Recurrent Neural Networks (RNNs), a type of machine learning technique, have recently drawn a lot of interest in numerous fields, including epidemiology. Implementing public health interventions in the field of epidemiology depends on efficient modeling and outbreak prediction. Because RNNs can capture sequential dependencies in data, they have become highly effective tools in this field. In this paper, the use of RNNs in epidemic modeling is examined, with a focus on the extent to which they can handle the inherent temporal dynamics in the spread of diseases. The mathematical representation of epidemics requires taking time-dependent variables into account, such as the rate at which infections spread and the long-term effects of interventions. The goal of this study is to use an intelligent computing solution based on RNNs to provide numerical performances and interpretations for the SEIR nonlinear system based on the propagation of the Zika virus (SEIRS-PZV) model. The four patient dynamics, namely susceptible patients S(y), exposed patients admitted in a hospital E(y), the fraction of infective individuals I(y), and recovered patients R(y), are represented by the epidemic version of the nonlinear system, or the SEIR model. SEIRS-PZV is represented by ordinary differential equations (ODEs), which are then solved by the Adams method using the Mathematica software to generate a dataset. The dataset was used as an output for the RNN to train the model and examine results such as regressions, correlations, error histograms, etc. For RNN, we used 100% to train the model with 15 hidden layers and a delay of 2 seconds. The input for the RNN is a time series sequence from 0 to 5, with a step size of 0.05. In the end, we compared the approximated solution with the exact solution by plotting them on the same graph and generating the absolute error plot for each of the 4 cases of SEIRS-PZV. Predictions made by the model appeared to be become more accurate when the mean squared error (MSE) decreased. An increased fit to the observed data was suggested by this decrease in the MSE, which suggested that the variance between the model's predicted values and the actual values was dropping. A minimal absolute error almost equal to zero was obtained, which further supports the usefulness of the suggested strategy. A small absolute error shows the degree to which the model's predictions matches the ground truth values, thus indicating the level of accuracy and precision for the model's output.
Citation: Kottakkaran Sooppy Nisar, Muhammad Wajahat Anjum, Muhammad Asif Zahoor Raja, Muhammad Shoaib. Recurrent neural network for the dynamics of Zika virus spreading[J]. AIMS Public Health, 2024, 11(2): 432-458. doi: 10.3934/publichealth.2024022
| [1] | Bo An . Construction and application of Chinese breast cancer knowledge graph based on multi-source heterogeneous data. Mathematical Biosciences and Engineering, 2023, 20(4): 6776-6799. doi: 10.3934/mbe.2023292 |
| [2] | Jiajia Jiao, Xiao Xiao, Zhiyu Li . dm-GAN: Distributed multi-latent code inversion enhanced GAN for fast and accurate breast X-ray image automatic generation. Mathematical Biosciences and Engineering, 2023, 20(11): 19485-19503. doi: 10.3934/mbe.2023863 |
| [3] | Lingli Gan, Xiaoling Yin, Jiating Huang, Bin Jia . Transcranial Doppler analysis based on computer and artificial intelligence for acute cerebrovascular disease. Mathematical Biosciences and Engineering, 2023, 20(2): 1695-1715. doi: 10.3934/mbe.2023077 |
| [4] | Yali Ouyang, Zhuhuang Zhou, Weiwei Wu, Jin Tian, Feng Xu, Shuicai Wu, Po-Hsiang Tsui . A review of ultrasound detection methods for breast microcalcification. Mathematical Biosciences and Engineering, 2019, 16(4): 1761-1785. doi: 10.3934/mbe.2019085 |
| [5] | Jun Gao, Qian Jiang, Bo Zhou, Daozheng Chen . Convolutional neural networks for computer-aided detection or diagnosis in medical image analysis: An overview. Mathematical Biosciences and Engineering, 2019, 16(6): 6536-6561. doi: 10.3934/mbe.2019326 |
| [6] | Muhammad Bilal Shoaib Khan, Atta-ur-Rahman, Muhammad Saqib Nawaz, Rashad Ahmed, Muhammad Adnan Khan, Amir Mosavi . Intelligent breast cancer diagnostic system empowered by deep extreme gradient descent optimization. Mathematical Biosciences and Engineering, 2022, 19(8): 7978-8002. doi: 10.3934/mbe.2022373 |
| [7] | Jian-xue Tian, Jue Zhang . Breast cancer diagnosis using feature extraction and boosted C5.0 decision tree algorithm with penalty factor. Mathematical Biosciences and Engineering, 2022, 19(3): 2193-2205. doi: 10.3934/mbe.2022102 |
| [8] | Xiao Zou, Jintao Zhai, Shengyou Qian, Ang Li, Feng Tian, Xiaofei Cao, Runmin Wang . Improved breast ultrasound tumor classification using dual-input CNN with GAP-guided attention loss. Mathematical Biosciences and Engineering, 2023, 20(8): 15244-15264. doi: 10.3934/mbe.2023682 |
| [9] | Xiaoli Zhang, Ziying Yu . Pathological analysis of hesperetin-derived small cell lung cancer by artificial intelligence technology under fiberoptic bronchoscopy. Mathematical Biosciences and Engineering, 2021, 18(6): 8538-8558. doi: 10.3934/mbe.2021423 |
| [10] | Xi Lu, Xuedong Zhu . Automatic segmentation of breast cancer histological images based on dual-path feature extraction network. Mathematical Biosciences and Engineering, 2022, 19(11): 11137-11153. doi: 10.3934/mbe.2022519 |
Recurrent Neural Networks (RNNs), a type of machine learning technique, have recently drawn a lot of interest in numerous fields, including epidemiology. Implementing public health interventions in the field of epidemiology depends on efficient modeling and outbreak prediction. Because RNNs can capture sequential dependencies in data, they have become highly effective tools in this field. In this paper, the use of RNNs in epidemic modeling is examined, with a focus on the extent to which they can handle the inherent temporal dynamics in the spread of diseases. The mathematical representation of epidemics requires taking time-dependent variables into account, such as the rate at which infections spread and the long-term effects of interventions. The goal of this study is to use an intelligent computing solution based on RNNs to provide numerical performances and interpretations for the SEIR nonlinear system based on the propagation of the Zika virus (SEIRS-PZV) model. The four patient dynamics, namely susceptible patients S(y), exposed patients admitted in a hospital E(y), the fraction of infective individuals I(y), and recovered patients R(y), are represented by the epidemic version of the nonlinear system, or the SEIR model. SEIRS-PZV is represented by ordinary differential equations (ODEs), which are then solved by the Adams method using the Mathematica software to generate a dataset. The dataset was used as an output for the RNN to train the model and examine results such as regressions, correlations, error histograms, etc. For RNN, we used 100% to train the model with 15 hidden layers and a delay of 2 seconds. The input for the RNN is a time series sequence from 0 to 5, with a step size of 0.05. In the end, we compared the approximated solution with the exact solution by plotting them on the same graph and generating the absolute error plot for each of the 4 cases of SEIRS-PZV. Predictions made by the model appeared to be become more accurate when the mean squared error (MSE) decreased. An increased fit to the observed data was suggested by this decrease in the MSE, which suggested that the variance between the model's predicted values and the actual values was dropping. A minimal absolute error almost equal to zero was obtained, which further supports the usefulness of the suggested strategy. A small absolute error shows the degree to which the model's predictions matches the ground truth values, thus indicating the level of accuracy and precision for the model's output.
The breast tumor is a common clinical disease in women. Ultrasound has always been considered the most appropriate method for breast examination and tumor screening [1]. However, ultrasound has a strong technical dependence on the examiners, and the results of different examiners are different, which has been considered the shortcomings of ultrasound [2]. With the imaging technology development, more and more methods have been used to objectively analyze ultrasound images to help diagnose breast tumors [3], such as elastic ultrasound, computer-aided diagnosis technology, etc.
The S-Detect artificial intelligence system is a set of auxiliary ultrasound imaging in diagnosing a deep learning system [4,5,6]. This system extracts the morphological features from the breast imaging reporting data system recommended by the American Society of Radiology (BI-RADS). Combined with pathological results of mass, the information can be used to diagnose breast lumps automatically. Two-dimensional breast ultrasound is widely used in diagnosing breast lesions. Still, due to its strong operator dependence, the accuracy and repeatability of BI-RADS classification results between different operators need to be improved [7,8,9]. Artificial intelligence-assisted diagnosis system eliminates the influence of many human factors. It can carry out image analysis objectively and efficiently to improve doctors' working efficiency and diagnostic efficiency [10,11,12].
One of the main problems encountered in ultrasound diagnosis is that senior doctors' diagnostic level is significantly higher than that of junior doctors. The training of a junior doctor often takes four years or more. The use of AI will significantly reduce the difficulty of training doctors. The purpose of this study was to evaluate the value of the S-Detect artificial intelligence system in the auxiliary diagnosis of benign and malignant breast masses.
From November 2019 to June 2020, the patients with breast masses undergoing ultrasound examination in the ultrasound imaging department of our hospital were randomly collected.
Inclusion criteria: The diagnosis had definite pathological support; adult patients; hospitalized patients.
Exclusion criteria: The mass boundary was not clear; there were blood flow, arrow, and text on the image; patients who can't cooperate with the exam.
Finally, 40 patients were enrolled in the study cohort. All the patients were female, with an age of (50.9 ± 13.9) years old. These patients were diagnosed with single or multiple breast lesions. However, only one typical image was selected from a patient. This study was approved by the ethics committee of the First People's Hospital of Anqing City. The written informed consent was obtained from patients.
The Samsung RS80A ultrasonic diagnostic instrument was used to detect the breast, with an L3-12A probe, frequency 3~12MHz.The patient was placed in the supine position, with his hands over his head, to expose the mammary glands and axilla's scanning area as large as possible. The scan was executed by two ultrasound doctors (engaged in breast ultrasound > 3 years) for regular breast two-dimensional (2D) gray-scale and color doppler ultrasound. The 2D images were collected, and the location, size, shape, boundary, internal echo, rear and side, blood flow, and spectrum of the echo were observed. The information was evaluated by the BI-RADS classification.
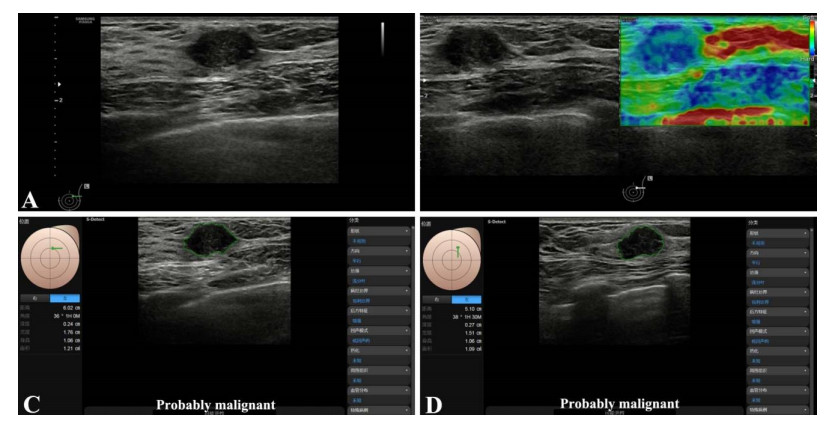
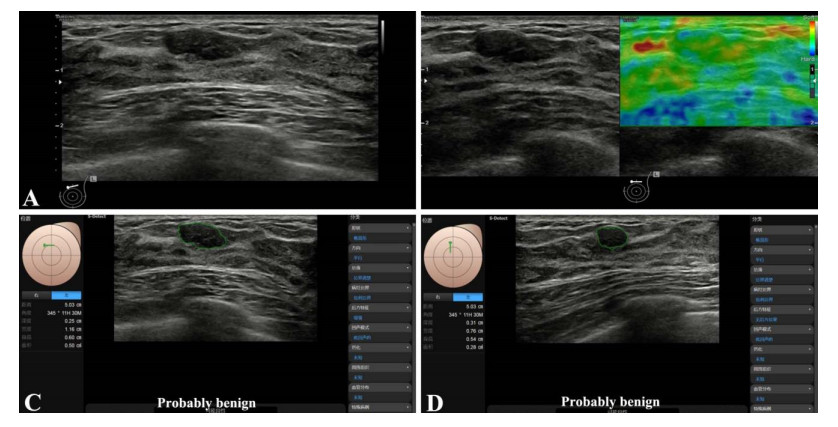
Then, the S-Detect mammary gland mode was selected to display the 2D horizontal and vertical gray-scale section of the mass. After clicking the center of lesion, the system automatically outlined the lesion boundary as the region of interest. If the border drawn automatically did not match the mass's solid edge, the operator could readjust and sketch the frame. After selecting the most appropriate boundary, the system automatically listed the various characteristics of the mass (size, depth, shape, boundary, internal echo, and so on) and the diagnostic results, which probably benign or malignant (Figures 1–3).
Finally, the model was converted to breast elasticity, and the patient was asked to hold their breath without pressure. Satisfactory elastic images were collected. The color of elastography was divided into green and blue, which reflects the hardness of the tissue. If the lesion is very hard, it is dark blue and tends to be malignant. The lesions were soft, light green, and managed to be benign.
The BI-RADS classification results for each breast mass were divided into the senior physician (engaged in breast ultrasound > 15 years) and the junior group. Both of them were classified into two groups based on whether the auxiliary S-Detect AI were used. One group was the BI-RADS initial classification of each mass by sonographers based on conventional ultrasound images; another group was the BI-RADS classification for each mass again performed for the physician combined with S-Detect assisted diagnosis.
Since each mass was randomly examined more than twice before the final surgical biopsy, the multiple BI-RADS classifications for all lesions obtained by multiple sonographers were defined in this study as a junior set of results. The S-Detect system diagnosis results were also combined with the junior set of products. The BI-RADS classification diagnosis results Category ≤ 4a was defined as benign lesions, while Category ≥ 4b was defined as malignant lesions.
The S-Detect diagnosis of each breast mass in both transverse and longitudinal sections was recorded as B/B, and both areas were likely benign. The diagnosis results of the two regions are different, which was denoted as M/B. Both sections are likely malignant, denoted as M/M. The S-Detect diagnostic result B/B was defined as benign lesions, while the M/B or M/M were defined as malignant lesions.
According to the standards of elastic imaging Guidelines, the Tsukuba score (Elasticity Score) was performed for each breast mass, with the ES value as the ES group data. The ES ≤ 3 points of Tsukuba were defined as benign lesions, while ES ≥ 4 points were defined as malignant lesions.
The SPSS 21.0 software was used for statistical analysis of the data. The qualitative data were presented in terms of rate and the results of different diagnostic methods for the same lesion were compared by paired chi-square test.
Based on the pathological results, the receiver operator characteristics (ROC) curves were drawn for multiple groups of diagnostic products to obtain the area under curve area (AUC), sensitivity, specificity, and diagnostic accuracy. Kappa test was used to analyze the consistency of diagnostic results of different groups of data. The accuracy was calculated by the following formula:
| accuracy=a+dn×100%(1) |
In the formula, the "a" represents the true positive number, "d" represents the true negative number, and "n" represents the total number.
Among the 40 patients, benign lesions accounted for 40.0% (16/40), and malignant lesions accounted for 60.0% (24/40). In the benign lesions, there were 4 cases of mammary gland disease, 8 cases of fibroadenoma, 2 cases of papilloma, and 2 cases of other benign tumors. In the malignant lesions, there were 17 cases of invasive ductal carcinoma, 1 case of mucinous carcinoma, 1 case of papillary carcinoma, 3 case of intraductal carcinoma, 1 cases of invasive lobular carcinoma, and 1 case of intraductal carcinoma in situ..
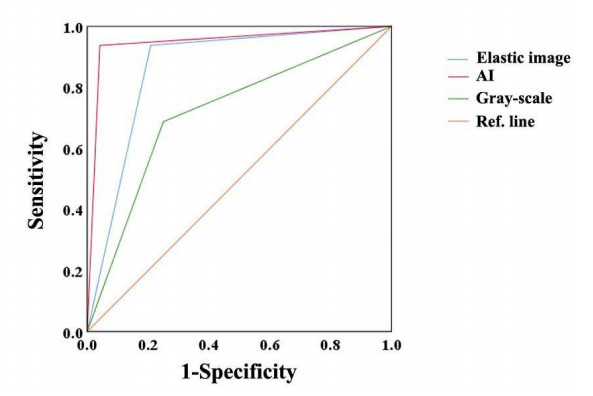
When the results of pathological examination were used as the gold standard, the S-Detect AI system itself had high sensitivity, specificity, and accuracy (95.8%, 93.8%, 89.6%) for the identification of benign and malignant breast masses. The diagnostic efficiency of two-dimensional gray-scale ultrasound was the lowest, with the sensitivity and specificity was lower than 80%. The elastic image had a relatively high specificity (93.8%) and a relatively lower sensitivity (79.2%). The detail was shown in Table 1 and Figure 4.
| Group | AUC | Sensitivity (%) | Specificity (%) | Accuracy (%) |
| AI | 0.948 | 95.8 | 93.8 | 89.6 |
| Elastic image | 0.865 | 79.2 | 93.8 | 73.0 |
| Gray-scale | 0.719 | 75.0 | 68.8 | 43.8 |
DownLoad:
CSV
Before the S-Detect AI system was used, the BI-RADS classification of 40 patients was category 3 in 8 cases, Category 4a in 13 cases, category 4b in 11 cases, and category 4c in 6 cases, and category 5 in 2 cases. From Table 2, doctors primarily use the S-Detect auxiliary system for escalation and degradation of category 4a and class 4b. For BI-RADS3, 4C, and 5 breast lesions, the S-Detect system diagnostic difference did not substantially affect the judgment of doctor.
| Before assistance | S-Detect | After assistance |
| Category 8 | B/B 7 | Category 3 8 |
| M/B 1 | ||
| Category4a 13 | B/B 6 | Category 3 3 |
| M/B 4 | Category 4a 4 | |
| M/M 3 | Category 4b 4 | |
| Category 4c 2 | ||
| Category 4b 11 | B/B 2 | Category 4a 2 |
| M/B 4 | Category 4b 6 | |
| M/M 5 | Category 4c 3 | |
| Category 4c 6 | M/B 2 | Category 4c 6 |
| M/M 4 | ||
| Category 5 2 | M/M 2 | Category 5 2 |
DownLoad:
CSV
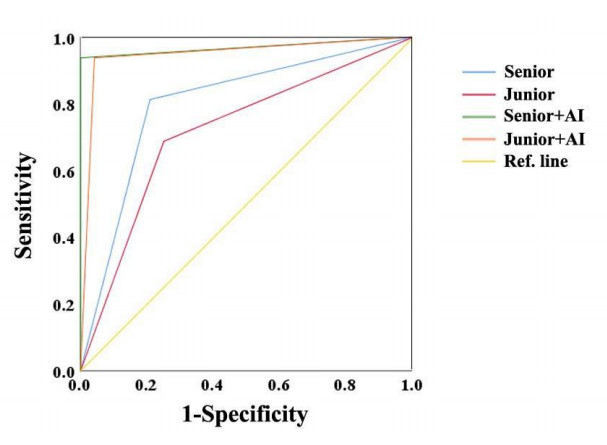
With the assistance of S-Detect AI system, the accuracy of BI-RADS classification was improved significantly. The detail was showed in Table 3 and Figure 5.
| Group | Area under ROC curve | Sensitivity (%) | Specificity (%) | Accuracy (%) |
| Senior | 0.802 | 79.2 | 81.3 | 60.5 |
| Junior | 0.719 | 75.0 | 68.8 | 43.8 |
| Senior+AI | 0.969 | 100.0 | 93.8 | 93.8 |
| Junior+AI | 0.948 | 95.8 | 93.8 | 89.6 |
DownLoad:
CSV
The S-Detect artificial intelligence system can effectively differentiate benign from malignant breast lesions. With the assistance of this technology, the BI-RADS classification diagnostic performance of senior sonographers has been on the rise [13]. The S-Detect auxiliary diagnosis results combined with the results of random ultrasound examination can significantly improve diagnostic accuracy. Therefore, this clinical diagnosis technique can enhance the quality of random breast ultrasound examinations obtained by patients and reduce missed diagnoses and misdiagnosis. The S-Detect technique and the elasticity scoring technique can improve the sonographer's diagnostic efficiency in breast cancer diagnosis. Still, there are some differences between the diagnostic ability and the auxiliary diagnostic ability [14].
Artificial intelligence technology based on big data and deep learning algorithm is widely integrated into the medical field [15,16,17]. Combining medical imaging with it can reduce the work burden of imaging physicians and improve the overall work efficiency. Computer aided diagnosis system has the advantages of objectivity, stability and high repeatability, and has been used in the early research and application of lung mass, breast mass and cardiovascular disease. The S-Detect auxiliary diagnostic system is intelligent and efficient, using the physico-acoustic characteristics of 2D gray-scale images of breast lesions for rapid differential diagnosis. In this study, the S-Detect technique has a high diagnostic efficiency, with differential diagnosis sensitivity, specificity and accuracy exceeding 90%. With the aid of this technology, the diagnostic efficiency of senior doctors has an upward trend. As a result, the S-Detect technology can provide effective advice and increase diagnostic confidence for junior sonographer in the diagnosis of 2D gray-scale images.
According to the BI-RADS classification standards recommended by the American Society of Radiology, Category3mass for the possibility of malignant 2% or less, Category 4a mass is the possibility of malignant is 2~10%. In this study, of the 16 benign groups, 93.8% (15/16) were classified as Category 3 or 4a, and the pathological findings were mostly adenopathy or fibroadenoma of the breast. The clinician will perform a pathological biopsy or surgical excision of masses classified as Category 4a or above, most of which pathologically turn out benign. The S-Detect technology has higher diagnostic performance for BI-RADS4a and 4B mass upgrades and downgrades, improving the accuracy of the random ultrasound surgeon's diagnosis and reducing unnecessary biopsies and surgeries to BI-RADS4a diagnosis. The operators in 2D ultrasound examination of the breast are highly dependent. The operation technique and image discrimination ability of ultrasound doctors with different years of experience lead to poor repeatability among the operators [18,19,20]. The S-Detect system automatically analyzes a 2D gray-scale image of a breast mass, allowing for more objective identification of a breast mass, regardless of the operator's years of service, experience, resolution, etc. In this study, to our hospital patients randomized to breast ultrasound, diagnostic accuracy was less than 80%. With the help of artificial intelligence, the joint diagnosis accuracy up to 90%, both in the senior and junior doctor. The results reduced the low qualification doctor with high qualification doctor diagnosis efficiency of the differences, thus improving the hospital's overall quality [10].
Elasticity score technology reflects the hardness characteristics of breast masses [21,22]. It uses the elasticity information of breast masses to improve the diagnostic efficiency of ultrasound doctors. The S-Detect technique uses the same physical-acoustic information and diagnostic classification as conventional breast ultrasound complements and optimizes the two-dimensional information of conventional ultrasound. In this study, the elasticity scoring technique was less sensitive than the S-Detect technique, and the accuracy was similar to the S-Detect technique. Still, the combination of the elasticity scoring with routine breast ultrasound was slightly better than the S-Detect technique, possibly due to the complementarity of physical information from different directions.
In summary, the S-Detect artificial intelligence system can improve breast cancer diagnosis accuracy by ultrasound physicians and improve the quality of routine breast ultrasound diagnosis. In the future, multi-center and more extensive sample size studies are needed to verify the artificial intelligence system's clinical application prospect. The S-Detect technology has its limitations. Its characteristic analysis does not include important information such as blood flow signals, calcifications, and mass hardness, and the system has errors in the identification of masses < 1 cm in diameter.
All the doctors in the ultrasound department of the first people's Hospital of Anqing city were acknowledged for their data collection contribution.
All authors declare no conflicts of interest in this paper.
| [1] |
Dick GW, Kitchen SF, Haddow AJ (1952) Zika virus (I). Isolations and serological specificity. Trans R Soc Trop Med Hyg 46: 509-520. https://doi.org/10.1016/0035-9203(52)90042-4 
|
| [2] |
Simpson David IH (1964) Zika virus infection in man. Trans R Soc Trop Med Hyg 58: 335-338. https://doi.org/10.1016/0035-9203(64)90201-9
|
| [3] |
Moore DÁ, Causey OR, Carey DE, et al. (1975) Arthropod-borne viral infections of man in Nigeria, 1964–1970. Ann Trop Med Parasitol 69: 49-64. https://doi.org/10.1080/00034983.1975.11686983
|
| [4] |
Olson J, Ksiazek TG (1981) Zika virus, a cause of fever in Central Java, Indonesia. Trans R Soc Trop Med Hyg 75: 389-393. https://doi.org/10.1016/0035-9203(81)90100-0
|
| [5] |
Lyle R. Petersen, Denise J. Jamieson, Ann M. Powers, et al. (2016) Zika virus. New Engl J Med 374: 1552-1563. https://doi.org/10.1056/NEJMra1602113
|
| [6] |
Duffy MR, Chen TH, Hancock WT, et al. (2009) Zika virus outbreak on Yap Island, federated states of Micronesia. New Engl J Med 360: 2536-2543. https://doi.org/10.1056/NEJMoa0805715
|
| [7] |
Zanluca C, Melo VCAD, Mosimann ALP, et al. (2015) First report of autochthonous transmission of Zika virus in Brazil. Mem Inst Oswaldo Cruz 110: 569-572. https://doi.org/10.1590/0074-02760150192
|
| [8] |
Rasmussen SA, Jamieson DJ, Honein MA, et al. (2016) Zika virus and birth defects—reviewing the evidence for causality. New Engl J Med 374: 1981-1987. https://doi.org/10.1056/NEJMsr1604338
|
| [9] |
Serrato IM, Moreno-Aguilera D, Caicedo PA, et al. (2022) Vector competence of lambda-cyhalothrin resistant Aedes aegypti strains for dengue-2, Zika and chikungunya viruses in Colombia. Plos One 17: e0276493. https://doi.org/10.1371/journal.pone.0276493
|
| [10] | World Health OrganizationSituation report: Zika virus, microcephaly, Guillain-Barré syndrome (2016). Available from: https://iris.who.int/handle/10665/250295 |
| [11] | Secretaría de Salud.Boletín epidemiológico. Sistema Nacional de Vigilancia Epidemiológica. Sistema Único Información (2019) 52: p.57. https://www.gob.mx/salud/acciones-y-programas/direccion-general-de-epidemiologia-boletin-epidemiologico |
| [12] |
Liu L (2014) Fields Virology, 6th Edition. Clin Infec Dis 59: 613-613. https://doi.org/10.1093/cid/ciu346
|
| [13] |
Pierson TC, Diamond MS (2012) Degrees of maturity: The complex structure and biology of flaviviruses. Curr Opin Virol 2: 168-175. https://doi.org/10.1016/j.coviro.2012.02.011
|
| [14] |
Yu IM, Zhang W, Holdaway HA, et al. (2008) Structure of the immature dengue virus at low pH primes proteolytic maturation. Science 319: 1834-1837. https://doi.org/10.1126/science.1153264
|
| [15] |
Boigard H, Alimova A, Martin GR, et al. (2017) Zika virus-like particle (VLP) based vaccine. PLoS Negl Trop Dis 11: e0005608. https://doi.org/10.1371/journal.pntd.0005608
|
| [16] |
Crill Wayne D, Gwong-Jen J Chang (2004) Localization and characterization of flavivirus envelope glycoprotein cross-reactive epitopes. J Virol 78: 13975-13986. https://doi.org/10.1128/JVI.78.24.13975-13986.2004
|
| [17] |
Lindenbach BD, Rice CM (2003) Molecular biology of flaviviruses. Adv Virus Res 59: 23-62. https://doi.org/10.1016/S0065-3527(03)59002-9
|
| [18] |
Dowd KA, Ko SY, Morabito KM (2016) Rapid development of a DNA vaccine for Zika virus. Science 354: 237-240. https://doi.org/10.1126/science.aai9137
|
| [19] |
Muthumani K, Griffin BD, Agarwal S, et al. (2016) In vivo protection against ZIKV infection and pathogenesis through passive antibody transfer and active immunisation with a prMEnv DNA vaccine. NPJ Vaccines 1: 16021. https://doi.org/10.1038/npjvaccines.2016.21
|
| [20] |
Nour AM, Li Y, Wolenski J, et al. (2013) Viral membrane fusion and nucleocapsid delivery into the cytoplasm are distinct events in some flaviviruses. PLoS Pathog 9: e1003585. https://doi.org/10.1371/journal.ppat.1003585
|
| [21] |
Topal AO, Chitic R, Leprévost F (2023) One evolutionary algorithm deceives humans and ten convolutional neural networks trained on ImageNet at image recognition. Appl Soft Comput 143: 110397. https://doi.org/10.1016/j.asoc.2023.110397
|
| [22] |
Shin J, Musa Miah AS, Hasan MAM, et al. (2023) Korean Sign Language Recognition Using Transformer-Based Deep Neural Network. Appl Sci 13: 3029. https://doi.org/10.3390/app13053029
|
| [23] |
Kanwisher N, Khosla M, Dobs K (2023) Using artificial neural networks to ask ‘why’questions of minds and brains. Trends Neurosci 46: 240-254. https://doi.org/10.1016/j.tins.2022.12.008
|
| [24] | Jorgenson DW, Weitzman ML, Zhang YX, et al. (2024) Can neural networks predict stock market?. AC Inv Res J 220. Available from: https://www.ademcetinkaya.com/p/how-does-rating-model-work.html |
| [25] |
Bi K, Xie L, Zhang H, et al. (2023) Accurate medium-range global weather forecasting with 3D neural networks. Nature 619: 533-538. https://doi.org/10.1038/s41586-023-06185-3
|
| [26] |
Veličković P (2023) Everything is connected: Graph neural networks. Curr Opin Struct Biol 79: 102538. https://doi.org/10.1016/j.sbi.2023.102538
|
| [27] |
Sabir Z, Bhat SA, Raja MAZ, et al. (2023) A swarming neural network computing approach to solve the Zika virus model. Eng Appl Artif Intel 126: 106924. https://doi.org/10.1016/j.engappai.2023.106924
|
| [28] | Rubio-Solis A, Musah A, Dos Santos P, et al. ZIKA virus: Prediction of Aedes mosquito larvae occurrence in Recife (Brazil) using online extreme learning machine and neural networks. In Proceedings of the 9th international conference on digital public health; c2019 : p. 101-110. https://doi.org/10.1145/3357729.3357738 |
| [29] | Mahalakshmi B, Suseendran G (2019) Prediction of zika virus by multilayer perceptron neural network (MLPNN) using cloud. Int J Recent Technol Eng (IJRTE) 8: 1-6. https://doi.org/10.35940/ijrte.B1041.0982S1119 |
| [30] |
Yin R, Luusua E, Dabrowski J, et al. (2020) Tempel: Time-series mutation prediction of influenza A viruses via attention-based recurrent neural networks. Bioinformatics 36: 2697-2704. https://doi.org/10.1093/bioinformatics/btaa050
|
| [31] | Gupta AK, Srinivasulu A, Hiran KK, et al. (2022) Prediction of omicron virus using combined extended convolutional and recurrent neural networks technique on CT-scan images. Interdiscip Perspect Infect Dis 2022: 1525615. https://doi.org/10.1155/2022/1525615 |
| [32] | Bonet I, García MM, Saeys Y, et al. (2007) Predicting human immunodeficiency virus (HIV) drug resistance using recurrent neural networks. In International Work-Conference on the Interplay Between Natural and Artificial Computation; c2007 : p. 234-243. https://doi.org/10.1007/978-3-540-73053-8_23 |
| [33] |
Sindhu TN, Çolak AB, Lone SA, et al. (2024) A decreasing failure rate model with a novel approach to enhance the artificial neural network's structure for engineering and disease data analysis. Tribol Int 192: 109231. https://doi.org/10.1016/j.triboint.2023.109231
|
| [34] | Shafiq Anum, Andaç Batur Çolak, Tabassum Naz Sindhu, et al. (2023) Modeling and survival exploration of breast carcinoma: A statistical, maximum likelihood estimation, and artificial neural network perspective. Artif Intel Life Sci 4: 100082. https://doi.org/10.1016/j.ailsci.2023.100082 |
| [35] |
Shafiq A, Çolak AB, Sindhu TN (2024) Comparative analysis to study the Darcy–Forchheimer Tangent hyperbolic flow towards cylindrical surface using artificial neural network: An application to Parabolic Trough Solar Collector. Math Comput Simulat 216: 213-230. https://doi.org/10.1016/j.matcom.2023.09.014
|
| [36] | Shafiq A, Çolak AB, Sindhu TN (2023) Development of an intelligent computing system using neural networks for modeling bioconvection flow of second-grade nanofluid with gyrotactic microorganisms. Numerical Heat Transfer Part B: Fundamentals. https://doi.org/10.1080/10407790.2023.2273512 |
| [37] |
Shafiq A, Çolak AB, Sindhu TN (2023) Significance of EMHD graphene oxide (GO) water ethylene glycol nanofluid flow in a Darcy–Forchheimer medium by machine learning algorithm. Eur Phys J Plus 138: 213. https://doi.org/10.1140/epjp/s13360-023-03798-5
|
| [38] |
Shafiq A, Çolak AB, Sindhu TN (2023) Analyzing activation energy and binary chemical reaction effects with artificial intelligence approach in axisymmetric flow of third grade nanofluid subject to Soret and Dufour effects. Heat Transf Re 54: 75-94. https://doi.org/10.1615/HeatTransRes.2022045008
|
| [39] |
Shafiq A, Çolak AB, Sindhu TN (2023) Optimization of the numerical treatment of the Darcy–Forchheimer flow of Ree–Eyring fluid with chemical reaction by using artificial neural networks. Int J Numer Meth Fl 95: 176-192. https://doi.org/10.1002/fld.5147
|
| [40] |
Çolak AB, Sindhu TN, Lone SA, et al. (2024) A comparative analysis of maximum likelihood estimation and artificial neural network modeling to assess electrical component reliability. Qual Reliab Eng Int 40: 91-114. https://doi.org/10.1002/qre.3233
|
| [41] |
Çolak AB, Sindhu TN, Lone SA, et al. (2023) Reliability study of generalized Rayleigh distribution based on inverse power law using artificial neural network with Bayesian regularization. Tribol Int 185: 108544. https://doi.org/10.1016/j.triboint.2023.108544
|
| [42] |
Shafiq A, Çolak AB, Swarup C, et al. (2022) Reliability analysis based on mixture of lindley distributions with artificial neural network. Adv Theor Simul 5: 2200100. https://doi.org/10.1002/adts.202200100
|
| [43] |
Shafiq A, Çolak AB, Sindhu TN, et al. (2022) Comparative study of artificial neural network versus parametric method in COVID-19 data analysis. Results Phys 38: 105613. https://doi.org/10.1016/j.rinp.2022.105613
|
| [44] |
Shafiq A, Çolak AB, Naz Sindhu T (2021) Designing artificial neural network of nanoparticle diameter and solid–fluid interfacial layer on single-walled carbon nanotubes/ethylene glycol nanofluid flow on thin slendering needles. Int J Numer Meth Fl 93: 3384-3404. https://doi.org/10.1002/fld.5038
|
| [45] |
Shafiq A, Çolak AB, Sindhu TN, et al. (2021) Estimation of unsteady hydromagnetic Williamson fluid flow in a radiative surface through numerical and artificial neural network modeling. Sci Rep 11: 14509. https://doi.org/10.1038/s41598-021-93790-9
|
| [46] | Rezapour S, Mohammadi H, Jajarmi A (2020) A new mathematical model for Zika virus transmission. Adv Differ Equ 589: 1-15. https://doi.org/10.1186/s13662-020-03044-7 |
| [47] |
Sabir Z (2022) Stochastic numerical investigations for nonlinear three-species food chain system. Int J Biomath 15: 2250005. https://doi.org/10.1142/S179352452250005X
|
| [48] |
Shoaib M, Zubair G, Nisar KS, et al. (2021) Ohmic heating effects and entropy generation for nanofluidic system of Ree-Eyring fluid: Intelligent computing paradigm. Int Commun Heat Mass 129: 105683. https://doi.org/10.1016/j.icheatmasstransfer.2021.105683
|
| [49] |
Nisar KS, Anjum MW, Raja MAZ, et al. (2024) Homogeneous-heterogeneous reactions on Darcy-Forchheimer nanofluid flow system. Case Stud Therm Eng 53: 103882. https://doi.org/10.1016/j.csite.2023.103882
|
| [50] |
Sabir Z, Wahab HA, Ali MR, et al. (2022) Neuron analysis of the two-point singular boundary value problems arising in the thermal explosion's theory. Neural Process Lett 54: 4297-4324. https://doi.org/10.1007/s11063-022-10809-6
|
| [51] |
Sabir Z, Wahab HA (2021) Evolutionary heuristic with Gudermannian neural networks for the nonlinear singular models of third kind. Phys Scripta 96: 125261. https://doi.org/10.1088/1402-4896/ac3c56
|
| [52] |
Sabir Z, Raja MAZ, Baleanu D (2021) Fractional mayer neuro-swarm heuristic solver for multi-fractional order doubly singular model based on lane–emden equation. Fractals 29: 2140017. https://doi.org/10.1142/S0218348X2140017X
|
| 1. | Peng Xue, Mingyu Si, Dongxu Qin, Bingrui Wei, Samuel Seery, Zichen Ye, Mingyang Chen, Sumeng Wang, Cheng Song, Bo Zhang, Ming Ding, Wenling Zhang, Anying Bai, Huijiao Yan, Le Dang, Yuqian Zhao, Remila Rezhake, Shaokai Zhang, Youlin Qiao, Yimin Qu, Yu Jiang, Unassisted Clinicians Versus Deep Learning–Assisted Clinicians in Image-Based Cancer Diagnostics: Systematic Review With Meta-analysis, 2023, 25, 1438-8871, e43832, 10.2196/43832 | |
| 2. | Anastasia-Maria Leventi-Peetz, Kai Weber, Probabilistic machine learning for breast cancer classification, 2022, 20, 1551-0018, 624, 10.3934/mbe.2023029 | |
| 3. | Peng-fei Lyu, Yu Wang, Qing-Xiang Meng, Ping-ming Fan, Ke Ma, Sha Xiao, Xun-chen Cao, Guang-Xun Lin, Si-yuan Dong, Mapping intellectual structures and research hotspots in the application of artificial intelligence in cancer: A bibliometric analysis, 2022, 12, 2234-943X, 10.3389/fonc.2022.955668 | |
| 4. | Xiaolei Wang, Shuang Meng, Diagnostic accuracy of S-Detect to breast cancer on ultrasonography: A meta-analysis (PRISMA), 2022, 101, 1536-5964, e30359, 10.1097/MD.0000000000030359 | |
| 5. | Samara Acosta-Jiménez, Javier Camarillo-Cisneros, Abimael Guzmán-Pando, Susana Aideé González-Chávez, Jorge Issac Galván-Tejada, Graciela Ramírez-Alonso, César Francisco Pacheco-Tena, Rosa Elena Ochoa-Albiztegui, 2023, Chapter 8, 978-3-031-18255-6, 83, 10.1007/978-3-031-18256-3_8 | |
| 6. | Yuqun Wang, Lei Tang, Pingping Chen, Man Chen, The Role of a Deep Learning-Based Computer-Aided Diagnosis System and Elastography in Reducing Unnecessary Breast Lesion Biopsies, 2022, 15268209, 10.1016/j.clbc.2022.12.016 | |
| 7. | Peizhen Huang, Bin Zheng, Mengyi Li, Lin Xu, Sajjad Rabbani, Abdulilah Mohammad Mayet, Chengchun Chen, Beishu Zhan, He Jun, Ateeq Ur Rehman, The Diagnostic Value of Artificial Intelligence Ultrasound S-Detect Technology for Thyroid Nodules, 2022, 2022, 1687-5273, 1, 10.1155/2022/3656572 | |
| 8. | Qiyu Liu, Meijing Qu, Lipeng Sun, Hui Wang, Accuracy of ultrasonic artificial intelligence in diagnosing benign and malignant breast diseases, 2021, 100, 0025-7974, e28289, 10.1097/MD.0000000000028289 | |
| 9. | N.N. Bayandina, E.N. Slavnova, A cytological method in the early diagnosis of cervical cancer: evolution, principles, technologies, prospects, 2023, 12, 2305-218X, 49, 10.17116/onkolog20231202149 | |
| 10. | Peng Sun, 2023, Chapter 35, 978-981-19-9372-5, 323, 10.1007/978-981-19-9373-2_35 | |
| 11. | Hee Jeong Kim, Hak Hee Kim, Ki Hwan Kim, Ji Sung Lee, Woo Jung Choi, Eun Young Chae, Hee Jung Shin, Joo Hee Cha, Woo Hyun Shim, Use of a commercial artificial intelligence-based mammography analysis software for improving breast ultrasound interpretations, 2024, 34, 1432-1084, 6320, 10.1007/s00330-024-10718-3 | |
| 12. | E. L. Teodozova, E. Yu. Khomutova, Artificial intelligence in radial diagnostics of breast cancer, 2023, 3, 2782-3024, 26, 10.61634/2782-3024-2023-12-26-35 | |
| 13. | Xiao Zou, Jintao Zhai, Shengyou Qian, Ang Li, Feng Tian, Xiaofei Cao, Runmin Wang, Improved breast ultrasound tumor classification using dual-input CNN with GAP-guided attention loss, 2023, 20, 1551-0018, 15244, 10.3934/mbe.2023682 | |
| 14. | Lu Li, Hongyan Deng, Xinhua Ye, Yong Li, Jie Wang, Comparison of the diagnostic efficacy of mathematical models in distinguishing ultrasound imaging of breast nodules, 2023, 13, 2045-2322, 10.1038/s41598-023-42937-x | |
| 15. | Na Li, Wanling Liu, Yunyun Zhan, Yu Bi, Xiabi Wu, Mei Peng, Value of S-Detect combined with multimodal ultrasound in differentiating malignant from benign breast masses, 2024, 55, 2090-4762, 10.1186/s43055-023-01183-x | |
| 16. | Pengjie Song, Li Zhang, Longmei Bai, Qing Wang, Yanlei Wang, Diagnostic performance of ultrasound with computer-aided diagnostic system in detecting breast cancer, 2023, 9, 24058440, e20712, 10.1016/j.heliyon.2023.e20712 | |
| 17. | Qing Dan, Ziting Xu, Hannah Burrows, Jennifer Bissram, Jeffrey S. A. Stringer, Yingjia Li, Diagnostic performance of deep learning in ultrasound diagnosis of breast cancer: a systematic review, 2024, 8, 2397-768X, 10.1038/s41698-024-00514-z | |
| 18. | Panpan Zhang, Min Zhang, Menglin Lu, Chaoying Jin, Gang Wang, Xianfang Lin, Comparative Analysis of the Diagnostic Value of S-Detect Technology in Different Planes Versus the BI-RADS Classification for Breast Lesions, 2024, 10766332, 10.1016/j.acra.2024.08.005 | |
| 19. | Zhuohua Lin, Ligang Cui, Yan Xu, Qiang Fu, Youjing Sun, Feasibility and potential of intraoperative ultrasound in arthroscopy of femoroacetabular impingement, 2024, 2054-8397, 10.1093/jhps/hnad050 | |
| 20. | Manisha Bahl, Jung Min Chang, Lisa A. Mullen, Wendie A. Berg, Artificial Intelligence for Breast Ultrasound: AJR Expert Panel Narrative Review, 2024, 0361-803X, 1, 10.2214/AJR.23.30645 | |
| 21. | Jie He, Nan Liu, Li Zhao, New progress in imaging diagnosis and immunotherapy of breast cancer, 2025, 16, 1664-3224, 10.3389/fimmu.2025.1560257 |
Figures(13) / Tables(3)
Kottakkaran Sooppy Nisar, Muhammad Wajahat Anjum, Muhammad Asif Zahoor Raja, Muhammad Shoaib. Recurrent neural network for the dynamics of Zika virus spreading[J]. AIMS Public Health, 2024, 11(2): 432-458. doi: 10.3934/publichealth.2024022
| Group | AUC | Sensitivity (%) | Specificity (%) | Accuracy (%) |
| AI | 0.948 | 95.8 | 93.8 | 89.6 |
| Elastic image | 0.865 | 79.2 | 93.8 | 73.0 |
| Gray-scale | 0.719 | 75.0 | 68.8 | 43.8 |
DownLoad:
CSV
| Before assistance | S-Detect | After assistance |
| Category 8 | B/B 7 | Category 3 8 |
| M/B 1 | ||
| Category4a 13 | B/B 6 | Category 3 3 |
| M/B 4 | Category 4a 4 | |
| M/M 3 | Category 4b 4 | |
| Category 4c 2 | ||
| Category 4b 11 | B/B 2 | Category 4a 2 |
| M/B 4 | Category 4b 6 | |
| M/M 5 | Category 4c 3 | |
| Category 4c 6 | M/B 2 | Category 4c 6 |
| M/M 4 | ||
| Category 5 2 | M/M 2 | Category 5 2 |
DownLoad:
CSV
| Group | Area under ROC curve | Sensitivity (%) | Specificity (%) | Accuracy (%) |
| Senior | 0.802 | 79.2 | 81.3 | 60.5 |
| Junior | 0.719 | 75.0 | 68.8 | 43.8 |
| Senior+AI | 0.969 | 100.0 | 93.8 | 93.8 |
| Junior+AI | 0.948 | 95.8 | 93.8 | 89.6 |
DownLoad:
CSV
| Group | AUC | Sensitivity (%) | Specificity (%) | Accuracy (%) |
| AI | 0.948 | 95.8 | 93.8 | 89.6 |
| Elastic image | 0.865 | 79.2 | 93.8 | 73.0 |
| Gray-scale | 0.719 | 75.0 | 68.8 | 43.8 |
| Before assistance | S-Detect | After assistance |
| Category 8 | B/B 7 | Category 3 8 |
| M/B 1 | ||
| Category4a 13 | B/B 6 | Category 3 3 |
| M/B 4 | Category 4a 4 | |
| M/M 3 | Category 4b 4 | |
| Category 4c 2 | ||
| Category 4b 11 | B/B 2 | Category 4a 2 |
| M/B 4 | Category 4b 6 | |
| M/M 5 | Category 4c 3 | |
| Category 4c 6 | M/B 2 | Category 4c 6 |
| M/M 4 | ||
| Category 5 2 | M/M 2 | Category 5 2 |
| Group | Area under ROC curve | Sensitivity (%) | Specificity (%) | Accuracy (%) |
| Senior | 0.802 | 79.2 | 81.3 | 60.5 |
| Junior | 0.719 | 75.0 | 68.8 | 43.8 |
| Senior+AI | 0.969 | 100.0 | 93.8 | 93.8 |
| Junior+AI | 0.948 | 95.8 | 93.8 | 89.6 |
