A monotonicity approach to Pogorelov's Hessian estimates for Monge- Ampère equation

1.   Introduction

Short-sale price restriction means a trading regulation that short sale of stock is restricted from being placed on a specified price in the market price of the shares. This regulation is often adopted to prevent the short selling of shares during declining market conditions with the intention of restricting the extent of a market slump. Restrictions on short selling may affect stock returns due to factors including market efficiency, liquidity, and overpricing, among others. It is interesting to find the connection between the short-sale restriction of trading stock and the investor's herding behavior because with short-sale restriction comes liquidity changes and market inefficiency and liquidity changes and market inefficiency could intensify the herding behavior. Sias (2004) and Roon et al. (2013) noted that association between liquidity status and herding. Puckett and Yan (2010) support that herding affect the efficiency by using weekly data. Osamor et al. (2019) show that stock market liquidity had significant impact on herding behavior during high and low market liquidity. El and Hefnaou (2019) also support the low liquidity and low level of transparency encourage investors to herd. Therefore, the short selling ban, accompanying with liquidity change and market inefficiency, motivates us to observe their impact of herding.

Most studies published over the past two decades have identified a negative connection between short-sale price restrictions and stock returns that is attributable to inability to arbitrage or other friction costs. These latter studies include Miller (1997), Eric et al. (2007), Haruvy and Noussair (2006), Bris (2008), Boulton and Braga-Alves (2010), Autore et al. (2011) and Coakley et al. (2018). A substantial body of research documents that short sale constraints often cause stocks to be overpriced and thus to have lower future return potential. However, a paucity of literature on the connection of short sale constraints and investor's herding behavior. This study we present in the present paper is an attempt to supplement the finding of these earlier researches and to find evidence of relationship of short sale constraints and investor's herding behavior.

Employing a special case of Taiwan to observe the main issue as mentioned above, there are three objectives to develop this paper. Although the literature has examined extensively the impact on price return of retaining and incurring short-sale restrictions (Miller, 1977; Bris, 2008; Beber and Pagano, 2011; Coakley et al., 2018), no published research has examined the impact of removing these restrictions. Thus, the first objective of the present study is to examine the effect on stock sales of removing short-sale restrictions.

Secondly, as previous empirical investigations have clarified the impact on stock returns of maintaining short sale constraints, the present study will endeavor to assess how "lifting" these constraints affect the "decisions of investors" (such as herding behavior). Bohl et al. (2011) studied the data of six stock indices during the current global financial crisis (2008–2009) and found no association between selling prohibitions and herding behavior in financial markets. However, the effect of herding behavior on individual stocks rather than on stock indices has yet to be addressed in the literature. Scholarly discussion to date on this topic lacks the systematic consideration of investor trading behavior in light of either continuing or lifting short-sale price restrictions. Thus, clarifying the currently poorly understood relationship between herding behavior and the continued implementation or lifting of short-sale price constraints is the second objective of the present study.

Short-sale constraints such as short-sale price restrictions may produce special insights into the pricing process of relatively high or low stock returns because herding behavior is more pronounced in cases of extreme stock return (Christie and Huang, 1995; Chiang et al., 2000, Caparrelli et al., 2010), when stocks reveal asymmetric returns (Zheng et al., 2010; Chiang et al., 2000; Hwang and Salmon, 2004; Sharma et al., 2015). Thus, the third objective of the present study is to investigate the effects of lifting short-sale constraints under the condition of extreme stock returns. In addressing this issue, a quantile regression model is used to observe both edges of stock returns.

The present research focuses on the Taiwan stock market. Similar to South Korea's stock market, the Taiwan stock market is a typical emerging stock market in which individual investors rather than institutional investors commonly dominate trading. Hwang and Salmon (2001) found that herd behavior tends to be stronger in emerging markets than in mature markets such as the UK or US. Apart from offering an opportunity to examine conditions in a stock market that is not dominated by institutional investors, the Taiwan stock market offers a unique opportunity to observe the distinct impact of the lifting of bans on short selling on the stocks of large "blue-chip" firms and all listed firms, respectively. Short-sale price restrictions were first rescinded in Taiwan in May 2005 for the constituent stocks of FTSE Taiwan Stock Exchange (TWSE) Taiwan 50 Index, which includes the top-50 domestic "blue-chip" companies by market capitalization¹. Eight years later, short-sale price restrictions were rescinded in September 2013 for all TWSE-listed stocks.

¹ The 50 of the most highly capitalized stocks represent nearly 70% of the Taiwanese stock market which has about 800 listed stocks.

The short-sale price restrictions in Taiwan were similar to those in the United States. Under US Securities and Exchange Commission (SEC) rules, short sales are permitted only on upticks or zero-plus ticks. An uptick is defined as a trade that is executed at a price that is higher than the trade immediately before. A zero-plus tick is a trade that is executed at a price that is the same as the trade immediately before. In Taiwan, short sales must be placed at a price that is at or above the closing price of the previous day.

As noted above, Taiwan offers a unique opportunity to assess empirical results in the context of different-sized firms. There are three main reasons to do this. First, past literature indicates that the level of herding in significantly higher in small stocks than in large stocks (Wermer, 1999). Second, this allows an examination of the impact of substitutions for short selling under conditions of short-sale price restrictions. While finding substitutions for short selling the stocks of small firms is difficult, short selling TAIWAN 50 Index stocks may be a substitute for short selling the stocks of large firms in Taiwan. Battalio and Schultz (2011) and Grundy et al. (2012) revealed that the option market provides a substitute to short selling when short-sale restrictions are in force. Finally, Bai et al. (2006) mentioned that the asymmetric information on stocks for short sellers when short-sale restrictions are in force may lead these sellers to over or undervalue a stock depending on the degree of information asymmetry. Therefore, as asymmetric information is a crucial factor for the short sellers, observing a two-stage lifting of short-sale restriction regulations allows a closer examination/assessment of this effect.

In summary, the main aims of the present study are to deepen scholarly understanding of the connection between herding and the ending of short-sale constraints and of whether this connection is influenced by investor type (individual vs. institutional) or by firm size. Briefly, most academic studies to date have addressed only the impact of short selling bans on stock returns, with little attention given to the relationship between herding behavior and stock returns under the condition of short-sell restrictions. Thus, the present paper investigates the effects of the herding behavior of individual investors and institutions both before and after the ending of short-sale restriction in terms of small and large firms, respectively.

The present study constructed an index of the TSE 50 lowest capitalized stocks as a comparison group for the Taiwan 50 Index in order to estimate the potential asymmetric empirical results based on firm size. The empirical results revealed that lower levels of herding behavior were associated with larger stock returns for small firms under short-sale price restrictions and that there was no connection between herding and stock returns for large firms. The results confirmed the conjecture that short-sale constraints impact small enterprises more significantly than large enterprises. That may be because small and illiquid stocks are not attractive shorting targets for traders. Another more important reason may be that indirect short sales of the constituent stocks of the Taiwan 50 Index has been allowed via the Taiwan 50 exchange traded fund (ETF) since June 2003. The Taiwan 50 ETF is the most active ETF on the Taiwan stock market. Conversely, small firms cannot be shorted outside of short-sale price constraints, either through direct trades or through indirect trades via an index ETF.

Besides, after lifting short-sale price restrictions, individual herding maintained an effect only in a low quantile of small stock returns. The effects of institutional herding behavior were no longer observable after the comprehensive ending of short-sale price restrictions on firms of all sizes.

To the best of the authors' knowledge, no study has previously been conducted on the relationship between herding behavior and stock returns after the ending of short-selling restrictions. The present paper offers several scholarly contributions. First, this study helps bridge a gap in the literature regarding to the comparative impact of herding behavior in emerging markets before and after short-sale price restrictions have ended. Secondly, a herding measurement that was defined by Lakonishok et al. (1992) and that is based on purchasing and sale orders was used for the first time in the present study, which complements and extends the current literature on this topic. Thirdly, the application of a quantile regression model permits the observation of effects in detail under various quantiles. Finally, the present paper confirms that the effect of herding behavior on stock returns differs between small and large firms.

The following sections review the relevant literature, describe the dataset, explain the empirical results, and present the conclusions.

2.   Literature review

This section reviews the literature on herding behavior and then introduces the connection between herding and short-sale price restrictions and herding behavior under extreme positive or negative returns.

Nofsinger and Sias (1999) defined "herding" as a group of investors trading in the same direction over a period of time. During herding, investors tend to ignore their private information or beliefs in favor of imitating the behavior of other investors, whether this behavior is rational or not. Herding in financial markets is now a widely accepted concept in the literature (Christie and Huang, 1995; Chang et al., 2000; Hwang and Salmon, 2004).

Herding-induced mispricing and market inefficiencies are issues that have been previously addressed in the literature (Banerjee, 1992; Bikhchanadani et al., 1992; Avery and Zemsky, 1998). As ruling out the presence of a herding influence is difficult using theoretical analysis (Hwang and Salmon, 2004), empirical results are necessary to confirm the influence of herding on stock returns.

Leaving aside the issue of whether spurious herding may be rationally judged using fundamental analysis, it is worth investigating how rule restrictions affect the relationship between herding and stock returns because the evidences of past literature show that a strong consensus regarding the impacts of short-sale restriction regulations on market efficiency, liquidity, and overpricing. The extensive early theoretical work in this area includes Miller (1977), who introduces the overvaluation hypothesis and investigated market condition under short-sale restrictions. Bris (2008) provided empirical results from the US that supported the overvaluation hypothesis, while Beber and Pagano (2011) investigated 30 countries and found the overvaluation problem to be insignificant. Besides the problem of overpricing, short-sale price restrictions could cause other market conditions change. The lower stock market liquidity (Amihud and Mendelson, 1986; Helmes et al., 2010; Marsh and Payne, 2012; and Beber and Pagano, 2013) and lower efficiency (Diamond and Verrecchia, 1987; Bris, 2008) that results from short-sale price restrictions results in different types of investors adopting different investment perspectives. Devenow and Welch (1996) explain three reasons for herding and the first reason is payoff externalities. Investors try to trade at the same time to benefit from a deeper liquidity (Admati and Pfleiderer, 1988; Dow, 2004; Merli and Roger, 2013). Jlassi and BenSaida (2014) found contemporaneous herding is a deterministic factor for increasing trading volume, but trading volume cannot generate herding behavior, except for liquid market, by applying VAR and Granger causality test. Due to the work of Osamor et al. (2019), using data of fifteen years from 2001–2015 to show that high and low stock market liquidity goes along with herding behavior, it is intuitively to link short-sale price restrictions and herding behavior.

Although few studies address the relationship between herding behavior and short-selling restrictions, several possible explanations support the likelihood of this relationship. First, restrictions on short selling prevent an arbitrageur from shorting an overpriced security, while allowing investors to buy that security. The short-selling restriction thus limits the liquidity and freedom of those investors who want to sell or short stocks. As this condition prevents a specific group of investors from freely expressing their pessimism about the value of a stock, overpricing of asset may occur. For the research on stock indices, Bohl et al. (2011) studied the data of six stock indices during the current global financial crisis (2008–2009) and found no association between selling prohibitions and herding behavior in financial markets. However, for the individual stocks, the connection of short sale restriction and herding has not yet to be addressed in the literature.

Lobao and Serra (2006) supported that on the consequences of herding behavior on asset valuation will help to shed some light on current debates over market efficiency. Guney et al. (2017) found that herding is present on African frontier markets and it is attributed to the low levels of transparency to reduce the quality of their information environment. Herding allow them to deduce information from their peers' transactions and leads investors to consider herding as a feasible option. Cipriani and Guarino (2014) developed a model of informational herding to agree herding also causes important informational inefficiencies in the market on a NYSE stock (Ashland Inc.) during 1995. Huang and Yang (2019) argued that managers' overconfidence and herd behavior would lead to more non-efficient investment in Chinese stock market. Moreover, prior studies concur that uncertain conditions increase the likelihood that investors will mimic the actions of other investors. Herding that occurs under conditions of a short-selling ban may then lead to a more seriously mispriced asset, as herding causes problems of mispricing and market inefficiency (Banerjee, 1992; Bikhchanadani et al., 1992; Avery and Zemsky, 1998; Puckett and Yan, 2010). The level of herding should be larger under the uncertainty of less liquidity/lacking arbitrage opportunities due to short-sale price restrictions than under conditions that place no restrictions on short-sale prices. Unfortunately, only a few studies have considered alleviating the problem of herding as an argument for ending short-selling restrictions. Bohl et al. (2011) compared the stock markets of six countries² that were affected by bans on short selling during the global financial crisis of 2008–2009 and found surprisingly that short-selling restrictions had no influence on herding formation. However, the herding measure of Bohl et al. (2011) was based on the average deviation of single stock returns from the market return and not on the actual trading orders of investors. Thus, their measure of herding behavior differs from that used in the present paper. Moreover, the 2011 study does not address the comparative effect of herding under during and after short-sale price constraints. The present paper attempts to add new scholarly insight on this issue.

² United States, the United Kingdom, Germany, France, South Korea, and Australia.

Besides, the setting or lifting short sale price restrictions may affect the herding through changes of investor emotion. Filiz et al. (2019) show that mood really does have an influence on the tendency towards herding behavior.

The related works related to herding measures may be classified into two primary categories. The first category, introduced by Lakonishok et al. (1992), conceptualizes herding as buyer power related to all trades. The detailed records of individual trading activities represent a distinct advantage for measurements in this category, showing the herding situation for each security rather than for the whole market or group of stocks. Besides, those that place orders may be introduced into the herding measurement. The second category, first proposed by Christie and Huang (1995) and Chang et al. (2000), is based on the average deviation of single stock returns from market returns and is most appropriate for examining overall herding behavior in a stock market.

Herding measurements that are based on trading orders (i.e., first category measurements) have the advantage of being able to observe herding behavior in a subetaoup of stocks. Several studies agree that a much higher level of herding exists in small firms (Lakonishok et al., 1992; Wermer, 1999; Bikhchandani and Sharma, 2001; Sias, 2004; Wylie, 2005). This may be due to the phenomenon of asymmetric information leading investors in emerging market to engage in herding (Calvo and Mendoz, 2000), with small firms typically being less transparent to investors than large firms. Many prior studies agree that firm size affects herding behavior. Therefore, the present paper applied Lakonishok et al. (1992)'s measurement in order to elicit a better understanding of herding in various size-based subetaoups of firms. Besides, the smaller firm may exist lower liquidity.

Christie and Huang (1995) and Chang et al. (2000) pointed out that short-sale constraints affect the herding behavior of institutional investors. This observation matches our intuition, as institutional investors rely extensively on short sales. Besides, incentives exist for institutions to mimic the behavior of others that do not exist for individuals. Trueman (1994), Olsen (1996), and De Bondt & Forbes (1999) argued that herding behavior occurs when financial analysts submit their earning forecasts in order to avoid the risk of inaccurate forecasts. Graham (1999) argued that financial analysts herd because they are concerned regarding their future labor market reputation relative to their colleagues. Brown et al. (2013) supported that institutional investors' herding behavior. Many studies that have investigated herding behavior in different groups of investors (e.g., individual and institutional investors) include Wermers (1999), Chiang (2008), Choi and Sias (2009), Puckett and Yan (2010), Gavriilidis et al. (2013), Kremer and Nautz (2013), Lakshman et al. (2013); and Zheng et al. (2015). However, there has been a dearth of scholarly discussion in the literature on the herding behavior of different types of investors under short-sale restrictions.

While a handful of studies do not support the existence of herding behavior during extreme market movements³, most agree that extreme prices or returns are accompanied by herding behavior. Falkenstein (1996) and many others have documented that funds are averse to stocks that have dropped significantly in price. Wermer (1999) found higher levels of herding in stocks that have extremely high or low past returns in comparison with the average. Hwang and Salmon (2004) provided empirical results of changing herding towards the market conditions in the US and South Korean stock markets. They found evidence of herding towards the market portfolio in both bull and bear markets. The finding of herding on extreme returns has important implications due to the positive-feedback effect between herding and stock returns. The common strategy of "momentum" that is adopted by investors has attracted considerable scholarly attention. Caparrelli et al. (2010) argued that a non-linear relationship exists between return dispersions and market returns in order to illustrate that herding behavior exists in the Italian stock market, especially during extreme market conditions. Recently, Sharma et al. (2015) investigating the presence of herding behavior in the Chinese stock market and found support for the asymmetric effect, with a greater magnitude of herding in up markets than in down markets. It is hoped that employing quantile regression will contribute to the general understanding of herding behavior under extreme positive and negative returns.

³ Gleason et al. (2004) examined nine sector ETFs that were traded on the American Stock Exchange. They find no evidence to support the existence of herding behavior during periods of extreme market movements using ETFs.

3.   Methodology

Two structure-change tests were employed to detect herding towards the lifting short sale price restriction. The Chow test was used when the specified date of an event was known and the DQ test was used when this date was not known.

We proposed the following equations for the Chow test to examine the impact of herding on stock returns:

The dependent variable, ARt, is the average abnormal returns for the Taiwan 50 small index and the Taiwan 50 Index, which represents 50 large firms at day t. We employ the event study of "Market-adjusted Model" to measure the abnormal returns: $A{R_{it}} = {R_{ii}} - R_{mi}^{}$. The expected return represents the contemporaneous return of a market index, and the abnormal returns are calculated by subtracting the return of a market index. The $\gamma$ is the coefficient that is used for estimation, Herding is the herding measurements that are distinguished by individual orders or institutional orders, and ut is the error term. Because the signs of the herding variable may have differing responses to the dependent variable, the coefficients under both periods are estimated. The different meanings of the coefficients may be clarified by comparing the two periods (short-sale price constraints in effect and short-sale price constraints ended).

In order to clarify the relationship between herding behavior and stock returns after short-sale constraints have ended, the present study employs a structural change regression model based on a quantile model rather than an ordinary least squares (OLS) model. The quantile regression model observes coefficients under different quantiles and may consider the characteristics of different quantiles. Thus, more information from the sample is shown in this model than the OLS model.

Short-sale constraints may cause stocks to become overpriced (Seneca, 1967; Miller, 1977, Figlewski, 1981; Aitken et al., 1998; Desai et al., 2002; Jones and Lamont 2002; Asquith et al., 2005, Boehme et al., 2006, and Harris et al., 2013) and the overpriced stock returns are placed on the lower quantiles. Besides, the relatively higher and lower quantiles allow observation of herding behavior on the relatively higher and lower stock returns. This echoes the finding in previous studies that higher levels of herding exist in stocks with extreme returns than in other stocks (Wermer, 1999; Chang et al., 2000; Christie and Huang, 1995).

As mentioned above, the Chow test was used when the date of an event was known. The Chow test is appropriate for data with known event dates. For data with unassigned event dates, the DQ test was used to find the potential structure change point in the quantile model. Thus, the present paper estimates structural changes that occur at unknown dates in conditional quantile functions.

The DQ test is based on a dynamic comparison of the residual sum of squares from each k breaks and k+1 breaks model. That is, we estimate the break dates and other parameters jointly by minimizing the check function over all permissible break dates:

where ${\rho _\tau }(u)$ is the check function given by ${\rho _\tau }(u) = u(\tau - 1(u < 0))$. Qu (2008) and Oka and Qu (2010) introduced the DQ test to provide a unified treatment of estimation, inference, and computation in quantile regression with unknown breaks. The limiting distribution of the estimator confidence interval is assessed using simulations and critical values that are provided in Qu (2008). It is important to examine whether and how the stock return differential between different herd levels has changed over time. Thus, the conditional quantiles should be considered.

Related to herding measurement, we employed the definition that was suggested by Lakonishok et al. (1992). Herding conceptualizes the buyer power that is related to all trades. The advantage of this herding measurement is that it shows the herding situation for each security and identifies the individual that places the order. In order to calculate the daily herding measurement as equation (5), the intra-day data of buys or sell orders of individual or institutional investors for firm I are used.

Investors hold different information perspectives on public information (Flglewski, 1982). Therefore, the present study distinguishes between the herding behavior of individuals and that of institutions. k represents either individuals or institutions in equation (5), while Bi, k and Si, k in equation (6) represent the buying and selling orders, respectively, of firm i of investor type k.

4.   Empirical results

4.1.   Data

The dataset comprised the daily data on stocks that are currently traded on the Taiwanese stock market. The sample period covers two distinct two-year periods, respectively, for the Taiwan 50 Index and the 50 small-sized firms index. The sample period was 2004/5/14–2006/5/15 for the Taiwan 50 Index, and 2012/9/23–2014/9/22 for the small firms index, with a total of approximately 495 days in each period.

The 50 small-sized firms index was constructed for the present study as a valid contrast to the Taiwan 50 Index in order to estimate the empirical results. The firms included in this latter group were chosen carefully.

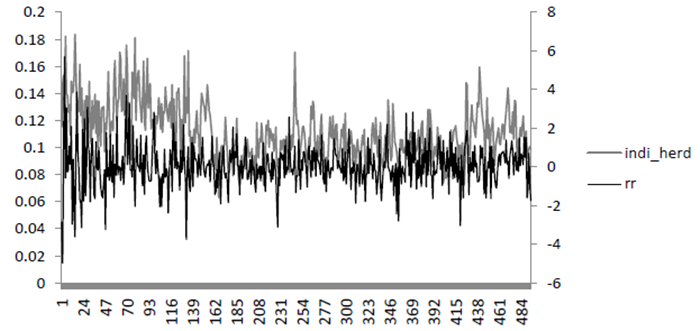
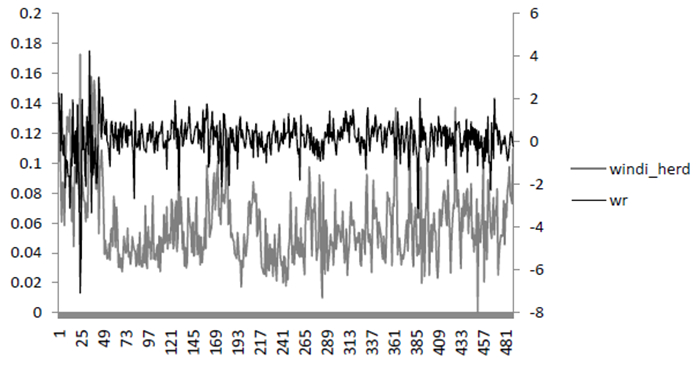
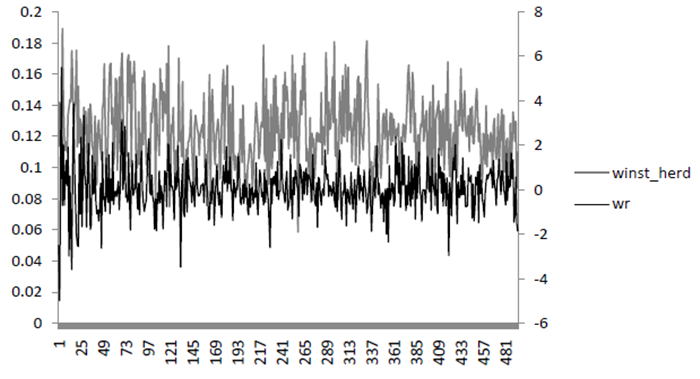
We draw the returns of ETF50 index calculated by market value or equally-weighted and herding measurements defined by individual investor behavior of the component stock of ETF 50 index in Figure 1A and 1B, respectively. Also, those patterns of top 50 smallest firms are shown in Figure 2A and 2B. Though it is difficult to judge a regular pattern from these lines, we can find the larger volatility of individual-herding measurements companied with the larger swings of returns for small-sized firms in figure 2A and 2B, which can be observed before time point 1 to time point 50.

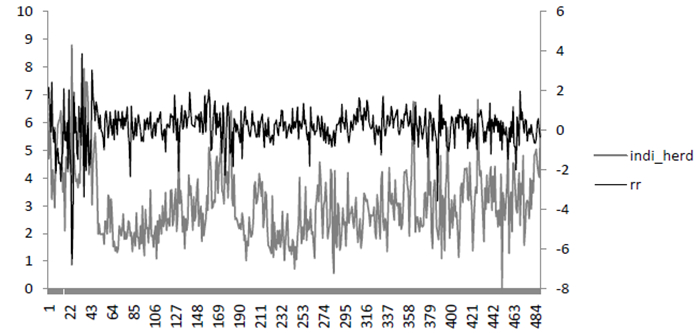
Then, we used the institutional orders to calculate herding measurements, and featured the lines of institutional-herding measurements and returns of ETF50 index in Figure 3. For comparing, the Figure 4 reports the lines of institutional-herding measurements and returns of small-sized firms. Similarly to Figure 2, we can observe the larger volatility of institutional-herding measurements companied with the larger swings of returns for small-sized firms in the initial part in Figure 4A and 4B. The patterns hints the connection of return of small-sized firms and herding measurements need to pay our attentions.

4.2.   Empirical results

To get the power evidence, descriptive statistics are reported in Table 1 and the OLS and quantile regression models are estimated and reported in Tables 2–6.

In terms of empirical results in Table 1, the skewness of daily returns across constituent firms of the Taiwan 50 Index was 0.146 and −0.082 prior to and after the ending of short-sale price restrictions, respectively. The level of skewness for large firms was smaller than that for small firms. By contrast, the average skewness of daily returns for small firms was −1.49 and −0.74 prior to and after the ending of short-sale price restrictions, respectively.

To learn whether the structure change model is appropriate, we show the relevant statistics in Table 2 for the abnormal return. Table 2 shows the F-statistics for the Chow Test, which indicates the appropriateness of the model of structural change. The F statistics were 0.58 and 1.16, which do not reject the hypothesis of no break for Taiwan 50 Index. When the institutional herding measurement was used as the explanation variable for small firms, similar results were observed. However, the F-statistic was 3.426, which rejects the hypothesis of no breaks for small firms when the individual herding measurement was used as the dependent variable. This implies that the structural change model is more appropriate than the OLS model. The model that allows dynamic searching of the break of date is shown on Panel B of Table 2. The break of date is 2013/1/14, which is earlier than the date when the policy was changed. The bottom row in Table 2 is a confidence interval ranging from 2012/10/30 to 2013/3/11. As a supplementary test, the dynamic structure point test justifies the need to distinguish between the time when short-sale restrictions were in force and the time when these restrictions had been lifted, although the structure point does not match perfectly with the specified date.

Table 3 reports the estimated coefficients of individual herding that were obtained using the structural change models that were developed, respectively, using the OLS method and the quantile regression model. Although the estimated results for all of the samples are reported in Panel B, the results that are shown in Table 2 suggest that the coefficients that were distinguished, respectively, before and after the removal of short-sale price restrictions are statistically preferable. Panel A of Table 3 shows that the short-sale-restriction-based bias in stock prices and the valuing of overpriced stock returns below expected returns are both associated with lower levels of individual herding behavior and larger stock returns for small firms. The relevant coefficients for the 0.2 and 0.35 quantiles are −18.25 and −13.05, respectively. However, this association disappears after short-sale price restrictions are lifted, with the exception of the 0.2 quantile (coefficient = −4.878). In other words, restricting the short selling of small stocks may increase herding behavior in individuals. This finding is consistent with the herding behavior that is frequently observed under conditions of extreme returns, especially extremely low returns (Wermer, 1999; Chang et al., 2000; Christie and Huang, 1995), affecting investors who are relatively averse to stocks that have recently dropped in price (Falkenstein, 1996). That may due to the problems of liquidity changes and market inefficiency which are caused by short-sale restriction of trading stock (Sias, 2004; Roon et al., 2013; Osamor et al., 2019). Besides, the problems is more deeply for small stock and the empirical results work in concert with El and Hefnaou (2019). They pointed that the low liquidity and low level of transparency encourage investors to herd. The resultant disappearance of small-firm-focused herding behavior may result from problems of liquidity and from market inefficiencies that were caused by the ending of short-term selling restrictions. Looking deeper, smaller stocks are harder to short and short-sale price constraints tend to impede the transmission of private information (Diamond and Verrecchia, 1987). Therefore, smaller stocks may become overpriced (Jones and Lamont 2002).

Table 4 shows the results of testing the conjecture regarding the effect of short-sale constraints on the herding behavior of institutional investors. Employing the quantile regression model, the coefficients in Panel A of Table 4 reveal that the impact of the herding variable is greater under the condition of short-sale price constraints. For example, values are significant at −4.672 for the 0.2 quantile, 2.381 for the 0.65 quantile, and 6.134 for the 0.8 quantile, although the coefficient does not reach significance in the OLS model. The positive coefficient of the herding variable means that small firms with higher levels of herding behavior tend to earn more, with these benefits increasing as the quantile increases (e.g., the 6.134 for the 0.8 quantile is larger than the 2.381 for the 0.6 quantile). This result contrasts with the findings of Lakonishok et al. (1992), which used a quarterly portfolio of 769 equity pension funds and found no evidence of herding behavior in small stocks. Similarly, Filip et al. (2015) supported that herding behavior of investors may lead to deviations of the stocks prices from their fundamental value. Thus, the deviations from true value let us observe the small companies with higher levels of herding behavior tend to have higher return.

The results shown in Panel B of Table 4 also conform to the position that herding behavior impacts negatively on low quantiles and positively on high quantiles. Both Table 3 and Table 4 agree that herding behavior significantly affects small firms under short-sale price constraints. The positive association between level of individual herding behavior and stock returns disappears after short-sale price restrictions end. This finding is consistent with Lakonishok et al. (1992), with the significantly higher level of herding in small stocks possibly due to the asymmetric information for short sellers under short-sale restrictions (Bai et al. (2006), Chang and Wang, 2006) or due to the lack of alternatives to short selling.

Table 5 shows the estimates for the constituent firms of the Taiwan 50 Index. Again, a quantile regression model with a given break date was used to capture the cases in which coefficients may differ for the two periods (i.e., before and after the lifting of short-sale price constraints). Surprisingly, most of the coefficients of individual herding measurements are insignificant in Table 5, regardless of period. This finding provides no evidence that herding by individual investors affected the stock returns of firms on the Taiwan 50 Index. Although the empirical results for small sized firms in Table 3, which support a herding-behavior bias, suggest that overpricing due to short-sale constraints is an important issue to individual investors, this suggestion is not supported for the large firms in Table 5.

The insignificance of herding variables in Table 6 is the same as that in Table 5. This empirical result contradicts the findings of Christie and Huang (1995) and Chang et al. (2000) that short-sale constraints affect the herding behavior of institutional investors. However, our finding of no significant effect of institutional herding behavior on stock returns echoes the finding of Bohl et al. (2013) that short-selling restrictions do not influence herding. Briefly, for Table 5 and Table 6, the hypothesis that the relationship between herding and stock returns differs based on the existence (yes/no) of short-sale price constraints is not supported for large firms in terms of either individual or institutional herding measurements.

Note that providing a robustness check, we had employed the beta risk as a single factor market model and famous Fama-French three-factors model which included market return, SMB, and HML factors. The results, which are not reported here, have identical evidences for the small-sized companies, but not for the large-sized companies when the lifting short-sale restriction event. We can not read evidence to say there is a connection of herding behavior and the larger firms for the lifting short-sale restriction event. However, before lifting the short-sale price constraints, there is negative coefficients of herding variable to return for small-sized firms and the results agree that restricting the short selling of small stocks may increase herding behavior in individuals.

5.   Conclusion

The effect of herding behavior on stock returns has been widely discussed in the literature in recent years. Although few studies have mentioned that no evidence currently exists to associate level of herding behavior and short-selling restrictions, no published research provides the empirical results necessary to assess the impact on herding behavior of lifting these restrictions. Thus, the present paper assesses whether the ending of restrictions on short selling changes the relationship between herding behavior and stock returns.

We employed a structural change model that included the Chow test and the DQ test. The first considers only known event days, while the latter allows the use of unspecified event days under quantile regression. Using the quantile regression model and the intra-day buying and selling orders of individual and institutional investors, the present paper observes how the herding behavior of these two distinct categories of investors affect stock returns under the events.

In particular, the two-stage elimination of short-selling restrictions in Taiwan were used in the present study to examine the discrete effects on highly capitalized blue-chip stocks and on all listed stocks, respectively. This elimination lifted short-sale restrictions for the constituent stocks of the Taiwan 50 Index in May 2005 and for all listed stocks in September 2013, allowing for short sales on any price tick, whether up or down. Our findings confirmed a negative relationship between individual herding behavior and the stock returns of small firms, particularly those in the low quantile of stock returns during the period of short-sale price restrictions. However, the lifting of short-sale price restrictions did not impact individual or institutional herding measurements. The present study thus supports that lifting short-sale price restrictions causes structural changes in herding behavior only for small firms and that these restrictions strengthen the association between the stock returns and the herding behavior of small firms.

Acknowledgements

This study was funded by the Taiwan Ministry of Science and Technology under project number MOST104-2410-H-025-008.

Conflict of interest

All authors declare that: (ⅰ) no support, financial or otherwise, has been received from any organization that may have an interest in the submitted work; and (ⅱ) there are no other relationships or activities that could appear to have influenced the submitted work.