1.
Introduction
Maize (Zea mays L.), a cereal crop adapted to a wide range of ecological conditions, is cultivated in all agroecologies of West and Central Africa (WCA) but primarily produced in the savannas. The savannas of WCA offer the highest productive environment for maize because of relatively high incidence of solar radiation, low night temperature and reduced occurrence of pests and diseases during the cropping season [1]. In 2018, the global maize production was 1.15 billion tonnes, 78.9 million tonnes came from Africa and 10.2 million tonnes from Nigeria [2]. The area under maize production in the savannas of WCA has increased at the expense of other traditionally cultivated cereal crops such as sorghum (Sorghum bicolor L.) and millet (Pennisetum glaucum L.) [3]. The acceptability of maize by farmers and its potential to combat food security challenges posed by population increase in WCA have greatly improved due to its high yield potential, wider adaptability to different environments, and relative ease of cultivation, processing, storage, and transportation [4,5]. However, maize production in most agroecological zones of WCA is constrained by three stresses: drought, low soil nitrogen (low N), and Striga infestation [3,6,7,8]. Presently, the extra-early (85–90 days to maturity) and early (90–95 days to maturity) maize hybrids, that combined high yield potentials with resistance/tolerance to the three stresses are available in sub-Saharan Africa (SSA) [3]. These maize hybrids have been adopted by the farmers in the region, although, grain yield in farmers' fields has averaged only 1 to 2 t/ha in contrast to the potential yields of about 5 to 7 t/ha reported for experimental stations [9]. Clearly, the heterotic advantage of the hybrids was not fully exploited. These hybrids are new to the farmers, and the existing agronomic recommendations such as N fertilizer rate, intra- and inter-row spacings, may not be appropriate to enable the full expression of grain yield potential of the hybrids.
Maize grain yield (GYLD) is largely influenced by the interaction between the genetic and management factors. Successful maize production depends on the adequate use of production inputs that will sustain the environment as well as agricultural production [10]. An adequate amount of N, phosphorus, and potassium must be supplied to maize crop for good development, growth and high yield [11]. Nitrogen performs an important role in crop life and is one of the most essential nutrients needed by maize plants in large quantities. Kamara et al. [12] reported severe yield losses in maize in Nigerian savannas when no mineral fertilizer was applied. Soils of SSA are characterized by low fertility due to continuous cultivation and heavy rainfall associated with the region. The quantity of fertilizer use in the region accounts for only 3% of global fertilizer use, an amount which has not improved over two decades [13]. Maize has potential for high yield and responds considerably to N fertilizer application. Generally, N-fertilizer application increases GYLD and yield components of maize. In many areas of Africa, improved maize cultivars are often grown with zero or inadequate rates of fertilizer. This may be one of the reasons for lower grain yield frequently obtained by the farmers in SSA. Correct application of N fertilizer and optimal use of plant density can maximally exploit the full grain yield potential of modern maize hybrids.
For improved maize production, optimal rate of plant density (PD) is an important factor. Low PD results in reduced GYLD, while high density leads to stress on the plants [14,15]. Plant density is dependent on both row width and intra- row spacing. In WCA, the intra-row spacing used by local farmers for open-pollinated maize varieties (OPVs) has been the same used for extra-early and early maturing maize hybrids (E-EH and EH) [16,17]. This also, could be associated with the low GYLD of the improved maize hybrids on the farmers' field. Furthermore, the use of high density under drought condition may heighten plant stress and reduce GYLD severely, specifically, if the drought coincides with the flowering and grain filling period [18]. Therefore, drought stress especially when combined with high PD can cause complete loss of grain production, if stress occurs during the tasselling and silking stages of production [19,20].
The use of hybrids that combine tolerance to drought and high density may be a promising production practice for the improvement of GYLD, particularly in drought-prone environments. The International Institute of Tropical Agriculture (IITA) and collaborators have developed and released E-EH and EH, that combine high yield potentials with combined tolerance to low soil-N and drought at flowering and grain filling periods, and have been adopted by the farmers in the sub-region. However, the tolerance of these hybrids to high density and N application has not been investigated. Such information can guide future breeding of new cultivars and cropping technique innovation. This study was conducted to (i) investigate the response of GYLD and other agronomic traits to PD and N rates of recently released four hybrids, belonging to extra-early and early-maturing groups, and the performance of each maturity group in different agroecologies of Nigeria, and (ii) partition the total variation in GYLD to its various components.
2.
Materials and methods
2.1. Experimental sites
This study was conducted during the growing season of 2015 at five locations, one location in each of five agroclimatic zones in Nigeria: Ile-Ife (Marginal rainforest—MRF: 07°28´N, 04°34´E), Ikenne (Rainforest—RF: 06°53´N, 03°42´E), Mokwa (Southern Guinea Savanna—SGS: 09°18´N, 05°40´E), Zaria (Northern Guinea Savanna—NGS: 12°00´N, 08°22´E) and Kadawa (Sudan Savanna—SS: 12°01´N, 08°19´E). The agroecologies of the experimental sites are the major maize growing zones in Nigeria. The characteristics of the experimental sites are described in supplementary Table S1. The climatic conditions that prevailed at each experimental site were variable but normal for all the agroecologies, although, there were more rainfall than usual during the evaluation period at Kadawa (SS) (Table S1). The SS agroecology is characterized as terminal drought-prone environment, but drought did not actually occur at the agroecology during the field evaluation period.
2.2. Germplasm and experimental design
Four maize hybrids (two each of extra-early and early maturity) recently released in Nigeria, Mali, and Ghana were evaluated. For each of the maturity group, one single-cross (SC) and one top-cross (TC) hybrids were evaluated. The hybrids are tolerant/resistant to low soil-N, drought and resistant to Striga with high yield potentials [21,22]. The detailed descriptions of the hybrids are presented in supplementary Table S2. Each experiment in each location was grown in a randomized complete block with a split-split-plot arrangement and three replications. The main plots were the urea fertilizer rates (90,120 and 150 kg N/ha), PDs (66,666; 88,888 and 133,333 plants/ha) were subplots, and the four hybrids were sub-subplots. The inter-row spacing of 0.75 m and three intra-row spacings of 0.4 m, 0.3 m and 0.2 m were used to obtain the three density levels. Each sub-subplot comprised four rows, 5 m long each. Three seeds were manually planted per hill and thinned to two plants/stand two weeks after emergence. The urea fertilizer was applied by side placement in two equal split applications at two and five weeks after planting. For each rate of the urea fertilizer, the initial soil N status (Table S1) of each experimental site was considered in determining the amount of N to be applied to ensure equal N level across the sites.
2.3. Data collection
Observations were made on the two central rows within each experimental plot. Data obtained included anthesis (ANTH) and silking (DYSLK) which, respectively were the number of days from planting to the date when 50% of the plants in a sub-subplot had shed pollen and emerged silks. Anthesis-silking interval (ASI) was computed as the difference between DYSLK and ANTH. Plant-height (PLHT) and ear-height (EHT) were measured as the distance from the base of the plant to the height of the first-tassel branch and to the node bearing the upper ear, respectively. Root-lodging (RL) was determined as the number of plants leaning about 45° or more from the upright position. The number of ears per plant (EPP) was determined by dividing the total number of ears per plot by the number of plants harvested. Plant aspect (PASP) was based on the overall plant appeal (visual), considering factors such as relative plant and ear heights, lodging, uniformity, reaction to diseases and insects and was scored on a scale of 1 to 9, where 1 = excellent plant type and 9 = poor plant type. Ear aspect (EASP) was based on freedom from disease and insect damage, ear size, uniformity of ears, and grain filling and was scored on a scale of 1 to 9, where 1 = clean, uniform, large, and well-filled ears and 9 = ears with undesirable features. Field weight was recorded as the weight in kg of all de-husked ears (cobs) in the sub-subplot. To determine grain moisture content, five representative cobs were selected and grains removed from their cobs. The moisture content of the grains was measured with grain moisture tester (Model PM-450, Kett Electric Laboratory). Grain yield in kg ha-1 was determined on field weight basis at 15% moisture content, and 80% shelling percentage was assumed for estimating the grain yield.
2.4. Statistical analysis
Analysis of variance (ANOVA), combined across trial environments was performed on plot means for the individual traits with PROC GLM in SAS using a RANDOM statement with the TEST option [23]. In the combined ANOVA, environment (E), and replication nested within E was considered as random effect for each trait, while N, PD, and genotype (G), and their interactions were considered as fixed effects, and interactions involving E were considered as independent effect. Comparison between and within maturity group was achieved by partitioning the G sum of squares into orthogonal contrasts. Linear regression was fitted to quantify the GYLD and other traits responses to PD. The proportion of total variation in GYLD accounted for by the different sources of variation in the combined ANOVA was manually computed by dividing the individual sum of square of each source of variation by the total sum of square, estimated in percentage. The estimates of broad-sense heritability (H2) for GYLD were computed for each environment. All the environments included in the present study revealed an H2 value of ≥ 0.30 (Table S1). The H2 of GYLD was estimated as follows:
where σg2 is the variance attributable to genotypic effects, σe2 is experimental error variance; and r = the number of replicates within each environment [24]. Repeatability (R) estimates of GYLD and other agronomic traits across environments were calculated on a hybrid-mean basis as follows according to Falconer and Mackay [25]:
where σge2 is the variance attributable to genotype x environment effects, and e is the number of environments; σg2, σ2, and r as defined above.
3.
Results
3.1. Field performance of extra-early and early hybrids and repeatability of traits
The combined ANOVA showed highly significant genotype (G), environment (E) and G x E interaction mean squares for grain yield and all other measured traits (Table 1). The contrast analysis for the two maturity groups (extra-early vs early maturing hybrids) showed highly significant mean squares for all the traits (Table 1). The between-group comparison for grain yield accounted for 56% of the variation among genotypes. The early maturing hybrids consistently out-yielded the extra- early maturing hybrids in all the agroclimatic zones with an average of 428 kg ha−1 (16%) across environments (Table 2). Furthermore, mean squares of the orthogonal comparison of single-cross (SC) extra-early versus top-cross (TC) extra-early, and SC early versus TC early (comparison within each maturity group), were highly significant for grain yield and most traits (Table 1). For each maturity group, the SC hybrid consistently out-yielded the TC hybrid in all the agroclimatic zones. Across agroclimatic zones, the SC out-yielded TC by 420,343 and 381 kg ha−1 for the extra-early, early maturing groups and across the maturity groups, respectively.
For plant and ear heights, the extra-early hybrids were consistently taller and had higher ear placement than the early maturing hybrids in all the agroclimatic zones, except in the MRF zone where the early hybrids were taller. The TC extra-early hybrid (TZEE-Y Pop DT SRT C5 x TZEEI 58) was consistently taller and had higher ear placement than the SC extra-early hybrid (TZEEI 29 x TZEEI 21) in all the agroclimatic zones, except in the MRF zone where SC had taller plants and higher ear placement (Table 2). Conversely, the SC early hybrid (TZEI 124 x TZEI 25) consistently had taller plants with lower ear placement when compared with the TC early hybrid (TZE-W Pop DT STR C4 x TZEI 7) in all the agroclimatic zones (Table 2). Also, root lodging was higher for the extra-early than for the early maturing hybrids, although, the lodging was more pronounced at the forest than the savanna locations (Table 2). In each maturity group, root lodging was higher for TC than for SC hybrids (Table 2).
It is striking to note that both maturity groups received good scores (in the range of 3 and 5) for plant aspect (PASP) in all the agroclimatic zones, except for extra-early hybrids that received a poor score (6) in the marginal rainforest (MRF) zone. For ear aspect (EASP), both maturity groups received poor scores (6) at the two forest locations, and good scores (4 and 5) at the savanna locations (Table 2). The repeatability estimate of grain yield was 0.83 (Table 1). The repeatability estimates of other agronomic traits varied from 0.40 for EASP to 0.90 for silking (DYSK) (Table 1). Most of the measured traits of the hybrids indicated high repeatability estimates (i.e., ≥ 60) across the three N levels, three plant densities (PDs) and five environments (Table 1).
3.2. Response of grain yield and agronomic traits to plant density
Plant density (PD) mean squares were highly significant for all traits except anthesis (ANTH), plant height (PLHT), and ear height (EHT). The environment x plant density (E x PD) interaction had a highly significant effect on grain yield (GYLD), ears per plant (EPP), PASP, and root lodging (RL). However, plant density x genotype (PD x G) interaction and other interactions involving PD had no significant effect on grain yield and most traits (Table 1).
Across locations, GYLD decreased significantly as PD increased although; the decrease was only about 6% from the lowest (66,666 plants ha−1) to the highest (133,333 plants ha−1) density (Table 3). The trend in PD response across genotypes and N rates was different within locations for grain yield. For each of the two forest locations, grain yields at 66,666 and 88,888 plants ha−1 were not significantly different but were significantly higher than grain yield at 133,333 plants ha−1. In contrast, grain yields at the three densities were about the same in each of the three savanna locations (Tables 3 and 4), suggesting that yield reductions associated with increased PD were lower in the savannas than in the forest locations. On average across densities, however, the savanna locations were 357 kg ha-1 (about 14.4%) higher yielding than the forest locations.
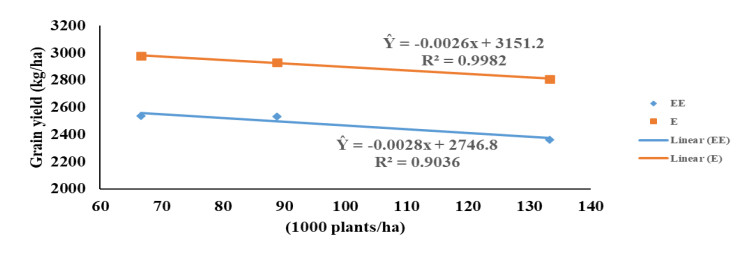
The overall grain yield of the hybrids of each maturity group across N rates and locations, showed a negative linear response to plant density (Figure 1). The predicted maximum grain yield from the negative linear response to PD was obtained at approximately 66,666 plants ha-1 for the range of plant densities used in this study (Figure 1). Extra-early and early hybrids had similar linear trends for PD, although, the regression parameters were higher for the early than extra-early hybrids (Figure 1). Rates of decrease in grain yield related to increased PD were roughly the same for all hybrids except for the SC extra-early hybrid, TZEEI 29 x TZEEI 21, which had a lower rate relative to others (Table 4). The R2 value (0.0616) for the linear response of TZEEI 29 x TZEEI 21 to PD was far lower than those obtained from the linear responses of other hybrids (Table 4).
The response of EPP to PD was similar to that of grain yield; that is, it decreased with increased PD (Table 3). On average across PD, the number of EPP produced in the savanna locations were about 9% higher than those produced in the two forest locations. The trait (EPP) exhibited a negative linear response to PD (Table 4). In contrast, the mean values of PASP and RL increased significantly with increased density, although the mean values were much lower in the savanna than in the forest locations (Table 3). The respective linear trends in the PD response (averaged across the four hybrids, three N rates, and five locations) for PASP, EASP, anthesis-silking interval (ASI), and RL were all positive (Table 4).
The coefficients of determination, R2 of the traits ranged from 89.29% for PASP and EASP to 100% for RL (Table 4), indicating high reliability of the linear regression models for the traits.
Interestingly, EASP and PASP had the same R2 value (89.29%) and the rate of increase in score value (2E−05) associated with increased PD, but the intercepts were quite different about 3.8 and 2.8 for EASP and PASP, respectively (Table 4).
3.3. Response of grain yield and agronomic traits to N rates
The combined ANOVA showed no statistical significance among the three rates of applied N on grain yield and all other measured traits (Table 1). The effect of the three N rates for grain yield across the three plant densities and five environments was the same for all the genotypes (Table 1). Although, across the four genotypes, three plant densities and five environments, the highest grain (2734.34 kg ha−1) was obtained at 120 kg N ha−1, but was not different statistically with grain yield (2681.43 kg ha−1) obtained at 90 kg N ha−1 (data not shown). Thus, the hybrids showed no response to N-fertilizer application rates above 90 kg N ha−1. N x PD interaction, as well as all other interactions involving N, had no significant effect on all the studied traits except in a few cases such as RL (Table 1).
3.4. Partitioning of the total variation in grain yield into its various components
The E, G, and G x E interaction mean squares in that order, were the most important contributors determining grain yield. Environment, the largest contributor, accounted for 23.5%. The variance accounted for by the G and G x E interaction sum of squares were 19.4% and 6.4%, respectively (Table 5). Partitioning of the variance contribution of the genotype sum of square revealed that orthogonal contrast between maturity groups (extra-early vs early hybrids) accounted for 55.5%, and within each maturity group accounted for 26.7% (SC vs TC extra-early hybrids) and 17.8% (SC vs TC early hybrids). The N and PD sum of squares accounted for 0.2 and 1.3%, respectively (Table 5). Thus, the PD sum of square contribution to the total variance in grain yield was more important relative to that of N. The variance magnitude accounted for by the remaining components ranged from 0.2% for N x PD and G x N interaction to 6.6% for Rep(Environment) sum of squares. The summation of the variance accounted for by the three Error terms (i.e., Error a + Error b + Error c) and replications gave approximately 38%. This proportion of the total Error variance was high, particularly, the magnitude of the residual, Error c (24%) (Table 5).
4.
Discussion
In an effort to combat the major stresses (drought, low soil N, and Striga infestation) constraining maize production in WCA, breeders have developed and commercialized extra-early and early-maturing maize hybrids that combine high yield potentials with tolerance/resistance to the stresses and are being adopted by the farmers in the sub-region. Hybrids of both maturity groups are new to the farmers; therefore, there is a rather urgent need to recommend appropriate agronomic practices for such hybrids in the whole of WCA. In this study, the lowest density level used (66,666 plant ha−1) and N fertilizer rate applied (90 kg N ha−1) were those presently recommended for the two maturity groups. The recommendations were based on open-pollinated varieties (OPVs) in the two maturity groups developed and evaluated in the NGS zones many years ago. It was desirable to increase the rates for hybrids of these maturity groups to take advantage of the heterosis to increase production. The results showed that the hybrids were intolerant of high plant densities and could not take advantage of higher N rates to increase production. The results of this rather preliminary study partly confirm and refute existing knowledge, and partly open new areas for further research on the agronomy of early and extra-early maize in the different agroclimatic zones of WCA. Our study confirmed the presence of significant differences in the performance of the hybrids both between and within maturity groups for grain yield and most other traits, as earlier reported by several researchers who worked in some of the agroecologies used in the present study [26,27 inter alia]. In another study, Oluwaranti et al., [28] found no significant differences among varieties within maturity groups for grain yield, vegetative and flowering traits. That study, which involved only OPVs, was conducted in the two seasons of the MRF agroecology used in the present study. The inconsistency of their findings with those obtained in the present study could be attributed to the difference in genetic materials and experimental design or methodology employ.
The significant G x E interaction effect observed for grain yield and other agronomic traits is another confirmation of existing common global knowledge of maize evaluation trials [21,29,30,31]. In this study, the significant G x E interaction mean squares for grain yield was magnitudinal rather than directional; that is, the differential grain yield performance of the genotypes in all the studied environments was only in magnitude (differences in the grain yield means) and not in ranking. The early hybrids were consistently higher yielding than the extra-early hybrids in all of the agroclimatic zones, even in the Sudan savanna location, a terminal drought-prone environment, where the extra-early hybrids would be expected to produce higher yield than the early hybrids, which are later maturing and could have been a victim of the terminal drought. This result was not surprising because terminal drought did not actually occur during the field evaluation at this location, as shown by the rainfall data for the location (Table S1).
Partitioning the existing G x E interaction into its components is desirable when efforts are directed to releasing varieties into the ecologies of their best adaptation. Results of this study clearly indicated that extra-early hybrids are not better adapted to the Sudan savanna agroecology, particularly, when drought does not actually occur during the growing seasons at the ecology. In general, early hybrids are only higher yielding in all agroecologies, including those which have longer rainy seasons, they are not necessarily better adapted to the ecologies than the extra-early hybrids. Early maturing hybrids take a longer period to complete necessary physiological processes and grain filling before physiological maturity than extra-early maturing hybrids. The results of this study confirmed the existing knowledge of the environmental physiology of maize that extra-early varieties, including hybrids, are not necessarily more suitable than the early varieties for the Sudan savanna and, by inference, other terminal drought-prone environments and short rainy season, such as the late season in the marginal forest agroecology of WCA, unless terminal drought really occurs [32,33,34].
Furthermore, the results of this study also, revealed that the yield performance of SC hybrids was superior to that of TC hybrids irrespective of the maturity group. The grain yield advantage of SC hybrids over the TC hybrids may be related to the variation in their genetic background and perhaps in the level of expression of heterosis. This is because SC hybrids give the maximum degree of heterosis. The higher grain yield performance of the SC hybrids may also be related to the consistent lower ear placement and reduced root lodging reported in this study (Table 2). Many researchers have reported that higher ear position could increase the susceptibility to root lodging, particularly in the extra-early, and consequently, a significant reduction in grain yield [35,36]. Therefore, the relatively lower grain yield of the TC hybrids in the present study may be linked with the higher ear placement, as well as higher root lodging consistently obtained for these hybrids in all the studied environments. Differential performance of the two hybrid types also contributed to the significant G x E that occurred in the present study. Whereas SC hybrids were about 21% higher yielding than the TC hybrids at Zaria in the Northern Guinea Savanna (NGS), they were about 17% higher yielding in the MRF and only about 13–14% better in all other agroecologies (Table 2).
Enhanced adaptation to high PD is key to maize grain yield improvement [29]. Optimization of plant density and fertilizer levels result in increased grain yield per unit land area. Investigating the PD and N fertilizer response of commercial hybrids offers invaluable information that can guide breeders in breeding new cultivars, and or in modelling innovative cropping techniques for grain yield improvement. In the present study, grain yield and other studied traits showed no significant response to N application. This result is at variance with the findings of other researchers such as Ahmad et al. [37], who reported significant differences among eight N rates (0, 30, 60, 90, 120, 150, 180 and 210 kg N ha−1). Al-Naggar et al. [38], also obtained significant differences among three N rates (0,285 and 570 kg N ha−1); and in another study by Qian et al. [39], significant differences were similarly obtained among four N rates (0,150,300 and 450 kg N ha−1). It was particularly striking that early and extra-early hybrids evaluated in our study did not respond to N fertilizer above 90 kg ha-1, whereas those evaluated in earlier studies, especially the more recent studies [37,38,39,40,41], responded to 150 kg ha−1 and higher rates. Because farmers in SSA, on average, apply less than 10 kg N ha−1 to maize [6,21], IITA and International Maize and Wheat Improvement Centre (CIMMYT) researchers, along with their national program collaborators are now developing low-N (about 30 kg N) tolerant maize germplasm. Recent studies by Badu-Apraku et al. [3,21,42], showed that, in addition to being low-N tolerant, the resulting germplasm had the value addition of being high yielding at a high level of N, usually 90 kg ha−1. The present study was the first attempt at evaluating such material at N rates higher than 90 kg ha−1. Perhaps the N response in the present study would have been more informative and adequate, if low N rates such as 0, 30 and 60 kg ha−1 had been included in the study. Seemingly, the greater challenge to maize breeders in SSA now is to develop hybrids that would respond to high N rates for increased grain yield in commercial farms that can afford high input levels. By implication, this challenge also extends to density response, along with the non-significant N x PD interaction mean square for grain yield both of which made it impossible to determine the response surface combinations of N and PD in our study.
Lack of significant G x PD interaction effect in this study indicated that the hybrids had similar response of reduced performance in grain yield and other traits as PD increased, a confirmation of results of earlier studies on the subject-matter [10,39,41]. Generally, the extra-early and early maturing hybrids were intolerant of high density. Therefore, selection and development of hybrids or lines under high plant population density may be a promising strategy to improve the tolerance and adaptation of hybrid maize to higher PD. In contrast to the hybrids, PD response within locations for grain yield, yield components, and few other agronomic traits varied significantly and this was indicated by highly significant PD x E mean squares for the traits; a valid justification for extensive evaluation of density response in multiple environments in order to draw conclusion and before recommendation could be made.
Across PD, grain yield performance in the savanna locations was about 14.4% higher than in the forest locations, thereby supporting Badu-Apraku et al. [1] that the savanna agroecologies are the most favorable environments for maize production in WCA. PASP and EASP score values increased with increased PD. This suggests that the general plant and ear phenotypic appeal becomes poorer with increased PD, implying that the overall plant and ear traits such as uniformity of stand, uniformity of plant and ear heights, lodging, resistance or reaction to diseases and pest, general growth and development of plant and ear, uniformity of ears, and flowering were all influenced by PD. This appears to be a general response by the plant during the growth and reproductive stages due to a reduction in photosynthate formed during these stages resulting from intense interplant competition for growth resources. Similarly, ASI value increased significantly with increased PD. Results from other researchers have consistently shown that increased ASI is associated with increased PD due to the increased number of days to silking after anthesis [43,44,45]. The increased ASI values associated with increased PD may be related to the stress imposed on the maize plant due to intense interplant competition for light, water, and nutrients resulting from increased plant population. Kamara et al. [46] reported that increased ASI is a useful indicator of density stress in maize and that, by implication, could be an effective trait to use for selecting density-tolerant varieties.
The savanna locations produced a higher number of ears per plant (EPP) than the forest locations, indicating that barrenness was more pronounced in the forest than in the savanna locations. This is probably one reason; higher grain yield was obtained in the savanna than forest locations in this study. Fakorede et al. [47], in yield trials conducted for four years, found that the yield advantage of the savanna over forest locations was due primarily to ear number. In the present study, EPP reduced and, by implication, barrenness increased with increased PD. Conversely, root lodging increased with increased PD. The increased root lodging obtained as PD increased may be attributed to stress resulting from interspecific competition for growth resources imposed by the increased plant population density. More so, the magnitude of root lodging was larger in the forest locations than in the savanna locations, implying that lodging is also largely dependent on the environment. The high repeatability estimates obtained for grain yield and most agronomic traits across the agroclimatic zones implied that the expression of the traits would be consistent under the levels of N fertilizer and plant densities.
Partitioning the total variance of multi-environment trial data into its various components among experimental factors and their interaction effects offers researchers the convenience to separate and compare the relative importance of the different variance components. In this study, E had the largest share of the total variance in grain yield but was only about 4% higher variation than the G. This is not surprising because the genotypes evaluated were improved cultivars and by implication optimization of the growing condition of the hybrids may result in a significant improvement of their grain yield. Also, the variance estimate of G was 13% higher than that of the G x E interaction. This trend of components of total variance; E ˃ G ˃ G x E has been consistently observed in earlier studies in WCA [8,42,48,49]. The closeness of G to E in this study is encouraging, an indication that proper management of the E as done in this study will reduce its masking effect on the performance of the genotypes. However, the unexpectedly large estimate of the total error variance obtained in this study suggested that more attention is still needed to minimize unexplainable sources of error in agronomic trials conducted in WCA. It is a common and routine practice of agronomists and breeders to conduct yield trials in multi-environments (locations and years) so as to identify high yielding and stable genotypes. It is, however, challenging that the effects of various management (M) practices on cultivar adaptation have not been given keen attention. Improved M practices are essential for improving grain yield particularly when the crop is managed under high plant population density. High PD generally results in increased inter-plant competition for growth resources. Such conditions can be improved with best M that involves effective control of pests, diseases, and weeds, uniformity of plant stands, and consequently, effective utilization of solar radiation, soil water, and nutrients by the maize crop. Breeding and agronomic decisions have primarily been based on G x E interaction but maize scientists seem to have neglected the significance of G x E x M.
5.
Conclusions
In summary, the DT extra-early and early-maturing maize hybrids were genetically distinct, with the early maturing hybrids producing higher grain yield than extra-early hybrids in all the studied environments. Irrespective of the maturity group, the single-cross hybrids expressed greater yield performance relative to the top-cross hybrids primarily due to the variability in their genetic background, as well as the lower ear placement and reduced root and stalk lodging associated with single-cross hybrids. Grain yield advantage of the savanna locations relative to the forest locations was attributed to the number of ears per plant. The E, G, and G x E were the most important factors determining variation in grain yield. No significant difference was found for grain yield and other traits among N rates of 90 to 150 kg N ha−1. Plant density, however, was found to affect grain yield and most of the studied agronomic traits significantly; grain yield and EPP exhibited negative linear responses, whereas ASI, PASP, EASP, and RL showed positive linear responses to plant density. The results of our investigation indicated that the genotypes were intolerant of high plant density. We suggested that breeding programs for the improvement of early and extra-early maize germplasm for high plant density tolerance should be initiated in the sub-region. The results of this study, however, showed that 90 kg N/ha and 66,666 plants/ha were optimal for the production of extra-early and early-maturing maize hybrids across the agroclimatic zones of Nigeria.
Acknowledgments
The authors are grateful for the financial support of the Drought Tolerant Maize for Africa (DTMA) project by Bill and Melinda Gates Foundation (OPP1134248) and IITA for this research. We are also grateful to the staff of the Maize Improvement Program of IITA for technical assistance.
Conflict of interest
The authors declare no conflict of interest.
Supplementary Tables









 DownLoad:
DownLoad: