Adaptive group testing in a compartmental model of COVID-19^*

1.   Introduction

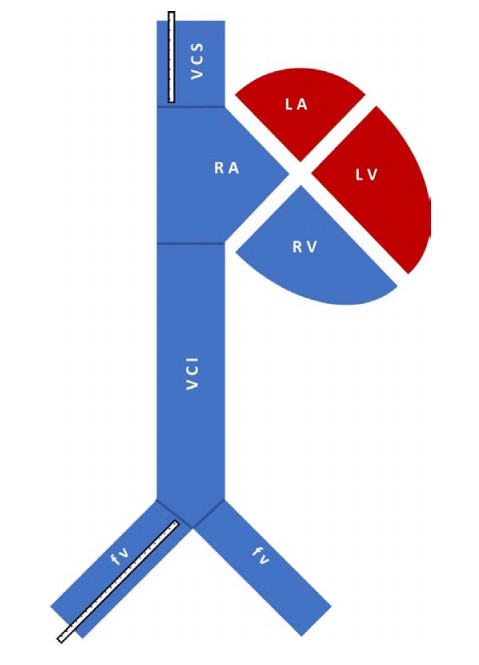
The hemodynamic situation of critically ill patients is often complex and can change rapidly. To optimize oxygenation and organ perfusion it is important to balance intravascular fluid demand against hypervolemia, which is associated with adverse effects like pulmonary oedema ^[1,2]. Advanced hemodynamic monitoring provides detailed information about the cardiovascular status ^[3] and consequently became a core element in the management of seriously ill patients ^[4,5]. Transpulmonary thermodilution (TPTD) was introduced more than 20 years ago and has evolved into a fundamental and widely used tool in the intensive care unit (ICU) setting ^[6]. Besides measurement of cardiac index (CI) and extra vascular lung water index (EVLWI), the TPTD technique provides global end-diastolic volume index (GEDVI) as a preload marker. This volumetric parameter corresponds to the blood volume in the heart, assuming that all four heart chambers are simultaneously in the diastolic phase. In contrast to stroke volume variation (SVV) and pulse pressure variation (PPV), both dynamic preload parameters obtained from pulse contour analysis, the static equivalent GEDVI is reliable, even when sinus rhythm and controlled mechanical ventilation are absent ^[7,8,9]. GEDVI is calculated based on the mean transit time (MTt), i.e. the period of time during which half of the indicator travels from the central venous catheter (CVC) to the arterial thermistor. Jugular or subclavian venous access is considered to be the gold standard for TPTD as the catheter tip is located in the superior vena cava (VCS) close to the right atrium and allows direct indicator injection into the right heart without any interposed circulatory segments. However, in 20 to 35 % of all cases jugular central venous access is not practical due to burns, polytrauma, thrombosis of the internal jugular vein or infections of the catheter site (Figure 1) ^[10,11]. The CVC is inserted in the femoral vein instead and the additional volume of the vena cava inferior (VCI) therefore enlarges the distance, which the indicator passes from the CVC tip to the aortic thermistor. This extends particularly the MTt and its subordinate parameters resulting in a marked overestimation of GEDVI ^[12,13].

Figure 1.  Transpulmonary thermodilution with jugular and femoral indicator injection. RA right atrium; RV right ventricle; LA left atrium; LV left ventricle; VCS vena cava superior; VCI vena cava inferior; fv femoral vein.

DownLoad: Full-Size Img PowerPoint

To take the femoral CVC position at TPTD measurements into account and adjust the artificially elevated GEDVI, a correction formula has been developed in a small collective of critically ill patients ^[14]. The latest generation of the PiCCO^® monitoring system (Pulsion Medical Systems PiCCO₂^® and PulsioFlex^® platform) adjusts GEDVI for femoral CVC placement ^[15,16]. A recent validation study confirms the benefit of the currently implemented correction function but suggests further investigations to achieve more accurate and precise measurement results in this modified setting ^[17]. Thus, our prospective study aims at first confirming and then further improving the efficacy of the correction formula using a large number of data sets. Finally, we validate the revised formula using retrospective data.

2.   Patients and methods

2.1.   Patients

This prospective study was carried out from November 2018 to January 2020 in the medical ICU of the German university hospital Klinikum Rechts der Isar, Technical University of Munich. In total, 38 adult patients were included in the study. All patients required hemodynamic monitoring as well as intravascular catheters for medical indication independently from the study. Approval was granted by the local ethics committee (Technical University of Munich, project number 3049/11s). All patients or their legal representatives gave their written informed consent according to the Declaration of Helsinki.

2.2.   Transpulmonary thermodilution measurements

For this study, patients were simultaneously equipped with three catheters: a thermistor-tipped arterial line in the femoral artery (PV2015L20-A PiCCO^® catheter; Pulsion Medical Systems SE, Feldkirchen, Germany) and two central venous lines. These were inserted in the jugular or subclavian and in the femoral vein respectively, with one catheter on each side of the diaphragm and their tips ending in the superior and inferior vena cava. Most of the patients enrolled received fluid and drugs via a CVC and required additional central venous access in the form of a 3 lumen Shaldon catheter for renal replacement therapy because of acute kidney failure.

Possible combinations were a CVC (Multicath 5; Vygon, Aachen, Germany) and a 3 lumen Shaldon catheter (GamCath, Gambro, Hechingen, Germany) or two CVCs. Occasionally, a CVC had to be transferred from a supra- to an infra-cardial position or vice versa, due to clinical reasons, such as suspected infection. In this case, TPTD measurements within the study were performed while both CVCs were in situ. Catheter type and position were chosen solely as a result of medical necessities. A PiCCO₂^® or PulsioFlex^® monitor (Pulsion Medical Systems SE, Feldkirchen Gemany) was used for the measurements according to the manufacturer's instructions.

Before TPTD measurement, arterial and central venous pressure transducers were calibrated to atmospheric pressure. Furthermore, basic information about the patient (date of birth, sex, height, body weight), CVP and the CVC site (jugular/femoral) were entered in the PiCCO^® software. For the thermodilution procedure, a bolus (15 ml) of ice-cold saline (0.9% sodium chloride, 4℃elsius) was injected quickly via the CVC or the small lumen of the Shaldon catheter, mixed with the bloodstream and passed through right heart, lung and left heart further into the aorta. The thermistor at the tip of the arterial line recorded the transient decline of blood temperature and thereby generated a thermodilution curve. After a minimum of three repetitions with comparable thermodilution curves, mean values for CI, GEDVI and EVLWI were calculated based on the TPTD curve. Indicator injection was performed either via the distal lumen of the CVC (volume 0.48 ml) or the third, small lumen for i.v. administration of the Shaldon catheter (volume 0.52 ml), as both catheter lumina have a similar diameter, length and consequently an equivalent volume.

Each measurement series (in technical triplicates) in this study consisted of three TPTD measurements: one jugular and one femoral measurement with the jugular CVC site selected in the device settings and one femoral measurement using the internal correction function for femoral indicator application by selecting the femoral CVC site in the device settings. These measurements were labelled as '_jug', '_fem_uncorr' and '_fem_corr', respectively. Thus, one TPTD measurement series consisted of 9 single thermodilution procedures and in total, 135 ml of fluid were injected per measurement series. Throughout the same series, respirator settings and catecholamine dose remained unchanged, the patient was laid flat and it was refrained from extensive fluid administration. The three TPTD measurements per series were carried out in random order to avoid systematic bias by potential volume effects of the previously administered fluid boli. For the same reason, the interval between two measurement series had to be minimum 24 hours and the injected volume was included in the daily volume balance of the patient.

2.3.   Mathematical background

GEDV is obtained by deducting the pulmonary thermal volume (PTV) from the intrathoracic thermal volume (ITTV). These volumes rest upon different parts of the thermodilution curve and can be further broken down (Eq 1).

(CO cardiac output; derived from the area under the curve; often displayed as cardiac index (CI) after adjustment to body surface area (BSA); Dst downslope time)

In case of indicator injection via the femoral vein, the indicator additionally passes the volume of the VCI and leads to an elongated mean transit time (MTt), which is the interval half of the time the indicator volume needs to travel from the injection site to the aortal thermistor. GEDVI (adjusted to BSA) therefore shows a tendency of overestimation, as its calculation partly rests upon MTt. Saugel et al. developed the following formula (Eq 2) to correct GEDVI in case of femoral indicator injection ^[14]:

(GEDVI_fem_corr global end-diastolic volume index derived from femoral indicator injection with application of the correction function (ml/m²); GEDVI_fem_uncorr global end-diastolic volume index derived from femoral indicator injection without applying the correction function (ml/m²); CI_fem cardiac index derived from femoral indicator injection; IBW ideal body weight (kg))

However, CI and extravascular lung water (index) (EVLW(Ⅰ); after indexation to body weight; deduced from the exponential decrease of the thermodilution curve, i.e. Dst) are barely affected by femoral indicator injection ^[14,18].

2.4.   Statistics

Categorial data is displayed as absolute and relative frequencies. Continuous data is presented as mean ± standard deviation (SD).

As each patient contributed to the dataset with a various number of measurement series (between one and five), it was necessary to level their impact and avoid skewing. We therefore included the first measurement series of all patients in one set (38 datasets; in the following referred to as set A) and the averaged values of each patient's measurement series (38 datasets; hereinafter called set B) in another set. Statistical testing with McNemar test and t-test for paired samples and further calculations were performed separately on each set.

In Bland-Altman analyses we compared the gold standard GEDVI, which is derived from jugular indicator injection (GEDVI_jug), to GEDVI results obtained from femoral indicator injection with (GEDVI_fem_corr) and without (GEDVI_fem_uncorr) application of the correction function ^[19].

To identify potential variables or their combinations for the development of a revised prediction formula, 9-fold cross validation was performed on the complete dataset of 98 TPTD measurements. This method was preferred, because all available samples can be utilized for both: first determining parameters for a new correction formula and afterwards developing the final version of it (Figure 2). Therefore, evaluation and predictions are based on the largest possible number of measurements.

Figure 2.  Steps of formula development and cross validation. a) The flow chart depicts the process of formula development. b) Cross validation (exemplarily shown with five folds): As a first step, a formula is developed with the datasets in the four coloured folds and then tested with the datasets of the crosshatched fold. Next, one of the coloured folds becomes the crosshatched test fold and the procedure of formula development and testing is repeated. The validation is completed when each fold has served as a test fold.

DownLoad: Full-Size Img PowerPoint

We applied a blocked subtype of the cross validation procedure, as it takes the data structure with partly repeated measurements into account. That means, all measurement series of a single patient were assigned to either the training or the test group of the cross validation model but did not contribute to both of them within the same fold. Subgroups were formed to ensure an even, proportional distribution of patients with the same number of measurement series to the test folds. Each of these subgroups contained patients contributing the same number of measurements (e.g. all those with three measurements etc.). The number of patients in every single subgroup was then evenly distributed among the nine folds of the cross validation model and the individual patients were randomly assigned to the preselected folds. This resulted in nine cross validation folds, each with ten to eleven TPTD measurement series derived from four to five patients. To evaluate the performance and prediction accuracy of various variables and their arrangements, we calculated the mean absolute error (MAE), root mean square error (RMSE), residual sum of squares (RSS), total sum of squares (TSS) and adjusted R² using the cross validation procedure described above. Pearson coefficient of correlation allowed comparison of different models with the gold standard GEDVI_jug. As a reference, cross validation was initially carried out with the parameters of the integrated correction formula (GEDVI_fem_uncorr, cardiac index derived from femoral indicator injection (CI_fem) and ideal body weight (IBW)) and its efficacy was assessed by the afore mentioned performance indicators, too. After selection of the most promising variables, we integrated these in a generalized estimating equation (GEE) model, which is aligned with different numbers of repeated measurements. For reasons of comparability the number of variables was limited to three, similar to the correction formula of Saugel et al. ^[14].The GEE model revealed the final version of the revised correction formula on the base of all available measurement series (n = 98). All random distributions of data were run with a random number generator using the algorithm 'Mersenne twister'. In general, p < 0.05 was assumed to be statistically significant, whereas p < 0.1 was accepted in case of the GEE model. Evaluation of the data was performed by IBM SPSS Statistics 27 (IBM Corp., Armonk, NY, USA).

3.   Results

3.1.   Patients and transpulmonary thermodilution measurements

In total, 38 critically ill patients were enrolled in this study, each of them contributing between one and five TPTD measurement series (2.6 on average). Altogether, 98 TPTD measurement series were performed, which is equivalent to 294 TPTD measurements.

Basic characteristics on patients and TPTD measurements are displayed in Table 1.

Table 1.  Characteristics, clinical data, reason for ICU admission and central venous catheters of the study population.

patients (n = 38)
patients' characteristics		reason for ICU admission, n (%)
sex (male : female, n (%))	22 : 16 (58 % : 42 %)	Sepsis	6 (16 %)
age (years)	64.8 ± 13.6	liver cirrhosis	6 (16 %)
height (cm)	173.8 ± 8.5	acute respiratory distress syndrome, pneumonia	8 (21 %)
weight (kg)	83.3 ± 17.6	cardiogenic shock	3 (8 %)
body mass index (kg/m2)	27.5 ± 5.2	central nervous system disorder	1 (3 %)
body surface area (m2)	1.97 ± 0.22	gastrointestinal (pancreatitis, gastrointestinal bleeding)	7 (18 %)
SOFA score	10.4 ± 3.1	Others	7 (18 %)
APACHE Ⅱ score	23.0 ± 6.3
ICU survival	27 survived, 11 died
measurements (n = 98)
clinical data		central venous catheters, n (%)
heart rate (bpm)	87 ± 16	Shaldon and CVC, in total	95 (97 %)
mean arterial pressure (mmHg)	83 ± 14	Shaldon jugular, CVC femoral	66 (67 %)
mechanical ventilation (n (%))	85 (87 %)	Shaldon femoral, CVC jugular	29 (30 %)
controlled mechanical ventilation (n (%))	34 (35 %)	2 CVCs	3 (3 %)
sinus rhythm (n (%))	83 (85 %)
atrial fibrillation (n (%))	14 (14 %)
pacemaker (n (%))	1 (1 %)
vasopressor therapy (n (%))	54 (55 %)
SOFA sepsis-related organ failure assessment score; APACHE acute physiology and chronic health evaluation; CVC central venous catheter, ICU intensive care unit.

---

 | Show Table

DownLoad: CSV

3.2.   Evaluation of the current correction formula

Bivariate analysis revealed a strong association of GEDVI_jug, GEDVI_fem_uncorr and GEDVI_fem_corr. For the values obtained from the two different injection sites and under different settings, large correlation coefficients were observed, as we compared the first measurement series of each patient among each other (set A) as well as all available measurement series (averaged, set B). Detailed results are depicted in Figure 3.

Figure 3.  Scatter plots illustrating the correlation of GEDVI_jug with GEDVI_fem_uncorr (dark dots) and GEDVI_fem_corr (light grey dots). Database: a) 1st measurement series (set A) and b) all available measurement series of each patient (set B). Set A: correlation of GEDVI_jug with GEDVI_fem_uncorr 0.85^** and with GEDVI_fem_corr 0.76^**; correlation of GEDVI_fem_uncorr with GEDVI_fem_corr 0.82^**. Set B: correlation of GEDVI_jug with GEDVI_fem_uncorr 0.90^** and with GEDVI_fem_corr 0.85^**; correlation of GEDVI_fem_uncorr with GEDVI_fem_corr 0.83^**. ^** p < 0.001; GEDVI_jug global end-diastolic volume index after jugular indicator injection (ml/m²); GEDVI_fem_uncorr global end-diastolic volume index derived from femoral indicator injection without applying the correction function (ml/m²); GEDVI_fem_corr global end-diastolic volume index derived from femoral indicator injection with application of the correction function (ml/m²).

DownLoad: Full-Size Img PowerPoint

Considering both first measurement series (set A) and all available measurements of each patient (set B), the means of GEDVI_jug and GEDVI_fem_uncorr deviated significantly. In contrast, the means of GEDVI_jug and GEDVI_fem_corr did not substantially differ. These results are provided in Table 2.

Table 2.  Comparison of GEDVI derived from different injection sites and PiCCO^® device settings.

	1st measurement series (set A; n = 38)	all available measurement series (set B; n = 38)
	means ± SD	means ± SD
GEDVI_jug (ml/m2)	829 ± 178	833 ± 175
GEDVI_fem_uncorr (ml/m2)	1071 ± 244	1080 ± 229
GEDVI_fem_corr (ml/m2)	805 ± 147	820 ± 144
GEDVI_jug global end-diastolic volume index after jugular indicator injection (ml/m2); GEDVI_fem_uncorr global end-diastolic volume index derived from femoral indicator injection without applying the correction function (ml/m2); GEDVI_fem_corr global end-diastolic volume index derived from femoral indicator injection with application of the correction function (ml/m2)

---

 | Show Table

DownLoad: CSV

Comparison of the gold standard GEDVI_jug with the GEDVI_fem_uncorr yielded a relatively large bias in Bland-Altman analysis. In contrast, applying the integrated correction function (GEDVI_fem_corr) reduced this bias considerably. Exact numbers, including limits of agreement, are to be found in Figure 4.

Figure 4.  Bland-Altman plots demonstrating the agreement between GEDVI_jug vs. GEDVI_fem_uncorr and GEDVI_fem_corr, respectively. Bias is visualized as the middle continuous line. 95% limits of agreement (LoA) are shown as the upper and lower broken lines. Database: 1st measurement series (set A) without (a)) and with (b)) application of the correction function; all available measurement series of each patient (set B) without (c)) and with (d)) application of the correction function. GEDVI_jug global end-diastolic volume index after jugular indicator injection (ml/m²); GEDVI_fem_uncorr global end-diastolic volume index derived from femoral indicator injection without applying the correction function (ml/m²); GEDVI_fem_corr global end-diastolic volume index derived from femoral indicator injection with application of the correction function (ml/m²); LoA limits of agreement.

DownLoad: Full-Size Img PowerPoint

Moreover, applying the correction function resulted in a decrease of the percentage error of initially 29.2% (set A) and 29.7% (set B) to 10.1% (set A) and 8.7% (set B), respectively.

In clinical practice GEDVI is classified as decreased (<680 ml/m²), normal (680–800 ml/m²) or increased (>800 ml/m²). Applying these categories on GEDVI_fem_uncorr, only 56 of 98 measurements (57.1 %) were assigned to the same category as the gold standard measurement, GEDVI_jug. The use of the correction function classified more than 60% of the initially wrong categorizations correctly according to the gold standard GEDVI_jug and led to a significantly better agreement with 70 out of 98 proper classifications (71.4 %, McNemar test p = 0.034).

3.3.   Development of the new correction formula – general overview

Applying a two-step procedure, comprising identification of the most suitable variables (Ⅰ) and consecutive integration into a formula (Ⅱ), led to a revised correction formula.

3.4.   Formula development I – determination of variables

We checked out a broad variety of biometric and other factors (inter alia age, sex, size, actual bodyweight, body mass index (BMI), IBW, cardiac index derived from femoral indicator injection (CI_fem) and body surface area based on actual (BSA) and predicted (BSA_pred) body weight) in different constellations, which might be causally related to the individual configuration of the VCI, that is additionally passed through in case of femoral indicator injection and hence affects MTt and GEDVI.

Finally, GEDVI_fem_uncorr, age and BSA convinced as the pivotal determinants for the new correction formula resting upon our dataset, as this combination reduces the MAE, the RMSE and the RSS most efficiently of all assessed parameters and combinations, respectively.

Pearson correlation coefficient demonstrates the highest agreement with GEDVI_jug when using the aforementioned variables for correction of GEDVI_fem_uncorr in cross validation. With the highest adjusted R², GEDVI_fem_uncorr, age and BSA can explain a larger percentage of variation than any other variable or combination of variables.

Besides, more measurements with femoral indicator injection were assigned to the same category (decreased/normal/increased) as the gold standard, GEDVI_jug, when the above-mentioned variable combination was considered for correcting GEDVI_fem_uncorr.

In all examination criteria, these variables performed better compared to the currently implemented correction function. Table 3 lists a precise display of the final evaluation results.

Table 3.  Model performance.

	uncorrected	corrected	corrected_new
included parameters	none	GEDVI_fem_uncorr CI_fem IBW	GEDVI_fem_uncorr age BSA
MAE (mL /m2)	247	68	61
RMSE (mL /m2)	270	86	77
RSS (mL /m2) 2	821225	90133	71240
correlation coefficient (Pearson)	0.892	0.899	0.908
adjusted R2	-1.57	0.72	0.78
agreement (n (%))	56 (57.1 %)	71 (72.4 %)	73 (74.5 %)
GEDVI_fem_uncorr global end-diastolic volume index derived from femoral indicator injection without applying the correction function (ml/m2); CI_fem cardiac index derived from femoral indicator injection (l/min/m2); BSA body surface area (m2); IBW ideal body weight (kg); MAE mean absolute error; RMSE root mean square error; RSS residual sum of squares.

---

 | Show Table

DownLoad: CSV

3.5.   Formula development Ⅱ – determination of numerical factors

In a second step, a GEE model provided the final correction formula (Eq 3) on the base of all available measurements of our dataset (n = 98) including GEDVI_fem_uncorr (p < 0.001), BSA (p < 0.001) and age (p = 0.074) as covariates:

GEDVI derived from femoral indicator injection and corrected by our new formula is defined as GEDVI_fem_corr_new.

3.6.   Validation of the new correction formula (GEDVI_fem_corr_new)

To evaluate the performance of GEDVI_fem_corr_new (Eq 3) in an independent data set, this newly developed correction formula was applied on the data of the validation group in the study, which proposed the first correction formula (Saugel et al. ^[14]). BSA was calculated based on the mean height (178 cm) and weight (93.6 kg), whereas mean age (57.2 years) was given. The original bias of GEDVI_jug (720 ml/m² ± 76 ml) and GEDVI_fem_uncorr (896 ml/m² ± 126 ml) was 176 ml/m², equivalent to 20% of GEDVI_fem_uncorr. The formula suggested by Saugel et al. reduced the bias to 50 ml/m² or 6%. GEDVI_fem_corr_new performed better than the existing GEDVI_fem_corr as a further reduction of the bias from 6% to 2% (20 ml/m²) of GEDVI_fem_uncorr was achieved by our revised correction formula.

4.   Discussion

4.1.   Necessity of GEDVI correction in femoral indicator injection

In this study, we validate the currently applied correction formula in the PiCCO^® device and point out parameters that can further improve this formula. Even if dynamic preload parameters, like SVV and PPV, are more accurate in the assessment of fluid responsiveness than their static equivalent GEDVI, there are constraints due to multiple mandatory preconditions, such as controlled mechanical ventilation of the patient, a minimal tidal volume of 8ml/kg bodyweight and the absence of cardiac arrhythmias ^[20]. In our study, only 2 % (2/98) of all patients met these requirements, which matches the results of a previously published systematic review ^[20].

GEDVI provides reliable results on preload status regardless of criteria like sinus rhythm and respiratory conditions ^[21]. It is measured after application of a cold saline bolus, which is usually administered into a jugular or subclavian vein, but in up to one third of all patients needs to be injected via femoral vein for various clinical reasons ^[10,11].

In that case, the indicator passes an additional distance through the VCI which consecutively lengthens the MTt and ultimately leads to an overestimated GEDVI. Consequently, we observed that only 57% of the femoral measurements were correctly assigned to the same category (decreased / normal / increased) as the gold standard, GEDVI_jug, which would lead to clinical misperception and hence inadequate, potentially harmful fluid resuscitation in more than two fifth of these critically ill patients.

4.2.   Performance of the current correction function

In the latest PiCCO^® algorithm, an internal correction function, which was developed with a rather small number of TPTD measurement series conducted in few patients, reduces the degree of overestimation ^[14]. In accordance with the findings of a recent validation study, we observed a significant correlation of GEDVI_jug, GEDVI_fem_uncorr and GEDVI_fem_corr in our study ^[17]. Applying the internal correction function of the PiCCO^® device reduces the bias markedly, which allows us to affirm the validity of the correction function in a larger collective.

4.3.   Performance of the new correction formula

Despite of the correlation, the absolute mean of GEDVI_fem_uncorr is, however, far apart from those of GEDVI_fem_corr and gold standard GEDVI_jug. We therefore assumed that this discrepancy can be explained by determined variables associated with the configuration of the VCI. By applying a cross validation procedure we successfully identified three promising covariates and subsequently developed a revised correction formula on the base of the whole dataset, which includes approximately 50 % more patients compared to the first study on this topic (n = 24 vs. n = 38) and about double the number of measurement series (n = 48 vs. n = 98).

The most suitable combination of the investigated parameters, GEDVI_fem_uncorr, age and BSA, resulted in a slight, but consistent improvement including an increased adjusted R², when compared to the cross validation results derived from the variables of the currently integrated correction formula (GEDVI_fem_uncorr, CI_fem and IBW). However, and most important from a clinical perspective, our favoured combination of covariates assigned more measurements to the same category as the gold standard GEDVI_jug. It thus allows the clinician to more precisely decide whether a change of the therapeutical fluid management is indicated.

Individual VCI configuration determines the increase in MTt in case of femoral indicator injection and seems to be associated with age and BSA, which takes the patient's height and actual body weight into account. While VCI volume might also alter ITTV and intrathoracic blood volume (ITBV), subtraction of both volumes diminishes overestimation in the calculation of EVLWI as shown by Saugel et al. ^[14].

Reviewing our new correction formula and comparing it to the current internal correction function on the independent validation dataset of Saugel et al. allowed us to further validate its efficacy ^[14]. Since our correction formula achieved a reduction of bias to 2 % and hereby exceeded the correction function of Saugel et. al. (reduction of bias to only 6 %), we assume an acceptable robustness, satisfying performance and universal applicability ^[14]. A reason for the improved performance might be the larger size of the dataset the revised correction formula was developed on.

4.4.   Outlook

In summary, the new correction formula for GEDVI derived from femoral indicator injection can predict the gold standard GEDVI_jug more precisely than the previously published formula. Nevertheless, a remarkable part of the variability remains unexplained by other variables than the ones used in both correction formulas.

These individual discrepancies are obviously difficult to compensate for. An alternative option to offset the femoral CVC placement could be a technical approach. A catheter, which is inserted in the femoral vein and ends near the right atrium, might be a possible alternative. Known length and diameter of such a catheter and consequently known flow velocity and temperature change of the indicator bolus could provide constant conditions, that are less dependent of circulation and individual patient characteristics. However, this would require additional catheter equipment. As an invasive, long, permanently intravasal catheter system, comparable with the pulmonary artery catheter, it would probably be associated with a higher number and more severe complications, like clot forming and looping. Overall, this might result in a reduced applicability and outweigh the advantages of TPTD and should therefore only be used in selected patients.

4.5.   Limitations

Although the three consecutive TPTD measurements in one series were performed within less than 20 minutes and under steady conditions, there are minor natural fluctuations within an organism inevitably affecting the results and their comparability.

Changes of GEDVI were not investigated over time in this study. While Biais et al. demonstrated that a mini-volume challenge (100 ml) can impact hemodynamics, Aya et al. showed that at least a bolus of 321 ml is required for a significant effect ^[22,23]. In addition, measurement of the specific parameter was performed in random order. Consequently, we do not think, but cannot completely exclude, that the total fluid bolus of up to 135 ml has affected the measurements.

This single centre study was carried out in a medical ICU. The new correction formula was validated retrospectively using the means of only a small dataset, which diminishes its informative value. The findings should thus be confirmed with data from different ICU populations in a prospective multicentric study design.

5.   Conclusions

Femoral indicator injection in TPTD measurements leads to a fundamental overestimation of GEDVI. A previously recommended correction formula approximates GEDVI_fem to the gold standard GEDVI_jug. Our novel correction formula, which is based on a larger data set, further reduces this difference, and therefore increases the predictive power of GEDVI_fem.

Acknowledgments

In remembrance of Prof. Wolfgang Huber who initiated this project but unfortunately deceased before study completion.

Conflict of interest

Tobias Lahmer received travel grants from Gilead, Pfizer and MSD and lecture fees from CytoSorbents. Sebastian Rasch received travel grants from Gilead and lecture fees from CytoSorbents. All other authors declare that they have no conflict of interests.