1. Historical Considerations on Protein Synthesis
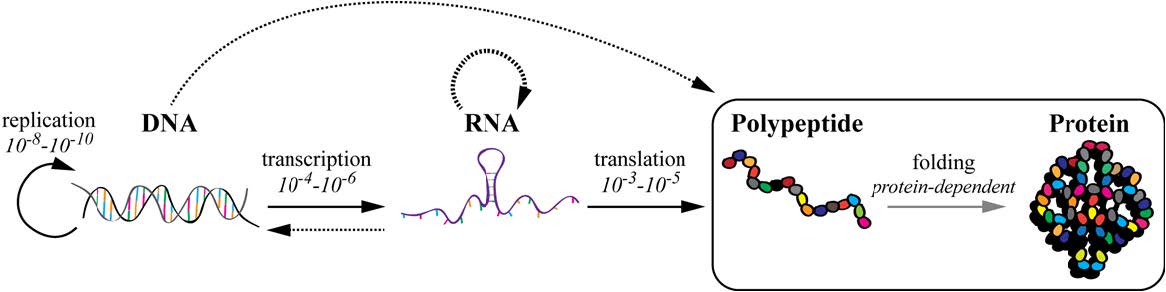
Production of any protein requires that the information for the specific sequence of its amino acids is transcribed from DNA into an mRNA molecule. The mRNA will then be translated by the complex machinery of the ribosome and its co-factors into a polypeptide chain that must be folded, with assistance of chaperones and folding enzymes, in its unique architecture. First thoughts on the flow of genetic information were enounced by Francis Crick in 1956, when he exposed his “Ideas on protein synthesis” [1]. These were later published and partially revisited in a Nature article in 1970 defining what he pompously (and inappropriately, as himself later admitted) defined as “Central dogma of molecular biology” [2] (Figure 1).
Although the (mis) use of the word “dogma” generated some misunderstanding with colleagues, the principles he wanted to highlight were rather simple: once the information, intended as the sequence of amino acids in a protein is transferred from DNA into proteins, it cannot go back. In 1970, Crick proposed a scenario where the flow of information would generally occur from DNA to DNA, DNA to RNA and from RNA to protein (what he called “general transfer”). RNA replication and flow of information from RNA to DNA and from DNA directly to protein were defined as “special transfers” meaning that they could happen under particular conditions. “Unknown transfers” were the ones from protein to protein as well as the reverse flow of information from protein to RNA or DNA. At the time, there were no experimental evidences for the latter and thus Crick hypothesized that these transfers were improbable to exist [2].
As science moved on, the concepts highlighted in Crick’s “central dogma” have dramatically changed and experimental evidences have proven the existence of “unknown transfers” such as reverse transcription (RNA to DNA) by Temin and Baltimore [3,4] and the description of the prion-mediated inheritance [5].
In any case, and independently of what Crick meant to say at the time he posted the “Ideas on protein synthesis” [1] and later on [2], it remains that translation and attainment of the correct ternary and quaternary structure of polypeptides are crucial steps in the flow of genetic information. Proteins are the active molecules that carry out almost every function in a living organism, while DNA stores the information to produce each one of them.
2. The Ribosomal Machinery: from Nucleotides to Amino Acids
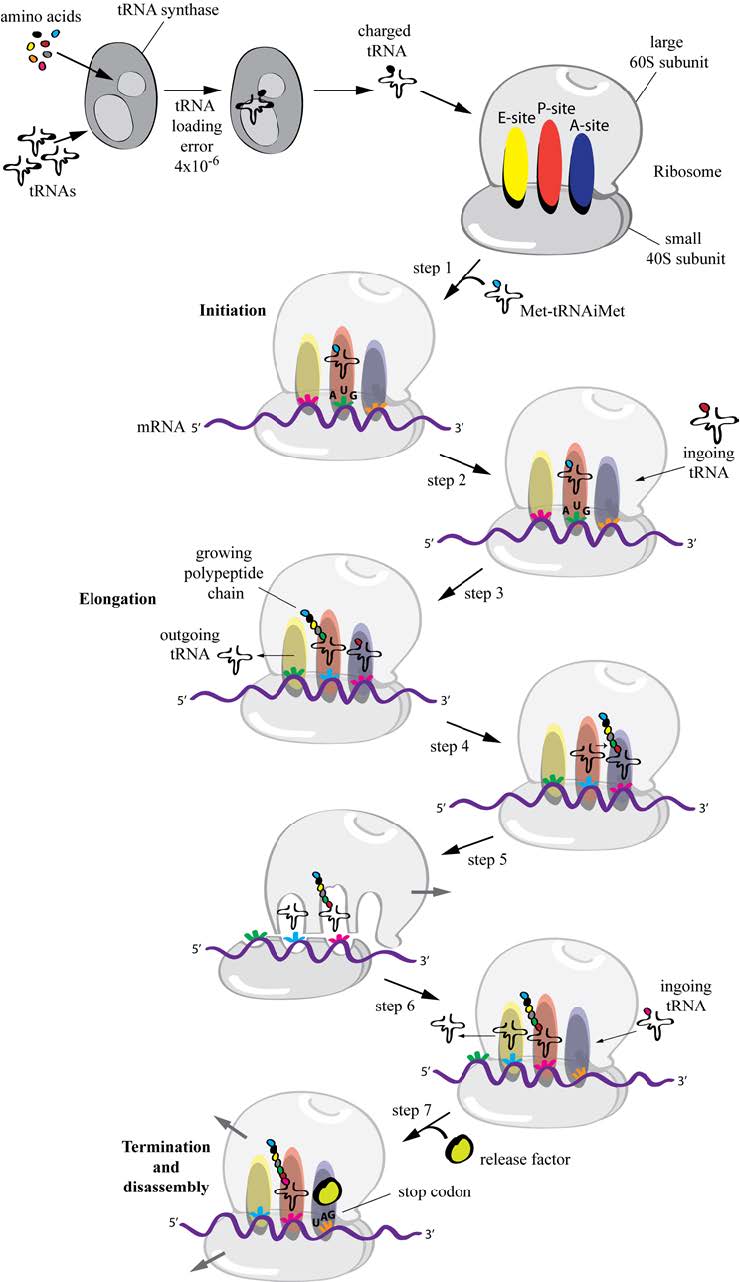
Translation of genetic information (sequences of nucleotides) into a polypeptide chain (sequence of amino acids) is mediated by ribosomes. These are complex structures that act as RNA-based enzymes (ribozymes) to catalyze peptide bond formation. Eukaryotic 80S ribosomes are large (4.3 MDa for the human ribosome) and complex structures composed of a large 60S subunit, which contains 47 proteins and 3 ribosomal (r)RNAs, and a small 40S subunit containing 33 proteins and 1 rRNA molecule [6,7].
Translation can be divided in 3 steps: Initiation, elongation and termination (Figure 2).
i) Initiation: Amino acids reach the ribosome conjugated with a specific transfer (t)RNA molecule (amino-acyl-tRNAs) that possesses a complementary anticodon for the codon encoding the specific amino acid. In eukaryotes, at least eleven different initiation factors are required to properly initiate translation. These ensure that the methionyl-initiator tRNA (Met-tRNAiMet) is brought in the P site of the ribosomal complex to the initiator AUG codon of an engaged mRNA [8] (Figure 2, step 1).
ii) Elongation: Successive amino-acyl-tRNAs are recruited to the ribosome on the A-site (Figure 2, step 2). While the mRNA molecule is pulled through the ribosome, codon-matching amino-acyl-tRNAs are recruited one after the other in order to link the amino acids into a peptide chain. During elongation the tRNA is recruited to the A-site while an adjacent tRNA in the P-site is covalently bound to the growing amino acid chain (Figure 2, step 3).
At this point a peptidyl transferase contained in the large ribosomal subunit breaks the high energy bond between the tRNA in the P-site and its amino acid, and links it to the free amino acid group on the tRNA in the A-site (Figure 2, step 4). This reaction causes a conformational shift of the large ribosomal subunit relative to the mRNA (which is bound by the small ribosomal subunit) leading to the shift of the bound tRNAs from the P-to the E-site and from the A-to the P-site (Figure 2, step 5). Next, the small ribosomal subunit undergoes a conformational change and moves, together with the mRNA, exactly 3 nucleotides. This resets the ribosomal structure leaving an empty A-site that can now accommodate the next complementary amino-acyl-tRNA, which binding will lead to the release of the “empty” tRNA from the E-site (Figure 2, step 6) [9].
iii) Termination: Translation gets terminated when a stop codon (UAA, UAG or UGA) is encountered by the A-site of the ribosome. These codons stop translation by engaging so called release factors that bind the A-site and force the peptidyl-transferase to catalyze the addition of a water molecule to the C-terminal peptidyl-tRNA thereby releasing the carboxyl terminus from its tRNA and leading to the disassembly of the large and small subunits of the ribosome (Figure 2, step 7) [10].
Translation is a rapid process. The catalytic activity of the ribozyme elongates the polypeptide chain at a rate of 3-10 amino acids per second in eukaryotes [11,12] and up to 10-20 amino acids per second in E. coli [13,14,15].
Every step in the biosynthetic process of proteins from DNA replication to protein folding (including transcription, amino-acyl-tRNA synthesis and translation) is error prone. Following chapters will focus on the fidelity of the mentioned processes and on quality control and repair mechanisms that operate in order to minimize the insertion of errors in the flow of genetic information from DNA to the mature protein. We will also discuss how cells maintain proteostasis i.e., how they ensure production of the proteome in correct amount and appropriate quality.
3. Fidelity of Genetic Information Flow: Error Prone Steps and Dedicated Quality Controls
3.1. Replication: from DNA to DNA
DNA replication is performed with very high fidelity since the correct maintenance of the genetic material is fundamental for the survival not only of organisms but, most importantly, for species. DNA polymerases (the enzymes that catalyze the synthesis of new DNA strands) and their co-factors have evolved in order to minimize the insertion of wrong nucleotides. In fact, DNA replication machineries are estimated to insert a mismatched base every 104-105 nucleotides, but through the combined actions of proofreading and post-replication mismatch repair mechanisms, eukaryotic cells can replicate their genome with remarkable fidelity of less than 1 mutation per genome per cell division (error rate of 10-8-10-10) [16,17,18,19,20] (Figure 1). Although DNA replication error rate is exquisitely low, nucleotide mis-incorporation is fundamental for adaptation to changing environmental conditions and for driving evolution [20].
3.2. Transcription: from DNA to mRNA
mRNA is the principal template molecule used for protein synthesis. Genes encoded in genomic DNA are transcribed, in the nucleus, by multi-component transcription machineries, which comprise RNA polymerases (RNA pol) as principal component, into a “transient” single stranded nucleotides chain (mRNA). This is transported into the cytosol where it can be engaged by the ribosomes (for reviews see [21,22,23]). The mRNA molecule has a relatively short half-life (around 9 h) and is re-synthesized constantly (typically 17 copies/gene are present) [24]. The continuous renewal of the mRNA pool implies that errors during transcription have less severe consequences than errors occurring during DNA replication and also fidelity of the process is less stringent (about 1 wrong nucleotide every 104-106 bases both in vitro and in vivo)[25,26,27] (Figure 1).
3.3. Translation: from mRNA to proteins
When it comes to translation of mRNA polynucleotides into amino acid chains, the error rate increases. Generally, the error rate for translation is one order of magnitude higher than that for transcription, laying between a wrong amino acid every 103-105 codons [28,29,30,31,32] (Figure 1). An evolutionary interpretation for the lower error rate in transcription than in translation is that an error in transcription would lead to many erroneous protein copies (on average 17 mRNA/gene code for 50, 000 proteins/gene [24], meaning ca. 2950 proteins/mRNA), whereas an error in translation affects only one polypeptide chain if the error is due to stochastic mis-incorporation (and not a defective machinery). Note that in addition to the mistranslation of mRNAs, the protein synthesis efficiency can also be hampered by the incorrect charging of the tRNAs themselves. Incorporation of the wrong amino acid on a given tRNA has been measured to occur with error rates of 10-4-10-5 [28] (Figure 2).
3.4. Folding: from a sequence of amino acids to an active protein
Protein biogenesis is not terminated upon translation. Most proteins must achieve a specific three dimensional structure, which is determined by its amino acid sequence. The final, or native, conformation of a polypeptide chain is generally the one that minimizes its free energy and can occur naturally in a test tube [33]. In the living cell, however, the polypeptide chain emerging from ribosomes has to undergo an active, chaperone-assisted process of folding (see 4.2), and in some cases of oligomerization, i.e., of pairing with other polypeptide chains, in order to achieve its functional conformation (tertiary and quaternary structures). Only then, proteins can be transported to their final destination. As one can imagine, the process of folding is all but error-free and needs strict quality assessment in order to permit the synthesis of a functional proteome.
The efficiency of polypeptide folding, as well as the rate, varies substantially for different gene products. Intuitively, short, single domain proteins achieve their native structure rapidly and efficiently, whereas long, multidomain and multi-subunit complexes have folding pathways that can be aborted at any time. Moreover, efficiency and rate of folding are substantially affected or even fully blocked by mutations in the polypeptide chain or by perturbation of the environmental and homeostatic conditions of the folding environment (e.g., the ER) making it very difficult to make trustable predictions (Figure 1). As such, although it is generally thought that the folding process is efficient for most proteins [34,35] there are controversial results/opinions. For example a study reported that ca. 30% of the newly synthesized proteome was rapidly degraded by the proteasome as a possible indication of low efficiency [36], whereas another reported diametrically different conclusions [37].
3.5. Targeting: the right protein in the right place
In order to perform their functions, proteins and protein complexes must be transported to the right intra-or extra-cellular place. Protein targeting is as important as any other step in protein synthesis and as such, defect in protein localization have been linked to several medical conditions or diseases [38]. Approximately half of the synthesized cellular proteins need to be transported across or into a membrane [39] and regulation of most trafficking processes is based on specific signal sequences displayed by the polypeptide chains [40]. Protein transport within cells is carried out by both bulk (e.g., unselective ER export via COPII vesicles) and specific, receptor based mechanisms that enrich select cargos into COPII vesicles (e.g., ERGIC53-based export of secretory glycoproteins from the ER) [41,42]. Protein translocation across organelle membranes might happen post-translationally, as for mitochondrial, peroxisomal, secretory and nuclear proteins, or co-translationally, as in the case of proteins of the secretory pathway and integral membrane proteins, which are synthesized directly into the ER. Protein trafficking into organelles involves specific mechanisms for each compartment. As a general rule, proteins carry a specific address tag for the organelle they are sent to. This tag is recognized by a receptor (bound on the organelle membrane or soluble), which engages the necessary import machinery. Protein import is energy driven and occurs through membrane-spanning channels (e.g., the TOM and TIM complexes of the outer and inner mitochondrial membrane [43] or the Sec61/Sec62/Sec63 complex of the ER membrane [44]). In the case of the nuclear envelope, small proteins up to 40 kDa can also traffic by simple diffusion [45]. The signal sequence may, or may not be removed upon translocation by signal peptidases. Studies on the fidelity of the cellular trafficking processes have not been performed, but several factors have been shown to be important and their loss significantly affects transport efficacy and precision and ultimately leads to disease [38]. As an example, two distinct mutations in the ERGIC-53 gene were shown to underlie the autosomal recessive bleeding disorder caused by combined factor V and factor VIII deficiency. Homozygous patients completely lack the expression of coagulation factors V and VIII due to their impaired export from the ER via COPII vesicles [46,47].
4. Dedicated Quality Control Mechanisms Monitor the Biosynthesis of Proteins
Protein folding occurs in cellular compartments with different chimico-physical properties (i.e., cytosol, mitochondria, ER), which contain compartment-specific chaperones and enzymes that maximize protein folding efficiency. Cells have developed dedicated mechanisms that screen the quality of the proteome during its synthesis and intervene when a protein is not able to reach its native conformation by promoting degradation of the erroneous product. Degradation of cellular proteins generally occurs in the cytosol and nucleus via the ubiquitin proteasome system (UPS) [48,49,50,51,52] and additionally by lysosomal pathways upon engulfment of the cytosolic proteins or organelles by the process of autophagy [51,52,53]. Following chapters will describe quality control and degradation machineries that act during the proteins synthesis process, from translation to protein folding and secretion.
4.1. Ribosome quality control complex (RQC)
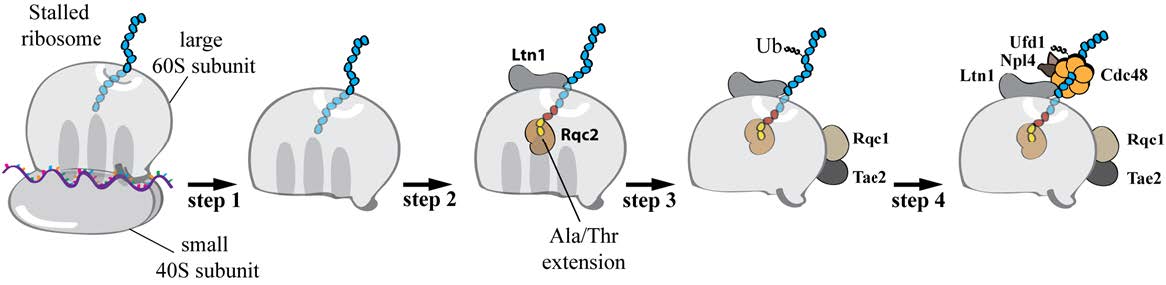
The quality of newly synthesized proteins is constantly monitored from the moment they start emerging from the ribosome up to their degradation [54]. The earliest defective ribosomal products (DRiPs) can be selected for degradation when they are still emerging from the ribosome. This mechanism of co-translational degradation takes place when the translation machinery is defective or engages faulty mRNAs, which leads to ribosomal/translational stalling and the formation of incomplete nascent chains (Figure 3).
Recent work in yeast showed that stalled 80S ribosomes carrying unfinished polypeptide chains are engaged by the ribosome quality control complex (RQC) which mediates ubiquitination and degradation of the incomplete nascent chain [55,56,57,58]. Molecular components of the RQC are the E3 ubiquitin ligase Listerin (Ltn1), Tae2, Rqc1, Rqc2 and Cdc48 with its cofactors Ufd1 and Npl14 [55,59]. Importantly, a recent study described a novel mechanism by which the RQC, in association with the 60S ribosomal subunit, adds polypeptide stretches to the stalled nascent chain and eventually ubiquitinates and targets the polypeptide for degradation via the proteasome [59] (Figure 3). In particular, ribosome stalling leads to the dissociation of the 60S and 40S subunits (Figure 3, step 1), followed by association of Ltn1 and Rqc2 with the peptidyl-tRNA-60S species (Figure 3, step 2). Here Rqc2 through binding of Alanyl-and Threonyl-tRNA orchestrates the, nota bene mRNA free and 40S free, incorporation of carboxy-terminal Ala and Thr extension (termed CAT tails) into the incomplete protein, followed by subsequent ubiquitination by Ltn1 (Figure 3, step 3) and extraction through the Cdc48 complex (Figure 3, step 4).
4.2. A select example: protein folding and quality control in the ER
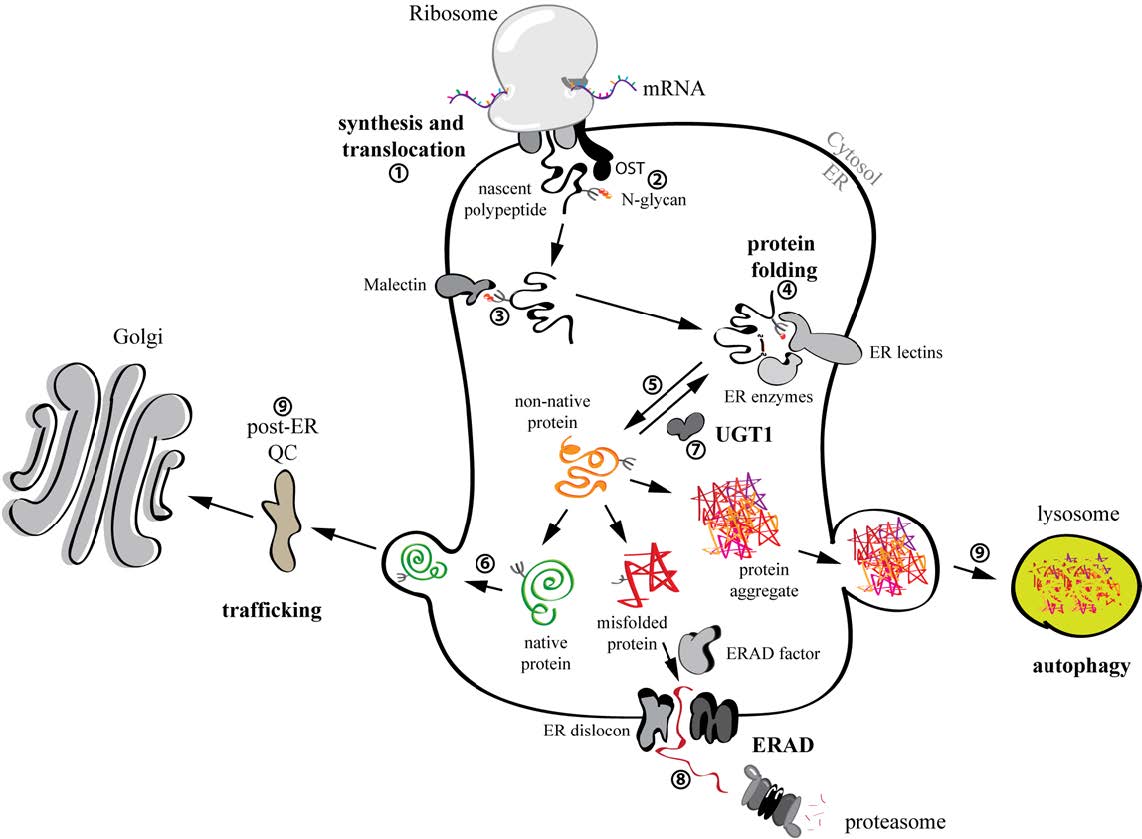
The ER plays a crucial role in the maintenance of a quantitatively and qualitatively correct proteome. In fact, it is the organelle where the synthesis and folding of most membrane bound and secreted proteins (which make up ca. 1/3 of a cell’s proteome [60]) occurs (Figure 4). Most proteins that are synthesized into the ER (Figure 4, step 1) may be subjected to the addition of a complex glycan, composed of three residues of glucose, nine mannoses and two N-acetylglucosamines (Glc3Man9GlcNAc2) on side chains of asparagine residues in the sequon Asn-Xxx-Ser/Thr/Cys (Xxx all but Pro or Asp). N-glycosylation is catalyzed by a multiprotein complex oligosaccharyltransferase (OST) [61,62] (Figure 4, step 2). The attached sugar increases solubility of nascent and folding polypeptides since it shields hydrophobic regions. Moreover, it engages folding, quality control and degradation factors to determine the fate of the folding protein [62,63,64]. For non-glycosylated proteins the processes that regulate folding and degradation are far less known and thus not mentioned in the following chapters.
4.2.1 N-glycan dependent protein folding and quality control
Early upon addition of the glycan, the outermost glucose is trimmed by the ER enzyme α-glucosidase I. The di-glucosylated protein-bound oligosaccharide engages Malectin [65] (Figure 4, step 3) a type I membrane-bound ER lectin, which was shown to preferentially associate with misfolded proteins [66]. Malectin operates the first quality control checkpoint for sequestration and delivery of misfolded proteins for ER-associated degradation (ERAD, see below). Elimination of the second glucose by α-glucosidase II leads to association of the maturing protein with the lectins Calnexin (CNX) and Calreticulin (CRT), which in turn engage enzymes necessary for proper folding of the bound polypeptide: protein disulfide isomerases (PDIs) like PDI, ERp57, ERp44, TMX1[67] and others, and peptidyl-prolyl isomerases (PPIs) like cyclophilin B (CYPB) (for a review see [62]) (Figure 4, step 4). Trimming of the third glucose by α-glucosidase II releases the substrate from CNX/CRT (Figure 4, step 5). This is a crucial step in protein folding in the ER. In fact, if a protein collapses to its proper conformation after release from CNX/CRT, it will be transported through the Golgi apparatus to reach its final intra-or extra-cellular destination [41,62] (Figure 4, step 6). On the contrary, if a protein is still misfolded after release from Cnx/Crt there are two options: (1) the N-glycans are re-glucosylated by the glucosyltransferase UGT1 and the polypeptide enters another round of folding in association with CNX/CRT (hence CNX/CRT cycle, [62,64,68]) (Figure 4, step 7), or (2) the N-glycans get further trimmed by ER mannosidases such as ER α1, 2-mannosidase I [69], EDEM1 [70], EDEM2 [71] and/or EDEM3 [72,73]. Removal of the mannoses impedes the re-glucosylation by UGT1 [70] and enables the recruitment of OS-9 and XTP3B [74,75], two lectins that deliver misfolded proteins to retro-translocation channels (dislocons) built around E3 ubiquitin ligases such as HRD1 and GP78 embedded in the ER membrane [76] (Figure 4, step 8). Proteins that escape retention-based quality control in the ER might be retained in post-ER compartments. This relies on the intervention of UGT1 and p97 as has recently been shown for proteins displaying ionizable residues in the intramembrane domain [77] (Figure 4, step 9).
4.2.2 ER-associated degradation (ERAD)
Since protein folding is liable to error and its efficiency differs from protein to protein, misfolded products constantly form in the ER. These must be rapidly eliminated to avoid the formation of toxic aggregates and disturbances of ER homeostasis. Misfolded proteins generally undergo selection for degradation via de-mannosylation of their N-glycans by EDEM proteins and associate with the ER lectins OS-9 and XTP3B [78]. As mentioned above, these two ERAD lectins act as shuttles that deliver the misfolded polypeptide to dislocons. Once there, misfolded proteins are retrotranslocated into the cytosol, poly-ubiquitinated by E3 ligases, extracted from the membrane by the AAA-ATPase p97 and its co-factors, and eventually targeted to the 26S proteasome for extensive proteolysis [78,79] (Figure 4, step 8). ERAD activity must be carefully regulated to prevent excessive or inefficient degradation, which can in turn result in the onset of loss-or gain-of-function disorders, respectively. Indeed, the turnover and the intracellular level of some ERAD factors such as EDEM1, OS-9 and SEL1L, is controlled in a post-translational manner through what has been defined as ERAD tuning [79,80,81].
4.3. The role of autophagy in degradation of misfolded proteins
As mentioned above, degradation of misfolded translational products not only happens through the UPS, but also via autophagy. Autophagy is a cellular degradative pathway that removes proteins, aggregates and even entire organelles, by engulfing them into double-membrane vesicles (the autophagosomes) that then fuse with lysosomes containing hydrolases (Figure 4, step 9). There are several types of autophagy (e.g., macroautophagy, microautophagy and chaperone mediated autophagy (CMA), for a review see [82]). They are mediated by the autophagy-related genes (ATG) and their associated enzymes [83,84,85]. Autophagy was first studied as a response to starvation, but several lines of research in the last decades made it clear that autophagy exists in many flavors and plays crucial roles in cell and organelle homeostasis [86] and, as such, is involved in human health and disease [87].
In line with this, the degradation of misfolded proteins through macroautophagy or both UPS and macroautophagy has been reported. Examples of disease-related proteins degraded via the autophagic machinery are mutant Huntingtin (HTT) protein (Huntington’s disease) [88], α-synuclein (Parkinson’s disease) [89], Aβ and tau (Alzheimer’s disease) [90,91]. Other well-characterized autophagy substrate is the Z-variant of α-1-antitrypsin (ATZ) [92] and cystic-fibrosis conductance regulator (CFTR) aggregates [93].
Although autophagic degradation of cytosolic components might happen stochastically as a bulk phenomenon, several types of highly selective autophagy have recently been described. Autophagy receptors are essential for such targeted degradation, which allow the recognition of the specific cargo and its coupling to the autophagic machinery [94]. Specific degradation of parts of the ER (and its content) have been shown to be crucial both during homeostasis [95] and recovery from an unfolded protein response (UPR, see below), during which excess ER chaperones and folding factors are degraded via the lysosome in a Sec62 dependent manner [96].
5. Keeping Protein Biosynthesis in Balance
The maintenance of proteostasis is of fundamental importance for the integrity of cells and organisms. As described above, cells are equipped with quality control and clearance mechanisms that ensure removal of misfolded proteins. These machineries that work efficiently under homeostatic conditions might need to be adjusted to fluctuations in cargo load in order to permit cells to adapt to, and tolerate stressful stimuli. Mutated or defective gene products might lead to loss-of-function or gain-of-toxic-function phenotypes. Fluctuations in protein synthesis due to environmental conditions or pathogen attacks can create conditions of overload of the quality control systems. Pathogens may subvert cellular proteostasis by hijacking the protein synthesis and quality control machineries for the purpose of their reproduction and immune escape [78,97,98]. In addition to quality control and degradation system for monitoring the quality of their proteome, cells have developed responses to various stresses in order to preserve and restore protein homeostasis. If maintenance or recovery of homeostatic conditions is not possible (i.e., in case of pathologies) and cellular viability is threatened, modulation of protein synthesis, protein folding, quality control and/or stress responses via chemical and biological compounds might be an efficient strategy to counteract these diseases.
5.1. The unfolded protein response: modulation of protein synthesis and quality control
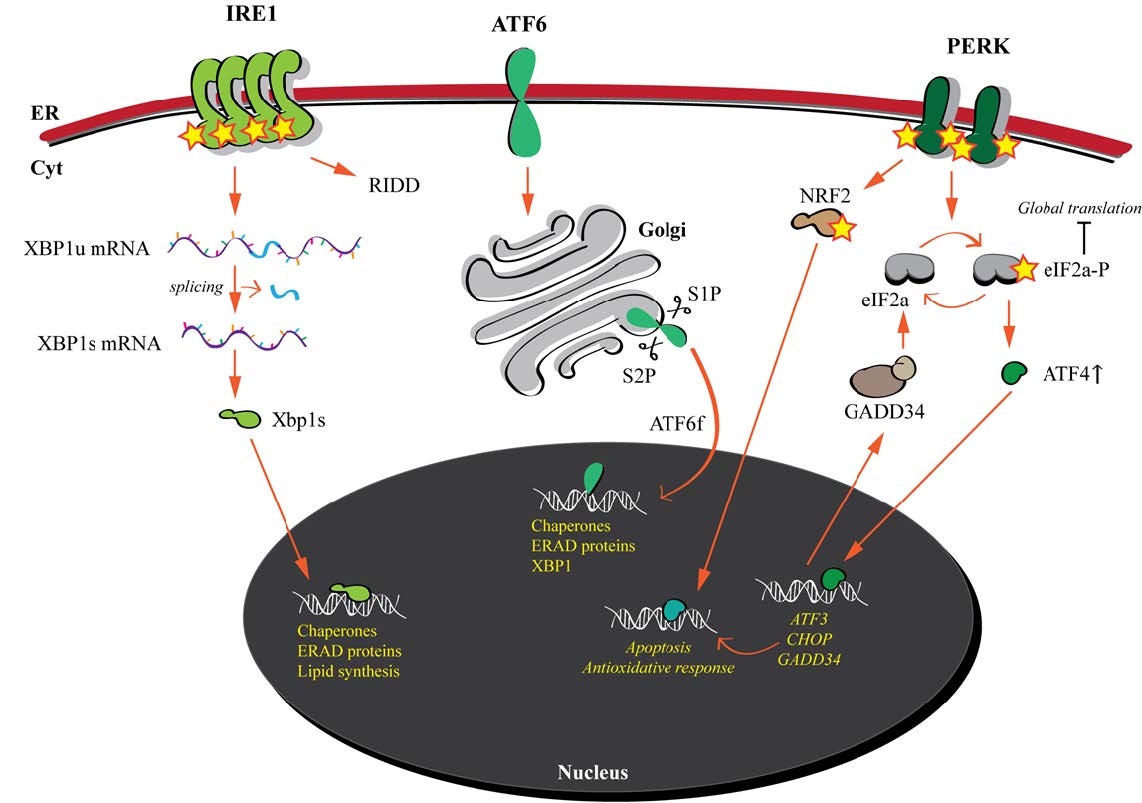
A striking example of cellular stress response is the unfolded protein response (UPR) elicited by the accumulation of misfolded proteins in the ER (Figure 5). Since the flux of nascent chains into the ER is dynamic and might change due to fluctuating environmental conditions, genetic mutations and attack by pathogens, a cell has to tune the level of its folding, quality control and ERAD components in order to preserve the production of membrane and secreted cargo proteins. Such homeostatic control is achieved by the constant monitoring of “ER load” by ER membrane embedded stress sensors, namely inositol-requiring-protein-1 (IRE1), activating transcription factor-6 (ATF6) and protein kinase RNA (PKR)-like ER kinase (PERK). These sensors are activated upon accumulation of misfolded proteins in the ER and subsequently orchestrate a signaling cascade from the ER to the nucleus [99] (Figure 5).
5.1.1 IRE1 and ATF6: modulation of protein folding and ERAD capacity
5.1.1.1. IRE1
The IRE1 branch of the UPR is the most conserved (found in yeast, plants and metazoans [100,101]). IRE1 is a type 1 protein with a lumenal N-terminal domain and a cytosolic C-terminal region that possesses kinase and RNase activities. Mammalian cells possess two homologs, namely IRE1α and IRE1β characterized by tissue-specific distribution. IRE1α is activated upon accumulation of misfolded proteins, which leads to its oligomerization, trans-autophosphorylation with consequent activation of its RNase domain. IRE1α signaling occurs via the cleavage of its principal substrate, XBP1 (X-box binding protein-1) mRNA (Figure 5). An unconventional splicing event leads to the excision of an intron and subsequent ligation leads to the formation of the XBP1s mRNA that translates into a potent bZIP transcription factor that upregulates transcription of ER quality control components and enzymes for lipid synthesis, which controls ER membrane expansion [102] (Figure 5). In addition to XBP1 splicing, IRE1 is responsible for the cleavage of a specific subset of mRNAs, mechanism termed Regulated IRE1-Dependent Decay (RIDD), leading to the reduction of ER cargo protein synthesis [103] (Figure 5).
5.1.1.2. ATF6
ATF6 is a metazoan specific stress sensor with a type II topology consisting of a luminal carboxyl-terminal stress sensing domain and a cytoplasmic amino-terminal bZIP transcription factor domain. Upon ER stress, ATF6 is transported to the Golgi apparatus, where it is sequentially cleaved by site 1 and site 2 proteases (S1P and S2P) leading to the release of the bZIP domain (ATF6f), which translocates to the nucleus and enhances transcription of ER quality control and ERAD components [102,104] (Figure 5). Interestingly, XBP1s and ATF6 upregulate UPR target genes with similar functions (e.g., chaperones and PDIs) and even share some of their targets, which upregulation mainly aims at enhancing folding and degradation capacity of the ER [102,105]. Additionally XBP1 is also a target gene of the ATF6 pathway, thus tightly linking the IRE1 and the ATF6 branches of the UPR.
5.1.2 PERK
The third branch of the UPR differs in its outcome compared to the IRE1 and ATF6 pathways. PERK is a type I membrane protein with a luminal stress sensing domain, similar to the one of IRE1 [106], and a cytosolic kinase domain. Upon activation by ER stress, PERK oligomerizes and phosphorylates itself and other targets. Importantly, PERK phosphorylates the α-subunit of eukaryotic initiation factor 2 (eIF2α) at Ser51, which results in a diminished global protein synthesis, thus reducing burden of ER cargo proteins [107]. Phosphorylation of eIF2α specifically upregulates the translation of activating transcription factor 4 (ATF4) from an open reading frame in its 5’ untranslated region [108,109]. Important among ATF4 target genes are activating transcription factor 3 (ATF3), transcription factor C/EBP homologous protein (CHOP) and DNA damage-inducible 34 (GADD34). ATF3 targets both CHOP and GADD34 [110] (Figure 5). CHOP is a transcription factor responsible for the upregulated transcription of several apoptotic genes, while GADD34, induced late during ER stress, encodes a phosphatase, which counteracts eIF2α phosphorylation (reducing translational inhibition). Thus, the PERK pathway plays a dual role during UPR: induction of apoptosis if the ER stress cannot be resolved and recovery of protein synthesis if eIF2α phosphorylation is reduced via both reduction of ER stress (decreased PERK activation) and GADD34. Additionally, PERK phosphorylates NRF2 which induces genes responsible for antioxidant responses [111] (Figure 5)
6. Pathological Conditions and Therapeutic Options
Several cellular machineries ensure the synthesis of the proper amount and quality of the proteome. Moreover, quality control mechanisms operate at every stage of protein biogenesis in order to maintain proteostasis and to respond to perturbations of various origins by inducing stress responses.
6.1. Conformational diseases and chemical/pharmacological chaperones
Mutations in the genome can give rise to ribosomal products that can elude the quality control systems. Amino acid substitutions in a newly synthesized polypeptide might destabilize the entire protein’s structure [112] causing protein aggregation and deposition (gain-of-toxic-function), or resulting in rapid degradation of the aberrant product (loss-of-function) [113,114,115]. Such pathological conditions are referred to as conformational diseases [114,116]. The aggregation or misfolding of a single species of proteins can affect maturation of other cellular proteins that follow or engage similar pathways. This means that defects in a single protein might spread to several other protein species and induce their misfolding and aggregation, thus harnessing the entire proteostasis network [117,118]. If activation of stress response pathways cannot re-establish proteostasis, consequences will be deleterious for single cells, organs and the entire organism.
Therapeutic approaches are available to restore the folding of proteins or to inhibit their aggregation by delivering molecules that facilitate folding of mutant proteins, stabilize their structure or enhance their solubility. Chemical chaperones are membrane permeable molecules that may non-selectively rescue the folding and functionality of mutant misfolded proteins [114]. Pharmacological chaperones, which have the same biological function, specifically bind only to certain proteins. The specificity makes pharmacological chaperones very suitable for clinical use [119,120,121].
6.2. Modulation of the UPR as treatment option for several diseases
The importance of the UPR is underlined by its implication in many diseases. Cancer cells undergo somatic mutations that are not detected by their DNA damage machineries, which enable them to ignore growth control and disable apoptotic signaling. Especially solid-tumor cells, which are poorly vascularized, might also encounter other type of stresses such as hypoxia, nutrient deprivation and pH changes [122]. These conditions can alter ER homeostasis and interfere with the physiological protein folding environment leading to the activation of the UPR. Activation of the IRE1/XBP1s and the ATF6 branches has been shown in several cancer types such as breast tumors [123], hepatocellular carcinomas [124], gastric tumors [125] and several cancer cell lines [126]. ER chaperone levels were shown to correlate with tumor aggressiveness and alterations in reaction to chemical agents. It is not clear if apoptotic signaling induced by the UPR is escaped due to specific activation of the IRE1 and ATF6 branches or to inactivation of the apoptotic program. In any case, cytoprotective branches of the UPR enable cancer cells to produce and fold large numbers of the proteins that they produce. Thus inhibition of the UPR might be a promising way to treat some types of cancer [127].
In contrast, other conditions are linked to an insufficient activation of the UPR, meaning that induction of the UPR, especially the IRE1 and/or ATF6 branches, could increase ER protein folding and export capacity decreasing aberrant ERAD of unstable, mutant proteins, thus ameliorating loss-of-function diseases such as cystic fibrosis or lysosomal storage diseases [128,129,130]. Similarly, increasing ERAD activity could attenuate the secretion of destabilized, aggregation-prone proteins as in the case of human amyloid diseases [113,131,132,133]. UPR signaling pathways are thereby promising targets for pharmacological intervention in a heterogeneous variety of human diseases. Small molecules, which selectively activate or inhibit only one branch of the UPR have been described and successfully used to influence pathological states [134,135,136,137,138]. Most recently, ATF6 specific modulators were described. Ceapins selectively inhibit the ATF6 pathway by blocking ATF6 delivery (and cleavage) to the Golgi apparatus [139,140]. Moreover, several ATF6 activators that enable the reduction of extracellular protein aggregates were described [141]. The palette of available chemical compounds offers the possibility to specifically modulate one or more branches of the UPR (at least in cultured cells) paving the road for the development of new therapeutic options for a variety of medical conditions including cancer and conformational diseases.
7. Perspectives
Maintenance of proteostasis is of fundamental importance for cellular viability. Through the combined action of a highly interconnected network of signaling pathways, which modulate gene expression, translation, protein folding, trafficking and degradation, cells tightly control the abundance and quality of the produced proteome and adapt it to stressful conditions. Nevertheless, pathological conditions, such as protein conformational diseases, may arise due to defective protein folding and transport.
The social and medical impact of protein misfolding diseases (most often rare genetic conditions that affect children) highlight the importance of understanding basic molecular mechanisms of cellular functions. Common pathways can be engaged by gene products at the basis of completely different pathological phenotypes (e.g. cancer vs lysosomal storage diseases). It will therefore be crucial in future to continue working on mechanisms involved in protein folding, ERAD and UPR in order to describe new targets for pharmaceutical intervention. Several open questions remain to be answered: What are the chimico-physical features of a protein that determine the engagement of specific folding or clearance machineries? How important are certain mechanisms in different cell types or tissues and how differently are they regulated? Do cells experiencing stresses communicate with and influence surrounding cells/tissues? Which are the components involved in the recovery from stresses? Do cells remember experiencing stressful conditions in order to adapt to future insults (epigenetics)? And of course how can these pathways be targeted in order to restore proteostasis?
To the latter question the recently developed clustered regularly interspaced short palindromic repeats (CRISPR)-Cas9 (CRISPR associated protein 9) gene editing technology seems very promising to correct gene defects [142]. Gene-editing as a therapeutic option has the advantage of eliminating the problem at its source, but what if the intervention causes unpredictable side effects? In contrast to pharmacologic interventions, whose use can be promptly interrupted in case of unwanted side effects, irreversibility is the major drawback in “genetic surgery”. Thus, basic research aimed at identify new possible targets regulating processes involved in protein biogenesis (in its larger meaning) remains of fundamental importance.
Acknowledgements
M. M. is supported by Signora Alessandra, the Foundation for Research on Neurodegenerative Diseases, the Swiss National Science Foundation (SNF), the Sinergia program of the SNF and the Comel, Gabriele and Gelu Foundations.
Conflict of Interest
The authors declare no conflict of interest.









 DownLoad:
DownLoad: