Degree based models of granular computing under fuzzy indiscernibility relations

Muhammad Akram; Ahmad N. Al-Kenani; Anam Luqman; Muhammad Akram; Ahmad N. Al-Kenani; Anam Luqman

doi:10.3934/mbe.2021417

Mathematical Biosciences and Engineering

2021, Volume 18, Issue 6: 8415-8443. doi: 10.3934/mbe.2021417

Previous Article Next Article

Research article Special Issues

Degree based models of granular computing under fuzzy indiscernibility relations

1.
Department of Mathematics, University of the Punjab, New Campus, Lahore, Pakistan
2.
Department of Mathematics, Faculty of Science, King Abdulaziz University, P.O. Box 80219, Jeddah 21589, Saudi Arabia

Academic Editor: Antonello Rizzi

Received: 29 July 2021 Accepted: 23 September 2021 Published: 27 September 2021

The aim of this research work is to put forward fuzzy models of granular computing based on fuzzy relation and fuzzy indiscernibility relation. Thanks to fuzzy information granulation to provide multi-level visualization of problems that include uncertain information. In such a granulation, fuzzy sets and fuzzy graphs help us to represent relationships among granules, groups or clusters. We consider the fuzzy indiscernibility relation of a fuzzy knowledge representation system ( $\mathcal{I}$ ). We describe the granular structures of $\mathcal{I}$ , including discernibility, core, reduct and essentiality of $\mathcal{I}$ . Then we examine the contribution of these structures to granular computing. Moreover, we introduce certain granular structures using fuzzy graph models and discuss degree based model of fuzzy granular structures. Granulation of network models based on fuzzy information effectively handles real life data which possesses uncertainty and vagueness. Finally, certain algorithms of proposed models are developed and implemented to solve real life problems involving uncertain granularities. We also present a concise comparison of the models developed in our work with other existing methodologies.

Keywords:

Citation: Muhammad Akram, Ahmad N. Al-Kenani, Anam Luqman. Degree based models of granular computing under fuzzy indiscernibility relations[J]. Mathematical Biosciences and Engineering, 2021, 18(6): 8415-8443. doi: 10.3934/mbe.2021417

Related Papers:

[1]	Ansheng Ye, Xiangbing Zhou, Kai Weng, Yu Gong, Fang Miao, Huimin Zhao . Image classification of hyperspectral remote sensing using semi-supervised learning algorithm. Mathematical Biosciences and Engineering, 2023, 20(6): 11502-11527. doi: 10.3934/mbe.2023510
[2]	Wenjun Xu, Zihao Zhao, Hongwei Zhang, Minglei Hu, Ning Yang, Hui Wang, Chao Wang, Jun Jiao, Lichuan Gu . Deep neural learning based protein function prediction. Mathematical Biosciences and Engineering, 2022, 19(3): 2471-2488. doi: 10.3934/mbe.2022114
[3]	Lili Jiang, Sirong Chen, Yuanhui Wu, Da Zhou, Lihua Duan . Prediction of coronary heart disease in gout patients using machine learning models. Mathematical Biosciences and Engineering, 2023, 20(3): 4574-4591. doi: 10.3934/mbe.2023212
[4]	Shun Li, Lu Yuan, Yuming Ma, Yihui Liu . WG-ICRN: Protein 8-state secondary structure prediction based on Wasserstein generative adversarial networks and residual networks with Inception modules. Mathematical Biosciences and Engineering, 2023, 20(5): 7721-7737. doi: 10.3934/mbe.2023333
[5]	Xiaoman Zhao, Xue Wang, Zhou Jin, Rujing Wang . A normalized differential sequence feature encoding method based on amino acid sequences. Mathematical Biosciences and Engineering, 2023, 20(8): 14734-14755. doi: 10.3934/mbe.2023659
[6]	Mingju Chen, Zhongxiao Lan, Zhengxu Duan, Sihang Yi, Qin Su . HDS-YOLOv5: An improved safety harness hook detection algorithm based on YOLOv5s. Mathematical Biosciences and Engineering, 2023, 20(8): 15476-15495. doi: 10.3934/mbe.2023691
[7]	Jingren Niu, Qing Tan, Xiufen Zou, Suoqin Jin . Accurate prediction of glioma grades from radiomics using a multi-filter and multi-objective-based method. Mathematical Biosciences and Engineering, 2023, 20(2): 2890-2907. doi: 10.3934/mbe.2023136
[8]	Natalya Shakhovska, Vitaliy Yakovyna, Valentyna Chopyak . A new hybrid ensemble machine-learning model for severity risk assessment and post-COVID prediction system. Mathematical Biosciences and Engineering, 2022, 19(6): 6102-6123. doi: 10.3934/mbe.2022285
[9]	Lu Yuan, Yuming Ma, Yihui Liu . Protein secondary structure prediction based on Wasserstein generative adversarial networks and temporal convolutional networks with convolutional block attention modules. Mathematical Biosciences and Engineering, 2023, 20(2): 2203-2218. doi: 10.3934/mbe.2023102
[10]	Hong Yuan, Jing Huang, Jin Li . Protein-ligand binding affinity prediction model based on graph attention network. Mathematical Biosciences and Engineering, 2021, 18(6): 9148-9162. doi: 10.3934/mbe.2021451

Abstract

1. Introduction

Neurotoxins are toxic substances that are destructive to nerve tissue, such as AF64A, 6-hydroxydopamine, and kainic acid. In principle, the toxins act on the ion channels in the nerve-muscle junction, destroying cholinergic neurons, inhibiting the release of acetylcholine, blocking nerve-muscle conduction, causing muscle weakness, and thus making the muscle unable to contract. In severe cases, these toxins can cause suffocation and death. Neurotoxins can be classified into presynaptic and postsynaptic types according to their mechanism of action ^[1]. Presynaptic neurotoxins mainly act on the presynaptic membrane ^[2]; due to the specificity of enzyme activity, they typically block neuromuscular transmission and inhibit the release of neurotransmitters. The targets of postsynaptic neurotoxins are located in the postsynaptic membrane and can bind to acetylcholine receptors ^[3]. For example, β-methylamino-L-alanine, also known as BMAA, can damage motor neurons and has been implicated in Parkinson's syndrome. Cobra neurotoxin is a short-chain neurotoxin, the most important lethal component in cobra venom, a mainly postsynaptic neurotoxin. Because cobra venom neurotoxin is nonaddictive and non-drug resistant, it has broad prospects for persons with a drug addiction in detoxification. Therefore, the study of presynaptic and postsynaptic neurotoxins will contribute to the development of medicine, for example, to provide important clues for drug design ^[4,5,6].

Neurotoxins are a type of protein, and while their structure and function can be correctly predicted through biochemical experiments, the work is time-consuming and expensive ^[7,8,9,10]. In the genome era, many biological sequences are available ^[11], giving us a variety of methods to predict protein structure and function ^{[12,13,14,15]}. The key to correctly predicting protein structure and function is how to analyze these features using computational methods. Therefore, we can use machine learning methods for protein type prediction ^[16]. Generally, the use of machine learning to predict biological sequences mainly includes the following steps: feature extraction, model construction, and performance evaluation ^{[17,18,19,20,21,22]}. In 2009, a diversity-based method of identifying presynaptic and postsynaptic neurotoxins was proposed. The algorithm is based on the composition of amino acids and pseudo-amino acids ^[23]. To further improve the accuracy of prediction, Hua Tang et al. proposed a new feature selection technique based on the principle of variance analysis (ANOVA) ^[7,24]. In this article, we constructed a predictive model, Neu_LR, to correctly identify presynaptic and postsynaptic neurotoxins. The monoMonokGap method was used to extract the frequency characteristics of presynaptic and postsynaptic neurotoxin sequences and carry out feature selection. Then, based on the important features obtained after dimensionality reduction, the logistic regression algorithm is used to construct the prediction model Neu_LR.

2. Material and methods

2.1. The main flow of the article

As the effectiveness of machine learning technology has been continuously verified in recent years, the prediction of protein classification using machine learning-related technology has become a new research category ^[25,26]. The key to protein classification prediction with the help of machine learning technology lies in data processing and classification algorithms. The general prediction process is to first use the algorithm to extract the features of the protein and then use different classifiers to predict the protein. Therefore, the effective combination of feature extraction algorithms and classifiers has been extensively studied ^{[27,28,29,30,31]}.

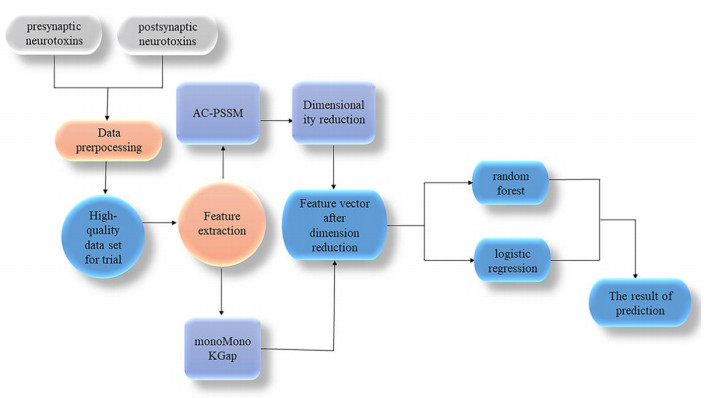
The research contents of this study include. We first download the presynaptic and postsynaptic neurotoxins from the UniProt database, and then the monoMonokGap feature expression algorithm is used to extract and select features from the data set to get the optimal features. Second, the feature vector obtained by dimensionality reduction was taken as the input, and the model was built using a logistic regression algorithm, and ten-fold cross-validation and independent test set validation was carried out. Figure 1 shows the flow chart of building the model in this paper ^[32,33].

Figure 1. Main flowchart for predicting presynaptic and postsynaptic neurotoxin proteins.

DownLoad: Full-Size Img PowerPoint

2.2. Dataset

High-quality data sets are the basis for building reliable and accurate models ^[34,35]. The UniProt database provides the scientific community with a single, centralized, authoritative source of protein sequence and functional information ^[36]. The data set used in this article is also applied to the research of Hua Tang et al. A total of 91 presynaptic and 165 postsynaptic neurotoxins were downloaded from the UniProt database. Since fuzzy information will reduce the quality of the benchmark data set and will cause the predicted model to become unreliable, we must eliminate unknown residues in the protein sequence (such as "X", "Z", "J", "O" and "B"). Because of the highly similar protein sequences in the data set, the results can be overestimated; therefore, the cut-off value of sequence identity is set to 80%. According to the results of the above screening, our data set contains 90 presynaptic neurotoxins, 165 postsynaptic neurotoxins and a total of 255 types of neurotoxin samples can be expressed by the following formula:

$S = S_{p r} \cup S_{p o}$

where subset $S_{p r}$ is a collection of 90 presynaptic neurotoxins and $S_{p o}$ is a collection of 165 postsynaptic neurotoxins.

Each protein sequence can be expressed by the following formula:

$R = r_{1} r_{2} r_{3} \cdots r_{L}$

R stands for protein sequence, $r_{i}$ stands for representative residue, and L is the length of the protein sequence. Since some machine learning methods cannot directly learn R, the protein sequences have to be converted to fixed-length vectors ^[37].

2.3. Feature selection

As the first step in building a biological sequence analysis model, feature extraction is an important part of the correct prediction of protein sequences. Generally, we can use the feature extraction method to convert the input neurotoxin sample order into a fixed-length digital vector and then use MRMD2.0 to reduce the dimensionality of the obtained feature vector as needed. Finally, we use the reduced dimensionality vector as the input vector of the classifier model and classify and process the result ^[38].

2.3.1. monoMonoKGap

The monoMonoKGap is a feature extraction method, and the best features can be generated from a large number of previously generated features, monoMonoKGap considers kGap in the nucleotide sub-sequence, frequencies of these sub-sequences are treated as prediction features. It can be used for feature extraction of DNA sequences, RNA sequences, and protein sequences ^[39]. The selection range of kGap in DNA sequences and RNA sequences is 1 to 5, and the selection range of kGap in protein sequences is 1 to 10. When the kGap value is small, the formation of the feature set is small, and the frequency of occurrence of these features retains the partial or short information sequence order, and when the kGap value is moderately large, more features will be generated to retain long sequence information ^[40]. Specifically, when kGap = 1, the sequence can be encoded as frequencies of X_X, Simultaneously generate 4×1×4 dimensional features, or 20×1×20 dimensional features, when kGap = 2, generate 4×2×4 dimensional features, or 20×2×20 dimensional features and so on ^[41]. That is when kGap = n, the DNA sequence and RNA sequence will produce 4×4×n features, and the protein sequence will produce 20×20×n features. The generated feature format is as follows:

when kGap = 1, the characteristic structure is X_X;

when kGap = 2, the characteristic structure is X_X and X__X; and so on.

where X is defined as:

$X = \left\{\begin{array}{l} \{A, C, G, T\}, \\ \{A, C, G, U\} \\ \{A, C, D, E, F, G, H, I, K, L, M, N, P, Q, R, S, T, V, W, Y\} \end{array}\right.$

This is followed by the DNA sequence, RNA sequence, and protein sequence.

For feature selection, to reduce the negative impact of dimensionality and to maintain information features, the AdaBoost classification model can be used to calculate the average impurity reduction. AdaBoost is a popular boosting classification algorithm in data mining. The core idea is to train multiple weak classifiers on the same training set, set these weak classifiers, and finally build a strong classifier ^[42]. Since Freund and Schapire proposed the AdaBoost algorithm ^[43,44,45], the improvement of AdaBoost has mainly involved two aspects: 1) adjusting the weight of the weak classifier in a new way and 2) improving the training method to reduce the error rate of classifier or save the training time.

2.3.2. Profile-based Auto covariance (AC-PSSM)

Each residue in the protein sequence has many physical and chemical properties, so the protein sequence can be regarded as a time series with corresponding properties ^[46]. PSI-BIAST is run to compare the data set and parameters to generate the outline of each sequence.

AC-PSSM ^[47] uses PSI-BIAST ^[48] as a search tool. PSI-BIAST provides a means to detect distant relationships between proteins. It is a protein sequence profile search method used to compare more sensitive protein sequences to other protein sequences. The database used is the nr library.

AC-PSSM can convert PSSMs of different lengths into vectors of fixed length. AC measures the correlation between two residues with the same property, which can be expressed as:

$\left\{\begin{array}{l} A C(i, l a g) = \sum\limits_{j = 1}^{L-l a g}\left(S_{i, j}-\bar{S}_{i}\right)\left(S_{i, j+\operatorname{lag}}-\bar{S}_{i}\right) /(L-l a g) \\ \bar{S}_{i} = \sum\limits_{j = 1}^{L} S_{i, j} / L \end{array}\right.$

(1)

where i is one of the residues, L is the length of the protein sequence, ${{S_{i, j}}}$ is the PSSM fraction of amino acid i at the jth position, and ${{{\bar S}_i}}$ is the average fraction of amino acid i in the entire protein sequence. In this way, the number of ACs can be calculated by 20×LAG, where LAG is the maximum hysteresis, where the value of lag is all integers from 1 to LAG. In this article, we set the value of LAG to the default value of 2, that is, the maximum hysteresis is 2. Figure 2 shows the flowchart for generating AC-PSSM when the hysteresis is 2. That is, when LAG = 2, AC-PSSM can generate 40 columns of feature vectors. The first 20 columns represent the characteristics of the lagging item in the PSSM matrix when lag = 1, expressed as

$(A, A, 1), (R, R, 1), (N, N, 1), \cdots, (V, V, 1)$

Figure 2. A flowchart of AC-PSSM generation. When LAG = 2, a 40-column feature vector is generated. (A) First generate the first 20 columns, that is, the features when one item is lagging. (B) Secondly, the characteristics of the last 20 columns that are lagging two items are generated.

DownLoad: Full-Size Img PowerPoint

The last 20 columns represent the characteristics of the two lags in the PSSM matrix when lag = 2, expressed as

$(A, A, 2), (R, R, 2), (N, N, 2), \cdots, (V, V, 2)$

2.3.3. Maximum correlation maximum distance (MRMD2.0)

Feature selection is also known as variable selection or attribute selection, defined as the process of selecting the feature that contributes the most to the predictor variable or output of interest. After extracting features from the sequence, the MRMD2.0 algorithm is used for feature selection.

MRMD2.0 is based on the PageRank algorithm. It is not only a Python-based tool for reducing the dimensionality of data sets but also draws performance curves based on feature dimensions. Accuracy can be sacrificed for fewer features by selecting dimensions from the performance curve ^[49,50].

2.4. Classification options and tools

The basic idea of classification is to learn the parameters of the classifier through training data, and the goal of classification is to train the parameters of the classifier with the training set with the smallest loss of accuracy ^[51,52]. Weka (3.8.5) can be used for data mining and prediction models. There are many different classification modes, such as random forest and Bayesian classifiers ^[27,49,53].

2.4.1. Random forest (RF)

The random forest algorithm is an ensemble algorithm, which is composed of multiple decision tree classifiers, and each subclassifier is a CART classification regression tree; therefore, classification and regression are performed using random forest. This algorithm is highly resistant to overfitting: the risk of overfitting can be reduced by averaging the decision tree ^[54,55]. The advantages are its simple implementation, high accuracy, fast training speed, strong anti-overfitting ability, and suitability as a benchmark model. The disadvantage is that the model is prone to overfitting on some sample sets with relatively large noise. When there are many decision trees, the training time and space will be relatively large.

2.4.2. Logistic regression (LR)

Logistic regression is a machine learning method used to solve binary classification problems, and it is a generalized linear regression ^[56]. A hyperplane can be established to classify samples, which can be described by the following formula:

$h_{\theta}(x) = g\left(\theta^{T} x\right) = \frac{1}{1+e^{-\theta T x}}$

(2)

where X is the sample $x = \left[x_{1}, x_{2}, \cdots, x_{n}\right]$ is a n-dimensional vector, g is a logistic function, and the general form is $g(z) = \frac{1}{1+e^{-z}}$ . The advantages are that the computational cost is not high and it is easy to understand and implement, but the disadvantages are that it is easy to underfit and the classification accuracy may not be high.

2.5. Performance evaluation

In this article, k-fold cross-validation was chosen to test predictions. Specificity (SP), sensitivity (SN), and accuracy (ACC) ^{[57,58,59,60,61]} were used to evaluate our proposed method ^[62]; they can be expressed as:

$\begin{gathered} S N = 1-\frac{N_{p o}^{p r}}{N^{p r}} \end{gathered}$

(3)

$\begin{gathered} S P = 1-\frac{N_{p r}^{p o}}{N^{p o}} \end{gathered}$

(4)

$\begin{gathered} A C C = 1-\frac{N_{p o}^{p r}+N_{p r}^{p o}}{N^{p r}+N^{p o}} \end{gathered}$

(5)

where ${{N^{pr}}}$ and ${{N^{po}}}$ represent presynaptic neurotoxin and postsynaptic neurotoxin, respectively; ${N_{po}^{pr}}$ indicates that the presynaptic neurotoxin was incorrectly predicted as a postsynaptic neurotoxin; and ${N_{pr}^{po}}$ indicates that a postsynaptic neurotoxin was incorrectly predicted as a presynaptic neurotoxin.

3. Results and discussion

Previous studies have shown that feature extraction is very important for predictor variables, and optimized features can improve the accuracy of model prediction ^{[63,64,65,66,67]}. Especially in some high-dimensional data, there may be some noise and redundant information, which will have some negative effects on the prediction.

3.1. Performance comparison of different feature expressions

In this section, the prediction results of monoMonokGap, AC-PSSM, 188D characteristics, Geary-related characteristics, and amino acid composition (AAC) ^[68,69] characteristics under logistic regression and random forest were compared. The results are shown in Tables 1 and 2 (where the maximum value is indicated in bold). It can be seen from Tables 1 and 2 that the monoMonokGap feature used in this model has the best performance in all indicators. Under the random forest algorithm, when kGap = 7, monoMonokGap (kGap = 7) performs the best; Under the logistic regression algorithm, when kGap = 9, monoMonokGap (kGap = 9) achieves the best result. Compared with the two results, kGap = 9 had the best performance on LR, among which the values of ACC, AUC, SP, and SN were 99.6078%, 0.996, 0.998, and 0.996, respectively. This also proves the effectiveness of monoMonokGap (kGap = 9), so we choose monoMonokGap (kGap = 9) as the feature expression method of the model.

Table 1. Different feature representation methods are used to obtain the results of using RF classifier under 5-fold cross-validation.

Method	SN	SP	AUC	ACC (%)
monoMonokGap (kGap=1)	0.910	0.870	0.966	90.9804
monoMonokGap (kGap=3)	0.941	0.912	0.985	94.1176
monoMonokGap (kGap=5)	0.945	0.920	0.992	94.5098
monoMonokGap (kGap=7)	0.957	0.936	0.991	95.6863
monoMonokGap (kGap=9)	0.949	0.917	0.992	94.902
AC-PSSM	0.839	0.776	0.868	83.9216
188D	0.710	0.609	0.794	70.9804
Geary	0.533	0.417	0.481	53.3333
AAC	0.686	0.602	0.736	68.6275

| Show Table

DownLoad: CSV

Table 2. Different feature representation methods are used to obtain the results of using LR classifier under 10-fold cross-validation.

Method	SN	SP	AUC	ACC (%)
monoMonokGap (kGap=1)	0.929	0.901	0.971	92.9412
monoMonokGap (kGap=3)	0.925	0.889	0.988	92.549
monoMonokGap (kGap=5)	0.984	0.986	0.994	98.4314
monoMonokGap (kGap=7)	0.973	0.980	0.995	97.2549
monoMonokGap (kGap=9)	0.996	0.998	0.996	99.6078
AC-PSSM	0.780	0.663	0.789	78.040
188D	0.631	0.37	0.603	63.1373
Geary	0.627	0.428	0.684	62.7451
AAC	0.667	0.460	0.637	66.6667

| Show Table

DownLoad: CSV

To further verify the stability of monoMonokGap, we tested it with an independent test set ^[70] and compared it with AC-PSSM, 188D characteristics, Geary-related characteristics, AAC and other feature expression methods. The results are shown in Tables 3 and 4. Among them, 80% of the randomly selected data sets are used to train the prediction model, and the remaining 20% are used to test the model.

Table 3. The performance comparison of different feature algorithms under LR was verified by an independent test set.

Method	SN	SP	AUC	ACC (%)
monoMonokGap (kGap=1)	0.824	0.828	0.931	82.3592
monoMonokGap (kGap=3)	0.882	0.835	0.894	88.22353
monoMonokGap (kGap=5)	0.980	0.989	0.985	98.0392
monoMonokGap (kGap=7)	0.961	0.979	0.998	96.0784
monoMonokGap (kGap=9)	0.941	0.968	0.977	94.1176
AC-PSSM	0.686	0.677	0.709	68.6275
188D	0.373	0.658	0.608	37.2549
Geary	0.588	0.397	0.396	58.8235
AAC	0.604	0.445	0.548	60.3774

| Show Table

DownLoad: CSV

Table 4. The performance comparison of different feature algorithms under RF was verified by an independent test set.

Method	SN	SP	AUC	ACC (%)
monoMonokGap (kGap=1)	0.804	0.742	0.872	80.3922
monoMonokGap (kGap=3)	0.804	0.716	0.923	80.3922
monoMonokGap (kGap=5)	0.902	0.846	0.966	90.1961
monoMonokGap (kGap=7)	0.882	0.810	0.977	88.2353
monoMonokGap (kGap=9)	0.922	0.881	0.973	92.1569
AC-PSSM	0.725	0.598	0.697	72.549
188D	0.510	0.707	0.786	50.9804
Geary	0.529	0.390	0.412	52.9412
AAC	0.566	0.501	0.553	56.6038

| Show Table

DownLoad: CSV

It can be seen from Tables 3 and 4 that, compared with other feature expression methods, the monoMonokGap feature expression method selected in this article has little difference in the results of independent verification set to test and the results of ten-fold cross-validation, which also proves that the feature expression method selected in this paper does not have over-fitting and has good stability.

3.2. Comparison with other classifiers

In this section, the performance of the model constructed in this article is compared with RF, Logical Model Tree (LMT), J48, Bayesnet, NaiveBayes, Sequential Minimal Optimization (SMO), and other classifiers. The results of ten-fold cross-validation are shown in Table 5 (the maximum value is indicated in bold). It can be seen from Table 5 that the results of the model constructed in this paper are significantly better than those of other classifiers in terms of various indicators, with the values of ACC, AUC, SP, and SN being 99.6078%, 0.996, 0.998 and 0.996 respectively. This also proves the validity of the model constructed in this article.

Table 5. Comparison of performance of different classification algorithms under 10-fold cross-validation.

Classifier	SN	SP	AUC	ACC (%)
Neu_LR	0.996	0.998	0.996	99.6078
RF	0969	0.948	0.995	96.8627
LMT	0.918	0.884	0.971	91.7647
J48	0.863	0.804	0.877	86.2745
BayesNet	0.929	0.891	0.987	92.9412
NaiveBayes	0.863	0.779	0.945	86.2745
SMO	0.973	0.965	0.969	97.2549

| Show Table

DownLoad: CSV

To further verify the robustness of the model constructed in this article, we conducted independent test set verification on it and compared its performance with RF, LMT, J48, Bayesnet, NaiveBayes, SMO, and other classifiers. The results are shown in Table 6. Among them, 80% of the randomly selected data sets are used to train the prediction model, and the remaining 20% are used to test the model.

Table 6. The performance comparison of different classification algorithms was verified by an independent test set.

Classifier	SN	SP	AUC	ACC (%)
Neu_LR	0.941	0.968	0.977	94.1176
RF	0.922	0.881	0.972	92.1569
LMT	0.784	0.756	0.886	78.4314
J48	0.843	0.788	0.824	84.3137
BayesNet	0.902	0.846	0.948	90.1961
NaiveBayes	0.804	0.691	0.920	80.3922
SMO	0.922	0.932	0.927	92.1569

| Show Table

DownLoad: CSV

It can be seen from Table 6 that, compared to other algorithms, the model constructed in this paper achieves the best prediction article, and the values of ACC, AUC, SP, and SN are 94.1176%, 0.977, 0.968, and 0.941, respectively. Moreover, compared with the results of 10-fold cross-validation, the difference is very small, which also proves that the prediction performance of the model constructed in this article does not exist overfitting and has good stability.

3.3. Comparison with state-of-the-art predictors

This section compares the model constructed in this paper with other existing methods. The comparison results are shown in Table 7, where the results of ID ^[23] and ANOVA ^[7] are obtained directly from literature. It can be seen from Table 7 that the model Neu_LR constructed in this paper has the best performance in all indicators, among which ACC, SP, and SN reach the maximum value of 99.6078%, 0.998, and 0.996 respectively, and the effect is better than the other two methods, which also proves the effectiveness of the model Neu_LR constructed in this paper ^[71].

Table 7. Comparison of different methods for predicting presynaptic and postsynaptic neurotoxins.

Method	SN	SP	ACC (%)
ID ^[23]	88.46	91.30	89.80
ANOVA ^[7]	94.51	95.15	94.92
Neu_LR	0.996	0.998	99.6078

| Show Table

DownLoad: CSV

4. Conclusions

The correct understanding of presynaptic and postsynaptic neurotoxins is an essential first step in the discovery of drug targets and drug design. And protein prediction mainly involves two aspects, feature extraction, and selection of classification algorithms. Therefore, the prediction model Neu_LR was constructed in this article. The monoMonokGap method was used to extract the frequency characteristics of presynaptic and postsynaptic neurotoxin sequences, and the feature selection was carried out. Then, based on the important features obtained after dimension-reduction, the logistic regression algorithm was used to construct the prediction model Neu_LR. In this paper, we use 10-fold cross-validation and independent test set validation to judge whether the Neu_LR model is good or not. In the 10-fold cross-validation, we achieved 99.6078% accuracy, and in the independent test set validation, we achieved 94.1176% accuracy, which shows that our model is feasible and effective.

Acknowledgments

This work was supported by the National Nature Science Foundation of China (Grant Nos 61863010, 11926205, 11926412, and 61873076), National Key R & D Program of China (No.2020YFB2104400) and Natural Science Foundation of Hainan, China (Grant Nos. 119MS036 and 120RC588), and Hainan Normal University 2020 Graduate Student Innovation Research Project (hsyx2020-41), the Special Science Foundation of Quzhou (2020D003).

Conflict of interest

All authors declare no conflicts of interest in this paper.

References

[1]	T. Y. Lin, Granular computing, Announcement of the BISC Special Interest Group on Granular Computing, 1997.
[2]	Y. Y. Yao, N. Zhong, Granular computing using finite information systems, In Data Mining, Rough Sets and Granular Computing, Physica-Verlag, 2002,102–124.
[3]	Y. Y. Yao, A partition model of granular computing, In Transactions on rough sets I, Springer, Berlin, Heidelberg, 2004,232–253.
[4]	Y. Y. Yao, J. T. Yao, Granular computing as a basis for consistent classification problems, Proc. PAKDD 02 Workshop on Foundations of Data Mining, 2 (2002), 101–106.
[5]	J. T. Yao, Y. Y. Yao, A granular computing approach to machine learning, FSKD, 2 (2002), 732–736.
[6]	G. Chiaselotti, D. Ciucci, T. Gentile, F. G. Infusino, The granular partition lattice of an information table, Inf. Sci., 373 (2016), 57–78. doi: 10.1016/j.ins.2016.08.037
[7]	S. K. M. Wong, D. Wu, Automated mining of granular database scheme, In Proc. IEEE Int. Conf. on Fuzzy Syst., 2002,690–694.
[8]	C. Bisi, G. Chiaselotti, D. Ciucci, T. Gentile, F. G. Infusino, Micro and macro models of granular computing induced by the indiscernibility relation, Inf. Sci., 388 (2017), 247–273.
[9]	C. Berge, Graphs and hypergraphs, Amsterdam: North-Holland Publishing Company, 1973.
[10]	J. G. Stell, Granulation for graphs, In Int. Conf. Spat. Inf. Theor., Springer, Berlin, Heidelberg, 1999,417–432.
[11]	F. M. Bianchi, L. Livi, A. Rizzi, A. Sadeghian, A Granular computing approach to the design of optimized graph classification systems, Soft Comput., 18 (2014), 393–412. doi: 10.1007/s00500-013-1065-z
[12]	G. Chen, N. Zhong, Granular structures in graphs, In Int. Conf. RSKT, Springer, Berlin, Heidelberg, 2011,649–658.
[13]	G. Chen, N. Zhong, Three granular structure models in graphs, In Int. Conf. RSKT, Springer, Berlin, Heidelberg, 2012,351–358.
[14]	G. Chiaselotti, D. Ciucci, T. Gentile, Simple graphs in granular computing, Inf. Sci., 340 (2016), 279–304.
[15]	G. Chen, N. Zhong, Y. Yao, A hypergraph model of granular computing, In IEEE Int. Conf. Granular Comput., 2008,130–135.
[16]	J. G. Stell, Relational granularity for hypergraphs, In Int. Conf. Rough Sets Curr. Trends Comput., Springer, Berlin, Heidelberg, (2010), 267–276.
[17]	L. A. Zadeh, Fuzzy sets and information granularity, Adv. Fuzzy Set Theor. Appl., North-Holland, Amsterdam, (1979), 3–18.
[18]	L. A. Zadeh, Towards a theory of fuzzy information granulation and its centrality in human reasoning and fuzzy logic, Fuzzy Sets. Syst., 19 (1997), 111–127.
[19]	L. Yang, S. Zhang, L. Zhu, Hypergraph clustering model based on fuzzy frequent item sets applied in management of agricultural land evaluation, In 2010 3rd Int. Conf. Inf. Manage. Innov. Manage. Ind. Eng., 3 (2010), 569–572.
[20]	Q. Wang, Z. Gong, An application of fuzzy hypergraphs and hypergraphs in granular computing, Inf. Sci., 429 (2018), 296–314. doi: 10.1016/j.ins.2017.11.024
[21]	Z. Gong, Q. Wang, On the connection of fuzzy hypergraph with fuzzy information system, J. Intell. Fuzzy Syst., 33 (2017), 1665–1676. doi: 10.3233/JIFS-16468
[22]	P. K. Singh, A. C. Kumar, J. Li, Knowledge representation using interval-valued fuzzy formal concept lattice, Soft Comput., 20 (2016), 1485–1502. doi: 10.1007/s00500-015-1600-1
[23]	B. $\check{ S}$ e $\check{s}$ elja, A. Tepav $\check{s}$ evi $\acute{c}$ , Fuzzy ordering relation and fuzzy poset, Pattern Recognition and Machine Intelligence, PReMI 2007, Lecture Notes in Computer Science, Springer, Berlin, Heidelberg, 4815, (2007).
[24]	A. Pedrycz, K. Hirota, W. Pedrycz, F. Dong, Granular representation and granular computing with fuzzy sets, Fuzzy Sets. Syst., 203 (2012), 17–32. doi: 10.1016/j.fss.2012.03.009
[25]	M. Akram, A. Luqman, J. C. R. Alcantud, Risk evaluation in failure modes and effects analysis: hybrid TOPSIS and ELECTRE I solutions with Pythagorean fuzzy information, Neural Comput. Appl., 33 (2021), 5675–5703. doi: 10.1007/s00521-020-05350-3
[26]	A. Luqman, M. Akram, J. C. R. Alcantud, Digraph and matrix approach for risk evaluations under Pythagorean fuzzy information, Expert Syst. Appl., 170 (2021), 114518. doi: 10.1016/j.eswa.2020.114518
[27]	M. Akram, C. Kahraman, K. Zahid, Group decision-making based on complex spherical fuzzy VIKOR approach, Know. Based Syst., (2021), 106793.
[28]	Z. Pawlak, Rough sets, Theoretical Aspects of Reasoning About Data, Kluwer Academic Publisher, 1991.
[29]	Z. Pawlak, Granularity of knowledge, indiscernibility and rough sets, Proc.1998 IEEE Int. Conf. Fuzzy Syst., (1998), 106–110.
[30]	C. Kaur, R. Kumar, A fuzzy hierarchy-based pattern matching technique for melody classification, Soft Comput., 2 (2019), 7375–7392.
[31]	T. O. William-West, D. Singh, Information granulation for rough fuzzy hypergraphs, Granul. Comput., 3 (2018), 75–92. doi: 10.1007/s41066-017-0057-2
[32]	Y. Y. Yao, Information granulation and rough set approximation, Int. J. Intell. Syst., 16 (2001), 87–104. doi: 10.1002/1098-111X(200101)16:1<87::AID-INT7>3.0.CO;2-S
[33]	Y. Y. Yao, Information granulation and approximation in a decision-theoretical model of rough sets, In Pal S.K., Polkowski L., Skowron A. (eds) Rough-Neural Computing. Cognitive Technologies, Springer, Berlin, Heidelberg, (2004).
[34]	A. Luqman, M. Akram, A. N. A. Koam, An $m$ -polar fuzzy hypergraph model of granular computing, Symmetry, 11 (2019), 483. doi: 10.3390/sym11040483
[35]	A. Luqman, M. Akram, A. N. A. Koam, Granulation of hypernetwork models under the $q$ -rung picture fuzzy environment, Mathematics, 7 (2019), 496. doi: 10.3390/math7060496
[36]	M. Akram, A. Luqman, A. N. Al-Kenani, Certain models of granular computing based on rough fuzzy approximations, J. Intell. Fuzzy Syst., 39 (2020), 2797–2816. doi: 10.3233/JIFS-191165
[37]	M. Akram, A. Luqman, Granulation of ecological networks under fuzzy soft environment, Soft Comput., 24 (2020), 11867–11892. doi: 10.1007/s00500-020-05083-4
[38]	W. Pedrycz, Granular computing as a framework of system modeling, J. Control Autom. Electr. Syst., 24 (2013), 81–86. doi: 10.1007/s40313-013-0010-9
[39]	W. Pedrycz, Allocation of information granularity in optimization anddecision-making models: Towards building the foundationsof Granular Computing, Eur. J. Operat. Res., 232 (2014), 137–145. doi: 10.1016/j.ejor.2012.03.038
[40]	L. A. Zadeh, Fuzzy sets, Inf. Cont., 8 (1965), 338–353.
[41]	L. A. Zadeh, Similarity relations and fuzzy orderings, Inf. Sci., 3 (1971), 177–200. doi: 10.1016/S0020-0255(71)80005-1
[42]	Z. Gong, Q. Wang, On the connection of fuzzy hypergraph with fuzzy information system, J. Intell. Fuzzy Syst., 33 (2017), 1665–1676. doi: 10.3233/JIFS-16468
[43]	J. N. Mordeson, P. S. Nair, Fuzzy graphs and fuzzy hypergraphs, Physica Verlag, Heidelberg, Second Edition, 46 (2000), 1–250.
[44]	A. Rosenfeld, Fuzzy graphs, In fuzzy Sets and their applications, L. A. Zadeh, K. S. Fu, and M. Shimura, Eds., Academic Press, New York, USA, 1975, 77–95.
[45]	K. Radha, N. Kumaravel, The degree of an edge in Cartesian product and composition of two fuzzy graphs, Int. J. App. Math. Stat. Sci., 2 (2013), 65–78.
[46]	A. Luqman, Granulation of network models under fuzzy hybrid information, Higher Education Commission, PhD Thesis, 2020.
[47]	H. S. Nawaz, M. Akram, J. C. R. Alcantud, An algorithm to compute the strength of competing interactions in the Bering Sea based on Pythagorean fuzzy hypergraphs, Neural Comput. Appl., (2021), 1–23.
[48]	M. Akram, H. S. Nawaz, Inter-specific competition among trees in Pythagorean fuzzy soft environment, Complex Intell. Syst., (2021), 1–22.
[49]	F. Zafar, M. Akram, A novel decision-making method based on rough fuzzy information, Int. J. Fuzzy Syst., 20 (2018), 1000–1014. doi: 10.1007/s40815-017-0368-0
[50]	M. Akram, F. Zafar, Hybrid soft computing models applied to graph theory, Springer International Publishing, 2020, 1–434.

This article has been cited by:

1.	Hao Wan, Qing Liu, Ying Ju, Utilize a few features to classify presynaptic and postsynaptic neurotoxins, 2023, 152, 00104825, 106380, 10.1016/j.compbiomed.2022.106380
2.	Muhammad Nabeel Asim, Muhammad Ali Ibrahim, Ahtisham Fazeel, Andreas Dengel, Sheraz Ahmed, DNA-MP: a generalized DNA modifications predictor for multiple species based on powerful sequence encoding method, 2023, 24, 1467-5463, 10.1093/bib/bbac546
3.	Hongwei Zhang, Yan Shi, Yapeng Wang, Xu Yang, Kefeng Li, Sio-Kei Im, Yu Han, LMFE: A Novel Method for Predicting Plant LncRNA Based on Multi-Feature Fusion and Ensemble Learning, 2025, 16, 2073-4425, 424, 10.3390/genes16040424

Reader Comments

Your name:*

Email:*
© 2021 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)