Strain-induced packing transition of Ih Cu_n@Ag_55-n(n = 0, 1, 13, 43) clusters from atomic simulations

1.   Introduction

Nanometallic clusters present different physical and chemical properties from their bulk counterparts ^[1,2,3,4,5]. As the cluster size decreases, the ratio of the atomic number in the surface to the total number increases in these nanoclusters, where the surface atoms have fewer coordination atoms and higher migration ability than those atoms in the interior. When these nanoclusters contain two or more components of metals, they are named as alloying clusters ^[6]. Dating back to the 19th century, Michael Faraday carried out a study on the size-dependent optical properties of Au and Ag colloids or nanoclusters ^[7]. During recent decades, bimetallic nanoclusters have attracted much attention of enterprises and scholars due to their wide applications in magnetism, optics and catalysis ^[8,9,10]. Nanoclusters exhibit great morphological differences with different temperatures and cluster’s sizes, where the morphologies of these nanoclusters are determined by geometric structure and chemical ordering. In fact, the distribution of component atoms in these clusters is not only a scientific issue, but also a practical technical problem ^[11].

From the point of view of basic research, bimetallic clusters can be used as a model system to provide the knowledge of surface effect on thermodynamics of alloys at nanometer scale. In practical applications, the anisotropy of magnetic crystals in nano-devices can be enhanced by changing the distribution of components ^[12]. In the optical field, the plasma resonance of nanoparticles can be fine-tuned by adjusting the metallic composition ^[13,14,15]. Moreover, the activity, reaction effectiveness, and selectivity of the catalyst mainly depend on how the catalytic components are arranged in several atomic layers on the surface of a nanocluster. Ag nanoclusters have been used in ethylene oxidation reaction for many years, and the addition of Cu is beneficial to the choices of oxidation path. Therefore, it is necessary to understand the arrangements of Ag and Cu atoms in catalyst at high temperatures ^[16,17,18]. The solid solution of binary Ag-Cu alloy is unstable, where the Ag-Cu alloy can be separated into Cu phase and Ag phase because of the large miscibility gap and mixing enthalpy in the eutectic system. When the binary system is in a low dimensional form, a Ag segregation strongly occurs in the surface of this system. In recent years, great attention has been paid to the substitution of Cu atoms for Ag in these Ag nanoclusters. This is due to the lower price and better electrical conductivity of Cu metals compared to Ag ones, and the excellently electrical, optical, and catalytic properties of Cu. When these two kinds of the atoms form an alloyed cluster, the Cu atoms tend to occupy core positions of the cluster, while the Ag atoms are on the surface of the Cu nucleus to form a thermodynamically stable arrangement of a Cu core coated with a Ag shell, which is identified as a Cu@Ag structure. Because the atoms in the core region have smaller radius than those in the shell, the strain release being related to atomic stress leads to a stable morphology, which prefers to the icosahedron configuration for small size clusters. In studying the importance of kinetic trapping and the role of atomic stress in structural transformation, Emanuele Panizon and Riccardo Ferrando performed atomistic and density-functional theory (DFT) calculations for strain-induced restructuring of the surface in core@shell nanoalloys comprising Co@Au, Co@Pt, Co@Ag, Ni@Ag, Cu@Au, Ni@Rh and Ni@Pd ^[19]. Recently, Diana Nelli and Riccardo Ferrando simulated the evolution of AuCo, AgNi and AgCu nanoalloys with the aim of comparing their evolution pathways towards equilibrium from initially disordered configurations ^[20]. In addition to sensors and catalysts, the Cu@Ag alloy clusters can be also used as low-temperature lead-free solders for electronic devices owing to their excellent conductivity, thermal conductivity and low melting point ^[21]. Mass spectrometric analysis showed that some clusters containing specific atomic numbers had a highly structural stability ^[22], that the number was named as “Magic Number”. In these magic clusters, the cluster containing 55 atoms has a typical icosahedron structure, which of particular interest to researchers ^[23,24,25]. It has been shown that the Ag₅₅ cluster has ferromagnetic properties, and its surface plasma mode can be tuned by doping Cu atoms. Ag₅₅ cluster has many potential applications, especially for solar cells and sensors ^[26].

In this work, atomic simulations using molecular dynamics were performed to explore the changes of atom packing and local strain of Ag₅₅, Cu₁Ag₅₄, Cu₁₃Ag₄₂, and Cu₄₃Ag₁₂ clusters with differences in core atoms at elevated temperatures. Here, local structural changes and rips of these clusters were demonstrated by potential energy per atom, shape factors, pair distribution functions, and strain distribution as well as visual atomic packing within the framework of embedded atom method (EAM).

2.   Model and simulation

In this paper, the interaction of atoms is described by the EAM form, which was proposed by Williams ^[27]. The total potential energy of the system E_tot is determined by

where ${V_{ij}}\left({{r_{ij}}} \right)$ is the potential energy between atoms i and j having a distance of r_ij, ${F_i}\left({{{\bar \rho }_i}} \right)$ is the embedded energy with an electron density of $\overline {{\rho _i}}$ at the position of the atom i. The density value is obtained from the superposition and sum of the electron density from the nearest neighbor atoms of the atom i. ${\rho _j}$ is the electron density of the neighbor atom j of the atom i. In the present EAM simulations, when the atomic packing changes, the total potential energy changes accordingly. In structural relaxation processes at a temperature after a certain time steps, the atoms present thermal movements around their equilibrium positions. Therefore, the energy changes in an oscillating mode. At each temperature, we determine the configuration with the lowest energy in the last time steps of 3N-6 (N = 55), which is the freedom of one particle.

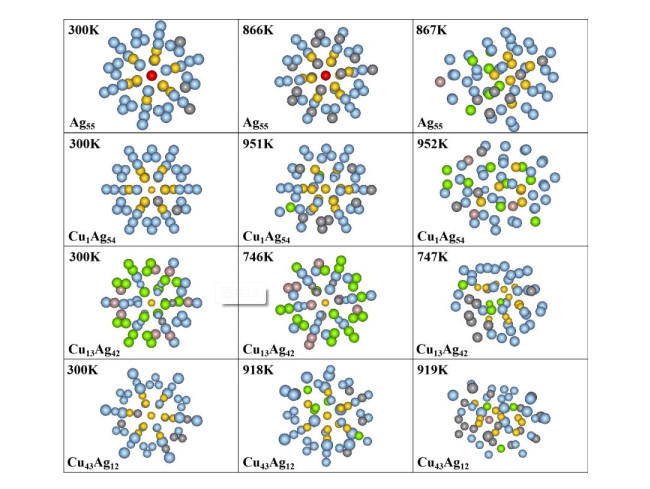
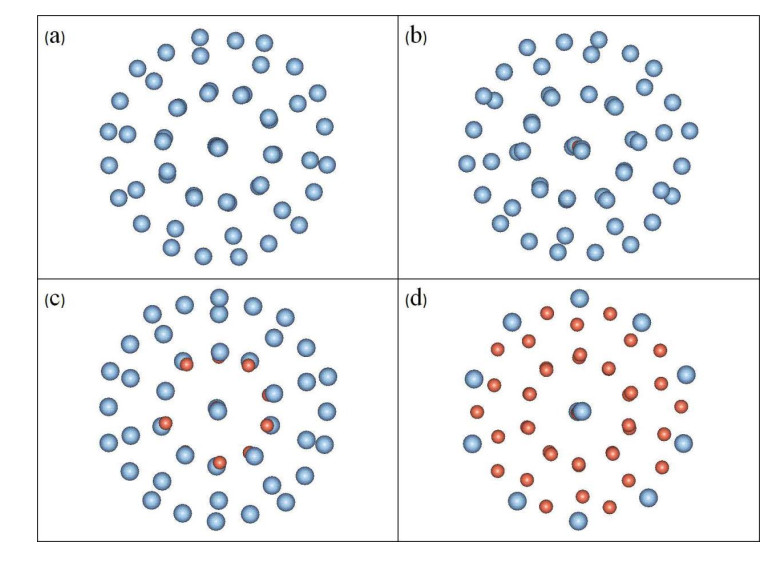
The simulations were carried out in the NVT ensemble using Andersen thermostat. By solving Newton’s motion equations, we could obtain the positions and velocities of each atom, and a predictor-corrector algorithm was used to integrate equations of motion. Throughout the simulations, a time step of 1.6 × 10^-15 s is used. At each temperature, the system was first fully equilibrated in 980, 000 time steps before running to accumulate statistics，and the atomic trajectories and energy recorded in the subsequent 20, 000 time steps were used to obtain thermodynamic equilibrium values. Initially, we constructed a 20a₀ × 20a₀ × 20a₀ bulk faced center cubic (FCC) Ag crystal (the lattice constant a₀ is 4.09 Å), and 55 atoms are extracted from this constructed Ag crystal. The 20a₀ × 20a₀ × 20a₀ is the size of the MD simulation cell. Here, the box size of the simulated central cell is enough large to avoid the interaction of the atoms in this central cell with the other atoms in its 26 neighbor imaging cells under periodic boundary conditions. The Ag₅₅ cluster has an icosahedron (Ih) configuration by structural relaxation at 300K. Three kinds of Cu@Ag clusters including Cu₁Ag₅₄, Cu₁₃Ag₄₂ and Cu₄₃Ag₁₂ were obtained by replacing the Ag atoms in the core region of the pure metal clusters with 1, 13 and 43 Cu atoms respectively. Then, these three clusters were subjected to structural relaxation at 300K. Figure 1 shows the atomic packing of Ag_55, Cu₁Ag₅₄, Cu₁₃Ag₄₂ and Cu₄₃Ag₁₂ clusters with the Ih configurations at this temperature, where the red balls are used to represent Cu atoms, and the silver-gray spheres Ag atoms. The simulations were performed by starting with the optimal structure at 300K, then increasing gradually the temperature to 1300K at an increment of 50K. The initial structures at a temperature above 350K were from the coordinates of the last time step of the previous temperature.

Figure 1.  Packing of the clusters at the temperature of 300K. (a) Ag₅₅, (b) Cu₁Ag₅₄, (c) Cu₁₃Ag₄₂, (d) Cu₄₃Ag₁₂.

DownLoad: Full-Size Img PowerPoint

The following values were determined in the simulations.

where $^{\left\langle - \right\rangle }$ denotes the average over the entire trajectory, and N is the atom number in this cell. g(r) is the pair distribution function, and gives the possibility of finding the atom pairs at a given distance r. When $r = {r_{ij}}$, δ is 1, whereas $r \ne {r_{ij}}$, it zero.

The moment of inertia can reflect atomic positions and mass distribution. As a tensor, it can be determined by：

where m_Cu/Ag is the mass of Cu atom or Ag atom，${x_c}$ is the mass center，${x^i}$ or ${x^j}$ is the coordinate of the iatom. i (or j) is 1, 2, 3 corresponds to the x, y and z axes, respectively. Three values of principle axes I¹, I² and I³ are obtained by diagonalization of tensor component, where the I¹ and I³ are the maximum and minimum values. The shape factor can be defined as:

If the shape factor value is closer to 1, the cluster has one nearly spherical shape.

The atomic stress is given by

where $r_{ij}^a$ and $r_{ij}^b$ (with a, b = x, y, z) are the Cartesian components of the vector ${r_{ij}}$, and ${r_{ij}}$ is its modulus. ${V_i}$ is the atomic volume ^[11]. The isotropic atomic pressure ${P_i}$ is related to the ${\sigma _i}$ as follows，

3.   Results and discussions

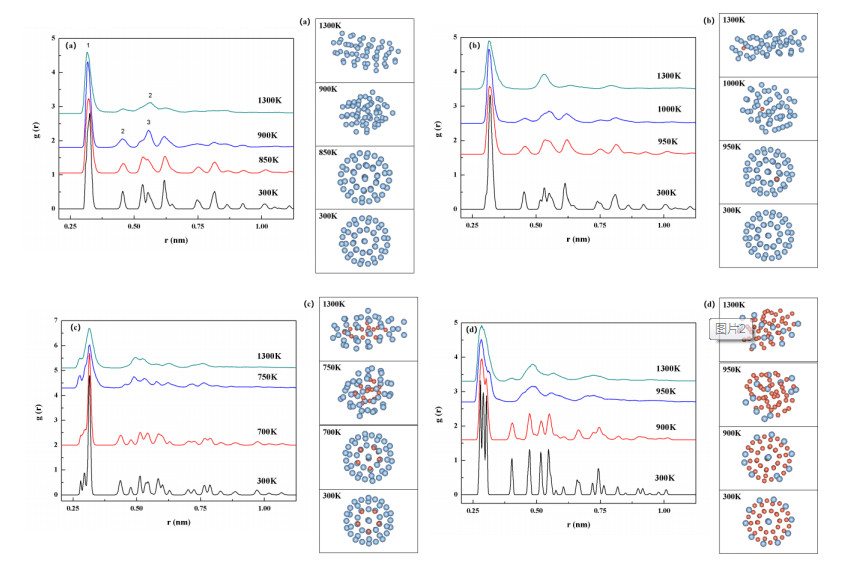
As given in the Eq (1), both of the embedding energy and potential energy of paired atoms contribute to the total potential energy of the simulated clusters. If the atomic packing changes, the electron charge density given in the Eq (2) and the distance between a pair of atoms change accordingly, also resulting in the changes of the embedding energy and potential energy. In the meantime, the cluster’s shape change correspondingly. As illustrated in Figure 2, the potential energy of one copper core-silver shell cluster at 300K is lower than that of the pure Ag cluster, which indicates that the substitution of Ag atoms by Cu atoms contributes to the stability of the alloy system. For the simulated Ag₅₅, Cu₁Ag₅₄, Cu₁₃Ag₄₂, or Cu₄₃Ag₁₂ clusters, the energy jumps significantly at the temperature of 900K, 1000K, 750K, or 950K, suggesting the apparent transition of the atomic packing. Accompanying the transition, the cluster is obviously elongated. Below the transition temperature, the cluster can hold its nearly spherical shape, whereas above this temperature, it appears as a rod. It can be noted that the energy of Ag₅₅ has a jump at 700K, and then it has restored its icosahedral configuration. Among these clusters, Cu₁Ag₅₄ has the highest elongation rate at 900K owing to the movements of the Cu atom. For the Cu₁Ag₅₄ and Cu₁₃Ag₄₂ clusters, the apparent changes of the energy occur in a temperature range being close to these transition temperatures, indicating the position changes of a few of atoms.

Figure 2.  Variation of internal energy per atom and shape factor in Ag₅₅, Cu₁Ag₅₄, Cu₁₃Ag₄₂, Cu₄₃Ag₁₂ clusters with temperature.

DownLoad: Full-Size Img PowerPoint

In Figure 3a, pair distribution function (PDF) of the Ag₅₅ cluster present the features of discrete peaks, suggesting the orderly packing of the atoms. With the increase of temperature, thermal movements of atoms around their equilibrium positions lead to the broadening of the peaks. In the meantime, there are not obvious changes of the positions and shape of these peaks. When the temperature reaches 900K, the changes of the shape and position of the third peak (labeled as 3 in this figure) indicate differences of the atomic packing from those below this temperature. Above 900K, the apparently discrete peaks suggest that most of the atoms are still orderly packed as shown in the visually packing pictures in the right of this figure. It should be noted that the shape of the first peak (labeled as 1) of this Ag₅₅ cluster does not present symmetrical form, which is due to the small differences in the atom distances between the atoms forming pairs corresponding to the first nearest neighbors. Here, the distances in inner region of this cluster is smaller than those in the outer region. The visually atomic packing shows that a few of atoms in the surface adjust their positions at 850K. At 900K, although this cluster loses its Ih configuration, most of the atoms still are packed into orderly local structures. For the Cu₁Ag₅₄ cluster, the position and shape of the peaks at 300K are similar to those of the pure Ag cluster, but there is one tiny peak at the left side of the first nearest peak. In addition, there exists a split of the peak at the position of 0.525 nm. The reason is that the occupied volume of the Cu atom at the core of the cluster is smaller than that of the Ag atom. Meanwhile, the pairs’ distances formed by the Cu atom and other Ag atoms are mainly near the positions of the third nearest neighbor peak. With increasing the number of Cu atoms to 13, although the PDF at 300K still presents the features of orderly packing of the atoms, there are many small peaks. For this Cu₁₃Ag₄₂ cluster, there are three kinds of pairs including Cu-Cu, Cu-Ag, and Ag-Ag. Therefore, two small peaks occur on the left side of the first main peak of the PDF curve. As the number of the Cu atoms in the core region increases to 43, the positions of the peaks shift significantly to the left. In addition, the number and shape of these peaks are also different from those of the other three clusters. As the temperature increases, most of the atoms in these Ih clusters hold their orderly packing. For the Cu₁Ag₅₄ cluster at 950K, it can be found that the Cu atom has moved away from the central position, while one Ag atom occupied this position. At a certain high temperature, these clusters lose their icosahedral configurations, which have been indicated from the shape factor changes of Figure 2b. Here, these particles are stretched, and their atomic packing will be present in the following Figure 4. Further calculations show the transition temperatures are 867K, 952K, 747K and 919K for the Ag₅₅, Cu₁Ag₅₄, Cu₁₃Ag₄₂ and Cu₄₃Ag₁₂ clusters respectively. Also we found that the Cu₁Ag₅₄ lost its Ih configuration at a higher temperature compared with its pure Ag counterpart. The phenomenon comes from the fact that the single Cu impurity helps stress release at the central site, resulting in increasing the thermal stability of the Ih structure as previously observed by C. Mottet et al. ^[28]. Here, the calculation of structurally transition point is performed at some temperatures by a “bisection” algorithm to narrow the search range until the point is found.

Figure 3.  Pair-distribution functions (PDFs) of the clusters at different temperatures. (a) Ag55, (b) Cu1Ag54, (c) Cu13Ag42, (d) Cu43Ag12.

DownLoad: Full-Size Img PowerPoint

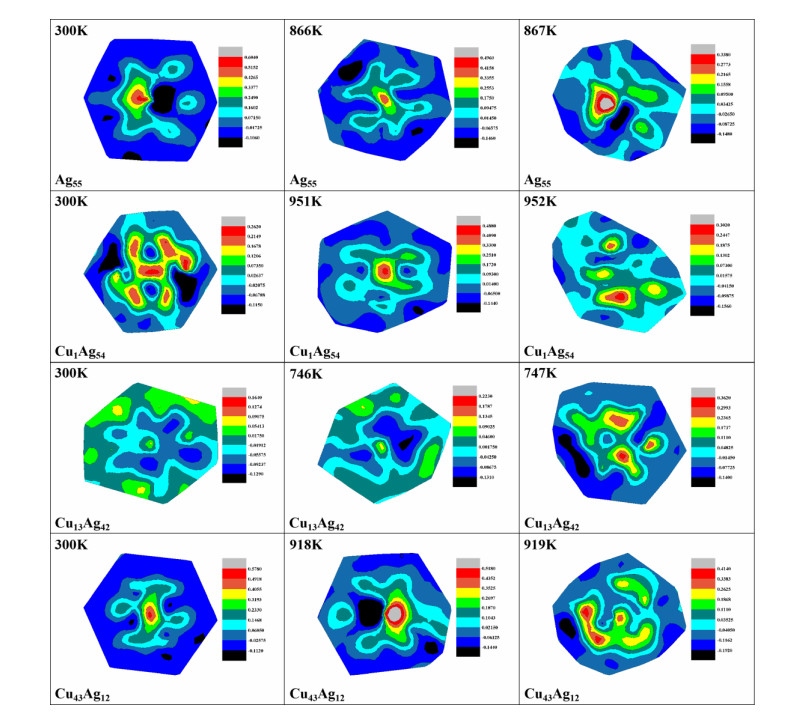
Figure 4 shows the pressure distribution on the atoms at room temperature, and the temperatures on both sides of the transition temperature. In these pictures, the value of pressure on red, yellow, green and brown balls are positive. Here the red atom is under the maximum positive pressure, yellow takes the second place, green the third place, while the brown one is the smallest. These atoms are subjected to the compression. The values of pressure on blue and black balls are negative, where the black atom is heavily stretched. Among them, the pressure value of green, brown and blue balls are very small. In these figures, small balls represent the Cu atoms, and large balls the Ag atoms. At 300K, we can see that the interior regions of the Ag₅₅, Cu₁Ag₅₄ and Cu₄₃Ag₁₂ clusters are obviously compressed. In the present simulations, at a certain temperature, there exist the rotations of the particles owing to the thermal movements. The rotation has apparent effect on average values of atomic coordinates in these small clusters, and we use the instantaneously trajectories in the statistics time steps to analysis the structure information at this temperature. Therefore, a few of atoms in the surface have relatively large deviation from their equilibrium positions, and they undergo negative pressure, which are stretched. For the Cu₄₃Ag₁₂ cluster, some positions in sub-surface are occupied by the Cu atoms, and they are under tensile. For the Cu₁Ag₅₄ cluster, a considerable number of atoms in the cluster suffer from compressive strains, due to the occupied smaller volume of the Cu atom in the core, resulting in the shrinking to the core for the Ag atoms. In the outer layer, the tension is obviously reduced. With increasing the temperature to the points of packing transition for the Ag₅₅, Cu₁Ag₅₄ and Cu₄₃Ag₁₂ clusters, although the inner regions still undergo compression, a considerable number of atoms in the outer layer are under strong negative pressure, where the tensile strain mainly occurs in the regions occupied by the Cu atoms. The strong tension at a high temperature results in the transition from the Ih configuration to locally ordered packing for the Ag₅₅, Cu₁Ag₅₄, and Cu₄₃Ag₁₂ clusters. Correspondingly, the shape of them becomes elongation. Under the case of the Cu₁₃Ag₄₂ cluster, these Cu atoms in the core region is helpful in releasing the strain of the whole cluster, where most of the Cu and Ag atoms do not undergo the strong negative or positive pressure. But in Cu/Ag interfaces, there are some strain regions occupied by the Cu atoms. At 747K, most of the Cu atoms are still in the inner regions of this cluster, and they are compressed. To indicate the differences of the strains in these clusters, Figure 5 shows contour images of atomic pressure in these four clusters at different temperatures. At 300K, we can find that the core regions present deep red, suggesting that these regions are heavily compressed, whereas the outer regions stretched. It can be noted that there are large red area for the Cu₁Ag₅₄ cluster due to the high-stress. As the temperature increases, the concentrated compressive strain area becomes smaller, and fragmented in these clusters containing Cu atoms. Upon heating, island morphologies occur for different stress regions.

Figure 4.  Atomic pressure in four clusters at different temperatures.

DownLoad: Full-Size Img PowerPoint

Figure 5.  The projections of atomic pressure in four clusters at different temperatures.

DownLoad: Full-Size Img PowerPoint

From the above images, we can get the implication that even the doping of one Cu atom can greatly affect the structural transformation of silver clusters. Accounting for the fact that properties of nano-clusters are highly sensitive to their geometries on an atom-by-atom basis, chemical composition and ordering of atoms provide some additional degrees of freedom for engineering their properties. When researchers understand the thermodynamics of such core-shell structure of the CuAg nanosystems, it could benefit functionalities in nano dimension.

4.   Conclusions

Comparing the results obtained for simulated clusters without or with different number of Cu atoms in the core regions of Ih Ag₅₅ clusters, inevitable conclusion is that the core atoms affect the strain distribution in these clusters, resulting in the different temperature of packing transition. Simulation finding addresses different packing changes on the results of the energy variations, shape factor, PDF curves, pressure’ analysis together with the visually cross-sectional images. For the cluster with high ratio of copper or pure silver, when the core atoms undergo strong compression, there exists apparent tension in the outer-shell regions. As the temperature increase, extended tensile strain zones in the surface induce the packing transition of the Ag₅₅ and Cu₄₃Ag₁₂ clusters. The similar phenomena occur for packing transition of the Cu₁@Ag₅₄ cluster at a high temperature. Here, there is not strong strain in the surface regions at room temperature because of small inward contraction owing to the smaller atomic volume of the central Cu atom compared to that of the replaced Ag atom. For a certain number of Cu atoms located in the core of the cluster, high strain zones exist on the Cu atom side of the Cu/Ag interfaces in the inner of the cluster, resulting in the lowest transition temperature of the atomic packing in the four simulated clusters.

Acknowledgements

We acknowledge the financial support from the National Natural Science Foundation of China (No. 51671051) and the National Key R & D Program of China (Grant No. 2016YFB0701304).

Conflict of interest

All authors declare no conflicts of interest in this paper.