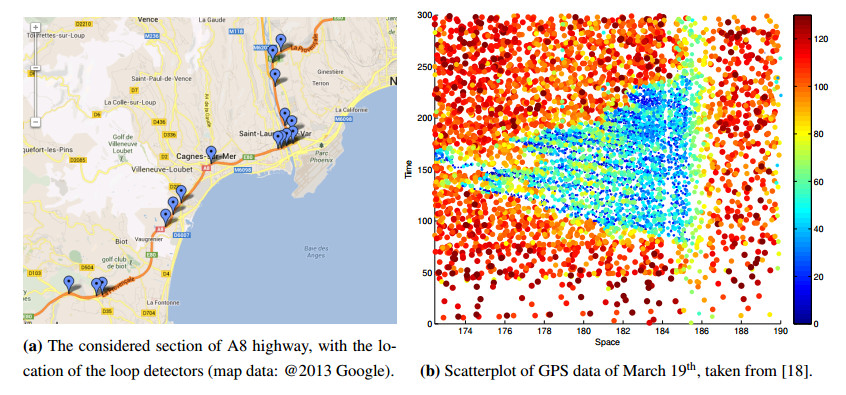
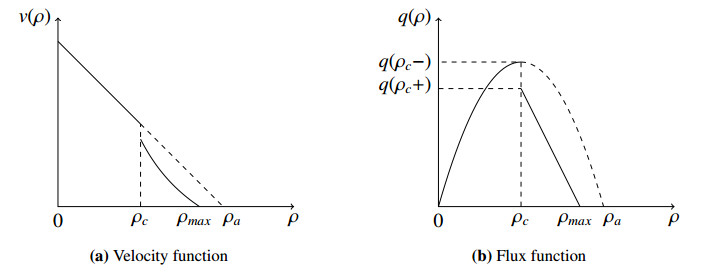
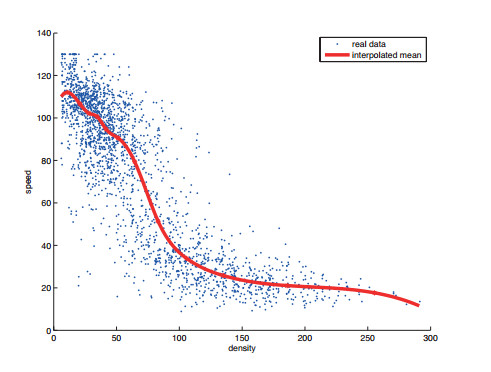
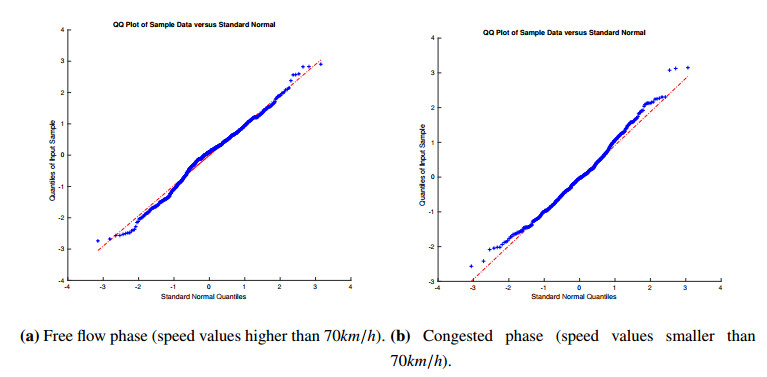
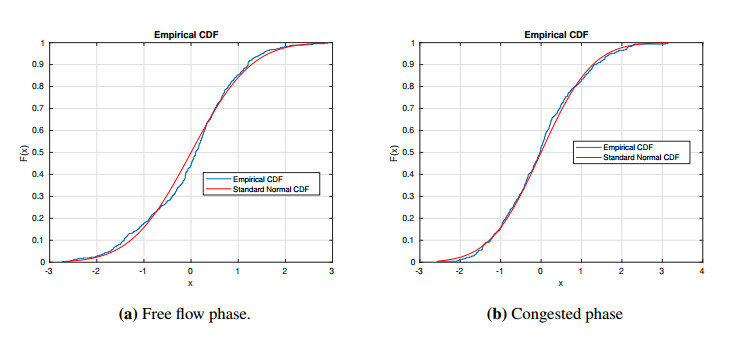
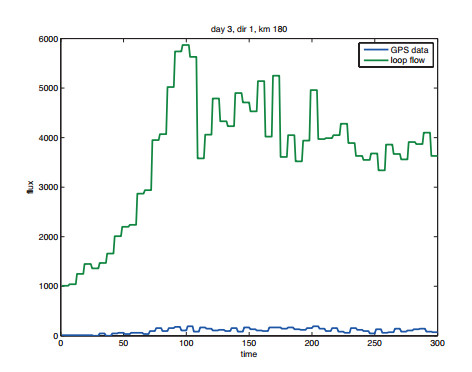
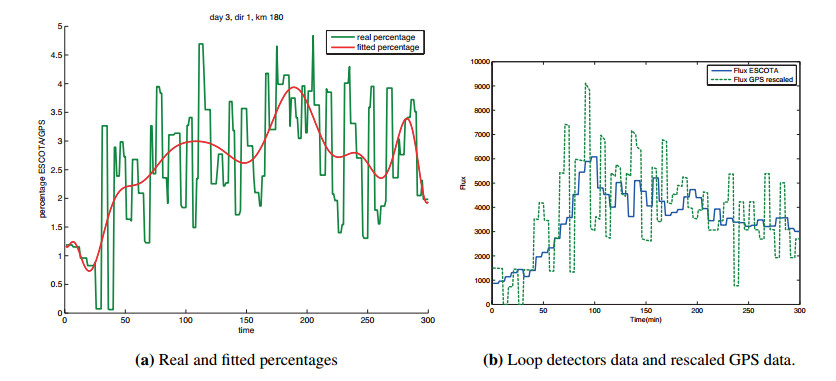
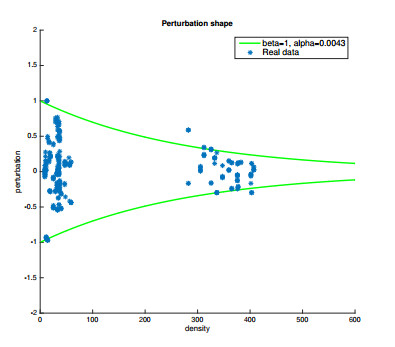
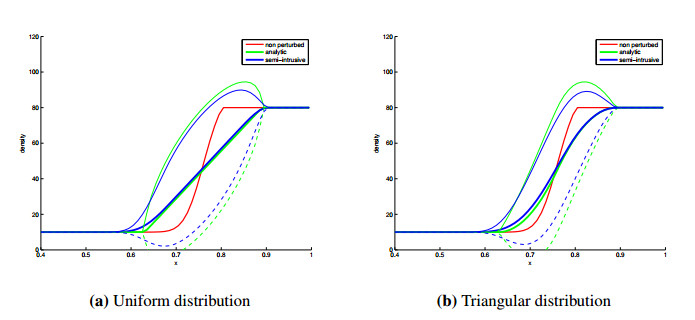
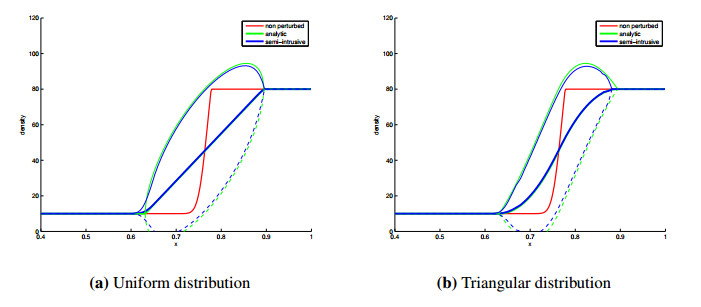
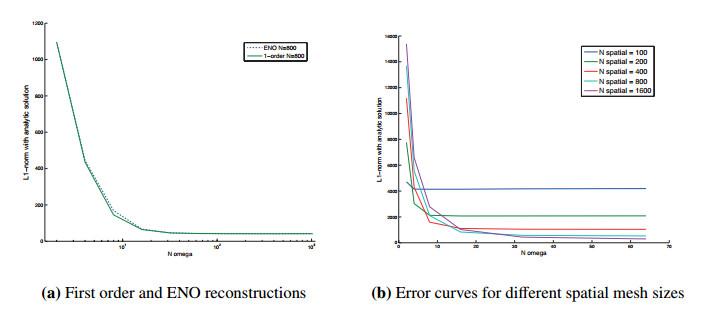
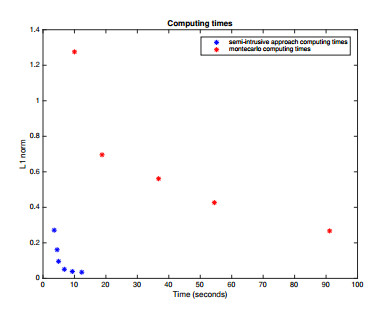
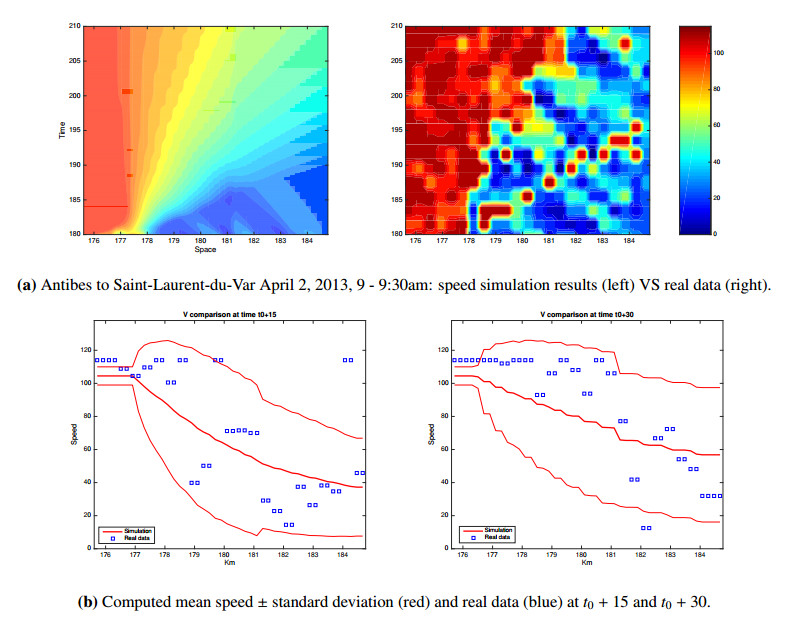
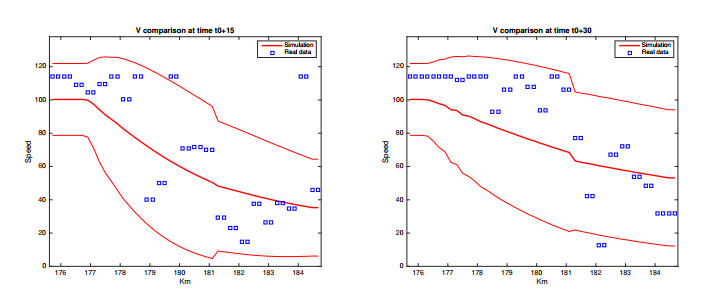
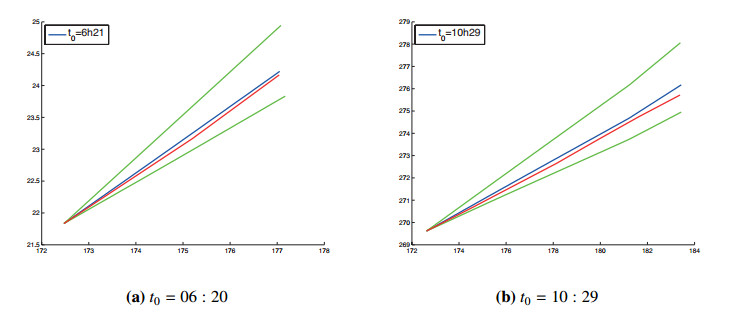
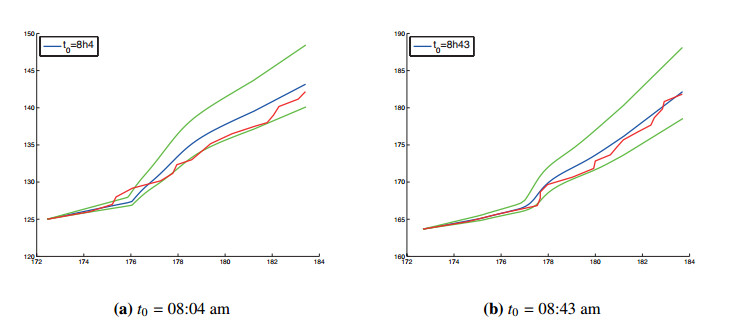
The objective of this paper is to analyze the inclusion of one or more random parameters into the deterministic Lighthill-Whitham-Richards traffic flow model and use a semi-intrusive approach to quantify uncertainty propagation. To verify the validity of the method, we test it against real data coming from vehicle embedded GPS systems, provided by Autoroutes Trafic.
1.
Introduction
A turbidostat, similar to a chemostat or an auxostat, is a basic piece of laboratory apparatus used to cultivate microorganisms, and it has feedback between the concentration of the fluid in the culture dish and the dilution rate [1,2,3,4,5]. Compared with the chemostat [6,7], the turbidostat can feed back the state values to the controller through the photoelectric system to control the flow rate of the limiting nutrient in the fresh inflowing medium according to the concentration of the fluid in the culture dish, so as to achieve the purpose of high-efficiency culture. The device used to measure the concentration of the liquid is called the optical sensor, which can measure both the microbial species concentration and the substrate concentration [8,9]. At present, there are a variety of microorganisms that can be continuously fermented by large-size turbidostats according to this principle in the fermentation industry. Microbial metabolites in balance with the bacteria can also be produced by continuous fermentation with a turbidostat. In consideration of the important value of the turbidostat in theory and practical application, the research on the kinetic model of the turbidostat has attracted the attention of many biologists, experimental technicians and mathematicians [10,11,12,13,14,15,16]. Leenheer and Smith [10] investigated the coexistence of two species in a turbidostat model with monotonic uptake functions by dint of feedback control of the dilution rate. In [11], a turbidostat model of exploitative competition for an inhibitory growth-limiting nutrient between two species of microorganisms was considered. Further, the author of [12] analyzed the dynamics of a turbidostat model with a non-monotonic response function and found that the coexistence can be dependent on the original condition if the washout equilibrium and the interior equilibrium are asymptotically stable synchronously.
However, in reality, no matter how sensitive a piece of online equipment used to measure nutrient or microorganism concentrations is, there invariably exists a time delay in the measurement of the concentration of the fluid and when this signal can be available to regulate the input flow rate. That is, online equipment typically only provides delayed discrete measurements. In [17], Mikheev et al. considered a digital controlled continuous-time system as a continuous-time system with time-varying delayed control input, and then Fridman [18] further researched the control system which can be described in the form of differential equations with after-effects. Subsequently, turbidostat systems with delayed feedback control have been investigated by multitudinous researchers [19,20,21,22,23,24,25]. Yuan et al. [22] revealed that the time delay may lead to the loss of stability and generate various oscillations and periodic solutions under the condition that the dilution rate depends on the turbidity of two competing species. In [19], by taking the dilution rate related to the substrate concentration as a feedback control variable, the author studied the asymptotic stabilization for a turbidostat by considering a delay on its output. A feedback analysis for turbidostats whose growth functions can be nonmonotonic was carried out in [23], where the dilution rate rests with the substrate concentration with piecewise constant delayed measurements.
Inevitably, population dynamics are susceptible to environmental noise in almost all ecological systems, which primarily involves continuous white noise. Under well-controlled laboratory conditions, even if the observed experimental results are very consistent with the theoretical behaviors of ordinary differential equations in the turbidostat system, the possible differences under operational conditions cannot be neglected. By introducing white noise, the dynamics of turbidostat models can be better understood [26,27,28,29,30,31]. The persistence in mean and stochastic persistence of a stochastic turbidostat model were obtained [32], as white noise is introduced by the maximal growth rate. Mu et al. [33] obtained sufficient conditions for competitive exclusion among microorganisms. In [34], Li et al. derived conditions of mean persistence and extinction for the population and showed that the species may survive when the disturbance is small enough.
Some researchers have taken into account both delay and stochastic phenomena in turbidostat for microbial culture [35,36]. However, for all we know, there are few works of literature considering the stochastic turbidostat model with piecewise constant delayed feedback control. In our work, we delve into the dynamical behaviors of the species in the turbidostat with both piecewise constant delayed feedback control and environmental random fluctuation. The rest of this paper is furnished as follows: In Section 2, we put forward the stochastic turbidostat model with a controllable dilution rate, where feedback depends on piecewise constant delayed measurements of the substrate level. The existence and uniqueness of the global positive solution of the model are presented in Section 3. We probe into the extinction and persistence of the species separately in Section 4. Section 5 deals with the stochastic asymptotical stability of the washout equilibrium and the asymptotic behavior of the stochastic delayed model around the interior equilibrium of the corresponding deterministic delayed model. Section 6 offers some numerical examples. The meanings of our results are briefly discussed in the final section.
2.
The model
Gonzalo [19] considered a turbidostat model with discrete delay τ>0 on the output:
where S(t) and x(t) depict the substance and the microbial species levels at time t, respectively. sin>0 represents the input concentration of the substrate. τ is the delay of the optical sensor in the measurement of the concentration of the fluid. In the turbidostat, the concentration of the limiting nutrient in the container possesses a feedback control effect on the input rate of the fresh nutrient. Therefore, the input flow rate D(S(t−τ))>0 can be manipulated and depends on the substrate level at time t−τ. The function μ:R+↦R+ stands for the uptake rate at which nutrient is absorbed by the species and is assumed to be a continuously differentiable function.
The measurements of the concentration of the fluid in [19] are assumed to be continuous. However, given that the sample data provided by online devices are discrete, we consider the following piecewise-linear delay. Fix any two positive numbers ε1,ε2>ε1 such that a sequence of real numbers {ti} satisfies 0<ε1≤ti+1−ti≤ε2 for every i∈N∪{0}, where t0=0 and N={1,2,…}. Given an arbitrary constant τf≥0, we give the following function τ(t):
Employing the above definition, it follows that t−τ(t)=t−(τf+t−tj)=tj−τf for every j≥0 and t∈[tj+τf,tj+1+τf), which means t−τ(t) is piecewise constant. In the particular case of τf=0, t−τ(t)=tj for any t∈[tj,tj+1),j≥0. In addition, for every t≥0, one obtains 0≤τ(t)≤τM, where τM=2τf+ε2. In this case, the measurement available S(t−τ) in model (2.1) can be rewritten as S(t−τ(t)).
Microorganisms consume nutrients for growth, which leads to a decrease in nutrients in the turbidostat. We adopt the function of the form
to describe the consumption, where m is the maximal uptake rate. The derivative of f(S(t)) satisfies f′(S(t))>0, and f1(S(t)) is a bounded continuous function on any finite interval. The presence of ambient noise makes the arguments contained in model (2.1) always swing near some average values rather than reaching fixed values over the time evolution. As one of the pivotal arguments in the turbidostat model, the maximum uptake rate of the organism is more susceptible to environmental noise [26,27,31,32]. Under the circumstances, randomness can be introduced into the maximal uptake rate m such that m→m+σB(t), where the B(t)∈(Ω,F,Ft≥0,P) applied to imitate the stochastic effect is standard Brownian motion, and σ reflects the intensity of the white noise. In consequence, we use m+σB(t) instead of m in the deterministic model (2.1) to obtain the following stochastic differential equation model with piecewise constant delayed measurements of the substrate level:
with initial value conditions
The only control variable, the dilution rate D(S(t−τ(t))), can be defined by D(S(t−τ(t)))=h(S∗−S(t−τ(t))) so that the nutrient concentration can approach S∗ with an appropriate feedback control law, where S∗ is the desired substrate level. Suppose that the function h fulfills the following conditions:
(A1) The continuous differentiable function h:R↦R+ is positive, bounded, increasing and satisfies mf(S∗)=h(0).
(A2) There is a unique root S∗∈(0,sin) such that the equation mf(S)−h(S∗−S)=0 holds.
Quite evidently, this type of feedback control is set up so that the corresponding deterministic delayed model of model (2.2) possesses a washout equilibrium E0=(sin,0) and a unique positive equilibrium E∗=(S∗,x∗), where x∗=sin−S∗. The positive equilibrium point can be set in advance, which means that we can control and achieve our expectations by imposing some conditions. Hence, the control problem we consider is to find sufficient conditions for the persistence of the species, even to make the concentration of the species close to sin−S∗ under the influence of delayed measurements and random perturbation. These questions are to be answered next.
3.
Existence and uniqueness of the positive solution
In consideration of ecological and mathematical significance, whether there exists a global unique positive solution is our primary concern. For this purpose, it is generally required that the arguments of (2.2) fulfill the local Lipschitz condition and the linear growth condition [37]. Whereas the parameters of model (2.2) do not satisfy the linear growth condition, the solutions may explode in a limited time. In this section, we analyze the existence of the unique global positive solution of model (2.2).
Theorem 3.1. For any initial value (2.3), model (2.2) admits a unique global solution (S(t),x(t)) on t≥−τM, and the solution will remain in R2+ with probability one; that is, (S(t),x(t))∈R2+ for all t≥−τM almost surely (a.s.).
Proof. In the light of approaches in [37], model (2.2) possesses a unique local positive solution (S(t),x(t)) on t∈[−τM,ςe), where ςe is the blow up time, which means the trajectories may diverge to infinity as t goes to the finite time ςe. In order to get globality of the solution, we only need to demonstrate ςe=∞ a.s.
Let z(t)=sin−S(t)−x(t). Then,
whose solution is expressed as
which results in S(t)+x(t)≤max{φ1(0)+φ2(0),2sin−φ1(0)−φ2(0)}=M for all t<ς0, where
It is clear that ς0≤ςe a.s. In order to get ςe=∞, we only need to show that ς0=∞ a.s. Now, we select ϵ0>0 such that φ1(ϑ)>ϵ0,φ2(ϑ)>ϵ0. For 0<ϵ≤ϵ0, define the following stopping time:
The empty set is generally denoted as ∅. Let inf∅=∞ all through this paper. There is no doubt that ςϵ is increasing as ϵ approaches 0. Therefore, ς0=limϵ→0ςϵ a.s. Next, the proof can be accomplished if we get ς0=∞ a.s.
For proof by contradiction, assume that there exists a pair of positive numbers δ∈(0,1) and T∈[ti,ti+1) such that P{ς0≤T}>δ, where i∈N∪{0}. Thus, there is a constant ϵ1∈(0,ϵ0) such that P{ςϵ≤T}>δ for any 0<ϵ≤ϵ1.
Additionally, define a C2-function V:R2+→R+ by
Obviously, V(S,x) is positive definite for all S,x>0. Using Itô's formula to model (2.2), one derives
where
where ˜M is the upper bound of the function f1(S) on [0,M]. Accordingly, we obtain
Integrating and taking the expectation of both sides of (3.3) yield
and
Superimposing the above inequations, we can get
For any 0<ϵ≤ϵ1, we set Ωϵ={ςϵ≤T}, and then P(Ωϵ)>δ. On the grounds of the definition of the blow up time, there exists S(ςϵ,ω), or x(ςϵ,ω) equals ϵ for every ω∈Ωϵ, and then
Via (3.4), one infers
This leads to a contradiction as we let ϵ→0,
So, we get ς0=∞ a.s. The proof is completed.
Define
It suffices from (3.2) to display that Λ is the positive invariant set of model (2.2). Throughout this paper, we always assume that the starting value (φ1,φ2) is constrained in Λ.
4.
Extinction and permanence
This section provides sufficient conditions for strong stochastic persistence and extinction of the microorganism in the turbidostat. For the sake of convenience, denote
Then, we define
and
4.1. Extinction
The following theorem is about the extinction of the microorganism.
Theorem 4.1. The biomass x(t) will die out exponentially, namely, limt→∞x(t)=0 a.s., provided
(i) h(S∗−sin)<mf(sin)≤2h(S∗−sin) and λ1<0 (i.e. σ>√2(mf(sin)−h(S∗−sin))f(sin)); or
(ii) mf(sin)>2h(S∗−sin) and σ>m√2h(S∗−sin).
Proof. In order to discuss the extinction of the biomass, applying Itˆo's formula to W1(x)=lnx yields
By integrating on the both sides of the above inequality from 0 to t, we can obtain
which further results in
where ¯W1 is an upper bound of the function LW1=f(S)(m−12σ2f(S))−h(S∗−sin), and ψ(t)=1t[lnx(0)+∫t0σf(S(r))dB(r)]. According to the strong law of large numbers for local martingale, we get limt→∞ψ(t)=0 a.s., which means lim supt→∞lnx(t)t≤¯W1 a.s. To get the extinction of x(t), we need to show ¯W1<0.
Next, we consider two cases by treating the function f(S)(m−12σ2f(S)) as a quadratic function about f(S). Case (i): On the premise of h(S∗−sin)<mf(sin)≤2h(S∗−sin), when σ2≤mf(sin), the function f(S)(m−12σ2f(S)) is increasing for S∈[0,sin]. Then, we have LW1≤mf(sin)−h(S∗−sin)−12σ2f2(sin)=λ1<0, which means ¯W1<0 if mf(sin)−h(S∗−sin)<σ2≤mf(sin). For σ2>mf(sin), the function f(S)(m−12σ2f(S)) reaches the maximum value when f(S)=mσ2, and then we have LW1≤m22σ2−h(S∗−sin)<mf(sin)2−h(S∗−sin)<0. To sum up, if h(S∗−sin)<mf(sin)≤2h(S∗−sin) and λ1<0, the biomass x(t) will die out. Case (ii): In the case of mf(sin)>2h(S∗−sin), one obtains LW1≤mf(sin)−h(S∗−sin)−12σ2f2(sin) when σ2≤mf(sin); nevertheless, at this point mf(sin)−h(S∗−sin)−12σ2f2(sin)>0. On the contrary, when σ2>mf(sin), we have LW1≤m22σ2−h(S∗−sin)<0 owing to σ>m√2h(S∗−sin)>√mf(sin). Hence, if mf(sin)>2h(S∗−sin) and σ>m√2h(S∗−sin), the microorganism x(t) will be extinct. The proof is completed.
In line with Theorem 4.1, it can be validated that stochasticity will result in the disappearance of the species of model (2.2) when the magnitude of stochasticity is large enough. In contrast, how does the biomass change in the turbidostat in the case of small intensity of disturbance? In the following, we discuss the stochastic strong permanence of the microorganism.
4.2. Stochastic strong permanence
This subsection is intended for stochastic strong permanence of the biomass of model (2.2) under the influence of small noise. To figure out the permanence of the microorganism for model (2.2), we introduce the equation
with initial value φ2. Let us first prepare the following useful lemma.
Lemma 4.1. Denote ∂Λ:={(S,x)∈Λ:x=0}. Then, there is a T>0 such that
for any (φ1,φ2)∈∂Λ, where ˜F(S(t))=−12σ2f2(S)+mf(S)−h(S∗).
Proof. If φ2=0, then x(t)=0 for all t≥−τM, and the first equation of model (2.2) turns into
Apparently, the solution of (4.3) converges to sin. Consequently,
for every (φ1,φ2)∈∂Λ. Then, we can find a positive number T fulfilling (4.2).
Theorem 4.2. If λ2>0, then X(t) of model (4.1) is strongly stochastically permanent, that is, for any γ>0, there exists a α1>0 such that
Proof. Introduce a Lyapunov function Wθ=Xθ, where θ∈R is a constant that needs to be specified. Simple calculations for Wθ on the grounds of Itˆo's formula give
Define Oθ=sup(S,X)∈Λ{θ[mf(S)−h(S∗)+θ−12σ2f2(S)]}. Then, for any starting value φ2, we get from (4.4) that LWθ≤OθXθ. By applying Itˆo's formula and taking expectations, we obtain
for any t≥0, φ2>0. On the basis of Itˆo's formula, (4.1) becomes
Integrating both sides of the above equality from 0 to t yields
Define W2(t)=lnX(0)−lnX(t). From (4.6), one has
According to Lemma 4.1 and Feller property, it then follows from the above equation that we can find a sufficiently small δ1>0 such that
for φ2<δ1. In the light of (4.5), for any fixed t≥0, we get
It then suffices from [38, Lemma 2.2] to show that
where ˜O is a constant which is dependent on T, O−1 and O1. If θ is sufficiently small and fulfills ˜Oθ2≤λ2θT4, we get from (4.7) that
which further implies
for φ2<δ1, where c1=exp(−λ2θT4). Noting (4.5), we obtain
for any φ2>δ1. This, combined with (4.8), means that
for any φ2. Considering that the Markov property, the following inequation
holds for any φ2. By the method of recursion, we obtain
This together with (4.5) results in
Consequently,
as n→∞. The theorem easily follows from an application of Markov's inequality to the above inequation.
Combining Theorem 4.2 and the comparison principle, we conclude the following theorem about the permanence of the microorganism x(t) of model (2.2).
Theorem 4.3. The microorganism x(t) is strongly stochastically permanent provided λ2>0.
In the contents above, the microorganism may die out in the case that the magnitude of stochasticity is sufficiently large, and the microorganism will be strongly stochastically permanent when the magnitude is small enough. Nevertheless, what we're more curious about is whether the microorganism fluctuates near the wanted biomass concentration sin−S∗ under the combined action of delayed measurements and random disturbance. Therefore, in the rest of this article, we will make an investigation on the asymptotic behaviors of model (2.2) around the steady states of the corresponding deterministic delayed model.
5.
Analysis of asymptotic behaviors
In this section, we look into the asymptotic stability of the washout equilibrium and asymptotic behavior of model (2.2) around the interior equilibrium E∗ of its corresponding deterministic delayed model.
Theorem 5.1. Assume that λ1<0; then, the washout equilibrium E0=(sin,0) is asymptotically stable in probability.
Proof. Set the Lyapunov function
where p∈(0,1) will be determined later. Applying Itˆo's formula to (5.1) yields
Noticing that λ1<0, we can choose sufficiently small positive numbers p,ν such that −2h(S∗−S(t−τ(t)))≤−2h(S∗−sin)≤p(λ1+ν)<0. Let Dδ1:=(sin−δ1,sin]×[0,δ1) for δ1∈(0,sin). For any (S,x)∈Dδ1, x has a tendency to 0 as δ1 approaches to 0+, which combined with the continuity of F(S,x) leads to
If p and δ1 are small enough, the following inequation holds:
for any (S,x)∈Dδ1, where 0<ν1,ν2<ν and ν=ν1+ν2. Hence, for any (S,x)∈Dδ1, we obtain
By virtue of [38, Theorem 3.1], we deduce that the microorganism-free equilibrium (sin,0) is asymptotically stable in probability.
Our control target is to make the random paths close to E∗ with a delayed state feedback of the form h(S∗−S(t−τ(t))). Whereas, E∗ is no longer the equilibrium of stochastic delayed model (2.2), the asymptotic behavior of model (2.2) around the steady state E∗ will be discussed.
For convenience of the analysis below, we introduce some notations:
Theorem 5.2. Suppose that λ2>0 and
Then, there exists T2>2τM such that
where
Proof. Noting that λ2>0, by Theorem 4.3, we can choose a pair of positive numbers T1 and α2<min{sin−S∗,α1} satisfying x(t)≥α2 a.s. for all t≥T1. Thus,
It follows that Sa∈(S∗,sin) owing to 0<α2<sin−S∗<sin for t≥T1. On account of the mean value theorem, we can get that
where ξ∈[0,S∗−S(t−τ(t))] or [S∗−S(t−τ(t)),0]. By the inequality 2ab≤a2+b2, we infer
for all t≥T1+2τM.
We define a Lyapunov functional U1 as follows:
which is nonnegative and belongs to class C1 over [0,sin). It follows from (5.3) that its derivative along all trajectories of model (2.2) for any t≥T1+2τM satisfies
where η∈[S∗,S(t)] or [S(t),S∗].
Construct a differentiable function U2, whose specific mathematical expression is
A preliminary calculation gives, for any t≥T1+2τM,
Taking the integral from T2>T1+2τM to t and then taking the expectation, we have
On the basis of the Burkholder-Davis-Gundy inequality, we get
It suffices from Fubini's Theorem to obtain
Hence,
Taking the superior limit of both sides of (5.5) leads to
This completes the proof of Theorem 5.2.
Remark 5.1. In view of Theorem 5.2, when the magnitude of stochasticity and the delay of measurements are small, the expected time average of the distance between the equilibrium E∗ and the stochastic solution (S(t),x(t)) will finally bind in a narrow area. That is, the solutions of stochastic delayed model (2.2) will be swinging around a point near the steady state E∗ of the corresponding deterministic delayed model when the magnitude of stochasticity and the delay of measurements are small, and it shows the biologically intuitive fact that the smaller the magnitude of stochasticity and the delay of measurements are, the closerS(t) will be to S∗. Meanwhile, x(t) will be closer to x∗.
6.
Numerical simulations
In this section, we carry out some numerical simulations to support our theoretical findings. In order to be more consistent with the actual situation, we fit model (2.2) to the experimental data of Espie and Macchietto [39], which is yeast culture in a fermenter by continuously adding the limiting nutrient containing glucose. Model (2.2) is obtained by substitution of variables, and then the original form of (2.2) is as follows:
To clarify our results, we consider the growth function f(S) in the form of the classical Monod function [40,41]
In the case of τf=0,tj=τMj, we establish the following feedback control law:
where ⌊A⌋=max{j∈{0,1,2,…}:j≤A} is the floor function. Obviously, τM⌊tτM⌋=τMj when t∈[τMj,τM(j+1)), which means τM⌊tτM⌋ is an increasing piecewise constant function. h(S∗−S(t−τ(t))) is a decreasing function of S due to the hyperbolic tangent function.
In order to estimate the parameter values of the corresponding deterministic delayed model of model (6.1), we initially use the least squares method to identify the parameters that best suit the deterministic model, aiming to minimize the sum of the squared differences between the output of the deterministic model and the laboratory data of S and x in [39]. Denote the collection of parameters Π=(l,ν,S∗,τM,sin,β,m,α)T. Let h(Z(t),Π)=(h(S∗−S(t−τ(t)))(sin−S(t))−mαf(S(t))x(t),x(t)(mf(S(t))−h(S∗−S(t−τ(t)))))T, where Z(t)=(S(t),x(t))T. For the given set of experimental data points of [37] (t1,Y1),(t2,Y2),…,(tn,Yn), the objective function of minimizing the squared sum of errors is
In order to achieve a satisfactory agreement between the output of the stochastic model and the experimental data, we search for an appropriate noise intensity of the stochastic model after estimating the parameters for the deterministic model. Ξ1, the sum of the squares of the distances of the experimental data points from the mean of their ordinate values (Ξ2), and the corresponding r-squared value [42] for experimental data and stochastic simulation data are calculated in the search for an appropriate noise strength. The statistical measure fitting r2 can be calculated using the relation r2=1−Ξ1Ξ2, which quantifies goodness of fit. We investigated 100 possible random noise intensity values σ∈[0,1], and the stochastic model was then simulated 100 times for each of these 100 possible values. The correlation coefficient r2 between the experimental data and the average output of the 100 stochastic simulations was calculated. Finally, the best estimated parameter values are reported in Table 1. Using these parameter values, we plotted the substrate and the species (see Figure 1c, d) obtained from the average of 100 simulations of the stochastic model (6.1). The blue dots represent the experimental data, the cyan solid lines are the trajectories after 100 simulations of the stochastic model and the green lines are the mean values among these trajectories (see Figure 1a, b). By consulting some information [43,44], we learned that the response time of the optical sensor is very short, only about a few seconds (1s≈2.778×10−4h). Our estimated delay parameter τM is 3×10−4, which is in line with the actual situation.
In the case of the estimated parameter values, the positive equilibrium of the corresponding deterministic model of model (6.1) is E∗=(1.425,16.7348). After a simple calculation, we obtain λ2>0 and τM=3×10−4<2(ρl+mϖl)ρu(1+x∗+m+mx∗+h2(S∗)+mf2(sin))=3.2982×10−4, which satisfies the conditions in Theorem 5.2. As a result, the biomass is strongly stochastically permanent, and the average distance between the positive equilibrium E∗ and (S(t),x(t)) ultimately becomes very small, i.e.,
Through numerical simulations, we know that with the value of the delay parameter τM gradually increasing and crossing the value τ0M≈2.3, a periodic solution of the corresponding deterministic model will bifurcate from the positive equilibrium E∗, which means positive equilibrium E∗ will become unstable. As is shown in Figure 2, the solution of nutrient S(t) and microorganism x(t) of model (6.1) will fluctuate around the stable periodic solutions of the deterministic model when τM=3>τ0M. By comparing Figure 1d and Figure 2b, the delay of measurements may affect the extent to which the microbial concentration deviates from the desired concentration when the magnitude of stochasticity is small.
To reveal the effect of parameters σ on the dynamics of model (6.1), we perform some simulations with the estimated parameter values except to σ. Set σ=0.4, and we have h(S∗−sin)=0.261<mf(sin)=0.3381 and λ1=−0.002<0, which is consistent with conditions in Theorem 4.1. In this case, microorganisms eventually die out exponentially, even if τM increases to 3 (see Figure 3b, d). It follows that relatively strong disturbances in the environment exert a destructive influence on the survival of the population, leading to the extinction of the population.
7.
Conclusions
This paper puts forward and explores a stochastic turbidostat model (2.2) with feedback control on its output, in which the feedback merely relies on the substrate level with piecewise constant delayed measurements. Such a stochastic model well depicts the effects of laboratory equipment and stochasticity on microbial culture. For the sake of governing the dynamics of the microorganism for model (2.2), we explore sufficient conditions of stochastic strong permanence and extinction of the species. In addition, the stochastic asymptotical stability of the washout equilibrium and the asymptotic behavior of model (2.2) around E∗ are discussed. More particular, the mean distance between E∗ and stochastic solution (S(t),x(t)) is dependent on the magnitude of stochasticity and delay of measurements, and the random paths are closer to E∗ the less the stochastic disturbance intensity and delay of measurements are. In summary, the magnitude of stochasticity could determine the survival of microorganisms, while the delay of measurements may affect the extent to which the microbial concentration deviates from the desired concentration when the magnitude of stochasticity is small. In microbial culture, the earlier the stabilization is, the better to improve efficiency. Hence, the smaller the delay and noise intensity are, the easier it is to achieve the goal, which means sophisticated laboratory instruments are more instrumental in achieving our control objectives.
As a result of these findings, we clarify that stochasticity and delayed measurements play an important role in a nutrient-microorganism model. Our research contributes to a better understanding of microbial culture. On the basis of the work, it is of great significance to study the effects of environmental noise and delayed measurements on the dynamics of the multi-nutrient or multi-microorganism models. We leave these as future work.
Acknowledgments
Research is supported by the National Natural Science Foundation of China (No. 12071293).
Conflict of interest
The authors declare there is no conflict of interest.









 DownLoad:
DownLoad: