Nonlinear autoregressive sieve bootstrap based on extreme learning machines

Michele La Rocca; Cira Perna; Michele La Rocca; Cira Perna

doi:10.3934/mbe.2020033

Mathematical Biosciences and Engineering

2020, Volume 17, Issue 1: 636-653. doi: 10.3934/mbe.2020033

Previous Article Next Article

Research article Special Issues

Nonlinear autoregressive sieve bootstrap based on extreme learning machines

Michele La Rocca ,
Cira Perna ^,

Department of Economics and Statistics, University of Salerno, via Giovanni Paolo II, Fisciano 84084, Italy

Received: 29 May 2019 Accepted: 09 October 2019 Published: 22 October 2019

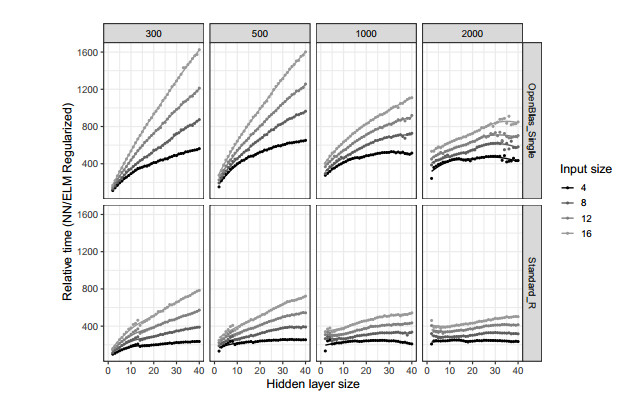
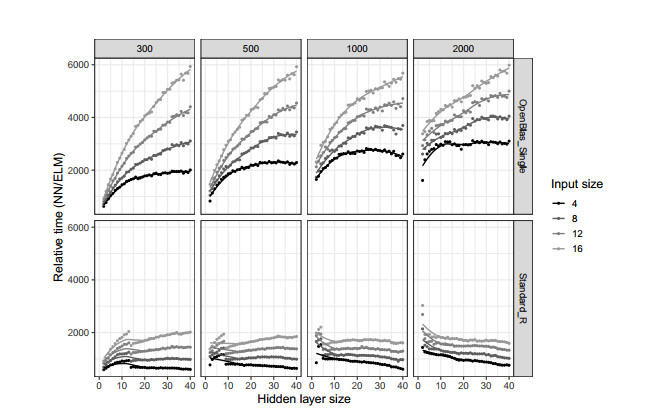
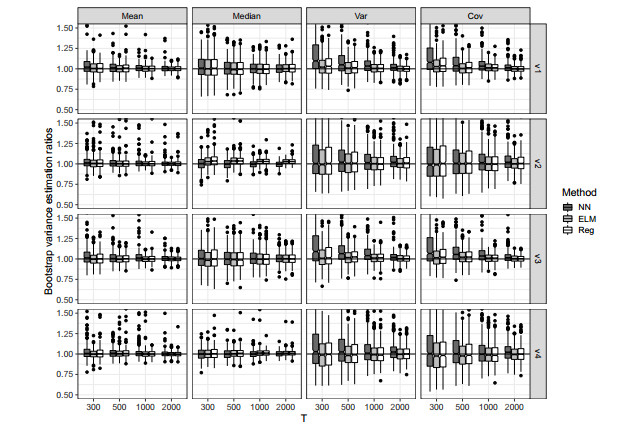
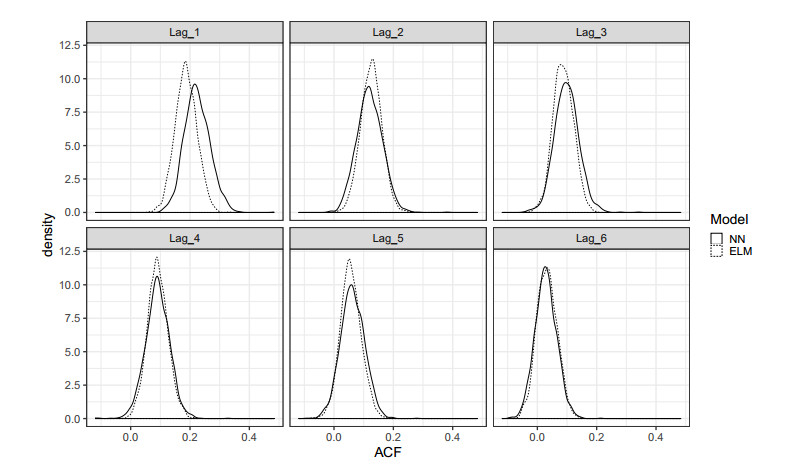
The aim of the paper is to propose and discuss a sieve bootstrap scheme based on Extreme Learning Machines for non linear time series. The procedure is fully nonparametric in its spirit and retains the conceptual simplicity of the residual bootstrap. Using Extreme Learning Machines in the resampling scheme can dramatically reduce the computational burden of the bootstrap procedure, with performances comparable to the NN-Sieve bootstrap and computing time similar to the ARSieve bootstrap. A Monte Carlo simulation experiment has been implemented, in order to evaluate the performance of the proposed procedure and to compare it with the NN-Sieve bootstrap. The distributions of the bootstrap variance estimators appear to be consistent, delivering good results both in terms of accuracy and bias, for either linear and nonlinear statistics (such as the mean and the median) and smooth functions of means (such as the variance and the covariance).

Keywords:

Citation: Michele La Rocca, Cira Perna. Nonlinear autoregressive sieve bootstrap based on extreme learning machines[J]. Mathematical Biosciences and Engineering, 2020, 17(1): 636-653. doi: 10.3934/mbe.2020033

Related Papers:

[1]	Shiqiang Feng, Dapeng Gao . Existence of traveling wave solutions for a delayed nonlocal dispersal SIR epidemic model with the critical wave speed. Mathematical Biosciences and Engineering, 2021, 18(6): 9357-9380. doi: 10.3934/mbe.2021460
[2]	Zhihong Zhao, Yan Li, Zhaosheng Feng . Traveling wave phenomena in a nonlocal dispersal predator-prey system with the Beddington-DeAngelis functional response and harvesting. Mathematical Biosciences and Engineering, 2021, 18(2): 1629-1652. doi: 10.3934/mbe.2021084
[3]	Jian Fang, Na Li, Chenhe Xu . A nonlocal population model for the invasion of Canada goldenrod. Mathematical Biosciences and Engineering, 2022, 19(10): 9915-9937. doi: 10.3934/mbe.2022462
[4]	Guo Lin, Shuxia Pan, Xiang-Ping Yan . Spreading speeds of epidemic models with nonlocal delays. Mathematical Biosciences and Engineering, 2019, 16(6): 7562-7588. doi: 10.3934/mbe.2019380
[5]	Xixia Ma, Rongsong Liu, Liming Cai . Stability of traveling wave solutions for a nonlocal Lotka-Volterra model. Mathematical Biosciences and Engineering, 2024, 21(1): 444-473. doi: 10.3934/mbe.2024020
[6]	Xiao-Min Huang, Xiang-ShengWang . Traveling waves of di usive disease models with time delay and degeneracy. Mathematical Biosciences and Engineering, 2019, 16(4): 2391-2410. doi: 10.3934/mbe.2019120
[7]	Qiaoling Chen, Fengquan Li, Sanyi Tang, Feng Wang . Free boundary problem for a nonlocal time-periodic diffusive competition model. Mathematical Biosciences and Engineering, 2023, 20(9): 16471-16505. doi: 10.3934/mbe.2023735
[8]	Wenhao Chen, Guo Lin, Shuxia Pan . Propagation dynamics in an SIRS model with general incidence functions. Mathematical Biosciences and Engineering, 2023, 20(4): 6751-6775. doi: 10.3934/mbe.2023291
[9]	Max-Olivier Hongler, Roger Filliger, Olivier Gallay . Local versus nonlocal barycentric interactions in 1D agent dynamics. Mathematical Biosciences and Engineering, 2014, 11(2): 303-315. doi: 10.3934/mbe.2014.11.303
[10]	Tong Li, Zhi-An Wang . Traveling wave solutions of a singular Keller-Segel system with logistic source. Mathematical Biosciences and Engineering, 2022, 19(8): 8107-8131. doi: 10.3934/mbe.2022379

Abstract

1. Introduction

As one of the most basic models in modeling infectious diseases, the SIR epidemiological model was introduced by Kermack and McKendrick ^[1] in 1927. Since then, a lot of differential equations have been studied as models for the spread of infectious diseases. Considering a continuous vaccination strategy, let $V$ be a new group of vaccinated individuals, Liu et al. ^[2] formulated the following system of ordinary differential equations:

$\begin{equation} \label{PrModel1}\left\{ \begin{array}{l} \displaystyle \frac{\rm{d} S(t)}{\rm{d} t} = \Lambda - \beta_1 S(t)I(t) - \alpha S(t) - \mu_1 S(t), \\ \displaystyle \frac{\rm{d} V(t)}{\rm{d} t} = \alpha S(t) - \beta_2 V(t)I(t) - (\gamma_1 + \mu_1) V(x, t), \\ \displaystyle \frac{\rm{d} I(t)}{\rm{d} t} = \beta_1 S(t)I(t) + \beta_2 V(t)I(t) - \gamma I(x, t) - \mu_3 I(x, t), \\ \displaystyle \frac{\rm{d} R(t)}{\rm{d} t} = \gamma_1V(t) + \gamma I(t)- \mu_1 R(t), \end{array}\right. \end{equation}$

(1.1)

where $S(t), \ V(t), \ I(t)$ and $R(t)$ denote the densities of susceptible, vaccinated, infective and removed individuals at time $t$ , respectively. $\Lambda$ denote the recruitment rate of susceptible individuals, $\mu_1$ denote the natural death rate. $\beta_1$ is the rate of disease transmission between susceptible and infectious individuals, and $\beta_2$ is the rate of disease transmission between vaccinated and infected individuals. $\gamma$ denote the recovery rate, $\alpha$ is the vaccination rate and $\gamma_1$ is the rate at which a vaccinated individual obtains immunity. In ^[2], the authors shown that the global dynamics of model (1.1) is completely determined by the basic reproduction number: that is, if the number is less than unity, then the disease-free equilibrium is globally asymptotically stable, while if the number is greater than unity, then a positive endemic equilibrium exists and it is globally asymptotically stable. Moreover, it was observed in Liu et al. ^[2] that vaccination has an effect of decreasing the basic reproduction number. By using the classical method of Lyapunov and graph-theoretic approach, Kuniya ^[3] studied the global stability of a multi-group SVIR epidemic model. Xu et.al ^[4] formulated a multi-group epidemic model with distributed delay and vaccination age, the authors established the global stability of the model, furthermore, the stochastic perturbation of the model is studied and it is proved that the endemic equilibrium of the stochastic model is stochastically asymptotically stable in the large under certain conditions. In ^[5,6,7], the global stability of different SVIR models with age structure are investigated.

On the other hand, in order to understand the geographic spread of infectious disease, the spatial effect would give insights into disease spread and control. Due to this fact, many literatures have studied the spatial effects on epidemics by using reaction-diffusion equations (see, for instance, ^{[8,9,10,11,12,13,14,15,16,17]} and the references therein). In the study of reaction-diffusion models, the Laplacian operator describes the random diffusion of each individual, but it can not describe the long range diffusion. Therefore, a nonlocal dispersal term has been established, which is by a convolution operator:

$\begin{equation}\label{J} J*\phi(x)-\phi(x) = \int_{{\rm IR}}J(x-y)\phi(y)\rm{d} y - \phi(x), \end{equation}$

(1.2)

where $\phi(x)$ denote the densities of individuals at position $x$ , $J(x-y)$ is interpreted as the probability of jumping from position $y$ to position $x$ , the convolution $\int_{{\rm IR}}J(x-y)\phi(y)\rm{d} y$ is the rate at which individuals arrive at position $x$ from all other positions, while $-\int_{{\rm IR}}J(x-y)\phi(x)\rm{d} y = -\phi(x)$ is the rate at which they leave position $x$ to reach any other position. Problems involving such operators are called nonlocal diffusion problems and have appeared in various references ^{[18,19,20,21,22,23,24,25,26,27,28,29,30,31]}.

Recently, Li et al. ^[23] proposed a nonlocal dispersal SIR model with delay:

$\begin{equation} \label{PrModel2}\left\{ \begin{array}{l} \displaystyle \frac{\partial S(x, t)}{\partial t} = d_1(J*S(x, t)-S(x, t)) +\Lambda - \frac{\beta_1 S(x, t)I(x, t-\tau)}{1+\theta I(x, t-\tau)} - \mu_1 S(x, t), \\ \displaystyle \frac{\partial I(x, t)}{\partial t} = d_2(J*I(x, t)-I(x, t)) + \frac{\beta_1 S(x, t)I(x, t-\tau)}{1+\theta I(x, t-\tau)} - \gamma I(x, t) - \mu_3 I(x, t), \\ \displaystyle \frac{\partial R(x, t)}{\partial t} = d_3(J*R(x, t)-R(x, t)) + \gamma I(x, t) - \mu_1 R(x, t), \end{array}\right. \end{equation}$

(1.3)

where $S(x, t), \ I(x, t)$ and $R(x, t)$ denote the densities of susceptible, infective and removed individuals at position $x$ and time $t$ , respectively. $\theta$ measures the saturation level. $d_i(i = 1, 2, 3)$ describes the spatial motility of each compartments. The biological meaning of other parameters are the same as in model (1.1). The authors find that there exists traveling wave solution if the basic reproduction number $\Re_0>1$ and the wave speed $c\geq c^*$ , where $c^*$ is the minimal wave speed. They also obtain the nonexistence of traveling wave solution for $\Re_0>1$ and any $0<c<c^*$ or $\Re_0<1$ .

Motivated by ^[2] and ^[23], in this paper, we consider a nonlocal dispersal epidemic model with vaccination and delay. Precisely, we study the following model.

$\begin{equation} \label{Model1}\left\{ \begin{array}{l} \displaystyle \frac{\partial S(x, t)}{\partial t} = d_1(J*S(x, t)-S(x, t)) +\Lambda - \beta_1 S(x, t)I(x, t-\tau) - \alpha S(x, t) - \mu_1 S(x, t), \\ \displaystyle \frac{\partial V(x, t)}{\partial t} = d_2(J*V(x, t)-V(x, t)) - \beta_2 V(x, t)I(x, t-\tau) + \alpha S(x, t) - (\gamma_1 + \mu_1) V(x, t), \\ \displaystyle \frac{\partial I(x, t)}{\partial t} = d_3(J*I(x, t)-I(x, t)) + \beta_1 S(x, t)I(x, t-\tau) + \beta_2 V(x, t)I(x, t-\tau) - \gamma I(x, t) - \mu_3 I(x, t), \\ \displaystyle \frac{\partial R(x, t)}{\partial t} = d_4(J*R(x, t)-R(x, t)) + \beta_1 S(x, t)I(x, t-\tau) + \gamma_1 V(x, t) + \gamma I(x, t) - \mu_4 R(x, t), \end{array}\right. \end{equation}$

(1.4)

where $S(x, t), \ V(x, t), \ I(x, t)$ and $R(x, t)$ denote the densities of susceptible, vaccinated, infective and removed individuals at position $x$ and time $t$ , respectively. $d_i(i = 1, 2, 3, 4)$ describes the spatial motility of each compartments. The biological meaning of other parameters are the same as in model (1.1). $J$ is the standard convolution operator satisfying the following assumptions.

Assumption 1.1. ^[23,24] The kernel function $J$ satisfies

(J1) $J\in C^1({\rm IR}), \ J(x)\geq0, \ \ J(x) = J(-x), \ \ \int_{{\rm IR}}J(x)\rm{d} x = 1$ and $J$ is compactly supported.

(J2) There exists a constant $\lambda_M\in(0, +\infty)$ such that

$\int_{{\rm IR}}J(x)e^{-\lambda x}{\rm d} x \lt +\infty, \ \ for\ \ any\ \ \lambda\in[0, \lambda_{\rm M})$

and

$\lim\limits_{\lambda\rightarrow \lambda_M-0}\int_{{\rm IR}}J(x)e^{-\lambda x}\rm{d} x\rightarrow+\infty.$

The organization of this paper is as follows. In section 2, we proved the existence of traveling wave solutions of (1.4) for $c>c^*$ by applying Schauder's fixed point theorem and Lyapunov method. In section 3, we show that the existence of traveling wave solutions of (1.4) for $c = c^*$ . Furthermore, we investigate the nonexistence of traveling wave solutions under some conditions in section 4. At last, there is a brief discussion.

2. Existence of traveling wave solutions for $c>c^*$

In this section, we study the existence of traveling wave solutions of system (1.4). Since we have assumed that the recovered have gained permanent immunity and $R(x, t)$ is decoupled from other equations, we indeed need to study the following subsystem of (1.4)

$\begin{equation} \label{Model}\left\{ \begin{array}{l} \displaystyle \frac{\partial S(x, t)}{\partial t} = d_1(J*S(x, t)-S(x, t)) +\Lambda - \beta_1 S(x, t)I(x, t-\tau) - \alpha S(x, t) - \mu_1 S(x, t), \\ \displaystyle \frac{\partial V(x, t)}{\partial t} = d_2(J*V(x, t)-V(x, t)) - \beta_2 V(x, t)I(x, t-\tau) + \alpha S(x, t) - \mu_2 V(x, t), \\ \displaystyle \frac{\partial I(x, t)}{\partial t} = d_3(J*I(x, t)-I(x, t)) + (\beta_1 S(x, t) + \beta_2 V(x, t))I(x, t-\tau) - \gamma I(x, t) - \mu_3 I(x, t). \end{array}\right. \end{equation}$

(2.1)

where $\mu_2 = \gamma_1 + \mu_1$ . Obviously, system (2.1) always has a disease-free equilibrium $E_0 = (S_0, V_0, 0) = \left(\frac{\Lambda}{\mu_1+\alpha}, \frac{\Lambda\alpha}{\mu_2(\mu_1+\alpha)}, 0\right)$ . Denote the basic reproduction number as following:

$\begin{align}\label{R_0} \Re_{0} = \frac{\beta_1S_0+\beta_2V_0}{\mu_3+\gamma}. \end{align}$

(2.2)

Furthermore, there exists another equilibrium $E^* = (S^*, V^*, I^*)$ satisfying

$\begin{equation} \label{PosiEqu}\left\{ \begin{array}{l} \displaystyle \Lambda - \beta_1 S^*I^* - \alpha S^* - \mu_1 S^* = 0, \\ \displaystyle \beta_2 V^*I^* + \alpha S^* - \mu_2 V^* = 0, \\ \displaystyle (\beta_1 S^* + \beta_2 V^*)I^* - \gamma I^* - \mu_3 I^* = 0. \end{array}\right. \end{equation}$

(2.3)

From [2,Theorem 2.1], system (2.1) has a unique positive equilibrium $E^*$ if $\Re_0>1$ .

Let $\xi = x+ct$ and substituting $\xi$ into system (2.1), then we obtain the wave form equations as

$\begin{equation} \label{TransModel}\left\{ \begin{array}{l} \displaystyle cS'(\xi) = d_1(J*S(\xi)-S(\xi))+\Lambda - \beta_1 S(\xi)I(\xi-c\tau) - \alpha S(\xi) - \mu_1 S(\xi), \\ \displaystyle cV'(\xi) = d_2(J*V(\xi)-V(\xi)) + \alpha S(\xi)- \beta_2 V(\xi)I(\xi-c\tau) - \mu_2 V(\xi), \\ \displaystyle cI'(\xi) = d_3(J*I(\xi)-I(\xi)) + \beta_1 S(\xi)I(\xi-c\tau) + \beta_2 V(\xi)I(\xi-c\tau) - \gamma I(\xi) - \mu_3 I(\xi). \end{array}\right. \end{equation}$

(2.4)

We want to find traveling wave solutions with the following asymptotic boundary conditions:

$\begin{equation}\label{Bound1} \lim\limits_{\xi\rightarrow-\infty}(S(\xi), V(\xi), I(\xi)) = (S_0, V_0, 0) \end{equation}$

(2.5)

and

$\begin{equation} \label{Bound2} \lim\limits_{\xi\rightarrow+\infty}(S(\xi), V(\xi), I(\xi)) = (S^*, V^*, I^*). \end{equation}$

(2.6)

Consider the following linear system of system (2.4) at infection-free equilibrium $(S_0, V_0, 0),$

$\begin{equation} \label{LinearModel} cI'(\xi) = d_3(J*I(\xi)-I(\xi)) + \beta_1S_0I(\xi-c\tau) + \beta_2V_0I(\xi-c\tau)-(\gamma+\mu_3)I(\xi). \end{equation}$

(2.7)

Let $I(\xi) = e^{\lambda \xi}$ , we have

$\begin{align} \Delta(\lambda, c)\triangleq d_3\int_{{\rm IR}}J(x)e^{-\lambda x}\rm{d} x-(d_3+\gamma+\mu_3)-c\lambda+\beta_1S_0e^{-c\tau\lambda}+\beta_2V_0e^{-c\tau\lambda} = 0. \end{align}$

(2.8)

By some calculations, we obtain

$\begin{align*} &\Delta(0, c) = \beta_1S_0+\beta_2V_0-\gamma-\mu_3, \ \ \ \lim\limits_{c\rightarrow+\infty}\Delta(\lambda, c) = -\infty\ \ \textrm{for}\ \ \lambda \gt 0, \\ &\frac{\partial \Delta(\lambda, c)}{\partial\lambda}\bigg|_{(0, c)} = -c-c\tau(\beta_1S_0+\beta_2V_0) \lt 0\ \ \textrm{for}\ \ c \gt 0, \\ &\frac{\partial \Delta(\lambda, c)}{\partial c} = -\lambda-\tau\lambda e^{-c\tau\lambda}(\beta_1S_0+\beta_2V_0) \lt 0\ \ \textrm{for}\ \ \lambda \gt 0, \\ &\frac{\partial^2 \Delta(\lambda, c)}{\partial\lambda^2} = d_3\int_{{\rm IR}}J(x)x^2e^{-\lambda x}\rm{d} x+(c\tau)^2e^{-c\tau\lambda}(\beta_1S_0+\beta_2V_0) \gt 0. \end{align*}$

For any $c\in{\rm IR},$ $\Delta(0, c) = \Re_0 -1,$ gives us $\Delta(0, c)>0$ if $\Re_0>1.$ Then there exist $c^*>0$ and $\lambda^*>0$ such that $\frac{\partial \Delta(\lambda, c)}{\partial\lambda}\big|_{(\lambda^*, c^*)} = 0$ , we have the following lemma.

Lemma 2.1. Let $\Re_{0}>1$ , we have

(ⅰ) If $c = c^*,$ then $\Delta(\lambda, c) = 0$ has two same positive real roots $\lambda^*;$

(ⅱ) If $0<c<c^*,$ then $\Delta(\lambda, c)>0$ for all $\lambda\in(0, \lambda_{c, \tau}),$ where $\lambda_{c, \tau}\in(0, +\infty]$ ;

(ⅲ) If $c>c^*,$ then $\Delta(\lambda, c) = 0$ has two positive real roots $\lambda_1(c),$ $\lambda_2(c).$

Denote $\lambda_c = \lambda_1(c)$ , from Lemma 2.1, we have

$0 \lt \lambda_c \lt \lambda^* \lt \lambda_2(c) \lt \lambda_{c, \tau}.$

For the followings in this section, we always fix $c>c^*$ and $\Re_0>1.$ Define the following functions:

$\begin{equation*} \label{UpLowSolution}\left\{ \begin{array}{l} \displaystyle \overline{S}(\xi) = S_0, \\ \displaystyle \overline{V}(\xi) = V_0, \\ \displaystyle \overline{I}(\xi) = e^{\lambda_c\xi}, \end{array}\right. \ \ \left\{ \begin{array}{l} \displaystyle \underline{S}(\xi) = \max\{S_0-M_1 e^{\varepsilon_1 \xi}, 0\}, \\ \displaystyle \underline{V}(\xi) = \max\{V_0-M_2 e^{\varepsilon_2 \xi}, 0\}, \\ \displaystyle \underline{I}(\xi) = \max\{e^{\lambda_c\xi}(1-M_3e^{\varepsilon_3 \xi}), 0\}, \end{array}\right. \end{equation*}$

where $M_i$ and $\varepsilon_i(i = 1, 2, 3)$ are some positive constants to be determined in the following lemmas.

Lemma 2.2. The function $\overline{I}(\xi) = e^{\lambda_c\xi}$ satisfies

$\begin{equation}\label{upI} cI'(\xi) \geq d_3(J*I(\xi)-I(\xi)) + \beta_1 S_0I(\xi-c\tau) + \beta_2 V_0I(\xi-c\tau) - \gamma I(\xi) - \mu_3 I(\xi). \end{equation}$

(2.9)

Lemma 2.3. The functions $\overline{S}(\xi) = S_0$ and $\overline{V}(\xi) = V_0$ satisfy

$\begin{equation} \left\{ \begin{array}{l} \displaystyle cS'(\xi)\geq d_1(J*S(\xi)-S(\xi))+\Lambda - \beta_1 S(\xi)\underline{I}(\xi-c\tau) - \alpha S(\xi) - \mu_1 S(\xi), \\ \displaystyle cV'(\xi)\geq d_2(J*V(\xi)-V(\xi)) + \alpha S(\xi)- \beta_2 V(\xi)\underline{I}(\xi-c\tau) - \mu_2 V(\xi). \end{array}\right. \end{equation}$

(2.10)

The proof is trivial, so we omitted the above two lemmas.

Lemma 2.4. For each $0<\varepsilon_1<\lambda_c$ sufficiently small and $M_1$ large enough, the function $\underline{S}(\xi) = \max\{S_0-M_1 e^{\varepsilon_1 \xi}, 0\}$ satisfies

$\begin{equation}\label{lowS} cS'(\xi) \leq d_1(J*S(\xi)-S(\xi))+\Lambda - \beta_1S(\xi)\overline{I}(\xi-c\tau)- (\mu_1+\alpha) S(\xi), \end{equation}$

(2.11)

with $\xi\neq\mathfrak{X}_1\triangleq\frac{1}{\varepsilon_1}\ln\frac{S_0}{M_1}$ .

Proof. See Appendix A.

Lemma 2.5. For each $0<\varepsilon_2<\lambda_c$ sufficiently small and $M_2$ large enough, the function $\underline{V}(\xi) = \max\{V_0-M_2 e^{\varepsilon_2 \xi}, 0\}$ satisfies

$\begin{equation}\label{lowV} cV'(\xi) \leq d_2(J*V(\xi)-V(\xi))+\alpha \underline{S}(\xi) - \beta_2V(\xi)\overline{I}(\xi-c\tau)- \mu_2 V(\xi), \end{equation}$

(2.12)

with $\xi\neq\mathfrak{X}_2\triangleq\frac{1}{\varepsilon_2}\ln\frac{V_0}{M_2}$ .

The proof is similar with Lemma 2.4.

Lemma 2.6. Let $0<\varepsilon_3<\min\{\varepsilon_1/2, \varepsilon_2/2\}$ and $M_3>\max\{S_0, V_0\}$ is large enough, then the function $\underline{I}(\xi) = \max\{e^{\lambda_c\xi}(1-M_3e^{\varepsilon_3 \xi}), 0\}$ satisfies

$\begin{equation}\label{lowIEqu} cI'(\xi)\leq d_3(J*I(\xi)-I(\xi)) + \beta_1 \underline{S}(\xi)I(\xi-c\tau) + \beta_2 \underline{V}(\xi)I(\xi-c\tau) - \gamma I(\xi) - \mu_3 I(\xi), \end{equation}$

(2.13)

with $\xi\neq\mathfrak{X}_3\triangleq\frac{1}{\varepsilon_3}\ln\frac{1}{M_3}$ .

Proof. See Appendix B.

Let $X>\max\{\mathfrak{X}_1, \mathfrak{X}_2, \mathfrak{X}_3\}$ , define

$\begin{equation*} \label{Gamma} \Gamma_X = \left\{\left( \begin{array}{c} \phi \\ \varphi \\ \psi \\ \end{array} \right) \in C([-X, X], {\rm IR}^3)\left| \begin{array}{l} \displaystyle \underline{S}(\xi)\leq \phi(\xi)\leq S_0, \ \ \phi(-X) = \underline{S}(-X), \ \ \mathrm{for}\ \xi\in [-X, X];\\ \displaystyle \underline{V}(\xi)\leq \varphi(\xi)\leq V_0, \ \ \varphi(-X) = \underline{V}(X), \ \ \mathrm{for}\ \xi\in [-X, X];\\ \displaystyle \underline{I}(\xi)\leq \psi(\xi)\leq \overline{I}(\xi), \ \ \psi(-X) = \underline{I}(X), \ \ \mathrm{for}\ \xi\in [-X, X].\\ \end{array}\right.\right\}. \end{equation*}$

For given $(\phi(\xi), \varphi(\xi), \psi(\xi))\in \Gamma_X,$ define

$\begin{equation*} \label{hat1} \hat{\phi}(\xi) = \left\{ \begin{array}{l} \displaystyle \phi(X), \ \ \mathrm{for}\ \xi \gt X, \\ \displaystyle \phi(\xi), \ \ \ \mathrm{for}\ \xi\in[-X-c\tau, X], \\ \displaystyle \underline{S}(\xi), \ \ \ \mathrm{for}\ \xi\leq -X-c\tau, \\ \end{array}\right.\ \ \ \hat{\varphi}(\xi) = \left\{ \begin{array}{l} \displaystyle \varphi(X), \ \ \mathrm{for}\ \xi \gt X, \\ \displaystyle \varphi(\xi), \ \ \ \mathrm{for}\ \xi\in[-X-c\tau, X], \\ \displaystyle \underline{V}(\xi), \ \ \ \mathrm{for}\ \xi\leq -X-c\tau, \\ \end{array}\right. \end{equation*}$

and

$\begin{equation*} \label{hat2} \hat{\psi}(\xi) = \left\{ \begin{array}{l} \displaystyle \psi(X), \ \ \mathrm{for}\ \xi \gt X, \\ \displaystyle \psi(\xi), \ \ \ \mathrm{for}\ \xi\in[-X-c\tau, X], \\ \displaystyle \underline{I}(\xi), \ \ \ \ \mathrm{for}\ \xi\leq -X-c\tau.\\ \end{array}\right. \end{equation*}$

We have

$\begin{equation*} \label{hat3} \left\{ \begin{array}{l} \displaystyle \underline{S}(\xi)\leq\hat{\phi}(\xi)\leq S_0, \\ \displaystyle \underline{V}(\xi)\leq\hat{\varphi}(\xi)\leq V_0, \\ \displaystyle \underline{I}(\xi)\leq\hat{\psi}(\xi)\leq \overline{I}(\xi). \end{array}\right. \end{equation*}$

For any $\xi\in[-X, X],$ consider the following initial value problem

$\begin{equation} \label{Initial}\left\{ \begin{array}{l} \displaystyle cS'(\xi) = d_1\int_{{\rm IR}}J(y)\hat{\phi}(\xi-y)\rm{d} y + \Lambda - \beta_1S(\xi)\psi(\xi-c\tau) - (d_1+\mu_1+\alpha) S(\xi), \\ \displaystyle cV'(\xi) = d_2\int_{{\rm IR}}J(y)\hat{\varphi}(\xi-y)\rm{d} y + \alpha\phi(\xi)- \beta_2V(\xi)\psi(\xi-c\tau) - (d_2+\mu_2) V(\xi), \\ \displaystyle cI'(\xi) = d_3\int_{{\rm IR}}J(y)\hat{\psi}(\xi-y)\rm{d} y + \beta_1\phi(\xi)\psi(\xi-c\tau) +\beta_2\varphi(\xi)\psi(\xi-c\tau) - (d_3+\gamma+\mu_3) I(\xi), \\ \displaystyle S(-X) = \underline{S}(-X), \ \ \ V(-X) = \underline{V}(-X), \ \ \ I(-X) = \underline{I}(-X). \end{array}\right. \end{equation}$

(2.14)

From the standard theory of functional differential equations (see ^[32]), the initial value problem (2.14) admits a unique solution $(S_X(\xi), V_X(\xi), I_X(\xi))$ satisfying

$(S_X, V_X, I_X)\in C^1([-X, X]),$

this defines an operator $\mathcal{A} = (\mathcal{A}_1, \mathcal{A}_2, \mathcal{A}_3):\Gamma_X\rightarrow C^1([-X, X])$ as

$S_X = \mathcal{A}_1(\phi, \varphi, \psi), \ V_X = \mathcal{A}_2(\phi, \varphi, \psi), \ I_X = \mathcal{A}_3(\phi, \varphi, \psi).$

Next we show the operator $\mathcal{A} = (\mathcal{A}_1, \mathcal{A}_2, \mathcal{A}_3)$ has a fixed point in $\Gamma_X$ .

Lemma 2.7. The operator $\mathcal{A} = (\mathcal{A}_1, \mathcal{A}_2, \mathcal{A}_3)$ maps $\Gamma_X$ into itself.

Proof. Firstly, we show that $\underline{S}(\xi)\leq S_X(\xi)$ for any $\xi\in[-X, X].$ If $\xi\in(\mathfrak{X}_1, X),$ $\underline{S}(\xi) = 0$ is a lower solution of the first equation of (2.14). If $\xi\in(-X, \mathfrak{X}_1),$ $\underline{S}(\xi) = S_0-M_1 e^{\varepsilon_1 \xi}$ , by Lemma 2.4, we have

$\begin{align*} &c\underline{S}'(\xi) - d_1\int_{{\rm IR}}J(y)\hat{\phi}(\xi-y)\rm{d} y - \Lambda + \beta_1\underline{S}(\xi)\psi(\xi-c\tau) - (d_1+\mu_1+\alpha) \underline{S}(\xi)\\ \nonumber \leq&c\underline{S}'(\xi) - d_1\int_{{\rm IR}}J(y)\underline{S}(\xi-y)\rm{d} y - \Lambda + \beta_1\underline{S}(\xi)\overline{I}(\xi-c\tau) - (d_1+\mu_1+\alpha) \underline{S}(\xi))\\ \leq&0, \nonumber \end{align*}$

which implies that $\underline{S}(\xi) = S_0-M_1 e^{\varepsilon_1 \xi}$ is a lower solution of the first equation of (2.14). Thus $\underline{S}(\xi)\leq S_X(\xi)$ for any $\xi\in[-X, X].$

Secondly, we show that $S_X(\xi)\leq \overline{S}(\xi) = S_0$ for any $\xi\in[-X, X].$ In fact,

$\begin{align*} &d_1\int_{{\rm IR}}J(y)\hat{\phi}(\xi-y)\rm{d} y + \Lambda - \beta_1S_0\psi(\xi-c\tau) - (d_1+\mu_1+\alpha) S_0\\ \nonumber \leq& d_1\int_{{\rm IR}}J(y)S_0\rm{d} y + \Lambda - \beta_1S_0\underline{I}(\xi-c\tau) - (d_1+\mu_1+\alpha) S_0\\ \leq&0, \nonumber \end{align*}$

thus $\overline{S}(\xi) = S_0$ is an upper solution to the first equation of (2.14), which gives us $S_X(\xi)\leq S_0$ for any $\xi\in[-X, X].$

Similarly, $\underline{V}(\xi)\leq V_X(\xi)\leq \overline{V}(\xi)$ and $\underline{I}(\xi)\leq I_X(\xi)\leq \overline{I}(\xi)$ for any $\xi\in[-X, X].$

Lemma 2.8. The operator $\mathcal{A}$ is completely continuous.

Proof. Suppose $(\phi_i(\xi), \varphi_i(\xi), \psi_i(\xi))\in\Gamma_X, \ i = 1, 2.$

$\begin{align*} S_{X, i}(\xi)& = \mathcal{A}_1(\phi_i(\xi), \varphi_i(\xi), \psi_i(\xi)), \\ V_{X, i}(\xi)& = \mathcal{A}_2(\phi_i(\xi), \varphi_i(\xi), \psi_i(\xi)), \\ I_{X, i}(\xi)& = \mathcal{A}_3(\phi_i(\xi), \varphi_i(\xi), \psi_i(\xi)), \end{align*}$

We show the operator $\mathcal{A}$ is continuous. By direct calculation, we have

$\begin{align*} S_X(\xi) = &\underline{S}(-X) \exp \left\{-\frac{1}{c}\int_{-X}^\xi(d_1 +\mu_1+\alpha+\beta_1\psi(s-c\tau))\rm{d} s\right\}\\ &+ \frac{1}{c}\int_{-X}^\xi\exp \left\{-\frac{1}{c}\int_{\eta}^\xi(d_1 +\mu_1+\alpha+\beta_1\psi(s-c\tau))\rm{d} s\right\}f_{\phi}(\eta)\rm{d} \eta, \end{align*}$

$\begin{align*} V_X(\xi) = &\underline{V}(-X) \exp \left\{-\frac{1}{c}\int_{-X}^\xi(d_2 +\mu_2+\beta_2\psi(s-c\tau))\rm{d} s\right\}\\ &+ \frac{1}{c}\int_{-X}^\xi\exp \left\{-\frac{1}{c}\int_{\eta}^\xi(d_2 +\mu_2+\beta_2\psi(s-c\tau))\rm{d} s\right\}f_{\varphi}(\eta)\rm{d} \eta, \end{align*}$

and

$\begin{align*} I_X(\xi) = &\underline{I}(-X) \exp \left\{-\frac{(d_3+\gamma+\mu_3)(\xi+X)}{c}\right\}\\ &+ \frac{1}{c}\int_{-X}^\xi\exp \left\{-\frac{(d_3+\gamma+\mu_3)(\xi-\eta)}{c}\right\}f_{\psi}(\eta)\rm{d} \eta. \end{align*}$

where

$\begin{align*} f_{\phi}(\eta) & = d_1\int_{{\rm IR}}J(\eta-y)\hat{\phi}(y)\rm{d} y + \Lambda, \\ f_{\varphi}(\eta) & = d_2\int_{{\rm IR}}J(\eta-y)\hat{\varphi}(y)\rm{d} y + \alpha\phi(\eta), \\ f_{\psi}(\eta) & = d_3\int_{{\rm IR}}J(\eta-y)\hat{\psi}(y)\rm{d} y + (\beta_1\phi(\eta)+\beta_2\varphi(\eta))\psi(\eta-c\tau). \end{align*}$

For any $(\phi_i, \varphi_i, \psi_i)\in\Gamma_X$ , $i = 1, 2$ , we have

$\begin{align*} |f_{\phi_1}(\eta) - f_{\phi_2}(\eta)| = &d_1\left|\int_{{\rm IR}}J(\eta-y)[\hat{\phi}_1(y) - \hat{\phi}_2(y)]\rm{d} y\right|\\ \leq &d_1\left|\int_{-X}^X J(\xi-y)(\phi_1(y)-\phi_2(y))\rm{d} y\right|+d_1\left|\int_X^\infty J(\xi-y)(\phi_1(X)-\phi_2(X))\rm{d} y\right|\\ \leq &2d_1\max\limits_{y\in[-X, X]}|\phi_1(y)-\phi_2(y)|, \end{align*}$

$\begin{align*} |f_{\varphi_1}(\eta) - f_{\varphi_2}(\eta)| = &d_2\left|\int_{{\rm IR}}J(\eta-y)[\hat{\varphi}_1(y) - \hat{\varphi}_2(y)]\rm{d} y + \alpha(\phi_1(\eta) - \phi_2(\eta))\right|\\ \leq &2d_2\max\limits_{y\in[-X, X]}|\varphi_1(y)-\varphi_2(y)| + \alpha \max\limits_{y\in[-X, X]}|\phi_1(y)-\phi_2(y)|, \end{align*}$

$\begin{align*} |f_{\psi_1}(\eta) - f_{\psi_2}(\eta)|\leq &(2d_2+\beta_1S_0+\beta_2V_0)\max\limits_{y\in[-X, X]}|\psi_1(y)-\psi_2(y)|\\ &+\beta_1e^{\lambda_c\xi}\max\limits_{y\in[-X, X]}|\phi_1(y)-\phi_2(y)|+\beta_2e^{\lambda_c\xi}\max\limits_{y\in[-X, X]}|\varphi_1(y)-\varphi_2(y)|. \end{align*}$

Here we use

$\begin{align*} &\left|\beta_1\phi_2(\xi)\psi_2(\xi-c\tau)-\beta_1\phi_1(\xi)\psi_1(\xi-c\tau)\right|\\ \leq &\left|\beta_1\phi_2(\xi)\psi_2(\xi-c\tau)-\beta_1\phi_2(\xi)\psi_1(\xi-c\tau)\right| +\left|\beta_1\phi_2(\xi)\psi_1(\xi-c\tau)-\beta_1\phi_1(\xi)\psi_1(\xi-c\tau)\right|\\ \leq &\beta_1S_0\max\limits_{y\in[-X, X]}|\psi_1(y)-\psi_2(y)|+\beta_1e^{\lambda_c\xi}\max\limits_{y\in[-X, X]}|\phi_1(y)-\phi_2(y)|. \end{align*}$

and

$\begin{align*} &\left|\beta_2\varphi_2(\xi)\psi_2(\xi-c\tau)-\beta_2\varphi_1(\xi)\psi_1(\xi-c\tau)\right|\\ \leq &\beta_2V_0\max\limits_{y\in[-X, X]}|\psi_1(y)-\psi_2(y)|+\beta_2e^{\lambda_c\xi}\max\limits_{y\in[-X, X]}|\varphi_1(y)-\varphi_2(y)|. \end{align*}$

Thus, we obtain that the operator $\mathcal{A}$ is continuous. Next, we show $\mathcal{A}$ is compact. Indeed, since $S_X$ , $V_X$ and $I_X$ are class of $C^1([-X, X])$ , note that

$\begin{align*} &c(S_{X, 1}'(\xi)-S_{X, 2}'(\xi))+(d_1+\mu_1+\alpha)(S_{X, 1}(\xi)-S_{X, 2}(\xi))\\ = &d_1\int_{{\rm IR}}J(\xi-y)(\hat{\phi}_1(y)-\hat{\phi}_2(y))\rm{d} y+ \beta_1\phi_2(\xi)\psi_2(\xi-c\tau)-\beta_1\phi_1(\xi)\psi_1(\xi-c\tau)\\ \leq&\left(2d_1+\beta_1e^{\lambda_c\xi}\right)\max\limits_{y\in[-X, X]}|\phi_1(y)-\phi_2(y)|+\beta_1S_0\max\limits_{y\in[-X, X]}|\psi_1(y)-\psi_2(y)|. \end{align*}$

Same arguments with $V_X'$ and $I_X'$ , give us $S_X'$ , $V_X'$ and $I_X'$ are bounded. Then $\mathcal{A}$ is compact and the operator $\mathcal{A}$ is completely continuous. This ends the proof.

Obviously, $\Gamma_X$ is a bounded closed convex set, applying the Schauder's fixed point theorem (^[33] Corollary 2.3.10), we have the following theorem.

Theorem 2.1. There exists $(S_X, V_X, I_X)\in \Gamma_X$ such that

$(S_X(\xi), V_X(\xi), I_X(\xi)) = \mathcal{A}(S_X, V_X, I_X)(\xi)$

for $\xi\in[-X, X].$

Now we are in position to show the existence of traveling wave solutions, before that we do some estimates for $S_X(\cdot), \ V_X(\cdot)$ and $I_X(\cdot).$

Define

$C^{1, 1}([-X, X]) = \{u\in C^1([-X, X])|u, u' \textrm{are Lipschitz continuous}\}$

with norm

$\begin{gather*} \|u\|_{C^{1, 1}([-X, X])} = \max\limits_{x\in[-X, X]}|u|+\max\limits_{x\in[-X, X]}|u'|+ \sup\limits_{\begin{subarray}{c} x, y\in [-X, X] \\ x\neq y \end{subarray}}\frac{|u'(x)-u'(y)|}{|x-y|}. \end{gather*}$

Lemma 2.9. There exists a constant $C(Y)>0$ such that

$\begin{equation*} \|S_X\|_{C^{1, 1}([-Y, Y])}\leq C(Y), \ \ \|V_X\|_{C^{1, 1}([-Y, Y])}\leq C(Y), \ \ \|I_X\|_{C^{1, 1}([-Y, Y])}\leq C(Y) \end{equation*}$

for $Y<X$ and $X>\max\{\mathfrak{X}_1, \mathfrak{X}_2, \mathfrak{X}_3\}.$

Proof. Recall that $(S_X, E_X, I_X)$ is the fixed point of the operator $\mathcal{A},$ then

$cS_X'(\xi) = d_1\int_{-\infty}^{+\infty}J(y)\hat{S}_X(\xi-y)\rm{d} y + \Lambda - \beta_1S_X(\xi)I_X(\xi-c\tau) - (d_1+\mu_1+\alpha) S_X(\xi),$

(2.15)

$cV_X'(\xi) = d_2\int_{-\infty}^{+\infty}J(y)\hat{V}_X(\xi-y)\rm{d} y + \alpha S_X(\xi)- \beta_2V_X(\xi)I_X(\xi-c\tau) - (d_2+\mu_2)V_X(\xi),$

(2.16)

$cI_X'(\xi) = d_3\int_{-\infty}^{+\infty}J(y)\hat{I}_X(\xi-y)\rm{d} y + \beta_1S_X(\xi)I_X(\xi-c\tau) + \beta_2V_X(\xi)I_X(\xi-c\tau) - (d_3+\mu_3) I_X(\xi),$

(2.17)

where

$\begin{equation*} (\hat{S}_X(\xi), \hat{V}_X(\xi), \hat{I}_X(\xi)) = \left\{ \begin{array}{l} \displaystyle (S_X(X), V_X(X), I_X(X)), \ \ \mathrm{for}\ \xi \gt X, \\ \displaystyle (S_X(\xi), V_X(\xi), I_X(\xi)), \ \ \ \ \ \ \mathrm{for}\ \xi\in [-X-c\tau, X], \\ \displaystyle (\underline{S}(\xi), \underline{V}(\xi), \underline{I}(\xi)), \ \ \ \ \ \ \ \ \ \ \ \ \mathrm{for}\ \xi\leq -X-c\tau, \\ \end{array}\right. \end{equation*}$

following that $S_X(\xi)\leq S_0, \ \ V_X(\xi)\leq V_0, \ \ I_X(\xi)\leq e^{\lambda_c Y}$ for any $\xi\in[-Y, Y].$ Then

$\begin{align*} |S'_X(\xi)|\leq &\frac{d_1}{c}\left|\int_{-\infty}^{+\infty}J(y)\hat{S}_X(\xi-y)\rm{d} y\right|+\frac{\Lambda}{c}+\frac{d_1+\mu_1+\alpha}{c}|S_X(\xi)|+\frac{\beta_1}{c}|S_X(\xi)||I_X(\xi-c\tau)|\\ \leq&\frac{2d_1+\mu_1+\alpha}{c} S_0+\frac{\Lambda}{c}+\frac{\beta_1S_0}{c}e^{\lambda_c Y}, \\ |V'_X(\xi)|\leq &\frac{2d_2+\mu_2}{c}V_0+\frac{\alpha S_0}{c}+\frac{\beta_2V_0}{c}e^{\lambda_c Y}, \\ |I'_X(\xi)|\leq &\left(\frac{d_3+\mu_3}{c}+\frac{\beta_1S_0}{c}+\frac{\beta_2V_0}{c}\right)e^{\lambda_c Y}. \end{align*}$

Thus, there exists some constant $C_1(Y)>0$ such that

$\begin{equation*} \|S_X\|_{C^{1}([-Y, Y])}\leq C_1(Y), \ \ \|V_X\|_{C^{1}([-Y, Y])}\leq C_1(Y), \ \ \|I_X\|_{C^{1}([-Y, Y])}\leq C_1(Y). \end{equation*}$

Then for any $\xi_1, \xi_2\in[-Y, Y]$ such that

$\begin{equation*} |S_X(\xi_1)-S_X(\xi_2)|\leq C_1(Y)|\xi_1-\xi_2|, \ \ |V_X(\xi_1)-V_X(\xi_2)|\leq C_1(Y)|\xi_1-\xi_2|, \ \ |I_X(x_1)-I_X(x_2)|\leq C_1(Y)|\xi_1-\xi_2|. \end{equation*}$

From (2.15), we have

$\begin{align*} c|S'_X(\xi_1)-S'_X(\xi_2)|\leq&d_1\left|\int_{-\infty}^{+\infty}J(y)(\hat{S}_X(\xi_1-y)-\hat{S}_X(\xi_2-y))\rm{d} y\right|\\ &+ (d_1+\mu_1+\alpha)|S_X(\xi_1)-S_X(\xi_2)| + S_0|I_X(\xi_1)-I_X(\xi_2)|. \end{align*}$

Recall (J1) of Assumption 1.1, we know $J$ is Lipschitz continuous and compactly supported on ${\rm IR}$ , let $L$ be the Lipschitz constant for $J$ and $R$ be the radius of supp $J$ . Then

$\begin{align*} &d_1\left|\int_{-\infty}^{+\infty}J(y)(\hat{S}_X(\xi_1-y)-\hat{S}_X(\xi_2-y))\rm{d} y\right|\\ = &d_1\left|\int_{-R}^{R}J(y)\hat{S}_X(\xi_1-y)\rm{d} y-\int_{-R}^{R}J(y)\hat{S}_X(\xi_2-y)\rm{d} y\right|\\ = &d_1\left|\int_{\xi_1-R}^{\xi_1+R}J(\xi_1-y)\hat{S}_X(y)\rm{d} y-\int_{\xi_2-R}^{\xi_2+R}J(y)\hat{S}_X(y)\rm{d} y\right|\\ = &d_1\left|\left(\int_{\xi_1-R}^{\xi_2-R}+\int_{\xi_2-R}^{\xi_2+R}+\int_{\xi_2+R}^{\xi_1+R}\right)J(\xi_1-y)\hat{S}_X(y)\rm{d} y-\int_{\xi_2-R}^{\xi_2+R}J(y)\hat{S}_X(y)\rm{d} y\right|\\ \leq& d_1\left|\int_{\xi_2+R}^{\xi_1+R}J(\xi_1-y)\hat{S}_X(y)\rm{d} y\right|+d_1\left|\int_{\xi_1-R}^{\xi_2-R}J(\xi_1-y)\hat{S}_X(y)\rm{d} y\right|\\ &+d_1\left|\int_{\xi_2-R}^{\xi_2+R}(J(\xi_1-y)-J(\xi_2-y))\hat{S}_X(y)\rm{d} y\right|\\ \leq& d_1(2S_0\|J\|_{L^\infty}+2RLS_0)|\xi_1-\xi_2|. \end{align*}$

Thus there exists some constant $C_2(Y)>0$ such that

$|S'_X(\xi_1)-S'_X(\xi_2)|\leq C_2(Y)|\xi_1-\xi_2|.$

Similarly

From the above discussion, there exists some constant $C(Y)>0$ for any $Y<X$ that is independent of $X$ such that

$\begin{equation*} \|S_X\|_{C^{1, 1}([-Y, Y])}\leq C(Y), \ \ \|V_X\|_{C^{1, 1}([-Y, Y])}\leq C(Y), \ \ \|I_X\|_{C^{1, 1}([-Y, Y])}\leq C(Y). \end{equation*}$

Now let $\{X_n\}_{n = 1}^{+\infty}$ be an increasing sequence such that $X_n\geq\{\mathfrak{X}_1, \mathfrak{X}_2, \mathfrak{X}_3\}$ , $X_n>Y+R$ for each $n$ and $\lim\limits X_n = +\infty$ , where $R$ is the radius of supp $J$ . For every $c>c^*$ , we have $(S_{X_n}, V_{X_n}, I_{X_n})\in\Gamma_{X_n}$ satisfying Lemma 2.9 and Equations (2.15)-(2.17).For the sequence $(S_{X_n}, V_{X_n}, I_{X_n})$ , we can extract a subsequence denoted by $\{S_{X_{n_k}}\}_{k\in{\rm IN}}$ , $\{V_{X_{n_k}}\}_{k\in{\rm IN}}$ and $\{I_{X_{n_k}}\}_{k\in{\rm IN}}$ tending to functions $(S, V, I)\in C^1({\rm IR})$ in the following topologies

$S_{X_{n_k}}\rightarrow S, \ \ V_{X_{n_k}}\rightarrow V\ \ \textrm{and}\ \ I_{X_{n_k}}\rightarrow I\ \ \textrm{in}\ \ C^1_{\textrm{loc}}({\rm IR})\ \ \textrm{as}\ \ k\rightarrow+\infty.$

Since $J$ is compactly supported, applying the dominated convergence theorem, thus

$\lim\limits_{k\rightarrow+\infty}\int_{{\rm IR}}J(y)\hat{S}_{X_{n_k}}(\xi-y)\rm{d} y = \int_{{\rm IR}}J(y)S(\xi-y)\rm{d} y = J*S(\xi),$

$\lim\limits_{k\rightarrow+\infty}\int_{{\rm IR}}J(y)\hat{V}_{X_{n_k}}(\xi-y)\rm{d} y = \int_{{\rm IR}}J(y)V(\xi-y)\rm{d} y = J*V(\xi)$

and

$\lim\limits_{k\rightarrow+\infty}\int_{{\rm IR}}J(y)\hat{I}_{X_{n_k}}(\xi-y)\rm{d} y = \int_{{\rm IR}}J(y)I(\xi-y)\rm{d} y = J*I(\xi).$

Moreover, $(S, V, I)$ satisfies system (2.4) and

$\underline{S}(\xi)\leq S(\xi)\leq S_0, \ \ \underline{V}(\xi)\leq V(\xi)\leq V_0, \ \ \underline{I}(\xi)\leq I(\xi)\leq e^{\lambda_c \xi}.$

Next, we show that $I(\xi)$ is bounded in ${\rm IR}$ by the method in ^[34] (see also ^[35,36]).

Lemma 2.10. There exists some positive constant $C$ such that

$\int_{{\rm IR}} J(y)\frac{I(\xi-y)}{I(\xi)}\rm{d} y \lt C, \ \ \ \frac{I(\xi-c\tau)}{I(\xi)} \lt C \ \ \ \mathit{and}\ \ \ \bigg|\frac{I'(\xi)}{I(\xi)}\bigg| \lt C.$

Proof. Let $\theta(\xi) = \frac{I'(\xi)}{I(\xi)}$ , from the third equation of (2.4), we have

$\begin{align*} \theta(\xi) &\geq \frac{d_3}{c}\left(\int_{{\rm IR}} J(y)\frac{I(\xi-y)}{I(\xi)}\rm{d} y-1\right) - \frac{\gamma + \mu_3}{c}\\ & = \frac{d_3}{c} \int_{{\rm IR}} J(y) e^{\int_{\xi}^{\xi-y}\theta(s)\rm{d} s}\rm{d} y - \left(\frac{d_3+\gamma+\mu_3}{c}\right). \end{align*}$

Set $\varpi = \left(\frac{d_3+\gamma+\mu_3}{c}\right)$ and $W(\xi) = \exp\left\{\varpi\xi + \int_0^{\xi}\theta(s)\rm{d} s\right\}$ , thus

$W'(\xi) = (\varpi + \theta(\xi))W(\xi) \geq \frac{d_3}{c} \int_{{\rm IR}} J(y) e^{\int_{\xi}^{\xi-y}\theta(s)\rm{d} s}\rm{d} y W(\xi),$

that is $W(\xi)$ is non-decreasing. We can take some $R_0>0$ with $2R_0<R$ , where $R$ is the radius of supp $J$ . Then by the same argument in [34,Lemma 2.2], we have

$W(\xi)\geq \frac{d_3}{c}R_0\int_{{\rm IR}}J(y)e^{\varpi y}W(\xi-R_0-y)\rm{d} y$

and

$W(\xi+R_0)\leq \sigma_0 W(\xi)\ \ \ \textrm{for}\ \ \textrm{all}\ \ \xi\in{\rm IR},$

where

$\sigma_0 \triangleq \frac{d_3}{cR_0\int_{-\infty}^{-2R_0}J(y)e^{\varpi y}\rm{d} y}.$

Thus

$\begin{align*} \int_{{\rm IR}} J(y)\frac{I(\xi-y)}{I(\xi)}\rm{d} y = &\int_{-\infty}^0 J(y)\frac{I(\xi-y)}{I(\xi)}\rm{d} y + \int_0^{+\infty} J(y)\frac{I(\xi-y)}{I(\xi)}\rm{d} y\\ = &\int_{-\infty}^0 J(y)e^{\varpi y}\frac{W(\xi-y)}{W(\xi)}\rm{d} y + \int_0^{+\infty} J(y)e^{\varpi y}\frac{W(\xi-y)}{W(\xi)}\rm{d} y\\ \leq&\sigma_0\int_{-\infty}^0 J(y)e^{\varpi y}\frac{W(\xi-y-R_0)}{W(\xi)}\rm{d} y + \int_0^{+\infty} J(y)e^{\varpi y}\rm{d} y\\ \leq&\frac{c\sigma_0}{d_3R_0} + \int_0^{+\infty} J(y)e^{\varpi y}\rm{d} y. \end{align*}$

Again with the third equation of (2.4), we have

$I'(\xi) + \varpi I(\xi) = d_3J*I(\xi) + \beta_1S(\xi)I(\xi-c\tau) + \beta_2V(\xi)I(\xi-c\tau) \gt 0\ \ \ \textrm{for}\ \ \textrm{all}\ \ \xi\in{\rm IR}.$

Let $U(\xi) = e^{\varpi\xi} I(\xi)$ , then $U'(\xi)\geq0$ , it follows that

$\frac{I(\xi-c\tau)}{I(\xi)}\leq e^{\varpi c\tau}.$

Furthermore,

$\bigg|\frac{I'(\xi)}{I(\xi)}\bigg|\leq \frac{d_3}{c}\int_{{\rm IR}} J(y)\frac{I(\xi-y)}{I(\xi)}\rm{d} y + (\beta_1S_0+\beta_2V_0)\frac{I(\xi-c\tau)}{I(\xi)} + \varpi.$

This completes the proof.

Lemma 2.11. Choose $c_k\in(c^*, c^*+1)$ and let $\{c_k, S_k, V_k, I_k\}$ be a sequence of traveling waves of (2.1) with speeds $\{c_k\}$ . If there is a sequence $\{\xi_k\}$ such that $I_k(\xi_k)\rightarrow+\infty$ as $k\rightarrow+\infty$ , then $S_k(\xi_k)\rightarrow0$ and $V_k(\xi_k)\rightarrow0$ as $k\rightarrow+\infty$ .

Proof. Assume that there is a subsequence of $\{\xi_k\}_{k\in{\rm IN}}$ again denoted by $\xi_k$ , such that $I_k(\xi_k)\rightarrow+\infty$ as $k\rightarrow+\infty$ and $S_k(\xi_k)\geq\varepsilon$ in ${\rm IR}$ for all $k\in{\rm IN}$ with some positive constant $\varepsilon$ . From the first equation of (2.4), we have

$S'_k(\xi)\leq \frac{2d_1S_0+\Lambda}{c^*} \triangleq \rm{d}elta_0\ \ \textrm{in}\ \ {\rm IR}.$

It follows that

$S_k(\xi)\geq\frac{\varepsilon}{2}, \ \ \ \forall\xi\in[\xi_k-\rm{d}elta, \xi_k],$

for all $k\in{\rm IN}$ , where $\rm{d}elta = \frac{\varepsilon}{\rm{d}elta_0}$ . By Lemma 2.10, we have $\bigg|\frac{I'_k}{I_k}\bigg|<C_0$ for some $C_0>0$ . Then

$\frac{I_k(\xi_k)}{I_k(\xi-c\tau)} = \exp\left\{\int_{\xi-c\tau}^{\xi_k}\frac{I'_k(s)}{I_k(s)}\rm{d} s\right\}\leq e^{C_0(c\tau+\rm{d}elta)}, \ \ \forall\xi\in[\xi_k-\rm{d}elta, \xi_k]$

for all $k\in{\rm IN}$ . Thus

$\min\limits_{\xi\in[\xi_k-\rm{d}elta, \xi_k]}I_k(\xi-c\tau)\geq e^{-C_0(c\tau+\rm{d}elta)}I_k(\xi_k),$

which give us

$\min\limits_{\xi\in[\xi_k-\rm{d}elta, \xi_k]}I_k(\xi-c\tau) \rightarrow +\infty\ \ \textrm{as}\ \ k\rightarrow+\infty$

since $I_k(\xi_k)\rightarrow+\infty$ as $k\rightarrow+\infty$ . Recalling the first equation of (2.4), one can have

$\max\limits_{\xi\in[\xi_k-\rm{d}elta, \xi_k]}S'_k(\xi)\leq \rm{d}elta_0 - \frac{\beta_1\varepsilon}{2}\min\limits_{\xi\in[\xi_k-\rm{d}elta, \xi_k]}I_k(\xi-c\tau)\rightarrow-\infty\ \ \textrm{as}\ \ k\rightarrow+\infty.$

Moreover, there exists some $K>0$ such that

$S'_k(\xi)\leq - \frac{2S_0}{\rm{d}elta}, \ \ \forall k\geq K\ \ \textrm{and}\ \ \xi\in[\xi_k-\rm{d}elta, \xi_k].$

Note that $S_k<S_0$ in ${\rm IR}$ for each $k\in{\rm IN}$ . Hence $S_k(\xi_k)\leq-S_0$ for all $k\geq K$ , which reduces to a contradiction since $S_k(\xi_k)\geq\varepsilon$ in ${\rm IR}$ for all $k\in{\rm IN}$ with some positive constant $\varepsilon$ . Similarly, we can show that $V_k(\xi_k)\rightarrow0$ as $k\rightarrow+\infty$ . This completes the proof.

Lemma 2.12. If $\limsup\limits_{\xi\rightarrow\infty}I(\xi) = \infty$ , then $\lim\limits_{\xi\rightarrow\infty}I(\xi) = \infty$ .

The proof is similar to that of [34,Lemma 2.4], so we omit the details. With the previous lemmas, we can show that $I(\xi)$ is bounded in ${\rm IR}$ .

Theorem 2.2. $I(\xi)$ is bounded in ${\rm IR}$ .

Proof. Assume that $\limsup\limits_{\xi\rightarrow\infty}I(\xi) = \infty$ , then we have $\lim\limits_{\xi\rightarrow\infty}S(\xi) = 0$ and $\lim\limits_{\xi\rightarrow\infty}V(\xi) = 0$ from Lemma 2.11 and Lemma 2.12. Set $\theta(\xi) = \frac{I'(\xi)}{I(\xi)}$ , from the third equation of (2.4), we have

$c\theta(\xi) = d_3\int_{{\rm IR}}J(y)e^{\int_\xi^{\xi-y}\theta(s)\rm{d} s} - (d_3+\gamma+\mu_3) + B(\xi),$

where

$B(\xi) = [\beta_1S(\xi)+\beta_2V(\xi)]\frac{I(\xi-c\tau)}{I(\xi)}.$

Since $\frac{I(\xi-c\tau)}{I(\xi)}<C$ for some positive constant $C$ from Lemma 2.10. By using [34,Lemma 2.5], we can get that $\lim\limits_{\xi\rightarrow+\infty}\theta(\xi)$ exists and satisfies the following equation

$f(\lambda, c) \triangleq d_3\left(\int_{\rm IR}J(y)e^{-\lambda y}-1\right)-c\lambda-(\gamma+\mu_3).$

By some calculations, we obtain

$f(0, c) \lt 0, \ \ \frac{\partial f(\lambda, c)}{\partial \lambda}\bigg|_{\lambda = 0} \lt 0, \ \ \frac{\partial^2 f(\lambda, c)}{\partial \lambda^2} \gt 0\ \ \textrm{and}\ \ \lim\limits_{\lambda\rightarrow+\infty}f(\lambda, c) = -\infty.$

Thus, $I(\xi)$ is bounded by using the same arguments in [34,Theorem 2.6]. This ends the proof.

Since $I(\xi)$ is bounded in ${\rm IR}$ , we assume that there exists a positive constant $\rho<\infty$ such that $I(\xi)<\rho$ . Furthermore, it can be verified $\frac{\Lambda}{\mu_1+\alpha+\beta_1\rho}$ is a lower solution of $S$ and $\frac{\alpha\Lambda}{(\mu_1+\alpha+\beta_1\rho)(\mu_2+\beta_2\rho)}$ is a lower solution of $V$ . Then we have the following proposition.

Proposition 2.1. $S(\xi), V(\xi)$ and $I(\xi)$ satisfy

$\begin{align*} \frac{\Lambda}{\mu_1+\alpha+\beta_1\rho}\leq S(\xi)\leq S_0, \ \ \frac{\alpha\Lambda}{(\mu_1+\alpha+\beta_1\rho)(\mu_2+\beta_2\rho)}\leq V(\xi)\leq V_0, \ \ \underline{I}(\xi)\leq I(\xi)\leq\rho \end{align*}$

for $\xi\in {\rm IR}$ .

The following lemma is to show that $I(\xi)$ cannot approach $0$ .

Lemma 2.13. Assume that $\Re_0>1$ , then for each $c>c^*$ , we have

$\liminf\limits_{\xi\rightarrow\infty}I(\xi) \gt 0.$

Proof. We only need to show that if $I(\xi)\leq\varepsilon_0$ for some small enough constant $\varepsilon_0>0$ , then $I'(\xi)>0$ for all $\xi\in {\rm IR}$ . Assume by way of contradiction that there is no such $\varepsilon_0$ , that is there exist some sequence $\{\xi_k\}_{k\in{\rm IN}}$ such that $I(\xi_k)\rightarrow0$ as $k\rightarrow+\infty$ and $I'(\xi_k)\leq0$ . Denote

$S_k(\xi)\triangleq S(\xi_k+\xi), \ \ V_k(\xi)\triangleq V(\xi_k+\xi)\ \ \textrm{and}\ \ I_k(\xi)\triangleq I(\xi_k+\xi).$

Thus we have $I_k(0)\rightarrow0$ as $k\rightarrow+\infty$ and $I_k(\xi)\rightarrow0$ locally uniformly in ${\rm IR}$ as $k\rightarrow+\infty$ . As a consequence, there also holds that $I_k'(\xi)\rightarrow0$ locally uniformly in ${\rm IR}$ as $k\rightarrow+\infty$ by the third equation of (2.4). From the argument in [25,Theorem 2.9], we can obtain that $S_\infty = S_0$ and $V_\infty = V_0$ .

Let $\psi_k(\xi)\triangleq\frac{I_k(\xi)}{I_k(0)}$ . By Lemma 2.10, and in the view of

$\psi_k'(\xi) = \frac{I_k'(\xi)}{I_k(0)} = \frac{I_k'(\xi)}{I_k(\xi)}\psi_k(\xi),$

we have $\psi_k(\xi)$ and $\psi_k'(\xi)$ are also locally uniformly in ${\rm IR}$ as $k\rightarrow+\infty$ . Letting $k\rightarrow+\infty$ , thus

$c\psi_\infty'(\xi) = d_3\int_{\rm IR}J(y)\psi_\infty(\xi-y)\rm{d} y + (\beta_1 S_0+\beta_2 V_0)\psi_\infty(\xi-c\tau) - (d_3+\gamma+\mu_3) \psi_\infty(\xi).$

One can have $\psi_\infty(\xi)>0$ in ${\rm IR}$ . In fact, if there exist some $\xi_0$ such that $\psi_\infty(\xi_0) = 0$ and $\psi_\infty(\xi)>0$ for all $\xi<\xi_0$ , then

$0 = d_3\int_{\rm IR}J(y)\psi_\infty(\xi_0-y)\rm{d} y + (\beta_1 S_0+\beta_2 V_0)\psi_\infty(\xi_0-c\tau) \gt 0,$

which is a contradiction.

Denote $Z(\xi)\triangleq\frac{\psi_\infty'(\xi)}{\psi_\infty(\xi)}$ , it is easy to verify $Z(\xi)$ satisfies

$\begin{equation}\label{Z} cZ(\xi) = d_3\int_{\rm IR}J(y)e^{\int^{\xi-y}_\xi Z(s)\rm{d} s}\rm{d} y + (\beta_1 S_0+\beta_2 V_0)e^{\int^{\xi-c\tau}_\xi Z(s)\rm{d} s} - (d_3+\gamma+\mu_3). \end{equation}$

(2.18)

Then by similar discussion in [25,Theorem 2.9], for $\Re_0>1$ and $c>c^*$ , we have

$0 \lt \psi_\infty'(0) = \lim\limits_{k\rightarrow+\infty}\psi_n'(0) = \lim\limits_{k\rightarrow+\infty}\frac{I_n'(0)}{I_n(0)}.$

Thus, $I'(\xi_k) = I_n'(0)>0$ , which is a contradiction. This completes the proof.

Remark 2.1. In the proof of Lemma 2.13, we need to show that $Z(\pm\infty)$ exist in Equation (2.18). In ^[25], the authors applying [37,Lemma 3.4] to show that $Z(\pm\infty)$ exist. There is a time delay term in Equation (2.18) which is different from [37,Lemma 3.4], but we can still using the method in [37,Lemma 3.4] to proof $Z(\pm\infty)$ exist. The proof is trivial, so we omitted it.

Now, we can give the main result in this section.

Theorem 2.3. Suppose $\Re_0>1,$ then for every $c>c^*$ , system (2.1) admits a nontrivial traveling wave solution $(S(x+ct), V(x+ct), I(x+ct))$ satisfying the asymptotic boundary condition (2.5) and (2.6).

Proof. First, it is easy to verify that $S(-\infty) = S_0, V(-\infty) = V_0, I(-\infty) = 0$ by Lemmas 2.4, 2.5 and 2.6.

Next, we will show $(S(\xi), V(\xi), I(\xi)) = (S^*, V^*, I^*)$ as $\xi\rightarrow +\infty$ by using Lyapunov function. From Proposition 2.1 and Lemma 2.13, we have $S(\xi)>0, \ V(\xi)>0$ and $I(\xi)>0$ .

Let $g(x) = x-1-\ln x,$ $\alpha^+(y) = \int_y^{+\infty}J(x)\rm{d} x,$ $\alpha^-(y) = \int_{-\infty}^y J(x)\rm{d} x.$ Since $J$ is compactly supported, and recall that $R$ is the radius of supp $J$ , hence

$\begin{equation}\label{suppJ} \alpha^+(y) \equiv 0\ \ \textrm{and}\ \ \alpha^-(y) \equiv 0\ \ \textrm{for}\ \ \ |y|\geq R. \end{equation}$

(2.19)

Define the following Lyapunov functional

$L(S, V, I)(\xi) = cS^*L_1(\xi)+cV^*L_2(\xi)+cI^*L_3(\xi)+d_1S^*U_1(\xi)+d_2V^*U_2(\xi)+d_3I^*U_3(\xi)$

where

$\begin{align*} L_1(\xi) = &g\left(\frac{S(\xi)}{S^*}\right);\ \ \ L_2(\xi) = g\left(\frac{V(\xi)}{V^*}\right);\\ L_3(\xi) = &g\left(\frac{I(\xi)}{I^*}\right) + (\mu_3+\gamma)I^*\int_0^{c\tau}g\left(\frac{I(\xi-\theta)}{I^*}\right)\rm{d} \theta;\\ U_1(\xi) = &\int_0^{+\infty}\alpha^+(y)g\left(\frac{S(\xi-y)}{S^*}\right)\rm{d} y-\int_{-\infty}^0\alpha^-(y)g\left(\frac{S(\xi-y)}{S^*}\right)\rm{d} y;\\ U_2(\xi) = &\int_0^{+\infty}\alpha^+(y)g\left(\frac{V(\xi-y)}{V^*}\right)\rm{d} y-\int_{-\infty}^0\alpha^-(y)g\left(\frac{V(\xi-y)}{V^*}\right)\rm{d} y;\\ U_3(\xi) = &\int_0^{+\infty}\alpha^+(y)g\left(\frac{I(\xi-y)}{I^*}\right)\rm{d} y-\int_{-\infty}^0\alpha^-(y)g\left(\frac{I(\xi-y)}{I^*}\right)\rm{d} y. \end{align*}$

Thanks to [38,Theorem 1] and $S(\xi)>0$ , $V(\xi)>0$ , $I(\xi)>0$ , we can get that $L_1(\xi)$ , $L_2(\xi)$ and $L_3(\xi)$ are bounded from below. Furthermore, by using (2.19), Proposition 2.1 and Lemma 2.13, we can claim that $U_1(\xi)$ , $U_2(\xi)$ and $U_3(\xi)$ is bounded from below. Thus $L(S, V, I)(\xi)$ is well defined and bounded from below. Note that $\alpha^{\pm} = \frac{1}{2},$ $\frac{\rm{d} \alpha^{+}(y)}{\rm{d} y} = J(y)$ and $\frac{\rm{d} \alpha^{-}(y)}{\rm{d} y} = -J(y)$ , we have

$\begin{align*} \frac{\rm{d} U_1(\xi)}{\rm{d} \xi} = &\frac{\rm{d}}{\rm{d} \xi}\int_0^{+\infty}\alpha^+(y)g\left(\frac{S(\xi-y)}{S^*}\right)\rm{d} y-\frac{\rm{d}}{\rm{d} \xi}\int_{-\infty}^0\alpha^-(y)g\left(\frac{S(\xi-y)}{S^*}\right)\rm{d} y\\ = &\int_0^{+\infty}\alpha^+(y)\frac{\rm{d}}{\rm{d} \xi}g\left(\frac{S(\xi-y)}{S^*}\right)\rm{d} y-\int_{-\infty}^0\alpha^-(y)\frac{\rm{d}}{\rm{d} \xi}g\left(\frac{S(\xi-y)}{S^*}\right)\rm{d} y\\ = &-\int_0^{+\infty}\alpha^+(y)\frac{\rm{d}}{\rm{d} y}g\left(\frac{S(\xi-y)}{S^*}\right)\rm{d} y+\int_{-\infty}^0\alpha^-(y)\frac{\rm{d}}{\rm{d} y}g\left(\frac{S(\xi-y)}{S^*}\right)\rm{d} y\\ = &g\left(\frac{S(\xi)}{S^*}\right)-\int_{-\infty}^{+\infty}J(y)g\left(\frac{S(\xi-y)}{S^*}\right)\rm{d} y. \end{align*}$

Similarly,

$\begin{align*} \frac{\rm{d} U_2(\xi)}{\rm{d} \xi} = g\left(\frac{V(\xi)}{V^*}\right)-\int_{-\infty}^{+\infty}J(y)g\left(\frac{V(\xi-y)}{V^*}\right)\rm{d} y;\\ \frac{\rm{d} U_3(\xi)}{\rm{d} \xi} = g\left(\frac{I(\xi)}{I^*}\right)-\int_{-\infty}^{+\infty}J(y)g\left(\frac{I(\xi-y)}{I^*}\right)\rm{d} y. \end{align*}$

By some calculations, it can be shown that

$\begin{align*} \nonumber\frac{\rm{d}}{\rm{d} \xi}\int_0^{c\tau}g\left(\frac{I(\xi-\theta)}{I^*}\right)\rm{d} \theta = &\int_0^{c\tau}\frac{\rm{d}}{\rm{d} \xi}g\left(\frac{I(\xi-\theta)}{I^*}\right)\rm{d} \theta = -\int_0^{c\tau}\frac{\rm{d}}{\rm{d} \theta}g\left(\frac{I(\xi-\theta)}{I^*}\right)\rm{d} \theta\\ = &\frac{I(\xi)}{I^*}-\frac{I(\xi-c\tau)}{I^*}+\ln \frac{I(\xi-c\tau)}{I(\xi)}. \end{align*}$

Thus

$\begin{align*} \frac{\rm{d} L(\xi)}{\rm{d} \xi} = &\left(1-\frac{S^*}{S(\xi)}\right)(d_1(J*S(\xi)-S(\xi))+\Lambda-\beta_1S(\xi)I(\xi-c\tau)-(\alpha+\mu_1)S(\xi))\\ &+\left(1-\frac{V^*}{V(\xi)}\right)(d_2(J*V(\xi)-V(\xi))+\alpha S(\xi) - \beta_2V(\xi)I(\xi-c\tau)- \mu_2E(\xi))\\ &+\left(1-\frac{I^*}{I(\xi)}\right)(d_3(J*I(\xi)-I(\xi))+\beta_1S(\xi)I(\xi-c\tau)+\beta_2V(\xi)I(\xi-c\tau)-(\gamma+\mu_3)I(\xi))\\ &+(\mu_3+\gamma)I^*\left(\frac{I(\xi)}{I^*}-\frac{I(\xi-c\tau)}{I^*}+\ln \frac{I(\xi-c\tau)}{I(\xi)}\right)\\ &+d_1S^*g\left(\frac{S(\xi)}{S^*}\right)-d_1S^*\int_{-\infty}^{+\infty}J(y)g\left(\frac{S(\xi-y)}{S^*}\right)\rm{d} y\\ &+d_2V^*g\left(\frac{V(\xi)}{V^*}\right)-d_2V^*\int_{-\infty}^{+\infty}J(y)g\left(\frac{V(\xi-y)}{V^*}\right)\rm{d} y\\ &+d_3I^*g\left(\frac{I(\xi)}{I^*}\right)-d_3I^*\int_{-\infty}^{+\infty}J(y)g\left(\frac{I(\xi-y)}{I^*}\right)\rm{d} y\\ \triangleq&B_1+B_2, \end{align*}$

where

$\begin{align*} B_1 = &\left(1-\frac{S^*}{S(\xi)}\right)d_1(J*S(\xi)-S(\xi))+d_1S^*g\left(\frac{S(\xi)}{S^*}\right)-d_1S^*\int_{-\infty}^{+\infty}J(y)g\left(\frac{S(\xi-y)}{S^*}\right)\rm{d} y\\ &+\left(1-\frac{V^*}{V(\xi)}\right)d_2(J*V(\xi)-V(\xi))+d_2V^*g\left(\frac{V(\xi)}{V^*}\right)-d_2V^*\int_{-\infty}^{+\infty}J(y)g\left(\frac{V(\xi-y)}{V^*}\right)\rm{d} y\\ &+\left(1-\frac{I^*}{I(\xi)}\right)d_3(J*I(\xi)-I(\xi))+d_3I^*g\left(\frac{I(\xi)}{I^*}\right)-d_3I^*\int_{-\infty}^{+\infty}J(y)g\left(\frac{I(\xi-y)}{I^*}\right)\rm{d} y, \\ \end{align*}$

and

$\begin{align*} B_2 = &\left(1-\frac{S^*}{S(\xi)}\right)(\Lambda-\beta_1S(\xi)I(\xi-c\tau)-(\alpha+\mu_1)S(\xi))\\ &+\left(1-\frac{V^*}{V(\xi)}\right)(\alpha S(\xi) - \beta_2V(\xi)I(\xi-c\tau)- \mu_2E(\xi))\\ &+\left(1-\frac{I^*}{I(\xi)}\right)(\beta_1S(\xi)I(\xi-c\tau)+\beta_2V(\xi)I(\xi-c\tau)-(\gamma+\mu_3)I(\xi))\\ &+(\mu_3+\gamma)I^*\left(\frac{I(\xi)}{I^*}-\frac{I(\xi-c\tau)}{I^*}+\ln \frac{I(\xi-c\tau)}{I(\xi)}\right). \end{align*}$

For $B_1$ , using $\ln\frac{S(\xi)}{S^*} = \ln\frac{S(\xi-y)}{S^*}-\ln\frac{S(\xi-y)}{S(\xi)},$ thus

$\begin{align*} &\left(1-\frac{S^*}{S(\xi)}\right)d_1(J*S(\xi)-S(\xi))+d_1S^*g\left(\frac{S(\xi)}{S^*}\right)-d_1S^*\int_{-\infty}^{+\infty}J(y)g\left(\frac{S(\xi-y)}{S^*}\right)\rm{d} y\\ = &d_1S^*\int_{-\infty}^{+\infty}J(y)\left[\frac{S(\xi-y)}{S^*}-\frac{S(\xi-y)}{S(\xi)}-\ln \frac{S(\xi)}{S^*}\right]-d_1S^*\int_{-\infty}^{+\infty}J(y)g\left(\frac{S(\xi-y)}{S^*}\right)\rm{d} y\\ = &d_1S^*\int_{-\infty}^{+\infty}J(y)\left[g\left(\frac{S(\xi-y)}{S^*}\right)-g\left(\frac{S(\xi-y)}{S(\xi)}\right)\right]-d_1S^*\int_{-\infty}^{+\infty}J(y)g\left(\frac{S(\xi-y)}{S^*}\right)\rm{d} y\\ = &-d_1S^*\int_{-\infty}^{+\infty}J(y)g\left(\frac{S(\xi-y)}{S(\xi)}\right)\rm{d} y. \end{align*}$

Then

$\begin{align} \nonumber B_1 = &-d_1S^*\int_{-\infty}^{+\infty}J(y)g\left(\frac{S(\xi-y)}{S(\xi)}\right)\rm{d} y-d_2V^*\int_{-\infty}^{+\infty}J(y)g\left(\frac{V(\xi-y)}{V(\xi)}\right)\rm{d} y\\ &-d_3I^*\int_{-\infty}^{+\infty}J(y)g\left(\frac{I(\xi-y)}{I(\xi)}\right)\rm{d} y. \end{align}$

(2.20)

For $B_2$ , by some calculation yields

$\begin{align*} B_2 = &\mu_1S^*\left(2-\frac{S(\xi)}{S^*}-\frac{S^*}{S(\xi)}\right)-\beta_1S^*I^*g\left(\frac{S(\xi)I(\xi-c\tau)}{S^*I(\xi)}\right)\\ &-\beta_2V^*I^*\left[g\left(\frac{V(\xi)I(\xi-c\tau)}{V^*I(\xi)}\right)+g\left(\frac{S(\xi)V^*}{S^*V(\xi)}\right)\right]\\ &-\mu_2V^*\left[g\left(\frac{V(\xi)}{V^*}\right)+g\left(\frac{S(\xi)V^*}{S^*V(\xi)}\right)\right]\\ &-(\alpha S^*+\beta_1S^*I^*)g\left(\frac{S^*}{S(\xi)}\right), \end{align*}$

here we use $(\mu_3+\gamma)I^* = \beta_1S^*I^*+\beta_2V^*I^*$ and $\alpha S(\xi)\frac{V^*}{V(\xi)} = (\beta_2V^*I^*+\mu_2V^*)\frac{S(\xi)V^*}{S^*V(\xi)}.$ Combining $B_1$ and $B_2,$ we obtain $L(\xi)$ is decreasing in $\xi$ .

Consider an increasing sequence $\{\xi_n\}_{n\geq 0}$ with $\xi_n>0$ such that $\xi_n\rightarrow+\infty$ when $n\rightarrow+\infty$ and denote

$\{S_n(\xi) = S(\xi+\xi_n)\}_{n\geq 0}, \ \ \{V_n(\xi) = V(\xi+\xi_n)\}_{n\geq 0, }\ \ \textrm{and}\ \ \{I_n(\xi) = I(\xi+\xi_n)\}_{n\geq 0}.$

We can assume that $S_n, \ V_n$ and $I_n$ converge to some nonnegative functions $S_\infty, \ V_\infty$ and $I_\infty.$ Furthermore, since $L(S, V, I)(\xi)$ is non-increasing on $\xi$ , then there exists a constant $\hat{C}$ and large $n$ such that

$\hat{C}\leq L(S_n, V_n, I_n)(\xi) = L(S, V, I)(\xi+\xi_n)\leq L(S, V, I)(\xi).$

Therefore there exists some $\tilde{\rm{d}elta}\in {\rm IR}$ such that $\lim_{n\rightarrow\infty} L(S_n, V_n, I_n)(\xi) = \tilde{\rm{d}elta}$ for any $\xi\in {\rm IR}$ . By Lebegue dominated convergence theorem, gives us

$\lim\limits_{n\rightarrow+\infty}L(S_n, V_n, I_n)(\xi) = L(S_\infty, V_\infty, I_\infty)(\xi), \ \xi\in {\rm IR}.$

Thus

$L(S_\infty, V_\infty, I_\infty)(\xi) = \tilde{\rm{d}elta}.$

Note that $\frac{\rm{d} L}{\rm{d} \xi} = 0$ if and only if $S(\xi)\equiv S^*$ , $V(\xi)\equiv V^*$ and $I(\xi)\equiv I^*$ , it follows that

$(S_\infty, V_\infty, I_\infty)\equiv (S^*, V^*, I^*).$

This completes the proof.

3. Existence of traveling wave solutions for $c=c^*$

In this section, we investigate the existence of traveling wave solutions for the case $c = c^*$ by a limiting argument(see ^[23,39]).

Theorem 3.1. Suppose $\Re_0>1,$ then for every $c = c^*$ , system (2.1) admits a nontrivial traveling wave solution $(S(x+c^*t), V(x+c^*t), I(x+c^*t))$ satisfying

$\lim\limits_{\xi\rightarrow+\infty}(S(\xi), V(\xi), I(\xi)) = (S^*, V^*, I^*).$

Furthermore, if we assume that $S(-\infty)$ and $V(-\infty)$ exist, then $(S(x+c^*t), V(x+c^*t), I(x+c^*t))$ also satisfying

$\lim\limits_{\xi\rightarrow-\infty}(S(\xi), V(\xi), I(\xi)) = (S_0, V_0, 0).$

Proof. Let $\{c_n\}\subset(c^*, c^*+1)$ be a decreasing sequence such that $\lim\limits_{n\rightarrow\infty}c_n = c^*$ . Then for each $c_n$ , there exists a traveling wave solution $(S_n(\cdot), V_n(\cdot), I_n(\cdot))$ of system (2.4) with asymptotic boundary condition (2.5) and (2.6). Since $(S_n(\cdot+a), V_n(\cdot+a), I_n(\cdot+a))$ are also solutions of (2.4) for any $a\in{\rm IR}$ , we can assume that

$I_n(0) = \rm{d}elta^*, \ \ I_n(\xi)\leq\rm{d}elta^*, \ \ \xi \lt 0$

with $0<\rm{d}elta<I^*$ is small enough.

Similar to ^[23,39], we can find a subsequence of $(S_n, V_n, I_n)$ , again denoted by $(S_n, V_n, I_n)$ , such that $(S_n, V_n, I_n)$ and $(S'_n, V'_n, I'_n)$ converge uniformly on every bounded interval to function $(S, V, I)$ and $(S', V', I')$ , respectively. Applying the Lebesgue dominated convergence theorem, it then follows that

$\lim\limits_{n\rightarrow\infty}J*S_n = J*S, \ \ \lim\limits_{n\rightarrow\infty}J*V_n = J*V, \ \ \textrm{and}\ \ \lim\limits_{n\rightarrow\infty}J*I_n = J*I$

on every bounded interval. Then we get that $(S, V, I)$ satisfies system (2.4). From the proof of Theorem 2.3, the Lyapunov functional is independent of $c$ . By the same argument in the proof of Theorem 2.3, we claim that $I(\xi)>0$ for any $\xi\in{\rm IR}$ . Hence, we can still get that

$\lim\limits_{\xi\rightarrow+\infty}S(\xi) = S^*, \ \ \lim\limits_{\xi\rightarrow+\infty}V(\xi) = V^*, \ \ \lim\limits_{\xi\rightarrow+\infty}I(\xi) = I^*.$

Moreover, we have

$I(0) = \rm{d}elta^*, \ \ I(\xi)\leq\rm{d}elta^*, \ \ \xi \lt 0.$

Let

$S_{sup} = \limsup\limits_{\xi\rightarrow-\infty}S(\xi), \ \ V_{sup} = \limsup\limits_{\xi\rightarrow-\infty}V(\xi), \ \ I_{sup} = \limsup\limits_{\xi\rightarrow-\infty}I(\xi)$

and

$S_{inf} = \liminf\limits_{\xi\rightarrow-\infty}S(\xi), \ \ V_{inf} = \liminf\limits_{\xi\rightarrow-\infty}V(\xi), \ \ I_{inf} = \liminf\limits_{\xi\rightarrow-\infty}I(\xi).$

Next, we show that $I(-\infty)$ exists. By way of contradiction, assume that $I_{inf} < I_{sup}$ . Then there exist sequences $\{x_n\}$ and $\{y_n\}$ satisfying $x_n, \ y_n\rightarrow-\infty$ as $n\rightarrow+\infty$ such that

$\lim\limits_{n\rightarrow+\infty}I(x_n) = I_{inf}\ \ \textrm{}\ \ \lim\limits_{n\rightarrow+\infty}I(y_n) = I_{sup}.$

Since we assumed that $S(-\infty)$ and $V(-\infty)$ exist, then $S_{sup} = S_{inf} = S(-\infty)$ and $V_{sup} = V_{inf} = V(-\infty)$ . From [40,Lemma 2.3], we can obtain that $S'(-\infty) = 0$ and $V'(-\infty) = 0$ . For any sequence $\{\xi_n\}$ , $\xi_n\rightarrow -\infty$ as $n\rightarrow+\infty$ , using Fatou Lemma, one have that

$S(-\infty)\leq\liminf\limits_{n\rightarrow\infty}J*S(\xi_n)\leq\limsup\limits_{n\rightarrow\infty}J*S(\xi_n)\leq S(-\infty).$

and

$V(-\infty)\leq\liminf\limits_{n\rightarrow\infty}J*V(\xi_n)\leq\limsup\limits_{n\rightarrow\infty}J*V(\xi_n)\leq V(-\infty).$

Thus, we have

$\lim\limits_{n\rightarrow\infty}[J*S(\xi_n)-S(\xi_n)] = 0\ \ \textrm{and}\ \ \lim\limits_{n\rightarrow\infty}[J*V(\xi_n)-V(\xi_n)] = 0$

Taking $\xi = x_n$ and $\xi = y_n$ in the first equation of system 2.4, and letting $n\rightarrow\infty$ , we obtain that $I_{inf} = I_{sup}$ , which is a contradiction. Hence, $I(-\infty)$ exists and $I(-\infty)<\rm{d}elta^*$ . From system (2.4) and [40,Lemma 2.3], we obtain

$\begin{equation} \left\{ \begin{array}{l} \displaystyle \Lambda - \beta_1 S(-\infty)I(-\infty) - \alpha S(-\infty) - \mu_1 S(-\infty = 0), \\ \displaystyle \alpha S(-\infty)- \beta_2 V(-\infty)I(-\infty) - \mu_2 V(-\infty) = 0, \\ \displaystyle \beta_1 S(-\infty)I(-\infty) + \beta_2 V(-\infty)I(-\infty)) - \gamma I(-\infty) - \mu_3 I(-\infty) = 0. \end{array}\right. \end{equation}$

(3.1)

In the view of $\rm{d}elta^*<I^*$ , it follows that

$\lim\limits_{\xi\rightarrow-\infty}S(\xi) = S_0, \ \ \lim\limits_{\xi\rightarrow-\infty}V(\xi) = V_0, \ \ \lim\limits_{\xi\rightarrow-\infty}I(\xi) = 0.$

This completes the proof.

Remark 3.1. For the case $c = c^*$ , there is a priori condition assuming $S(-\infty)$ and $V(-\infty)$ exist. This condition is only necessary for the difficulty in mathematics. In ^[34], the authors have given some results for the case $c = c^*$ in a nonlocal diffusive SIR model without constant recruitment, but some estimates is much more difficult for our model with constant recruitment and time delay as in [34,Section 3]. Thus, how to extend the methods in ^[34] to our model, it will be an interesting problem for further investigation.

4. Nonexistence of traveling wave solutions

In this section, we show the nonexistence of traveling waves when $\Re_0>1$ with $0<c<c^*$ .

Theorem 4.1. If $\Re_0>1$ and $0<c<c^*$ , then there exists no nontrivial positive solutions of (2.4) with (2.5) and (2.6).

Proof. Since $\Re_0>1$ gives us $\beta_1S_0+\beta_2V_0>\mu_3+\gamma$ . Assume there exists nontrivial positive solution $(S, V, I)$ of (2.4) with (2.5) and (2.6). Then there exists a positive constant $K>0$ large enough such that, for any $\xi<-K$ , we have

$\begin{equation}\label{Equ1} c I'(\xi) \geq d_3(J*I(\xi) - I(\xi)) +\frac{\beta_1S_0 + \beta_2V_0 - (\gamma + \mu_3)}{2}I(\xi-c\tau) +(\gamma+\mu_3)(I(\xi-c\tau) - I(\xi)) \end{equation}$

(4.1)

holds. Let $K(\xi) = \int_{-\infty}^\xi I(\eta)\rm{d} \eta$ . By Fubini theorem, thus

$\begin{align}\label{Equ2} d_3\int_{-\infty}^{\xi}J * I(s)\rm{d} s = &d_3\int_{-\infty}^{\xi} \int_{{\rm IR}} J(y) I(s-y)\rm{d} y\rm{d} s\\ \nonumber = &d_3\int_{{\rm IR}} \int_{-\infty}^{\xi} J(y) I(s-y)\rm{d} s\rm{d} y\\ \nonumber = &d_3\int_{{\rm IR}} J(y) \int_{-\infty}^{\xi} I(s-y)\rm{d} s\rm{d} y\\ \nonumber = & d_3 J * K(\xi).\nonumber \end{align}$

(4.2)

Integrating the both sides of (4.1) from $-\infty$ to $\xi$ with $\xi\leq-K$ , we have

$\begin{align}\label{Equ3} \nonumber cI(\xi) \geq &d_3(J*K(\xi) - K(\xi)) + (\gamma + \mu_3)[K(\xi-c\tau) - K(\xi)]\\ & + \frac{\beta_1S_0 + \beta_2V_0 - (\gamma + \mu_3)}{2} K(\xi-c\tau). \end{align}$

(4.3)

Furthermore, the following two equations hold.

$\begin{align}\label{Equ4} \nonumber\int_{-\infty}^\xi[K(\eta-c\tau) - K(\eta)]\rm{d} \eta = &\int_{-\infty}^\xi (-c\tau) \int_0^1\frac{\partial K(\eta-c\tau s)}{\partial s}\rm{d} s\rm{d} \eta\\ = &- c\tau \int_0^1 K(\xi-c\tau s)\rm{d} s \end{align}$

(4.4)

and

$\begin{align}\label{Equ5} d_3\nonumber\int_{-\infty}^\xi[J*K(\eta) - K(\eta)]\rm{d} \eta = &d_3\int_{-\infty}^\xi \int_{-\infty}^{+\infty}(-x)J(x)\int_0^1\frac{\partial K(\eta-x s)}{\partial s}\rm{d} s\rm{d} x\rm{d} \eta\\ = &d_3\int_{-\infty}^{+\infty} (-x) J(x)\int_0^1 K(\xi-x s)\rm{d} s \rm{d} x. \end{align}$

(4.5)

Integrating both sides of inequality (4.3) from $-\infty$ to $\xi$ , and combining Equations (4.4) and (4.5) yield

$\begin{align}\label{Equ6} \nonumber&\frac{\beta_1S_0 + \beta_2V_0 - (\gamma + \mu_3)}{2} \int_{-\infty}^\xi K(\eta-c\tau)\rm{d} \eta\\ \nonumber\leq&c K(\xi) + (\gamma+\mu_3)c\tau \int_0^1 K(\xi-c\tau s)\rm{d} s\\ \nonumber& + d_3\int_{-\infty}^{+\infty} x J(x)\int_0^1 K(\xi-x s)\rm{d} s \rm{d} x\\ \leq&\left(c+d_3\int_{{\rm IR}}xJ(x)\rm{d} x+(\gamma+\mu_3)c\tau\right)K(\xi), \end{align}$

(4.6)

Here we use $xK(\xi-sx)$ as a non-increasing function with $s\in(0, 1)$ . By (J1) of Assumption 1.1, we have $\int_{{\rm IR}} x J(x)\rm{d} x = 0$ . Then for $\xi<-K,$ we have

$\begin{align}\label{Equ7} \nonumber&\frac{\beta_1S_0 + \beta_2V_0 - (\gamma + \mu_3)}{2} \int_0^{+\infty} K(\xi - \eta - c\tau)\rm{d} \eta\\ \leq&(c+(\gamma+\mu_3)c\tau)K(\xi), \end{align}$

(4.7)

For the non-decreasing function $K(\xi),$ there exists some $\tilde{\eta}$ with $\tilde{\eta} + c\tau > 0$ such that

$\begin{align}\label{Equ8} \nonumber&\frac{\beta_1S_0 + \beta_2V_0 - (\gamma + \mu_3)}{2} (\tilde{\eta} + c\tau) K(\xi - \tilde{\eta} - c\tau)\\ \leq&(c+(\gamma+\mu_3)c\tau)K(\xi), \end{align}$

(4.8)

Thus there exists a sufficiently large constant $\theta>-c\tau$ and some constant $\varepsilon\in (0, 1),$ such that

$K(\xi-\theta -c\tau)\leq\varepsilon K(\xi), \ \ \xi\leq-M.$

Let

$p(\xi) = K(\xi)e^{-\nu\xi},$

where

$0 \lt \nu\triangleq\frac{1}{\theta+c\tau}\ln \frac{1}{\varepsilon} \lt \lambda_c,$

By some simple calculation, we have

$p(\xi-\theta-c\tau)\leq p(\xi).$

Using L'Hospital's rule yields

$\lim\limits_{\xi\rightarrow+\infty}p(\xi) = \lim\limits_{\xi\rightarrow+\infty}\frac{K(\xi)}{e^{\nu\xi}} = \lim\limits_{\xi\rightarrow+\infty}\frac{I(\xi)}{\nu e^{\nu\xi}} = 0,$

Note that $p(\xi)\geq 0$ , thus there exists a constant $p_0$ such that

$\begin{align}\label{Equ9} p(\xi) = K(\xi)e^{-\nu\xi}\leq p_0, \ \ \xi\in {\rm IR}. \end{align}$

(4.9)

On the other hand, since $S(\xi)\leq S_0$ and $V(\xi)\leq V_0$ for $\xi\in{\rm IR}$ , recall the third equation of (2.4), we have

$\begin{align}\label{Equ91} \nonumber cI'(\xi) = &d_3(J*I(\xi)-I(\xi)) + \beta_1 S(\xi)I(\xi-c\tau) + \beta_2 V(\xi)I(\xi-c\tau) - \gamma I(\xi) - \mu_3 I(\xi)\\ \leq&d_3(J*I(\xi)-I(\xi)) + \beta_1 S_0I(\xi-c\tau) + \beta_2 V_0I(\xi-c\tau) - \gamma I(\xi) - \mu_3 I(\xi). \end{align}$

(4.10)

Integrating the both sides of (4.10) from $-\infty$ to $\xi$ yields

$\begin{align}\label{Equ10} cI(\xi)\leq d_3J*K(\xi)-(\gamma+\mu_3+d_3)K(\xi) + (\beta_1S_0+\beta_2V_0)K(\xi-c\tau). \end{align}$

(4.11)

From (4.9), using $J$ is compactly supported, for $\xi\in{\rm IR},$ there exists a positive constant $M_1$ such that

$\begin{align}\label{Equ11} \nonumber (d_3J*K(\xi))e^{-\nu \xi} = &d_3\int_{{\rm IR}}J(y)e^{-\nu \xi}K(\xi-y)\rm{d} y\\ = & d_3\int_{{\rm IR}}J(y)e^{-\nu y}K(\xi-y) e^{-\nu (\xi-y)}\rm{d} y\\ \nonumber\leq& d_3p_0\int_{{\rm IR}} J(y)e^{-\nu y} \rm{d} y\\ \nonumber\leq&M_1. \end{align}$

(4.12)

Thus there exists a constant $M_2>0$ such that

$\begin{align} I(\xi) e^{-\nu \xi}\leq M_2, \ \ \xi\in{\rm IR}, \end{align}$

(4.13)

since (4.9), (4.11) and (4.12) hold. Then

$\begin{align} \sup\limits_{\xi\in{\rm IR}}\{I(\xi) e^{-\nu \xi}\} \lt +\infty. \end{align}$

(4.14)

By the same procedure in (4.12), there exists a positive constant $M_2$ such that

$\begin{align}\label{Equ12} (d_3J*I(\xi))e^{-\nu \xi}\leq&M_2. \end{align}$

(4.15)

Hence

$\begin{align} \sup\limits_{\xi\in{\rm IR}}\{I'(\xi) e^{-\nu \xi}\} \lt +\infty. \end{align}$

(4.16)

For $\lambda\in{\rm IC}$ with $0<\textrm{Re}\lambda<\nu,$ define the following two-side Laplace transform of $I(\xi)$ ,

$\begin{align} \nonumber \mathcal{L}_I(\lambda):& = \int_{{\rm IR}}I(\xi)e^{-\lambda \xi}\rm{d} \xi. \end{align}$

From (2.4), we have

$\begin{align} \nonumber &d_3(J*I(\xi)-I(\xi)) - cI'(\xi) + (\beta_1 S_0 + \beta_2V_0) I(\xi-c\tau) - (\gamma+\mu_3) I(\xi)\\ = &\beta_1 (S_0 - S(\xi))I(\xi-c\tau) + \beta_2 (V_0 - V(\xi))I(\xi-c\tau). \end{align}$

(4.17)

Take the two-side Laplace transform to the above equation, thus

$\begin{align}\label{Equ13} \Delta(\lambda, c)\mathcal{L}_I(\lambda) = \int_{\rm IR}e^{-\lambda \xi}[\beta_1 (S_0 - S(\xi))I(\xi-c\tau) + \beta_2 (V_0 - V(\xi))I(\xi-c\tau)]\rm{d} \xi \end{align}$

(4.18)

for $\lambda \in {\rm IC}$ with $0<\textrm{Re}\lambda<\nu$ . Let $L(\xi) = S_0 -S(\xi)$ , we have $0\leq L(\xi)\leq S_0$ and $\lim_{\xi\rightarrow -\infty} L(\xi) = 0$ . Then from the first equation of (2.4), we have

$c L'(\xi) = d_1 (J*L(\xi)-L(\xi)) + \beta_1 S_(\xi)I(\xi-c\tau) + (\alpha+\mu_1)S(\xi).$

Let $\eta\in C^{\infty}({\rm IR}, [0, 1])$ be a nonnegative nondecreasing function, $\eta(x)\equiv0$ in $(-\infty, -2]$ and $\eta(x)\equiv1$ in $[-1, +\infty)$ . For $N\in{\rm IN}$ , set $\eta_N = \eta\left(\frac{x}{N}\right)$ . Then, taking $0\leq \nu_0\leq\nu$ , we have

$c \int_{{\rm IR}}L'(\xi)e^{-\nu_0 \xi}\eta_N\rm{d} \xi = d_1\int_{{\rm IR}}(J*L(\xi)-L(\xi))e^{-\nu_0 \xi}\eta_N\rm{d} \xi + \int_{{\rm IR}}S(\xi)[\beta_1I(\xi-c\tau) + \alpha + \mu_1] e^{-\nu_0 \xi}\eta_N\rm{d} \xi.$

By the argument in [22,Theorem 3.1], there exists a constant $\Xi>0$ dependent on $\nu_0$ such that

$\int_{{\rm IR}}L(\xi)e^{-\nu_0 \xi}\rm{d} \xi \leq \Xi.$

Thus,

$\int_{{\rm IR}}\beta_1(S_0 - S(\xi))I(\xi-c\tau)e^{-(\nu+\nu_0)\xi}\rm{d} \xi\leq \beta_1\sup\limits_{\xi\in{\rm IR}}\{I(\xi) e^{-\nu \xi}\}\int_{{\rm IR}}L(\xi)e^{-\nu_0 \xi}\rm{d} \xi \lt \infty.$

Similarly,

$\int_{{\rm IR}}\beta_2(V_0 - V(\xi))I(\xi-c\tau)e^{-(\nu+\nu_0)\xi}\rm{d} \xi \lt \infty.$

From the property of Laplace transform ^[41], $\mathcal{L}_I(\lambda)$ is well defined with $\textrm{Re}\lambda>0$ . Note that Equation (4.18) can be rewritten as

$\begin{align}\label{Equ16} \int_{\rm IR}e^{-\lambda \xi}\left[\Delta(\lambda, c)I(\xi)+\beta_1 (S_0 - S(\xi))I(\xi-c\tau) + \beta_2 (V_0 - V(\xi))I(\xi-c\tau)\right]\rm{d} \xi = 0. \end{align}$

(4.19)

Recall (J2) of Assumption 1.1, then $\Delta(\lambda, c)\rightarrow+\infty$ as $\xi\rightarrow+\infty$ for $c\in(0, c^*)$ which is a contradiction of (4.19). This completes the proof.

5. Discussion

As traveling wave solutions describe the transition from disease-free equilibrium to endemic equilibrium when the wave speed is larger than the minimal wave speed. Now, we focus on how the parameters in system (2.1) can affect the wave speed. Suppose $(\hat{\lambda}, \hat{c})$ be a zero root of $\Delta(\lambda, c)$ , recall that $V_0 = \frac{\Lambda\alpha}{\mu_2(\mu_1+\alpha)}$ and $\mu_2 = \mu_1 +\gamma_1$ , we have

$\Delta(\hat{\lambda}, \hat{c}) = d_3\int_{{\rm IR}}J(x)e^{-\hat{\lambda} x}\rm{d} x-(d_3+\gamma+\mu_3)-\hat{c}\hat{\lambda}+\beta_1S_0e^{-\hat{c}\tau\hat{\lambda}}+\frac{\beta_2\Lambda\alpha}{(\mu_1 +\gamma_1)(\mu_1+\alpha)}e^{-\hat{c}\tau\hat{\lambda}} = 0.$

By some calculations, we obtain

$\frac{\rm{d} \hat{c}}{\rm{d} d_3} = \frac{\int_{{\rm IR}}J(x)[e^{-\hat\lambda x}-1]\rm{d} x}{\hat\lambda(1+ [\beta_1 S_0 + \beta_2 V_0] \tau e^{-\hat{c}\tau\hat{\lambda}})} \gt 0, \ \ \frac{\rm{d} \hat{c}}{\rm{d} \tau} = -\frac{\beta_1S_0 + \beta_2V_0}{e^{\hat{c}\tau\hat{\lambda}} + \beta_1S_0\tau + \beta_2V_0\tau} \lt 0,$

$\frac{\rm{d} \hat{c}}{\rm{d} \beta_1} = \frac{S_0e^{-\hat{c}\tau\hat{\lambda}}}{\hat\lambda(1+ [\beta_1 S_0 + \beta_2 V_0] \tau e^{-\hat{c}\tau\hat{\lambda}})} \gt 0, \ \ \frac{\rm{d} \hat{c}}{\rm{d} \beta_2} = \frac{V_0e^{-\hat{c}\tau\hat{\lambda}}}{\hat\lambda(1+ [\beta_1 S_0 + \beta_2 V_0] \tau e^{-\hat{c}\tau\hat{\lambda}})} \gt 0,$

and

$\frac{\rm{d} \hat{c}}{\rm{d} \gamma_1} = -\frac{\beta_2V_0 e^{-\hat{c}\tau\hat{\lambda}}}{(\mu_1+\gamma_1)\hat\lambda(1+ [\beta_1 S_0 + \beta_2 V_0] \tau e^{-\hat{c}\tau\hat{\lambda}})} \lt 0,$

that is, $\hat{c}$ is a decreasing function on $\gamma_1$ and $\tau$ , while $\hat{c}$ is an increasing function on $d_3$ , $\beta_1$ and $\beta_2$ . From the biological point of view, this indicates the following four scenarios:

Ⅰ. The more successful the vaccination, the slower the disease spreads;

Ⅱ. The longer the latent period, the slower the disease spreads;

Ⅲ. The faster infected individuals move, the faster the disease spreads;

Ⅳ. The more effective the infections are, the faster the disease spreads.

Now, we are in a position to make the following summary:

Mathematically, we investigated a nonlocal dispersal epidemic model with vaccination and delay; The existence of traveling wave solutions is studied by applying Schauder fixed point theorem with upper-lower solutions, that is there exists traveling wave solutions when $\Re_0>1$ with $c>c^*$ . Furthermore, the boundary asymptotic behaviour of traveling wave solutions at $+\infty$ was established by the methods of constructing suitable Lyapunov like function. We also showed that there exists traveling wave solutions when $\Re_0>1$ with $c = c^*$ . Finally, we proved the nonexistence of traveling wave solutions under the assumptions $\Re_0>1$ and $0<c<c^*$ .

Biologically, our results imply that the nonlocal dispersal and infection ability of infected individuals can accelerate the spreading of infectious disease, while the latent period and successful rate of vaccination can slow down the disease spreads.

Acknowledgments

The authors are very grateful to the editors and three reviewers for their valuable comments and suggestions that have helped us improving the presentation of this paper. We would also very grateful to Prof.Shigui Ruan, Dr. Sanhong Liu and Dr.Wen-Bing Xu for their valuable comments and helpful advice. This work is supported by Natural Science Foundation of China (No.11871179; No.11771374), and the first author was also partially supported by China Scholarship Council (No.201706120216). R. Zhang acknowledges the kind hospitality received from the Department of Mathematics at the University of Miami, where part of the work was completed.

Conflict of interest

All authors declare no conflicts of interest in this paper.

Appendix A: Proof of Lemma 2.4

Proof. If $\xi>\mathfrak{X}_1$ , then $\underline{S}(\xi) = 0,$ equation (2.11) holds. If $\xi<\mathfrak{X}_1$ , then $\underline{S}(\xi) = S_0-M_1 e^{\varepsilon_1 \xi},$ we have

$\begin{align*} &c{\underline{S}}'(\xi)- d_1(J*\underline{S}(\xi)-\underline{S}(\xi)) - \Lambda + \beta_1\underline{S}(\xi)\overline{I}(\xi-c\tau) +(\mu_1+\alpha) \underline{S}(\xi)\\ = &-c\varepsilon_1M_1e^{\varepsilon_1\xi}+d_1M_1e^{\varepsilon_1\xi}\int_{{\rm IR}}J(x)e^{-\varepsilon_1x}\rm{d} x-d_1M_1e^{\varepsilon_1\xi}-\Lambda\\ &+\beta_1(S_0-M_1e^{\varepsilon_1\xi})e^{\lambda_c(\xi-c\tau)}+(\mu_1+\alpha)(S_0-M_1 e^{\varepsilon_1 \xi})\\ \leq&e^{\varepsilon_1\xi}\left[-c\varepsilon_1M_1e^{\varepsilon_1\xi}+d_1M_1e^{\varepsilon_1\xi}\int_{{\rm IR}}J(x)e^{-\varepsilon_1x}\rm{d} x-d_1M_1e^{\varepsilon_1\xi}+\beta_1S_0\left(\frac{S_0}{M_1}\right)^{\frac{\lambda-\varepsilon_1}{\varepsilon_1}}\right]. \end{align*}$

Here we use

$e^{(\lambda_c-\varepsilon_1)\xi} \lt \left(\frac{S_0}{M_1}\right)^{\frac{\lambda_c-\varepsilon_1}{\varepsilon_1}}\ \ \ \textrm{for}\ \ \ \xi \lt \mathfrak{X}_1.$

Keeping $\varepsilon_1M_1 = 1$ , letting $M_1\rightarrow+\infty$ for some $M_1>S_0$ large enough and $\varepsilon_1$ small enough, we have

$\begin{equation*} c{\underline{S}}'(\xi)- d_1(J*\underline{S}(\xi)-\underline{S}(\xi)) - \Lambda + \beta_1\underline{S}(\xi)\overline{I}(\xi-c\tau) +(\mu_1+\alpha) \underline{S}(\xi)\leq0. \end{equation*}$

This completes the proof.

Appendix B: Proof of Lemma 2.6

Proof. If $\xi > \frac{1}{\varepsilon_3}\ln \frac{1}{M_3}$ , the Equation (2.13) holds since $\underline{I}(\xi) = 0$ . If $\xi < \frac{1}{\varepsilon_3}\ln \frac{1}{M_3}$ , then $\underline{I}(\xi) = e^{\lambda_c\xi}(1-M_3e^{\varepsilon_3 \xi})$ , we have the following four cases.

Case Ⅰ: $\xi>\max\{\mathfrak{X}_1, \mathfrak{X}_2\}$ .

In this case, $\underline{S}(\xi) = \underline{V}(\xi) = 0$ . Thus, Equation (2.13) is equivalent to

$c{\underline{I}}'(\xi) \leq d_3(J*\underline{I}(\xi)-\underline{I}(\xi)) - \gamma \underline{I}(\xi) - \mu_3 \underline{I}(\xi),$

that is

$\begin{align*} &c\lambda_c-d_3\int_{{\rm IR}}J(y)e^{-\lambda_cy}\rm{d} y+d_3+\gamma+\mu_3\\ \leq& M_3e^{\varepsilon_3\xi}\left[c(\lambda+\varepsilon_3)-d_3\int_{{\rm IR}}J(y)e^{-(\lambda_c+\varepsilon_3)y}\rm{d} y+d_3+\gamma+\mu_3\right]. \end{align*}$

From $\Delta(\lambda_c, c) = 0$ and $\Delta(\lambda_c+\varepsilon_3, c)<0,$ we have

$\beta_1S_0e^{-c\tau\lambda_c}+\beta_2V_0e^{-c\tau\lambda_c}\\ \leq M_3e^{\varepsilon_3\xi}\left[-\Delta(\lambda_c+\varepsilon_3, c)+\beta_1S_0e^{-c\tau(\lambda_c+\varepsilon_3)}+\beta_2V_0e^{-c\tau(\lambda_c+\varepsilon_3)}\right],$

Because $\tau>0,$ $\lambda_c>0,$ it suffices to prove

$\begin{align*} \beta_1S_0+\beta_2V_0\leq M_3e^{\varepsilon_3\xi}\left[-\Delta(\lambda_c+\varepsilon_3, c)+\beta_1S_0e^{-c\tau(\lambda_c+\varepsilon_3)}+\beta_2V_0e^{-c\tau(\lambda_c+\varepsilon_3)}\right]. \end{align*}$

Since $\xi>\max\{\mathfrak{X}_1, \mathfrak{X}_2\},$ $M_3>\max\{S_0, V_0\}$ and $0<\varepsilon_3<\min\{\varepsilon_1/2, \varepsilon_2/2\},$ note that $e^{\varepsilon_3\xi}\geq\left(\frac{S_0}{M_1}\right)^{\frac{1}{2}}\left(\frac{V_0}{M_2}\right)^{\frac{1}{2}},$ then we only need to ensure

$\beta_1S_0+\beta_2V_0\leq-\Delta(\lambda_c+\varepsilon_3, c)M_3\left(\frac{S_0}{M_1}\right)^{\frac{1}{2}}\left(\frac{V_0}{M_2}\right)^{\frac{1}{2}}.$

Thus, Equation (2.13) holds for sufficiently large $M_3>0$ with

$M_3\geq \frac{\beta_1S_0+\beta_2V_0}{-\Delta(\lambda_c+\varepsilon_3, c)}\sqrt{\frac{S_0}{M_1}}\sqrt{\frac{V_0}{M_2}}\triangleq\Pi_1.$

Case Ⅱ: $\mathfrak{X}_1>\xi>\mathfrak{X}_2$ .

In this case, $\underline{S}(\xi) = S_0-M_1e^{\varepsilon_1\xi}$ and $\underline{V}(\xi) = 0.$ Hence, Equation (2.13) is equivalent to

$c{\underline{I}}'(\xi) \leq d_3(J*\underline{I}(\xi)-\underline{I}(\xi)) - \gamma \underline{I}(\xi) - \mu_3 \underline{I}(\xi) + \beta_1\underline{S}(\xi)\underline{I}(\xi-c\tau),$

that is

$\begin{align*} &c\lambda_c-d_3\int_{{\rm IR}}J(y)e^{-\lambda_cy}\rm{d} y+d_3+\gamma+\mu_3-\beta_1S_0e^{-\lambda_cc\tau}+\beta_1M_1e^{\varepsilon_1\xi-\lambda_cc\tau}\\ \leq& M_3e^{\varepsilon_3\xi}\left[c(\lambda+\varepsilon_3)-d_3\int_{{\rm IR}}J(y)e^{-(\lambda_c+\varepsilon_3)y}\rm{d} y+d_3+\gamma+\mu_3-\beta_1S_0e^{-(\varepsilon_3+\lambda_c)c\tau}+\beta_1M_1e^{\varepsilon_1\xi-(\varepsilon_3+\lambda_c)c\tau}\right], \end{align*}$

we need to prove

$\begin{equation*} \beta V_0\leq -\Delta(\lambda_c+\varepsilon_3, c)M_3e^{\varepsilon_3\xi}. \end{equation*}$

Choose $M_3$ large enough with

$M_3\geq \frac{\beta_2 \sqrt{V_0M_2}}{-\Delta(\lambda_c+\varepsilon_3, c)}\triangleq\Pi_2.$

Case Ⅲ: $\mathfrak{X}_2>\xi>\mathfrak{X}_1$ .

In this case, $\underline{V}(\xi) = V_0-M_2e^{\varepsilon_2\xi}$ and $\underline{S}(\xi) = 0.$ Similar to Case Ⅱ, Equation (2.13) holds if we choose

$M_3\geq \frac{\beta_1 \sqrt{S_0M_1}}{-\Delta(\lambda_c+\varepsilon_3, c)}\triangleq\Pi_3$

large enough.

Case Ⅵ: $\xi<\min\{\mathfrak{X}_1, \mathfrak{X}_2\}$ .

In this case, $\underline{S}(\xi) = S_0-M_1e^{\varepsilon_1\xi}$ and $\underline{V}(\xi) = V_0-M_2e^{\varepsilon_2\xi}$ , Equation (2.13) is equivalent to

$c{\underline{I}}'(\xi) \leq d_3(J*\underline{I}(\xi)-\underline{I}(\xi)) - \gamma \underline{I}(\xi) - \mu_3 \underline{I}(\xi) + \beta_1\underline{S}(\xi)\underline{I}(\xi-c\tau) + \beta_2\underline{V}(\xi)\underline{I}(\xi-c\tau),$

that is

$\begin{align*} c\lambda_c-d_3&\int_{{\rm IR}}J(y)e^{-\lambda_cy}\rm{d} y+d_3+\gamma+\mu_3-\beta_1S_0e^{-\lambda_cc\tau}-\beta_2V_0e^{-\lambda_cc\tau}+\beta_1M_1e^{\varepsilon_1\xi-\lambda_cc\tau}+\beta_2M_2e^{\varepsilon_2\xi-\lambda_cc\tau}\\ \leq M_3e^{\varepsilon_3\xi}&\left(c(\lambda+\varepsilon_3)-d_3\int_{{\rm IR}}J(y)e^{-(\lambda_c+\varepsilon_3)y}\rm{d} y+d_3+\gamma+\mu_3-\beta_1S_0e^{-(\varepsilon_3+\lambda_c)c\tau}\right.\\ &+\left.\beta_1M_1e^{\varepsilon_1\xi-(\varepsilon_3+\lambda_c)c\tau}-\beta_2V_0e^{-(\varepsilon_3+\lambda_c)c\tau}+\beta_2M_2e^{\varepsilon_1\xi-(\varepsilon_3+\lambda_c)c\tau}\right) \end{align*}$

we only need to ensure

$M_3\geq\frac{\beta_1M_1e^{(\varepsilon_1-\varepsilon_3)\xi-\lambda_cc\tau}+\beta_2M_2e^{(\varepsilon_2-\varepsilon_3)\xi-\lambda_cc\tau}}{-\Delta(\lambda_c+\varepsilon_3, c)+\beta_1M_1e^{\varepsilon_1\xi-(\varepsilon_3+\lambda_c)c\tau}+\beta_2M_2e^{\varepsilon_2\xi-(\varepsilon_3+\lambda_c)c\tau}}$

Since $\xi<\min\{\mathfrak{X}_1, \mathfrak{X}_2\},$ $0<S_0<M_3,$ $0<V_0<M_3$ , $\varepsilon_3<\min\{\varepsilon_1/2, \varepsilon_2/2\}$ and $\tau>0,$ we have

$\frac{\beta_1M_1e^{(\varepsilon_1-\varepsilon_3)\xi-\lambda_cc\tau}+\beta_2M_2e^{(\varepsilon_2-\varepsilon_3)\xi-\lambda_cc\tau}}{-\Delta(\lambda_c+\varepsilon_3, c)+\beta_1M_1e^{\varepsilon_1\xi-(\varepsilon_3+\lambda_c)c\tau}+\beta_2M_2e^{\varepsilon_2\xi-(\varepsilon_3+\lambda_c)c\tau}} \lt \frac{\beta_1\sqrt{S_0M_1}+\beta_2\sqrt{V_0M_2}}{-\Delta(\lambda_c+\varepsilon_3, c)}.$

Then Equation (2.13) holds if we choose $M_3$ large enough with

$M_3\geq\frac{\beta_1\sqrt{S_0M_1}+\beta_2\sqrt{V_0M_2}}{-\Delta(\lambda_c+\varepsilon_3, c)}\triangleq\Pi_4.$

Through the above discussion, Equation (2.13) holds if we choose $M_3\geq\max\{\Pi_1, \Pi_2, \Pi_3, \Pi_4\}$ large enough for all $\xi\in{\rm IR}$ . Here we completes the proof.

References

[1]	J. P. Kreiss, Bootstrap procedures for AR(∞)-processes, in Bootstrapping and Related Techniques (eds. K.-H. Jockel, G. Rothe and W. Sendler), Springer, Heidelberg, (1992), 107-113.
[2]	P. Bühlmann, Sieve bootstrap for time series, Bernoulli, 3 (1997), 123-148.
[3]	P. J. Bickel and P. Bühlmann, A new mixing notion and functional central limit theorems for a sieve bootstrap in time series, Bernoulli, 5 (1999), 413-446.
[4]	A. M. Alonso, D. Peña and J. Romo, Forecasting time series with sieve bootstrap, J. Stat. Plann. Infer., 100 (2002), 1-11.
[5]	A. M. Alonso, D. Peña and J. Romo, On sieve bootstrap prediction intervals, Stat. Probabili. Lett., 65 (2003), 13-20.
[6]	A. Zagdanski, On the construction and properties of bootstrap-t prediction intervals for stationary time series, Probab. Math. Stati. PWN, 25 (2005), 133-154.
[7]	A. M. Alonso and A. E. Sipols, A time series bootstrap procedure for interpolation intervals, Comput. Stat. Data Anal., 52 (2008), 1792-1805.
[8]	P. Mukhopadhyay and V. A. Samaranayake, Prediction intervals for time series: a modified sieve bootstrap approach, Commun. Stat. Simul. Comput., 39 (2010), 517-538.
[9]	G. Ulloa, H. Allende-Cid and H. Allende Robust sieve bootstrap prediction intervals for contaminated time series, Int. J. Pattern Recognit. Artif. Intell., 28 (2014).
[10]	Y. Chang and J. Y. Park, A sieve bootstrap for the test of a unit root, J. Time Ser. Anal., 24 (2003), 379-400.
[11]	Z. Psaradakis, Blockwise bootstrap testing for stationarity, Stat. Probabili. Lett., 76 (2006), 562 -570.
[12]	D. S. Poskitt, Properties of the sieve bootstrap for fractionally integrated and non-invertible processes, J. Time Ser. Anal., 29 (2008), 224-250.
[13]	D. S. Poskitt, G. M. Martin and S. D. Grose, Bias reduction of long memory parameter estimators via the pre-filtered sieve bootstrap, arXiv preprint arXiv, 2014 (2014).
[14]	E. Paparoditis, Sieve bootstrap for functional time series, Ann. Stat., 46 (2018), 3510-3538.
[15]	M. Meyer, C. Jentsch and J. P. Kreiss Baxter's inequality and sieve bootstrap for random fields, Bernoulli, 23 (2017), 2988-3020.
[16]	J. P. Kreiss, E. Paparoditis and D. N. Politis, On the range of validity of the autoregressive sieve bootstrap, Ann. Stat., 39 (2011), 2103-2130.
[17]	M. Fragkeskou and E. Paparoditis, Extending the Range of Validity of the Autoregressive (Sieve) Bootstrap, J. Time Ser. Anal., 39 (2018), 356-379.
[18]	F. Giordano, M. La Rocca and C. Perna, Forecasting nonlinear time series with neural network sieve bootstrap, Comput. Stat. Data Anal., 51 (2007), 3871-3884.
[19]	F. Giordano, M. La Rocca and C. Perna, Properties of the neural network sieve bootstrap, J. Nonparametr. Stat., 23 (2011), 803-817.
[20]	G. B. Huang, Q. Y. Zhu and C. K. Siew, Extreme learning machine: theory and applications, Neurocomputing, 70 (2006), 489-501.
[21]	G. B. Huang, H. Zhou, X. Ding, et al., Extreme learning machine for regression and multiclass classification, IEEE Trans. Syst. Man Cybern. Part B, 42 (2012), 513-529.
[22]	W. Haerdle and A. Tsybakov, Local polynomial estimators of the volatility function in nonparametric autoregression, J. Econometrics, 81 (1997), 223-242.
[23]	J. Franke and M. Diagne, Estimating market risk with neural network, Stat. Decisions, 24 (2006), 233-253.
[24]	A. R. Barron, Universal approximation bounds for superpositions of a sigmoidal function, IEEE Trans. Inf. Theory, 39 (1993), 930-945.
[25]	K. Hornik, M. Stinchcombe and P. Auer, Degree of approximation results for feedforward networks approximating unknown mappings and their derivatives, Neural Comput., 6 (1994), 1262-1275.
[26]	Y. Makovoz, Random approximates and neural networks, J. Approximation Theory, 85 (1994), 98-109.
[27]	X. Chen and H. White, Improved Rates and Asymptotic Normality for Nonparametric Neural Network Estimators, IEEE Trans. Inf. Theory, 45 (1999), 682-691.
[28]	X. Chen and X. Shen, Asymptotic Properties of Sieve Extremum Estimates for Weakly Dependent Data with Applications, Econometrica, 66 (1998), 299-315.
[29]	J. Zhang, Sieve Estimates via Neural Network for Strong Mixing Processes, Stat. Inference Stochastic Processes, 7 (2004), 115-135.
[30]	S. F. Crone and N. Kourentzes, Feature selection for time series prediction-A combined filter and wrapper approach for neural networks, Neurocomputing, 7 (2010), 1923-1936.
[31]	C. Wang, Y. Qi, M. Shao, et al., A fitting model for feature selection with fuzzy rough sets, IEEE Trans. Fuzzy Syst., 25 (2017), 741-753.
[32]	D. Yu and L. Deng, Efficient and effective algorithms for training single hidden- layer neural networks, Pattern Recognit. Lett., 33 (2012), 554-558.
[33]	K. Li, J. X. Peng and G. W. Irwin, A fast nonlinear model identification method, IEEE Trans. Autom. Control, 50 (2005), 1211-1216.
[34]	X. Yao, A review of evolutionary artificial neural networks, Int. J. Intell. Syst., 8 (1993), 539-567.
[35]	G. B. Huang, D. H. Wang and Y. Lan, Extreme learning machines: a survey, Int. J. Mach. Learn. Cybern., 2 (2011), 107-122.
[36]	S. Ding, H. Zhao, Y. Zhang, et al. Extreme learning machine: algorithm, theory and applications, Artif. Intell. Rev., 44 (2015), 103-115. doi: 10.1007/s10462-013-9405-z
[37]	G. Huang, G. B. Huang, S. Song, et al., Trends in extreme learning machines: A review, Neural Networks, 61 (2015), 32-48.
[38]	G. H. Huang, H. Zhou, X. Ding, et al., Extreme learning machine for regression and multiclass classification, IEEE Trans. Syst. Man Cybern. Part B, 42 (2012), 513-529.
[39]	G. B. Huang, L. Chen and C. K. Siew, Universal approximation using incremental constructive feedforward networks with random hidden nodes, IEEE Trans. Neural Networks, 17 (2006), 879-892.
[40]	G. B. Huang and L. Chen, Convex incremental extreme learning machine, Neurocomputing, 70 (2007), 3056-3062.
[41]	G. B. Huang and L. Chen, Enhanced random search based incremental extreme learning machine, Neurocomputing, 71 (2008), 3460-3468.
[42]	J. Lin, J. Yin, Z. Cai, et al., A secure and practical mechanism of outsourcing extreme learning machine in cloud computing, IEEE Intell. Syst., 28 (1999), 35-38.
[43]	E. Cule and S. Moritz, ridge: Ridge Regression with Automatic Selection of the Penalty Parameter, R package version, (2019), https://CRAN.R-project.org/package=ridge.
[44]	Z. Cai, J. Fan and Q. Yao, Functional-coefficient regression models for nonlinear time series, J. Am. Stat. Assoc., 95 (2000), 941-956.
[45]	H. Kuswanto and P. Sibbertsen, Can we distinguish between common nonlinear time series models and long memory?, Discussion papers//School of Economics and Management of the Hanover Leibniz University., (2007).

This article has been cited by:

1.	Kaiyan Zhao, Shaojuan Ma, Qualitative analysis of a two-group SVIR epidemic model with random effect, 2021, 2021, 1687-1847, 10.1186/s13662-021-03332-w
2.	Jinhu Xu, Yan Geng, Dimitri Volchenkov, Dynamics of a Diffusive Multigroup SVIR Model with Nonlinear Incidence, 2020, 2020, 1099-0526, 1, 10.1155/2020/8847023
3.	Shu Liao, Weiming Yang, Fang Fang, Traveling waves for a cholera vaccination model with nonlocal dispersal, 2021, 44, 0170-4214, 5150, 10.1002/mma.7099
4.	JIANGBO ZHOU, JINGHUAN LI, JINGDONG WEI, LIXIN TIAN, Wave propagation in a diffusive SAIV epidemic model with time delays, 2022, 33, 0956-7925, 674, 10.1017/S0956792521000188
5.	Daozhou Gao, Yuan Lou, Impact of State-Dependent Dispersal on Disease Prevalence, 2021, 31, 0938-8974, 10.1007/s00332-021-09731-3
6.	Chunyue Wang, Jinliang Wang, Ran Zhang, Global analysis on an age‐space structured vaccination model with Neumann boundary condition, 2022, 45, 0170-4214, 1640, 10.1002/mma.7879
7.	Jinliang Wang, Ran Zhang, Toshikazu Kuniya, A reaction–diffusion Susceptible–Vaccinated–Infected–Recovered model in a spatially heterogeneous environment with Dirichlet boundary condition, 2021, 190, 03784754, 848, 10.1016/j.matcom.2021.06.020
8.	Ran Zhang, Dan Li, Hongquan Sun, Traveling wave solutions for a discrete diffusive epidemic model with asymptomatic carriers, 2023, 16, 1793-5245, 10.1142/S1793524522500796
9.	Yahui Wang, Xinjian Wang, Guo Lin, Propagation thresholds in a diffusive epidemic model with latency and vaccination, 2023, 74, 0044-2275, 10.1007/s00033-022-01935-1
10.	Soufiane Bentout, Salih Djilali, Toshikazu Kuniya, Jinliang Wang, Mathematical analysis of a vaccination epidemic model with nonlocal diffusion, 2023, 0170-4214, 10.1002/mma.9162
11.	Ran Zhang, Shengqiang Liu, WAVE PROPAGATION FOR A DISCRETE DIFFUSIVE VACCINATION EPIDEMIC MODEL WITH BILINEAR INCIDENCE, 2023, 13, 2156-907X, 715, 10.11948/20220040
12.	Rukhsar Ikram, Amir Khan, Aeshah A. Raezah, Impact of supervise neural network on a stochastic epidemic model with Levy noise, 2024, 9, 2473-6988, 21273, 10.3934/math.20241033
13.	Ran Zhang, Hongyong Zhao, Traveling wave solutions for a three-component noncooperative systems arising in nonlocal diffusive biological models, 2024, 17, 1793-5245, 10.1142/S1793524523500675
14.	Lianwen Wang, Xingyu Wang, Zhijun Liu, Yating Wang, Global dynamics and traveling waves for a diffusive SEIVS epidemic model with distributed delays, 2024, 128, 10075704, 107638, 10.1016/j.cnsns.2023.107638
15.	Xinzhi Ren, Lili Liu, Tianran Zhang, Xianning Liu, Minimal Wave Speed for a Nonlocal Viral Infection Dynamical Model, 2024, 8, 2504-3110, 135, 10.3390/fractalfract8030135
16.	Liwen Song, Sanyi Tang, Changcheng Xiang, Robert A. Cheke, Sha He, Modelling and bifurcation analysis of spatiotemporal hormetic effects on pest control, 2023, 177, 09600779, 114194, 10.1016/j.chaos.2023.114194
17.	Jinxin Wang, Shi-Liang Wu, Mingdi Huang, Haiqin Zhao, Spatial spread for a delayed and nonlocal foot-and-mouth disease model, 2024, 76, 14681218, 104006, 10.1016/j.nonrwa.2023.104006

Reader Comments

Your name:*

Email:*
© 2020 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)