In this paper, we focus on the study of the dynamics of a certain age structured epidemic model. Our aim is to investigate the proposed model, which is based on the classical SIR epidemic model, with a general class of nonlinear incidence rate with some other generalization. We are interested to the asymptotic behavior of the system. For this, we have introduced the basic reproduction number R0 of model and we prove that this threshold shows completely the stability of each steady state. Our approach is the use of general constructed Lyapunov functional with some results on the persistence theory. The conclusion is that the system has a trivial disease-free equilibrium which is globally asymptotically stable for R0<1 and that the system has only a unique positive endemic equilibrium which is globally asymptotically stable whenever R0>1. Several numerical simulations are given to illustrate our results.
1.
Introduction
Mathematical models for the spread of epidemic infectious diseases in populations have been studied for a long time [1]. One of the most classical epidemic models is the SIR epidemic model in which the total population is divided into three classes called susceptible, infected and removed [2]. Some types of SIR epidemic models without age structure are nonlinear systems of ordinary differential equations, and it is relatively easy to show that the long time behavior of its solution is completely determined by a threshold value R0: if R0<1, then the trivial equilibrium in which there is no infected individual (the disease-free equilibrium) is globally asymptotically stable, whereas if R0>1, then the nontrivial equilibrium in which the infected individuals persist (the endemic equilibrium) is globally asymptotically stable [3,4]. The threshold value R0 is called the basic reproduction number and it plays an important role as an indicator of the intensity of diseases since it implies the expected number of secondary cases produced by a typical infected individual during its entire period of infectiousness in a fully susceptible population [3,4].
Some types of SIR epidemic models with age structure are nonlinear systems of partial differential equations, and the mathematical analysis for them is generally more difficult than that for the models without age structure. The most classical SIR epidemic model studied by Kermack and McKendrick [2] has the structure of infection age (time elapsed since the infection), and the complete global stability analysis for an infection age-structured SIR epidemic model was recently done by Magal et al, [5]. That is, they showed that the disease-free equilibrium in their model is globally asymptotically stable if R0≤1, whereas the endemic equilibrium is so if R0>1. Their method of constructing a suitable Lyapunov function in a compact global attractor has been applied for the analysis of various epidemic models with age structure [6,7,8,9,10]. Recently, the global behavior of various kinds of age-structured models in biology has been studied [11,12,13].
In basic epidemic models, the incidence rate is often assumed to take the bilinear form such as βSI, where β>0 is the disease-transmission coefficient and S and I are the populations of susceptible and infected individuals, respectively. As a generalization, Capasso and Serio [14] studied the incidence rate with the form Sg(I), where g(⋅) is a continuous bounded function that takes into account either the saturation phenomenon or the psychological effects. After their work, epidemic models with more general incidence rates such as f(S)g(I) and f(S,I) have been studied by many authors [15,16,17,18,19,20,21,22,23,24,25,26].
In [27], Bentout and Touaoula established an infection age-structured SIR epidemic model with a general incidence rate. They proved for their model that if R0≤1, then the disease-free equilibrium is globally asymptotically stable, whereas if R0>1, then the endemic equilibrium is globally asymptotically stable. The purpose of this study is to generalize their results for a new epidemic model. Specifically, we assume for our model that the removed individuals do not stay permanently in the removed class and can return to the infected class. This kind of assumption can be seen in models for diseases with relapse [7,10,25,26,28], drug epidemics [15,29,31,30,32,33] and (although it is a fiction) zombie epidemics [34]. Because of the complexity in the model structure, we need more advanced techniques for the mathematical analysis of our model. In this paper, we obtain the basic reproduction number R0 for our model and show that the disease-free equilibrium is globally asymptotically stable if R0<1, whereas the endemic equilibrium uniquely exists and it is globally asymptotically stable if R0>1.
The organization of this paper is as follows. In Section 2, we establish our main model. In Section 3, we define the basic reproduction number R0 and show that the disease-free equilibrium is globally asymptotically stable if R0<1. In Section 4, we define the semiflow generated by the solution of our model and show the existence of a compact attractor. In Section 5, we prove the existence and uniqueness of the endemic equilibrium, uniform persistence of the system and global asymptotic stability of the endemic equilibrium for R0>1. In Section 6, we perform numerical simulations that illustrate our theoretical results. Finally, Section 7 is devoted to the summary.
2.
The model
Let S(t), i(t,a) and R(t) denote the population of susceptible, infected with infection age a and removed individuals at time t, respectively. The function θ(a) is the age-dependent per-capita removal rate of infected individuals with age a. The parameters μ, δ and k are respectively the natural death rate, the relapse rate and the fraction at which removed individuals directly return to the infected class. The parameter A represents the entering flux into the class S. The main model of this paper is formulated as the following system with infection age and with a general class class of nonlinear incidence rate, for t>0,
where J is the contact function given by,
The system (2.1) is completed by the following boundary and initial conditions,
For instance, diseases with relapse such as herpes simplex virus type 2 (HSV-2) [28] can be modeled by system (2.1). Throughout this paper, we make the following hypotheses on β and θ.
● The function β∈CBU(R+,R+) (where CBU(R+,R+) is the set of all bounded and uniformly continuous function from R+ to R+) and the function θ∈L∞+(R+) (where L∞+(R+) is the set of all essentially bounded functions from R+ to R+).
● The parameters A and μ are positive and k∈[0,1).
The boundedness of β implies that there is a maximum of infectivity of infected individuals. The uniform continuity of β is sufficient for the practical application since any continuous functions with a closed bounded support is uniformly continuous (see Section 6). For the above system, we make also the following assumptions on the infection response function f.
● (H0) The function f(⋅,J) is increasing for J>0 and f(S,⋅) is increasing for S>0 with f(0,J)=f(S,0)=0 for all S,J≥0.
● (H1) For all S>0 the function f(S,J) is concave with respect to J.
● (H2) The function ∂f(⋅,0)/∂J is continuous positive on every compact set K⊂R+.
● (H3) The function f is locally Lipschitz continuous in S and J, that is, for every C>0 there exists some L:=LC>0 such that
whenever 0≤S2,S1,J2,J1≤C.
Now, let us define the functional space X:=R×L1(R+)×R equipped with the norm
We put X+=R+×L1+(R+)×R+, which denotes the positive cone of X. The problem of existence, positivity and uniqueness of the solution is classical and can be treated by using the Banach-Picard fixed point theorem in an appropriate Banach space on X (see, for instance, [27], [39]). Then, we have the following theorem.
Theorem 2.1. Let consider an initial condition belonging to X+. Then, the system (2.1) and (2.2) has a unique nonnegative solution (S,i,R)∈C1(R+)×C(R+,L1(R+))×C1(R+).
We omit the proof of Theorem 2.1 as it is similar to the proof of [27,Theorem 2.2] except for a simple modification. We set, N(t)=S(t)+∫+∞0i(t,a)da+R(t). Then, from the system (2.1), we have, for t>0,
So, for t>0, we obtain
with N(0)=S0+∫+∞0i0(a)da+r0, and
On the other hand, we can check that (L is the Lipschitz constant defined in (2.3)),
3.
Global stability of the disease-free equilibrium
In this section, we show the local and the global stability of the disease-free equilibrium. First, we begin by studying the local stability. We denote by
We can estimate the basic reproduction number by renewal process, which is the spectral radius of the next generation matrix. For more details, we refer the reader to [3]. The basic reproduction number R0 for our model (2.1) is given by
For the system (2.1), it is easy to see that the disease-free steady state always exists and it is given by E0=(A/μ,0,0)∈X+. The linearization of system (2.1) near the equilibrium E0=(A/μ,0,0) is given by, for t>0,
Next, the characteristic equation of (3.2) at E0 is given by the following equation,
where
and
We have the following theorem.
Theorem 3.1. If R0<1, then the disease-free equilibrium E0=(A/μ,0,0) is locally asymptotically stable and unstable for R0>1.
Proof. The characteristic equation of the disease free equilibrium E0 is given by
where
Obviously, we can see that −μ is a negative real root of (3.3) and all other roots are obtained from H(λ)=0. We assume first that R0<1. We claim that H(λ)=0 has no root with nonnegative real part. By contradiction, we suppose that there exists λ0∈C with Re(λ0)≥0 such that H(λ0)=0, then,
Since Re(λ0)≥0 and R0<1, so
Then,
Therefore,
We obtain that R0≥1 and which is a contradiction with the hypothesis. Consequently, the disease-free equilibrium E0 is locally asymptotically stable for R0<1. Next, we suppose that R0>1. Then, for λ∈R, we have
This ensures to the existence of a positive real root of (3.3). Hence, E0 is unstable if R0>1.
Now, we focus on the global stability of E0. Let ˆi(h):=i(t+h,a+h) and ˆθ(h):=θ(a+h). By the second equation of (2.1), we have
Hence, ˆi(h)=ˆi(0)e−∫h0(μ+ˆθ(s))ds and thus,
Considering two cases a<t and a≥t, we have
Hence, we obtain
with B(t):=i(t,0). Using (3.4), we obtain
and
We prove the following theorem.
Theorem 3.2. The disease-free equilibrium E0 is globally asymptotically stable whenever R0<1.
Proof. It suffices to prove the global attractivity of E0 because of the local stability of this disease-free equilibrium. We set
By using the fluctuation lemma (see [37], Lemma A.14), there exist tn,sn,rn tend to infinity as n tends to infinity such that S(tn)→S∞, S′(tn)→0, B(sn)→B∞ and R(rn)→R∞ and R′(rn)→0. Next substituting rn in (3.7), and letting n tends to infinity we find,
Similarly using (3.5), (3.6) and the hypothesis (H0), we obtain
Now, combining (3.8), (3.9) and the fact that f(S∞,J)≤f(A/μ,J), we get
Since S∞≤A/μ and f is an increasing function with respect to S, then
Next, from (H1), we easily obtain
By combining (3.10) with (3.11) and the second equation of (3.9), we get
Since R0<1, then necessarily B∞=0. This implies that J∞=R∞=0. Hence, f(S(t),J(t))→0 as t→+∞ and the asymptotic behavior of S obyes the equation dS(t)/dt=A−μS(t). This implies that S(t)→A/μ as t→+∞. The theorem is proved.
4.
The semiflow and global attractor
In this section, we prove the existence of a compact attractor of all bounded subset of X+. First, we define the semiflow Φ:R+×X+→X+ such that
which is generated by the unique solution of system (2.1). So, it is not difficult to show that this semiflow is continuous.
Theorem 4.1. The semiflow Φ has a compact attractor A of bounded subsets of X+.
Proof. Following Theorem 2.33 in [37], we need to check some properties of the semiflow Φ, namely, the point-dissipativity, eventually bounded on bounded sets on X and asymptotically smoothness properties. By theorem 2.1, we can show that the first two properties are satisfied. Now, we apply Theorem 2.46 of [37] to show the asymptotic smoothness. For this, we define
where
and
Let C be a bounded closed subset of initial data in X+, that is forward invariant under Φ. We set
Using the same arguments as in Theorem 2.1 in [18], we have Ψ1→0 as t→+∞ uniformly for all initial data in C. Next, we have to prove that Ψ2 has a compact closure. We need only to check the condition (ⅲ) of Theorem B.2 in [37] or Theorem 3.1 in [27] because the other conditions are readily checked. Thus,
Notice that, for t≥0, we have
for all initial data in C. Then, the second term of (4.3) tends to 0 when h→0 uniformly in C. We set
From the system (2.1) and (4.2), we have, for t≥0,
and
Now, let us define Ah for h>0 as
Using the fact that the function f is Lipschitz, we get
By (4.6) and (4.7), we can easily show that the first and the last term of Ah tend to 0 as h tends to 0+ uniformly for all initial data in C. Now we focus on the second term of Ah. Denote by,
For t≥0, we set J(t)=J1(t)+J2(t), where
Thus, for s,h≥0,
After a change of variable in (4.9), we obtain
Therefore, for s,h≥0,
Consequently, we can readily checked that these last terms tend to 0 as h→0+ uniformly for all s≥0 and for all initial data in C. Using the definition of J2 in (4.9), we get
Using the fact that β is uniformly continuous and using (4.2), we can see that all above terms go to 0 as h→0+ uniformly for all s≥0 and for all initial data on C. Finally, employing the same arguments as above, we show that,
as h→0+ uniformly for all initial data in C. The theorem is established.
Next, we describe the total trajectories of system (2.1). Let ϕ:R→X+ be the total Φ-trajectories, ϕ(t)=(S(t),i(t,⋅),R(t)). Then, ϕ(t+r)=Φ(t,ϕ(r)),t≥0,r∈R. By using the same arguments as in [37], we have, for all t∈R,
The following lemma gives some estimates on the total trajectories.
Lemma 4.2. For all (S0,i0(⋅),r0)∈A, we have the following estimates, for all t∈R
with ξ:=(L‖β‖∞+k‖θ‖∞+δ)(A/μ) and L is Lipschitz constant of f defined in (2.3).
Proof. We set,
After a change of variable, for t∈R,
By differentiating I, we get
Combining this equation with the system (4.10), we obtain,
Thus, for t∈R
Moreover, from the equation of S in (4.10) and the hypothesis (H3), we have
so,
On the other hand, using the fact that f(S,0)=0 and (H3), we find
According to (4.11), we conclude that
where ξ:=(L‖β‖∞+k‖θ‖∞+δ)(A/μ).
5.
Global stability of the endemic equilibrium
The main purpose of this section is to study the global asymptotic stability of the endemic equilibrium. We first need to prove the strong uniform persistence of the solution of problem (4.10).
We begin by the following lemma which concern the existence of a positive equilibria.
Lemma 5.1. Assume that
If R0>1, then the system (2.1) has a positive steady state.
Proof. Let E∗:=(S∗,i∗(.),R∗) be a positive fixed point of the semiflow Φ. Then,
From (4.1) and (3.4), we have
and
Remark that if we consider i∗(a) from the first expression of (5.1), it satisfies also the second one of (5.1). Indeed, for t<a<2t, we have
and thus
We can proceed by iteration in order to prove the result. Therefore,
Combining (5.2) and (5.3), we get
with
thus,
Moreover, from (5.2) and (5.5), we obtain
where M:=∫+∞0β(a)π(a)da and D is defined in (5.4). Following the same arguments as in [22] and [23], we prove the existence of positive steady state. The following lemma is readily to prove it
Lemma 5.2. Under (H0) and (H1), we have following assertions,
and
Now we focus on the uniform persistence of the solution of problem (4.10). We define,
and
We set
Lemma 5.3. If (S0,i0(⋅),r0)∈X0, then there exists t0>0 such that B(t):=i(t,0)>0 for all t>t0. If (S0,i0(⋅),r0)∈X+∖X0, then B(t)=0 for all t≥0.
Proof. Recall that S(t)>0 for all t>0 and that
The third equation of system (2.1) implies that, for t≥0,
We can rewrite B as,
So, from (3.4),
If r0>0 then we have B(t)>0 for all t≥0. Next, we suppose that ∫+∞0i0(a)ˉθ(a)da>0, thus we set,
By integrating I0 it yields,
In the following, we will use translations of solutions: for r≥0, we define Sr(t)=S(t+r) Jr(t)=J(t+r), I0r(t)=I0(t+r) and Br(t)=B(t+r). By (5.9), (5.10),
Since ∫+∞0I0(t)dt>0 then for some small r>0, I0ris not zero almost everywhere on [r,+∞). Consequently, from Corollary B.6 in [37], there exists t0>0 such that B(t)>0 for all t>t0. Using the same arguments as above in the case where ∫+∞0i0(a)ˉβ(a)da>0.
Now, if (S0,i0(⋅),r0)∈X+∖X0, then according to (3.4), (5.9), we have
From the definition of the space X+∖X0 and the fact that the function f is locally Lipschitz and that f(S,0)=0 for all S>0, the equation (5.11) yields,
We can apply the Fubini's Theorem to the last term and using the assumptions on β and θ, we get
By Gronwall's inequality, we obtain B(t)=0 for all t≥0.
We define the persistence function ρ:X+→R+ by
By definition, we have,
Lemma 5.4. Assume that (5.7) holds. If x∈X0, then there exists some ϵ>0 such that
for all solutions of (2.1) provided that R0>1.
Proof. We suppose that the function ρ is not uniformly weakly persistent, so, there exists an arbitrarily small ϵ>0 such that
By Theorem 2.1, we have S(t)>0 for all t>0, so, from the above expression there exists some small ϵ0>0 such that
Let S∞=lim inft→+∞S(t). Then, there exists a sequence tk→+∞ such that S(tk)→S∞ and S′(tk)→0 as tk→+∞ see ([37], Lemma A.14). Combining this with the continuity of the function f, we have (for large t)
Therefore,
with ψ(ϵ):=ϵ/μ. By using the fact that R0>1, then we can choose a small ϵ1>0 such that
From system (2.1), we have, for all t≥0,
Therefore, for t≥0,
Next, we introduce the Laplace transform to this inequality, which is given by, for λ>0,
Thus, we get
where ˆB and ˆθ are respectively the Laplace transforms of B and θ, defined by, for λ>0,
Moreover, since there exists T>0 such that J(t)<ϵ0 for all t≥T, then by (5.7), we obtain
Then, for x∈X0, we write
so, for t≥T,
Similarly, we apply the Laplace transform to the last inequality, we get, for λ>0,
where ˆβ is the Laplace transform of β defined as
By using (5.13), we obtain
Since, ˆB(λ)>0 (See, Lemma 5.3), then
We can choose λ=ϵ0 and we obtain
which contradicts (5.12). This completes the proof.
To prove the uniform strong ρ−persistence from Lemma 5.4, we will use Theorem 3.5 in [37]. To this end, we need to prove that there exists no total trajectory ϕ:R→X+0, ϕ is defined in (4.10), such that ρ(ϕ(0))=0 and ρ(ϕ(−r))>0 and ρ(ϕ(t))>0 for some r,t∈R+ (see (H1) in [37], Chapter 5). Therefore, we next prove the following lemma.
Lemma 5.5. If ρ(ϕ(t))=0 for all t≤0, then ρ(ϕ(t))=0 for all t>0.
Proof. Assume that ρ(ϕ(t))=B(t)=0 for all t≤0 with B(t) is defined in (4.10). Then, applying the same arguments as in the proof of Lemma 5.3, we have
thus since B(t)=0 for t≤0,
So,
Finally, according to Fubini's Theorem,
Applying the Gronwall's inequality, we get
Lemma 5.6. The following alternative holds: either B is 0 everywhere on R or B is positive everywhere on R.
Proof. From Lemma 5.5, we can deduce that for each r∈R, if B(t)=0 for all t≤r then B(t)=0 for all t≥r. This means that either B(t)=0 for all t∈R or there exists a sequence tn→−∞ as n→∞ with B(tn)>0. Let, Bn(t):=B(t+tn), by Lemma 4.2 and (5.9),
After a change of variable,
where Bn(0):=B(tn)>0, Now, suppose that there exists ϵ>0 such that Bn(ϵ)=0 and Bn(t)>0 for all t∈[0,ϵ), so, we obtain
Then, Bn(s)=0 for all s∈[0,ϵ), this leads to a contradiction. Then, Bn(t)>0 for all t>0 and since tn→−∞ as n→+∞, B(t)>0 for all t∈R.
Now we are ready to prove the strong uniform persistence of the disease.
Theorem 5.7. Assume that R0>1, Then, each solution of systems (2.1)-(2.2) is strongly uniformly ρ−persistent for initial data belonging to X0, that is there exists an ϵ>0 such that lim inft→+∞ρ(Φ(t)x0)>ϵ provided x0∈X0.
Proof. By Lemmas 5.4, 5.5 and 5.6, we can apply Theorem 5.2 in [37] to conclude that uniform weak ρ−persistence implies the uniform strong ρ−persistence. The proof is completed.
From Theorem 5.7 in [37], we have the following result.
Theorem 5.8. There exists a compact attractor A1 that attracts every solution with initial condition in X0. Moreover A1 is uniformly ρ−positive, i.e there exists some positive constant Γ, such that
We will need the following estimates later.
Lemma 5.9. For all (S0,i0(⋅),r0)∈A1, the following estimates hold
with Γ0=ΓD/f(S∗,J∗) and η2=((1−k)Γ∫+∞0θ(a)π(a)da)/(μ+δ).
Proof. Recall that the compact attractor of bounded set is the union of bounded total trajectories, see Proposition 2.34 in [37]. Thus, there exists a total trajectory Ψ:R→A1, Ψ(t)=(S(t),i(t,⋅),R(t)), S(0)=S0,i(0,a)=i0(a). In view of (5.14), we get
In addition, from the equation of R in (4.10), and Theorem 5.8,
Finally, by a straightforward computation, we find
with η2:=(1−k)Γ∫+∞0θ(a)π(a)da/(μ+δ).
Now, we are ready to prove the global asymptotic stability of the unique positive endemic equilibrium.
Theorem 5.10. Suppose that R0>1. The problem (4.10) has a unique positive endemic equilibrium (S∗,i∗(.),R∗) which is globally asymptotically stable in X0.
Proof. Let Ψ:R→A1 be a total Φ−trajectory such that Ψ(t)=(S(t),i(t,.),R(t)), S(0)=S0, i(0,.)=i0(.), where (S(t),i(t,a),R(t)) is the solution of problem (4.10). Let, for a≥0,
and, for y>0,
Then, for Ψ(t)=(S(t),i(t,.),R(t))∈A1, t∈R, we consider the following Lyapunov functional V(Ψ(t))=V1(Ψ(t))+V2(Ψ(t))+V3(Ψ(t)) where
and
First, using the equation of S in (4.10), we get
By using the same arguments as in the proof of Lemma 9.18 in [37], we find
where
In view of the third equation of (4.10), by
we have Δ1+Δ2+Δ3=1. The convexity of the function H implies
By adding V′1 and V′2 and using the expression of H, we have
We reorder these terms, and using the fact that
we obtain
On the other hand,
By adding and subtracting the same term
it yields,
Next, by summing V′=V′1+V′2+V′3, we get
Using the fact (μ+δ)R∗=(1−k)∫+∞0θ(a)i∗(a)da, the addition of the two last terms of the above expression vanishes. Moreover, by employing the Jensen inequality, we have
Thus, by combining this with the fact that
we obtain
Now, for the values of t such that J(t)<J∗. From Lemma 5.2 and Jensen inequality, we have
This implies that
Finally, in view of the expression of ϕ′ in (5.15), we find
Since −ln(1/x)−x+1≤0 and H(b)−H(a)+(a−b)H′(b)≤0 then the second and the third terms are negative. As a consequence,
By applying the same arguments for the values of t satisfying J(t)>J∗. we conclude that V′(Ψ(t))≤0. Now, since V is bounded on Ψ, the alpha limit and omega limit set of Ψ must be contained in M the largest invariant subset of {V′=0}. Notice that V′(Ψ(t))=0 implies that S(t)=S∗, and i(t,a)/i∗(a)=R(t)/R∗. From (4.10) and the fact that i∗(a)=π(a)i∗(0), we have
this implies that B(t−a)=B(t) for all a≥0, and so i(t,a)=i(t,0) for all a≥0. Next, from the equation of S in (4.10) and the fact that S(t)=S∗, we have
Moreover, from equations of equilibrium we know that
thus we obtain f(S∗,J∗)=f(S∗,J(t)). From the monotonicity of f and (4.10) we obtain i(t,0)∫+∞0β(a)π(a)da=i∗(0)∫+∞0β(a)π(a)da, and so, B(t):=i(t,0)=i∗(0) for all t∈R. Finally, from (5.16), we get R(t)=R∗ and i(t,.)=i∗(.) for all t∈R. Consequently, M consists of only the endemic equilibrium.
Now, since A1 is compact, the omega limit and alpha limit are non-empty, compact, invariant and attract Ψ(t) as t→±∞, respectively. Since V(Ψ(t)) is nonincreasing on t, V is constant on the omega and alpha limit and these both sets contain only the endemic equilibrium. Consequently Ψ(t)→(S∗,i∗(.),R∗) as t→±∞, and hence
On the other hand, we have
for all t∈R, then V(Ψ(t))=V(S∗,i∗(.),R∗) for all t∈R and so Ψ(t)=(S∗,i∗(.),R∗) for all t∈R. Therefore the compact attractor A1 is reduced to the endemic equilibrium. In addition, by Theorem 2.39 in [37] the endemic equilibrium is also locally asymptotically stable. The theorem is proved.
6.
Numerical simulation
In this section, the results of the previous sections are illustrated by numerical simulations. We use the following numerical method: we discretize our problem by the upwind method for solving hyperbolic partial differential equation. For instance, the approximation uni=u(t,a) is given by
The equations of susceptible and recover are solving by explicit Euler method for the ODE. The non-local terms are approximated by one of the composite integration formulas.
Let's consider the Beddington-Deangelis functional response defined by
We note that this function f satisfies all the assumption of the paper. The system (2.1) has two equilibria and the condition of stability is given by the basic reproduction number R0. In the case of the Beddington-Deangelis functional response, the basic reproduction number R0 is given by
We consider the following values of parameters
with the initial conditions
The functions β and θ are chosen to be
and
with τ1=10 and τ2=12. The parameter τ1 represents the end of the exposed period at a=τ1 (witch means that the individual is infected but he does not transmit the disease from a=0 to a=τ1), when a≥τ1 the infectious period starts. The time required to leave the infected class is described by the parameter τ2.
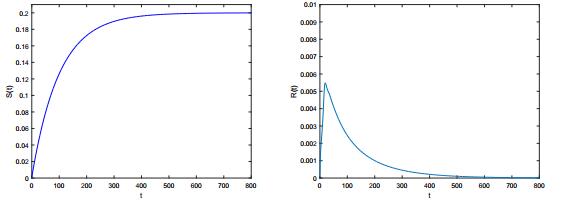
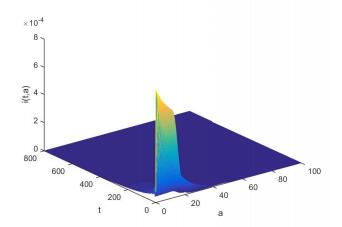
In first time, we choose k=0.1. The basic reproduction number is computed and we have R0=0.8624<1. According to the result stated, the disease-free equilibrium is globally asymptotically stable as illustrated in Figures 2 and 3.
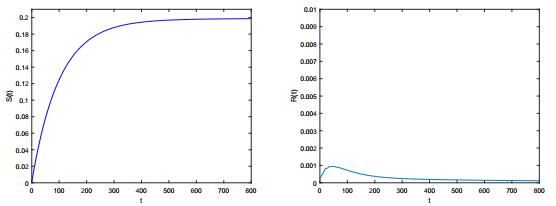
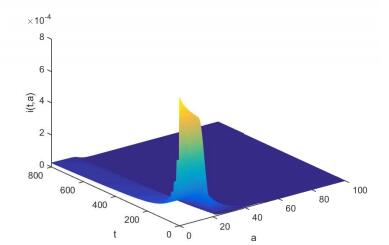
In second time, we take k=0.9 and then the basic reproduction number R0=1.1849>1, that mean that, the positive endemic equilibrium is globally asymptotically stable as illustrated in Figures 4, 5 and 6.
7.
Summary
In this paper, we proposed and analyzed an infection age-structured SIR epidemic model with a general incidence rate. We showed the basic characters of the solution including existence, uniqueness and positivity. We obtained the basic reproduction number R0 by renewal process and showed that the disease-free equilibrium E0 is globally asymptotically stable if R0<1. The main contribution of this paper was devoted to the global asymptotic stability of the endemic equilibrium E∗. We prove that the unique endemic equilibrium E∗ exists, the system is uniformly persistent and E∗ is globally asymptotically stable if R0>1. Our results showed that the asymptotic behavior of the solution of our model can be determined by the basic reproduction number R0, allowing to separate the situations into the extinction and the persistence of diseases.
In this paper, we have not considered the case where R0=1. By some previous results on basic epidemic models (see for instance [4,Section 5.5.2]), we can expect that the disease-free equilibrium is globally asymptotically stable for R0=1. However, it is not trivial and a future work. From the application viewpoint, our results for R0≠1 are thought to be sufficient since R0 estimated by real data unlikely to be just equal to 1.
Acknowledgments
The authors would like to thank the associate editor and the anonymous reviewers for their careful reading and helpful comments. TK was supported by Grant-in-Aid for Young Scientists (B) of Japan Society for the Promotion of Science (Grant No. 15K17585).
Conflict of interest
All authors declare no conflicts of interest in this paper.









 DownLoad:
DownLoad: