1.
Introduction
Migration is a process motivated by an individual's desire to improve the quality of life, including job, income and career prospects, living conditions, well-being, etc. Bulgaria is among the latest EU member countries (since 2007) with the lowest standard of living and hence, it is among those Eastern European countries that traditionally send emigrants rather than host immigrants. According to data from the National Statistical Institute, the country has been characterized by negative migration rates for many years now, especially among young people. In 2021, the number of emigrants, who officially declared a relocation to another country, was 26755. More than 1/3 of them (10825 people) were young individuals aged 20-34 years [1].
Young people are the main force both for efficient functioning of the labor market and for increasing birth rates, especially in the context of severe demographic crisis, caused by the large emigration flows from Bulgaria, the low birth rates and also by depopulation of the rural areas in the country. Therefore, the issue of permanent relocation of young Bulgarian citizens abroad and the discourse on how to enhance their return migration are of key importance and great interest to researchers, policy-makers and the general public.
Most studies on human migration are economic, sociological and demographic in nature. They are driven mostly by the researchers' interest in the role of migrant capital for efficient functioning of the labor markets [2]. However, these studies and theories do not provide a comprehensive picture of migration potential and behavior beyond external factors, such as income levels, unemployment rates, economic growth, political instability, social inequalities, etc.
It is due to the dynamically changing external factors and due to the low predictability of current events such as pandemics, wars, global economic crisis, large migration flows, etc., that we have decided to examine the psychological construct of emigration attitudes, rather than emigration behavior. Furthermore, since migration is driven mostly by individuals' striving for a better life and well-being, we have focused onto the role of perceived/subjective psychological well-being and its main components—optimistic expectations and life satisfaction, in shaping emigration attitudes. We considered this approach more reliable and valid to understand in depth the pre-emigration stage of decision-making (emigration potential) and hence, contribute to the knowledge about how to change positive emigration attitudes in order to prevent mass emigration from the country.
Not a few recent studies have been focused on the effect of subjective/psychological well-being on emigration. Researchers were interested if people, who were less happy and less satisfied with life, were more or less likely to relocate abroad, compared to individuals who were happier and more satisfied with life [3,4,5,6,7,8].
Most of the previous research evidence supports the notion that individuals with lower degrees of subjective well-being are more likely to emigrate in comparison with those who experience higher subjective well-being. However, the findings are still contradictory or at least more complicated than they seem to be (See Paragraph 1.4 below). On one hand, it is due to the ambiguous conceptualization and operationalization of the constructs of life satisfaction, optimism, happiness and subjective psychological well-being that are often considered equivalent. On the other hand, it may be due to the wide range of tools used by different researchers to measure the same construct. On the third hand, it may be because of ignoring the differences between individual-level, group-level and country-level findings. On the fourth hand, it may be because most surveys have been conducted in countries with positive net migration rates.
Although the relationship between migration (attitudes) and satisfaction with life and has been studied in many countries, it has not been examined to date neither in the light of optimistic expectations for individual flourishing in an Eastern European country such as Bulgaria nor in terms of differences between generations/age groups. Furthermore, age differences in emigration attitudes have not been scrutinized through the prism of prior emigration experience, education, income and marital status.
The objective of this paper is to examine both optimism (in terms of optimistic expectations) and satisfaction with life in the country of origin and residence—Bulgaria, as psychological antecedents of emigration attitudes of young Bulgarians with regard to their generational belonging and age differences (i.e. Generation Y or Millennials, born between 1981-1995/6 and Generation Z or Zoomers, born between 1996/7-2012), as well as to study the generational differences in emigration attitudes through the lens of prior emigration experience, income, education and marital status.
We decided to examine the antecedents of emigration attitudes of Generation Y and Generation Z, rather than use age as a continuous variable, because not a few differences between the two age groups are outlined in the scientific literature, even though they are considered to have some characteristics in common, as well. Research evidence from different European countries suggests that younger generations have more positive attitudes towards mobility and emigration in comparison to older generations, possibly due to the better educational and employment opportunities abroad, the greater exposure to globalization, international influences and experience [9,10,11]. Some researchers argue that compared to Millennials, Zoomers have lower levels of optimism and psychological well-being, and they are more likely to experience anxiety and depression, due to a range of factors, including economic uncertainty, political instability, the rise of social media use, and/or the pressure to succeed [12,13]. Some other researchers found that there were not significant differences in psychological well-being and happiness between the age cohorts [14]. Furthermore, Zoomers were found to be more achievement-oriented, entrepreneurial and risk-taking than older generations [15,16]. Compared to Millennials, Generation Z is more likely to seek out meaningful, purpose-driven work and to be less motivated by financial gains. This age cohort values social justice, diversity, and environmentalism more highly. These values may be shaped by the social and political events of their time, including the increasing use of digital technologies, increasing income inequality, and climate change [16,17]. Most studies suggest that there are indeed differences both within and between Generations Y and Z shaped by cultural and historical factors, as well as economic and social contexts.
1.1. Optimism & optimistic expectations
More than any other scientific discipline, psychology has been focused on studies of optimism (also called an optimistic tendency, attitude, expectations) as a personality characteristic. As far as the first half of the 20th century, pioneers such as Z. Freud and Е. Erickson have argued that optimism is an inborn personality trait [18]. From an evolutionary point of view some sociologists, including the anthropologist Tiger, considered it a key factor for personal development and achievement [19].
Tiger defined optimism as "a mood or attitude associated with an expectation about the social or material future—one which the evaluator regards as socially desirable, to his [or her] advantage, or for his [or her] pleasure" [20,19,18]. Consequently, optimism is considered a cognitive, affective and motivational construct [18].
Optimism is also regarded as overall positive expectations for future events [21,22]. The expectations, which one has about what will (not) happen for future, are important, since these expectations determine the goals that they set for themselves, the efforts they invest, and the response to certain events. Future expectations underlie any decision that one makes—from important decisions, such as what career to pursue or whom to marry, to ordinary ones such as where to dine, etc. [23].
Optimists tend to believe that future will be auspicious to them, whereas pessimists are inclined to expect unpleasant future experiences. Pessimists are likely to act in a way that enables them to get prepared for the worst future scenario. Hence, optimistic and pessimistic expectations act like powerful cognitive filters that change individual perceptions of the world and influence the way people adapt to unfamiliar situations, handle challenges and stressful events [24].
A significant positive correlation was found between optimism, using social support and cognitive restructuring of stressful situations as coping strategies. By using certain coping strategies optimism has an indirect effect on the quality of life [25]. More optimistic individuals have a higher quality of life and higher satisfaction with life in comparison with less optimistic and more pessimistic individuals. Optimism is likely to improve psychological well-being and physical health through enhancement of health-wise lifestyle, as well through adaptive behaviour and cognitive responses characterized by increased flexibility, decision-making ability, and effective management of unpleasant events.
Peterson et al. [26,27] delved into the fundamentals of pessimism, which led them to its oppositional concept—"the optimistic attributional style", characterized by the tendency to believe that negative events are nonrepetitive, external (i.e. independent of an individual's will and control), and specific (i.e. having effect on a limited scope of areas and activities in one's life). In other words, individuals, who score high on optimism, tend to believe that positive experiences are more likely to occur than negative experiences. These individuals think that they can solve most daily hassles by not allowing these hassles to upset them and hence, they handle stressful events more efficiently in comparison to individuals who score low on optimism [24].
According to Forgeard and Seligman, the essence of optimism consists of the special attribution style that explains determinants of both success and failure. More optimistic individuals have several advantages. They tend to be more proactive, more energetic, and less likely to experience depressive moods compared to less optimistic/more pessimistic persons [25].
One of the most widespread and strong biases, which impacts individuals' decisions, is having overly optimistic expectations [28]. The optimistic bias can be regarded as a tendency of expecting only positive events to occur given equal other conditions [29]. This bias is also known as wishful thinking, unrealistic optimism, desirability bias, comparative optimism, etc., but all of these constructs share the common ground that people, in general, have optimistic expectations for positive outcomes. Interestingly, this bias persists even when positive and negative events have exactly the same chances of occurring, when individuals know the chances and when they cannot control the outcome [30]. According to some researchers, people, on average, expect good things to happen and their expectations are more optimistic than the reality of events, rather than everyone has overly optimistic expectations for every event [31].
Both stability and spread of optimistic expectations across individuals and situations suggest that there is some process or a "cognitive characteristic" that contributes to maintain the optimistic bias [32]. The cognitive processes that underlie optimistic expectations have not been clearly identified yet. Still, focusing on a more important and desirable outcome rather than less important and undesirable one, while trying to predict the future outcome, is considered a possible explanation for the optimistic bias [33]. Another explanation emphasizes the role of emotional responses. Sometimes, people make decisions based on their emotions rather than reason. Consequently, they tend to evaluate a positive outcome as more likely than negative one, when they are positively attuned [34]. The reverse is also true—thinking about a positive outcome is also likely to breed positive emotions and hence, optimistic expectations. Obviously, both cognitive and emotional processes, as well as their interaction, contribute to maintain optimistic expectations.
Some authors argue that optimistic expectations about future events have at least two advantages over realistic and not so optimistic expectations. The first advantage is that optimistic expectations serve to set a goal and pursue it before the event actually occurs and hence, they strengthen individual motivation and increase the likeliness to obtain positive results [32]. The second advantage of optimistic expectations is that they contribute to reduce stress before the event actually takes place.
A number of studies found that optimism and the general tendency to expect positive outcomes are predictive of low (dis)stress levels, high adaptation levels, and also of using effective coping strategies [35].
1.2. Life satisfaction and allied concepts
In the last decades, psychologists worldwide have increasingly been focusing their research efforts on the issue of subjective psychological well-being, its components and allied concepts—life satisfaction, optimism and happiness. All these concepts are often used interchangeably without being clearly defined and distinguished.
Argyle [21,36] argued that happiness was an independent factor in an individual's experience that consisted of three other relatively independent factors: life satisfaction, presence of positive emotions, and absence of negative emotions. Among the commonly mentioned major determinants of the state of happiness are the following: optimism as an attitude to the positive development of events, availability of a life goal, high and solid self-esteem. These three determinants are regarded as fundamental components of positive thinking.
On one side, most researchers consider optimism the key ingredient of happiness. Argyle argues that most people have an overall positive attitude towards the world. Yet, some individuals are more optimistic in their expectations than others.
On the other side, happiness is regarded by most researchers, including us, as the affective component of subjective psychological well-being (i.e. the presence/absence of positive/negative emotions), whereas life satisfaction is considered its cognitive component [37,38,39,40,41].
Life satisfaction is "the degree to which a person positively evaluates the overall quality of his/her life as a whole." [39] or "[A]n overall assessment of feelings and attitudes about one's life at a particular point in time ranging from negative to positive" [37]. It represents the difference between individual expectations, intentions, needs and desires and the degree to which these are met [27].
Life satisfaction is a global evaluation of one's life. It is perceived as an overall psychological situation. Unlike the state of happiness, which is immediate and short, life satisfaction is not grounded in any specific life domain or at any particular moment [39].
Obviously, meeting individual needs and anticipations results in satisfaction with life, while the reverse—in dissatisfaction with life.
There are two main types of theory on life satisfaction, which are closely related, yet debated:
1. Bottom-up theories: life satisfaction is an overall result of satisfaction with many areas of life —work, relationships, family and friends, personal development, health and fitness.
2. Top-down theories: overall life satisfaction determines domain-specific satisfaction [42].
Anyway, life satisfaction is associated with individual experiences, daily life and future expectations in various areas of life. Furthermore, changes that an individual would like to implement in life are influenced by their efforts to distinguish their own life from the attitudes and behaviour of others towards them and their life [38].
Life satisfaction can be boosted through a number of factors such as setting feasible goals and plans for future, having pleasant and peaceful work and home environments, enough spare time, hobbies, exercise activities, close friendships, good social-economic standing, good health and health-wise behaviour.
As long as migration is driven mostly by an individual's desire to improve their well-being, we have focused this particular study onto the role of life satisfaction and optimistic expectations as main components of subjective psychological well-being in shaping emigration attitudes.
1.3. Emigration attitudes and emigration behaviour
In broad terms, migration is the movement of an individual from one place of residence to another. For demographic purposes, it is usually regarded as a movement that results in a long-term or permanent change in the usual place of residence [43,44]. The act of changing one's place of residence out of the political boundaries of a country with the intention to reside in another country, is considered emigration in view of the sending country, but immigration in view of the host country. Most countries apply a 183-day rule (6 months) to determine if someone should be considered a resident for tax purposes. Otherwise, the broader term mobility is usually used to indicate a shorter term of relocation.
There are not a few theories of attitudes and concepts about how they relate to behavior, which may be applied to emigration attitudes as well. Both conceptualization and operationalization of emigration attitudes in this study are based on the classical Tripartile Theory of Attitudes, also called the ABC model [45,46,47,48], and also on the extension of Schwarz's Construal Model [49].
According to the tripartile theory, an attitude has its affective, cognitive and behavioral components. The affective component refers to an individual's emotional or affective response to a particular object, person, or idea. For example, an individual may have a positive or negative emotional response to the idea of emigration. The behavioral component refers to an individual's behavioral tendencies/intentions towards a particular object, person, or idea. For example, an individual may have a tendency/intention to emigrate for future. The cognitive component refers to an individual's beliefs, knowledge, and thoughts about a particular object, person, or idea. For example, an individual may have a set of positive beliefs about emigration, emigrants, other countries, languages, etc.
The tripartite theory of attitudes suggests that these three components work together to form an individual's overall attitude towards a particular object, person, or idea such as emigration. Moreover, the theory proposes that each of these components can be measured separately and can influence behavior in different ways [46,47]. For instance, an individual's affective component may be more influential in shaping their emigration behavior in the short term, whereas their cognitive component may play a more significant role in shaping their emigration behavior over the long term. Although this classical theory has been subjected to not a few further extensions, understanding the tripartite nature of emigration attitudes can still help researchers suggest measures to prevent emigration behavior through changing emigration attitudes.
The construct of migration attitude is very similar to the so-called "migration potential" [50]. Gallup World Poll [50] used a few questions to capture different aspects of migration potential, including an individual's desire to migrate, their certain plans to migrate in the near future, and their preparation for migration. The desire, plan and preparation to move are also regarded as key aspects of the process of emigration decision-making, also called "pre-migration stage".
Attitudes may be positive, negative [51] or neutral. It is important to note that positive migration attitudes may or may not result in actual migration behavior [52] due to a complex of factors that are not only economic, political, sociocultural, but also psychological in nature.
The extension of Schwarz's Construal Model [49], which we have also employed in the study, is among the latest theories trying to explain the attitude-behaviour relationship. The model emphasizes the importance of the context in which attitudes are shaped and expressed. Schwarz argues that attitudes are dynamically changing constructs that are continually constructed and reconstructed in response to the current situation. The theory suggests that attitudes are formed and expressed based on three types of information: (1) information about the attitudinal object, (2) information about the context in which the attitude is shaped and expressed, and (3) information about the person who is forming and expressing the attitude.
Furthermore, the model suggests that the same attitude object can be evaluated differently depending on the context in which it is presented. For example, an attitude toward emigration may differ depending on whether it is shaped and expressed under pandemic, war or other specific conditions; depending on conditions in the destination country; depending on the stage of planning and preparation for emigration, etc [49].
Of course, there are other recent sociocognitive theories such as the Theory of Reasoned Action [53] and its extension—the Theory of Planned Behaviour [54] that also try to explain the relationship between attitudes and behaviour and that can also be applied to emigration.
According to the Theory of Reasoned Action (TRA) an individual's behaviour is determined by their intention to perform that behaviour, which is in turn influenced both by their attitude towards the behaviour and by subjective norms. The theory suggests that an attitude is formed by an individual's beliefs about the likely outcomes of performing a behaviour and the evaluation of those outcomes.
In terms of emigration, the TRA would suggest that an individual's intention to emigrate is influenced by their attitude towards emigration, which is formed by their beliefs about the likely outcomes of emigration and the evaluation of those outcomes. For example, an individual who believes that emigration will lead to a better standard of living is more likely to have a positive attitude towards emigration than someone who believes that emigration will lead to negative outcomes.
According to TRA, subjective norms are based on an individual's perception of the social pressure to emigrate or not emigrate, based on the beliefs of important others such as family members, friends, and peers. For example, an individual who perceives that their family and friends support emigration may have a higher intention to emigrate than someone who perceives that their family and friends are against emigration [53].
According to the Theory of Planned Behaviour (TPB) an individual's intention to perform certain behaviour, which then can lead to actual behaviour, is determined not only by their attitude and subjective norms, but also by the perceived behavioural control, i.e. the individual's perceived ability (ease or difficulty) to perform the behaviour in question. In terms of emigration, the theory proposes that the stronger an individual's intention to emigrate, the more likely they are to actually emigrate. However, the theory also recognizes that there are other factors that may influence behaviour, such as external factors beyond an individual's control, habits, and emotional factors [54].
Both TRA and TPB are useful frameworks for understanding and predicting human behaviour, particularly in relation to behaviors that are intentional and under an individual's control. Their limitation is that they assume individuals are rational decision-makers who consider all factors when deciding whether or not to engage in a particular behaviour.
Some researchers suggest that due to complexity of the phenomenon of emigration attitude and its relation to emigration behaviour it is important to distinguish between active emigrants and ideological but impeded emigrants [55].
There are a few studies on generational differences in emigration attitudes of young people. These studies suggest that younger generations have more positive attitudes towards mobility and emigration in comparison to older generations, possibly due to their better opportunities abroad, the greater exposure to globalization, international influences and experience [9,10,11]. However, research evidence on generational differences in emigration attitudes of young Bulgarians is not available to date.
1.4. Life satisfaction, optimistic expectations and emigration attitudes: previous research evidence on the interrelations
There are several reasons to gain a better understanding of the relationship between life satisfaction and optimistic expectations of young people attuned to emigration. Firstly, regional and national authorities around the world have become increasingly aware that subjective well-being is a key factor for the development of policies for individual and societal welfare [56]. Secondly, it was found that the higher degrees of subjective psychological well-being result in higher productivity [57] and creativity [7].
Some researchers explored if an experience with emigration would make people happier [5] and if emigration would have an effect on the welfare of both sending and receiving countries [58], on physical health [59], on sociability, the quality of social relations, social capital and social behaviour [60], on the likeliness to be employed and promoted, and also on the probability to obtain higher education and income [7].
On one hand, any receiving country should be interested in the degree of immigrants' well-being. More productive, healthier, and more sociable immigrants are likely to exert less pressure over the host country's social system, for they can integrate themselves easier into the accepting society in comparison to those who are less productive, ill and uncommunicative. On the other hand, the receiving countries may gain well-being through immigrants, while the sending countries may lose well-being through emigrants.
Most studies, which explore the relationship of subjective well-being (happiness and life satisfaction) and emigration intentions at the individual level, show that people who score lower on psychological well-being have stronger desire to emigrate in comparison to those who score higher.
For example, secondary data analyses performed by Cai et al. [4] on Gallup World Survey data from 116 countries showed a negative correlation between life satisfaction and the desire to emigrate. In other words, a lower degree of satisfaction with life was associated with stronger desire to emigrate. Similar were the findings from secondary data analysis of survey data from 5 rounds (2004, 2006-2009), including 18 countries and over 90000 individual interviews with respondents from Latin America. The higher degree of happiness is associated with lower willingness to emigrate [7,61,62].
Ivlevs (2015) [7] examined the differences in the relationship of life satisfaction and emigration intentions between the 15 old EU member countries (from Western Europe) and the 10 latest EU member countries (from Central and Eastern Europe, including Bulgaria), which accessed the EU in 2004 and 2007. The differences in emigration intentions between people who were the least satisfied and the most satisfied with life turned out larger in the western EU countries. This finding suggests that dissatisfaction with life is a stronger drive for emigration in wealthier countries.
Furthermore, individuals who are not so happy and satisfied with life, are more likely to emigrate, if they are highly educated. Personality tendencies such as achievement motivation and risk propensity may also influence subjective well-being and emigration desire [6,7].
In line with the findings above, cross-cultural comparisons based on Gallup's data [50] showed that subjective well-being was a stronger negative predictor of emigration desire for wealthier countries compared to poorer countries. At the continental level, the negative relation between subjective well-being and emigration desire was found to be stronger in Europe compared to the other continents. Subjective well-being was also found to be a stronger predictor of emigration desire in comparison to household income, still considering income a key component of subjective well-being [4].
Otrachshenko & Popova [3] carried out secondary analyses of Eurobarometer data to find out that citizens of Central and Eastern Europe were less satisfied with life and more inclined to emigrate either abroad or within their country of origin in comparison to those from Western European countries.
Somewhat more complicated was the data obtained by Polgreen & Simpson [63], who studied the interrelation of life satisfaction and emigration at the country level. The researchers found out that emigration rates from countries with a relatively low index of subjective well-being were decreasing, whereas the average degree of happiness in the countries was increasing. And the reverse was also true for countries with a relatively high index of well-being. Consequently, the highest emigration rates were observed for the countries with the highest and the lowest index of subjective well-being. In other words, serious intentions to relocate abroad were expressed by those who were the most and the least satisfied with life. The interrelation of life satisfaction and emigration varied a bit across countries and cultures. These findings suggested a U-shaped relationship between life satisfaction and emigration attitudes, i.e. very satisfied and very unsatisfied people were most likely to emigrate over the next year, whereas people close to the mean value of the distribution curve of life satisfaction were the least likely to emigrate.
Some seemingly contradictory findings on the relationship between life satisfaction and emigration attitudes can be explained by the push-pull theory of migration, developed by E. Lee [43]. According to the theory, the reasons for migration are determined by the so-called "push" and "pull" factors. These factors are either necessity-driven or opportunity-driven and these can be some economic, political, cultural and/or environmental conditions.
Push factors are regarded as conditions that can force individuals to leave their place of residence. These are usually conditions in the country from which a person migrates, such as a low quality of life and poor living conditions, low income and poverty, poor healthcare, loss of wealth/job, rapid population growth that exceeds available resources or depopulation that leaves people without means of livelihood/occupation, (fear of) political, religious or otherwise persecution, famines, droughts, desertification, natural disasters, etc.
Pull factors are exactly the opposite of push factors. They attract people to move to a certain place and are perceived as opportunities. Some examples of pull factors are better living conditions and job opportunities, higher income, superior system of education and healthcare, better transportation and communication facilities, political, religious and otherwise freedoms, easy availability of land for settling, stress-free environment, security, etc.
De Jong [64] argued that the intention to relocate was the basic determinant of migration behaviour, while Burda and colleagues [65] argued that these intentions were a function of the variables that motivated emigration. Of course, the intention, plan and desire to emigrate does not necessarily result in an act of emigration. For example, Van Dalen & Henkens [66] found out that only one third of the Dutch citizens, who declared their desire to move abroad, have relocated in another country over the following five years. Still, emigration attitudes, incl. desire, intention and plan to emigrate, can be good predictors of emigration behaviour.
Economists and other social scientists usually regard emigration decision-making as an investment: one will move abroad if gains outweigh losses. According to this hypothesis, emigration gains and losses are usually represented by the overall income that one can gain in their own country and in another country. The basic assumption is that the poorer are the people the more likely they are to emigrate. However, income is not the only thing that individuals would like to maximize. For example, if we consider that: 1) they increase their life satisfaction rather than their income and 2) they believe that the higher degree of life satisfaction will be accomplished abroad, we will assume that individuals, who score low on life satisfaction, are more likely to emigrate compared to those who are satisfied with life, since the former may get more life satisfaction with their relocation ([7], p. 6). Of course, it is based on the assumption that one believes that they will be more satisfied with life only if they move abroad. Perhaps, individual beliefs (expectations) are shaped by friends, family members and the media that convey messages about the experience of others who have already emigrated. Still, these experiences may suggest either higher or lower life satisfaction while living abroad.
As long as the losses from emigration are concerned and according to most income hypotheses, the poorest people often do not emigrate, because they need certain income to cover emigration expenses (travelling, lodging, etc.). Similarly, we may assume that a certain degree of well-being/life satisfaction is needed to enhance emigration [63,67]. It is still controversial whether emigrants need to be optimistic, self-confident, open and sociable to cross the borders and to adapt to new environments. Since these personality traits are relating to well-being, life satisfaction and happiness [36,21], we can also expect a positive correlation between well-being (life satisfaction) and emigration.
Some studies support the hypothesis that emigrants have certain personality traits associated with well-being. For example, Boneva & Frieze [68] found out that emigrants from Eastern Europe had higher achievement motivation (willingness to excel, to meet challenges, to do something unique) and also a stronger drive to be recognized by others, in comparison to non-emigrants. Polgreen & Simpson [63] also argue that happier people tend to have more optimistic expectations, to be more adventurous, and ready to look for new opportunities abroad compared to individuals who are not happy [63,68].
Another possibility to illustrate the positive relationship between life satisfaction and emigration is to look back to the traditional version of the neoclassical model—the assumption that emigration results in higher satisfaction with life. Since many people associate emigration with higher income, they actually increase their income and hence, indirectly their life satisfaction and well-being [60]. Consequently, the positive association between emigration (attitudes) and life satisfaction is more likely in poor countries, because income can be increased with relocation to another country. Furthermore, in countries, which hinder individual development through ineffective governmental policies and restricted mobility opportunities, people who are achievement-oriented, entrepreneurial, and freedom-loving may consider emigration a strategy for improving their social-economic standing [69].
In brief, either negative or positive relationship between subjective well-being (incl. life satisfaction, optimism and happiness), emigration attitudes and the act of emigration is possible. This relation may prove to be stronger in poorer countries, and also in countries with ineffective governmental authorities and limited opportunities for personal and career growth.
Analyses by social-demographic characteristics [7] show that women, elders, individuals in marital/committed relationships, and those with health issues report a weaker desire for emigration in comparison to men, young and healthy people, singles, and individuals who have contacts with emigrants.
In terms of personality tendencies, the preparation for emigration may temporarily make an individual more or less happy and/or satisfied with life in comparison with their baseline of happiness and/or life satisfaction. For example, a prospect emigrant, who anticipates separation from friends and family, is likely to feel insecure and worried, and hence to report a lower degree of subjective well-being than usual. Still, their happiness and/or life satisfaction is likely to switch back to the baseline after relocation [69].
Changes in individual circumstances such as worsening relationships with family members or problems at the workplace may make people think about relocation to another country and at the same time they may temporarily decrease life satisfaction and the experience of well-being [7].
2.
Methods
2.1. Research design and sampling
This study is а part of a larger research project (See Acknowledgements) aimed at exploring psychological determinants of young people's attitudes to emigration and life planning in the context of demographic challenges in Bulgaria. To implement this particular cross-sectional study, we performed a quantitative data analysis of a structured questionnaire survey.
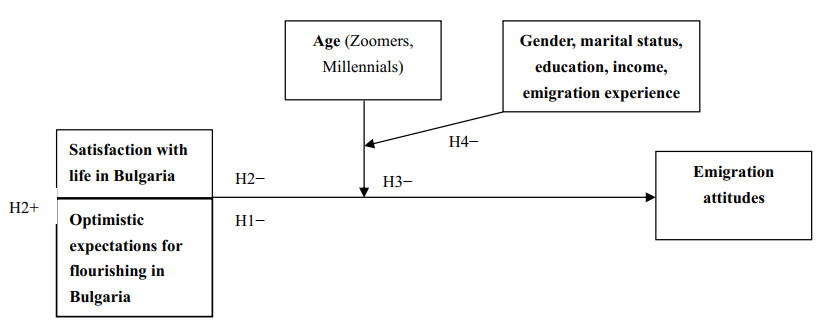
Grounding on literature overview, previous research evidence and common sense, we hypothesized that [H1] optimistic expectations of young people for their own development in Bulgaria would be a significant antecedent of their emigration attitudes and also that the relationship between the two constructs would be negative. We also assumed that [H2] satisfaction of young people with life in Bulgaria would be a significant predictor of their emigration attitudes and also that life satisfaction would be negatively associated with emigration attitudes and positively with optimistic expectations. Furthermore, we hypothesized that [H3] there would be differences in the effects of optimistic expectations and life satisfaction on emigration attitudes between Bulgarian Zoomers and Millennials, and also that age (generation) would moderate these effects significantly. And finally, we assumed that [H4] Zoomers, men, singles, young people who had previous emigration experience, lower level of education, no (good) income would be significantly more likely to emigrate from Bulgaria compared to Millennials, women, young people in marital/committed relationships, without prior emigration experience, higher level of education, and good/high income (See Figure 1).
The structured survey questionnaire, which we have employed, included measures and scales developed on the basis of both literature overview and qualitative (thematic and relational content) data analysis of the semi-structured calendar interviews (N = 45), carried out in June - November 2020 among young Bulgarian men (N = 21) and women (N = 24) aged 18-35 years from large, middle and small towns/villages in Bulgaria.
In June 2021, an online piloting survey on the social media was carried out among young Bulgarians aged 18-35 years (N = 79) using both convenience and snowball sampling methods to test psychometric properties of the scales. In September-October 2021, using the improved versions of the scales, we conducted a large survey (N = 1200), representative of the young Bulgarians aged 18-35 years, who lived in the country at the time of the research. Although the representative sample has not been controlled for generational criteria, it is large enough to provide data for analyzing age differences between Bulgarian Generation Y (Millennials), born between 1981-1995/6 (N = 756) and aged 26-35 years at the time of the study, and Bulgarian Generation Z (Zoomers), born between 1996/7-2012 (N = 444) and aged 18-25 years at the time of the study.
The representative data collection was subcontracted to an agency for social, political and marketing research, which had their own network of interviewers and supervisors on the territory of the country. The data was collected via face-to-face structured interviews in the respondents' places of residence, observing all anti-pandemic measures at the time of research. A two-stage probability sampling method was employed. Firstly, 240 clusters (voting sections) were selected from large, middle and small Bulgarian towns, which were proportional to the number of their population. Secondly, a systematic sampling method was employed at regular intervals from the sampling frame, including 5 respondents from each cluster controlled for gender and age so that the interviewees were equally distributed by gender for any settlement type. This two-stage sampling procedure ensured decreased risk of sampling bias, i.e. ± 2, 83% at 95% CI for a relative share of 50%. Furthermore, the pre-existing stratification of the sampling database by place of residence ensured proportional representation of all regions in the country.
Herein below, we present only those measures and scales from the survey questionnaire that were needed to test the hypotheses and to carry out the quantitative data analysis relating to the objective of this particular study: Optimistic Expectations for Flourishing in Bulgaria Scale, Satisfaction with Life in Bulgaria Scale, and Emigration Attitudes Scale.
2.2. Optimistic Expectation for Flourishing in Bulgaria Scale (OEFBS)
The Optimistic expectations for Flourishing in Bulgaria Scale (OEFBS) was developed on the basis of the literature overview and content analysis of the calendar interviews data. It consists of 5 items measured on a 5-point Likert scale ranging from 1 = Strongly Disagree to 5 = Strongly Agree.
The scale is characterized by good psychometric properties, including reliability (Cronbach's α = 0.75), external and convergent validity. All inter-item correlations were significant (p < 0.01) and moderate in strength. PCA showed 50.26% cumulative loadings onto a single extracted factor that was identified as "optimistic expectations for flourishing in Bulgaria" (See Table 1).
1 The structured survey questionnaire, including Optimistic Expectations for Flourishing in Bulgaria Scale (OEFB), Satisfaction with Life in Bulgaria Scale (SLBS), Emigration Attitudes Scale (EAS), was administered in Bulgarian language. All items of the original scales were (back)translated by the authors and two independent experts.
2.3. Satisfaction with Life in Bulgaria Scale (SLBS)
The Satisfaction with Life in Bulgaria Scale (SLBS) is also an original scale that consists of 4 items measured on a 5-point Likert scale ranging from 1 = Strongly Disagree to 5 = Strongly Agree.
The scale is characterized by good psychometric properties, including reliability (Cronbach's α = 0.75), external and convergent validity. All inter-item correlations were significant (p < 0.01) and moderate in strength. PCA showed 57.63% cumulative loadings onto a single extracted factor that was identified as "satisfaction with life in Bulgaria" (See Table 2).
2.4. Emigration Attitudes Scale
The Emigration Attitudes Scale (EAS) was also developed on the basis of the literature overview and content analysis of the calendar interviews data. It consists of 5 items measured on a 5-point Likert scale ranging from 1 = Strongly Disagree to 5 = Strongly Agree.
The scale is characterized by very good psychometric properties, including reliability (Cronbach's α = 0.86), external and convergent validity. All inter-item correlations were significant (p < 0.01), varying from moderate to strong −0.441 < r < 0.654. PCA showed 64.65% cumulative loadings on a single extracted factor "emigration attitudes" (See Table 3).
Consequently, all three measures and scales above are both reliable and valid enough for further analyses.
2.5. Sample distributions: sociodemographic characteristics
To test the last hypothesis, differences in emigration attitudes have been also analysed by sociodemographic characteristics such as gender, marital status, education, income, and previous emigration experience (See Table 4).
2.6. Statistical methods
The quantitative data of the study was processed using IBM SPSS v25.0. Firstly, we used reliability analysis, Pearson's r and principal component analysis (one component solution without rotation) to test psychometric properties (reliability and validity) of the measures and correlations between optimistic expectations, life satisfaction and emigration attitudes. Secondly, we used descriptive statistics, including normality tests (Shapiro-Wilk), and the Independent-Samples Mann-Whitney U Test for non-normal data distribution to examine age and gender differences in optimistic expectations, life satisfaction and emigration attitudes, as well as differences in emigration attitudes of Zoomers and Millennials depending on emigration experience, income, education and marital status. Thirdly, we employed linear regression (OLS) and multiple regression (stepwise method) to test our hypothesis about the effects of optimistic expectations and satisfaction with life in Bulgaria as predictors of emigration attitudes of young Bulgarians from both Z and Y generations. Finally, we set age as a moderator to examine the conditional effect of optimistic expectations and life satisfaction on emigration attitudes.
2.7. Ethics approval of research
The research was carried out in compliance with the Code of Conduct of the authors' affiliation—Department of Psychology, Institute for Population and Human Studies—Bulgarian Academy of Sciences, and also in conformity to the contractual agreements both with the funding institution (See Acknowledgments) and with the subcontractor's agency—a private company specialized in carrying out social, marketing and political research, and responsible for the quantitative data collection among the full-age young Bulgarians (N = 1200; 18-35 y.o.). Written consents were obtained by the subcontractor's agency in accordance with the agreement, the law and the regulations on personal data protection EU policies, applicable in Bulgaria as an EU member country. The categorization of respondents by socially constructed categories such as age, gender, marital status, education, income was based on the respondents' self-report.
3.
Results
Descriptive data analyses on optimistic expectations, life satisfaction and emigration attitudes of the Bulgarian population aged 18-35 years, including Shapiro-Wilk normality tests, revealed nonnormal data distributions. Consequently, we employed nonparametric tests (Independent-Samples Mann-Whitney U Test) to compare distributions across the two age groups—Zoomers and Millennials, considering that nonparametric tests do not assume that data follows the normal distribution (See Table 5).
The Independent-Samples U Tests showed that there were significant differences both in optimistic expectations and in emigration attitudes between Bulgarian Zoomers and Millennials. Zoomers, i.e. those aged 18-25 years, were found to be significantly more optimistic about their own future development in Bulgaria, but also significantly more likely to emigrate in comparison to Millennials, i.e. those aged 26-35 years. However, significant differences in satisfaction with life in Bulgaria between Generations Z and Y were not found (p > 0.05). Gender differences in optimistic expectations, life satisfaction and emigration attitudes were found neither (p > 0.05).
We carried out further Independent-Samples Mann-Whitney U Tests to examine differences in emigration attitudes of Zoomers and Millennials with regard to some other sociodemographic factors such as previous emigration experience, income, education and marital status (See Methods and Procedure). Significant differences were found for almost all studied sociodemographic factors with the exception of marital status (See Table 6).
The results showed that: 1. both Zoomers and Millennials with previous emigration experience were significantly more positive about emigration in comparison to those without prior emigration experience; 2. both Zoomers and Millennials with primary/secondary degree of education were significantly more attuned to emigration compared to those with Bachelor's or higher educational degree; 3. only Zoomers, but not Millennials, with no/low own income were significantly more positive about emigration in comparison to those Zoomers who had good/high own income. A specific pattern of missing values of the "income" variable was not observed.
To test our hypothesis about the direction of the relationship between optimistic expectations, life satisfaction and emigration attitudes, we performed Pearson's r correlation analysis that was appropriate for interval data in terms of overall Likert scale scores (See Table 7).
The results above show that there are significant correlations between the three constructs. Satisfaction with life in Bulgaria is positively and strongly associated with optimistic expectations of young people for their future development in the country and they both are negatively and moderately associated with young people's attitudes to move abroad.
Since the linearity assumption for the relationship between all studied variables was met, multiple linear regression (OLS, stepwise method) was employed to study the effect of both optimistic expectations and life satisfaction on emigration attitudes of the representative sample (18-35 y.o.), as long as the method did not require the normality assumption. Furthermore, no collinearity of the independent variables was observed and the residuals were normally distributed. Results showed that both Model 1 (optimistic expectations for flourishing in Bulgaria) and Model 2 (both optimistic expectations for flourishing in Bulgaria and satisfaction with life in Bulgaria) were fit. However, Model 1 was fitter −R2 = 0.09, F (1, 1198) = 111.63, p = 0.000, compared to Model 2 − R2 = 0.10, F (2, 1197) = 64.64, p = 0.000. In other words, optimistic expectations for flourishing in Bulgaria (Model 1) had a stronger (moderate and negative) main effect on emigration attitudes and it explained approximately 9% of the variance of emigration attitudes scores, compared to life satisfaction (included in Model 2 together with optimistic expectations), whose effect on emigration attitudes was also negative, but weak, and which added only 1% to the variance explanation (See Table 8).
Regarding age differences in the effect of both optimistic expectations and life satisfaction on emigration attitudes between Zoomers (Z) and Millennials (Y), multiple linear regression was employed by setting the 2-categorical variable "age" (Z/Y) as a selection variable. The results showed that both Model 1 and Model 2 for Zoomers and Millennials were different, even though all four models proved to be fit. Interestingly, Model 1 for Zoomers encompassed only the main effect of optimistic expectations −R2 = 0.11, F (1, 442) = 53.87, p = 0.000 that explained approximately 11% of the emigration attitudes' variance and Model 1 for Millennials included only the main effect of life satisfaction −R2 = 0.08, F (1, 754) = 65.38, p = 0.000 that explained approximately 8% of the construct variance. In Model 2 for Zoomers life satisfaction added only 1% to the explanation of the construct variance −R2 = 0.12, F (2, 441) = 29.89, p = 0.000 and in Model 2 for Millennials optimistic expectations also added approximately 1% to the construct variance explanation −R2 = 0.09, F (2, 753) = 38.81, p = 0.000 (See Table 9).
However, a significant interaction effect of either optimistic expectations or life satisfaction and age on emigration attitudes was not found (p > 0.05).
4.
Discussion
Results of this cross-sectional study representative of young people aged 18-35 years born and resident in Bulgaria - an Eastern EU country with longstanding and increasing negative net migration rates for that age group [1], showed that in October 2021 young Bulgarians held predominantly negative emigration attitudes, especially towards permanent emigration. In support to one of our hypotheses [H4] Zoomers (18-25 y.o.) turned out significantly more likely to move abroad compared to Millennials (26-35 y.o.).
Furthermore, Bulgarian Zoomers were found to be more optimistic about their educational opportunities, financial security and career prospects in the country, compared to Bulgarian Millennials. At the same time, both Zoomers and Millennials were similarly satisfied with life in Bulgaria, including the same areas of life. On one hand, these results are in contrast to research evidence from other countries showing lower optimism and well-being of Zoomers, compared to Millennials [12,13]. On the other hand, the findings come to support the notion that life satisfaction and optimism/happiness are clearly distinguished constructs, that should be measured separately and respectively regarded as cognitive and affective components of subjective well-being [37,38].
Contrary to the hypothesis above and to data from the National Statistical Institute [1], which showed a higher number of emigrant men than emigrant women from Bulgaria from 2014 to 2020 on an annual basis, we did not find gender differences in emigration attitudes of young Bulgarians in the autumn of 2021. Gender differences in neither optimistic expectations nor life satisfaction were found. On one hand, it is in line with the longstanding discussion about the incongruence between attitudes and behavior. Other researchers also found that migration attitudes might or might not result in actual migration behavior [52], but they could still be a good predictor of migration behavior [50]. On the other hand, the findings may imply that emigration attitudes of young Bulgarian men are more likely to translate into actual emigration behavior, compared to emigration attitudes of young Bulgarian women. On the third hand, emigration attitudes of people around the world, including those of young Bulgarians, should have been very dynamic in the latest years. In line with Schwarz's Construal Model, which emphasizes both contextual and dynamic nature of attitudes, emigration attitudes have been probably changing even more since October 2021 due to the fourth COVID-19 pandemic wave followed by the war in Ukraine from February 2022 onwards.
In support to our hypotheses [H1 and H2], as well as to most earlier research evidence [4,3,6,7] we found a positive relationship between optimistic expectations and life satisfaction, and they both turned out negatively associated with emigration attitudes. In other words, the higher degrees of optimism in young people for their social-economic prospects in Bulgaria are associated with stronger satisfaction with life in Bulgaria and also with more negative attitudes towards relocation abroad. Although both optimistic expectations and life satisfaction as personality characteristics were found to be significant psychological antecedents of emigration attitudes among young Bulgarians (18-35 y.o.), optimistic expectations turned out a stronger negative predictor of their attitudes compared to satisfaction with life in Bulgaria, especially among Zoomers (18-25 y.o.).
In support to our hypothesis [H3], we found age differences in the effect of both optimistic expectations and life satisfaction on emigration attitudes between Bulgarian Zoomers and Millennials. On one hand, optimistic expectations for future flourishing in the country turned out a stronger negative predictor of emigration attitudes of Zoomers (18-25 y.o.) than satisfaction with life. On the other hand, life satisfaction was found to be a stronger negative predictor of emigration attitudes among Millennials (26-35 y.o.) in comparison to optimistic expectations. Although a significant interaction effect of either optimistic expectations or life satisfaction and age on emigration attitudes was not found, the predictive power of both optimistic expectations and life satisfaction was weaker for Millennials compared to Zoomers. In other words, negative emigration attitudes of younger people (Zoomers) can better be explained by the stronger tendency to see their prospects for flourishing in the country through rose-coloured glasses, compared to the more mature individuals (Millennials), whose attitudes are determined by a more realistic and global evaluation of one's life [39], including assessment of the difference between individual expectations, intentions, needs and desires and the degree to which these are met [27]. On one hand, this finding may be interpreted in the light of the push-pull theory of migration [43], i.e. by the wider range of job and career opportunities available to younger Bulgarians compared to those available to older ones both in the country and abroad under the conditions of global crisis. On the other hand, the result may be interpreted in terms of perceived opportunities, rather than ones actually available to younger people, due to their tendency to be less experienced, more achievement-oriented and more opportunistic in various life domains, in comparison to older people [15]. These findings have implications for both theory and policies on human migration, since they outline age-specific psychological antecedents of emigration attitudes and hence, point to age-specific measures to change these attitudes so as to prevent potential emigration behaviour of young people.
As mentioned earlier, our hypothesis [H4] regarding some sociodemographic differences in emigration attitudes of young Bulgarians was partially supported. In support to the hypothesis both Zoomers and Millennials with previous emigration experience turned out significantly more positive about emigration in comparison to those without prior emigration experience. Furthermore, both Zoomers and Millennials with lower (primary or secondary) degree of education were significantly more likely to emigrate compared to those with Bachelor's or higher educational degree. However, only Zoomers with no/low own income were significantly more positive about emigration in comparison to those Zoomers who had good/high own income. In contrast to our assumptions and previous research evidence: 1. Millennials, who had no/low income were similarly attuned to emigration as those Millennials who had good/high income and 2. no significant within-group differences in emigration attitudes were found for both Zoomers and Millennials who were single/living alone and those who were married, in a committed relationship or in cohabitation with another person.
The absence of income differences in emigration attitudes of Millennials (unlike Zoomers) may be explained by some age or intergenerational value differences. Some researchers argue that Zoomers are more achievement-oriented, entrepreneurial and risk-taking than older generations [15]. According to our opinion these characteristics may turn out age-specific rather than generation-specific. Still, it may serve as an implication for future longitudinal research.
5.
Limitations and implications for further research
The study has some limitations. Firstly, two of the measures that we employed—Optimistic Expectation for Flourishing in Bulgaria Scale and Satisfaction with Life in Bulgaria Scale, were original, but context-specific. They were administered in Bulgarian language and were specifically designed to measure the two constructs in Bulgarian cultural conditions. Consequently, further replication research is advisable to test the external validity of both measures in other cultural contexts. Furthermore, the original Emigration Attitudes Scale may be expanded so as to encompass measures of subjective norms and perceived behavioral control in terms of the Theory of Planned Behavior [53], as well as some contextual measures in the light of Schwarz's Construal Model [49].
The second limitation of the study, which may also be regarded as its strength and implication for future research, is the fact that it is a cross-sectional study carried out under unprecedented global pandemic conditions. On the challenging side, the results may not apply to other (e.g. usual, non-pandemic or war) conditions. Neither they may apply to other cultural contexts, since the issue of emigration concerns different cultural contexts. On the positive side, carrying out research under such unusual pandemic conditions, just before a lockdown threat, provides any further replication studies with a rare chance to compare the findings and make a highly reliable meta-analysis of psychological antecedents of emigration attitudes under different conditions.
6.
Conclusions
In summary, in the autumn of 2021 young Bulgarians (18-35 y.o.) held predominantly negative emigration attitudes, especially towards permanent emigration. On average, they were rather optimistic about living and achieving in their country of origin. It is important to note that the findings outline young people's optimistic expectations about their own development in the country, rather than their optimistic expectations for advancement of the country itself.
However, significant age differences in emigration attitudes were found. On one hand, younger Bulgarians, the so called Zoomers, were found to be more positive about moving abroad, regardless of their gender and marital status, but also more optimistic about their own future flourishing in Bulgaria, in comparison to the more mature Millennials. On the other hand, beyond the objective economic and educational reasons, the willingness of young Bulgarians to emigrate could also be explained by psychological factors such as not so optimistic expectations for one's own development in the country, dissatisfaction with life in the country and any prior emigration experience. Generally speaking, the more pessimistic and dissatisfied with life in Bulgaria young people are, the more positive they are about relocation to another country, especially 18-25 y.o., individuals, who have some emigration background, no/low income and primary/secondary education.
The findings above, as well as the evidence that optimistic expectations for individual development in Bulgaria can serve as a better explanation of Zoomers' emigration attitudes compared to those of Millennials, and satisfaction with life in the country proves to be a better explanation of Millennials' emigration attitudes compared to those of Zoomers, can be used to develop age-specific measures to efficiently predict and change emigration attitudes of young Bulgarians and hence, try to prevent further deepening of the demographic crisis in terms of negative net migration rates for Bulgaria through development of appropriate social and economic policies and measures to enhance the quality of life, educational and career opportunities for young Bulgarians in their native country. These measures should address not only citizens and residents, but also returnees and circular migrants, who are still searching for their vocation and career, and who are still hopeful to flourish in Bulgaria.
Acknowledgments
This research was carried out within the framework of Project КП-06-Н35/4 "Psychological determinants of young people's attitudes to emigration and life planning in the context of demographic challenges in Bulgaria", funded by the Bulgarian National Science Fund, Ministry of Education and Science, Bulgaria; implemented by the Department of Psychology, Institute for Population and Human Studies—Bulgarian Academy of Sciences; 18 December 2019-18 June 2023.
Conflict of interest
The authors declare no conflicts of interest in this paper.









 DownLoad:
DownLoad:




