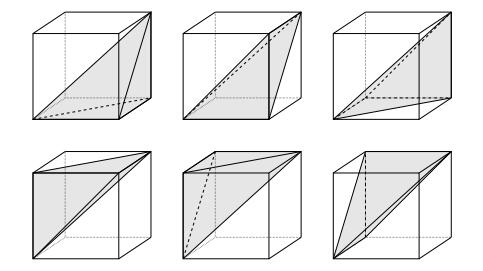
The six tetrahedra in the Kuhn decomposition of a three-dimensional cube
.The purpose of this paper is to investigate the oscillatory behaviour of a class of first-order sublinear and superlinear neutral difference equations. Some conditions are established by applying Banach's Contraction mapping principle, Knaster-Tarski fixed point theorem and using several inequalities. We provide some examples to illustrate the outreach of the main results.
Citation: Mohamed Altanji, Gokula Nanda Chhatria, Shyam Sundar Santra, Andrea Scapellato. Oscillation criteria for sublinear and superlinear first-order difference equations of neutral type with several delays[J]. AIMS Mathematics, 2022, 7(10): 17670-17684. doi: 10.3934/math.2022973
| [1] | Roberto Alicandro, Giuliano Lazzaroni, Mariapia Palombaro . Derivation of a rod theory from lattice systems with interactions beyond nearest neighbours. Networks and Heterogeneous Media, 2018, 13(1): 1-26. doi: 10.3934/nhm.2018001 |
| [2] | Roberto Alicandro, Andrea Braides, Marco Cicalese . Phase and anti-phase boundaries in binary discrete systems: a variational viewpoint. Networks and Heterogeneous Media, 2006, 1(1): 85-107. doi: 10.3934/nhm.2006.1.85 |
| [3] | Mathias Schäffner, Anja Schlömerkemper . On Lennard-Jones systems with finite range interactions and their asymptotic analysis. Networks and Heterogeneous Media, 2018, 13(1): 95-118. doi: 10.3934/nhm.2018005 |
| [4] | Thomas Hudson . Gamma-expansion for a 1D confined Lennard-Jones model with point defect. Networks and Heterogeneous Media, 2013, 8(2): 501-527. doi: 10.3934/nhm.2013.8.501 |
| [5] | Andrea Braides, Margherita Solci, Enrico Vitali . A derivation of linear elastic energies from pair-interaction atomistic systems. Networks and Heterogeneous Media, 2007, 2(3): 551-567. doi: 10.3934/nhm.2007.2.551 |
| [6] | Claudio Canuto, Anna Cattani . The derivation of continuum limits of neuronal networks with gap-junction couplings. Networks and Heterogeneous Media, 2014, 9(1): 111-133. doi: 10.3934/nhm.2014.9.111 |
| [7] | Bernd Schmidt . On the derivation of linear elasticity from atomistic models. Networks and Heterogeneous Media, 2009, 4(4): 789-812. doi: 10.3934/nhm.2009.4.789 |
| [8] | Michele Gianfelice, Marco Isopi . On the location of the 1-particle branch of the spectrum of the disordered stochastic Ising model. Networks and Heterogeneous Media, 2011, 6(1): 127-144. doi: 10.3934/nhm.2011.6.127 |
| [9] | Manuel Friedrich, Bernd Schmidt . On a discrete-to-continuum convergence result for a two dimensional brittle material in the small displacement regime. Networks and Heterogeneous Media, 2015, 10(2): 321-342. doi: 10.3934/nhm.2015.10.321 |
| [10] | Victor A. Eremeyev . Anti-plane interfacial waves in a square lattice. Networks and Heterogeneous Media, 2025, 20(1): 52-64. doi: 10.3934/nhm.2025004 |
The purpose of this paper is to investigate the oscillatory behaviour of a class of first-order sublinear and superlinear neutral difference equations. Some conditions are established by applying Banach's Contraction mapping principle, Knaster-Tarski fixed point theorem and using several inequalities. We provide some examples to illustrate the outreach of the main results.
In this paper we study an atomistic model for (possibly heterogeneous) nanowires. We consider a scaling of the energy that corresponds to a reduction of the system from
Specifically, in the homogeneous case, we study the asymptotic behaviour of the energy defined by
| εε(u):=∑i,j∈ZN|i−j|≤R(|u(εi)−u(εj)|ε−|i−j|)p, | (0.1) |
where
This model was first studied in [14,15] under the assumption that the admissible deformations satisfy the non-interpenetration condition, namely, that the Jacobian determinant of a suitably defined piecewise affine interpolation of
For the scaling of (0.1), we obtain a complete description of the
The
The paper is organised as follows. In Section 1 we introduce the model. In Section 1 we introduce the minimal costs to bridge different equilibria and study their dependence on the thickness of the nanowire. In Sections 3-5, performing a discrete to continuum limit and a dimension reduction simultaneously, we characterise the
Notation. We recall some basic notions of geometric measure theory for which we refer to [3]. Given a bounded open set
For
Finally,
In the paper, the same letter
We study the dimension reduction of a discrete model for heterogeneous nanowires. Let
| Lε(k):=εZN∩¯Ωkε, | (1.1) |
where
The bonds between the atoms are defined by means of the so-called Kuhn decomposition, which is relevant for modelling some specific Bravais lattices. (See [2,Remark 2.6] for details on the treatment of some lattices in dimension two and three, such as the hexagonal or equilateral triangular, the face-centred cubic, and the body-centred cubic.) First we define a partition
| {{0,ei1,ei1+ei2,…,ei1+ei2+⋯+eiN}:(12⋯Ni1i2⋯iN)∈SN}, |
where
| B1:={ξ∈RN:x and x+ξ are contiguous}. | (1.2) |
If both simplices
| B2:={ξ∈RN:x and x+ξ are opposite vertices}, | (1.3) |
and remark that, by periodicity,
We assume that
| L−ε(k):={x∈Lε(k):x1<0},L+ε(k):={x∈Lε(k):x1≥0}, |
respectively, where
| ε1,λε(u,k):=∑x∈L−ε(k)ξ∈B1∪B2x+εξ∈Lε(k)c(ξ)||u(x+εξ)−u(x)|ε−|Hξ||p+∑x∈L+ε(k)ξ∈B1∪B2x+εξ∈Lε(k)c(ξ)||u(x+εξ)−u(x)|ε−λ|Hξ||p, | (1.4) |
where
To simplify the presentation, we restrict our attention to the case of
| ∑x∈L−ε(k)ξ∈B1∪B2x+εξ∈Lε(k)ϕ1(ξ,|u(x+εξ)−u(x)|e−|Hξ|)+∑x∈L+ε(k)ξ∈B1∪B2x+εξ∈Lε(k)ϕλ(ξ,|u(x+εξ)−u(x)|e−λ|Hξ|), |
where
| C1|z|p≤ϕμ(ξ,z)≤C2|z|pfor μ=λ,1, |
for some positive constants
In principle, all the results that we present in the sequel extend to the case when the two components of the nanowire have equilibria of the form
We study the limit behaviour of
The main novelty of the present paper is that we remove the non-interpenetration assumption made in [14,15], allowing for changes of orientations. Furthermore, in the study of the
In the sequel of the paper we will often consider the rescaled domain
| Ωk,∞:=R×(−k,k)N−1. |
We define the associated lattice and subsets
| L∞(k):=ZN∩¯Ωk,∞,L−∞(k):={x∈L∞(k):x1<0},L+∞(k):={x∈L∞(k):x1≥0}, |
where
| E1,λ∞(u,k):=∑x∈L−∞(k)ξ∈B1∪B2x+ξ∈L∞(k)c(ξ)||u(x+ξ)−u(x)|−|Hξ||p+∑x∈L+∞(k)ξ∈B1∪B2x+ξ∈L∞(k)c(ξ)||u(x+ξ)−u(x)|−λ|Hξ||p. | (1.5) |
We identify every deformation
| Aε(Ωkε):={u∈C0(¯Ωkε;RN):u piecewise affine, ∇u constant on Ωkε∩εT ∀T∈T}. |
Similarly, for (1.5) we define
| A∞(Ωk,∞):={u∈C0(¯Ωk,∞;RN):u piecewise affine, ∇u constant on Ωk,∞∩T ∀T∈T}. |
As customary in dimension reduction problems, we rescale the domain
| Aε:=diag(1,ε,…,ε); | (1.6) |
i.e.,
| I1,λε(˜u,k):=E1,λε(u,k)for ˜u∈˜Aε(Ωk), | (1.7) |
with
| ˜Aε(Ωk):={˜u∈C0(A−1ε(¯Ωkε);RN):˜u piecewise affine, ∇˜u constant on Ωk∩(A−1εεT) ∀T∈T}. |
For later use it will be convenient to set the following notation:
| Ω−k:=(−L,0)×(−k,k)N−1,Ω+k:=(0,L)×(−k,k)N−1. |
We recall that, throughout the paper,
We will study the
| γ(P1,P2;k):=inf{E1,λ∞(v,k):M>0, v∈A∞(Ωk,∞),∇v=P1H for x1∈(−∞,−M),∇v=P2H for x1∈(M,+∞)}; | (2.1a) |
for
| γ(P1,P2;k):=inf{E1,1∞(v,k):M>0, v∈A∞(Ωk,∞),∇v=P1H for x1∈(−∞,−M),∇v=P2H for x1∈(M,+∞)}, | (2.1b) |
where
| E1,1∞(v,k):=∑x∈L∞(k)ξ∈B1∪B2x+ξ∈L∞(k)c(ξ)||v(x+ξ)−v(x)|−|Hξ||p; |
for
| γ(P1,P2;k):=inf{Eλ,λ∞(v,k):M>0,v∈A∞(Ωk,∞),∇v=P1Hforx1∈(−∞,−M),∇v=P2Hforx1∈(M,+∞)}, | (2.1c) |
where
| Eλ,λ∞(v,k):=∑x∈L∞(k)ξ∈B1∪B2x+ξ∈L∞(k)c(ξ)||v(x+ξ)−v(x)|−λ|Hξ||p. |
The next proposition shows that the relevant quantities defined through (2.1) are in fact four: the minimal costs of the transition at the interface between the energy wells
Proposition 1. For each
| γ(R,R′;k)=γ(Q,Q′;k)=γ(λR,λR′;k)=γ(λQ,λQ′;k)=0, | (2.2a) |
| γ(R,λR′;k)=γ(Q,λQ′;k)=γ(I,λI;k), | (2.2b) |
| γ(R,λQ;k)=γ(Q,λR;k)=γ(I,λJ;k), | (2.2c) |
| γ(R,Q;k)=γ(Q,R;k)=γ(I,J;k),and | (2.2d) |
| γ(λR,λQ;k)=γ(λQ,λR;k)=γ(λI,λJ;k). | (2.2e) |
Moreover,
| γ(λP1,λP2;k)=λpγ(P1,P2;k)forevery P1,P2∈O(N). | (2.3) |
Proof. First one notices that
We now prove estimates on the asymptotic behaviour of
Theorem 2.1.[2,Theorem 3.1] Let
| εN−1∑ξ∈B1∪B2∑x,x+εξ∈εZN∩(0,1)N||uε(x+εξ)−uε(x)|ε−|Hξ||p<C. | (2.4) |
Then there are a subsequence (not relabelled) and a function
| ∇u∈SBV((0,1)N;O(N)H). | (2.5) |
Specifically,
| u(x)=∑i∈N(RiHx+bi)χEi(x), | (2.6) |
where
We now prove the main result of this section.
Theorem 2.2. Let
| γ(P1,P2;k)≤CkN. | (2.7) |
Moreover,
| limk→∞γ(P1,P2;k)kN−1=+∞. | (2.8) |
Proof. The upper bound (2.7) is proven by comparing test functions for
For the proof of the lower bound (2.8) we will use Theorem 2.1 in each of the subsets
| 1kN−1jE1,λ∞(uj,kj)<C, | (2.9) |
for some positive
| Lj:=1kjZN∩¯Ω1,∞,L+j:=Lj∩{x1>0},L−j:=Lj∩{x1<0}. |
Expressing
| E1,λ∞(uj,k)=∑x∈L−jξ/kj∈B1∪B2x+ξ/kj∈Ljc(ξ)||vj(x+ξkj)−vj(x)|1kj−|Hξ||p+∑x∈L+jξ/kj∈B1∪B2x+ξ/kj∈Ljc(ξ)||vj(x+ξkj)−vj(x)|1kj−λ|Hξ||p. | (2.10) |
The above term controls the (piecewise constant) gradient of
| v(x)=∑i∈N(RiHx+ai)χEi(x)+∑j∈N(λQjHx+bj)χE+j(x), |
where
Remark 1. An estimate similar to (2.8) was proven in [14,15] (for a hexagonal lattice in dimension two and a class of three-dimensional lattices) via a different argument, based on the non-interpenetration condition. In fact, in [14,15] a stronger result is proven, namely, that
The non-interpenetration assumption turns out to be necessary if the energy involves only nearest neighbour interactions; indeed, in such a case, one can exhibit deformations that violate the non-interpenetration condition and for which (2.8) does not hold, see [14,Section 4.2]. Such deformations, which consist of suitable foldings of the lattice, would be energetically expensive (and, in particular, would not provide a counterexample to (2.8)) in the present setting, exactly because of the effect of the interactions across neighbouring cells. It is the latter ones that prevent folding phenomena and allow one to prove (2.8), via Theorem 2.1.
Before characterising the
Essential tools for the compactness and the lower bound are provided by the following rigidity estimates.
Theorem 3.1. [12,Theorem 3.1] Let
| ‖∇u−R‖Lp(U;MN×N)≤C(U)‖dist(∇u,SO(N))‖Lp(U). | (3.1) |
The constant
It is convenient to define the energy of a single simplex
| Ecell(uF;T):=N∑i≤j=0||F(xi−xj)|−|H(xi−xj)||pfor every F∈MN×N, |
where
Lemma 3.2. [2,Lemma 2.2] There exists a constant
| distp(F,SO(N)H)≤CEcell(uF;T)∀F∈MN×N:detF≥0, | (3.2a) |
| distp(F,(O(N)∖SO(N))H)≤CEcell(uF;T)∀F∈MN×N:detF≤0. | (3.2b) |
The next lemma asserts that if in two neighbouring simplices the sign of
| Ecell(u;S∪T):=N∑i≤j=0||u(xi)−u(xj)|−|H(xi−xj)||p |
| +N∑j=1||u(y0)−u(xj)|−|H(y0−xj)||p+||u(y0)−u(x0)|−|H(y0−x0)||p. |
Lemma 3.3. [2,Lemma 2.3] There exists a positive constant
| det(∇u|S)det(∇u|T)≤0, |
then
Lemma 3.2 will allow us to apply Theorem 3.1. More precisely, in the part of the wire with
Due to the fact that a minimum energy has to be paid for each change of orientation, see Lemma 3.3, the parts with positive determinant do not mix with those with negative determinant. Hence, passing to the weak* limit we obtain functions taking values in
Remark 2. It is well known that
| co(SO(2))={(α−ββα):α2+β2≤1},co(O(2)∖SO(2))={(αββ−α):α2+β2≤1}. |
In particular,
| co(SO(N))∪co(O(N)∖SO(N))⊊ |
for
Henceforth, the symbol
Proposition 2. Let
| \label{succ-limitata} \limsup\limits_{\varepsilon\to 0^{+}} \mathcal{I}_{\varepsilon }^{1, \lambda }({\tilde{u}}_\varepsilon, k) \leq C \, . | (3.3) |
Then there exist functions
| (3.4) |
and
| \label{charact} (\partial_1 {\tilde{u}} \, | \, d_2 \, | \cdots \, | d_N)\in \begin{cases} {\rm{co}}(SO(N))H &\;\;\;\;\; a.e.\;\;in\ (-L, 0)\cap U \, , \\ {\rm{co}}(O(N) {\setminus} SO(N))H &\;\;\;\;\; a.e.\;\;in\ (-L, 0)\setminus U\, , \\ \lambda\, {\rm{co}}(SO(N))H & \;\;\;\;\;a.e.\;\;in\ (0, L) \cap U \, , \\ \lambda\, {\rm{co}}(O(N) {\setminus} SO(N))H & \;\;\;\;\;a.e.\;\;in\ (0, L)\setminus U \, , \end{cases} | (3.5) |
and
| \label{gammaliminf} \begin{split} \liminf\limits_{\varepsilon\to 0^{+}} \mathcal{I}_{\varepsilon }^{1, \lambda }(\tilde u_\varepsilon, k)\geq&\ \gamma(I, J;k) \, \mathcal{H}^0(\partial U\cap(-L, 0)) + \gamma(\lambda I, \lambda J;k) \, \mathcal{H}^0(\partial U\cap(0, L)) \\ & + \gamma(I, \lambda I;k) \left[1-\chi_{\partial U}(0) \right] + \gamma(I, \lambda J;k)\, \chi_{\partial U}(0) \, . \end{split} | (3.6) |
Remark 3. The right-hand side of (3.6) contains different contributions. The first term corresponds to the minimal energy needed to bridge a rotation with a rotoreflection, or viceversa, in the left part of the nanowire; the energy spent depends on the number of changes of orientation, i.e., on the cardinality of
Proof. (Compactness) The assumption (3.3) implies that
In order to show
Specifically, for each
| \int_{({a_i},{a_i} + \varepsilon ) \times {{(k\varepsilon ,k\varepsilon )}^{N - 1}}} |\nabla {u_\varepsilon } - {P_\varepsilon }({a_i}){|^p}{\mkern 1mu} {\rm{d}}x \le C\int_{({a_i},{a_i} + \varepsilon ) \times {{(k\varepsilon ,k\varepsilon )}^{N - 1}}} \;{\rm{dis}}{{\rm{t}}^p}(\nabla {u_\varepsilon },O(N)H){\mkern 1mu} {\rm{d}}x{\mkern 1mu} , |
and for every
| \int_{({a_i},{a_i} + \varepsilon ) \times {{(k\varepsilon ,k\varepsilon )}^{N - 1}}} |\nabla {u_\varepsilon } - {P_\varepsilon }({a_i}){|^p}{\mkern 1mu} {\rm{d}}x \le C\int_{({a_i},{a_i} + \varepsilon ) \times {{(k\varepsilon ,k\varepsilon )}^{N - 1}}} \;\;{\rm{dis}}{{\rm{t}}^p}(\nabla {u_\varepsilon },\lambda {\mkern 1mu} O(N)H){\mkern 1mu} {\rm{d}}x{\mkern 1mu} . |
Moreover for
| \begin{align} &\int_{\Omega _k^-} |\nabla {\tilde{u}}_\varepsilon A_\varepsilon^{-1} - P_\varepsilon(x_1)|^{p} \, \text{d}x \leq C\!\! \int_{A_\varepsilon^{-1}(\overline{\Omega }_{k\varepsilon}) \cap\{x_1 < 0\}} \!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\! \text{dist}^{p}(\nabla {\tilde{u}}_\varepsilon A_\varepsilon^{-1}, O(N)H ) \, \text{d}x \leq C\varepsilon\, , \end{align} | (3.7a) |
| \begin{align}&\int_{\Omega _k^+} |\nabla {\tilde{u}}_\varepsilon A_\varepsilon^{-1} - P_\varepsilon(x_1)|^{p} \, \text{d}x \leq C\!\! \int_{A_\varepsilon^{-1}(\overline{\Omega }_{k\varepsilon}) \cap\{x_1 > 0\}} \!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\! \text{dist}^{p}(\nabla {\tilde{u}}_\varepsilon A_\varepsilon^{-1}, \lambda\, O(N) H ) \, \text{d}x \leq C\varepsilon\, , \end{align} | (3.7b) |
where the last inequality of each line follows by applying Lemma 3.2 to each subdomain with
We now define the sets
| \begin{align*} K_\varepsilon &: = \{ a_i^\varepsilon \in (-L, L) \colon P_\varepsilon(x_1) \in SO(N)H \cup \lambda\, SO(N) H \;\;\text{ for }\;\; x_1\in [a_i^\varepsilon, a_i^\varepsilon + \varepsilon)\} \, , \\ U_\varepsilon &: = \bigcup\limits_{a_i^\varepsilon\in K_\varepsilon} [a_i^\varepsilon, a_i^\varepsilon + \varepsilon)\, , \end{align*} |
and remark that Lemma 3.2, Lemma 3.3, and assumption (3.3) imply that the cardinality of
| \label{U} U = \bigcup\limits_{i = 1}^n (\alpha_i, \beta_i) \, , \;\;\;\;\;-L\le\alpha_1 < \beta_1 < \alpha_2 < \beta_2 < \dots < \alpha_n < \beta_n\le L \, . | (3.8) |
Since we can write
| \begin{split} P_\varepsilon(x_1) = & R_\varepsilon(x_1)\big(\chi_{U_\varepsilon\cap (-L, 0)} H + \chi_{U_\varepsilon\cap (0, L)}\lambda H \big) \\ &+ JR_\varepsilon(x_1)\big((1-\chi_{U_\varepsilon\cap (-L, 0)}) H + (1-\chi_{U_\varepsilon\cap (0, L)})\lambda H \big)\, , \end{split} |
where
(Lower bound) Inequality (3.6) is proven by a standard argument which can be found, for example, in [14,16,17]. We will briefly sketch the main ideas and refer the reader to [14,16,17] for full details. First recall that
| \begin{align}&(\alpha_i^\varepsilon -2\sigma, \alpha_i^\varepsilon - \sigma )\subset (-L, L)\setminus U_\varepsilon \, , \;\;\;\;\;(\alpha_i^\varepsilon +\sigma, \alpha_i^\varepsilon +2 \sigma ) \subset U_\varepsilon \, , \end{align} | (3.9a) |
| (\beta_i^\varepsilon -2\sigma, \beta_i^\varepsilon - \sigma ) \subset U_\varepsilon \, ,\;\;\;\;\; (\beta_i^\varepsilon +\sigma, \beta_i^\varepsilon +2 \sigma ) \subset (-L, L)\setminus U_\varepsilon \, . | (3.9b) |
Moreover, if
| v_\varepsilon(x_1, x_2, \dots, x_N) : = \tfrac{1}\varepsilon{\tilde{u}}_\varepsilon(\varepsilon x_1 + \alpha_i^\varepsilon, x_2, \dots, x_N) = \tfrac{1}\varepsilon u_\varepsilon(\varepsilon x_1 + \alpha_i^\varepsilon, \varepsilon x_2, \dots, \varepsilon x_N). |
Then,
| \label{ci-siamo-quasi} \begin{split}&\int_{(-\frac{2\sigma}\varepsilon, -\frac{\sigma}\varepsilon)\times (-k, k)^{N-1} } \text{dist}^{p}(\nabla v_\varepsilon, \lambda(O(N){\setminus} SO(N)) H ) \, \text{d}x \\&+ \int_{(\frac{\sigma}\varepsilon, \frac{2\sigma}\varepsilon)\times (-k, k)^{N-1}} \text{dist}^{p}((\nabla v_\varepsilon, \lambda\, SO(N)H) \, \text{d}x \leq C \, . \end{split} | (3.10) |
From (3.10), Theorem 3.1 and the Poincaré inequality, we deduce that there exists a unit interval contained in
| \mathcal{I}_\varepsilon ^{1,\lambda }({\tilde u_\varepsilon },k){|_{(\alpha _i^\varepsilon - 2\sigma ,\alpha _i^\varepsilon + 2\sigma ) \times {{( - k,k)}^{N - 1}}}} \ge \mathcal{E} _\infty ^{\lambda ,\lambda }({\hat v_\varepsilon },k) - C\frac{\varepsilon }{\sigma }{\mkern 1mu} , |
where
We prove that the bound (3.6) is in fact optimal.
Proposition 3. Let
| \label{cohull} F\in \begin{cases} {\rm{co}}(SO(N))H\;\;\;\;\; a.e.{\rm{ }}\;in\ (-L, 0)\cap U \, , \\ {\rm{co}}(O(N){\setminus} SO(N))H\;\;\;\;\; a.e.{\rm{ }}\;in\ (-L, 0)\setminus U \, , \\ \lambda\, {\rm{co}}(SO(N))H\;\;\;\;\; a.e.{\rm{ }}\;in\ (0, L)\cap U \, , \\ \lambda\, {\rm{co}}(O(N){\setminus} SO(N))H\;\;\;\;\; a.e.{\rm{ }}\;in\ (0, L)\setminus U \, . \end{cases} | (4.1) |
Then there exists a sequence
| \label{1328061} {\tilde{u}}_\varepsilon A_\varepsilon^{-1}\mathop * \limits_ \rightharpoonup F \;\;\;\;\; weakly*{\rm{ }}in\;\;\;\;\;\ L^{\infty}(\Omega _k;{{\mathbb{M}}^{N\times N}})\, , | (4.2) |
and
| \limsup\limits_{\varepsilon\to 0^{+}} \mathcal{I}_{\varepsilon }^{1, \lambda }(\tilde u_\varepsilon, k)\leq\ \gamma(I, J;k) \, \mathcal{H}^0(\partial U\cap(-L, 0)) + \gamma(\lambda I, \lambda J;k) \, \mathcal{H}^0(\partial U\cap(0, L)) \\+ \gamma(I, \lambda I;k) \left[1-\chi_{\partial U}(0) \right] + \gamma(I, \lambda J;k)\, \chi_{\partial U}(0) | (4.3) |
Proof. Using a standard approximation argument we may assume that
| F = \sum\limits_{i = m}^{-1}\chi_{(a_i, a_{i+1})}R_{i}H+ \sum\limits_{i = 0}^{n}\chi_{(a_i, a_{i+1})}\lambda R_{i}H |
and
| U = {\mathop{\rm int}} \bigcup \big\{ [a_i, a_{i+1}] \colon R_i\in SO(N) \, , \ m\le i\le n-1 \big\} \, . |
The following construction is similar to that in [14,Proposition 3.2], so we will show the details only for what concerns the changes of orientation. We introduce a mesoscale
We now complete the definition of
If
| \nabla v = R_{i-1}H \ \text{for} \ x_1\in(-\infty, -M)\, , \,\;\;\;\;\; \nabla v = R_{i}H \ \text{for} \ x_1\in(M, +\infty) |
and
| \mathcal{E} _{\infty }^{1, 1}(v, k) \leq \gamma (I, J;k) + \eta \, , |
where we used also Proposition 1. With this at hand, we define
| \tilde u_\varepsilon(x): = \varepsilon v(\tfrac{1}{\varepsilon}A_\varepsilon x) + b \, . |
The constant vector
The case
In the next theorem we characterise the
| \label{gammadomain} \begin{split} \mathcal{A}^{1,\lambda }(k): = \big\{ u\in W^{1, \infty}(\Omega _k;\mathbb{R}^N)\colon & \partial_{2} u = \dots = \partial_{N} u = 0 \ \text{a.e. in}\ \Omega _k\, , \\ & |\partial_1 u |\leq 1 \ \text{a.e. in}\ \Omega _k^- \, , \ |\partial_1 u |\leq \lambda \ \text{a.e. in}\ \Omega _k^+ \big\} \, . \end{split} | (5.1) |
We show that on such domain the
Theorem 5.1. The sequence of functionals
| \mathcal{I}^{1,\lambda }(u, k) = \begin{cases} \gamma (k) & \;\;\;\;\;if\ u\in\mathcal{A}^{1,\lambda }(k)\, , \\ +\infty &\;\;\;\;\; otherwise, \end{cases} | (5.2) |
with respect to the weak* convergence in
| \label{gammamin} \gamma(k): = \min\big\{ \gamma(I, \lambda I;k), \gamma(I, \lambda J;k) \big\} \, . | (5.3) |
Proof. (Liminf inequality) Let
| \mathcal{I}^{1,\lambda }(u, k)\leq \liminf\limits_{\varepsilon \to 0^+} \mathcal{I}_{\varepsilon }^{1,\lambda }({\tilde{u}}_\varepsilon, k) \, . |
We assume that
(Limsup inequality) Given a function
| \limsup\limits_{\varepsilon\to 0^+} \mathcal{I}_{\varepsilon }^{1,\lambda } ({\tilde{u}}_\varepsilon, k)\leq \mathcal{I}^{1,\lambda }(u, k) \, . | (5.4) |
We assume that
The construction of the recovery sequence depends on the precise value of the minimum in (5.3). Since we do not know such value, we explain how to proceed in the case when
| (\partial_1 u \, | \, d_2 \, | \, \cdots \, | \, d_N)\in \begin{cases} {\rm{co}}(SO(N))H & \text{a.e. in}\ \Omega _k^-\, , \\ \lambda\, {\rm{co}}(SO(N))H & \text{a.e. in}\ \Omega _k^+\, . \end{cases} |
| (\partial_1 u \, | \, d_2 \, | \, \cdots \, | \, d_N)\in \begin{cases} {\rm{co}}(SO(N))H & \text{a.e. in}\ \Omega _k^-\, , \\ \lambda\, {\rm{co}}(O(N){\setminus}SO(N))H & \text{a.e. in}\ \Omega _k^+\, . \end{cases} |
Proposition 3 can be now applied to
Remark 4. As long as the
Below we show that, if a stronger topology is chosen, the value of the
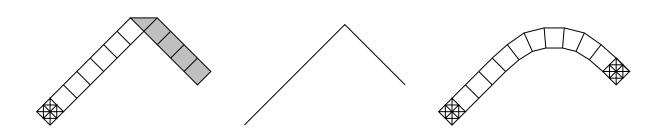
Two possible recovery sequences for the profile at the centre of the figure. Here we picture only a part of the wire containing just one species of atoms, therefore the transition at the interface is not represented. A kink in the profile may be reconstructed by folding the strip, i.e., mixing rotations and rotoreflections (left); or by a gradual transition involving only rotations or only rotoreflections (right). In the limit, the former recovery sequence gives a positive cost, while the latter gives no contribution. If the stronger topology is chosen, the appropriate recovery sequence will depend on the value of the internal variable, which defines the orientation of the wire
.We introduce the sequence of functionals defined for
| \widehat {\cal I}_\varepsilon ^{1,\lambda }(\tilde u,D,k): = \left\{ {\begin{array}{*{20}{l}} {{\cal I}_\varepsilon ^{1,\lambda }(\tilde u,k)\;\;\;\;\;{\rm{if}}\;\tilde u \in {{\widetilde {\cal A}}_\varepsilon }({\Omega _k})\;{\rm{and}}\;D = ({\partial _1}\tilde u{\mkern 1mu} |{\mkern 1mu} {\varepsilon ^{ - 1}}{\partial _2}\tilde u{\mkern 1mu} |{\mkern 1mu} \cdots {\mkern 1mu} |{\mkern 1mu} {\varepsilon ^{ - 1}}{\partial _N}\tilde u){\mkern 1mu} ,}\\ { + \infty \;\;\;\;\;{\rm{otherwise}}{\rm{.}}} \end{array}} \right. |
In the next theorem we study the
| \begin{align} & {{\widehat{\mathcal{A}}}^{1,\lambda }}(k):=\{(u,D):u\in {{\mathcal{A}}^{1,\lambda }}(k),\ D\in {{L}^{\infty }}({{\Omega }_{k}};{{\mathbb{M}}^{N\times N}}),\ \ \\ & \ \ \ D{{e}_{1}}={{\partial }_{1}}u,\ {{\partial }_{2}}D=\ldots ={{\partial }_{N}}D=0\ \text{a}\text{.e}\text{. in}\ {{\Omega }_{k}},\ \ \ \ \\ & \ D\in \text{co}(SO(N))H\cup \text{co}(O(N)\setminus SO(N))H\ \text{a}\text{.e}\text{. in}\ \Omega _{k}^{-}, \\ & D\in \lambda \text{co}(SO(N))H\cup \lambda \text{co}(O(N)\setminus SO(N))H\ \text{a}\text{.e}\text{. in}\ \Omega _{k}^{+}\}, \\ \end{align} |
where
Definition 5.2. Given
| \label{coso} D \in \begin{cases} {\rm{co}}(SO(N))H& \text{for a.e.}\ x_1\in(-L, 0)\cap U \, , \\ {\rm{co}}(O(N){\setminus} SO(N))H& \text{for a.e.}\ x_1\in(-L, 0)\setminus U \, , \\ \lambda\, {\rm{co}}(SO(N))H& \text{for a.e.}\ x_1\in(0, L)\cap U \, , \\ \lambda\, {\rm{co}}(O(N){\setminus} SO(N))H& \text{for a.e.}\ x_1\in(0, L)\setminus U \, . \end{cases} | (5.5) |
For
| \begin{split} \mathcal{J}(U) : = &\ \gamma(I, J;k) \, \mathcal{H}^0(\partial U\cap(-L, 0)) + \gamma(\lambda I, \lambda J;k) \, \mathcal{H}^0(\partial U\cap(0, L)) \\ & + \gamma(I, \lambda I;k) \left[1-\chi_{\partial U}(0) \right] + \gamma(I, \lambda J;k)\, \chi_{\partial U}(0) \end{split} |
and
| {\mathcal{J}_{\min }}(u, D) : = \min\limits_{U\in \mathcal{U}(u, D)} \mathcal{J}(U) \, . | (5.6) |
The last definition will be used to apply Propositions 2 and 3 towards the characterisation of the
| \begin{split} &\{ \mathcal{J}(U) \colon U \in \mathcal{U}(u, D) \} \\ \subset& \{ m_1 \gamma(I, J;k) + m_2 \gamma(I, \lambda I;k)+ m_3 \gamma(I, \lambda J;k)+ m_4 \gamma(\lambda I, \lambda J;k) \colon m_i\in \mathbb{N} \} \, . \end{split} |
A minimiser needs not be unique as shown in the following example.
Example 5.3. Fix
Theorem 5.4. The sequence of functionals
| {{\widehat{\mathcal{I}}}^{1,\lambda }}(u,D,k):=\left\{ \begin{array}{*{35}{l}} {{\mathcal{J}}_{\min }}(u,D) & if\ (u,D)\in {{\widehat{\mathcal{A}}}^{1,\lambda }}(k), \\ +\infty & otherwise\text{, } \\ \end{array} \right. | (5.7) |
with respect to the weak* convergence in
Proof. The liminf inequality is obtained by applying Proposition 2 and arguing as in Theorem 5.1. Also the derivation of the limsup inequality is similar to the one performed in Theorem 5.1; let us simply point out that, while in the proof of Theorem 5.1 the matrix field
Remark 5. We underline that Theorem 5.4 provides a nontrivial
In the present section we discuss how the previous results extend to the case when the functional (1.4) is complemented by boundary conditions or external forces. Although our considerations apply to the case of general
Boundary conditions. Let
| \begin{cases} \nabla u(x) = B^-x&\text{ if }-L < x_1 < -L +\varepsilon\, , \\ \nabla u(x) = B^+x&\text{ if }L -\varepsilon < x_1 < L \, . \end{cases} | (6.1) |
It is easy to see that while the compactness result of Proposition 2 remains valid, the
| \begin{split} \gamma(B^-, P;k): = \inf\big\{ \mathcal{E} _M^{1,1}(v, k) \colon&M > 0\, , \ v\in \mathcal{A}_{\infty}(\Omega _{k, \infty})\, , \\ & \nabla v = B^- \ \text{for} \ x_1\in(-\infty, -M)\, , \, \\& \nabla v = P \ \text{for} \ x_1\in(M, +\infty) \big\}\, , \end{split} | (6.2) |
| \begin{split} \gamma(P, B^+;k): = \inf\big\{ \mathcal{E} _M^{1,1}(v, k) \colon&M > 0\, , \ v\in \mathcal{A}_{\infty}(\Omega _{k, \infty})\, , \\ & \nabla v = P \ \text{for} \ x_1\in(-\infty, -M)\, , \, \\& \nabla v = B^+ \ \text{for} \ x_1\in(M, +\infty) \big\}\, , \end{split} | (6.3) |
where
Remark 6. By Proposition 2 and the properties of
| \gamma(I, B^{\pm};k)\to 0 \;\;\;\;\; \text{as} \;\;\;\;\; \text{dist}\;\;\;\;\;(B^{\pm};SO(N))\to 0 |
and therefore, as long as
External forces. We study a class of tangential/radial forces acting along the rod. Let
| \begin{align} & {{\mathcal{F}}_{\varepsilon }}(u,k):= \\ & \sum\limits_{(\pm {{L}_{\varepsilon }},{{x}_{2}},\ldots ,{{x}_{N}})\in {{\mathcal{L}}_{\varepsilon }}(k)}{}{{F}_{1}}\cdot (u({{L}_{\varepsilon }},{{x}_{2}},\ldots ,{{x}_{N}})-u(-{{L}_{\varepsilon }},{{x}_{2}},\ldots ,{{x}_{N}})) \\ & +\sum\limits_{({{x}_{1}},\pm \varepsilon k,\ldots ,{{x}_{N}})\in {{\mathcal{L}}_{\varepsilon }}(k)}{}{{F}_{2}}({{x}_{1}})\cdot (u({{x}_{1}},\varepsilon k,{{x}_{3}},\ldots ,{{x}_{N}})-u({{x}_{1}},-\varepsilon k,{{x}_{3}},\ldots ,{{x}_{N}}))+\cdots \\ & \ldots +\sum\limits_{({{x}_{1}},\ldots ,{{x}_{N-1}},\pm \varepsilon k)\in {{\mathcal{L}}_{\varepsilon }}(k)}{}{{F}_{N}}({{x}_{1}})\cdot (u({{x}_{1}},\ldots ,{{x}_{N-1}},\varepsilon k)-u({{x}_{1}},\ldots ,{{x}_{N-1}},-\varepsilon k)), \\ \end{align} | (6.4) |
where
| \begin{split} \mathcal{F}_\varepsilon(u, k) = \\& \sum\limits_{x_1 = -L_\varepsilon}^{L_\varepsilon-\varepsilon} \ \sum\limits_{(x_1, \dots, x_N)\in \mathcal{L}_\varepsilon(k)}\!\!\!\! \!\!\!\!\!\!\! F_1 {\cdot} \big( u(x_1+\varepsilon, \dots, x_N) - u(x_1, \dots, x_N) \big) \\ +& \sum\limits_{x_2 = -\varepsilon k}^{\varepsilon(k-1)} \ \sum\limits_{(x_1, \dots, x_N)\in \mathcal{L}_\varepsilon(k)}\!\!\!\!\!\!\!\!\!\!\! F_2(x_1) \cdot \big( u(x_1, x_2+\varepsilon, \dots, x_N) - u(x_1, x_2, \dots, x_N) \big)+ \cdots \\ \dots+& \sum\limits_{x_N = -\varepsilon k}^{\varepsilon(k-1)} \ \sum\limits_{(x_1, \dots, x_N)\in \mathcal{L}_\varepsilon(k)}\!\!\!\!\!\!\!\!\!\!\! F_N(x_1) \cdot \big( u(x_1, \dots, x_N+\varepsilon) - u(x_1, \dots, x_N) \big) \, , \end{split} |
hence we have that
Introducing the new variables
| \begin{split} &\ {\tilde{\mathcal{F}}}_\varepsilon({\tilde{u}}, k): = \\ &\!\!\!\!\!\!\! \sum\limits_{(\pm L_\varepsilon, x_2, \dots, x_N)\in A_\varepsilon^{-1}\mathcal{L}_\varepsilon(k)} \!\!\!\!\!\!\!\!\!\!\! F_1 {\cdot} \big( {\tilde{u}}(L_\varepsilon, x_2, \dots, x_N) - {\tilde{u}}(- L_\varepsilon, x_2, \dots, x_N) \big) \\ +& \!\!\!\!\!\!\! \sum\limits_{(x_1, \pm k, \dots, x_N)\in A_\varepsilon^{-1}\mathcal{L}_\varepsilon(k)} \!\!\!\!\!\!\!\!\!\!\! F_2(x_1) {\cdot} \big( {\tilde{u}}(x_1, k, x_3, \dots, x_N) - {\tilde{u}}(x_1, - k, x_3, \dots, x_N) \big)+ \dots \\ \dots+& \!\!\!\!\!\!\! \sum\limits_{(x_1, \dots, x_{N-1}, \pm k)\in A_\varepsilon^{-1}\mathcal{L}_\varepsilon(k)}\!\!\!\!\! \!\!\!\!\!\! F_N(x_1) {\cdot} \big( {\tilde{u}}(x_1, \dots, x_{N{-}1}, k) - {\tilde{u}}(x_1, \dots, x_{N-1}, - k) \big) \\ = &\ \mathcal{F}_\varepsilon(u, k)\, , \end{split} |
so that
| {{\mathcal{G}}_{\varepsilon }}(\tilde{u},D,k):=\widehat{\mathcal{I}}_{\varepsilon }^{1,1}(\tilde{u},D,k)-{{\widetilde{\mathcal{F}}}_{\varepsilon }}(\tilde{u},k),\ \ \ \ \tilde{u}\in {{\widetilde{\mathcal{A}}}_{\varepsilon }}({{\Omega }_{k}}),D\in {{L}^{\infty }}({{\Omega }_{k}};{{\mathbb{M}}^{N\times N}}). | (6.5) |
Note that in this context we cannot use the weak* convergence in
| \frac{C}\varepsilon \int_{\Omega _k} ( |\nabla {\tilde{u}}\, A_\varepsilon^{-1}|^p -1 ) \, \text{d}x \leq \frac{C}\varepsilon\int_{{\Omega }_{k}}\text{dist}^{p}(\nabla {\tilde{u}}\, A_\varepsilon^{-1}, O(N)) \, \text{d}x \leq \widehat{\mathcal{I}}_{\varepsilon }^{1,1}({\tilde{u}}, k) |
and
| {{\tilde{\mathcal{F}}}_{\varepsilon }}(\tilde{u},k)\le C(\int_{{{\Omega }_{k}}}{|}F{{|}^{{{p}'}}}\text{d}x+\int_{{{\Omega }_{k}}}{|}\nabla \tilde{u}A_{\varepsilon }^{-1}{{|}^{p}}\text{d}x). |
Let now
| \limsup\limits_{\varepsilon\to 0^+} \mathcal{G}_\varepsilon(\tilde u_\varepsilon, D_\varepsilon, k) \leq C\, . |
The previous inequalities imply that
Theorem 6.1. The following results hold:
(Compactness) Let
| \limsup\limits_{\varepsilon\to 0^+} \mathcal{G}_\varepsilon(\tilde u_\varepsilon, D_\varepsilon, k) \leq C\, . |
Then there exists
(
| \mathcal{G}(u,D,k):={{\widehat{\mathcal{I}}}^{1,1}}(u,D,k)-\tilde{\mathcal{F}}(D,k), | (6.6) |
with respect to the weak* convergence in
| \tilde{\mathcal{F}}(D,k):={{(2k)}^{N-1}}\int_{-L}^{L}{(}{{F}_{1}}\cdot {{d}_{1}}+\cdots +{{F}_{N}}\cdot {{d}_{N}})\text{d}{{x}_{1}} |
for
As a consequence of the previous theorem and the standard properties of
Corollary 1. We have that
| \begin{align} & \underset{\varepsilon \to 0}{\mathop{\lim }}\,\min \{{{\mathcal{G}}_{\varepsilon }}(u,D):(u,D)\in {{\widetilde{\mathcal{A}}}_{\varepsilon }}({{\Omega }_{k}})\times {{L}^{\infty }}({{\Omega }_{k}};{{\mathbb{M}}^{N\times N}})\} \\ & =\min \{\mathcal{G}(u,D,k):(u,D)\in {{\widehat{\mathcal{A}}}^{1,1}}(k)\}. \\ \end{align} |
Moreover if
| \lim\limits_{\varepsilon\to 0} \mathcal{G}_\varepsilon (u_\varepsilon, D_\varepsilon) = \lim\limits_{\varepsilon\to 0} \min\{\mathcal{G}_\varepsilon (u, D) \colon (u, D)\in {\tilde {\mathcal{A}}}_\varepsilon(\Omega _{k}) \times L^\infty(\Omega _k;{{\mathbb{M}}^{N\times N}}) \} \, , |
then any cluster point
We now come back to the question of the consistency of the model with the non-interpenetration condition. In this context we cannot expect that minimisers of (6.5) preserve orientation for the whole class of loads defined above. This is clarified in the following remark.
Remark 7. Minimisers of the functional defined by (6.6) may have transition points between the two wells
Define
| \begin{align} & C:=\underset{(u,D)\in \widehat{\mathcal{A}}_{0}^{1,1}(k)}{\mathop{\min }}\,-\tilde{F}(D,k) > -\tilde{F}(\bar{D},k) \\ & =-{{(2k)}^{N-1}}(\sum\limits_{i=1}^{N-1}{\int_{-L}^{L}{{{f}_{i}}}}\text{d}{{x}_{1}}+\int_{-L}^{a}{{{f}_{N}}}\text{d}{{x}_{1}}-\int_{a}^{L}{{{f}_{N}}}\text{d}{{x}_{1}}). \\ \end{align} |
Therefore, if
| -\widetilde F(\overline D, k) + \gamma(I, J;k) < C, |
then it is energetically preferred to have a transition at
The lattice mismatch in heterostructured materials, corresponding to
Following the ideas of [14], in dimension
| \mathcal{L}_\varepsilon(\rho, k): = \mathcal{L}_\varepsilon^-(1, k)\cup\mathcal{L}_\varepsilon^+(\rho, k) \, , |
where
| \begin{align*} \mathcal{L}_\varepsilon^-(1, k) &: = \phantom{\rho} \varepsilon \mathbb{Z}^2 \cap \overline\Omega _{k\varepsilon} \cap \{x_1 < 0\} \, , \\ \mathcal{L}_\varepsilon^+(\rho, k) &: = \rho \varepsilon \mathbb{Z}^2 \cap \overline\Omega _{k\varepsilon} \cap \{x_1\ge0\} \, , \end{align*} |
and
In presence of dislocations, the choice of the interactions and of the equilibria strongly depends on the lattice that one intends to model. Therefore, in this section we focus on the simplest situation of hexagonal (or equilateral triangular) Bravais lattices in dimension two and we fix
| H: = \begin{pmatrix} 1&-\tfrac12 \\ 0&\tfrac{\sqrt3}2 \end{pmatrix} \, . |
The lattice
The bonds between nearest and next-to-nearest neighbours are defined first in the lattice
Once the bonds in the lattice
| \begin{align*} B_1(x) &: = \{ \xi\in\mathbb{R}^N\colon Hx, \ H(x{+}\xi) \in H \mathcal{L}_\varepsilon(\rho, k) \ \text{are nearest neighbours} \} \, , \\ B_2(x) &: = \{ \xi\in\mathbb{R}^N\colon Hx, \ H(x{+}\xi) \in H \mathcal{L}_\varepsilon(\rho, k) \ \text{are next-to-nearest neighbours} \} \, . \end{align*} |
We remark that if
| \begin{split} &\mathcal{E} _{\varepsilon }^{1, \lambda }(u, \rho, k) : = \\ &\!\!\! \sum\limits_{\substack{ x\in \mathcal{L}^-_\varepsilon (\rho, k)\\ \xi\in B_1(x) }} \!\!\!\ c_1 \left|\frac{|u(x+\xi)-u(x)|}e-1\right|^p + \!\!\! \sum\limits_{\substack{ x\in \mathcal{L}^+_\varepsilon(\rho, k)\\ \xi\in B_1(x) }} \!\!\! c_1 \left|\frac{|u(x+ \xi)-u(x)|}e-\lambda \right|^p \\ +&\!\!\! \sum\limits_{\substack{ x\in \mathcal{L}^-_\varepsilon (\rho, k)\\ \xi\in B_2(x) }} \!\!\!\ c_2 \left|\frac{|u(x+\xi)-u(x)|}e-\sqrt3\right|^p + \!\!\! \sum\limits_{\substack{ x\in \mathcal{L}^+_\varepsilon(\rho, k)\\ \xi\in B_2(x) }} \!\!\! c_2 \left|\frac{|u(x+ \xi)-u(x)|}e-\sqrt3\lambda \right|^p \, . \end{split} |
Notice that away from the interface all bonds in the reference configuration are in equilibrium if
The results shown in detail in this paper for the defect-free case (corresponding to
| \begin{align*} \mathcal{L}_{\infty}(\rho, k)&: = \mathcal{L}_{\infty}^-(1, k)\cup\mathcal{L}_{\infty}^+(\rho, k) \, , \\ \mathcal{L}_{\infty}^-(1, k) &: = \phantom{\rho} \mathbb{Z}^2 \cap \overline\Omega _{k, \infty} \cap \{x_1 < 0\} \, , \\ \mathcal{L}_{\infty}^+(\rho, k) &: = \rho \mathbb{Z}^2 \cap \overline\Omega _{k, \infty} \cap \{x_1\ge0\} \, , \end{align*} |
where the triangulation is chosen in analogy with the one for
| \begin{split} \gamma(P_1, \lambda P_2;\rho, k): = \inf\big\{ &\mathcal{E} _{\infty }^{1, \lambda }(v, \rho, k) \colon M > 0\, , \ v\in \mathcal{A}_{\infty}(\Omega _{k, \infty})\, , \\ & \nabla v = P_1H \ \text{for} \ x_1\in(-\infty, -M)\, , \, \\&\nabla v = \tfrac\lambda\rho P_2 H \ \text{for} \ x_1\in(M, +\infty) \big\}\, , \end{split} |
with
| \begin{split} &\mathcal{E} _{\infty }^{1, \lambda }(u, \rho, k) : = \\ &\!\!\! \sum\limits_{\substack{ x\in \mathcal{L}^-_{\infty}(\rho, k)\\ \xi\in B_1(x) }} \!\!\!\ c_1 \Big||u(x+\xi)-u(x)|-1\Big|^p + \!\!\! \sum\limits_{\substack{ x\in \mathcal{L}^+_{\infty}(\rho, k)\\ \xi\in B_1(x) }} \!\!\! c_1 \Big||u(x+ \xi)-u(x)|-\lambda \Big|^p \\ +&\!\!\! \sum\limits_{\substack{ x\in \mathcal{L}^-_{\infty}(\rho, k)\\ \xi\in B_2(x) }} \!\!\!\ c_2 \Big||u(x+\xi)-u(x)|-\sqrt3\Big|^p + \!\!\! \sum\limits_{\substack{ x\in \mathcal{L}^+_{\infty}(\rho, k)\\ \xi\in B_2(x) }} \!\!\! c_2 \Big||u(x+ \xi)-u(x)|-\sqrt3\lambda \Big|^p \, . \end{split} |
Theorem 7.1. The sequence of functionals
| {{\mathcal{I}}^{1,\lambda }}(u,\rho ,k)=\left\{ \begin{array}{*{35}{l}} \gamma (\rho ,k) & if\ u\in {{\mathcal{A}}^{1,\lambda }}(\rho ,k), \\ +\infty & otherwise\text{, } \\ \end{array} \right. |
with respect to the weak* convergence in
| \begin{split} \mathcal{A}^{1,\lambda }(\rho, k): = \big\{ u\in W^{1, \infty}(\Omega _k;\mathbb{R}^N)\colon & \partial_{2} u = 0 \ a.e.\text{ }in\ \Omega _k\, , \\ & |\partial_1 u |\leq 1 \ a.e.\text{ }in\ \Omega _k^- \, , \ |\partial_1 u |\leq \tfrac\lambda\rho \ a.e.\text{ }in\ \Omega _k^+ \big\} \end{split} |
and
| \gamma(\rho, k): = \min\big\{ \gamma(I, \lambda I;\rho, k), \gamma(I, \lambda J;\rho, k) \big\} \, . |
The stronger topology introduced in Theorem 5.4 allows us to take into account the cost of "folding" the lattice using rotoreflections, giving deeper insight into deformations that bridge different equilibria. Indeed, it is possible to combine Theorems 5.4 and 7.1 giving the
Remark 8. It is easy to see that for
| C_1 k\le \gamma (\lambda, k)\le C_2 k |
for some constants
| \gamma (\rho, k)\le C_\rho \, k^2 \;\;\;\;\;\text{and} \;\;\;\;\;\lim\limits_{k\to\infty}\frac{\gamma (\rho, k)}{k} = +\infty \, . |
This gives a mathematical proof of the experimentally observed fact that dislocations are preferred in order to relieve the lattice mismatch when the thickness of the specimen is sufficiently large. We recall that a similar result was proven in [14,15] (under the non-interpenetration assumption), see also Remark 1.
The results sketched here for hexagonal lattices can be obtained also for other lattices by adapting the technique to each specific case. In particular, we refer to [15] for details on the rigidity of face-centred and body-centred cubic lattices in dimension three.
| [1] | R. P. Agarwal, Difference equations and inequalities: Theory, methods, and applications, Marcel Dekker, New York, 2000. |
| [2] | R. P. Agarwal, M. Bohner, S. R. Grace, D. O'Regan, Discrete oscillation theory, Hindawi Publishing Corporation, New York, 2005. |
| [3] | T. Candan, Existence of nonoscillatory solutions to first order neutral differential equations, Electon. J. Differ. Equ., 2016 (2016), 1–11. |
| [4] | G. E. Chatzarakis, G. N. Miliaras, Asymptotic behaviour in neutral difference equations with variable coefficients and more than one day arguments, J. Math. Comput. Sci., 1 (2012), 32–52. |
| [5] | L. H. Erbe, Q. Kong, B. G. Zhang, Oscillation theory for functional differential equations, Marcel Dekker Inc., New York, 1995. |
| [6] | Y. Gao, G. Zhang, Oscillation of nonlinear first order neutral difference equations, Appl. Math. E-Notes, 1 (2001), 5–10. |
| [7] |
D. A. Georgiou, E. A Grove, G. Ladas, Oscillations of neutral difference equations, Appl. Anal., 33 (1989), 243–253. https://doi.org/10.1080/00036818908839876 doi: 10.1080/00036818908839876

|
| [8] | G. Gomathi Jawahar, Oscillation properties of certain types of first order neutral delay difference equations, Int. J. Sci. Eng. Res., 4 (2013), 1197–1201. |
| [9] | I. Györi, G. Ladas, Oscillation theory of delay differential equations with applications, Clarendon Press, Oxford, 1991. |
| [10] | J. R. Graef, E. Thandapani, S. Elizabeth, Oscillation of first order nonlinear neutral difference equations, Indian J. Pure Appl. Math., 36 (2005), 503–512. |
| [11] | B. Karpuz, Sharp conditions for oscillation and nonoscillation of neutral difference equations, In: M. Bohner, S. Siegmund, R. Simon Hilscher, P. Stehlik, Difference equations and discrete dynamical systems with applications, ICDEA 2018, Springer Proceedings in Mathematics & Statistics, Vol. 312, Springer, Cham, 2020. https://doi.org/10.1007/978-3-030-35502-9_11 |
| [12] | V. Kolmanovski, A. Myshkis, Introduction to the theory and applications of functional differential equations, Kluwer, Dordrecht, 1999. |
| [13] | F. Kong, Existence of nonoscillatory solutions of a kind of first order neutral differential equation, Math. Commun., 22 (2017), 151–164. |
| [14] |
X. Lin, Oscillation of solutions of neutral difference equations with a nonlinear neutral term, Comput. Math. Appl., 52 (2006), 439–448. https://doi.org/10.1016/j.camwa.2006.02.009 doi: 10.1016/j.camwa.2006.02.009

|
| [15] |
Q. Li, C. Wang, F. Li, H. Liang, Z. Zhang, Oscillation of sublinear difference equations with positive neutral term, J. Appl. Math. Comput., 20 (2006), 305–314. https://doi.org/10.1007/BF02831940 doi: 10.1007/BF02831940

|
| [16] | A. Murugesan, R. Suganthi, Oscillation behaviour of first order nonlinear functional neutral delay difference equations, Int. J. Differ. Equ., 12 (2017), 1–13. |
| [17] | A. D. Myschkis, Lineare Differentialgleichungen mit nacheilendem Argument, VEB Deutscher Verlag der Wissenschaften, Berlin, 1955. |
| [18] |
S. S. Santra, D. Baleanu, K. M. Khedher, O. Moaaz, First-order impulsive differential systems: Sufficient and necessary conditions for oscillatory or asymptotic behavior, Adv. Differ. Equ., 2021 (2021), 283 https://doi.org/10.1186/s13662-021-03446-1 doi: 10.1186/s13662-021-03446-1

|
| [19] | O. Öcalan, Oscillation criteria for systems of difference equations with variable coefficients, Appl. Math. E-Notes, 6 (2006), 119–125. |
| [20] |
O. Öcalan, O. Duman, Oscillation analysis of neutral difference equations with delays, Chaos, Solitons, Fract., 39 (2009), 261–270. https://doi.org/10.1016/j.chaos.2007.01.094 doi: 10.1016/j.chaos.2007.01.094

|
| [21] | O. Öcalan, Linearized oscillation of nonlinear difference equation with advanced arguments, Arch. Math. (Brno), 45 (2009), 203–212. |
| [22] | N. Parhi, A. K. Tripathy, Oscillation of forced nonlinear neutral delay difference equations of first order, Czech. Math. J., 53 (2003), 83–101. |
| [23] |
N. Parhi, A. K. Tripathy, Oscillation of a class neutral difference equations of higher order, J. Math. Anal. Appl., 28 (2003), 756–774. https://doi.org/10.1016/S0022-247X(03)00298-1 doi: 10.1016/S0022-247X(03)00298-1

|
| [24] | H. Péics, Positive solutions of neutral delay difference equations, Novi. Sad. J. Math., 35 (2005), 111–122. |
| [25] | S. H. Saker, M. A. Arahet, New oscillation criteria of first order neutral delay difference equations of Emden-Fowler type, J. Comput. Anal. Appl., 29 (2021), 252–265. |
| [26] | S. S. Santra, D. Majumder, R. Bhattacharjee, T. Ghosh, Asymptotic behaviour for first-order difference equations I, In: Applications of networks, sensors and autonomous systems analytics, Studies in Autonomic, Data-driven and Industrial Computing, Singapore: Springer, 2022. https://doi.org/10.1007/978-981-16-7305-4_4 |
| [27] |
X. H. Tang, X. Lin, Necessary and sufficient conditions for oscillation of first-order nonlinear neutral difference equations, Comput. Math. Appl., 55 (2008), 1279–1292. https://doi.org/10.1016/j.camwa.2007.06.009 doi: 10.1016/j.camwa.2007.06.009

|
| [28] | M. K. Yildiz, H. Öğünmez, Oscillatory results of higher order nonlinear neutral delay difference equations with a nonlinear neutral term, Hacet. J. Math. Stat., 43 (2014), 809–814. |
| [29] | G. Zhang, Oscillation for nonlinear neutral difference equations, Appl. Math. E-Notes, 2 (2002), 22–24. |
| 1. | Silvio Fanzon, Marcello Ponsiglione, Riccardo Scala, Uniform distribution of dislocations in Peierls–Nabarro models for semi-coherent interfaces, 2020, 59, 0944-2669, 10.1007/s00526-020-01787-5 | |
| 2. | Prashant K. Jha, Timothy Breitzman, Kaushik Dayal, Discrete-to-Continuum Limits of Long-Range Electrical Interactions in Nanostructures, 2023, 247, 0003-9527, 10.1007/s00205-023-01869-6 |