1.
Introduction
Forest fires are a significant environmental problem that affects many regions of the world. These events can have devastating consequences on natural ecosystems, including habitat and biodiversity loss, soil degradation, increased greenhouse gas emissions, and also affect communities that depend on the biome for survival, becoming a major challenge for governments and communities all around the world. In addition, wildfires can be natural or even human-caused [1]. It occurs due to human activity mainly in regions where irresponsible agricultural practices are common. With the expansion of agriculture and cattle raising in the region, many areas of the Cerrado are being deforested for the creation of pastures and grain cultivation. This poses a risk to urban centers, potentially affecting thousands of people. Furthermore, significant climate change and natural causes such as high temperatures, dry weather, and lightning strikes create more conducive conditions for triggering fires and spreading fire.
The Cerrado biome, located in Brazil, is known for harboring a wide variety of species, with thousands of species already recorded. Additionally, it is important to highlight that many of these species are exclusive to this biome, making it one of the most diverse and rich savannas in the world. Cerrado is an example of an ecosystem that is particularly vulnerable to forest fires. This region is known for its unique flora and fauna and is considered a resilient biome due to its ability to recover quickly after fires, even attracting herbivorous animals in search of new fodder. Many species of these animals are capable of following wildfires and feeding on insects and reptiles affected by the fire [2]. However, during the dry season, forest fires can occur frequently and have significant environmental and economic impacts. For this reason, there is a need to develop effective tools and strategies for the management and prevention of forest fires in the Cerrado biome [3].
In this context, computational modeling has become an essential tool in many scientific fields, allowing researchers to simulate complex phenomena and predict the behavior of complex systems. In particular, cellular automata (CA) have proven to be a powerful tool in computational modeling due to their ability to simulate the evolution of systems through the interactions of individual cells. This approach has been successfully applied in many fields, including ecology, epidemiology, and physics, among others. One particularly important application of cellular automata is in modeling wildfires. By simulating the spread of fire through a forest or other vegetation, researchers can gain insight into the behavior of wildfires and develop strategies for prevention and control. This is particularly relevant in the Cerrado Biome in Brazil, which is known for its high incidence of wildfires and diverse vegetation.
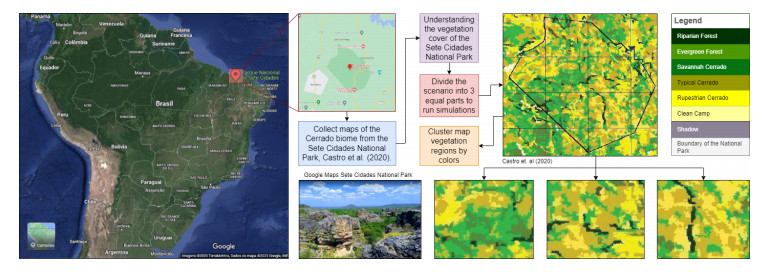
In this context, the paramount objective of this article is to offer a comprehensive model aimed at unraveling the complexities of forest fire propagation within the distinctive Cerrado biome. This innovative approach leans on the cellular automaton (CA) technique, where a dynamic grid of cells evolves over time, guided by a meticulously defined set of rules and parameters. The resultant advanced model has been seamlessly integrated into a dedicated website, empowering users to actively engage in wildfire simulations and to monitor real-time fire propagation dynamics within the Cerrado. Specifically, our study focuses on the Sete Cidades National Park in Piaui, Brazil, which encompasses a diverse range of scenarios [4,5].
The park's unique environment includes arid savanna forest (babassu forests) and transitional zones between savanna, arid savanna, and seasonal forest. It serves to safeguard a significant geological formation and conserve vital water resources in a region characterized by aridity. Notably, the park features remarkable geological monuments, as well as prehistoric cave paintings and inscriptions. Spanning an area of 7,700 hectares (19,000 acres), the Sete Cidades National Park is shared between the municipalities of Brasileira (26.21%) and Piracuruca (73.77%) within the state of Piauí*. It is enveloped by the Serra da Ibiapaba Environmental Protection Area, which covers a vast expanse of 1,592,550 hectares (3,935,300 acres) and was established in 1996. This holistic study encompasses an array of experiments, delving into different vegetation types and environmental conditions, ranging from homogeneous to heterogeneous scenarios, as detailed in the works of [6,7].
*Instituto Socioambiental (ISA), Protected Areas Monitoring Program: Environmnent Conservation Units (ECU) and Indigenous Lands (IL): https://uc.socioambiental.org/arp/1278.
2.
Theoretical foundation
In this section, we will cover the following topics related to the modeling of fire propagation in the Brazilian Cerrado biome: characteristics of the Cerrado biome, including its vegetation and weather patterns; fire spread in the Brazilian Cerrado biome, including factors that affect its behavior; definition of Cellular Automata, including its principles and how it can be used to simulate fire propagation; and finally, research on fire propagation using Cellular Automata simulations, including studies that have been conducted in the Cerrado biome and their findings.
2.1. Description characteristics of the Cerrado biome
The Cerrado biome has a great diversity of species, with about 1.23×104 registered species and a high rate of endemism, making it one of the most diverse and rich savannas in the world. However, it has been destroyed at an alarming rate due to its accelerated occupation, especially since the 1960s, making it highly threatened. Although it is not yet legally considered a national heritage, the Cerrado is a valuable heritage that has lost about 50% of its original coverage. Most of the remaining vegetation has been modified by human activities such as agriculture. Physiognomically, the Cerrado is characterized by typically savanna vegetation, with a lower occurrence of forest and grassland formations [8]. The Cerrado stricto sensu is one of the most prominent physiognomies, with tree cover ranging from 10 to 60%. This type of cerrado has been subdivided into dense cerrado, typical cerrado, sparse cerrado, and rupestrian cerrado, based on the density of shrub-tree and subshrub-herbaceous components and substrate properties.
The Northwest's Cerrado is known as "distal marginal cerrados" and represent one of the biodiversity supercenters of this biome. In this region, there is one of the largest concentrations of cerrados in Northeast Brazil, corresponding to about 14% of the total area of the Northeast Region and 10.8% of all Brazilian cerrado lato sensu. There are several conservation units in Brazil aimed at protecting the maintenance of ecological conditions and the perpetuation of existing species, and the Cerrado has 9.4% of its domains protected by these units. Indirect use conservation units completely restrict the exploitation or use of natural resources, allowing only the indirect use of their benefits.
The creation of plans for the conservation and management of natural areas relies on research in plant ecology, which allows for obtaining qualitative and quantitative information about the area in question and making informed decisions. These studies also provide important information on the structure and dynamics of plant communities, which are essential for the regeneration of natural formations and for understanding their classification, stratification, abundance, associations, and conservation status. Castro's work aimed to characterize the floristic composition and structural properties of a typical cerrado community in the Sete Cidades National Park. The Sete Cidades National Park is a Brazilian conservation unit for the integral protection of nature located in the northern region of the state of Piauí, Brazil, according to Figure 1. The park's territory is distributed between the municipalities of Piracuruca and Brasileira. Its area of 7.7×103 hectares occupies 73.77% of the municipality of Piracuruca and 26.21% of the municipality of Brasileira. It is located 130 kilometers from Parnaíba and receives an average of 2.5×105 visitors per year. The park is managed by ICMBio (Chico Mendes Institute for Biodiversity Conservation).
In our work, on the other hand, we aim to present a probabilistic CA-based fire propagation model that utilizes the realistic characterization from Castro's work, with the goal of supporting decision-making and aiding in the development of public policies for the conservation and restoration of this natural area.
2.2. Spread of fires in the Brazilian Cerrado biome
The spread of fires in the Brazilian Cerrado biome is a common phenomenon and can occur due to natural causes, such as lightning, or human actions, such as uncontrolled burnings. It is important to highlight that the Cerrado is a resilient biome, meaning it is capable of recovering after a fire, but only if the intensity and frequency of fires are not too high. When the fire is too intense and recurrent, it can lead to vegetation degradation and loss of biodiversity.
Therefore, it is crucial to avoid burnings in the Cerrado, especially during dry periods when the risk of fires is higher. Uncontrolled burnings can harm both fauna and flora, as well as contribute to the emission of greenhouse gases in the atmosphere, further exacerbating the impacts of climate change (Figure 2). Additionally, it is important to note that the Cerrado harbors rich biodiversity, with several endemic species, meaning they only occur in this biome, which reinforces the importance of its preservation.
In Brazil, fires are becoming more frequent due to deforestation for the expansion of pasture areas and agribusiness, along with hot and dry weather, especially in the Cerrado region which is naturally hot [2]. Climate change exacerbates the situation by providing increasingly hot and dry weather, which, combined with winds, can lead to devastating fires. For example, in recent years some of the major fires in the Cerrado of Brazil have been documented: Figure 2a in 2020, the Pantanal†, which encompasses parts of the Cerrado, Caatinga, and tropical forests, suffered from the largest forest fires in recent years. The most affected municipalities were Poconé and Cáceres in Mato Grosso, and Corumbá in Mato Grosso do Sul. Figure 2b in 2017, a major fire hit the Serra da Canastra in Minas Gerais [9], causing the death of animals and destroying a large part of the native vegetation. The closest city to Serra da Canastra is São Roque de Minas. Figure 2c in 2015, a large-scale fire destroyed a Cerrado area in the Jalapão State Park in Tocantins [10], affecting the biodiversity of the region. The nearby cities to the park are Mateiros and São Félix do Tocantins. The absence of rain during the dry season further amplifies the risk of large-scale fires in the Cerrado [2].
†Canal Rural: Municipalities in the Pantanal lead the ranking of wildfires and producers reinforce prevention. Access available through the link: https://blogs.canalrural.com.br/canalruralmatogrosso/2020/07/31/municipios-do-pantanal-lideram-ranking-de-queimadas-e-produtores-reforcam-prevencao/.
2.3. Cellular automata definition
Cellular automata (CA) are computational systems based on sets of cells that interact with each other following predefined rules where each cell is represented by a state. They were proposed as mathematical models to simulate the complexity of natural systems like the one that will be treated in the article. The basic principle of CA consists of cells undergoing a state transformation (from the states of neighboring cells), where the evolution of the system is determined through transition rules: deterministic or probabilistic [11]. Cellular automata are widely used in several areas of science to develop the spatial modeling of complex systems that have a large number of local interactions and that can exhibit unpredictable behavior. Among them, we can mention robotics [12,13], modeling of diseases [14], and even forest fire modeling [15], which is the focus of our work. Different works have already been proposed with the objective of modeling fires and forest fires through CA. Among them, we can mention the work of [15] in which CA was used in a two-dimensional form.
The CA can be represented by a vector or matrix L and are classified according to the number of dimensions in which the cells (xij∈L) are arranged, and can be one-dimensional (1D) when the cells are arranged in a line, two-dimensional (2D) when the cells form a grid, or three-dimensional (3D) when the cells are arranged in a row, which means that the cells form a cube. Herein we will study 2D cellular automata applied to fire modeling, which is a complex task because it is influenced by several variables such as topography, climate, vegetation type, region and wind, among others. CA can simulate these factors on a smaller scale, allowing for more accurate modeling of the fire propagation process.
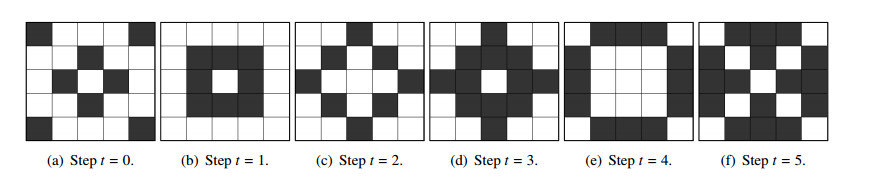
Thus, one of the best-known cellular automata in literature is the Game of Life (GL) proposed by John Conway in 1970. The Cellular Automaton Game of Life, created by John Conway, has the following update rules: (ⅰ) any live cell (black) with fewer than two live neighbors dies (white), as if caused by underpopulation; (ⅱ) any live cell with two or three live neighbors lives on to the next generation; (ⅲ) any live cell with more than three live neighbors dies, as if by overpopulation; and (ⅳ) any dead cell with exactly three live neighbors becomes a live cell, as if by reproduction. Figure 3 represents the T=6 CA-rule evolution for L5×5 considering t=0 (see Figure 3a), t=1 (see Figure 3b), t=2 (see Figure 3c), t=3 (see Figure 3d), t=4 (see Figure 3e), and t=5 (see Figure 3f).
These rules are applied simultaneously to every cell in the grid, and each generation is created based on the previous generation's configuration. The result is a dynamic pattern of live and dead cells that can exhibit complex behavior over time. The Game of Life is a classic example of emergent complexity in complex systems.
Cellular automata are a valuable computational tool for modeling dynamic and complex systems [15]. CA can simulate complex systems with realism, replacing numerical simulations from differential equations. By using a transition rule, either deterministic or probabilistic, the state transformation of CA cells can simulate the dynamic action of different systems [16]. CA has been applied in various contexts, including robotics [11,12], disease modeling [14,17,18], cryptography [19,20,21], and even modeling fires [15] or pedestrian evacuation in panic [22,23,24].
2.4. Research on fire propagation utilizing Cellular Automata simulation
Cellular automata modeling can be an important tool for environmental agencies to predict fire behavior and make informed decisions. Several works have proposed CA models for simulating fires, such as probabilistic 2D-CA or 3D-CA for simulating different fire outbreaks in homogeneous or heterogeneous forests. Herein we conducted a Systematic Literature Review (SLR) to analyze papers from 2017–2022 using StArt Tool (State of Art). From this SLR methodology we selected 20 papers, as as are shown in Table 1.
The term "level", signifies the manual classification we, as authors, assigned when reviewing each article. We established a hierarchical order of priority based on our assessments. On the other hand, "score" corresponds to the rating provided by StArt. In this context, StArt assigns a score to each word in TAK (title, abstract, and keywords). Specifically, it assigns a score of 5 for every word from the search string appearing in the title, 3 for each word found in the keywords, and 2 for every word from the search string present in the abstract.
A key distinction between our model and the model presented by the authors in Table 1 lies in the consideration of six distinct vegetation types, each characterized by unique propagation probabilities, accounting for variations in humidity and dryness. Additionally, we incorporate a preference matrix for wind direction, derived from an evacuation matrix designed for pedestrian movement [44]. Notably, our model is grounded in a real-world scenario, specifically the nuanced landscape of Sete Cidades National Park. This incorporation of real-world conditions adds a layer of authenticity and applicability to our simulation.
The first study analyzed, described in [25], improved the model for real-world integration, introducing a wind flow model that accurately calculates wind parameters based on topography and land use. Authors tested the model with data from northern Morocco, showing the wind's dynamic effect on fire spread. In the work by Ferreira et al. [1], the authors proposed the utilization of genetic algorithms for the automatic adjustment of fire propagation model parameters. This research built upon Lima and Lima's previous work [15] by introducing genetic algorithms to enhance a parameter related to a preference matrix used for wind simulation [15]. Additionally, it extended their prior studies on wildfire simulation using CA and stochastic rules [15,22], as well as their use of 2D-CA for modeling fires in confined environments to facilitate safe evacuations [22]. Experimental results conducted across various scenarios validated the practicality of employing evolutionary computation for parameter optimization in fire spread models.
In [26], authors propose a data-driven approach to derive cell burning probabilities from historical forest fire data, converting them into time estimates for complete cell burning. This method enables simulation of varied fire spread speeds under diverse geographical conditions. Experimental results suggest the effectiveness of this approach for forest fire simulation. The authors of [27] developed a cellular automata-based forest fire spread model for predicting and assessing forest fires. They used field data from Yaji Mountain, Beijing, processed with ArcMap to create an accurate 3D terrain model for simulations, yielding reliable results. Meanwhile, [28] study enhances forest fire spread simulation by combining cellular automata with an existing model. It adapts the time step through a speed change rate index based on meteorological factors and current fire conditions, ensuring 96.9% simulation accuracy. This adaptable model is a valuable tool for fire spread prediction and decision-making.
In the study by [29], a GIS-based CA model was used to simulate wildfire propagation, considering dynamic factors like wind, vegetation, and topography. Calibration employed a genetic algorithm (GA), and model validation using overall accuracy (OA) and the Kappa coefficient indicated effective wildfire spread simulation, providing valuable tools for fire management. [30] discussed the long-lasting impacts of Australian wildfires and the successful use of drones by the Victorian government for fire surveillance. Their paper utilized cellular automata to simulate forest fire initiation and propagation, allowing parameter control for fire frequency and intensity. Historical data categorized regions by importance, enabling variable attention levels. In [31], the complexity of forest fire spread was addressed, highlighting the limitations of CA. To enhance precision, they integrated the Rothermel forest fire rate formula with CA, forming a multi-dimensional cellular automata (MD-CA) model with distinct combustion properties per cell. Formulas for fire spread rates in eight directions were derived from training datasets. Testing showed MD-CA closely approximates real forest fire spread, with area errors of 9.42%–15.63% and perimeter errors of 4.21%–8.99%, surpassing CA. In summary, the Rothermel-based MD-CA effectively simulated controlled fire spread, with ongoing error reduction as a future challenge.
In [32], an advanced hierarchical clustering model was developed for the Amazon forest fire situation. It employed diffusion simulations using a CA model derived from the hierarchical clustering approach. This model analyzes diffusion speed and range based on various brightness parameters. Furthermore, a particle system simulates three-dimensional fire and smoke effects. An entropy-weighted TOPSIS model optimizes wildfire risk ranking with latitude and longitude data, producing a relationship diagram. Simulation results validated the method's feasibility and rationality. In [33], the authors addressed control in a CA model using actuators and attributes. They introduced "attributes" and adapted actuator definitions for CA in connection with attributes. The control method relies on attributes rather than the system's state, as seen in earlier elementary CA works. The study illustrated this approach through forest fire control within a 3D-CA model. In [34], the study aimed to identify and predict mangrove forest changes pre- and post-forest fire incidents. They integrated a Markov Chain and CA model, and employed remote sensing technology, analyzing Landsat satellite imagery from 1989, 1998, 2002, and 2015. The results reveal a 9.6% forest decrease from 1989–1998 due to fires, an 8.4% increase from 1998–2002, and a 2.3% increase from 2002–2015. Projections indicated a continuous expansion in mangrove area, estimating a 27.4% to 31% increase (7974.8 ha) from 2015–2028. This suggests ongoing changes in mangrove ecosystems, potentially influenced by effective government management.
In [35], a physical model was used to simulate fire spread in response to the transboundary haze issue in Southeast Asia, focusing on Dumai as a significant contributor to the problem. Unlike traditional models using Huygens' wave propagation, this model employs cellular automata to predict firefighting interventions, considering the fire's spatial dynamics. It aligns with cellular automata, widely used in diverse scientific fields. The study's findings resemble real-world observations, providing insights into factors influencing fire spread under varying conditions. In [36], forest fire spread was simulated by varying burnability (BurnProbability) from 10% to 80%. For each setting, 17 iterations calculated the percentage of burned forest. The results show that increased BurnProbability leads to more extensive forest burning. At 80%, nearly the entire forest (97.23%) is consumed by fire. In [37], layers like height, slope, aspect, vegetation density, roads, rivers, and climate data were integrated to assess the Golestan region's forests. The wildfire spread model achieved 65% general accuracy and a 59% Cohen's kappa coefficient.
In [38], the devised model coupled CA with an existing forest fire model to improve time accuracy in fire spread simulations. It assesses the impact of time steps on accuracy to identify an optimal value. Validation used a May 2006 forest fire at Daxing'an Mountain, determining the optimal time step as 1/8 of cellular combustion time. Compared to Landsat Thematic Mapper (TM) fire data, the model exhibited high temporal and spatial consistency, with an average Kappa coefficient of 0.6352 and mean accuracy of 87.89%. This algorithm simulates and predicts fire spread and identifies fire source points. In [39], a CA model simulated two small wildland fires in Sardinia, Italy, during the summer of 2010. Derived from a detailed model for large-scale wildfires in Greece, it accounted for landscape features, flammability distribution, and precise wind field data. Qualitatively, simulation results resembled actual data, but significant quantitative disparities occured for small burned areas. Discrepancies between model outcomes and those from Greece, where simulations closely matched fire evolution, are attributed to the fires' small size.
In [40], the paper presented a parallel analysis of forest fire simulations using CA. Two simulations, one with topography and wind effects, were conducted applying Von Neumann's CA rule. Numerical simulations covered grid sizes of 102,202,402,802, and 1602. For a grid size of 1602, serial and parallel codes with topography effect had CPU times of 6076.31 and 2670.43 seconds, respectively. In contrast, CPU times for serial and parallel simulations with wind effect were 186.325 and 128.793 seconds for 1602. Speedup and efficiency for topography effect simulations were reported as 2.27 and 56%, while those for wind effect simulations were 1.44 and 36%, respectively. In [41], a novel approach integrated Extreme Learning Machine (ELM) with CA for simulating forest fire spread. Unlike traditional CA models requiring in-depth studies for local transition rules, the ELM predicts each cell's igniting probability. Validated with data from five western United States fires, the ELM-CA approach aptly describes wind velocity's impact on fire spreading patterns, demonstrating acceptable performance and often outperforming previous studies.
In [42], a CA Forest Fire Forecast System in MATLAB incorporated rules for combustible materials, wind, temperature, and terrain. Simulation results demonstrated efficacy in forecasting forest fire spreading trends under various conditions. In [43], a 3D cellular space fire spread model using genetic algorithms was proposed. Addressing 2D CA model limitations, the model incorporated main factors influencing forest fire spread, improving the initial mode with a 3D spherical cell space. This resulted in reduced iterations and running time, enhancing operational efficiency. The CA termination condition is explicit, and simulation results closely mirrored the actual forest fire spreading process.
3.
Proposal
In this section, we will present three models for the spread of fires. First, a basic model of CA rule updating will be introduced. Then, a model that considers the action of wind will be presented. Finally, a model that takes into account the different types of vegetation that affect fire propagation will be discussed.
3.1. Initial base model
Initially, as shown in Figure 4, the CA states are set in TA (t=0). Later, a fire focus is allocated to the grid L5×5. At this moment, a fire is started in the lattice TBi, 1≤i≤4. In other words, the fire intensity assumes 4 possible states (colors) depending on how long the vegetation has been burning. Thus, in this example, tbi=2 (burning time), the fire changes its intensity every t=2 steps. If cell xij has one or more neighboring cells on fire, then it has a non-zero probability (p(xij)) of catching fire (TA→TB) at a later time; in cases of homogeneous forests, p(xij)=0.6. Finally, when the fire reaches a certain burning state, the tree is completely destroyed (dead) and goes to the state TD.
3.2. Variation of wind speed
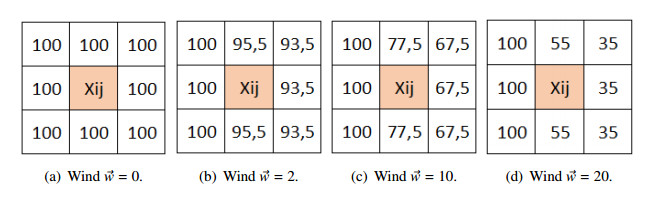
When developing 2D CA models to simulate wildfires, it is crucial to consider the impact of wind on fire spread. Wind plays a major role in determining the direction, speed, size, and shape of fire fronts. Thus, incorporating wind speed and direction into CA models can enhance the accuracy and realism of simulations. A matrix field can be used to represent wind in a CA model, indicating the wind's speed and direction at each cell, and the matrix remains constant throughout the simulation. This matrix field can then be used to calculate the probability of a cell igniting and spreading fire based on the wind's direction and speed. The wind preference matrix used in this study was based on previous works [1,15,44], and can be observed in Figure 5.
There are different directions and velocities that wind can assume, for example: wind (→w=0) in Figure 5a, wind (→w=2) in Figure 5b, wind (→w=10) in Figure 5c, and wind (→w=20) in Figure 5d. Cells downwind from burning cells are more likely to ignite, while cells upwind are less likely to ignite. In addition, the wind matrix can also affect the shape and size of the fire front. For example, when wind blows in a perpendicular direction to the fire front, it can cause the fire to spread faster and in a more elongated shape, while wind blowing parallel to the fire front can cause the fire to spread in a more circular shape.
Besides that, wind plays a crucial role in the propagation of fires in heterogeneous landscapes. In such landscapes, the fire's spread can be influenced by the direction of wind blowing across patches of different vegetation types. For example, a wildfire in a forest with varying types of vegetation can spread faster through areas with highly flammable vegetation, such as dry grasslands or dead wood, when blown by winds in the right direction. However, wind can also slow down or prevent fire spread in areas with less flammable vegetation, such as wetlands or regions with dense vegetation cover. Therefore, the inclusion of wind in CA models is essential to creating precise simulations of wildfire propagation. This can lead to a better understanding of fire behavior in various environments and help develop effective strategies for preventing and controlling wildfires.
3.3. Impact of vegetation type
The spread of forest fires is strongly influenced by the type of vegetation present in the affected area. Vegetation types most susceptible to fire, such as dry grasslands and forests with large amounts of dead wood, are at greater risk of ignition and rapid spread. Additionally, other factors such as fuel, temperature, humidity, and wind can also contribute to the occurrence and spread of forest fires.
When using CA models to simulate wildfires, it is crucial to consider the type of vegetation in the area being modeled. This can be achieved by creating models with homogeneous or heterogeneous vegetation cover. Homogeneous models are useful for studying fire behavior in a specific type of vegetation, since all cells have the same characteristics. On the other hand, heterogeneous models provide more realistic simulations of real-world scenarios, where various types of vegetation and landscape features interact with each other in complex ways.
In a heterogeneous model, the presence of different types of vegetation and landscape characteristics can affect the behavior of forest fires. For example, a river can act as a natural barrier that prevents fire from spreading to neighboring patches of vegetation. Likewise, the presence of different types of vegetation with different levels of flammability can create firebreaks that can slow or prevent the spread of fire. Therefore, a heterogeneous model can provide more accurate and realistic wildfire simulations than a homogeneous model. To incorporate different vegetation types into the model, we introduce a heterogeneous state approach, where different vegetation types are assigned different burn probabilities based on their properties. For example, dry forests are more likely to burn than temperate forests with higher vegetation, while very wet forests have zero probability. In our model, we are considering the Sete Cidades National Park with 6 different types of vegetation. By considering vegetation diversity and other landscape features, CA models can improve simulations and help develop effective wildfire prevention and control strategies.
In this sense, there may be areas where the vegetation is more heterogeneous, presenting a combination of characteristics from different types of vegetation. In these cases, it is possible that the vegetation presents an intermediate state between the predominant types, with characteristics of both. This intermediate state can be described as "mixed" or "transitional", indicating that the vegetation has characteristics of two or more different types. This diversity of vegetation types is common in natural environments and can be influenced by factors such as topography, water availability, solar intensity and the presence of other organisms.
The presence of different types of vegetation in a forest can affect the spread of forest fires, with drier areas presenting a greater fire risk than wetter areas. Therefore, it is important to consider vegetation heterogeneity when modeling wildfire spread. When using CA to model wildfires, it is important to consider the type of vegetation present in the environment, as well as the presence of other landscape features that may influence fire spread. In this study we take into account the realistic shape of a specific scenario and the type of vegetation in the Sete Cidades National Park. This has helped us create more accurate and useful simulations, which in turn can contribute to the development of better wildfire prevention and control strategies. To better understand the geographic data and each vegetation type of Sete Cidades National Park, we use an image clustering approach, which will be described below.
3.4. Clustering Cerrado real maps
The selected image of Sete Cidades National Park was used to create a matrix that represents the lattice L of CA, after collecting each pixel in RGB (red, green, and blue) format. Subsequently, clustering was performed to transform each cell xij of the matrix into a different state, representing distinct types of vegetation. Six vegetation types were found, including mesophytic cerrado, flooded gallery forest, semideciduous dry forest, typical cerrado, rocky cerrado, and open grassland. Figure 1 shows the colors for each of these states. This analysis is important for understanding the heterogeneity of the characteristic vegetation of the Cerrado, which was used in modeling real scenarios using stochastic two-dimensional cellular automata.
In terms of territorial extension, the typical savanna occupied the first position (37.6% of the area), followed by the mesophytic savanna (24.3%) and open field (14.3%), with a predominance, therefore, of savanna formations, confirming that the vegetation is predominantly Cerrado, as confirmed by its oreadic vegetation [6]. However, since we only used three parts of the park in the simulation, as shown in Figure 1, these proportions may have been different.
Below we present an algorithm that represents the process of clustering the pixels of the images that represent the maps of the Sete Cidades National Park.
1. The selected image of Sete Cidades National Park was used to create a matrix representing the CA lattice L, after collecting each pixel (pRij, pGij, pBij) in RGB format.
2. Clustering was performed to transform each cell xij of the matrix L into a different state representing distinct types of vegetation (heterogeneous model).
(a) Check the colors of the pixels on the map of the Sete Cidades National Park (see Figure 1).
(b) Assign a reference RGB color (qRij, qGij, qBij) to each type of vegetation on the Sete Cidades National Park Map (initial points for starting the algorithm).
(c) Identify the different types of vegetation from the colors of the pixels (Figure 1).
i. Use the Euclidean distance in the clustering process to measure the similarity between pixel colors and group them according to their similarity. The Euclidean distance between two points p=(pRij,pGij,pBij) and q=(qRij,qGij,qBij) in an n-dimensional space is given by Eq 1:
ii. Assign one of six vegetation types to cell (xij): mesophytic cerrado, flooded gallery forest, semideciduous dry forest, typical cerrado, rocky cerrado, and open grassland.
(d) Carry out the clustering process to group each pixel to a certain type of vegetation (xij∈L).
3. Repeat the process until all the pixels of the images that represent the Sete Cidades National Park Maps are assigned to a state.
Thus, a CA-lattice L is created that serves as an initial input at time t=0 for the 2D-CA that represents the map of the Sete Cidades National Park, with clustering of pixels into vegetation types using Euclidean distance and attribution reference colors. Six vegetation types were identified and the process is repeated until all pixels are assigned a state.
3.5. Algorithm for Cerrado wildfire model
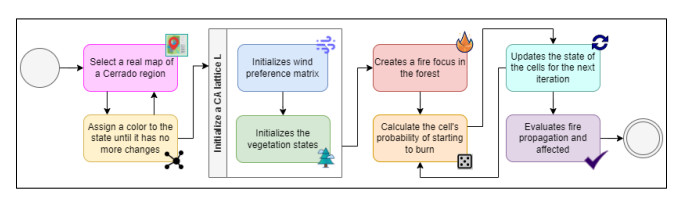
In this study, we utilize a methodology to simulate the spread of forest fires in the Cerrado biome. We employ the cellular automaton (CA) technique, which is a mathematical tool that can simulate complex and dynamic systems. We conduct various experiments by considering different types of vegetation, including both homogeneous and heterogeneous forests. The algorithm used for simulation can be summarized in a few steps:
1. Select a real map of a Cerrado region for which it is possible to simulate fire propagation and perform the clustering of the vegetation by RGB colors to create a realistic simulation using the Euclidean distance between maps pixels p and RGB reference colors q.
2. Repeat process 1 until all pixels in the image are assigned a single, distinct state with no color changes.
3. Initialize a matrix for CA lattice Lm×n, where each cell xij represents an area of vegetation and is initially filled with state 0 (live or intact area) for t=0;
(a) Initialize a preference matrix →W representing the wind vector (→w) and set the parameters for wind direction and velocity.
(b) Initialize the vegetation states, which can include heterogeneous vegetation (TD11) Rupestrian cerrado, (TD12) Clean camp, (TD13) Typical cerrado, (TD14) Dense cerrado, (TD15) Evergreen forest, and (TD16) Riparian forest, according to [45].
(c) Establish distinct fire probabilities for various land cover types: (TD11) Rupestrian cerrado with p(xij)=80, (TD12) Clean camp with p(xij)=65, (TD13) Typical cerrado with p(xij)=40, (TD14) Dense cerrado with p(xij)=25, (TD15) Evergreen forest with p(xij)=10, and (TD16) Riparian forest with p(xij)=0.
4. Initiate a fire focus in the forest by changing the state of the matrix cell to 1 (burning) for tbi∈TBi=1,2,3,4,5, representing different fire intensities.
5. For each cell that is in state 0, calculate the probability of starting to burn in the current iteration from the state of neighboring cells in the previous iteration, considering factors such as wind and type of vegetation.
6. Update the state of the cells for the next iteration, increasing the state by 1 for all cells that were burning in the previous iteration (t=t+1) and changing the state of the cells to fully burned, then TD.
7. Repeat steps 5 and 6 for T defined iterations, updating the states of L by the CA probabilistic rule.
8. Evaluate fire propagation and affected area metrics as the percentage of the total green area burned.
Figure 6 depicts the state diagram of the forest fire model, which encompasses the initialization of matrix and vegetation states, creation of the fire focus, calculation of the burning probability, updating cell states, and evaluation of the fire.
4.
Methodology
In this methodology section, we will comprehensively outline our robust research methods and carefully selected materials for an extensive analysis of wildfire behavior and vegetation burning rates within the study area, ensuring a comprehensive understanding of the complex interactions influencing these ecological processes.
4.1. Research methods
Our research paper adopts a qualiquantitative approach, which effectively combines both qualitative and quantitative methodologies, to provide a comprehensive understanding of the factors influencing wildfire behavior and vegetation burning rates. This approach is justified by the complexity of the subject matter, which involves multifaceted variables like wind conditions, vegetation types, and fire progression dynamics. We performed 100 different simulations for each scenario that ensures the robustness of the dataset, making it reliable for statistical analysis.
Wildfires are complex natural events influenced by a multitude of factors. A purely quantitative approach may not capture the nuanced interactions between these factors. By incorporating qualitative data, we gain a deeper understanding of the contextual nuances, such as the environmental conditions in Sete Cidades National Park, which are pivotal in comprehending wildfire behavior. A qualiquantitative approach combines both quantitative data, collected from sensors and measurements, and qualitative insights gained from field observations and expert opinions. This holistic approach is vital for a comprehensive understanding of wildfire scenarios, essential for broader implications like environmental conservation and wildfire management. We used qualitative information because it enriches the interpretation of quantitative results, providing meaningful context and real-world relevance in our paper. Addressing interdisciplinary aspects of wildfire management, our approach integrates knowledge from multiple fields to enhance our analysis.
4.2. Materials
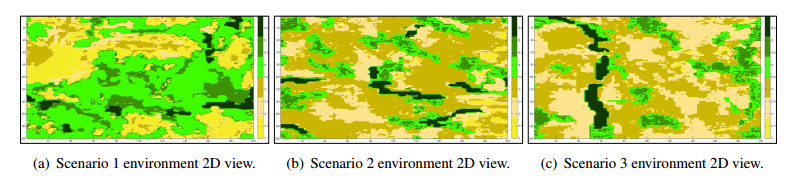
In a CA-lattice comprising |L|=200×200 cells, we encountered a diverse landscape representative of the unique ecosystem at hand. Figure 7 was generated using MATLAB's contourf(L) function, illustrating three distinct 2D-lattice scenarios, while Figure 8 presents the 3D-lattice vegetation visualization, also created using the surf(L) function in MATLAB. These scenarios are also visualized in Figure 1. In this study, Scenario 1 was employed. As illustrated in the 2D-lattice in Figure 7a and the 3D-lattice in Figure 8a, the Rupestrian Cerrado (Vegetation 11) spans 7953 lattice cells, representing around 19.88% of the lattice and highlighting the resilience of this specific vegetation type. Clean camp (vegetation 12) was present in 1809 lattice cells, comprising 4.52% of the area and contributing to the overall ecological diversity. The Typical Cerrado (vegetation 13), occupying 5638 lattice cells, made up 14.10% of the landscape, illustrating the variety within the Cerrado biome. The lattice's largest component was the Dense Cerrado (vegetation 14), with 16,940 lattice cells, covering approximately 42.35% of the area and emphasizing the dominant nature of this vegetation type. Evergreen Forest (vegetation 15), extending across 6139 lattice cells, represented 15.35% of the landscape and provided an essential habitat within this ecosystem. Lastly, Riparian Forest (vegetation 16), found in 1521 lattice cells, constituted 3.80% of the lattice, underlining the significance of riparian zones in this complex and biodiverse environment, as is possible to see in Table 2.
The study area's Scenario 2 showcases a diverse array of vegetation types, each making a unique contribution to the ecosystem. This diversity is visually depicted in both 2D (Figure 7b) and 3D (Figure 8b) representations. Notably, Rupestrian Cerrado (vegetation 11) covers approximately 3.03% of the region, characterized by its hardy and rocky terrain. Clean camp (vegetation 12), accounting for 16.82% of the area, represents open spaces typically associated with grasslands and meadows.
Typical Cerrado (Vegetation 13) dominates the landscape, covers a substantial 52.77% of the study area, offering both habitat and sustenance for various species. Dense Cerrado (Vegetation 14), making up 13.77%, contributes to the region's ecological complexity with its thick and lush growth. The evergreen forest (Vegetation 15), representing 9.05% of the landscape, is essential for maintaining green cover throughout the year. Lastly, the riparian forest (Vegetation 16), encompassing 4.56% of the region, plays a vital role in preserving water quality and supporting aquatic ecosystems along watercourses. This diverse array of vegetation types is described in Table 2.
In Scenario 3, as depicted in Figure 7c for the 2D-lattice and Figure 8c for the 3D lattice, the distribution of vegetation types is as follows: Rupestrian cerrado (Vegetation 11) covers 416 CA-cells, constituting approximately 1.04% of the area. Clean camp (Vegetation 12) occupies the largest portion, with 11,312 CA-cells, accounting for 28.28% of the landscape.
Typical cerrado (Vegetation 13) encompasses 18,455 CA-cells, representing 46.14% of the scenario. Dense cerrado (Vegetation 14) is found in 4,644 CA-cells, making up 11.61% of the area. Evergreen forest (Vegetation 15) is present in 3,488 CA-cells, contributing 8.72% to the landscape. Lastly, Riparian forest (Vegetation 16) is observed in 1,685 CA-cells, covering 4.21% of the scenario. This diverse vegetation distribution plays a crucial role in understanding the ecological dynamics of Scenario 3, as can be seen in Table 2.
5.
Results
The conducted experiments are designed to facilitate qualitative comparisons regarding the impact of varying parameters within the fire propagation model in homogeneous forests. This exploration employs a two-dimensional cellular automaton (2D-CA) with a lattice size of 200×200 cells, characterized by probabilistic transition rules and a non-periodic contour. We opted for fixed boundary conditions, a choice guided by the specific characteristics of our simulated system and the desired outcomes of the CA dynamics. Three distinct scenarios, as visualized in Figures 7 and 8, serve as the basis for our experiments. In the subsequent discussion, we will present both qualitative and quantitative analyses to illuminate the influences of Sete Cidades National Park, incorporating factors such as wind force and vegetation type.
5.1. Storyboards for qualitative analysis of various vegetation scenarios
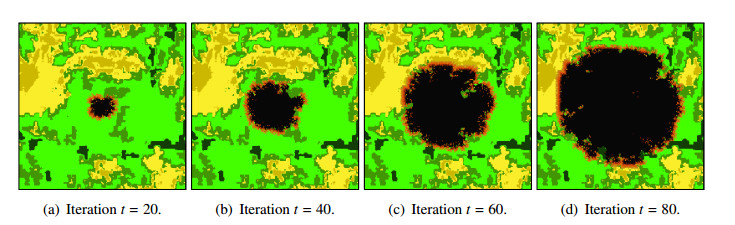
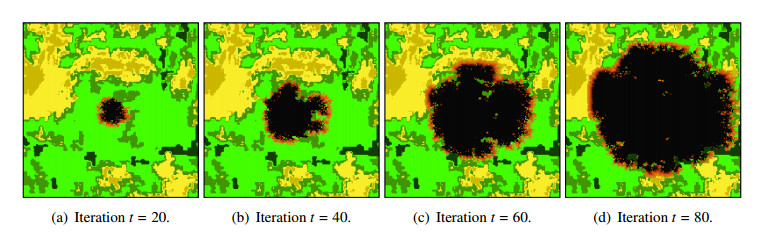
The sequence of images presented in Figure 9 illustrates the fire development in Scenario 1 under windless conditions (→w=0) across different temporal stages. At iteration t=20 (Figure 9a), the fire initiates with a limited impact, burning merely 1.12% of the vegetation. Progressing to iteration t=40 (Figure 9b), the fire intensifies, expanding its reach and consuming 5.82% of the total vegetation. By iteration t=60 (Figure 9c), the fire continues its advance, affecting a more substantial portion of the landscape, with 16.14% of the vegetation being consumed.
Finally, at iteration t=80 (Figure 9d), the fire has reached an advanced stage, significantly impacting the area, with 34.34% of the vegetation burned.
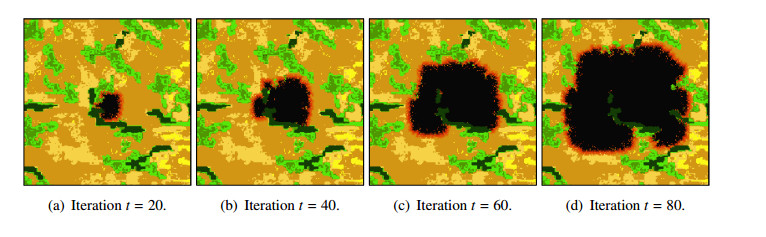
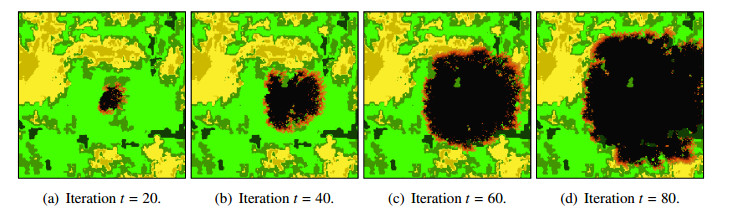
Figure 10 depict the evolution of the simulated fire spread in a scenario without wind (→w=0) at different iterations. As the iterations progress, we observe a significant increase in the burned cells, indicating the dynamic nature of fire propagation within the modeled landscape. In Figure 10a at iteration t=20, the fire is in its initial stages, affecting a relatively small area; in this case, the fire consumed 2.37% of the natural vegetation. Moving to iteration t=40 in Figure 10b, the fire has expanded, engulfing a larger portion of the vegetation, and the burned area now constitutes 8.48% of the total vegetation. Subsequently, at iteration t=60 in Figure 10c, the fire continues to spread, covering a substantial portion of the landscape, with the burned area extending to 20.87%.
Finally, at iteration t=80 in Figure 10d, the fire has reached a more extensive and intensified state (41.01%), resulting in a significant increase in burned cells. This simulation without wind provides a baseline understanding of fire behavior in the absence of wind influence, serving as a comparative reference for scenarios with varying wind conditions.
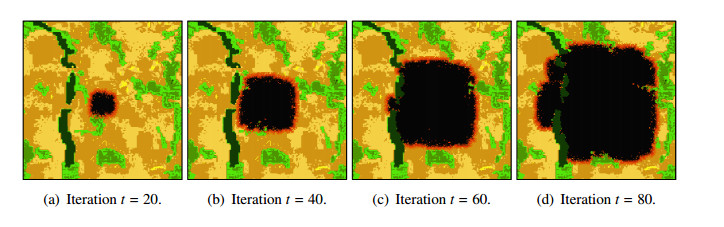
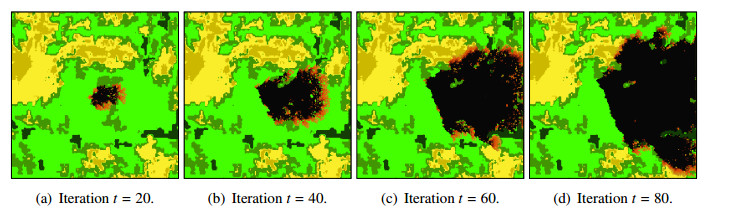
The series of simulations depicted in Figure 11 showcase the fire progression in Scenario 3 under windless conditions (→w=0) at different time steps. At iteration t=20 (Figure 11a), the fire initiates, affecting a relatively limited area, with 3.23% of the vegetation being consumed. Advancing to iteration t=40 (Figure 11b), the fire intensifies, leading to a larger burned area that now constitutes 13.45% of the total vegetation. By iteration t=60 (Figure 11c), the fire continues to spread, covering a considerable portion of the landscape, with 28.14% of the vegetation being consumed.
Finally, at iteration t=80 (Figure 11d), the fire has reached an advanced stage, impacting almost half of the vegetation (48.52%). This progression demonstrates the temporal evolution and increasing impact of the fire in Scenario 3 when wind is absent.
This visual representation provides insights into the temporal evolution of the fire and its increasing impact on Scenarios 1, 2, and 3 without wind influence. The escalating burned percentages highlight the progressive nature of the fire, underscoring the importance of understanding its dynamics for effective wildfire management in heterogeneous landscapes. Scenario 3 exhibits a higher propensity for a burning rate than Scenarios 1 and 2, and its limited spread is attributed to the presence of a riparian forest in the middle of the scenario. Scenario 1 is less prone to burning due to its specific vegetation type, while Scenario 2 represents an intermediate propensity for ignition and fire spread.
5.2. Qualitative analysis of wind intensity forces
The result of the simulation is a visual representation of fire spread, which can be used to analyze different scenarios and understand how fire spread is affected by different factors and variables. The simulation starts with a cell on fire and the fire spread is updated for each cell at each time step (iteration).
In the experiments carried out, the effect of varying the wind parameter on the propagation of fires in heterogeneous forests was compared. Throughout the experiment, the wind parameter was varied from (→w=0) to (→w=20), with →w being zero (0) wind and (20) being extremely strong wind. The effect of wind over time was also analyzed, and for this purpose the simulations in iterations t={20,40,60,80} were taken. Initially, the wind parameter was kept at zero (→w=0), and it was observed that the fire spread in all directions more uniformly, see Figure 11. The wind parameter was increased to (→w=5), and the simulation showed that the fire spread started to tend towards the right. The fire spread faster towards the wind direction, with the cells burning more often and more intensely, see Figure 12.
The wind parameter was increased to (→w=10), and the simulation showed that the fire spread became even more intense downwind, see Figure 13.
Cells burned with greater frequency and intensity towards the wind, while cells on the opposite side had a lower rate of burning.
The wind parameter was increased to (→w=15), see Figure 14, and the simulation showed that the fire spread continued to intensify and became even more selective.
Cells burned more frequently and intensely towards the wind, while cells on the opposite side had an even lower rate of burning. The wind parameter was increased to (→w=20), and the simulation showed that fire propagation was more intense than in previous iterations (Figure 15).
The fire spread faster towards the direction of the wind, and the cells burned more often and more intensely in that direction.
5.3. Relationship between wind force and scenario to evaluate burning rates
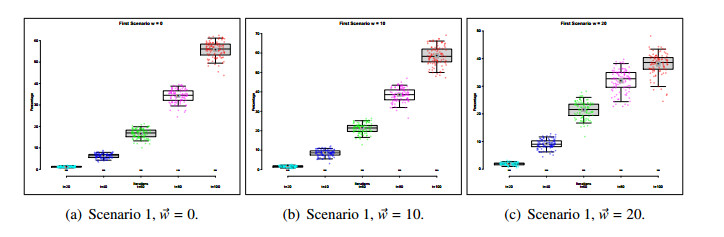
This section investigates this relationship by comparing statistical data derived from different wind forces (→w={0,10,20}). By analyzing the upper whisker, median, and lower whisker values for each wind force, we can gain insights into the influence of wind on fire behavior in Sete Cidades National Park.
The first scenario is shown in Figure 1. This study utilizes statistical analysis, including box plots, to examine the influence of different wind forces (0, 10, and 20) on fire progression within the park. We aims to understand the dynamic relationship between wind force and the rate of burning vegetation in Scenario 1, as is shown in Table 3. These statistics are pivotal for comprehending the dynamics of wildfires within Sete Cidades National Park. The key findings and patterns emerging from this data table underscore the influence of wind intensity and time steps on the rate of vegetation burning.
The upper whisker values reveal the upper limits of the percentage of burning for each scenario. Notably, we observe a substantial increase in burned vegetation from 1.92 at t=20 to 62.46 at t=100 under calm wind conditions (wind →w=0), see Figure 16a. This significant progression demonstrates the impact of time steps on fire behavior. The 3rd quartile values offer insights into the central 50% of the data. It is evident that the rate of vegetation burning steadily rises with both increased time steps and wind intensities. This finding highlights the role of time steps and wind in promoting fire spread. In Figure 16b, when wind is more intense (→w=10), the median values represent the midpoint of the data. Similar to the 3rd quartile, the median demonstrates a continuous increase in the rate of vegetation burning, further emphasizing the influence of time steps and wind intensities. The 1st quartile values reveal the central 25% of the data. They reflect the lower bounds of vegetation burning. These values also show a consistent increase, signifying the impact of time steps and wind on fire progression. The mean values provide an average perspective. They confirm the overall trend of increasing vegetation burning rate with higher time steps and wind intensities; in this case, when →w=10, the mean is bigger than →w=0. The lower whisker values indicate the minimum extent of burning for each scenario. Even in less windy conditions (wind →w=0), the rate of vegetation burning can be significant, reaching 48.19 at t=100. This data underscores the importance of understanding and managing wildfires under various conditions.
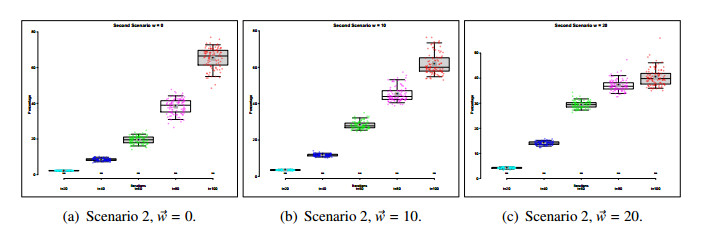
Figure 16c shows the results for wind →w=20. In this case, upper whisker of the box plot shows the highest values for burned areas. At t = 100, the upper whisker reaches 46.13, indicating that there are data points with considerably higher burned areas, which may represent more severe fire outbreaks. In Scenario 2, the 3rd quartile at t=100 is 41.9, suggesting that most data points experience relatively lower burned areas, but a quarter of the data surpasses this value.
At t=100, the median is 39.83 and the 1st quartile at t=100 is 37.64, implying that a quarter of the data has lower burned areas, while 75% of the data experiences higher values. At t=100, the lower whisker reaches 35.98, suggesting the presence of data points with relatively minimal burned areas. At t=100, the mean is 40.33, offering a central value that summarizes the overall fire progression, considering all data points.
Table 4 presents valuable statistics for evaluating the percentage of burning in scenario 2 at five different CA steps (t) and three distinct wind intensities (→w).
These statistics offer crucial insights into how wind conditions impact the rate of vegetation burning, which is of significant importance in wildfire management. For the scenario with no wind (→w=0), the upper whisker indicates an increase in burned vegetation from 2.46 at t=20 to 72.78 at t=100, highlighting substantial fire progression even in calm wind conditions, see Figure 17a.
The other quartiles and the median follow a similar increasing trend, emphasizing the continuous rise in the rate of burned vegetation.
Conversely, when moderate wind force (→w=10) is introduced, the upper whisker exhibits a more rapid fire spread, peaking at 73.41 at t=100, emphasizing the substantial influence of wind on the fire's behavior, see Figure 17b. Similar trends are observed in the other quartiles and the median, signifying a significant increase in the burned vegetation rate. In the presence of high wind force (→w=20), the upper whisker once again demonstrates rapid fire spread, peaking at 46.13 at t=100, see Figure 17c. This data confirms that strong winds can promote more extensive fires, as seen in the other quartiles and the median.
The data presented in Table 5 demonstrates how different wind intensities influence the percentage of burning in Scenario 3 at various time steps (t={20,40,60,80,100}).
This information is crucial for understanding the role of wind in fire spread simulations. The upper whisker, 3rd quartile, and median values consistently increase with time in all wind scenarios. For example, when →w=0 (without wind), see Figure 18a, the average burning percentages are lower than in case of →w, when we have more wind intensity, see Figure 18b. This suggests that as time progresses, the percentage of burning generally becomes higher, which aligns with the nature of wildfires as they tend to spread and intensify over time if not controlled.
Comparing the three wind intensities, it is evident that higher wind intensity (wind →w=20) generally results in a more significant spread of the fire, see Figure 18c. This is evident from the higher upper whisker, 3rd quartile, and median values in this wind scenario across all time steps. This indicates that stronger winds contribute to a more extensive area being affected by the fire. The upper whisker shows the presence of outliers with extremely high values. These outliers may represent critical situations where the fire spreads rapidly and extensively due to a combination of factors, including wind.
In the context of fire management and prevention, these findings highlight the importance of considering wind conditions when predicting and controlling wildfires. The data shows that increased wind intensity can substantially influence the extent of fire spread, making it a critical parameter for accurate simulations and decision-making. Managing wildfires effectively requires strategies that account for wind variations, especially in scenarios with wind intensities similar to the →w=20 scenario, where fire spread can be most severe.
5.4. Comparison of burning percentage between all scenarios
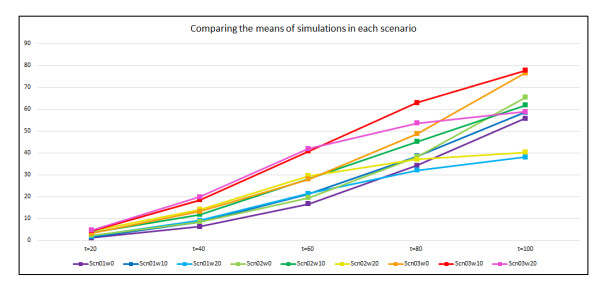
The means of burned vegetation for each scenario in the Sete Cidades National Park, considering different wind forces (0, 10, 20) and various cellular automata (CA) iterations (t), reveal important insights into the impact of these factors on fire propagation. In Scenario 1 (Scn01), the influence of different wind forces on fire propagation within the Sete Cidades National Park is evident. When wind force is absent (wind force 0), the progression of the fire is characterized by a relatively slow start at 1.18 at t=20, but it intensifies significantly over time, reaching 55.8 at t=100. However, introducing a moderate wind force (wind force 10) accelerates the spread of the fire, with the burned vegetation reaching 58.58 at t=100. Notably, when the wind force is further increased to 20, the fire initially peaks at 38.05 at t=60. However, after this point, its intensity decreases.
This intriguing observation suggests that extremely high wind forces may not consistently lead to more extensive fires, as there appears to be an optimal point where the fire's progress is most influenced by the wind. These findings underscore the complexity of fire behavior in the presence of different wind conditions.
In Scenario 2 (Scn02), the impact of wind force on fire behavior becomes evident through a series of simulations. When there is no wind (wind force 0), the fire exhibits steady growth, increasing from an initial 2.25 at t=20 to a substantial 65.36 at t=100. The progression is noticeable, highlighting the inherent vulnerability of the area to wildfires. When wind force is introduced at a moderate level (wind force 10), the fire's spread accelerates significantly. It reaches its peak at 61.88 at t=100, emphasizing the substantial role wind plays in driving fire propagation. However, it is essential to note that extreme wind forces (wind force 20) may not consistently result in the most severe fires. While the fire intensifies more rapidly, reaching 40.33 at t=80, it subsequently experiences a slight decrease. This observation suggests that there may be a threshold beyond which excessively strong winds hinder the fire's progression, underlining the complex interplay of environmental factors in wildfire dynamics.
In Scenario 3 (Scn03), a detailed examination of fire progression under varying wind forces and cellular automata (CA) iteration times reveals valuable insights into wildfire dynamics. When wind force is absent (→w=0), the fire gradually intensifies, escalating from 3.21 at t=20 to a substantial 76.58 at t=100. However, the introduction of moderate wind (→w=10) significantly amplifies the fire's spread, reaching a higher peak of 77.73 at t=100, underscoring the substantial impact of even moderate winds on fire behavior. Furthermore, under high wind force conditions (→w=20), the fire rapidly spreads, peaking at 58.85 at t=100. This data provides clear evidence that strong winds can indeed foster the propagation of more extensive and potentially destructive fires. These findings emphasize the importance of considering wind conditions and implementing effective wildfire prevention and management strategies, especially in areas prone to such natural disasters.
These results underline the impact of wind force and CA iteration time on the spread of wildfires in the Sete Cidades National Park. While wind can accelerate fire propagation, extremely high wind forces might not always lead to more severe fires, as there may be a point where it hinders the fire's progress. Additionally, the simulations underscore the need for effective fire prevention and management strategies, especially in areas susceptible to wildfires like the Sete Cidades National Park.
6.
Discussion
We embrace a qualiquantitative approach to thoroughly explore the myriad factors shaping wildfire behavior in Sete Cidades National Park, Brazil, aligning with the comprehensive investigations conducted in works such as [1,25,34]. Given the intricate interplay of numerous variables in our study area, effective wildfire management necessitates a holistic understanding of these elements, uniquely susceptible to fire [2]. This distinct context contrasts with studies in other biomes like the Amazon [32], Canada [43], Italy [39], and Indonesia [34]. The integration of qualitative and quantitative data in our analysis offers a comprehensive and insightful examination, valuable for researchers, policymakers, and environmental conservationists alike.
Our study reveals that under windy conditions and with extended time steps, the likelihood of vegetation burning significantly amplifies, echoing the findings in [40]. As time elapses, the fire gradually spreads, and when the wind intensifies, represented by →w=5,10,15,20, the fire undergoes rapid expansion. Notably, the type of vegetation exerts a considerable influence on this propagation. In specific instances, especially when the wind speed is →w=10,15,or20, the fire within the cellular automaton (CA) at time t=80 extends beyond the boundaries of the CA-grid. Despite the percentage calculation suggesting a seemingly reduced impact, this perception is deceptive, as the diminished proportion is solely a consequence of the fire being located outside the grid. This information is pivotal for effective wildfire management and environmental conservation efforts in the region, underscoring the necessity for inclusive strategies that account for both temporal dynamics and environmental factors. The consistency of these patterns across varying wind intensities emphasizes the decisive role of wind conditions in influencing the rate of burned vegetation in the studied scenario. Such insights are invaluable for assessing and managing wildfire risk, particularly in environments like Sete Cidades National Park, where diverse wind conditions can profoundly impact fire behavior.
Furthermore, it is crucial to recognize that vegetation scenarios play a vital role in influencing the likelihood of fire spreading across different types of vegetation. Dryer vegetation increases the probability of burning, while more humid vegetation exhibits controlled burning rates. This underscores the importance of contemplating urban sustainability, as environmental conditions can have a profound impact on fire dynamics. Despite the Cerrado being recognized as a resilient biome, neglecting environmental factors can lead to severe consequences.
Finally, our study focused on a specific scenario within Sete Cidades National Park, which encompasses six distinct vegetation types. It is imperative to acknowledge the potential inclusion of other vegetation types in similar analyses. In such scenarios, wind emerges as a crucial factor significantly influencing the speed of fire spread. Our findings highlight the critical importance of considering wind patterns when assessing and mitigating wildfire risks in the region.
7.
Conclusions
In conclusion, this study has delved into the complex dynamics of wildfire propagation in the unique ecological setting of Sete Cidades National Park, Brazil. Employing a qualiquantitative approach, we amalgamated qualitative and quantitative data to gain a comprehensive understanding of the multifaceted factors influencing wildfire behavior. Our investigation unveiled the substantial impact of wind conditions and extended time steps on the rapid spread of vegetation fires. The integration of these findings is crucial for the development of effective wildfire management strategies, emphasizing the necessity for inclusive approaches that consider both temporal dynamics and environmental factors.
Furthermore, the meticulous examination of diverse vegetation types within the selected scenario underscores the heterogeneous nature of the Brazilian Cerrado biome. Recognizing the varied responses of different vegetation types to fire spread is paramount for implementing tailored conservation and management practices. Notably, our study revealed instances where the fire extended beyond the computational grid, highlighting the need for nuanced analyses that account for such occurrences.
The consideration of six distinct vegetation types in our scenarios adds a layer of realism to our findings, paving the way for future research to explore additional vegetation variations. Specifically, further investigations could delve into the fire spread in three different scenarios of Sete Cidades National Park, each representing unique environmental conditions and vegetation compositions. This expanded scope would provide a more comprehensive understanding of the complex dynamics of fire propagation in diverse landscapes.
As we move forward, it becomes imperative to extend our insights to incorporate a broader range of scenarios, enabling a more comprehensive grasp of the factors influencing wildfire behavior in the Brazilian Cerrado. Such a nuanced approach ensures that conservation and management strategies are not only effective but also adaptable to the intricate interplay of environmental variables in different regions of the Cerrado biome.
Looking ahead, the insights gleaned from this research contribute to the broader field of wildfire modeling and management, particularly in regions characterized by diverse ecological factors. The significance of wind conditions and vegetation heterogeneity should not be underestimated in devising strategies for mitigating wildfire risks. In this case, we aim to explore the influence of other factors, including topography, varied wind directions, and diverse scenarios within Sete Cidades National Park. This study serves as a foundation for further exploration, encouraging interdisciplinary efforts that integrate ecological understanding, computational modeling, and conservation practices to foster more resilient ecosystems in the face of escalating wildfire challenges.
Use of AI tools declaration
The authors declare that they have not used artificial intelligence tools in the creation of this article.
Acknowledgments
The authors wish to extend their gratitude to the funding agencies that supported this research. DAL acknowledges the financial assistance from CNPq (National Council for Scientific and Technological Development), Grant Number Chamada/CNPq/MCTIC/FNDCT 18/2021-423105/2021-3. HCB acknowledges the support from FAPEMIG (Minas Gerais State Research Foundation), Grant Number Edital 04/2022-PIBIC. Additionally, DAL and HCB express their appreciation to the Federal Institute of Triangulo Mineiro (IFTM), Campus Patrocinio, for permitting the research to proceed during the COVID-19 pandemic and for providing invaluable support to the authors. Their contributions were instrumental to the success of this study.
Conflict of interest
The authors declare that there is no conflict of interest.









 DownLoad:
DownLoad: