1.
Introduction
A conic bundle is a proper flat morphism π:X→Z of non-singular varieties such that it is of relative dimension 1 and the anticanonical divisor −KX is relatively ample. We say that a variety X has a conic bundle structure if there exists a conic bundle π:X′→Z and a birational map X⇢X′.
Varieties with conic bundle structure play a very important role in the birational classification of algebraic varieties of negative Kodaira dimension. For example, any variety with rational curve fibration has a conic bundle structure [1]. For these varieties there are well-developed techniques to solve rationality problems [1,2,3,4].
Another important class of varieties of negative Kodaira dimension is the class of Q-Fano varieties. Recall that a projective variety X is called Q-Fano if it has only terminal Q-factorial singularities, the Picard number ρ(X) equals 1, and the anticanonical class −KX is ample. In fact, these two classes overlap. Moreover, Q-Fano varieties with conic bundle structures form a large subclass in the class of all Q-Fano varieties. It is very important for birational geometry to investigate and describe those Q-Fano varieties that do not have conic bundle structures. This paper is an attempt to do it in dimension three.
To formulate the main result we have to recall some definitions. A normal n-dimensional variety X with only canonical Gorenstein singularities is called a del Pezzo variety if there exists an ample Cartier divisor A such that −KX=(n−1)A (see [5]). Then the intersection number d(X):=An is called the degree of X. We consider only those del Pezzo varieties that have at worst terminal singularities. Del Pezzo varieties of degree ≤2 have special names:
(i) a del Pezzo variety X of degree 1 is called double Veronese cone;
(ii) a del Pezzo variety of degree 2 is called quartic double solid.
Recall that the Fano index qQ(X) of a Q-Fano variety is the maximal integer that divides the canonical class KX in the torsion free group Cl(X)/Tors (see 2.4). The following fact was proved in [6]:
1.1. Theorem
Let X be a Q-Fano threefold with qQ(X)>7.Then X is rational. In particular, X has a lot of conic bundle structures.
For a normal Q-factorial projective variety X, denote
Our main result is the following
1.2. Theorem
Let X be a Q-Fano threefold with qQ(X)>1.Assume that X has no conic bundle structures.Then we have.
(i) df(X)≤3.
(ii) If df(X)=3, then X is a smooth quartic double solid.
(iii) If df(X)=2, then there exists a birational transformation Ψ:X⇢X′ such that one of the following holds:
● X′ is a smooth quartic double solid,
● X′ is a smooth double Veronese cone and Ψ is an isomorphism, or
● X′ is a double Veronese cone with terminal Q-factorial singularities and X′ is singular.
Note that a smooth double Veronese cone has no conic bundle structures according to [7]. Existence of conic bundle structures on smooth quartic double solids and singular double Veronese cones is not known.
2.
Preliminaries
2.1. Notation and terminology
In this paper we always assume that the ground field k is algebraically closed and of characteristic zero. Also, we use the standard notations and definitions of the Minimal Model Program (MMP). When we say that a variety X has terminal (resp. canonical) singularities, this means that the singularities are not worse than that, in particular, X can be smooth.
For a variety X with terminal singularities B(X) denotes the basket of its singularities (see [8]). Typically, when describing a basket we list only indices of singularities. For example, B(X)=(r1,r2) means that B(X) contains two points of types 1r1(1,−1,b1) and 1r2(2,−2,b2). For any normal variety X, Cl(X) denotes the group of Weil divisors on X modulo linear equivalence. By Cl(X)T we denote the torsion subgroup of Cl(X). If M is a linear system, then Bs(M) denotes its base locus.
2.2. Singularities of linear systems
2.2.1. Definition
Let X be a normal variety and let M be a (not necessarily complete) linear system of Weil divisors. We assume that dim(M)>0 and M has no fixed components. Let μ be non-negative rational number such that KX+μM is Q-Cartier. For a birational morphism f:˜X→X, write
where ˜M:=f−1∗M is the proper transform of M on ˜X, Ei are prime exceptional divisors, and ei are rational numbers called discrepancies. Note that in this formula discrepancies are defined with respect to μM, where M∈M is a general member [9,Sect. 4]. We say that the pair (X,μM) canonical, resp. terminal if ei≥0 (resp. ei>0) for all i and for all birational morphisms f:˜X→X.
2.2.2. Lemma
(see [10], [9], [11]). Let (X,M) be a pair, where X is a threefold with at worst terminal singularities and M is a movable linear system on X.
(i) If (X,M) is canonical, then a general member S∈M has only Du Val singularities and in a neighborhood of each point P∈Bs(M) we have M∼−KX,
(ii) If (X,M) is terminal, then a general member S∈M is a smooth surface contained in the smooth locus of X, and dimBs(M)≤0.
2.2.3. Theorem
(see [10], [11]).Let X be a variety with terminal Q-factorial singularities and let M be a linear system on X without fixed components such that the pair (X,μM) is canonical for some μ≥0.Let
be a step of KX+μM-MMP, that is, φ is either a KX+μM-negative extremal divisorial contraction or a KX+μM-flip.Then the variety X′ again has terminal Q-factorial singularitiesand the pair (X′,μM′) is canonical.If moreover (X,μM) is terminal, then so is (X′,μM′).
2.2.4. Corollary
(see [10], [12], [11]). Let X be a normal variety and let M be a linear system on X without fixed components such that KX+μM is Q-Cartier for some μ≥0.Then there exists a projective birational morphism
where ˜M:=f−1∗M, such that the pair (˜X,μ˜M) is terminal, K˜X+μ˜M is f-nef, and ˜X is Q-factorial. Moreover,
where Ei are prime exceptional divisors and ei≥0 for all i.
Such a pair (˜X,μ˜M) is called terminal Q-factorial model of (X,μM).
2.2.5. Corollary
(see [13], [10], [11]). Let X be a variety with terminal Q-factorial singularities and let M be a linear system on X without fixed components such that the pair (X,μM) is canonical for some μ≥0.Then there exists a projective birational morphism
where ˜M:=f−1∗M, such that ˜X is terminal Q-factorial, the pair (˜X,μ˜M) is canonical,
and the exceptional locus of f is a prime divisor.
Such a morphism is called an extremal log crepant blowup of (X,μM).
2.2.6. Corollary
(see [12]). Let X be a normal projective variety and let M be a linear system on X without fixed components such that KX+μM is canonical for some μ≥0.Assume that KX+μM is not pseudo-effective.Then one can run KX+μM-MMP and end with a Mori fiber space.
Recall also the following well-known result.
2.2.7. Theorem
(see [14]). Let (X∋P) be a terminal quotient singularity of type1r(1,a,r−a), let f:˜X→X be a divisorial Mori contraction, and let E be the exceptional divisor.Then f(E)=P, the contraction f is a weighted blowup with weights (1,a,r−a), and the discrepancy of E equals a(E,X)=1/r.
2.3. Del Pezzo varieties
2.3.1. Proposition
(see [5], [15]).Let X be a three-dimensional del Pezzo variety with terminal singularities.Then
and a general member M∈|−12KX| is a smooth del Pezzo surface of degree d(X). Moreover, the pair (X,|−12KX|) is terminal.
2.3.2. Theorem
(see [16], [5], [15]). Let X be a three-dimensional del Pezzo variety with terminal singularities.
(i) If d(X)=1 (i.e., X is a double Veronese cone), then X can be realized as a hypersurface of degree 6 in the weighted projective space P(13,2,3). In this case, the projection
is a double cover whose branch divisor has degree 6 in P(13,2).
(ii) If d(X)=2 (i.e., X is a quartic double solid), then X can be realized as a hypersurface of degree 4 in the weighted projective space P(14,2). In this case, the projection
is a double cover whose branch divisor is a quartic hypersurface.
2.4. Q-Fano varieties
Now, let X be a Q-Fano variety. It follows from the definition that Cl(X) is a finitely generated abelian group of rank 1. The numbers
are called the Fano index and Fano-Weil index, respectively. Clearly, qW(X) divides qQ(X) and qQ(X)=qW(X) if Cl(X) is torsion free. For a Q-Fano variety X throughout this paper A will denote a Weil divisor on X such that −KX∼QqQ(X)A. If qQ(X)=qW(X), we take A so that −KX∼qQ(X)A. Since ρ(X)=1 and X is Q-factorial, for any Weil divisor M on X we can write −KX∼QλM. In this situation denote
Thus qQ(X)=λ(X,A).
2.4.1. Theorem
(see [17]). Let X be a Q-Fano threefold and let M be a smooth surface on Xwith ϰ(M)=−∞.Assume that X is not rational. Then one of the following holds:
(i) X is a hypersurface of degree 6 in P(12,22,3), M is a member of the linear system |OX(2)|=|−23KX|, and so λ(X,M)=3/2,
(ii) X is a del Pezzo threefold of degree d(X)≤3, M is a member of the linear system |−12KX|, and λ(X,M)=2.
Note that in [17] the surface M is supposed to have ample normal bundle. This is automatically satisfied in our case because ρ(X)=1 by our Q-Fano assumption.
2.4.2. Corollary
Let X be a Q-Fano threefold and let M be a linear system without fixed components on X such that λ(X,M)>1 and the pair (X,M) is terminal.Assume that X has no conic bundle structures.Then one of the following holds:
(i) X=X6⊂P(13,2,3) is a double Veronese cone,
(ii) X=X4⊂P(14,2) is a smooth quartic double solid.
Proof. By Lemma 2.2.2(ii) a general member M∈M is smooth and is contained in the smooth locus of X. By the adjunction formula M is a del Pezzo surface. In particular, M is rational. Thus we can apply Theorem 2.4.1.
Assume that X is such as in 2.4.1(i). Let ψ(x1,x′1,x2,x′2,x3)=0 be an equation of X=X6⊂P(12,22,3), where the subscript index of the variables xi and x′j is its degree. Since the singularities of X are terminal, ψ contains the term x23. Thus ψ can be written in the form
where γ3 is a homogeneous polynomial of degree 3 and ϕ does not contain cubic terms in x2,x′2. Clearly, γ3≠0 (otherwise X would be singular along the line {x1=x′1=x3=0}). Thus after a linear coordinate change we may assume that γ3=x2x′2(x2+x′2), x2x′22, or x′32. Then the projection
is a rational curve fibration because in the affine chart x′2=1 the equation ψ becomes quadratic in x3,x2. Hence X has a conic bundle structure in this case.
Thus we may assume that X is a del Pezzo threefold of degree d(X)≤3 (see 2.4.1(ii)). If d(X)=3, then X=X3⊂P4 is a cubic with terminal singularities and M is its smooth hyperplane section. In this case the projection X⇢P2 from a line l⊂X is a rational curve fibration.
It remains to consider the case d(X)=2, i.e., the case where X=X4⊂P(14,2) is a quartic double solid. Suppose that X has a singular point, say P∈X. Let ψ(x0,x1,x2,x3,y)=0 be an equation of X=X4⊂P(14,2), where deg(xi)=1 and deg(y)=2. Since the singularities of X are terminal Gorenstein, ψ contains the term y2. By an obvious coordinate change we may assume that ψ has the form
where deg(ϕ)=4. Clearly, P is contained in the hyperplane {y=0}. Hence, by a linear coordinate change we may assume that P=(1,0,0,0,0) and so
where deg(ϕi)=i. As above, the projection
is a rational curve fibration. Hence X has a conic bundle structure.
3.
Construction
We recall the construction used in the papers [18,19,20,21].
3.1. Assumptions
Let X be a Q-Fano threefold.Consider a linear system M on X without fixed components.Let c:=ct(X,M) be the canonical threshold of the pair (X,M) [13].Assume that λ(X,M)>c.
3.1.1. Lemma
(see [19,Lemma 4.2]). Let P∈X be a point of index r>1. Assume thatM∼−tKX near P, where 0<t<r. Then ct(X,M)≤1/t and β≥tα.
Consider an extremal log crepant blowup f:˜X→X with respect to KX+cM (Corollary 2.2.5). Let E be the exceptional divisor. Recall that ˜X has only terminal Q-factorial singularities. We can write
where α,β∈Q>0. Then c=α/β and
Since λ(X,M)>c, the divisor −(K˜X+c˜M) is nef. Take λ=λ(X,M). Then
Put δ:=α(λ/c−1). Then
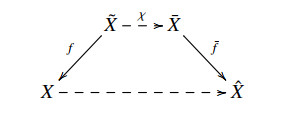
Run the MMP with respect to K˜X+c˜M. We obtain the following diagram (Sarkisov link)
Here the varieties ˜X and ˉX have only terminal Q-factorial singularities, χ is a composition of K˜X+c˜M-log flips, ρ(˜X)=ρ(ˉX)=2, and ˉf:ˉX→ˆX is an extremal KˉX-negative Mori contraction. In particular, rkCl(ˆX)=1.
In what follows, for a divisor (or a linear system) N on X by ˜N and ˉN we denote proper transforms of N on ˜X and ˉX respectively. If ˉf is birational, then ˆN denotes the proper transform of N on ˆX. Apply χ∗ to (3.1.3):
3.1.6. Lemma
(cf. [6,Lemma 4.7]). If M is not composed of a pencil and ˉf is not birational, then X has a conic bundle structure.
Proof. If dim(ˆX)=2, then ˉf is a Q-conic bundle and we are done. Assume that dim(ˆX)=1. Then ˉf is a del Pezzo fibration and ˆX≃P1. By our assumption dim(M)≥2 and a general member of M is irreducible. Hence M is not ˉf-horizontal, i.e., M is not a pull-back of a linear system on ˆX. Since the divisor ˉE is not movable, it is not contained in fibers and so ˉE is ˉf-ample. For a general fiber F by the adjunction formula and (3.1.5) we have
where both ˉM|F and ˉE|F are integral ample divisors. Since λ>1, the surface F is isomorphic either P2 or P1×P1. In this case, ˉX is rational and so it has a lot of conic bundle structures.
3.2. Birational contractions
Assume that the contraction ˉf is birational. Then ˆX is a Q-Fano threefold. Denote ˆq:=qQ(ˆX).
3.2.1. Lemma
If the contraction ˉf is birational, then the divisor ˉE is not contracted by ˉf.
Proof. Indeed, otherwise the map ˉf∘χ∘f−1:X⇢ˆX would be an isomorphism in codimension one. Hence it is an isomorphism. On the other hand, the number of KˆX+cˆM-crepant divisors on ˆX is strictly less than the number of KX+cM-crepant divisors on X, a contraction.
It follows from (3.1.5) that
Therefore,
3.3. Linear systems
Let Nk be a non-empty linear system on X such that qNk∼k(−KX) (here we allow Nk have fixed components). Let Θ be an ample Weil divisor on ˆX generating Cl(ˆX)/Cl(ˆX)T. We can write
where e∈Z>0 by Lemma 3.2.1 and sk∈Z≥0. Thus sk=0 if and only if dim(Nk)=0 and a unique element ˉN of ˉNk is ˉf-exceptional. As in (3.1), we write
The relations (3.1.2) and (3.3.1) give us
where qβk−kα is an integer. From this we obtain
3.4. Dimensions of linear systems via computer search
We need certain information on linear systems on Q-Fano threefolds of large Fano index. Most of these facts are contained in the Graded Ring Database [22] or can be obtained by direct computations using algorithms described in [6,19,23,24].
3.4.1. Proposition
Let X be a Q-Fano threefold and let M be a Weil divisor on X such that dim|M|≥2 and λ(X,M)>1.Then the linear system |M| is not composed of a pencil.
Proof. Assume the contrary, that is, |M|=D+m|L|, where D is the fixed part of |M| and |L| is a pencil without fixed components. Then dim|M|=m≥2. Replacing |M| with m|L| we may assume that D=0. Clearly, dim|2L|=2 and qQ(X)≥3 in our case. Now, running computer search for Q-Fano threefolds with dim|L|=1 and dim|2L|=2, we get a contradiction. Interested readers can find a simple PARI/GP code [25] on the author's webpage https://homepage.mi-ras.ru/ prokhoro/programs/pencil.gp.
As above, using computer search one can find that there are 30 possible Hilbert series of Q-Fano threefolds such that qQ(X)≥3, Cl(X)T=0, and df(X)=3. As a consequence of this list one obtains.
3.4.2. Proposition
Let X be a Q-Fano threefold with qQ(X)≥3, Cl(X)≃Z and df(X)=3. Then the following assertions hold.
(i) qQ(X)≤7, qQ(X)≠6, and the basket B(X) contains at most one point of index ≥8.
(ii) If qQ(X)=7, then |A|=∅ and dim|2A|=dim|3A|=0.
(iii) If qQ(X)=5, then dim|2A|=0 and dim|3A|=1.
(iv) If qQ(X)=4, then dim|A|≤0 and 1≤dim|2A|≤2.If moreover, dim|2A|=2, then dim|A|=0, A3=2/11 and B(X)=(11).
Similarly, we have.
3.4.3. Proposition
Let X be a Q-Fano threefold with qQ(X)≥3, df(X)=3 and Cl(X)T≃Z/nZ, where n>1.Let T be a generator of Cl(X)T. Then the following assertions hold.
(i) qQ(X)≤5,
(ii) If qQ(X)=5, then qQ(X)=5, n=2, B=(42,12), A3=1/12. Moreover, dim|A|=0, |A+T|=∅, dim|2A|=dim|2A+T|=0, dim|3A|=dim|3A+T|=1.
(iii) Assume that qQ(X)=4 and df(X)=3. Then n∈{2,5} and for a suitable choice of A we have |A|=∅, dim|2A|=1, and dim|3A+kT|=3 for any k. Moreover,
(a) if n=2, then dim|A+T|=0 and 0≤dim|2A+T|≤1;
(b) if n=5, then dim|A+kT|=0 and dim|2A+kT|=1 for k≢0mod5.
(iv) Assume that qQ(X)=3 and df(X)=3. Then n∈{2,3} and |A+Λ|≠∅ for anyΛ∈Cl(X)T. Moreover, if n=2 and dim|2A|<3, then A3=15/28 and B=(2,4,14), and |A|≠∅.
4.
Proof of Theorem 1.2
4.1. Assumptions
Let X be a Q-Fano threefold with qQ(X)>1 and df(X)≥2 and let M be a linear system without fixed components such that dim(M)=df(X) and λ(X,M)>1.Assume that X has no conic bundle structures.
4.1. Claim
There exists a sequence of links of the form (3.1.4)
whereeach X(i) is a Q-Fano threefold,
for M(i+1)=Ψi∗M(i), and the pair (X′,M′=M(n)) is terminal.
Proof. If the pair (X,M) is terminal, we are done. Thus we may assume that c:=ct(X,M)≤1. By Proposition 3.4.1 the linear system M is not composed of a pencil. Apply the construction (3.1.4) to (X,M). By Lemma 3.1.6 the contraction ˉf is birational and so we obtain a new pair (ˆX,ˆM), where ˆX is a Q-Fano threefold and ˆM:=Ψ∗M is a linear system without fixed components such that λ(ˆX,ˆM)>λ(X,M) (see (3.2.2)). If the pair (ˆX,ˆM) is terminal, we are done. Otherwise we can repeat the process applying the construction (3.1.4) to (ˆX,ˆM) and continue. We get a sequence of pairs
Since the set of all Q-Fano threefolds is bounded [29], the process terminates with a pair (X′,M′)=(X(n),M(n)) having terminal singularities.
By Corollary 2.4.2 X′ is a del Pezzo threefold of degree d(X′)≤2 and M′=Ψ∗M⊂|−12KX′|. In particular, dim(M)=dim(M′)≤3. This proves 1.2(i).
If X′=X′6⊂P(13,2,3) is a double Veronese cone, then dim(M)=dim(M′)=2. If, furthermore, X′ is smooth, then according to [7], any birational model of X′ which is a Mori fiber space non-isomorphic to X′ is a degree 1 del Pezzo fibration over P1. In particular, this implies that X≃X′. If X′ is a quartic double solid, then it must be smooth (see Corollary 2.4.2). This proves 1.2(iii).
It remains to prove the assertion of 1.2(ii), i.e., Ψ is an isomorphism in the case dim(M)=3. For this purpose we can consider the last step of (4.1.2). We show that X(n)≃X(n−1).
4.2. Assumptions
Thus we assume that dim(M)=3. We put X=X(n−1) and ˆX=X(n).Recall that ˆX is a smooth quartic double solid in our case. We are going to use all the notation of Sect. 3.Since (X,M) is not terminal, c=ct(X,M)≤1.Recall also the commutative diagram (3.1.4):
Denote by ˉF the ˉf-exceptional divisor and by ˜F⊂˜X its proper transform. By Lemma 3.2.1 ˉF≠ˉE. Hence F:=f(˜F) is a prime divisor on X. Write
Thus λ:=λ(X,M)=q/b>1. Clearly,
where a is the discrepancy of ˉF. Since ˆX is smooth, a is a positive integer.
4.2.2. Remark
● If ˉf(ˉF) is a point, then by [26] ˉf is a weighted blowup of ˉf(ˉF)∈ˆX with weights (1,w1,w2), where gcd(w1,w2)=1. In particular, a=w1+w2≥2. Moreover, a=2 if and only if ˉf is a usual blowup.
● If ˉf(ˉF) is a curve, then the contraction ˉf is the usual blowup at its general point. In particular, a=1.
4.2.3. Claim
2b=q+ad. In particular, q<2b.
Proof. Since Bs(ˆM)=∅, we have
Taking the pushforward of this relation to X, we get the desired equality.
4.2.4. Claim
gcd(b,d)=1 and Cl(X)T is a cyclic group of order e/d.
Proof. The group Cl(ˉX) is generated by the classes of ˉM and ˉE:
Hence, Cl(˜X)=Z⋅[˜M]⊕Z⋅[˜F] and we can write
Since E is contracted by f, we have Cl(X)=Cl(˜X)/Z⋅[E] and so Cl(X)=Z⊕Z/nZ, where n=gcd(u,v). Taking pushforward of (4.2.5) to X and ˆX we get ub+vd=0 and e=u, respectively. Since the classes of M and F generate Cl(X), we have gcd(b,d)=1. Hence, v=−nb and e=nd.
4.2.6. Claim
Assume that M∋tN, where N is an (effective) Weil divisor and t≥2.Then e=d=1, Cl(X) is torsion free, Supp(N)=F, ˉf(ˉF) is a point, and a≥2.
Proof. We can write ˜M∼t˜N+δE, where δ is an non-negative integer. Hence, ˆM∼tˉf∗ˉN+δˆE. Since the class of ˆM is not divisible in Cl(ˆX), we have Supp(ˉN)=ˉF and so δ=1 and ˆE∼M, i.e., e=1. By Claim 4.2.4 d=1 and Cl(X) is torsion free. Further,
where t≥2. If ˉf(ˉF) is a curve, then ˆE must be singular along ˉf(ˉF). This contradicts [27]. Hence ˉf(ˉF) is a point and a≥2 by Remark Remark 4.2.2.
4.2.7. Claim
Assume that M∋N1+N2, where N1 and N2 are effective non-zero Weil divisors without common components. If F≠Supp(N1), then F=Supp(N2)and N1 is a prime divisor.
Proof. As above, write ˜M∼˜N1+˜N2+δE, where δ≥0. Hence, ˆM∼ˉf∗ˉN1+ˉf∗ˉN2+δˆE. By our assumption f∗ˉN1≠0. Since the class of ˆM is not divisible in Cl(ˆX), we have δ=0, ˉf∗ˉN2=0, and ˉN1 is a prime divisor.
4.2.8. Claim
If a>1, then M∼N+δF, where δ is a positive integer and N is an effective Weil divisor such that dim|N|≥2.
Proof. By Remark Remark 4.2.2 ˉf(ˉF) is a point, say ˆP. Let ˆMP⊂ˆM be the linear subsystem consisting of all divisors from ˆM passing through ˆP. Then dim(ˆMP)=2 and
where δ≥1. Therefore, M∼MP+δF, where dim(MP)=2.
Now we are in position to prove Theorem 1.2(ii). We show that in our assumptions 4.2 the diagram 4.3 does not exist. Consider possibilities for ˆX case by case.
4.3. Case Cl(X)≃Z
Then e=d by Claim 4.2.4. Apply Proposition 3.4.2. We obtain the following subcases.
Subcase qQ(X)=7
Then by 3.4.2(ii) there are prime divisors M2 and M3 such that M∼3M2∼2M3. But then by Claim 4.2.6 we have M2=M3=F, a contradiction.
Subcase qQ(X)=5
Then dim|2A|=0 and dim|3A|=1 by 3.4.2(iii). Hence, b=4 and M∋2D, where D∈|2A|. By Claim 4.2.6 we have Supp(D)=F, d=1 and a≥2. Then by Claim 4.2.8 we have dim|kA|≥2, where k≤3, a contradiction.
Subcase qQ(X)=4.
It follows from Claim 4.2.3 that b=3 and ad=2. Thus M∼3A and s3=1. According to Proposition 3.4.2(iv) we have dim|A|≤0 and dim|2A|≥1. The relation (3.3.2) for k=3 has the form
where β3≥2α by Lemma 3.1.1. Hence α≤2/(5e)<1 and so P:=f(E) is a non-Gorenstein point of X. Let r be its index. Similarly, the relation (3.3.2) for k=2 has the form
Since dim|2A|>0, a general member of M2=|2A| is not contracted on ˆX, hence s2≥1. Therefore, β2≤α/2. Since rM2 is Cartier at P, rβ2 is an integer. So, α≥2/r. Hence f cannot be a Kawamata blowup of P∈X and P∈X is not a cyclic quotient singularity (see Theorem 2.2.7). By Proposition 3.4.2(i) we have r≥7. Then
By Claim 4.2.4 d=1, a=2, and dim|2A|≥2 by Claim 4.2.8. Then by 3.4.2(iv) we have only one possibility: A3=2/11 and B(X)=(11). But this means that P∈X is a cyclic quotient singularity, a contradiction.
Subcase qQ(X)=3
Then b=2 and d=a=1 by Claim 4.2.3. Hence, M∼2F because Cl(X)≃Z. Then we get a contradiction by Claim 4.2.6.
4.4. Case: Cl(X)T≠0
Let n be the order of Cl(X)T. Apply Proposition 3.4.3 and consider possibilities for qQ(X).
Subcase qQ(X)=5.
Then n=2. By 3.4.3(ii) we have
In particular, b=4. If a>1, then by Claim 4.2.8 we get a contradiction. Thus a=1 and d=3 (see Claim 4.2.3). If M=|4A|, then we get a contradiction by Claim 4.2.6 because |A|≠∅ (see 3.4.3(ii)). Let M=|4A+T|. Then M∼2N1+N2, where N1∈|A| and N2∈|2A+T| are prime divisors. By Claim 4.2.7 we have F=N1 and so d=1, a contradiction.
Subcase qQ(X)=4
Then n=2 or 5. It follows from Claim 4.2.3 that b=3 and ad=2. If a>1, we get a contradiction by Claim 4.2.8 and 3.4.3(iii). Thus, a=1 and d=2. By 3.4.3(iii) |A+T|≠∅ and dim|3A+3T|=3. Take M=|3A+3T| and apply the construction (3.3). Then by Claim 4.2.6 ˆX is not a smooth quartic double solid because M∋3N, N∈|A+T|. By (3.2.2) we have λ(ˆX,ˆM)>λ(X,M)=4/3. Therefore, qQ(ˆX)>4. Hence we can proceed with the sequence of Sarkisov links (4.1.2) so that λ(X(i),M(i))>4/3 and qQ(X(i))>4. By the above considered cases we get a contradiction.
Subcase qQ(X)=3.
It follows from Claim 4.2.3 that b=2, a=d=1. Hence, M∼Q2F. If M∼2F, we get a contradiction by Claim 4.2.6. Therefore, T:=M−2F is a non-trivial torsion element. We have KˉX+2ˉM−ˉF∼0. Hence −KX∼2M−F∼3F+2T. Moreover, the group Cl(X) is generated by the classes of F and T. By Claim 4.2.4 ˆE≁ˆM because d=1.
Assume that 3T∼0. Then M∼2(F+2T) and |F+2T|=∅ by Claim 4.2.6. This contradicts 3.4.3(iv). Therefore, 2T∼0. Hence, −KX∼3F. If dim|2F|=3, then we take M=|2F| and apply the construction (3.1.4). Then by Claim 4.2.6 ˆX is not a smooth quartic double solid. By (3.2.2) we have λ(ˆX,ˆM)>λ(X,M)=3/2. Therefore, qQ(ˆX)>3. Hence we can proceed with the sequence of Sarkisov links (4.1.2) so that λ(X(i),M(i))>3/2 and qQ(X(i))>3. By the above considered cases we get a contradiction.
Therefore, dim|2F|<3. Thus by 3.4.3(iv) we have B=(2,4,14). Then |F+T|≠∅ and for D∈|F+T| we have −KX∼M+D,
where γ≥β−α=α(c−1)≥0. Since D≠F, taking pushforward to ˆX we obtain −KˆX∼ˆM+ˆD+γˆE, where ˆD≠0. This is possible only if γ=0. Then the pair (X,M) is canonical. In this case by Lemma 2.2.2(i) near each singular point either D or M is Cartier. Since −4KX∼12D∼6M, the divisor 4KX is Cartier. This contradicts B=(2,4,14).
5.
Applications
In this section we consider applications of Theorem 1.2 to the existence of conic bundle structures on rationally connected threefolds.
5.1. Proposition
Let X be a normal projective threefold and let M be a non-empty linear system of Weil divisors on X such that
(i) M is not composed of a pencil,
(ii) −(KX+M)∼QΘ, where Θ is a Q-divisor which is Q-Cartier, and
(iii) either Θ>0 or Θ=0 and the pair (X,M) is not canonical.
Assume that the variety X has no conic bundle structures.Then there exists a birational transformation Ψ:X⇢X′, whereX′ is del Pezzo threefold as in 1.2(iii). Moreover,
Proof. We may assume that M has no fixed components. Let
be a terminal Q-factorial model of the pair (X,M) (see Corollary 2.2.4). Thus ˜M:=f−1∗M, the pair (˜X,˜M) is terminal, K˜X+˜M is f-nef, and the variety ˜X is Q-factorial. We can write
where E′ is the exceptional Q-divisor and E′≥0. Furthermore,
where is the proper transform of on and . Hence,
where . Moreover, by our assumptions 5.1(iii). In particular, is not nef. Run -MMP. The divisor cannot be contracted, so at the end we get a Mori fiber space :
where if -ample. If , then is a -conic bundle and then we are done. Assume that . Then is a del Pezzo fibration and . Since and a general member of is irreducible, is not -horizontal, i.e., is not a pull-back of a linear system on . For a general fiber by the adjunction formula we have
where both and are ample. Since is an (integral) Cartier divisor, the surface is either or . In this case, is rational and so it has a lot of conic bundle structures. Finally, assume that is a point. Then is a -Fano threefold such that and
where both and are ample, and . Thus we can apply Theorem 1.2 to .
Now we consider applications of Theorem 1.2 to -Fano threefolds. For simplicity we consider only -Fanos whose Weil divisor class group has no torsions, i.e., . The collection of invariants determines the Hilbert series
An abstract collection , where is a basket of terminal singularities, , and is called a numerical candidate if there are no numerical obstructions (like orbifold Riemann-Roch theorem [8] and Bogomolov-Miyaoka inequality [14]) for existence of a -Fano threefold with corresponding invariants.
Computer search by using algorithm described in [6,19,23,24] (see also [22]) shows that there are at most 472 numerical candidates with . Among them Theorem 1.2 is applicable in 313 cases. Similarly, there are at most 1 492 numerical candidates with and Theorem 1.2 is applicable in 382 cases. We expect that in most of the remaining cases the corresponding -Fano threefolds (if they exist) should have conic bundle structure. However proof of this needs a case by case considerations. Let us consider just one example.
5.2. Proposition
Let be a -Fano threefold with and (No. in [22]).Then is rational. In particular, has a conic bundle structure.
Note however that the existence of a -Fano threefold with these invariants is not known.
Proof. In this case the group is torsion free, , , and for . Apply construction (3.1.4) with . Then near the point of index we have . Hence, by Lemma 3.1.1 and so
The relation (3.2.2) for has the form
Since , we have and . Thus is the Kawamata blowup of a cyclic quotient singularity of index , or . If or , then near . Hence , where is an integer, . Then (5.2.1) gives us
This contradicts our assumptions. Thus and so near . Hence , where is a non-negative integer. Again, from (5.2.1) we obtain
Thus , , and . By Theorem 2.2.7 . Now, the relation (3.3.2) for has the form:
Since , . Since , this implies that the contraction is not birational, so it is a del Pezzo fibration. Since , we have and so is a fiber of multiplicity . By the main result of [28] the general fiber of is a del Pezzo surface of degree . But then must be rational, a contradiction.
Acknowledgments
The paper was partially supported by the HSE University Basic Research Program. The author would like to thank the referees for useful comments and suggestions.
Conflict of interest
The author declares there is no conflicts of interest.










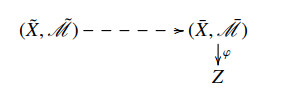



 DownLoad:
DownLoad: