Growing items inventory model for carbon emission under the permissible delay in payment with partially backlogging

1.   Introduction

Nowadays, the business of livestock farming is increasing rapidly. Once a farm animal enters the system, it is fed and allowed to grow to a maturity stage which leads to the increment in the weight of the animal and it is described as the growing inventory. The level of inventory keeps increasing until the time of slaughter which is the endpoint of the breeding period. It is also seen that the excessive growth of the animal also decreases the quality of the meat (Polidori, et al., 2017) which is needed to be controlled. Even pre-slaughter handling is as important as breeding to enhance the quality of the product (Składanowska-Baryza & Stanisz, 2019). Later, the period of consumption begins after slaughter items start to decrease due to demand and deterioration.

Deterioration of the growing inventory can be understood as the decay of the slaughter items that are stored to meet the demands of the customer. The rate of the deterioration may depend on the item, the storage facilities or on the length of the consumption period, etc. The concept has been widely used by researchers in the retail industries. Still, no new developments have yet been found in this area.

Although livestock farming is turned out to be a major source of GHG emission (Tullo, 2019), it is becoming a challenge for the government as well as the industry to reduce emissions, the main ground of these emissions is surfaced from the energy used to drive the slaughterhouse and from the degradation of the wastewater in the process of slaughtering. Wastewater contains many organic materials and when they decompose, they emit a large number of greenhouse gases.

Nowadays, the execution of cost management is going through modernization, rapid technological changes, and excessive cutthroat competitions. Inflation is essential to allow during the costing analysis as it can affect the whole replenishment policies. Incorporation of inflation during the analysis can provide accurate forecasting of the imminent cost of the system. In the present era, practitioners are supposed to give some credit to their customers to survive in the market. Delay in payment is the credit that is generally offered by the suppliers to motivate the retailers to buy more.

In the presented article, an economic order quantity (i.e. EOQ is a production formula used to determines the most efficient amount of goods that should be purchased based on ordering and carrying costs) inventory model has been studied for the growing items. Newborn animals are ordered and fed until they grow to the consumer-preferred weight and then slaughtered to meet the demand. It is assumed that the retailer is been offered the trade credit and clears the payment after completing the replenishment cycle. Here, the replenishment cycle is divided into two portions; breeding period and consumption period. During the breeding period, the level of inventory keeps increasing until the time of slaughter, which is the endpoint of the breeding period. Quality control is taken into account to manage the quality loss and pre-slaughter handling is also considered to enhance to quality of the item. Later, the period of consumption begins and the slaughter items are eliminated to zero due to the demand and deterioration. Shortages are allowed and fully backlogged.

The rest of the article is constructed as follows: section 2 provides a literature survey to explain the background of the work, to indicate the related gaps, and also to elucidate the contributions of the paper. Notation and assumptions are provided in section 3. Section 4 describes the mathematical formulation of the model. Section 5 is mentioned for the numerical verification of the formulated results. Sensitivity analysis and managerial insights are also shown in this section. Finally, the conclusion with the future research expansions is given in section 6.

2.   Literature review

In the last decades, only a few researchers investigated the area inventory problems of growing items under carbon-constrained. Hua et al. (2011) studied reduced carbon emissions to allay global warming. A numerical demonstration of this model was provided to examine the impacts of carbon constraints on a minimum total cost. Hu and Zhou (2014) examined the manufacture's joint carbon emission reduction and pricing policy under a carbon emission trade. They investigated the impact of a carbon emission policy to find the maximum profit. Saxena et al. (2017) derived a vendor buyer closed-loop supply chain model with the reverse channel of used products. In this model, the buyer was offered the credit in payment and it has been revealed that it is not always profitable to increase the delay period. Taleizadeh et al. (2020) investigated a pricing policy for the inventory model with allowable stock-out. Carbon emission has been considered to deal with environmental issues. The first paper was given by Goliomytis et al. (2003) which describes relative to the research of growing items in the inventory model. Law and Wee (2006) studied a two-echelon inventory model. The manufacturer buys growing goods and picks them up to be used as raw materials in their production system. Both the manufacturer and the retailer are facing declines. The most prevalent weakness is that the ordering policy is inconsistent throughout the series. Dem and Singh (2013) traced the advances in the area by formulating a production model for ameliorating items. In their research, the term amelioration only expresses quality improvement and the production process involves both perfect and imperfect items. Zhang et al. (2016) discussed inventory management problems of growing items under carbon-constrained. Nobil et al. (2018) derived an EOQ model for growing items for a condition with a permissible delay which is fully backordered.

Furthermore, the phenomenon of shortages in an inventory system is a real-life position. In most traditional inventory models, it is assumed that during the stock-out period, the shortage is either fully regained or lost altogether. Singh et al. (2012) have explained an inventory model with rework and flexibility under an allowable shortage. Singh and Rana (2020) derived an effect of inflation and variable holding cost on a lifetime inventory model with multivariable demand and lost sales. Ouyang et al. (2006) developed an inventory model for non-instantaneous deteriorating items where deterioration of the item starts after some time. Singh and Rana (2020) developed an optimal refill policy for new products and take-back quantity of used products with deteriorating items under inflation and lead time. Singh and Sharma (2016) derived a production reliable model for deteriorating products with random demand and inflation. Haley and Higgins (1973) were given the first paper with trade credit in operations management. Since then, the buyer's inventory policy under a given trade credit period has long been concerned. Mahata and De (2016) studied an EOQ framework for items with utility enhancement over time. Turki et al. (2018) have reported and the optimization of the inventory control problem with remanufacturing with carbon cap and trade policy. The amelioration is assumed as the opposite of deterioration and the items do not hold any features of growth. The demand rate is sales-dependent and two-level trade credits are applied to encourage sales. After all, the literature body of the problem is still infantile and underdeveloped. The concept has not been analyzed and a wide and varied variety of unrealistic and simplified assumptions is used to model the problem. This study is undertaken to compensate for some of the inefficiencies. Therefore, in this paper, we shall discuss the effects of carbon emissions on age-dependent breeding, holding costs, deterioration costs, and the total cost for growing items based on the EOQ (Economic Order Quantity) model.

3.   Notations and assumptions

3.1.   Notations

${\mathrm{C}}_{\mathrm{p}}$  Unit purchasing cost

${\mathrm{C}}_{\mathrm{d}}$  Deterioration cost per unit time

${\mathrm{C}}_{\mathrm{b}}$  Breeding (feeding) cost per unit item during the growth period

${\mathrm{C}}_{\mathrm{h}}$  Unit holding cost per unit time during the consumption period

${\mathrm{C}}_{\mathrm{o}}$  Fixed ordering cost per cycle

${\mathrm{w}}_{\mathrm{o}}$  Weight of each newborn item

${\mathrm{w}}_{{\mathrm{t}}_{1}}$  Weight of each grown item at the time${\mathrm{t}}_{1}$ of slaughtering

${\mathrm{w}}_{\mathrm{t}}$  Weight of a unit item at time t

$\lambda$$(.)$  Fraction of items gone useless during the growth period

$\alpha$  quality control parameter

I(t)   Inventory level at time t

$\theta$  Deterioration rate

${\mathrm{w}}_{\mathrm{e}}$  Average of carbon emission cost due to the storage

${\mathrm{d}}_{\mathrm{e}}$  Average of carbon emission cost due to the deterioration

${\mathrm{b}}_{\mathrm{e}}$  Average of carbon emission cost due to the breeding in the growth period

s  Shortage cost per unit

${\mathrm{C}}_{\mathrm{l}}$  lostsale cost per unit

$\mathrm{\delta }$   rate of partially backlogging

$r$   constant rate of inflation per unit time, where $0\le r < 1$

M  the permissible delay in settling account (i.e., the trade credit period),

${\mathrm{I}}_{\mathrm{\tau }}$  the interest charged per dollar in stocks per year by the supplier

${\mathrm{I}}_{\mathrm{e}}$  the interest earned per dollar per year, where ${\mathrm{I}}_{\mathrm{e}}\le {\mathrm{I}}_{\mathrm{\tau }}$.

x  Number of growing items purchased at the beginning of a cycle (unit items)

q  Order quantity (units)

Q  usable items

${\mathrm{t}}_{1}$  Breeding period

${\mathrm{t}}_{2}$  Consumption period

T  Replenishment cycle

TUC  Total unit cost

3.2.   Assumptions

The provided model is structured based on the following assumptions:

2. A linearly time-dependent demand rate $D\left(t\right) = m+nt$; where $m, n >0$; is considered.

4. Shortages are permitted which is partially backlogged.

5. The time-value of money and inflation are considered.

6. The slaughtered items are perceived to be deteriorating in nature and taken to time-dependent.

7. The growth is considered to be time-varying and is formulated as ${w}_{t} = {A(1+a{e}^{-kt})}^{-1}$, where $A$ is the maximum possible weight of the item, a is the integration constant, $k$ is a constant rate that determines the spread of the growth curve.

4.   Mathematical formulation

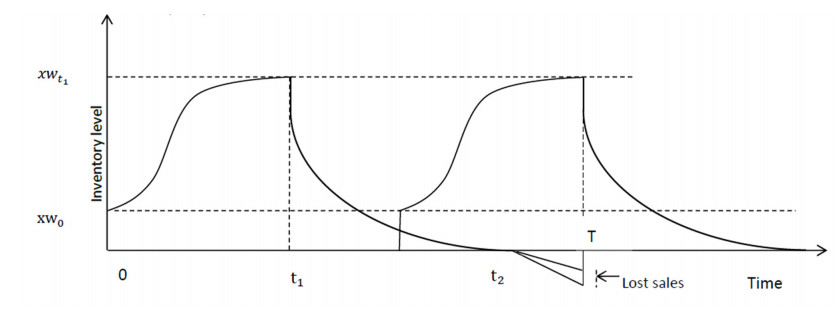
In this model, we have considered to buys newborn animals at the beginning of the cycle. Thereafter breed and raises them. After the breeding period, they are supposed to slaughter. As an example, consider young and fast-growing calves. Enter the inventory system each with a certain number of calves (say $x$) with an initial weight ${\mathrm{w}}_{0}$. Then the initial inventory level is $x{w}_{0}.$ During the breeding period, the items are fed and they grow to a target weight ${\mathrm{w}}_{{\mathrm{t}}_{1}}$ which is a function of time. After reaching the target weight of ${\mathrm{w}}_{{\mathrm{t}}_{1}}$, they are slaughtered. Accordingly, the inventory level rises to $x{w}_{{t}_{1}}$. During the consumption period, the inventory level depletes to zero due to linear demand and deterioration. Figure 1 projects this inventory system.

Suppose $x$ unit items are purchased at zero time. The weight of any newborn is assumed to ${w_0} = A{(1 + a)^{ - 1}}$.

Therefore, the initial inventory level (order quantity) is:

Likewise, at time ${t}_{1}$, the inventory level before inspection (which is shown by ${\rm{I'}}({{\rm{t}}_1})$) yields:

Since x represents the number of newborn animals, it must be considered an integer; which increases the complexity of the model. From Equations (1) and (2), we get

Accordingly, the inventory level at ${\rm{t}} \in [0,{{\rm{t}}_1})$ is

A fraction of the inventory loses its quality during the breeding period which is revealed by quality control of items at point ${\mathrm{t}}_{1}$. Separately, this fraction must be an increasing function of ${t}_{1}$. Moreover, it should hold two other features. Firstly, at time zero, this fraction is negligible(i.e.$\, \lambda (0) = 0)$. Secondly, as the breeding period takes very large values, this approaches one (i.e.${\mkern 1mu} \;{\lim _{{{\rm{t}}_1} - > \infty }}\lambda ({{\rm{t}}_1}) = 1).$The following function holds these features:

Thus the disposal quantity can be expressed as:

Then the inventory level of inspected and useable items is given as:

The above expression provides

During the time interval [${\mathrm{t}}_{1}$, ${\mathrm{t}}_{2}$], inventory level decreases due to the combined effect of demand and deterioration. At the end of period ${\mathrm{t}}_{2}$, the inventory level depletes up to zero. Again, during time interval [${\mathrm{t}}_{2}$, T] shortages start occurring and at T there are maximum shortages, due to partial backordering some sales are lost. The status of the inventory at any instant of time is governed by the following differential equation.

With boundary condition ${\mathrm{I}}_{2}\left({\mathrm{t}}_{2}\right) = 0$ and ${{\rm{I}}_2}({\rm{T}}) = - {\rm{R}}$

The solution of given Equations (8) and (9) are

From Equations (10) & (11), we have

Now, ${I}_{2}\left({t}_{1}\right)-{I}_{2}\left(T\right) = Q$ implies

From Equations (7) & (14), we have

The components of the total cost of the system are outlined as follows:

Pre-slaughter handling is a very essential factor that contributes to them eatable quality. Method, species, breed, and age should be considered during handling the animals before slaughtering. Moreover, the way animals of dealing with the animal during transportation, in the farm, and at the market create lots of stress. Improper handling can cause the death of animals. Therefore, the Pre slaughter handling cost is taken as follows:

where the first term is the constant cost of pre-slaughter handling which does not depend on the number of items while the second expression is the variable cost which depends on the number of items going to be slaughter.

Interest and Depreciation cost: This is $\mathrm{Y}\left({\mathrm{C}}_{\mathrm{o}}, \mathrm{\alpha }\right)$ total cost associated with the supplier's interest and depreciation per production cycle, where $u, b, c\ge 0$

Total expenses for interest rate along with the depreciation$Y\left({C}_{o}, \alpha \right) = v{C}_{o}^{-b}{(1-\alpha)}^{-c}$.

So that $Y\left({C}_{o}, \alpha \right)\to \infty$ as $\alpha \to 1$ to reflect the fact that the loss of quality will never be zero.

Opportunity cost due to the loss of the inventory: It is

where ${\mathrm{C}}_{\mathrm{o}}$ is the fixed ordering cost per cycle, $\lambda$$\left({\mathrm{t}}_{1}\right)$ is the fraction of items gone useless at time ${\mathrm{t}}_{1}$.

Purchasing cost: The cost of inventory, which includes the cost of purchased merchandise, fewer discounts that are taken, plus any duties and transportation costs paid by the purchaser. This cost is PC = $\frac{{\mathrm{C}}_{\mathrm{p}}\mathrm{q}}{\mathrm{T}}$, after substitution, it provides

Breeding cost: The buyer's breeding cost per unit time considering both traditional inventories carrying cost (${\mathrm{C}}_{\mathrm{b}}$) and carbon emission cost (${\mathrm{b}}_{\mathrm{e}}$) due to growing period is:

B(t) is an increasing function of time. There are several functions for B(t) in the literature; polynomial and exponential are the most vastly applied ones (Goliomytis et al., 2003). In this paper, the exponential function $B\left(t\right) = \left\{{e}^{\eta t}|\eta >0\right\}$ is selected. Then Equation (19) is rewritten as:

Holding cost: Holding costs are those associated with storing inventory that remains unsold. These costs are one component of total inventory costs, along with ordering and shortage costs. A firm's holding costs include the price of goods damaged or spoiled, as well as storage space, labor, and insurance.

The buyer's holding cost per unit time considering both traditional inventories carrying cost (${\mathrm{C}}_{\mathrm{h}}$) and carbon emission cost (${\mathrm{w}}_{\mathrm{e}}$) due to consumption period is:

Deterioration cost: Deterioration is defined as change, damage, decay, spoilage obsolescence, and loss of utility or loss of original value in a commodity that results in the decreasing usefulness from the original one.

The buyer's deteriorating cost per unit time considering both traditional deteriorating cost (${\mathrm{C}}_{\mathrm{d}}$) and carbon emission cost (${\mathrm{d}}_{\mathrm{e}}$) generated by deteriorating items is:

After substitutions, the above provides

Shortage cost: When demand exceeds the available inventory for an item, the demand and customer goodwill may be lost. The associate cost is called shortage cost.

The shortage cost of the inventory is given by

The lost sale cost is given by

CASE (a): M$\le {\mathit{t}}_{1}$

In this case, because the credit period M is shorter than or equal to the replenishment cycle time ${t}_{1}$, so the retailer begins to pay interest for the items in stock after time M with rate ${\mathrm{I}}_{\mathrm{p}}$. The interest earned by the retailer is $I{E}_{1} = 0$.

The interest paid by the retailer is

Hence, the total cost function per unit time is

Case b: When ${t}_{1}\le$M$\le {t}_{2}$, the interest earned and interest paid by the retailer are as follows:

Hence, the total cost function per unit time is

5.   Solutions procedure

Here, ${t}_{1}$, ${t}_{2}$ and T are decision variables. The quantities $q$ and Q are dependent variables. The objectives of the proposed model are to minimize the cost functions $\mathrm{T}{\mathrm{C}}_{\mathrm{i}}(i = \mathrm{1, 2})$ considering ${t}_{1}$, ${t}_{2}$ and T as decision variables.

The optimal values of the total cost function are calculated by using the following necessary and sufficient conditions:

The convexity of the total cost function is obtained by the well–known Hessian matrix. Here, the Hessian matrix of the total cost function for i = 1, 2

where the principal minors ${\rm{H}}({\rm{T}},{{\rm{t}}_1},{{\rm{t}}_2})$ are $\left| {{{\rm{H}}_1}} \right| > 0, \, \, \, \, \left| {{{\rm{H}}_2}} \right| > 0\, {\rm{and}}\, \, \left| {{{\rm{H}}_3}} \right| > 0$, which are all positive.

6.   Numerical examples

Examples1 (M$\le {\mathit{t}}_{1}$): In this section, the applicability and validity of the proposed model is illustrated through numerical results for a specific type of newborn growing animals: "Broiler Chicken". The parameters of the growth curve are estimated by Goliomytis et al. (2003). The values of the key parameters are as follows: A = 300, a = 48, k = 73, ${ C}_{o}$ = 800, ${C}_{p}$ = 2.7, ${C}_{h}$ = 1.7, ${C}_{l}$ = 0.12, ${C}_{d}$ = 1.5, ${C}_{b}$ = 0.31, m = 200, n = 65, r = .045, θ = 0.32, $\delta =$0.7, $\eta = 17$ and α = 3, ${w}_{e} =$ 0.28, ${d}_{e} =$ 0.43, and ${b}_{e} =$0.023, ${I}_{\tau }$ = 0.15, M = 0.025 and P = 0.17, respectively.

Solving the outlined problem, we have the optimal solution as follows: T${\mathrm{C}}_{1}$ = 562.344, ${t}_{1}$ = 0.0654, ${t}_{2}$ = 0.6070, T = 2.56 and Q = 4.2856.

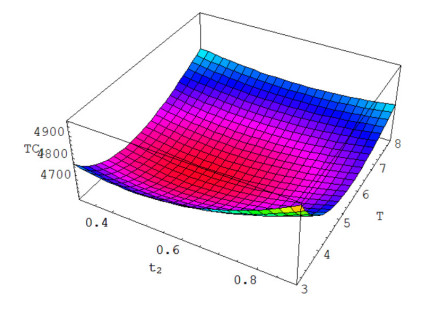
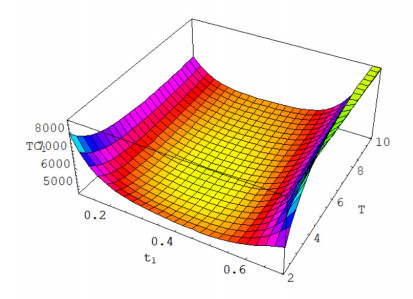
The above Figures 2, 3 & 4 show the convexity nature of the cost functions in different cases.

Examples 2 (${\mathit{t}}_{1}\le$M$\le {\mathit{t}}_{2}$): The data are the same as in Example 1 except M = 0.5. Solving the outlined problem, we have the following optimal solutions: T${\mathrm{C}}_{2}$ = 554.931, ${t}_{1}$ = 0.471, ${t}_{2}$ = 0.8264, T = 2.277 and Q = 7.449.

6.1.   Sensitivity analysis

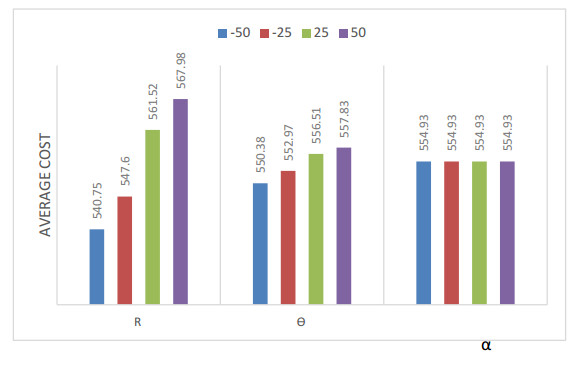
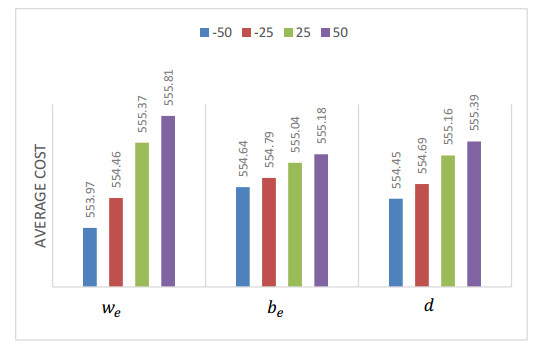
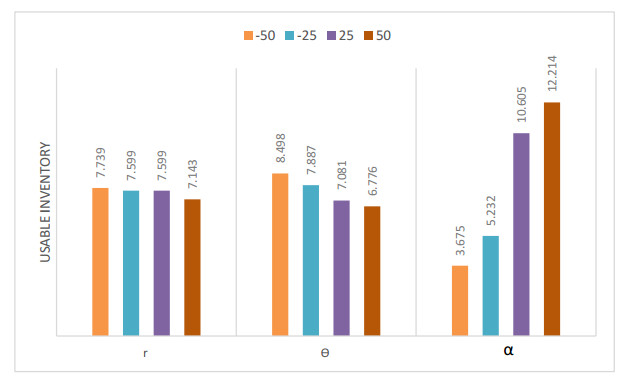
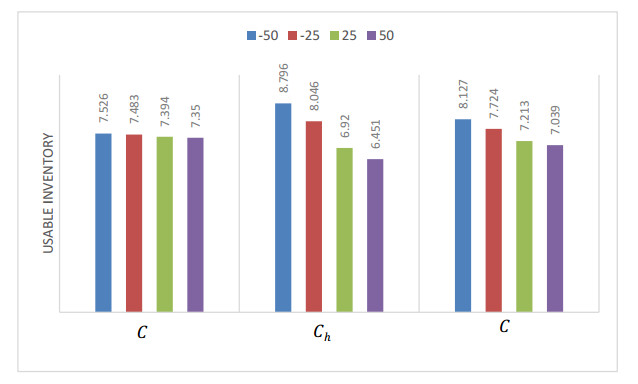
Sensitivity analysis is shown on selected parameters to investigate the effects that changes in those parameters have on the expected total profit per unit time and the economic lot size. Not all input parameters are investigated because the proposed inventory has numerous input parameters. The sensitivity analysis was only shown on nine input parameters, ${\mathrm{r}, \mathrm{\theta }, \mathrm{C}}_{\mathrm{p}}, {\mathrm{C}}_{\mathrm{b}}, {\mathrm{C}}_{\mathrm{h}}, \mathrm{a}, \mathrm{\alpha }, \mathrm{n}, \mathrm{ }\mathrm{m}, {\mathrm{b}}_{\mathrm{e}}, {\mathrm{d}}_{\mathrm{e}},$ respectively. The sensitivity analysis is carried out by variation each parameter by −50%, −25%, +25% and +50%, taking one parameter at a time and other parameters are constant.

6.2.   Observations

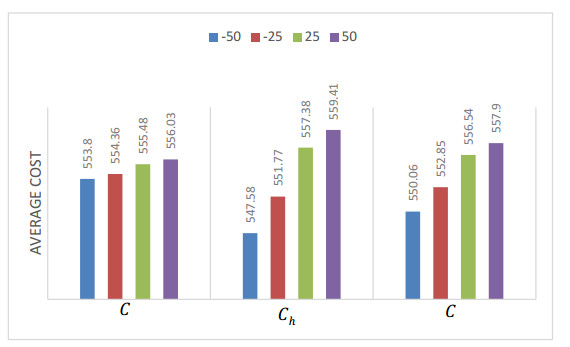
In Figure 6, it is noticed that the average cost is positively sensitive to the changes in the purchasing cost, holding cost, and the breeding cost, but it is relatively more sensitive to the holding cost and least sensitive to the purchasing cost.

In Figure 7, it is noticed that the average cost is highly positive sensitive to the changes in the inflation rate, while slightly positive sensitive to the deterioration rate. It was also observed that the increase in quality control parameters does not affect the average cost of the system.

In Figure 8, it is noticed that the average cost is positively sensitive to the changes in the carbon emission cost due to the holding, breeding, and deterioration, but it is relatively more sensitive to the changes in the carbon emission cost due to the holding than others and least sensitive to the changes due to the deterioration.

In Figure 9, it is noticed that the average cost is positively sensitive to the changes in the demand parameters m and n but slightly negatively sensitive to the changes in the weight formulation parameter. It is also noticed that them is highly sensitive to the changes in the demand parameter m.

In Figure 10, it is noticed that the usable inventory is slightly negative sensitive to the change in the inflation rate, moderately negative sensitive to the deterioration rate but highly positive sensitive to the changes in the quality control parameter.

Figure 11 reveals that the optimal number of items is negatively sensitive to the changes in the purchasing cost, holding cost, and breeding cost. It is noticed that the number of usable inventory is most sensitive to the change in the holding cost and moderately sensitive to the changes in the breeding cost, on the other hand, changes in the purchasing cost do not much affect the decisions regarding the number of items.

Figure 12 reveals that the changes in the weight can cause the fluctuation in the optimal usable inventory in both directions that is the optimal usable inventory first increase then decrease then again increase. While it is positively sensitive to the changes in the demand rate parameters where m is much more sensitive than parameter n.

It is noticed from Figure 13 that the optimum inventory is negatively sensitive to the changes in the carbon emission cost Whether it is emitted from any of the sources but it is quite considerable that the optimum inventory is most sensitive to the changes in the carbon emission cost due to the storage and least sensitive to the changes in the carbon emission cost due to the breeding.

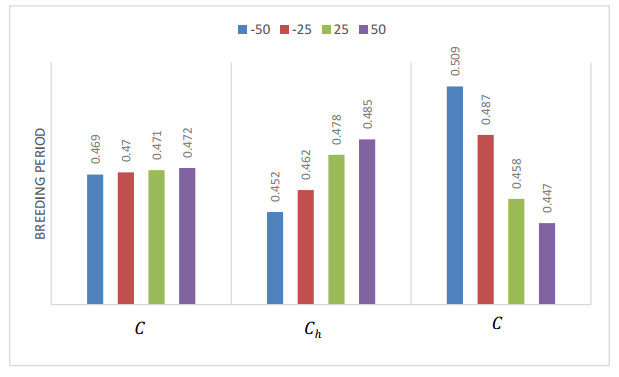
It is noticed from Figure 14 that the breeding period is positively sensitive to the changes in the purchasing cost and the holding cost that is the practitioners should increase the breeding period of the livestock if the holding cost is too higher. It is also noticed that the breeding period is negatively sensitive to the changes in the breeding cost. that is if the breeding cost is higher practitioners should reduce the breeding period these two facts reveal a trade-off between the holding and breeding cost. So, the manager should decide wisely.

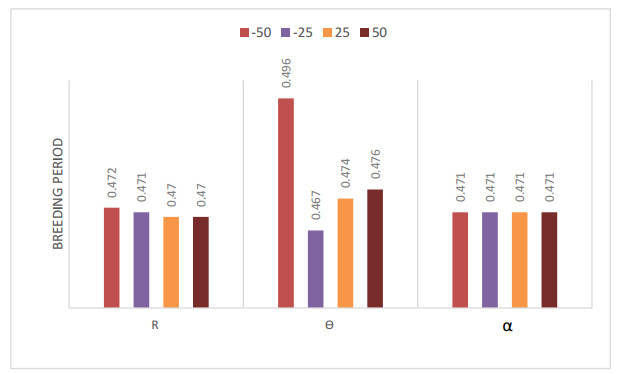
It is noticed from Figure 15 that the breeding period fluctuates with the changes in the deterioration rate, while it is negatively sensitive to the changes in the inflation rate. On the other hand, it is noticed that the breeding period is not affected by the quality control parameter.

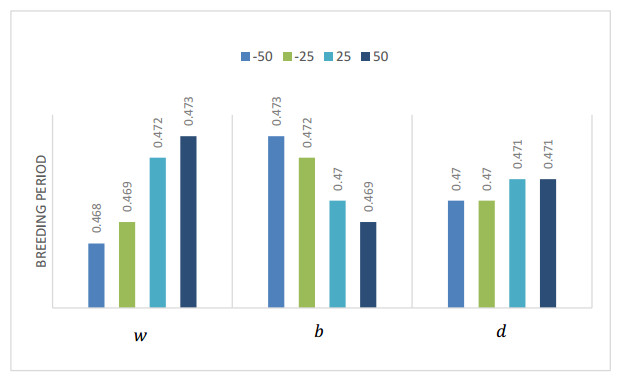
It is noticed from Figure 16 that the breeding period is positively sensitive to the changes in the carbon emission cost due to the storage hence the practitioners should increase the breeding period of the livestock if the holding cost is too higher. It is also noticed that the breeding period is negatively sensitive to the changes in the carbon emission cost due to breeding. It is noticeable that the breeding period is unaffected by the carbon emission cost due to the deterioration.

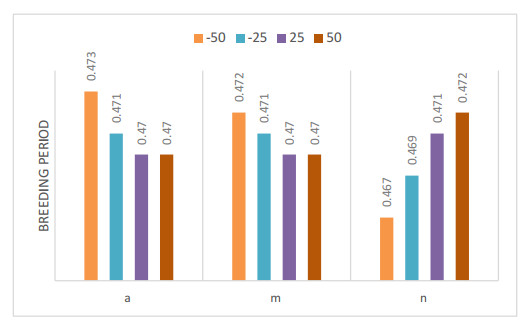
It is noticed from Figure 17 that the breeding period is negatively sensitive to the changes in the disposal rate and the demand parameter m while it is positively sensitive to the changes in the demand parameter n.

7.   Conclusions

In this model, the growing items are considered and it is assumed that the newborn animals are bought at the beginning of the cycle and after the breeding period these are supposed to be slaughtered. These (such as poultry, livestock) will produce carbon emissions during the breeding stage and the holding and deterioration is also a reason for excessive emission, which is very detrimental to the retailer. Many companies are looking for ways to reduce carbon emissions. In this paper, it is presumed that the growth rate of the livestock inventory is taken as a linear function of time. Shortages are assumed to be allowable in the system in the form of partially back-ordering. It is observed from the mathematical and numerical analyses that the total cost, the optimal breeding period, and the order quantity have increased by the effect of carbon emission. It is noticed that the optimum inventory is negatively sensitive to the changes in the carbon emission cost. It is emitted from any of the sources but it is quite considerable that the optimum inventory is most sensitive to the changes in the carbon emission cost due to the storage and least sensitive to the changes in the carbon emission cost due to the breeding. It is observed that the breeding period is positively sensitive to the changes in the purchasing cost and the holding cost that is the practitioners should increase the breeding period of the livestock if the holding cost is too higher. It is also noticed that the breeding period is negatively sensitive to the changes in the breeding cost; which implies that, if the breeding cost is higher practitioners should reduce the breeding period these two facts reveal a trade-off between the holding and breeding cost. So, the manager should decide wisely.

Major limitations of the proposed model are deterministic demand and production rates and all key parameters are static in nature. The model might be extended in future for stochastic demand and production rate of the lives stocks. The proposed model can be extended immediately in several ways considering the integrated system for multi items, block chain technology to improve trust-ability and the model in fuzzy scenarios. The effects of price, advertising and product quality are relaxed in demand function of this model. These factors might be included in future extension of the proposed model.

Conflict of interest

All authors declare no conflicts of interest in this paper.