Modeling of stress distribution on the basis of the measured values of strain and temperature changes

1.   Introduction

To keep pace with today's rapid development in construction and production technology, engineers need to be able to quickly adapt to new types of materials. Engineers should conduct extensive materials testing to maintain the reliability of the built-in components. It is desired to minimize two required factors (time and money) in production. For this reason, engineers and researchers are turning to the modeling and increasingly use models based on the finite element method ^[1,2,3,4]. Depending on what is wanted to be examined, models can be especially complex, as in the case of modeling of mechanical behavior of metal components that are under complex load.

During modeling, it is impossible to count all influencing parameters, so developed models always have some limitation. It is required to determine a significant number of parameters of material, for accurately simulation of real behavior of a certain component ^[3,4,5,6]. Linear regression is usually used to determine the interdependences of various parameters during modeling. Tikhonov-Morozov regularization is one of the most classic and important regularization method that has been efficiently utilized in literature to reduce the influence of measurement noise without notably distorting the measured data ^[7,8].

Researchers develop various models, ranging from modeling of some parameter behavior up to more complex ones for determining the material flow and stress distribution during loading ^[9,10,11]. Lately, there are new approaches in modeling of material flow by using digital image correlation (DIC). DIC method is mainly used for displacement and deformation analysis during deformation of materials ^[12,13]. Researchers can obtain a reliable data for construction of models on material flow with this method. It is so developed that it is even used to validate some developed models ^[13]. DIC method is fully optical, non-contact method and it is insensitive on the shape of the sample, what is one of its advantages.

Thermography is another method that is becoming more and more frequently used. This method is based on the premises that during the plastic deformation of the metals exists change in the internal energy of the deformed metal, which is manifested as the temperature change on the surface of sample in the deformation zone ^[14,15,16]. This change can be detected and measured by an infrared camera, and subsequent thermal analysis provides of the temperature distribution ^[12,16].

Clear insight on the metal flow, throughout the deformation zone, can be obtained from data achieved with thermography and DIC method ^[17]. During the analysis of results from DIC and thermography, the idea for a different and perhaps simpler approach to the modeling has emerged. The aim of this paper is to formulate a mathematical model, which can calculate the values of acting stresses from experimentally measured temperature changes, deformations and knowing stretching rates during tensile testing. The model will be formulated on the basis of experimentally determined values of deformation and temperature changes detected by the methods of digital image correlation and thermography. Once formulated, the model will be validated and verified by experimentally measured values.

2.   Materials and methods

To determine the stresses, strains and temperature changes in the deformation zone during the static tensile testing the deformation of samples were recorded with an infrared and optical digital camera. Subsequent analysis of recorded date was done using thermography and digital image correlation method in order to determine the maximum values of temperature changes and strains. The maximum stresses were determined from static tensile testing in the same points. The chemical composition of the tested steel is given in Table 1.

Table 1.  Chemical composition of the tested steel, wt%.

Element	C	Mn	Si	P	S	Al	Nb	N
Micro-alloyed steel	0.12	0.78	0.18	0.011	0.018	0.02	0.048	0.008

---

 | Show Table

DownLoad: CSV

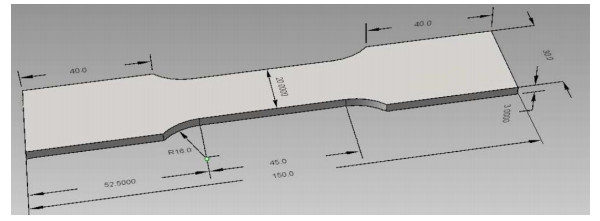
Tests were performed at four different stretching rates in range from 5 up to 30 mm·min⁻¹. The samples for static tensile testing were taken in rolling direction from 3 mm thick hot-rolled strip. Tests were performed on samples with rectangular cross-section, with gage length of 45 mm and gage width of 20 mm (Figure 1).

Figure 1.  Configuration of the samples for tensile testing.

DownLoad: Full-Size Img PowerPoint

Using the MathCAD and OriginPro software packages, the analysis of variance-ANOVA and χ² test was performed on values of measured results. Subsequently, the multiple linear regression analysis was used to formulate the mathematical model for stress calculation.

3.   Results and discussion

In the previous work ^[18], it was found out that stretching rate has influence on the measured values of temperature change, strain and stress. For that reason, the first step before formulating the model was to investigate interdependence of measured values at used stretching rates. The results of analysis on the influence of temperature change on the strain at all stretching rates are shown in Figure 2.

Figure 2.  Influence of stretching rate on the dependence between temperature change and strain.

DownLoad: Full-Size Img PowerPoint

Analysis shows that in the case of microalloyed steel with the 0.048% of Nb, the temperature change increases with strain increase at all stretching rates. The increase in temperature is linear with strain increase. Linear equations, for the dependence between temperature change and strains, were determined for each stretching rate:

It is clear that temperature change increase is even more pronounced by increasing the stretching rate from obtained dependences and equations. So this needs to be taken into account when formulating mathematical model. This indicates a close relationship between deformation (i.e., strain), stretching rate (i.e., strain rate) and temperature changes. In order to clarify the influence of stress on the temperature change, analyses were done on relationship between stress and temperature change at all used stretching rates (Figure 3).

Figure 3.  Influence of stretching rate on the dependence between stress and temperature change.

DownLoad: Full-Size Img PowerPoint

It is a reasonable assumption the maximum stresses are acting where maximum temperature change occurs at the place of the maximum deformation. Starting from this assumption, the stress values were determined from results of the tensile test. At the same points where the values of temperature change and strain were determined from thermography and DIC, the stress values were read out from the results of the tensile test. As can be seen in the above correlation diagram, the increase in stress has a significant effect on the temperature change. Influence of stretching rate is not so much pronounced as in the case of relation ΔT_max–ε, but it is still present. There is a higher temperature change increase by increasing stretching rate. Functional relationship between temperature change and stresses is represented by an exponential function at all used stretching rate.

From obtained dependencies in Figures 2 and 3, one can conclude that the measured temperature changes are closely related to the stretching rate, values of stress and strains achieved during the tensile test. Accordingly, the following relation can be set:

where is: σ—stress (MPa)

      ε—strain (mm·mm⁻¹)

      v—streching rate (mm·mm⁻¹)

By the further transformation of Eq 5, stress can be determined from measured values of temperature changes, strains and known stretching rate:

The functional relationships between the temperature changes, strains, stress and stretching rates was formulated using the multiple linear regression analysis. At first the multiple linear regressions was carried out on the experimentally obtained results from thermography, static tensile test and digital image correlation, at each stretching rate separately.

The general function of dependency of temperature change on the strain and corresponding stresses is in the form of:

where is: a, b, c—parameters dependent on stretching rate.

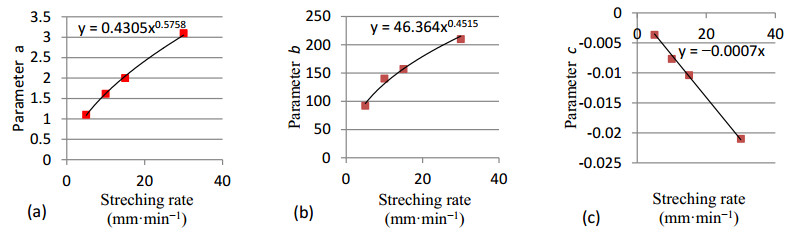
It is clear that by increasing stretching rate, there is the change in the values of the determined parameters a, b, and c (Table 2).

Table 2.  Determined values of paremeter a, b, and c at diferent streching rates for Nb microalloyed steel.

Streching rate (mm·min−1)	a	b	c
5	1.1	92.1	−0.0037
10	1.6	140.2	−0.0077
15	2.0	157.2	−0.0104
30	3.1	210.0	−0.0210

---

 | Show Table

DownLoad: CSV

Functional dependency of parameters on the stretching rate is shown by Figure 4. Dependences of parameters a, b and c on the stretching rate is given by following relations:

Figure 4.  Dependency of function parameters on the testing speed.

DownLoad: Full-Size Img PowerPoint

By incorporating functional dependences of parameters a, b and c as a function of stretching rate in the initial Eq 11, obtined by linear regresion, the function of temperature change is now:

By transformation of Eq 15 it is possible to express the stress as the function of temperature change, strains and stretching rate:

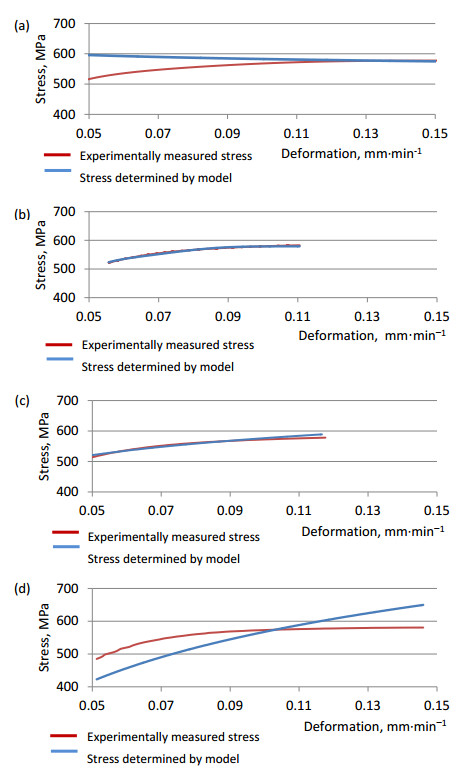
The mathematical model for stress calculation formulated on the basis of the above achieved regression equations was validated and verified with the measured values of strains and temperature changes at all used stretching rates. The comparison between the model and the experimentally measured values is shown in Figure 5.

Figure 5.  Comparison between the calculated values and the experimentally measured values. Testing speed: (a) 5 mm·min⁻¹, (b) 10 mm·min⁻¹, (c) 15 mm·min⁻¹ and (d) 30 mm·min⁻¹.

DownLoad: Full-Size Img PowerPoint

As it can be seen from given diagrams, it has been achieved a good agreement between model and experimentally measured values up to the stretching rate of 30 mm·min⁻¹. Some deviations of modeled values can be observed at 5 mm·min⁻¹. This is only in the beginning of plastic flow, and afterwards there is a good agreement between modeled and measured values.

4.   Conclusions

ANOVA and χ² test analysis conducted on experimentally obtained data established that there is strong interdependence between measured values of temperature changes, strain, stresses and stretching rate during deformation of the tested samples. The functional relationship was determined using multiple linear regression analysis between the tree independent variables temperature changes, starching rate and strains and the stresses as the dependent variable.

Formulated mathematical model enables the calculation of acting stresses in the deformation zone on the basis of the experimentally measured values of temperature changes, strains and known used starching rate. Model was validated and verified by comparing the calculated and experimentally determined values. Model accurately calculated stress values on the basis of measured values of temperature change and deformation up to the stretching rate of 30 mm·min⁻¹.

Acknowledgements

This work has been fully supported by the Croatian Science Foundation under the project number IP-2016-06-1270.

Conflict of interests

All authors declare no conflicts of interest in this paper.