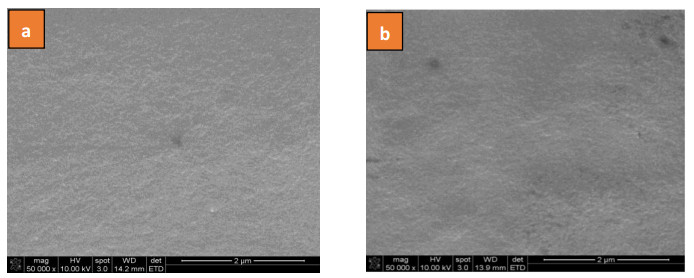
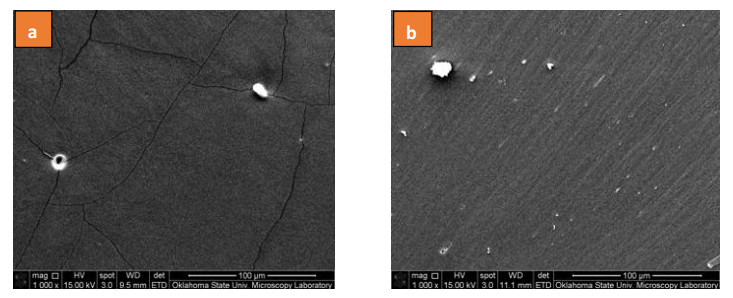
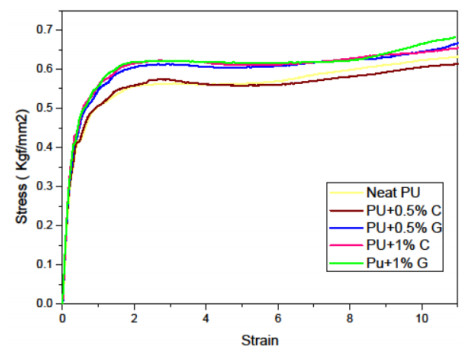
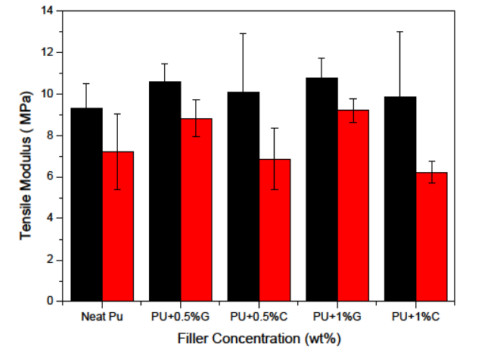
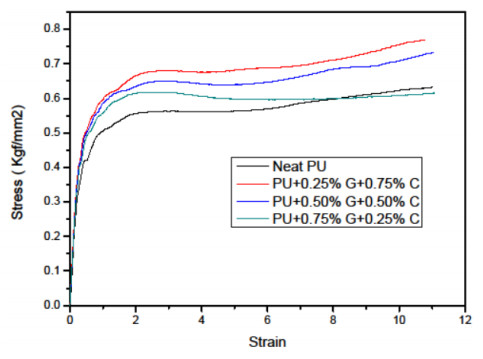
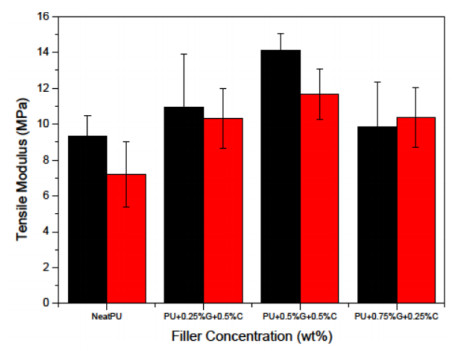
This research examines reduction of microcracks in polyurea by addition of graphene and nanoclay on account of potential improvement in barrier properties. Graphene and nanoclay were added in different wt. fractions to polyurea followed by thin film preparation. The prepared thin film samples were characterized using scanning electron microscopy. Surface images before and after exposure to cryogenic temperature indicate that controlled addition of graphene and nanoclay to polyurea thin film can lead to a reduction in microcracking caused by thermal shocks.
1.
Introduction
It is well known that the general approaches in fuzzy differential systems are established using the concept of fuzzy sets, as introduced by Zadeh [1] in 1965. The theory of fuzzy sets and some of their applications have been developed in a number of books and papers. We will refer the readers to [2,3,4] and the references cited there.
On the other hand, the notions of an H−Hukuhara derivative and H− differentiability were introduced in 1983 for fuzzy mappings [5], and the notion of integrals in [6]. Since then, the investigations of fuzzy differential equations have undergone rapid development. See, for example, [7,8,9,10,11,12,13,14] and the references therein. The theory of fuzzy differential equations is still a hot topic for research [15,16,17,18,19] including numerous applications considering fuzzy neural networks and fuzzy controllers [20,21,22,23,24,25].
It is also well known that the functionality of many complex engineering systems, as well as, the long life of their practical operation are provided under the conditions of uncertainties [26]. In fact, due to inaccuracy in the measurements of the model parameters, data input and different types of unpredictability, uncertain parameters occur in a real system [27]. It is, therefore, clear that the study of the effects of uncertain values of the parameters on the fundamental and qualitative behavior of a system is of significant interest for theory and applications [28,29,30,31,32,33,34], including fuzzy modeling [35]. Considering the high importance of considering uncertain parameters, the theory of uncertain fuzzy differential equations needs future development and this is the basic aim and contribution of our research.
This paper deals with systems of fuzzy differential equations that simulate the perturbed motion of a system with uncertain values of parameters that belong to a certain domain. A regularization procedure is proposed for the family of differential equations under consideration with respect to the uncertain parameter. An analysis of some fundamental properties of the solutions is performed for both, the original fuzzy system of differential equations and the intermediate families of differential equations. The introduced regularization scheme expands the horizon for the extension of the fundamental and qualitative theory of fuzzy differential equations to the equations involving uncertain parameters. The authors expect that the proposed results will be of particular interest to researchers in the study of the qualitative properties of such systems, including equations with delays and some modifications of the Lyapunov theory. In addition, the engineering applications of such results to the design of efficient fuzzy controllers are numerous.
The innovation and practical significance of our research are as follows:
(ⅰ) we introduce a new regularization scheme for uncertain fuzzy differential equations;
(ⅱ) new existence criteria of the solutions of the regularized fuzzy differential equations are proposed;
(ⅲ) the distance between two solutions is estimated;
(ⅳ) the offered regularization scheme reduces a family of fuzzy differential equations to a simple form that allows analysis of the properties of solutions of both the original fuzzy system of differential equations, as well as the intermediate families of differential equations.
The rest of the paper is organized according to the following scenario. In Section 2 we provide the necessary preliminary notes from the theory of fuzzy sets. Some main properties of fuzzy functions and H−Hukuhara derivatives are also given. In Section 3 we introduce a regularization procedure for uncertain fuzzy systems of differential equations, and the basic problem of analyzing such systems based on the developed procedure is presented. In Section 4 conditions for existence of the solutions of the regularized fuzzy differential equations are established. In Section 5 we offer an estimate of the distance between solutions of the regularized equations. The closing Section 6 provides some comments and future directions for research.
2.
Fuzzy sets and functions: Preliminary notations and results
This section is mainly based on the results in [2,4,14].
The use of fuzzy sets introduced in [1] has facilitated the mathematical modeling and analysis of real processes that involve uncertain parameters. Recently, the fuzzy set approach achieved significant development. In this section, we will present some elements from the fuzzy set theory and fuzzy functions that are necessary for the analysis of uncertain systems.
2.1. Fuzzy sets
Consider a basic set X with elements of an arbitrary nature. To each element x∈X a value of a membership function ξ(x) is assigned, and the function ξ(x) takes its values from the closed interval [0,1].
Following [1], for a function ξ:X→[0,1], we consider a fuzzy subset of the set X as a nonempty subset with elements {(x,ξ(x)):x∈X} from X×[0,1].
For a fuzzy set with a membership function ξ on X its ϰ-level sets [ξ]ϰ are defined by
The closure of the union of all ϰ-level sets for a fuzzy set with a membership function ξ in the general topological space X is called its support, and it is denoted by [ξ]0, i.e.,
Note that most often, the space X is the N-dimensional Euclidean space RN equipped with a norm ‖⋅‖.
Next, the Hausdorff distance between two nonempty subsets U and V of RN is defined as
where Vr(0)={x∈RN:‖x‖<r}, r≥0.
The above distance is symmetric with respect to both subsets U and V. For more properties of ΔH(U,V) we refer the reader to [2,4,14].
2.2. The space EN
We will next need the space EN of functions ξ:RN→[0,1], which satisfy the following conditions (cf. [2]):
1) ξ is upper semicontinuous;
2) there exists an x0∈RN such that ξ(x0)=1;
3) ξ is fuzzy convex, i. e.,
for any values of ν∈[0,1];
4) the closure of the set {x∈RN:ξ(x)>0} is a compact subset of RN.
It is well known that [7,8,9,10,11,12,13,14] and [15,16,17,18,19], if a fuzzy set with a membership function ξ is a fuzzy convex set, then [ξ]ϰ is convex in RN for any ϰ∈[0,1].
Since EN is a space of functions ξ:RN→[0,1], then a metric in it can be determined as
The least upper bound of ΔH on EN is defined by
for ξ,η∈EN. The above defined d[ξ,η] satisfies all requirements to be a metric in EN [2].
2.3. Properties of fuzzy functions
Consider a compact T=[α,β], β>α>0.
The mapping F:T→EN is strictly measurable, if for any ϰ∈[0,1] the multivalued mapping Fϰ:T→Pk(RN), defined as Fϰ(τ)=[F(τ)]ϰ, is measurable in the sense of Lebesgue under the condition that Pk(RN) is equipped with a topology generated by the Hausdorff metric, where Pk(RN) denotes the family of all nonempty compact convex subsets of RN [11,36].
The mapping F:T→EN is integrally bounded if there exists an integrable function ω(τ) such that ‖x‖≤ω(τ) for x∈F0(τ).
We will denote by β∫αF(τ)dτ the integral of F on the interval T defined as
for any 0<ϰ≤1.
The strictly measurable and integrally bounded mapping F:T→RN is integrable on I, if ∫TF(τ)dτ∈EN.
Numerous important properties of fuzzy functions are given in [2,4,11].
2.4. Hukuhara-type derivative
Let ξ,η∈EN. If there exists ζ∈EN such that ξ=η+ζ, ζ is determined as the Hukuhara-type difference of the subsets ξ and η and is denoted by ξ−η.
If both limits in the metric space (EN,Δ)
exist and are equal to L, then the mapping F:T→EN is differentiable at the point τ0∈T, and L=F′(τ0)∈EN.
The family {DHFϰ(τ):ϰ∈[0,1]} determines an element F′(τ)∈EN. If Fϰ is differentiable, then the multivalued mapping Fϰ is differentiable in the sense of Hukuhara for all ϰ∈[0,1] and
where DHFϰ is the Hukuhara-type derivative of Fϰ.
Several basic properties of differentiable mappings F:I→EN in the Hukuhara sense are given below:
1) F is continuous on T;
2) For τ1,τ2∈T and τ1≠τ2 there exists an ι∈EN such that F(τ2)=F(τ1)+ι;
3) If the derivative F′ is integrable on T, then
4) If θ0∈EN and θ0(x)={1,forx=0,0,x∈RN∖{0}, then
3.
Regularization scheme for systems of fuzzy differential equations
In this section, we will introduce a regularization scheme for a system of fuzzy differential equations with respect to an uncertain parameter.
Consider the following fuzzy system with an uncertain parameter
where τ0∈R+, ξ∈EN, f∈C(R+×EN×S,EN), μ∈S is an uncertain parameter, S is a compact set in Rl.
The parameter vector μ that represents the uncertainty in system (3.1) may vary in nature and can represent different characteristics. More precisely, the uncertainty parameter μ:
(a) may represent an uncertain value of a certain physical parameter;
(b) may describe an estimate of an external disturbance;
(c) may represent an inaccurate measured value of the input effect of one of the subsystems on the other one;
(d) may represent some nonlinear elements of the considered mechanical system that are too complicated to be measured accurately;
(e) may be an indicator of the existence of some inaccuracies in the system (3.1);
(f) may be a union of the characteristics (a)–(e).
Denote
and suppose that fm(τ,ξ), fM(τ,ξ)∈C(R+×EN,EN). It is clear that
for (τ,ξ,μ)∈R+×EN×S.
For (τ,η)∈R+×EN and 0≤ϰ≤1, we introduce a family of mappings fϰ(τ,η) by
and, we will introduce a system of fuzzy differential equations corresponding to the system (3.1) as
where fϰ∈C(T×EN,EN), T=[τ0,τ0+a], τ0≥0, a>0, ϰ∈[0,1].
We will say that the mapping η:T→EN is a solution of (3.6), if it is weakly continuous and satisfies the integral equation
for all τ∈T and any value of ϰ∈[0,1].
The introduced fuzzy system of differential equations (3.6) is considered as a regularized system of the system (3.1) with respect to the uncertain parameter.
It is clear that for all τ∈T we have diam[ξ(τ)]ϰ≥diam[ξ0]ϰ, for any value of ϰ∈[0,1], where diam denotes the set diameter of any level [2,4,11].
The main goal and contribution of the present paper are to investigate some fundamental properties of the regularized system (3.6) on T and [τ0,∞).
4.
Criteria for existence and uniqueness of solutions
In this section we will state criteria for the existence and uniqueness of the solutions of the introduced regularized problem (3.6).
Theorem 4.1. If the family fϰ(τ,η)∈C(T×EN,EN) for any ϰ∈[0,1] and there exists a positive constant Lϰ such that
then for the problem (3.6) there exists a unique solution defined on T for any ϰ∈[0,1].
Proof. We define a metric in C(T,EN) as:
for all η,ˉη∈EN, where λ=2maxLϰ, ϰ∈[0,1]. The completeness of (EN,d)implies the completeness of the space (C(T,EN),H).
Let ξϰ∈C(T,EN) for any ϰ∈[0,1] and the mapping Tξϰ is defined as
From the above definition we have that Tξϰ∈C(T,EN) and
Since d[η,ˉη]=H[η,ˉη]eλτ, we have
Given the choice of λ, from (4.1) we obtain
The last inequality implies the existence of a unique fixed point ξϰ(τ) for the operator Tξϰ which is the solution of the initial value problem (3.6) for ϰ∈[0,1].
Now, let us define a second metric in C(T,EN) as follows:
where η,ˉη∈EN, and consider a family of continuous functions that have equal variation over a given neighborhood. Such a family of functions is know to be equi-continuous [11].
Theorem 4.2. If the family fϰ(τ,η)∈C(T×EN,EN) for any ϰ∈[0,1] and there exists a positive constant Ωϰ>0 such that
then for the problem (3.6) there exists a unique solution defined on T for any ϰ∈[0,1].
Proof. Let the set B, B⊆C(T,EN) be bounded. Then, according to the definition of the mapping T, the set TB={Tξϰ:ξϰ∈B,ϰ∈[0,1]} is bounded, if it is equi-continuous, and for any τ∈T the set [TB](τ)={[Tξϰ](τ):τ∈T,ϰ∈[0,1]} is a bounded subset of EN.
For τ1<τ2∈T and η∈B, we have from (2.1) that
where ¯Ω=maxϰΩϰ. This implies the equi-continuity of the set TB.
Also, for any fixed τ∈T we have that
for τ1∈T, η∈B.
From the above inequality, we conclude that the set {[Tξϰ](τ):ξϰ∈B,ϰ∈[0,1]} is bounded in the space EN, which, according to the Arzela–Ascoli theorem, implies that the set TB is a relatively compact subset of C(T,EN).
Next, for ϰ∈[0,1] and M>0 we define B∗={ξϰ∈C(T,EN):H∗[ξϰ,θ0]<M¯Ω, B∗⊆(C(T,EN),H∗).
Obviously, TB⊂B∗, since ξϰ∈C(T,EN), ϰ∈[0,1] and d[Tξϰ(τ),Tξϰ(τ0)]=d[Tξϰ(τ),θ0]≤|τ−τ0|Ωϰ<M¯Ω, ϰ∈[0,1]. Let θ0(τ)=θ0 for τ∈T, where θ0(τ):T→EN. Then
Hence, T is compact. Therefore, it has a fixed point ξϰ(t), and according to the definition of T, ξϰ(t) is the solution of the initial value problem (3.6) for ϰ∈[0,1]. This completes the proof.
Remark 4.3. Theorems 4.1 and 4.2 offered new existence criteria for the regularized system (3.6). The proposed criteria show that the idea to use a family of mappings and regularize the fuzzy system (3.1) with respect to uncertain parameters greatly benefits its analysis. Note that due to some limitations and difficulties in the study of fuzzy differential systems with uncertain parameters, the published results in this direction are very few [11,18]. Hence, the proposed regularization procedure complements such published accomplishments and, due to the offered advantages is more appropriate for applications.
Remark 4.4. The proposed existence results also extend and generalize some recently published existence results for differential systems with initial and nonlocal boundary conditions [37], where the fixed-point argument plays a crucial role in manipulating the integral equation, to the fuzzy case.
The validity of Theorem 4.1 is demonstrated by the next example.
Example 4.5. Let fϰ(τ,η)=Aϰη+Bϰ for any value of ϰ∈[0,1] and Aϰ,Bϰ∈E1. Consider the initial value problem
It is easy to show that
where Lϰ=max∣Aϰ∣ for ϰ∈[0,1].
Therefore, all conditions of Theorem 4.1 are satisfied, and hence there exists a unique solution of the initial value problem (4.4)-(4.5). The unique solution is of the type
where
for any value of ϰ∈[0,1]. A solution in the form of (4.6) is compact for all t∈T and the union contains all upper and lower solutions of the problem (4.4)-(4.5). For the rationale for this approach, see [11], pp. 150–155).
Remark 4.6. The conditions of Theorem 4.1 imply the following estimate
for all t∈T, η,ˉη∈E1 and any value of ϰ∈[0,1]. Hence, for Ωϰ=12Lϰd[η,ˉη] all conditions of Theorem 4.1. are also satisfied. Therefore, the conditions of Theorem 4.2 are some modifications of these of Theorem 4.1.
5.
Distance between solutions
Given that the inequalities
are satisfied for all (τ,η)∈T×EN, it is important and interesting to evaluate the distance between two solutions η(τ), ˉη(τ) of the regularized system (3.6) depending on the initial data. This is the aim of the present section.
Theorem 5.1. Assume the following:
1) The family fϰ∈C(T×EN,EN) for any ϰ∈[0,1].
2) There exists a continuous function g(τ,ζ), g: T×R+→R, which is nondecreasing with respect to its second variable ζ for any τ∈T, and such that for (τ,η),(τ,ˉη)∈T×EN and ϰ∈[0,1],
3) The maximal solution u(τ,τ0,y0) of the scalar problem
exists on T.
4) The functions η(τ) and ˉη(τ) are any two solutions of the problem (3.6) defined on T, corresponding to initial data (η0,ˉη0) such that d[η0,ˉη0]≤y0.
Then,
Proof. Set d[η(τ),ˉη(τ)]=ρ(τ). Then, ρ(τ0)=d[η0,ˉη0]. Also, from (3.7), for any ϰ∈[0,1] we obtain
Using (5.2) we get
Applying to (5.3) Theorem 1.6.1 from [38], we conclude that the estimate (5.1) is satisfied for any τ∈T and ϰ∈[0,1].
Remark 5.2. The estimate offered in Theorem 5.1 is based on the integral equation, and on the existence and uniqueness results established in Section 4. Such estimations are crucial in the investigation of the qualitative properties of the solutions when the Lyapunov method is applied. Hence, the proposed result can be developed by the use of the Lyapunov technique in the study of the stability, periodicity and almost periodicity behavior of the states of the regularized system (3.6).
Condition 2 of Theorem 5.1 can be weakened while maintaining its statement.
Theorem 5.3. Assume that Condition 1 of Theorem 5.1 holds, and that the following are true:
1) There exists a family of functions gϰ∈C(T×R+,R) such that
for any ϰ∈[0,1] and (τ,η),(τ,ˉη)∈R+×EN.
2) The maximal solution uϰ(τ,τ0,y0) of the scalar problem
is defined on T.
Then,
where η(τ) and ˉη(τ) are any two solutions of the problem (3.6) defined on T, corresponding to initial data (η0,ˉη0) such that d[η0,ˉη0]≤y0 and ¯u(τ,τ0,y0)=maxϰuϰ(τ,τ0,y0).
Proof. Denote again ρ(t)=d[η(τ),ˉη(τ)]. Then, for the difference ρ(τ+h)−ρ(τ), h>0, we have
From the above inequalities, we get
The conclusion of Theorem 5.3 follows in the same way as in Theorem 5.1 by applying Theorem 1.6.1 from [38] to (5.5). Hence, for any ϰ∈[0,1] we get
and, therefore, d[η(τ),ˉη(τ)]≤¯u(τ,τ0,y0) for τ∈T. The proof of Theorem 5.2 is complete.
Theorems 5.1 and 5.3 offer also the opportunities for estimating the distance between an arbitrary solution η(τ) of the regularized problem (3.6) and the "steady state" θ0∈EN of (3.6).
Corollary 5.4. Assume that Condition 1 of Theorem 5.1 holds, and that the family of functions g∗ϰ∈C(T×R+,R) is such that
(a) d[fϰ(τ,η),θ0]≤g∗ϰ(τ,d[η,θ0]) or
(b) limsup{[d[η+hfϰ(τ,η),θ0]−d[η,θ0]]h−1:h→0+}≤g∗ϰ(τ,d[η,θ0]) for all τ∈T, ϰ∈[0,1].
Then d[η0,θ0]≤y0 implies
where ¯u(τ,τ0,y0)=maxϰuϰ(τ,τ0,y0), uϰ(τ,τ0,y0) is the maximal solution of the family of comparison problem
and η(τ) is an arbitrary solution of the problem (3.6) defined on T, corresponding to the initial value η0.
Corollary 5.5. If in Corollary 5.4, g∗ϰ(τ,d[η,θ0])=λ(τ)d[η,θ0] with λ(τ)>0 for τ∈T, then the estimate (5.6) has the form
for any ϰ∈[0,1].
Remark 5.6. All established results for the regularized system (3.6) can be applied to obtain corresponding results for the uncertain fuzzy problem (3.1). Thus, the proposed regularized scheme offers a new approach to study a class of fuzzy differential systems with uncertainties via systems of type (3.6), which significantly simplifies their analysis and is very appropriate for applied models of type (3.1). This will be demonstrated by the next example.
Remark 5.7. The proposed regularized scheme and the corresponding approach can be extended to more general systems considering delay effects, impulsive effects and fractional-order dynamics.
Example 5.8. We will apply Theorem 5.1 to estimate the distance between a solution of the problem (3.1) and the equilibrium state θ0. To this end, we consider a particular function g(τ,ζ) from Condition 2 of Theorem 5.1. We transform the fuzzy equation in (3.1) by using the regularized process to the form
where g(τ,ξ,μ)=f(τ,ξ,μ)−fϰ(τ,ξ) for all μ∈S. Further we will suppose that fϰ∈C(T×EN,EN) for all ϰ∈[0,1] and g∈C(T×EN×S,EN), T⊆[τ0,α], g(τ,0,μ)≠0 for all τ≥τ0.
Let fϰ(τ,ξ) and g(τ,ξ,μ) be such that for all τ∈T there exist continuous positive functions Ω(τ) and o(τ) satisfying the hypotheses
1) d[fϰ(τ,ξ),θ0]≤Ω(τ)d[ξ,θ0] for all ϰ∈[0,1];
2) d[g(τ,ξ,μ),θ0]≤o(τ)dq[ξ,θ0] for all μ∈S;
3) ψ(τ0,τ)=(q−1)dq−1[ξ0,θ0]τ∫τ0o(σ)exp[(q−1)σ∫τ0Ω(υ)dυ]dσ<1, q>1.
For the family of equations (5.7), we assume that Hypotheses 1–3 are fulfilled for all τ,σ∈[τ0,α].
Then, the deviations of any solution ξ(τ) of (5.7) from the state θ0∈EN are estimated as follows
for all τ∈[τ0,α].
From (5.7), we have
Let z(t)=d[ξ(τ),θ0]. Then z(τ0)=d[ξ0,θ0], and
In view of Hypotheses 1 and 2, the inequality (5.10) yields
or
Applying the estimation technique from [39] to inequality (5.11), we obtain the estimate
From (5.12) we obtain (5.8).
If the Hypotheses 1 and 2 are satisfied and 0<q<1, then by applying Theorem 2.2 from [40] to the inequality (5.11), we get the estimate (5.8) in the form
for all τ∈[τ0,α].
6.
Concluding remarks
In this paper we investigate some fundamental properties of fuzzy differential equations using a new approach. We introduce a regularization scheme for systems of fuzzy differential equations with uncertain parameters. Existence and uniqueness criteria for the regularized equations are established. Estimates about the distance between solutions of the regularized equations are also proposed. The proposed technique and the new results will allow us to consider the qualitative properties of the solutions such as stability, boundedness, periodicity, etc. The introduced approach can also be extended to study delayed systems and impulsive control systems via modifications of the Lyapunov theory [41,42,43,44]. The advantages of the proposed regularization procedure can be implemented in various fuzzy models such as fuzzy neural networks with uncertain parameters, fuzzy models in biology with uncertain parameters, fuzzy models in economics with uncertain parameters, and much more. Numerical applications of our finding in a way that is similar to [45] are also interesting and challenging further directions of research.
Conflict of interest
The authors declare no conflict of interest.














 DownLoad:
DownLoad: