Search Advanced

AIMS Materials Science

2019, Volume 6, Issue 1: 80-96. doi: 10.3934/matersci.2019.1.80
Previous Article Next Article
Research article

Optimization on dry sliding wear behavior of yellow brass using face centered composite design

  • Department of Mechanical Engineering, Qassim University, Buraydah, Saudi Arabia
  • Received: 13 October 2018 Accepted: 13 January 2019 Published: 15 February 2019

  • The proposed research has investigated the dry sliding wear behavior at ambient temperature atmosphere on yellow brass intended for locks, gears, bearings, ammunition casings, and valves. The experiments were conducted based on the central composite design. The wear experiments were performed using the pin-on-disc tribometer by varying the applied load, the sliding velocity, and the sliding distance. The obtained wear results were examined using the multiple regression non-linear model and then the results were validated by performing the confirmation test. It was found that the predicted wear based on the developed regression model was well correlated with the confirmation results. Further, the detailed microstructures of as-received and worn surfaces were examined and reported. In addition, the plotted surface graphs between the input independent parameters and the responses showed that the wear rate started to vary linearly with the function of load whereas it was varied non-linearly with the function of the sliding velocity and the sliding distance.

    Citation: Subbarayan Sivasankaran. Optimization on dry sliding wear behavior of yellow brass using face centered composite design[J]. AIMS Materials Science, 2019, 6(1): 80-96. doi: 10.3934/matersci.2019.1.80

    Related Papers:

    [1] Abdulkader A. Annaz, Saif S. Irhayyim, Mohanad L. Hamada, Hashim Sh. Hammood . Comparative study of mechanical performance between Al–Graphite and Cu–Graphite self-lubricating composites reinforced by nano-Ag particles. AIMS Materials Science, 2020, 7(5): 534-551. doi: 10.3934/matersci.2020.5.534
    [2] Nur Farah Bazilah Wakhi Anuar, Mohd Shukor Salleh, Mohd Zaidi Omar, Saifudin Hafiz Yahaya . Mechanical properties and dry sliding wear behaviour of Al–Si–Mg alloy by equal channel angular pressing. AIMS Materials Science, 2022, 9(5): 733-749. doi: 10.3934/matersci.2022045
    [3] Saif S. Irhayyim, Hashim Sh. Hammood, Anmar D. Mahdi . Mechanical and wear properties of hybrid aluminum matrix composite reinforced with graphite and nano MgO particles prepared by powder metallurgy technique. AIMS Materials Science, 2020, 7(1): 103-115. doi: 10.3934/matersci.2020.1.103
    [4] Vijaykumar S Jatti, Nitin K Khedkar, Vinaykumar S Jatti, Pawandeep Dhall . Investigating the effect of cryogenic treatment of workpieces and tools on electrical discharge machining performance. AIMS Materials Science, 2022, 9(6): 835-862. doi: 10.3934/matersci.2022051
    [5] Zarina Aringozhina, Nurtoleu Magazov, Bauyrzhan Rakhadilov, Gulzhaz Uazyrkhanova, Auezhan Amanov . Effect of ultrasonic nanocrystalline surface modification on hardness and elastic modulus of Ti-6Al-4V alloy. AIMS Materials Science, 2025, 12(1): 101-117. doi: 10.3934/matersci.2025008
    [6] Omar Bataineh, Abdullah F. Al-Dwairi, Zaid Ayoub, Mohammad Al-Omosh . DOE-based experimental investigation and optimization of hardness and corrosion rate for Cu-x%Al2O3 as processed by powder metallurgy. AIMS Materials Science, 2021, 8(3): 416-433. doi: 10.3934/matersci.2021026
    [7] Abdelmoneim El Naggar, Maged A. Youssef . Shape memory alloy heat activation: State of the art review. AIMS Materials Science, 2020, 7(6): 836-858. doi: 10.3934/matersci.2020.6.836
    [8] Bauyrzhan Rakhadilov, Ainur Zhassulan, Daryn Baizhan, Aibek Shynarbek, Kuanysh Ormanbekov, Tamara Aldabergenova . The effect of the electrolyte composition on the microstructure and properties of coatings formed on a titanium substrate by microarc oxidation. AIMS Materials Science, 2024, 11(3): 547-564. doi: 10.3934/matersci.2024027
    [9] M. Achyutha Kumar Reddy, V. Ranga Rao, K. Naga Chaitanya, Veerendrakumar C. Khed . Optimization of Bentocrete parameters using Response Surface Methodology (RSM). AIMS Materials Science, 2021, 8(2): 221-246. doi: 10.3934/matersci.2021015
    [10] Claude Stolz . Moving surfaces and interfaces : application to damage, fracture and wearcontact. AIMS Materials Science, 2016, 3(3): 881-907. doi: 10.3934/matersci.2016.3.881
  • The proposed research has investigated the dry sliding wear behavior at ambient temperature atmosphere on yellow brass intended for locks, gears, bearings, ammunition casings, and valves. The experiments were conducted based on the central composite design. The wear experiments were performed using the pin-on-disc tribometer by varying the applied load, the sliding velocity, and the sliding distance. The obtained wear results were examined using the multiple regression non-linear model and then the results were validated by performing the confirmation test. It was found that the predicted wear based on the developed regression model was well correlated with the confirmation results. Further, the detailed microstructures of as-received and worn surfaces were examined and reported. In addition, the plotted surface graphs between the input independent parameters and the responses showed that the wear rate started to vary linearly with the function of load whereas it was varied non-linearly with the function of the sliding velocity and the sliding distance.


    1.   Introduction

    In recent years, the non-ferrous alloys have played a major role in the manufacturing of most of the commodity items in which the brass (one of the copper-based alloys) is being applied more. The brass is consisting of copper (Cu) and zinc (Zn) elements [1,2,3,4,5]. The amount of incorporation of Zn element with the Cu has formed several types of brasses with different properties. The major applications of brasses are in the radiator tubes, the hydraulic brake tubes, the journal bearings, the carburetor, the fasteners, and in marine components [3,6,7,8,9]. The properties of brass can be enhanced by cold working which is available in different mechanical properties. Almost all the brasses have been excellent in corrosion resistance, higher in malleability, and have a relatively low melting point. Among the several brasses, the yellow brass has provided superior strength, more ductility and good casting properties which are necessary for the manufacturing of ornaments. Yellow brass is the alloy of 65% Cu and 35% Zn [10]. In real applications, the components made up of brasses have been subjected to mechanical rubbing action which creates abrasive action. Wear is one kind of metal removal from the contacting surfaces due to relative motions between the two mating parts [11,12]. The wear that occurred in the components was mainly based on the applied load, the operating temperature, the speed, the hardness of the alloy, the time duration of mechanical action, the atmospheric action, and the presence of foreign matters. Wear is a foremost issue in the engineering field in which 1 to 4% of gross national product (GNP) has consumed more direct cost during the usage of mechanical parts. Therefore, it is necessary to examine and study the wear behavior of brasses and its alloys [13,14,15].

    Tribological behavior, namely the wear rate, the wear resistance, and the coefficient of friction, has clarified the wear rate of materials which have played a major role in material removal [5,6,7,8,9,16]. This indicates the transfer of material from one surface to another owing to the relative motion between the contacting surfaces. A dry sliding wear test is the best experimental technique for many structural applications to find out the wear behavior. However, to explore in detail the wear behavior of any material, it is necessary to conduct a number of experiments in the conventional one-to-one method. This conventional method has needed more investment, but these issues can be solved by implementing the design and analysis of experimental technique by which the number of experiments can be minimized by considering more input parameters. The response surface methodology (RSM) is a powerful statistical tool employed to understand the performance of the process when multiple parameters are involved [8,9,10,11,12,13,14]. This technique has reduced the experimental time with the minimum number of experiments and it has a series of steps such as the prediction of the response for different combinations of parameters, development of regression coefficients estimation, fitting of the experimental data, prediction of response and checking the adequacy of the fitted model. The analysis can be carried out for a 95% confidence level and 5% significance level to determine the significance of the input parameters employed for the construction of the model [15]. The significance of the regression model, linear, square and interaction terms, as well as the lack of fit, can be known through this analysis. In order to ensure the consistency among the results, the central composite design (CCD) has paved a way by providing 6 numbers of experimental runs with the same process parameter which cannot be done through Box-Behnken, Taguchi and genetic algorithm methods [17,18,19,20,21,22,23,24]. Based on the literature, there was no report and detailed study on the dry sliding wear behavior of yellow brasses. Therefore, the present research work was focused to optimize the essential wear parameters such as the load, the sliding velocity and the sliding distance using central composite design in RSM technique to determine the appropriate input parameters to be used for the brass components/parts in real time applications which minimize the wear.

    2.   Experimental methodology and optimization technique

    2.1.   Yellow brass sample preparation and characterization

    The hot rolled commercial yellow brass was purchased from Kovai Metal Mart (P) Ltd., India. The chemical composition, the physical properties and the mechanical properties of as-received yellow brass are given in Table 1. The as-received yellow brass is a face-centered cubic (FCC) crystal system which usually consists of α-phase (majority) and some amount of β-phase. The microstructural images in as-received condition and the worn surfaces were examined using the FESEM (field emission scanning electron microscope) of S-3400 N, Hitachi, with the EDS (energy dispersive spectrometer) of XFlasj 5010, Bruker. The as-received hot rolled yellow brass was cut into several pieces of ø10 × 30 mm as pins for doing the wear test. Before, the samples were cleaned with acetone and then the samples were polished as per the metallographic procedure. The samples were mounted in a Bakelite thermosetting agent, polished with different SiC grit papers (400,600,800, 1000, 1200, 2000 SiC grits/inch2), and finally, the samples were lapped by 3 μm alumina paste. After polishing, the super finish samples were etched using HNO3 (50 mL) and distilled water (50 mL) to examine the microstructure.

    Table 1.  Physical and mechanical properties of as-received yellow brass.
    Parameter(s) Description(s)
    Chemical composition Cu = 65%, Zn = 35%
    Physical properties Density, ρ 8.42 g/cm3
    Melting point 900 ℃
    Coefficient of thermal expansion 20.1 μm/m ℃
    Thermal conductivity 112 W/mK
    Mechanical properties Hardness (HR30T) 16.5
    Ultimate tensile strength 320 MPa
    Tensile yield strength 105 MPa
    Elongation at failure 62%
    Rockwell hardness 55 HRF
     | Show Table
    DownLoad: CSV

    2.2.   Dry sliding wear test

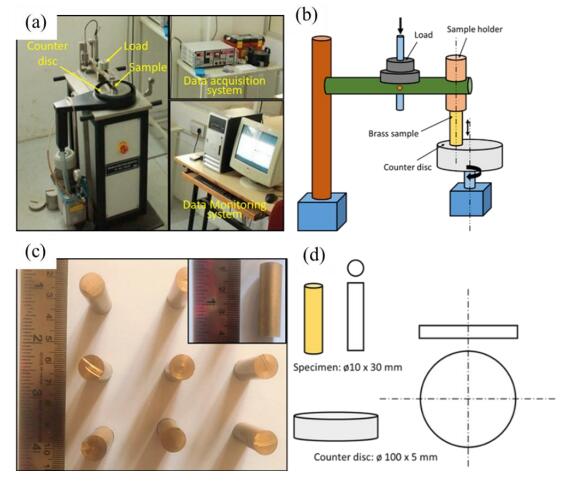
    The pin-on-disc sliding wear test equipment was used to study the tribological behavior of yellow brass under the dry condition at ambient temperature. Figure 1a shows the photograph of the pin-on-disc (supplied by DUCOM, India) wear test apparatus which consists of the counter disc, the sample holder, the sample, the applied load, the data acquisition system, and the data monitoring system. Further, the schematic of the sliding wear test is also shown in Figure 1b. The cylindrical counter disc was made of hardened stainless steel having 5 mm thickness and 100 mm diameter. The counter disc was initially ground and cleaned with acetone to eliminate the presence of oxide layers, foreign particles, and moisture. The prepared samples for wear test were weighed before and after the test by which the mass loss was determined. Based on the mass losses, the wear rate and the volume loss were determined using the following formula:

    Figure 1.  (a) Photograph of sliding wear test apparatus; (b) the schematic of sliding wear test; (c) photograph of yellow brass samples before sliding wear; (d) the dimensions of the counter disc and the pin.
    DownLoad: Full-Size Img PowerPoint
    Wearrate(mm3m)=Volumeloss(mm3)slidingdistance(m)×1000 (1)
    Volumeloss(mm3)=Weightloss(g)Density(g)(cm3) (2)

    2.3.   Design of experiments

    The wear test was conducted by varying the loads (10 N, 30 N and 50 N), the sliding velocities (1 m/s, 3 m/s and 5 m/s), and the sliding distances (500 m, 1500 m, and 2500 m). The experiments were conducted as per the face-centered central composite design (FCCD). The three independent input parameters with three levels were illustrated in Table 2. The photograph of yellow brass samples of before wear is shown in Figure 1c. Minitab (v15) statistical software was used to perform the design of experiments and analyses. In the FCCD, twenty experimental runs were used with three input parameters at three levels. The actual experimental design and the corresponding response are illustrated in Table 3. At least three replicas were used in each input condition and the average was used for the investigation. The analysis of variance (ANOVA) was applied to investigate the significant parameters and the individual parameter's percentage contribution. The input independent parameters (the load, the sliding velocity and the sliding distance) and the corresponding responses (the wear rate, and the coefficient of friction) were correlated by means of second ordered mathematical regression models which are expressed as below:

    Table 2.  Wear parameters and their levels used in pin-on-disc tribometer.
    Parameter Units Notation Level
    Load (N) L 10 30 50
    Velocity (m/s) V 1 3 5
    Sliding distance (m) SD 500 1500 2500
     | Show Table
    DownLoad: CSV
    Table 3.  Design matrix and experimental results on sliding wear of yellow brass.
    Experiment number Load, N Velocity, m/s Distance, m Wear rate, mm3/m Coefficient of friction, μ
    1 30 3 2500 0.002044 0.882
    2 10 5 2500 0.000457 0.917
    3 30 3 500 0.004040 0.829
    4 30 1 1500 0.003701 0.702
    5 30 3 1500 0.002387 0.682
    6 30 3 1500 0.002327 0.678
    7 50 5 500 0.001730 0.952
    8 30 3 1500 0.002332 0.681
    9 50 1 2500 0.003516 0.866
    10 10 1 500 0.003954 0.902
    11 30 3 1500 0.002339 0.679
    12 50 1 500 0.006020 0.867
    13 10 5 500 0.000762 0.939
    14 10 3 1500 0.001461 0.735
    15 30 3 1500 0.002347 0.68
    16 10 1 2500 0.002380 0.853
    17 50 5 2500 0.001192 0.97
    18 30 5 1500 0.000727 0.851
    19 30 3 1500 0.002341 0.679
    20 50 3 1500 0.002914 0.788
     | Show Table
    DownLoad: CSV
    Yu=b+∑biXiu+∑biiX2iu+∑bijXiuXju (3)

    where, Yu is the response, b, bi, bii and bij are the regression model coefficients. The above polynomial equation has indicated the linear effect (second term), square effect (third term), and the interaction effect (third term). The influence of input parameters on the responses was studied through the contour plots which were expected to minimize the wear rate and the coefficient of friction. In the present study, the experimental parameters were chosen based on commercial experiences. Based on the results, the optimum input parameter could be selected by which the wear of the yellow brass components could be diminished in the real dynamic applications.

    3.   Results and discussion

    3.1.   Microstructural examination

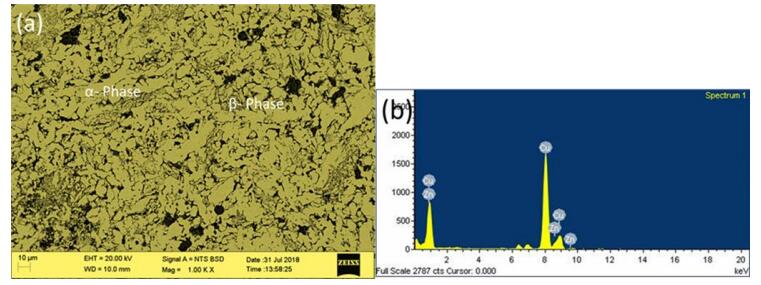
    Before the wear test, the SEM microstructure of as-received and the hot rolled brass are shown in Figure 2. From Figure 2a, more quantity of α-phases were observed and some amount of β-phases which were all related to the yellow brass. The white region indicated the α-phases (primary phase) and the dark grey region indicated the β-phases (secondary phase). The observed grains were almost equiaxed with random oriented ones which further showed the homogeneous nature. Based on several microstructural images, the average grain sizes were calculated for both the α-phase and β-phase. The average grain size of α-Cu phase was around 40 ± 5.3 μm which was calculated from 500 grains. In a similar manner, the average grain size of β-Zn phase was also computed from 200 grains which were around 22 ± 2.6 μm. In fact, the solid crystals with random orientation would have isotropic properties [4]. The energy dispersive spectroscopy (EDS) of the SEM (Figure 2b) also confirmed the presence of copper and zinc phases, and the chemical composition in terms of weight percentage [3,4] has well correlated with Table 1.

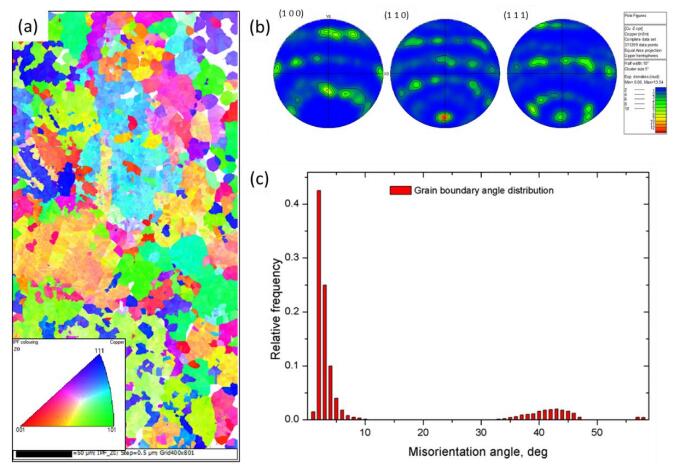
    Figure 2.  SEM microstructure of as-received yellow brass; (b) corresponding EDS.
    DownLoad: Full-Size Img PowerPoint

    The electron backscattered diffraction (EBSD) analyses were also carried out for the as-received yellow brass using FEI Quanta FEG SEM with TSL-OIM software to the colored grain distribution of α-Cu phase and β-Zn phase (Figure 3a). The corresponding inverse pole figure is also shown as inset of Figure 3a at the bottom left corner. Further, Figure 3b shows the pole figure in terms of mean uniform density (MUD) which indicated that one preferred texture orientation was observed in the legend of (1 1 0) that corresponds to {2 2 0} planes. In addition, from Figure 3c, binomial grain misorientation angle distribution (low angle grain boundaries and high-angle grain boundaries) was also observed which was expected in the presence of α-Cu phase and β-Zn phase.

    Figure 3.  (a) EBSD grain coloured maps of as-received yellow brass, inset at the bottom shows the inverse pole figure; (b) the pole figure of (a) indicates the texture orientation; (c) grain misorientation angle distribution.
    DownLoad: Full-Size Img PowerPoint

    3.2.   Investigation on dry sliding wear behavior on yellow brass

    3.2.1.   Influence of input variables on the wear rate

    Table 3 illustrated the wear rate and the coefficient of friction of yellow brass with the function of process parameters. The results revealed that the experiment number 2 exhibited the minimum wear rate of 0.000457 mm3/m with the applied load of 10 N, the sliding velocity of 5 m/s, and the sliding distance of 2500 m. The minimum coefficient of friction of 0.687 was obtained in experiment number 6 with the applied load of 30 N, the sliding velocity of 3 m/s, and the sliding distance of 1500 m. Based on these values, the optimum input parameters could be predicted which are near to the optimum region. However, these parameters would not be the optimum. The developed mathematical non-linear regression model (polynomial) is given in Eq 4 which can be used to predict the wear rate for the given input process parameters.

    Wearrate(mm3/m)=0.00051317+(0.0000942)×Load−(0.0005637)×Velocity−(0.0000029)×Distance−(0.000000624)×Load×Load−(0.0000557)×Velocity×Velocity+(0.000000000605)×Distance×Distance−(0.00000468)×Load×Velocity−(0.00000000728)×Load×Distance+(0.000000202)×Velocity×DistanceR2=98.947%,R2(adj)=98.04% (4)

    The various main factors (the load, the sliding velocity, and the sliding distance) and the corresponding interaction effect were taken for the investigation which was further classified into two categories such as significant factors and non-significant factors. The decision of whether the factor is significant and or non-significant was made based on the P-value of 0.05 which has a confidence level of 95%. If the p-value is less than 0.05, then the corresponding factor would give a major effect on the response which is called significant factor, and otherwise, the particular input parameter is called an insignificant factor. Table 4 illustrated the observed ANOVA results on the wear rate during the sliding wear test on yellow brass which indicated that almost all the linear terms, square terms, and the interaction terms were influenced much on the wear rate. Further, the developed regression model (Eq 4) was the R2 value of 98.947% and the adjusted R2 value of 98.04% which meant the developed model has more accuracy. From Table 4 and the model (Eq 4), among the linear terms, the sliding velocity was influenced much followed by the distance and the load. In a similar manner, among the square terms, only the square of distance was influenced much, little effect was produced by the square of the load, and the square of velocity was not influenced. Further, among the interaction terms, the term "Velocity × Distance" was influenced much followed by the terms of "Load × Velocity" and "Load × Distance".

    Table 4.  ANOVA test results for wear rate during sliding wear test on yellow brass.
    Source DF Seq SS Adj SS Adj MS F P
    Regression 9 0.000033 0.000033 0.000004 106.49 < 0.0001
    Linear terms 3 0.00003 0.00003 0.00001 292.85 < 0.0001
    Load (N) 1 0.000004 0.000004 0.000004 116.64 < 0.0001
    Velocity (m/s) 1 0.000022 0.000022 0.000022 623.84 < 0.0001
    Distance (m) 1 0.000005 0.000005 0.000005 138.08 < 0.0001
    Square terms 3 0.000001 0.000001 0.000000 9.71 0.0030
    Load × Load 1 0.000000 0.000000 0.000000 4.94 0.0510
    Velocity × Velocity 1 0.000000 0.000000 0.000000 3.94 0.0750
    Distance × Distance 1 0.000001 0.000001 0.000001 29.07 0.0000
    Interaction 3 0.000002 0.000002 0.000001 16.92 < 0.0001
    Load × Velocity 1 0.000000 0.000000 0.000000 8.1 0.0170
    Load × Distance 1 0.000000 0.000000 0.000000 4.89 0.0510
    Velocity × Distance 1 0.000001 0.000001 0.000001 37.76 < 0.0001
    Residual Error 10 0.000000 0.000000 0.000000
    Lack-of-Fit 5 0.000000 0.000000 0.000000 151.34 < 0.0001
    Pure Error 5 0.000000 0.000000 0.000000
    Total 19 0.000034
     | Show Table
    DownLoad: CSV

    Figure 4 explained the contour plots of wear rate during the sliding wear test on yellow brass at room temperature. From Figure 4a, the results indicated that the wear rate started to increase gradually when the function of the applied load for any sliding velocity remained constant. These results were attributed to the formation of more thrust forces between the pin (yellow brass) and the counter disc, and consequently, the materials were expected to decrease. However, at the lower load, when the velocity started to increase, the amount of formed thrust force was expected to be less and hence, less wear loss in the materials was expected to occur. In contrast, for instance, at higher load and higher value of sliding velocity, less wear rate was observed which was attributed to the domination of slipping phenomenon [25,26]. In general, as the sliding velocity was increased, the introduced/increased rotational forces in the materials were forced to move the sample in a faster manner, which might be expected to form the slippage called slipping phenomenon that nullified the thrust forces. Hence, less wear rate occurred at high velocity with a high load. The contour plot of Figure 4b drawn between the load and the sliding distance showed the similar behavior of Figure 4a. At a lower value of sliding distance, the wear rate started to increase gradually with the function of load due to the domination of thrust forces.

    Figure 4.  Contour plots of the wear rate of commercial yellow brass with the function of: (a) velocity vs. load; (b) distance vs. load; (c) distance vs. velocity.
    DownLoad: Full-Size Img PowerPoint

    However, at a higher value of sliding distance and at higher load, the observed wear rate increased due to more contact time of the sample over the counter disc and hence, more volume loss occurred. The result of Figure 4c revealed that the observed wear was more at a low velocity which was attributed to more adhesion and more contact time taken by the sample over the counter disc. Due to this, more plastic deformation occurred in the sample which led to more wear loss. However, at high velocity, the contact time between the sample and the counter disc decreased and hence, less wear occurred. According to Archard's law, the applied load and the sliding distance are directly proportional and influence the wear rate. Therefore, in the present work, the wear rate was highly influenced by the function of the load and the distance.

    3.2.2.   Influence of input variables on the coefficient of friction

    The coefficient of friction could be predicted by the following mathematical non-linear regression model which is given in Eq 5.

    Coefficientoffriction(CoF)=1.16854−(0.00657)×Load−(0.06711)×Velocity−(0.000415)×Distance+(0.00009)×Load×Load+(0.01275)×Velocity×Velocity+(0.00000013)×Distance×Distance+(0.000275)×Load×Velocity+(0.00000055)×Load×Distance+(0.00000288)×Velocity×DistanceR2=94.64%,R2(adj)=89.74% (5)

    Table 5 showed the observed ANOVA results on the coefficient of friction during the sliding wear test on yellow brass which indicated that the sliding velocity in the linear term was only highly influenced when compared to the other two linear terms. In the square terms, the square of distance was influenced much followed by the square of velocity. However, the square term of the load was not influenced in the coefficient of friction. Further, no interaction term has not influenced the coefficient of friction. Further, the developed regression model (Eq 5) possessed the R2 value of 94.64% and the adjusted R2 value of 89.74% which meant the developed model had more accuracy.

    Table 5.  ANOVA test results for the coefficient of friction during sliding wear test on yellow brass.
    Source DF Seq SS Adj SS Adj MS F P
    Regression 9 0.205275 0.205275 0.022808 19.47 < 0.0001
    Linear terms 3 0.020213 0.020213 0.006738 5.75 0.0150
    Load (N) 1 0.000941 0.000941 0.000941 0.8 0.3910
    Velocity (m/s) 1 0.019272 0.019272 0.019272 16.45 0.0020
    Distance (m) 1 0.000000 0.000000 0.000000 0.000 0.9930
    Square terms 3 0.182861 0.182861 0.060954 52.03 < 0.0001
    Load × Load 1 0.104546 0.003564 0.003564 3.04 0.112
    Velocity × Velocity 1 0.03184 0.007153 0.007153 6.11 0.033
    Distance × Distance 1 0.046475 0.046475 0.046475 39.67 < 0.0001
    Interaction 3 0.002201 0.002201 0.000734 0.63 0.614
    Load × Velocity 1 0.000968 0.000968 0.000968 0.83 0.385
    Load × Distance 1 0.000968 0.000968 0.000968 0.83 0.385
    Velocity × Distance 1 0.000265 0.000265 0.000265 0.23 0.645
    Residual Error 10 0.011716 0.011716 0.001172
    Lack-of-Fit 5 0.011705 0.011705 0.002341 1080.5 < 0.0001
    Pure Error 5 0.000011 0.000011 0.000002
    Total 19 0.000034
     | Show Table
    DownLoad: CSV

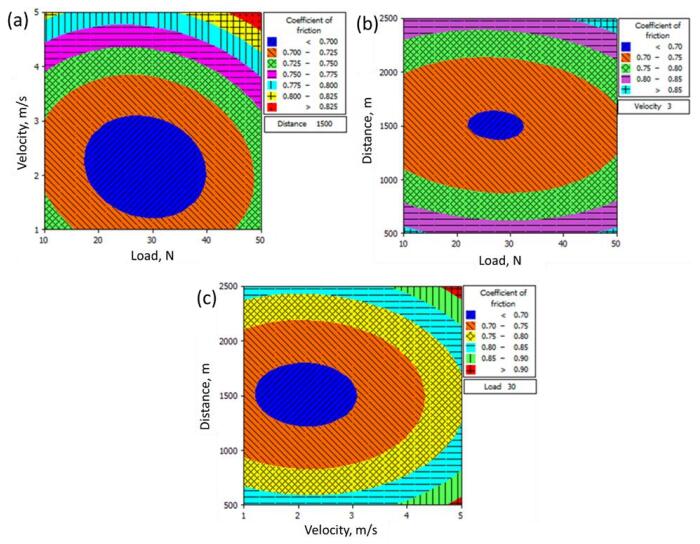
    Figure 5 showed the contour plot of the coefficient of friction with the function of the applied load, the sliding velocity, and the sliding distance. The result of Figure 5a explained that the average range of coefficient of friction value (0.700 to 0.725) was observed at the lower value of the applied load and the lower value of sliding velocity. At the middle value of the load, the observed coefficient of friction was low (<0.7). This meant the value of the coefficient of friction started to decrease with the function of the load up to the middle one which was attributed to less frictional force occurring. However, when the applied load and the sliding velocity increased further, the coefficient of friction increased due to a higher amount of thrust force which was caused by the increase of the load, and hence, a more frictional force due to heavy contact of the sample over the counter disc occurred. The result of Figure 5b demonstrated that the observed coefficient of friction value increased with the function of the load and the sliding distance. These results were attributed to more material contact with the disc and more time. This more material contact was expected to produce more plastic deformation which led to increasing the frictional forces. Similar behavior was observed in the result of Figure 5c.

    Figure 5.  Contour plots of the coefficient of commercial yellow brass with the function of (a) velocity vs. load; (b) distance vs. load; (c) distance vs. velocity.
    DownLoad: Full-Size Img PowerPoint

    Figure 6 depicted the normal probability plot of dry sliding wear behavior of yellow brass. The plot demonstrated that the maximum number of the residuals has coincided over the inclined fitted line which explained clearly the strong correlation between the model's prediction and the experimental one.

    Figure 6.  The measured and the predicted values on dry sliding wear behavior of yellow brass: (a) wear rate; (b) the coefficient of friction.
    DownLoad: Full-Size Img PowerPoint
    3.2.3.   Confirmatory test

    Table 6 illustrated the measured, the predicted results of the wear rate, and the coefficient of friction which was computed based on the confirmatory test and the developed mathematical polynomial model. From Table 6, it was clearly shown that all the predicted values were much closer to the experimental ones with a 95% confidence level.

    Table 6.  Confirmation experiment and regression wear rate.
    Load (N) Velocity (m/s) Sliding distance (m) Wear rate (mm3/m) Coefficient of friction
    Experimental Wear rate (mm3/m) Regression Wear rate (mm3/m) Error (%) Experimental Wear rate (mm3/m) Regression Wear rate (mm3/m) Error (%)
    15 2 750 0.003153 0.003300 4.655 0.7734 0.78765 1.85
    15 2 900 0.002893 0.003059 5.74 0.7401 0.7596 2.65
    10 4 750 0.001490 0.001394 6.40 0.8012 0.8330 3.97
     | Show Table
    DownLoad: CSV
    3.2.4.   Optimization of the responses

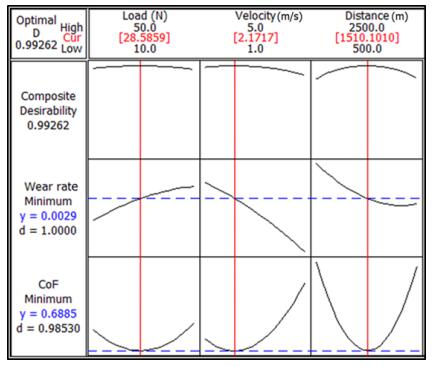
    The main feature of implementing the response surface methodology is that the optimization of all the responses based on the input parameters can be carried out. It is well known that the wear rate and the coefficient of friction mainly influenced the applied sliding parameters in the real-time applications based on which the service life of the components could be designed. It is necessary to do the optimization when we want to increase the service life the components. In the present research work, the wear rate and the coefficient of friction were optimized using the response optimization tool in the Minitab software. Both of these two responses were minimized, and hence, the target values were fixed based on minimum criteria function. Figure 7 showed the optimized plot of the two responses. From Figure 7, the optimum input parameters were the applied load of 28.5859 N, the sliding velocity of 2.1717 m/s, and the sliding distance of 1510.1010 m with the desirability of 0.99262.

    Figure 7.  Optimal conditions of process parameters during sliding wear test on yellow brass.
    DownLoad: Full-Size Img PowerPoint

    3.3.   Worn surface morphology

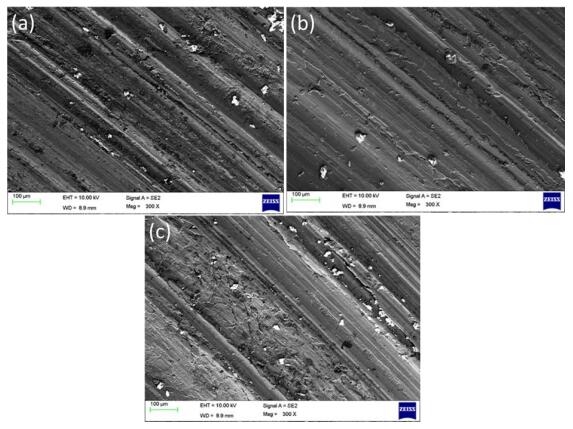
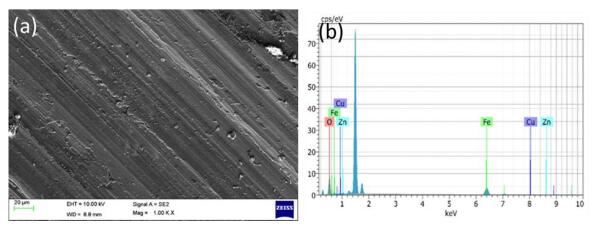
    Figure 8 showed the worn surface morphology of the sample with the function of load. It was clearly known that a lesser amount of cutting effect, the abrasion effect, and the plowing effect were observed when the load was 10 N. However, when the load started to increase, more amount of cutting lines, abrasion effect, and the plowing effect was formed due to more contact of the sample with the counter disc which was expected to introduce more friction [27,28]. Therefore, more plastic deformation has occurred at higher load which produced more damages in the sample. Figure 9 showed the worn surface morphology of the sample with the function of the sliding velocity and the distance. At a lower load and lower value of sliding velocity, severe debris, scratches, and plowing were observed in the sample due to more sample contact time with the counter disc. However, as the velocity increased, a good surface morphology was obtained. This result was attributed to the fact that the sample contact time started to decrease as the velocity increased which led to producing less wear [25,26]. These results were clearly seen in Figure 9b, c. Figure 10 showed the worn surface morphology of the optimized sample which was a good surface with little damages like the scratches, plowing, pitting, debris, etc. The energy spectroscopy of the optimized sample was also shown in Figure 10b which confirmed the presence of alloy system and some iron oxide particles which were expected to form from the chemical composition of the counter disc.

    Figure 8.  The worn surface morphology of dry sliding wear behavior of yellow brass with the function of the applied load: (a) load = 10 N, velocity = 1 m/s, distance = 500 m, Exp.No.10; (b) load = 30 N, velocity = 1 m/s, distance = 1500 m, Exp.No.4; (c) load = 50 N, velocity = 1 m/s, distance = 500 m, Exp.No.12.
    DownLoad: Full-Size Img PowerPoint
    Figure 9.  The worn surface morphology of dry sliding wear behavior of yellow brass with the function of the sliding velocity: (a) load = 10 N, velocity = 1 m/s, distance = 2500 m, Exp.No.16; (b) load = 10 N, velocity = 3 m/s, distance = 1500 m, Exp.No.14; (c) load = 10 N, velocity = 5 m/s, distance = 500 m, Exp.No.13.
    DownLoad: Full-Size Img PowerPoint
    Figure 10.  Worn surface morphology at the optimum condition with corresponding EDS analysis.
    DownLoad: Full-Size Img PowerPoint

    4.   Conclusions

    The sliding wear test on the commercial yellow brass was successfully conducted and the optimum process parameters were determined. From the present study, the following conclusions were drawn.

    ● The empirical relationship between the process parameters and the responses using the face-centered composite design was developed which can be used to examine the factor of the load, the sliding velocity and sliding distance on the wear rate and the coefficient of friction.

    ● The present research work can be used to predict the wear rate and the coefficient of friction at 5% significant level.

    ● The optimum value of wear rate was achieved at the applied load of 28.5859 N, the sliding velocity of 2.1717 m/s, and the sliding distance of 1510.1010 m with the desirability of 0.99262.

    ● Investigations with FESEM and EDAX had ensured the presence of some iron oxide particles in the base metal which might be expected to form from the counter disc.

    ● ANOVA results exposed that the sliding distance has a greater impact on the wear rate.

    Acknowledgments

    The corresponding author wishes to thank the Qassim University for all funding and support required to carry out this research.

    Conflicts of interest

    The author declares no conflict of interest.



    [1] Bénard AEB, Hernández DM, Reyes JGG, et al. (2014) Synthesis, characterization and cold workability of cast copper-magnesium-tin alloys. Metall Mater Trans A 45: 555–562.
    [2] Taha MA, El-Mahallawy NA, Hammouda RM, et al. (2012) Machinability characteristics of lead free-silicon brass alloys as correlated with microstructure and mechanical properties. Ain Shams Eng J 3: 383–392. doi: 10.1016/j.asej.2012.05.004
    [3] Kong XL, Qiao LJ, Liu YB (2003) Wear behavior of nanocrystalline Cu-Zn alloy. J Mater Eng Perform 12: 312–316. doi: 10.1361/105994903770343169
    [4] Sivasankaran S, Alaboodi AS, Al-Mufadi F (2018) Cold deformation of dezincification resistant yellow brass for plumbing applications. Mater Manuf Process 33: 1693–1700. doi: 10.1080/10426914.2018.1453147
    [5] Neishi K, Horita Z, Langdon TG (2001) Achieving superplasticity in a Cu–40% Zn alloy through severe plastic deformation. Scripta Mater 45: 965–970. doi: 10.1016/S1359-6462(01)01119-8
    [6] Zhang ZJ, An XH, Zhang P, et al. (2013) Effects of dislocation slip mode on high-cycle fatigue behaviors of ultrafine-grained Cu–Zn alloy processed by equal-channel angular pressing. Scripta Mater 68: 389–392. doi: 10.1016/j.scriptamat.2012.10.036
    [7] Vinogradov A, Vasilev E, Seleznev M, et al. (2016) On the limits of acoustic emission detectability for twinning. Mater Lett 183: 417–419. doi: 10.1016/j.matlet.2016.07.063
    [8] Tarasov SY, Filippov AV, Kolubaev EA, et al. (2017) Adhesion transfer in sliding a steel ball against an aluminum alloy. Tribol Int 115: 191–198. doi: 10.1016/j.triboint.2017.05.039
    [9] Filippov AV, Nikonov AY, Rubtsov VE, et al. (2017) Vibration and acoustic emission monitoring the stability of peakless tool turning: Experiment and modeling. J Mater Process Tech 246: 224–234. doi: 10.1016/j.jmatprotec.2017.03.030
    [10] Kato H, Todaka Y, Umemoto M, et al. (2015) Sliding wear behavior of sub-microcrystalline pure iron produced by high-pressure torsion straining. Wear 336–337: 58–68.
    [11] Konkova T, Mironov S, Korznikov A, et al. (2015) An EBSD investigation of cryogenically-rolled Cu–30% Zn brass. Mater Charact 101: 173–179. doi: 10.1016/j.matchar.2015.02.004
    [12] Kalita K, Shivakoti I, Ghadai RK (2017) Optimizing process parameters for laser beam micro-marking using genetic algorithm and particle swarm optimization. Mater Manuf Process 32: 1101–1108. doi: 10.1080/10426914.2017.1303156
    [13] Shah KB, Kumar S, Dwivedi DK (2007) Aging temperature and abrasive wear behaviour of cast Al–(4%, 12%, 20%) Si–0.3% Mg alloys. Mater Design 28: 1968–1974.
    [14] Tang C, Wang JM, Wen GW, et al. (2011) Bauschinger effect in wear of Cu–40Zn alloy and its variations with the wear condition. Wear 271: 1237–1243. doi: 10.1016/j.wear.2010.11.026
    [15] Mousavi SE, Meratian M, Rezaeian A (2017) Investigation of mechanical properties and fracture surfaces of dual-phase 60–40 brass alloy processed by warm equal-channel angular pressing. J Mater Sci 52: 8041–8051. doi: 10.1007/s10853-017-1006-9
    [16] Kim HS, Kim WY, Song KH (2012) Effect of post-heat-treatment in ECAP processed Cu–40% Zn brass. J Alloy Compd 536: S200–S203. doi: 10.1016/j.jallcom.2011.11.079
    [17] Seeman M, Ganesan G, Karthikeyan R, et al. (2010) Study on tool wear and surface roughness in machining of particulate aluminum metal matrix composite-response surface methodology approach. Int J Adv Manuf Tech 48: 613–624. doi: 10.1007/s00170-009-2297-z
    [18] Gupta MK, Sood PK, Sharma VS (2016) Machining parameters optimization of titanium alloy using response surface methodology and particle swarm optimization under minimum-quantity lubrication environment. Mater Manuf Process 31: 1671–1682. doi: 10.1080/10426914.2015.1117632
    [19] Kiaee N, Aghaie-Khafri M (2014) Optimization of gas tungsten arc welding process by response surface methodology. Mater Design 54: 25–31. doi: 10.1016/j.matdes.2013.08.032
    [20] Vidyarthy RS, Dwivedi DK, Muthukumaran V (2018) Optimization of A-TIG process parameters using response surface methodology. Mater Manuf Process 33: 709–717. doi: 10.1080/10426914.2017.1303154
    [21] Olivares EAG, Díaz VMV (2018) Study of the hot-wire TIG process with AISI-316L filler material, analysing the effect of magnetic arc blow on the dilution of the weld bead. Weld Int 32: 139–148. doi: 10.1080/09507116.2017.1347327
    [22] Bawazer LA, Ihli J, Comyn TP, et al. (2015) Genetic algorithm-Guided discovery of additive combinations that direct quantum dot assembly. Adv Mater 27: 223–227. doi: 10.1002/adma.201403185
    [23] Nagaraju S, Vasantharaja P, Chandrasekhar N, et al. (2016) Optimization of welding process parameters for 9Cr-1Mo steel using RSM and GA. Mater Manuf Process 31: 319–327. doi: 10.1080/10426914.2015.1025974
    [24] Radhika N, Raghu R (2018) Prediction of mechanical properties and modeling on sliding wear behavior of LM25/TiC composite using response surface methodology. Particul Sci Technol 36: 104–111. doi: 10.1080/02726351.2016.1223773
    [25] Nageswaran G, Natarajan S, Ramkumar KR (2018) Synthesis, structural characterization, mechanical and wear behaviour of Cu-TiO2-Gr hybrid composite through stir casting technique. J Alloy Compd 768: 733–741. doi: 10.1016/j.jallcom.2018.07.288
    [26] Ramkumar KR, Sivasankaran S, Alaboodi AS (2017) Effect of alumina content on microstructures, mechanical, wear and machining behavior of Cu-10Zn nanocomposite prepared by mechanical alloying and hot-pressing. J Alloy Compd 709: 129–141. doi: 10.1016/j.jallcom.2017.03.153
    [27] Thankachan T, Prakash KS, Loganathan M (2018) WEDM process parameter optimization of FSPed copper-BN composites. Mater Manuf Process 33: 350–358. doi: 10.1080/10426914.2017.1339311
    [28] Jeyaprakash N, Duraiselvam M, Aditya SV (2018) Numerical modeling of WC-12% Co laser alloyed cast iron in high temperature sliding wear condition using response surface methodology. Surf Rev Lett 25: 1950009. doi: 10.1142/S0218625X19500094

  • This article has been cited by:

    1. M. Seyf Eddine Bougoffa, M. Nabil Bachir bey, Chahrazed Benouali, T. Sayah, Mamoun Fellah, Mohammed Abdul Samad, Dry Sliding Friction and Wear Behavior of CuZn39Pb2 and AA7075 Under Industrial and Laboratory Conditions, 2021, 7, 2198-4220, 10.1007/s40735-021-00475-x
    2. ALİ KAYA GUR, CETİN OZAY, BUSRA ICEN, EVALUATİON OF B4C/Tİ COATİNG LAYER, İNVESTİGATİON OF ABRASİVE WEAR BEHAVİORS USİNG TAGUCHİ TECHNİQUE AND RESPONSE SURFACE METHODOLOGY, 2020, 27, 0218-625X, 1950225, 10.1142/S0218625X19502251
    3. Hany R. Ammar, Subbarayan Sivasankaran, Abdulaziz S. Alaboodi, The influence of ball milling processing variables on the microstructure and compaction behavior of Fe–Mn–Cu alloys, 2021, 39, 2083-134X, 410, 10.2478/msp-2021-0033
    4. M. Seyf Eddine Bougoffa, Sayhia Benchaa, Yamina Benkrima, Abdelhak Hadibi, Ouissem Benmesbah, Sihem Benkhaled, An Innovative Evaluation of the Friction and Wear Behavior of Ferrous and Non-Ferrous Metals Under Lab and Lathe Wear Tests, 2024, 2250-2122, 10.1007/s40033-024-00820-7
  • Reader Comments
  • © 2019 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)
通讯作者: 陈斌, bchen63@163.com
  • 1. 

    沈阳化工大学材料科学与工程学院 沈阳 110142

  1. 本站搜索
  2. 百度学术搜索
  3. 万方数据库搜索
  4. CNKI搜索
AIMS Materials Science
1.4 3.6

Metrics

Article views(4470) PDF downloads(813) Cited by(4)

Preview PDF
Download XML
Export Citation
Article outline
Abstract
   Introduction
   Experimental methodology and optimization technique
   Results and discussion
   Conclusions
Acknowledgments
Conflicts of interest
References

Figures and Tables

Figures(10)  /  Tables(6)

Other Articles By Authors

Related pages

Tools

Copyright © AIMS Press

/

DownLoad:  Full-Size Img  PowerPoint
Return
Return

Catalog