Figure 1.
An example of face degree.
Citation: Emmanuel Reyes-Uribe, Nathalia Serna-Marquez, Eduardo Perez Salazar. DDRs: receptors that mediate adhesion, migration and invasion in breast cancer cells[J]. AIMS Biophysics, 2015, 2(3): 303-317. doi: 10.3934/biophy.2015.3.303
| [1] | Huilin Ge, Yuewei Dai, Zhiyu Zhu, Biao Wang . Robust face recognition based on multi-task convolutional neural network. Mathematical Biosciences and Engineering, 2021, 18(5): 6638-6651. doi: 10.3934/mbe.2021329 |
| [2] | Chii-Dong Ho, Gwo-Geng Lin, Thiam Leng Chew, Li-Pang Lin . Conjugated heat transfer of power-law fluids in double-pass concentric circular heat exchangers with sinusoidal wall fluxes. Mathematical Biosciences and Engineering, 2021, 18(5): 5592-5613. doi: 10.3934/mbe.2021282 |
| [3] | José M. Sigarreta . Extremal problems on exponential vertex-degree-based topological indices. Mathematical Biosciences and Engineering, 2022, 19(7): 6985-6995. doi: 10.3934/mbe.2022329 |
| [4] | Qingqun Huang, Muhammad Labba, Muhammad Azeem, Muhammad Kamran Jamil, Ricai Luo . Tetrahedral sheets of clay minerals and their edge valency-based entropy measures. Mathematical Biosciences and Engineering, 2023, 20(5): 8068-8084. doi: 10.3934/mbe.2023350 |
| [5] | Hao Wang, Guangmin Sun, Kun Zheng, Hui Li, Jie Liu, Yu Bai . Privacy protection generalization with adversarial fusion. Mathematical Biosciences and Engineering, 2022, 19(7): 7314-7336. doi: 10.3934/mbe.2022345 |
| [6] | Meili Tang, Qian Pan, Yurong Qian, Yuan Tian, Najla Al-Nabhan, Xin Wang . Parallel label propagation algorithm based on weight and random walk. Mathematical Biosciences and Engineering, 2021, 18(2): 1609-1628. doi: 10.3934/mbe.2021083 |
| [7] | Xinmei Liu, Xinfeng Liang, Xianya Geng . Expected Value of Multiplicative Degree-Kirchhoff Index in Random Polygonal Chains. Mathematical Biosciences and Engineering, 2023, 20(1): 707-719. doi: 10.3934/mbe.2023032 |
| [8] | Fengwei Li, Qingfang Ye, Juan Rada . Extremal values of VDB topological indices over F-benzenoids with equal number of edges. Mathematical Biosciences and Engineering, 2023, 20(3): 5169-5193. doi: 10.3934/mbe.2023240 |
| [9] | Stefano Cosenza, Paolo Crucitti, Luigi Fortuna, Mattia Frasca, Manuela La Rosa, Cecilia Stagni, Lisa Usai . From Net Topology to Synchronization in HR Neuron Grids. Mathematical Biosciences and Engineering, 2005, 2(1): 53-77. doi: 10.3934/mbe.2005.2.53 |
| [10] | Qiming Li, Tongyue Tu . Large-pose facial makeup transfer based on generative adversarial network combined face alignment and face parsing. Mathematical Biosciences and Engineering, 2023, 20(1): 737-757. doi: 10.3934/mbe.2023034 |
To exemplify the phenomena of compounds scientifically, researchers utilize the contraption of the diagrammatic hypothesis, it is a well-known branch of geometrical science named graph theory. This division of numerical science provides its services in different fields of sciences. The particular example in networking [1], from electronics [2], and for the polymer industry, we refer to see [3]. Particularly in chemical graph theory, this division has extra ordinary assistance to study giant and microscope-able chemical compounds. For such a study, researchers made some transformation rules to transfer a chemical compound to a discrete pattern of shapes (graph). Like, an atom represents as a vertex and the covalent bonding between atoms symbolized as edges. Such transformation is known as molecular graph theory. A major importance of this alteration is that the hydrogen atoms are omitted. Some chemical structures and compounds conversion are presented in [4,5,6].
In cheminformatics, the topological index gains attraction due to its implementations. Various topological indices help to estimate a bio-activity and physicochemical characteristics of a chemical compound. Some interesting and useful topological indices for various chemical compounds are studied in [3,7,8]. A topological index modeled a molecular graph or a chemical compound into a numerical value. Since 1947, topological index implemented in chemistry [9], biology [10], and information science [11,12]. Sombor index and degree-related properties of simplicial networks [13], Nordhaus–Gaddum-type results for the Steiner Gutman index of graphs [14], Lower bounds for Gaussian Estrada index of graphs [15], On the sum and spread of reciprocal distance Laplacian eigenvalues of graphs in terms of Harary index [16], the expected values for the Gutman index, Schultz index, and some Sombor indices of a random cyclooctane chain [17,18,19], bounds on the partition dimension of convex polytopes [20,21], computing and analyzing the normalized Laplacian spectrum and spanning tree of the strong prism of the dicyclobutadieno derivative of linear phenylenes [22], on the generalized adjacency, Laplacian and signless Laplacian spectra of the weighted edge corona networks [23,24], Zagreb indices and multiplicative Zagreb indices of Eulerian graphs [25], Minimizing Kirchhoff index among graphs with a given vertex bipartiteness, [26], asymptotic Laplacian energy like invariant of lattices [27]. Few interesting studies regarding the chemical graph theory can be found in [28,29,30,31,32].
Recently, the researchers of [33] introduced a topological descriptor and called the face index. Moreover, the idea of computing structure-boiling point and energy of a structure, motivated them to introduced this parameter without heavy computation. They computed these parameters for different models compare the results with previous literature and found approximate solutions with comparatively less computations. This is all the blessings of face index of a graph. The major concepts of this research work are elaborated in the given below definitions.
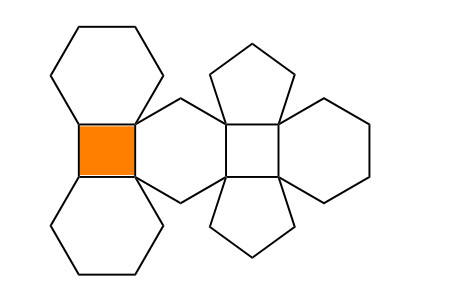
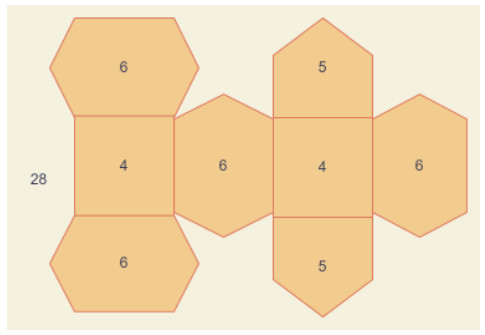
Definition 1.1. [33] Let a graph $ G = \left(V(G), E(G), F(G)\right) $ having face, edge and vertex sets notation with $ F(G), E(G), V(G), $ respectively. It is mandatory that the graph is connected, simple and planar. If $ e $ from the edge set $ E(G), $ is one of those edges which surrounds a face, then the face $ f $ from the face set $ F(G), $ is incident to the edge $ e. $ Likewise, if a vertex $ \alpha $ from the vertex set $ V(G) $ is at the end of those incident edges, then a face $ f $ is incident to that vertex. This face-vertex incident relation is symbolized here by the notation $ \alpha\sim f. $ The face degree of $ f $ in $ G $ is described as $ d(f) = \sum_{\alpha\sim f}{d\left(\alpha\right)}, $ which are elaborated in the Figure 1.
Definition 1.2. [33] The face index $ FI\left(G\right), $ for a graph $ G, $ is formulated as
|
$ FI(G)=∑f∈F(G)d(f)=∑α∼f,f∈F(G)d(α). $
|
In the Figure 1, we can see that there are two faces with degree $ 4, $ exactly two with five count and four with count of 6. Moreover, there is an external face with count of face degree $ 28, $ which is the count of vertices.
As the information given above that the face index is quite new and introduced in the year 2020, so there is not so much literature is available. A few recent studies on this topic are summarized here. A chemical compound of silicon carbides is elaborated with such novel definition in [34]. Some carbon nanotubes are discussed in [35]. Except for the face index, there are distance and degree-based graphical descriptors available in the literature. For example, distance-based descriptors of phenylene nanotube are studied in [36], and in [37] titania nanotubes are discussed with the same concept. Star networks are studied in [38], with the concept of degree-based descriptors. Bounds on the descriptors of some generalized graphs are discussed in [39]. General Sierpinski graph is discussed in [40], in terms of different topological descriptor aspects. The study of hyaluronic acid-doxorubicin ar found in [41], with the same concept of the index. The curvilinear regression model of the topological index for the COVID-19 treatment is discussed in [42]. For further reading and interesting advancements of topological indices, polynomials of zero-divisor structures are found in [43], zero divisor graph of commutative rings [44], swapped networks modeled by optical transpose interconnection system [45], metal trihalides network [46], some novel drugs used in the cancer treatment [47], para-line graph of Remdesivir used in the prevention of corona virus [48], tightest nonadjacently configured stable pentagonal structure of carbon nanocones [49]. In order to address a novel preventive category (P) in the HIV system known as the HIPV mathematical model, the goal of this study is to offer a design of a Morlet wavelet neural network (MWNN) [50].
In the next section, we discussed the newly developed face index or face-based index for different chemical compounds. Silicate network, triangular honeycomb network, carbon sheet, polyhedron generalized sheet, and generalized chain of silicate network are studied with the concept of the face-based index. Given that the face index is more versatile than vertex degree-based topological descriptors, this study will aid in understanding the structural characteristics of chemical networks. Only the difficulty authors will face to compute the face degree of a generalized network or structure, because it is more generalized version and taking degree based partition of edges into this umbrella of face index.
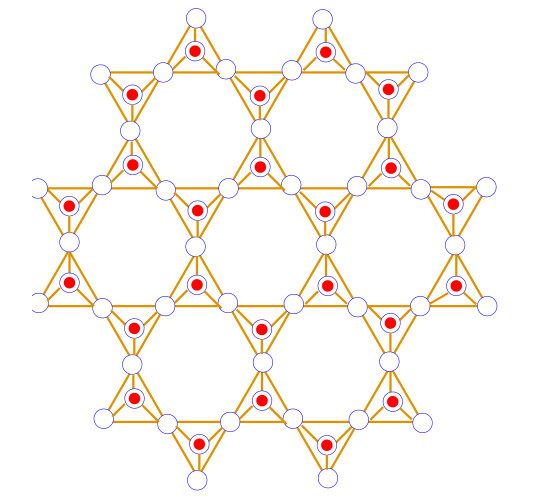
Silicates are formed when metal carbonates or metal oxides react with sand. The $ SiO_4, $ which has a tetrahedron structure, is the fundamental chemical unit of silicates. The central vertex of the $ SiO_4 $ tetrahedron is occupied by silicon ions, while the end vertices are occupied by oxygen ions [51,52,53]. A silicate sheet is made up of rings of tetrahedrons that are joined together in a two-dimensional plane by oxygen ions from one ring to the other to form a sheet-like structure. The silicate network $ SL_{n} $ symbol, where $ {n} $ represents the total number of hexagons occurring between the borderline and center of the silicate network $ SL_{n}. $ The silicate network of dimension one is depicted in Figure 2. It contain total $ 3{n}\left(5{n}+1\right) $ vertices are $ 36{n}^2 $ edges. Moreover, the result required is detailed are available in Table 1.
| Dimension | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{12}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{15}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{36}}}{\bf{|}} $ |
| $ 1 $ | $ 24 $ | $ 48 $ | $ 7 $ |
| $ 2 $ | $ 32 $ | $ 94 $ | $ 14 $ |
| $ 3 $ | $ 40 $ | $ 152 $ | $ 23 $ |
| $ 4 $ | $ 48 $ | $ 222 $ | $ 34 $ |
| $ 5 $ | $ 56 $ | $ 304 $ | $ 47 $ |
| $ 6 $ | $ 64 $ | $ 398 $ | $ 62 $ |
| $ 7 $ | $ 72 $ | $ 504 $ | $ 79 $ |
| $ 8 $ | $ 80 $ | $ 622 $ | $ 98 $ |
| . | . | . | . |
| . | . | . | . |
| . | . | . | . |
| $ {n} $ | $ 8{n}+16 $ | $ 6{n}^{2}+28{n}+14 $ | $ {n}^{2}+4{n}+2 $ |
DownLoad:
CSV
Theorem 2.1. Let $ SL_{n} $ be the silicate network of dimension $ n\geq1. $ Then the face index of $ SL_{n} $ is
|
$ FI(SLn)=126n2+720n+558. $
|
Proof. Consider $ SL_{n} $ the graph of silicate network with dimension $ {n}. $ Suppose $ f_{i} $ denotes the faces of graph $ SL_{n} $ having degree $ i. $ that is, $ d(f_{i}) = \sum_{\alpha\sim f_{i}}{d\left(\alpha\right)} = i $ and $ |f_{i}| $ denotes the number of faces with degree $ i. $ The graph $ SL_{n} $ contains three types of internal faces $ f_{12}, $ $ f_{15}, $ $ f_{36}, $ and single external face which is usually denoted by $ f^{\infty}. $
If $ SL_{n} $ has one dimension then sum of degree of vertices incident to the external face is $ 144 $ and when $ SL_{n} $ has two dimension then sum of degree of incident vertices to the external face is $ 204 $ whenever $ SL_{n} $ has three dimension then sum of degree of incident vertices to the external face is $ 264. $ Similarly, $ SL_{n} $ has $ n- $dimension then sum of degree of incident vertices to the external face is $ 60n+84. $
The number of internal faces with degree in each dimension is mentioned in Table 1.
By using the definition of face index $ FI $ we have
|
$ FI(SLn)=∑α∼f∈F(SLn)d(α)=∑α∼f12∈F(SLn)d(α)+∑α∼f15∈F(SLn)d(α)+∑α∼f36∈F(SLn)d(α)+∑α∼f∞∈F(SLn)d(α)=|f12|(12)+|f15|(15)+|f36|(36)+(60n+84)=(8n+16)(12)+(6n2+28n+14)(15)+(n2+4n+2)(36)+60n+84=126n2+72n+558. $
|
Hence, this is our required result.
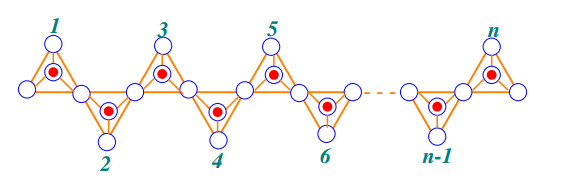
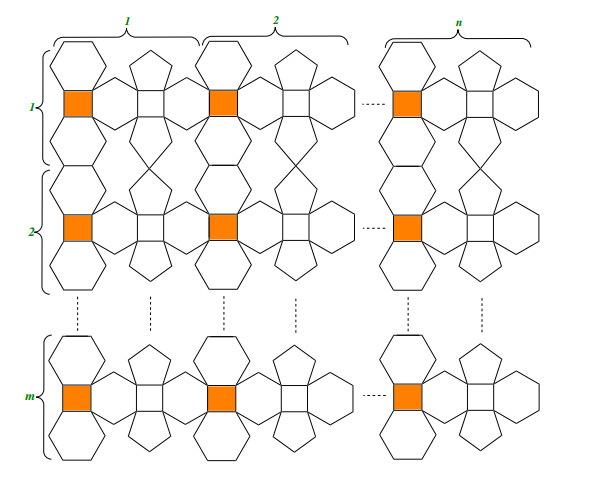
A chain silicate network of dimension $ (m, n) $ is symbolized as $ CSL\left(m, n\right) $ which is made by arranging $ (m, n) $ tetrahedron molecules linearly. A chain silicate network of dimension $ (m, n) $ with $ m, n\geq1 $ where $ m $ denotes the number of rows and each row has $ n $ number of tetrahedrons. The following theorem formulates the face index $ FI $ for chain silicate network.
Theorem 2.2. Let $ CSL\left(m, n\right) $ be the chain of silicate network of dimension $ m, n\geq1. $ Then the face index $ FI $ of the graph $ CSL\left(m, n\right) $ is
|
$ FI(CSL(m,n))={48n−12if m=1, n≥1;96m−12if n=1, m≥2;168m−60if n=2,m≥2;45m−9n+36mn−42if both m,n are even45m−9n+36mn−21otherwise. $
|
Proof. Let $ CSL\left(m, n\right) $ be the graph of chain silicate network of dimension $ (m, n) $ with $ m, n\geq1 $ where $ m $ represents the number of rows and $ n $ is the number of tetrahedrons in each row. A graph $ CSL\left(m, n\right) $ for $ m = 1 $ contains three type of internal faces $ f_{9}, \ f_{12} $ and $ f_{15} $ with one external face $ f^{\infty}. $ While for $ m\geq2, $ it has four type of internal faces $ f_{9}, \ f_{12}, \ f_{15} $ and $ f_{36} $ with one external face $ f^{\infty}. $ We want to evaluate the algorithm of face index $ FI $ for chain silicate network. We will discuss it in two different cases.
Case 1: When $ CSL\left(m, n\right) $ has one row $ (m = 1) $ with $ n $ number of tetrahedrons as shown in the Figure 3.
The graph has three type of internal faces $ f_{9}, \ f_{12} $ and $ f_{15} $ with one external face $ f^{\infty}. $ The sum of degree of incident vertices to the external face is $ 9n $ and number of faces are $ |f_{9}| = 2, \ |f_{12}| = 2n $ and $ |f_{15}| = n-2. $ Now the face index $ FI $ of the graph $ CSL\left(m, n\right) $ is given by
|
$ FI(CSL(m,n))=∑α∼f∈F(CSL(m,n))d(α)=∑α∼f9∈F(CSL(m,n))d(α)+∑α∼f12∈F(CSL(m,n))d(α)+∑α∼f15∈F(CSL(m,n))d(α)+∑α∼f∞∈F(CSL(m,n))d(α)=|f9|(9)+|f12|(12)+|f15|(15)+(9n)=(2)(9)+(2n)(12)+(n−2)(15)+9n=48n−12. $
|
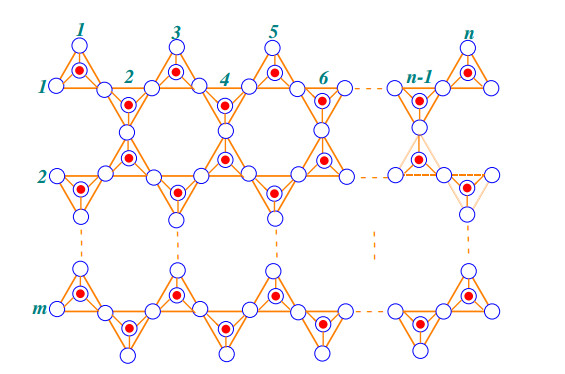
Case 2: When $ CSL\left(m, n\right) $ has more than one rows $ (m\neq1) $ with $ n $ number of tetrahedrons in each row as shown in the Figure 4.
The graph has four type of internal faces $ f_{9}, \ f_{12}, \ f_{15} $ and $ f_{36} $ with one external face $ f^{\infty}. $ The sum of degree of incident vertices to the external face is
|
$ ∑α∼f∞∈F(CSL(m,n))d(α)={18mif n=1, m≥1;27mif n=2, m≥1;30m+15n−30if both m,n are even30m+15n−33otherwise. $
|
The number of faces are $ |f_{9}|, \ |f_{12}|, \ f_{15} $ and $ |f_{36}| $ are given by
|
$ |f9|={2if m is odd3+(−1)nif m is even.|f12|={2(2m+n−1)if m is odd4(⌊n+12⌋+2m−1)if m is even|f15|=(3m−2)n−m|f36|={(m−12)(n−1)if m is odd(2n+(−1)n−14)(m−22)nif m is even. $
|
Now the face index $ FI $ of the graph $ CSL\left(m, n\right) $ is given by
|
$ FI(CSL(m,n))=∑α∼f∈F(CSL(m,n))d(α)=∑α∼f9∈F(CSL(m,n))d(α)+∑α∼f12∈F(CSL(m,n))d(α)+∑α∼f15∈F(CSL(m,n))d(α)+∑α∼f36∈F(CSL(m,n))d(α)+∑α∼f∞∈F(CSL(m,n))d(α)=|f9|(9)+|f12|(12)+|f15|(15)+|f36|(36)+∑α∼f∞∈F(CSL(m,n))d(α). $
|
After some mathematical simplifications, we can get
|
$ FI(CSL(m,n))={48n−12if m=196m−12if n=1,∀m168m−60if n=2,∀m45m−9n+36mn−42if both m,n are even45m−9n+36mn−21otherwise. $
|
There are three regular plane tessellations known to exist, each constituted from the same type of regular polygon: triangular, square, and hexagonal. The triangular tessellation is used to define the hexagonal network, which is extensively studied in [54]. A dimensioned hexagonal network $ TH_{k} $ has $ 3{k}^2-3{k}+1 $ vertices and $ 9{k}^2-15{k}+6 $ edges, where $ {k} $ is the number of vertices on one side of the hexagon. It has $ 2{k}-2 $ diameter. There are six vertices of degree three that are referred to as corner vertices. Moreover, the result required detailed are available in the Table 2.
| Dimension | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{12}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{14}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{17}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{18}}}{\bf{|}} $ |
| $ 1 $ | $ 6 $ | $ 0 $ | $ 0 $ | $ 0 $ |
| $ 2 $ | $ 6 $ | $ 12 $ | $ 12 $ | $ 12 $ |
| $ 3 $ | $ 6 $ | $ 24 $ | $ 24 $ | $ 60 $ |
| $ 4 $ | $ 6 $ | $ 36 $ | $ 36 $ | $ 144 $ |
| $ 5 $ | $ 6 $ | $ 48 $ | $ 48 $ | $ 264 $ |
| $ 6 $ | $ 6 $ | $ 60 $ | $ 60 $ | $ 420 $ |
| $ 7 $ | $ 6 $ | $ 72 $ | $ 72 $ | $ 612 $ |
| $ 8 $ | $ 6 $ | $ 84 $ | $ 84 $ | $ 840 $ |
| . | . | . | . | . |
| . | . | . | . | . |
| . | . | . | . | . |
| $ {k} $ | $ 6 $ | $ 12({k}-1) $ | $ 12({k}-1) $ | $ 18{k}^{2}-42{k}+24 $ |
DownLoad:
CSV
Theorem 2.3. Let $ TH_{k} $ be the triangular honeycomb network of dimension $ k\geq1. $ Then the face index of graph $ TH_{k} $ is
|
$ FI(THk)=324k2−336k+102. $
|
Proof. Consider $ TH_{k} $ be a graph of triangular honeycomb network. The graph $ TH_{1} $ has one internal and only one external face while graph $ TH_{k} $ with $ k\geq2, $ contains four types of internal faces $ f_{12}, $ $ f_{14}, $ $ f_{17}, $ and $ f_{18} $ with one external face $ f^{\infty}. $
For $ TH_{1} $ the sum of degree of incident vertices to the external face is $ 18 $ and in $ TH_{2} $ the sum of degree of incident vertices to the external face is $ 66. $ Whenever the graph $ TH_{3}, $ the sum of degree of incident vertices to the external face is $ 114. $ Similarly, for $ TH_{k} $ has $ n- $dimension then sum of degree of incident vertices to the external face is $ 48k-30. $
The number of internal faces with degree in each dimension is given in Table 2.
By using the definition of face index $ FI $ we have
|
$ FI(THk)=∑α∼f∈F(THk)d(α)=∑α∼f12∈F(THk)d(α)+∑α∼f14∈F(THk)d(α)+∑α∼f17∈F(THk)d(α)+∑α∼f18∈F(THk)d(α)+∑α∼f∞∈F(THk)d(α)=|f12|(12)+|f14|(14)+|f17|(17)+|f18|(18)+(48k−30)=(6)(12)+(12(k−1))(14)+(12(k−1))(17)+(18k2−42k+24)(18)+48k−30=324k2−336k+102. $
|
Hence, this is our required result.
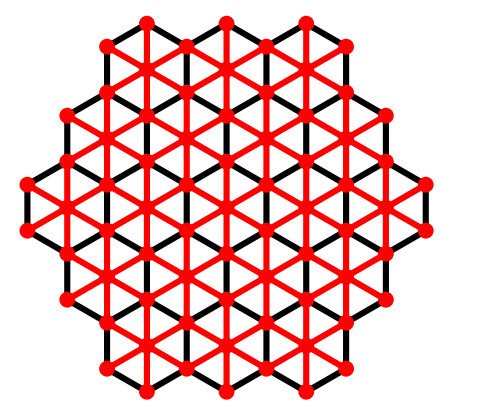
Given carbon sheet in the Figure 6, is made by grid of hexagons. There are few types of carbon sheets are given in [55,56]. The carbon sheet is symbolize as $ HCS_{{m}, {n}}, $ where $ {n} $ represents the total number of vertical hexagons and $ {m} $ denotes the horizontal hexagons. It contain total $ 4{m}{n}+2\left({n}+{m}\right)-1 $ vertices and $ 6{n}{m}+2{m}+{n}-2 $ edges. Moreover, the result required detailed are available in Tables 3 and 4.
| Dimension $ {{\boldsymbol{m}}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{15}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{16}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{18}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}^{{\bf{\infty}}}{\bf{|}} $ |
| $ 2 $ | $ 3 $ | $ 2\left({n}-1\right) $ | $ {n}-1 $ | $ 20{n}+7 $ |
DownLoad:
CSV
| Dimension $ {{\boldsymbol{m}}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{15}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{16}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{17}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{18}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}^{{\bf{\infty}}}{\bf{|}} $ |
| $ 2 $ | $ 3 $ | $ 2\left({n}-1\right) $ | $ 0 $ | $ {n}-1 $ | $ 20{n}+7 $ |
| $ 3 $ | $ 2 $ | $ 2{n} $ | $ 1 $ | $ 3\left({n}-1\right) $ | $ 20{n}+17 $ |
| $ 4 $ | $ 2 $ | $ 2{n} $ | $ 3 $ | $ 5\left({n}-1\right) $ | $ 20{n}+27 $ |
| $ 5 $ | $ 2 $ | $ 2{n} $ | $ 5 $ | $ 7\left({n}-1\right) $ | $ 20{n}+37 $ |
| $ 6 $ | $ 2 $ | $ 2{n} $ | $ 7 $ | $ 9\left({n}-1\right) $ | $ 20{n}+47 $ |
| . | . | . | . | . | . |
| . | . | . | . | . | . |
| . | . | . | . | . | . |
| $ {m} $ | $ 2 $ | $ 2{n} $ | $ 2{m}-5 $ | $ 2{m}{n}-2{m}-3{n}+3 $ | $ 20{n}+10{m}-13 $ |
DownLoad:
CSV
Theorem 2.4. Let $ HCS_{{m}, {n}} $ be the carbon sheet of dimension $ \left({m}, {n}\right) $ and $ {m}, {n}\geq2. $ Then the face index of $ HCS_{{m}, {n}} $ is
|
$ FI(HCSm,n)={70n+2ifm=236mn−14−2(n−4m)ifm≥3. $
|
Proof. Consider $ HCS_{{m}, {n}} $ be the carbon sheet of dimension $ \left({m}, {n}\right) $ and $ {m}, {n}\geq2. $ Let $ {f}_{i} $ denotes the faces of graph $ HCS_{{m}, {n}} $ having degree $ {i}, $ which is $ d(f_{i}) = \sum_{\alpha\sim f_{i}}{d\left(\alpha\right)} = i, $ and $ |f_{i}| $ denotes the number of faces with degree $ i. $ A graph $ HCS_{{m}, {n}} $ for a particular value of $ {m} = 2 $ contains three types of internal faces $ {f}_{15}, $ $ {f}_{16}, $ $ {f}_{17} $ and $ {f}_{18} $ with one external face $ {f}^{\infty}. $ While for the generalize values of $ {m}\geq3, $ it contain four types of internal faces $ {f}_{15}, $ $ {f}_{16} $ and $ {f}_{17} $ with one external face $ {f}^{\infty} $ in usual manner. For the face index of generalize nanotube, we will divide into two cases on the values of $ {m}. $
Case 1: When $ HCS_{{m}, {n}} $ has one row or $ HCS_{{2}, {n}}. $
A graph $ HCS_{{m}, {n}} $ for a this particular value of $ {m} = 2 $ contains three types of internal faces $ \left|{f}_{15}\right| = 3, $ $ \left|{f}_{16}\right| = 2\left({n}-1\right) $ and $ \left|{f}_{18}\right| = {n}-1 $ with one external face $ {f}^{\infty}. $ For the face index of carbon sheet, details are given in the Table 3. Now the face index $ FI $ of the graph $ NT_{{2}, {n}} $ is given by
|
$ FI(HCS2,n)=∑α∼f∈F(HCS2,n)d(α)=∑α∼f15∈F(HCS2,n)d(α)+∑α∼f16∈F(HCS2,n)d(α)+∑α∼f18∈F(HCS2,n)d(α)+∑α∼f∞∈F(HCS2,n)d(α)=|f15|(15)+|f16|(16)+|f18|(18)+20n+7.=3(15)+2(n−1)(16)+(n−1)(18)+20n+7.=70n+2. $
|
Case 2: When $ HCS_{{m}, {n}} $ has $ {m}\geq3 $ rows.
A graph $ HCS_{{m}, {n}} $ for generalize values of $ {m}\geq3 $ contains four types of internal faces $ \left|{f}_{15}\right| = 2, $ $ \left|{f}_{16}\right| = 2{n}, $ $ \left|{f}_{17}\right| = 2{m}-5 $ and $ \left|{f}_{18}\right| = 2{m}{n}-2{m}-3{n}+3 $ with one external face $ {f}^{\infty}. $ For the face index of carbon sheet, details are given in the Table 4. Now the face index $ FI $ of the graph $ NT_{{m}, {n}} $ is given by
|
$ FI(HCSm,n)=∑α∼f∈F(HCSm,n)d(α)=∑α∼f15∈F(HCSm,n)d(α)+∑α∼f16∈F(HCSm,n)d(α)+∑α∼f17∈F(HCSm,n)d(α)+∑α∼f18∈F(HCSm,n)d(α)+∑α∼f∞∈F(HCSm,n)d(α)=|f15|(15)+|f16|(16)+|f17|(17)+|f18|(18)+20n+10m−13.=36mn−2n+8m−14. $
|
Given structure of polyhedron generalized sheet of $ C^{\ast}_{28} $ in the Figure 7, is made by generalizing a $ C^{\ast}_{28} $ polyhedron structure which is shown in the Figure 8. This particular structure of $ C^{\ast}_{28} $ polyhedron are given in [57]. The polyhedron generalized sheet of $ C^{\ast}_{28} $ is as symbolize $ PHS_{{m}, {n}}, $ where $ {n} $ represents the total number of vertical $ C^{\ast}_{28} $ polyhedrons and $ {m} $ denotes the horizontal $ C^{\ast}_{28} $ polyhedrons. It contain total $ 23{n}{m}+3{n}+2{m} $ vertices and $ 33{n}{m}+{n}+{m} $ edges. Moreover, the result required detailed are available in Tables 3 and 5.
| $ {{\boldsymbol{m}}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{14}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{15}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{16}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{17}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{18}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{20}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{35}}}{\bf{|}} $ |
| $ 1 $ | $ 2{n}+1 $ | $ 2 $ | $ 4{n}-2 $ | $ 0 $ | $ 0 $ | $ 2{n}-1 $ | $ 0 $ |
| $ 2 $ | $ 2{n}+2 $ | $ 2 $ | $ 8{n}-2 $ | $ 2 $ | $ 2{n}-2 $ | $ 4{n}-2 $ | $ 2{n}-1 $ |
| $ 3 $ | $ 2{n}+3 $ | $ 2 $ | $ 12{n}-2 $ | $ 4 $ | $ 4{n}-4 $ | $ 6{n}-3 $ | $ 4{n}-2 $ |
| . | . | . | . | . | . | . | . |
| . | . | . | . | . | . | . | . |
| . | . | . | . | . | . | . | . |
| $ {m} $ | $ 2{n}+{m} $ | $ 2 $ | $ 4{m}{n}-2 $ | $ 2{m}-2 $ | $ 2{m}{n}-2\left({m}+{n}\right)+2 $ | $ 2{m}{n}-{m} $ | $ 2{m}{n}-\left({m}+2{n}\right)+1 $ |
DownLoad:
CSV
Theorem 2.5. Let $ PHS_{{m}, {n}} $ be the polyhedron generalized sheet of $ C^{\ast}_{28} $ of dimension $ \left({m}, {n}\right) $ and $ {m}, {n}\geq1. $ Then the face index of $ PHS_{{m}, {n}} $ is
|
$ FI(PHSm,n)=210mn−2(3m+5n). $
|
Proof. Consider $ PHS_{{m}, {n}} $ be the polyhedron generalized sheet of $ C^{\ast}_{28} $ of dimension $ \left({m}, {n}\right) $ and $ {m}, {n}\geq1. $ Let $ {f}_{i} $ denotes the faces of graph $ PHS_{{m}, {n}} $ having degree $ {i}, $ which is $ d(f_{i}) = \sum_{\alpha\sim f_{i}}{d\left(\alpha\right)} = i, $ and $ |f_{i}| $ denotes the number of faces with degree $ i. $ A graph $ PHS_{{m}, {n}} $ for the generalize values of $ {m}, {n}\geq1, $ it contain seven types of internal faces $ {f}_{14}, {f}_{15}, {f}_{16}, {f}_{17}, {f}_{18}, {f}_{20} $ and $ {f}_{35} $ with one external face $ {f}^{\infty} $ in usual manner. For the face index of polyhedron generalized sheet, details are given in the Table 5.
A graph $ PHS_{{m}, {n}} $ for generalize values of $ {m}, {n}\geq1 $ contains seven types of internal faces $ \left|{f}_{14}\right| = 2{n}+{m}, $ $ \left|{f}_{15}\right| = 2, $ $ \left|{f}_{16}\right| = 4{n}{m}-2, $ $ \left|{f}_{17}\right| = 2\left({m}-1\right), $ $ \left|{f}_{18}\right| = 2{n}{m}-2\left({m}+{n}\right)+2, $ $ \left|{f}_{20}\right| = 2{n}{m}-2{m}{n}-{m}, $ and $ \left|{f}_{35}\right| = 2{m}{n}-{m}-2{n}+1 $ with one external face $ {f}^{\infty}. $ Now the face index $ FI $ of the graph $ PHS_{{m}, {n}} $ is given by
|
$ FI(PHSm,n)=∑α∼f∈F(PHSm,n)d(α)=∑α∼f14∈F(PHSm,n)d(α)+∑α∼f15∈F(PHSm,n)d(α)+∑α∼f16∈F(PHSm,n)d(α)+∑α∼f17∈F(PHSm,n)d(α)+∑α∼f18∈F(PHSm,n)d(α)+∑α∼f20∈F(PHSm,n)d(α)+∑α∼f35∈F(PHSm,n)d(α)+∑α∼f∞∈F(PHSm,n)d(α)=|f14|(14)+|f15|(15)+|f16|(16)+|f17|(17)+|f18|(18)+|f20|(20)+|f35|(35)+37m+68n−35.=210mn−6m−10n. $
|
With the advancement of technology, types of equipment and apparatuses of studying different chemical compounds are evolved. But topological descriptors or indices are still preferable and useful tools to develop numerical science of compounds. Therefore, from time to time new topological indices are introduced to study different chemical compounds deeply. In this study, we discussed a newly developed tool of some silicate type networks and generalized sheets, carbon sheet, polyhedron generalized sheet, with the face index concept. It provides numerical values of these networks based on the information of faces. It also helps to study physicochemical characteristics based on the faces of silicate networks.
M. K. Jamil conceived of the presented idea. K. Dawood developed the theory and performed the computations. M. Azeem verified the analytical methods, R. Luo investigated and supervised the findings of this work. All authors discussed the results and contributed to the final manuscript.
This work was supported by the National Science Foundation of China (11961021 and 11561019), Guangxi Natural Science Foundation (2020GXNSFAA159084), and Hechi University Research Fund for Advanced Talents (2019GCC005).
The authors declare that they have no conflicts of interest.
| [1] |
Gjorevski N, Nelson CM (2011) Integrated morphodynamic signalling of the mammary gland. Nat Rev Mol Cell Biol 12: 581-593. doi: 10.1038/nrm3168
|
| [2] |
Forsyth IA, Neville MC (2009) Introduction: hormonal regulation of mammary development and milk protein gene expression at the whole animal and molecular levels. J Mammary Gland Biol Neoplasia 14: 317-319. doi: 10.1007/s10911-009-9146-4
|
| [3] | Polyak K, Kalluri R (2010) The role of the microenvironment in mammary gland development and cancer. Cold Spring Harb Perspect Biol 2: a003244. |
| [4] |
Vogel WF, Abdulhussein R, Ford CE (2006) Sensing extracellular matrix: an update on discoidin domain receptor function. Cell Signal 18: 1108-1116. doi: 10.1016/j.cellsig.2006.02.012
|
| [5] |
Ozbek S, Balasubramanian PG, Chiquet-Ehrismann R, et al. (2010) The evolution of extracellular matrix. Mol Biol Cell 21: 4300-4305. doi: 10.1091/mbc.E10-03-0251
|
| [6] |
Egeblad M, Rasch MG, Weaver VM (2010) Dynamic interplay between the collagen scaffold and tumor evolution. Curr Opin Cell Biol 22: 697-706. doi: 10.1016/j.ceb.2010.08.015
|
| [7] |
McNally S, Martin F (2011) Molecular regulators of pubertal mammary gland development. Ann Med 43: 212-234. doi: 10.3109/07853890.2011.554425
|
| [8] |
Hinck L, Silberstein GB (2005) Key stages in mammary gland development: the mammary end bud as a motile organ. Breast Cancer Res 7: 245-251. doi: 10.1186/bcr1331
|
| [9] | Watson CJ (2006) Post-lactational mammary gland regression: molecular basis and implications for breast cancer. Expert Rev Mol Med 8: 1-15. |
| [10] |
Brisken C, Kaur S, Chavarria TE, et al. (1999) Prolactin controls mammary gland development via direct and indirect mechanisms. Dev Biol 210: 96-106. doi: 10.1006/dbio.1999.9271
|
| [11] |
Oakes SR, Rogers RL, Naylor MJ, et al. (2008) Prolactin regulation of mammary gland development. J Mammary Gland Biol Neoplasia 13: 13-28. doi: 10.1007/s10911-008-9069-5
|
| [12] |
Sternlicht MD, Kouros-Mehr H, Lu P, et al. (2006) Hormonal and local control of mammary branching morphogenesis. Differentiation 74: 365-381. doi: 10.1111/j.1432-0436.2006.00105.x
|
| [13] | Fata JE, Werb Z, Bissell MJ (2004) Regulation of mammary gland branching morphogenesis by the extracellular matrix and its remodeling enzymes. Breast Cancer Res 6: 1-11. |
| [14] |
Myllyharju J, Kivirikko KI (2004) Collagens, modifying enzymes and their mutations in humans, flies and worms. Trends Genet 20: 33-43. doi: 10.1016/j.tig.2003.11.004
|
| [15] |
Yeh YC, Lin HH, Tang MJ (2012) A tale of two collagen receptors, integrin beta1 and discoidin domain receptor 1, in epithelial cell differentiation. Am J Physiol Cell Physiol 303: C1207-1217. doi: 10.1152/ajpcell.00253.2012
|
| [16] |
Carafoli F, Hohenester E (2013) Collagen recognition and transmembrane signalling by discoidin domain receptors. Biochim Biophys Acta 1834: 2187-2194. doi: 10.1016/j.bbapap.2012.10.014
|
| [17] |
Valiathan RR, Marco M, Leitinger B, et al. (2012) Discoidin domain receptor tyrosine kinases: new players in cancer progression. Cancer Metastasis Rev 31: 295-321. doi: 10.1007/s10555-012-9346-z
|
| [18] |
Ortega N, Werb Z (2002) New functional roles for non-collagenous domains of basement membrane collagens. J Cell Sci 115: 4201-4214. doi: 10.1242/jcs.00106
|
| [19] | Acerbi I, Cassereau L, Dean I, et al. Human breast cancer invasion and aggression correlates with ECM stiffening and immune cell infiltration. Integr Biol (Camb). [in press] |
| [20] | Tao G, Levay AK, Peacock JD, et al. Collagen XIV is important for growth and structural integrity of the myocardium. J Mol Cell Cardiol 53: 626-638. |
| [21] |
Mehner C, Radisky DC (2013) Triggering the landslide: The tumor-promotional effects of myofibroblasts. Exp Cell Res 319: 1657-1662. doi: 10.1016/j.yexcr.2013.03.015
|
| [22] |
Gehler S, Ponik SM, Riching KM, et al. (2013) Bi-directional signaling: extracellular matrix and integrin regulation of breast tumor progression. Crit Rev Eukaryot Gene Expr 23: 139-157. doi: 10.1615/CritRevEukarGeneExpr.2013006647
|
| [23] | Nistico P, Bissell MJ, Radisky DC (2012) Epithelial-mesenchymal transition: general principles and pathological relevance with special emphasis on the role of matrix metalloproteinases. Cold Spring Harb Perspect Biol 4. |
| [24] |
Favreau AJ, Vary CP, Brooks PC, et al. (2014) Cryptic collagen IV promotes cell migration and adhesion in myeloid leukemia. Cancer Med 3: 265-272. doi: 10.1002/cam4.203
|
| [25] |
Emsley J, Knight CG, Farndale RW, et al. (2000) Structural basis of collagen recognition by integrin alpha2beta1. Cell 101: 47-56. doi: 10.1016/S0092-8674(00)80622-4
|
| [26] |
Espinosa Neira R, Salazar EP (2012) Native type IV collagen induces an epithelial to mesenchymal transition-like process in mammary epithelial cells MCF10A. Int J Biochem Cell Biol 44: 2194-2203. doi: 10.1016/j.biocel.2012.08.018
|
| [27] |
Leitinger B (2011) Transmembrane collagen receptors. Annu Rev Cell Dev Biol 27: 265-290. doi: 10.1146/annurev-cellbio-092910-154013
|
| [28] |
Noordeen NA, Carafoli F, Hohenester E, et al. (2006) A transmembrane leucine zipper is required for activation of the dimeric receptor tyrosine kinase DDR1. J Biol Chem 281: 22744-22751. doi: 10.1074/jbc.M603233200
|
| [29] |
Mihai C, Chotani M, Elton TS, et al. (2009) Mapping of DDR1 distribution and oligomerization on the cell surface by FRET microscopy. J Mol Biol 385: 432-445. doi: 10.1016/j.jmb.2008.10.067
|
| [30] |
Shrivastava A, Radziejewski C, Campbell E, et al. (1997) An orphan receptor tyrosine kinase family whose members serve as nonintegrin collagen receptors. Mol Cell 1: 25-34. doi: 10.1016/S1097-2765(00)80004-0
|
| [31] |
Vogel W, Gish GD, Alves F, et al. (1997) The discoidin domain receptor tyrosine kinases are activated by collagen. Mol Cell 1: 13-23. doi: 10.1016/S1097-2765(00)80003-9
|
| [32] |
Canning P, Tan L, Chu K, et al. (2014) Structural mechanisms determining inhibition of the collagen receptor DDR1 by selective and multi-targeted type II kinase inhibitors. J Mol Biol 426: 2457-2470. doi: 10.1016/j.jmb.2014.04.014
|
| [33] | Barker KT, Martindale JE, Mitchell PJ, et al. (1995) Expression patterns of the novel receptor-like tyrosine kinase, DDR, in human breast tumours. Oncogene 10: 569-575. |
| [34] | Di Marco E, Cutuli N, Guerra L, et al. (1993) Molecular cloning of trkE, a novel trk-related putative tyrosine kinase receptor isolated from normal human keratinocytes and widely expressed by normal human tissues. J Biol Chem 268: 24290-24295. |
| [35] | Karn T, Holtrich U, Brauninger A, et al. (1993) Structure, expression and chromosomal mapping of TKT from man and mouse: a new subclass of receptor tyrosine kinases with a factor VIII-like domain. Oncogene 8: 3433-3440. |
| [36] |
Fu HL, Valiathan RR, Arkwright R, et al. (2013) Discoidin domain receptors: unique receptor tyrosine kinases in collagen-mediated signaling. J Biol Chem 288: 7430-7437. doi: 10.1074/jbc.R112.444158
|
| [37] |
Jin P, Zhang J, Sumariwalla PF, et al. (2008) Novel splice variants derived from the receptor tyrosine kinase superfamily are potential therapeutics for rheumatoid arthritis. Arthritis Res Ther 10: R73. doi: 10.1186/ar2447
|
| [38] |
Leitinger B (2014) Discoidin domain receptor functions in physiological and pathological conditions. Int Rev Cell Mol Biol 310: 39-87. doi: 10.1016/B978-0-12-800180-6.00002-5
|
| [39] |
Curat CA, Eck M, Dervillez X, et al. (2001) Mapping of epitopes in discoidin domain receptor 1 critical for collagen binding. J Biol Chem 276: 45952-45958. doi: 10.1074/jbc.M104360200
|
| [40] |
Abdulhussein R, McFadden C, Fuentes-Prior P, et al. (2004) Exploring the collagen-binding site of the DDR1 tyrosine kinase receptor. J Biol Chem 279: 31462-31470. doi: 10.1074/jbc.M400651200
|
| [41] | Leitinger B (2003) Molecular analysis of collagen binding by the human discoidin domain receptors, DDR1 and DDR2. Identification of collagen binding sites in DDR2. J Biol Chem 278: 16761-16769. |
| [42] |
Ichikawa O, Osawa M, Nishida N, et al. (2007) Structural basis of the collagen-binding mode of discoidin domain receptor 2. EMBO J 26: 4168-4176. doi: 10.1038/sj.emboj.7601833
|
| [43] |
Xu H, Raynal N, Stathopoulos S, et al. (2011) Collagen binding specificity of the discoidin domain receptors: binding sites on collagens II and III and molecular determinants for collagen IV recognition by DDR1. Matrix Biol 30: 16-26. doi: 10.1016/j.matbio.2010.10.004
|
| [44] |
Carafoli F, Mayer MC, Shiraishi K, et al. (2012) Structure of the discoidin domain receptor 1 extracellular region bound to an inhibitory Fab fragment reveals features important for signaling. Structure 20: 688-697. doi: 10.1016/j.str.2012.02.011
|
| [45] |
Leitinger B, Kwan AP (2006) The discoidin domain receptor DDR2 is a receptor for type X collagen. Matrix Biol 25: 355-364. doi: 10.1016/j.matbio.2006.05.006
|
| [46] |
Konitsiotis AD, Raynal N, Bihan D, et al. (2008) Characterization of high affinity binding motifs for the discoidin domain receptor DDR2 in collagen. J Biol Chem 283: 6861-6868. doi: 10.1074/jbc.M709290200
|
| [47] |
Koo DH, McFadden C, Huang Y, et al. (2006) Pinpointing phosphotyrosine-dependent interactions downstream of the collagen receptor DDR1. FEBS Lett 580: 15-22. doi: 10.1016/j.febslet.2005.11.035
|
| [48] |
Wang CZ, Su HW, Hsu YC, et al. (2006) A discoidin domain receptor 1/SHP-2 signaling complex inhibits alpha2beta1-integrin-mediated signal transducers and activators of transcription 1/3 activation and cell migration. Mol Biol Cell 17: 2839-2852. doi: 10.1091/mbc.E05-11-1068
|
| [49] |
Lemeer S, Bluwstein A, Wu Z, et al. (2012) Phosphotyrosine mediated protein interactions of the discoidin domain receptor 1. J Proteomics 75: 3465-3477. doi: 10.1016/j.jprot.2011.10.007
|
| [50] |
Ruiz PA, Jarai G (2011) Collagen I induces discoidin domain receptor (DDR) 1 expression through DDR2 and a JAK2-ERK1/2-mediated mechanism in primary human lung fibroblasts. J Biol Chem 286: 12912-12923. doi: 10.1074/jbc.M110.143693
|
| [51] | L'Hote C G, Thomas PH, Ganesan TS (2002) Functional analysis of discoidin domain receptor 1: effect of adhesion on DDR1 phosphorylation. FASEB J 16: 234-236. |
| [52] |
Huang Y, Arora P, McCulloch CA, et al. (2009) The collagen receptor DDR1 regulates cell spreading and motility by associating with myosin IIA. J Cell Sci 122: 1637-1646. doi: 10.1242/jcs.046219
|
| [53] |
Shintani Y, Fukumoto Y, Chaika N, et al. (2008) Collagen I-mediated up-regulation of N-cadherin requires cooperative signals from integrins and discoidin domain receptor 1. J Cell Biol 180: 1277-1289. doi: 10.1083/jcb.200708137
|
| [54] |
Hidalgo-Carcedo C, Hooper S, Chaudhry SI, et al. (2011) Collective cell migration requires suppression of actomyosin at cell-cell contacts mediated by DDR1 and the cell polarity regulators Par3 and Par6. Nat Cell Biol 13: 49-58. doi: 10.1038/ncb2133
|
| [55] |
Hansen C, Greengard P, Nairn AC, et al. (2006) Phosphorylation of DARPP-32 regulates breast cancer cell migration downstream of the receptor tyrosine kinase DDR1. Exp Cell Res 312: 4011-4018. doi: 10.1016/j.yexcr.2006.09.003
|
| [56] |
Hilton HN, Stanford PM, Harris J, et al. (2008) KIBRA interacts with discoidin domain receptor 1 to modulate collagen-induced signalling. Biochim Biophys Acta 1783: 383-393. doi: 10.1016/j.bbamcr.2007.12.007
|
| [57] | Dejmek J, Leandersson K, Manjer J, et al. (2005) Expression and signaling activity of Wnt-5a/discoidin domain receptor-1 and Syk plays distinct but decisive roles in breast cancer patient survival. Clin Cancer Res 11: 520-528. |
| [58] |
Kim HG, Hwang SY, Aaronson SA, et al. (2011) DDR1 receptor tyrosine kinase promotes prosurvival pathway through Notch1 activation. J Biol Chem 286: 17672-17681. doi: 10.1074/jbc.M111.236612
|
| [59] |
Lu KK, Trcka D, Bendeck MP (2011) Collagen stimulates discoidin domain receptor 1-mediated migration of smooth muscle cells through Src. Cardiovasc Pathol 20: 71-76. doi: 10.1016/j.carpath.2009.12.006
|
| [60] |
Ongusaha PP, Kim JI, Fang L, et al. (2003) p53 induction and activation of DDR1 kinase counteract p53-mediated apoptosis and influence p53 regulation through a positive feedback loop. EMBO J 22: 1289-1301. doi: 10.1093/emboj/cdg129
|
| [61] |
Das S, Ongusaha PP, Yang YS, et al. (2006) Discoidin domain receptor 1 receptor tyrosine kinase induces cyclooxygenase-2 and promotes chemoresistance through nuclear factor-kappaB pathway activation. Cancer Res 66: 8123-8130. doi: 10.1158/0008-5472.CAN-06-1215
|
| [62] |
Ikeda K, Wang LH, Torres R, et al. (2002) Discoidin domain receptor 2 interacts with Src and Shc following its activation by type I collagen. J Biol Chem 277: 19206-19212. doi: 10.1074/jbc.M201078200
|
| [63] |
Yang K, Kim JH, Kim HJ, et al. (2005) Tyrosine 740 phosphorylation of discoidin domain receptor 2 by Src stimulates intramolecular autophosphorylation and Shc signaling complex formation. J Biol Chem 280: 39058-39066. doi: 10.1074/jbc.M506921200
|
| [64] |
Olaso E, Labrador JP, Wang L, et al. (2002) Discoidin domain receptor 2 regulates fibroblast proliferation and migration through the extracellular matrix in association with transcriptional activation of matrix metalloproteinase-2. J Biol Chem 277: 3606-3613. doi: 10.1074/jbc.M107571200
|
| [65] | Marcel V, Catez F, Diaz JJ (2015) p53, a translational regulator: contribution to its tumour-suppressor activity. Oncogene. [in press] |
| [66] |
Petitjean A, Achatz MI, Borresen-Dale AL, et al. (2007) TP53 mutations in human cancers: functional selection and impact on cancer prognosis and outcomes. Oncogene 26: 2157-2165. doi: 10.1038/sj.onc.1210302
|
| [67] |
Vogel WF (2002) Ligand-induced shedding of discoidin domain receptor 1. FEBS Lett 514: 175-180. doi: 10.1016/S0014-5793(02)02360-8
|
| [68] |
Slack BE, Siniaia MS, Blusztajn JK (2006) Collagen type I selectively activates ectodomain shedding of the discoidin domain receptor 1: involvement of Src tyrosine kinase. J Cell Biochem 98: 672-684. doi: 10.1002/jcb.20812
|
| [69] |
Fu HL, Sohail A, Valiathan RR, et al. (2013) Shedding of discoidin domain receptor 1 by membrane-type matrix metalloproteinases. J Biol Chem 288: 12114-12129. doi: 10.1074/jbc.M112.409599
|
| [70] |
Shitomi Y, Thogersen IB, Ito N, et al. (2015) ADAM10 controls collagen signaling and cell migration on collagen by shedding the ectodomain of discoidin domain receptor 1 (DDR1). Mol Biol Cell 26: 659-673. doi: 10.1091/mbc.E14-10-1463
|
| [71] |
Huber MA, Kraut N, Beug H (2005) Molecular requirements for epithelial-mesenchymal transition during tumor progression. Curr Opin Cell Biol 17: 548-558. doi: 10.1016/j.ceb.2005.08.001
|
| [72] |
Knust E, Bossinger O (2002) Composition and formation of intercellular junctions in epithelial cells. Science 298: 1955-1959. doi: 10.1126/science.1072161
|
| [73] |
Yeh YC, Wu CC, Wang YK, et al. (2011) DDR1 triggers epithelial cell differentiation by promoting cell adhesion through stabilization of E-cadherin. Mol Biol Cell 22: 940-953. doi: 10.1091/mbc.E10-08-0678
|
| [74] |
Wang CZ, Yeh YC, Tang MJ (2009) DDR1/E-cadherin complex regulates the activation of DDR1 and cell spreading. Am J Physiol Cell Physiol 297: C419-429. doi: 10.1152/ajpcell.00101.2009
|
| [75] |
Yeh YC, Wang CZ, Tang MJ (2009) Discoidin domain receptor 1 activation suppresses alpha2beta1 integrin-dependent cell spreading through inhibition of Cdc42 activity. J Cell Physiol 218: 146-156. doi: 10.1002/jcp.21578
|
| [76] |
Xu H, Bihan D, Chang F, et al. (2012) Discoidin domain receptors promote alpha1beta1- and alpha2beta1-integrin mediated cell adhesion to collagen by enhancing integrin activation. PLoS One 7: e52209. doi: 10.1371/journal.pone.0052209
|
| [77] |
Imamichi Y, Menke A (2007) Signaling pathways involved in collagen-induced disruption of the E-cadherin complex during epithelial-mesenchymal transition. Cells Tissues Organs 185: 180-190. doi: 10.1159/000101319
|
| [78] |
Staudinger LA, Spano SJ, Lee W, et al. (2013) Interactions between the discoidin domain receptor 1 and beta1 integrin regulate attachment to collagen. Biol Open 2: 1148-1159. doi: 10.1242/bio.20135090
|
| [79] |
Rudra-Ganguly N, Lowe C, Mattie M, et al. (2014) Discoidin domain receptor 1 contributes to tumorigenesis through modulation of TGFBI expression. PLoS One 9: e111515. doi: 10.1371/journal.pone.0111515
|
| [80] |
Park CY, Min KN, Son JY, et al. (2014) An novel inhibitor of TGF-beta type I receptor, IN-1130, blocks breast cancer lung metastasis through inhibition of epithelial-mesenchymal transition. Cancer Lett 351: 72-80. doi: 10.1016/j.canlet.2014.05.006
|
| [81] |
Ozdamar B, Bose R, Barrios-Rodiles M, et al. (2005) Regulation of the polarity protein Par6 by TGFbeta receptors controls epithelial cell plasticity. Science 307: 1603-1609. doi: 10.1126/science.1105718
|
| [82] |
Buijs JT, Stayrook KR, Guise TA (2011) TGF-beta in the Bone Microenvironment: Role in Breast Cancer Metastases. Cancer Microenviron 4: 261-281. doi: 10.1007/s12307-011-0075-6
|
| [83] | de Jong JS, van Diest PJ, van der Valk P, et al. (1998) Expression of growth factors, growth-inhibiting factors, and their receptors in invasive breast cancer. II: Correlations with proliferation and angiogenesis. J Pathol 184: 53-57. |
| [84] |
Walsh LA, Nawshad A, Medici D (2011) Discoidin domain receptor 2 is a critical regulator of epithelial-mesenchymal transition. Matrix Biol 30: 243-247. doi: 10.1016/j.matbio.2011.03.007
|
| [85] |
Xu J, Lu W, Zhang S, et al. (2014) Overexpression of DDR2 contributes to cell invasion and migration in head and neck squamous cell carcinoma. Cancer Biol Ther 15: 612-622. doi: 10.4161/cbt.28181
|
| [86] |
Maeyama M, Koga H, Selvendiran K, et al. (2008) Switching in discoid domain receptor expressions in SLUG-induced epithelial-mesenchymal transition. Cancer 113: 2823-2831. doi: 10.1002/cncr.23900
|
| [87] |
Siziopikou KP (2013) Ductal carcinoma in situ of the breast: current concepts and future directions. Arch Pathol Lab Med 137: 462-466. doi: 10.5858/arpa.2012-0078-RA
|
| [88] |
Turashvili G, Bouchal J, Ehrmann J, et al. (2007) Novel immunohistochemical markers for the differentiation of lobular and ductal invasive breast carcinomas. Biomed Pap Med Fac Univ Palacky Olomouc Czech Repub 151: 59-64. doi: 10.5507/bp.2007.010
|
| [89] |
Toy KA, Valiathan RR, Nunez F, et al. (2015) Tyrosine kinase discoidin domain receptors DDR1 and DDR2 are coordinately deregulated in triple-negative breast cancer. Breast Cancer Res Treat 150: 9-18. doi: 10.1007/s10549-015-3285-7
|
| [90] |
Vogel WF, Aszodi A, Alves F, et al. (2001) Discoidin domain receptor 1 tyrosine kinase has an essential role in mammary gland development. Mol Cell Biol 21: 2906-2917. doi: 10.1128/MCB.21.8.2906-2917.2001
|
| [91] |
Ren T, Zhang J, Liu X, et al. (2013) Increased expression of discoidin domain receptor 2 (DDR2): a novel independent prognostic marker of worse outcome in breast cancer patients. Med Oncol 30: 397. doi: 10.1007/s12032-012-0397-3
|
| [92] |
Morikawa A, Takeuchi T, Kito Y, et al. (2015) Expression of Beclin-1 in the Microenvironment of Invasive Ductal Carcinoma of the Breast: Correlation with Prognosis and the Cancer-Stromal Interaction. PLoS One 10: e0125762. doi: 10.1371/journal.pone.0125762
|
| [93] |
Zhang K, Corsa CA, Ponik SM, et al. (2013) The collagen receptor discoidin domain receptor 2 stabilizes SNAIL1 to facilitate breast cancer metastasis. Nat Cell Biol 15: 677-687. doi: 10.1038/ncb2743
|
| [94] | Lodillinsky C, Infante E, Guichard A, et al. (2015) p63/MT1-MMP axis is required for in situ to invasive transition in basal-like breast cancer. Oncogene. [in press] |
| [95] |
Xiang S, Liu YM, Chen X, et al. (2015) ZEB1 Expression Is Correlated With Tumor Metastasis and Reduced Prognosis of Breast Carcinoma in Asian Patients. Cancer Invest 33: 225. doi: 10.3109/07357907.2015.1022258
|
| [96] |
Ling J, Kumar R (2012) Crosstalk between NFkB and glucocorticoid signaling: a potential target of breast cancer therapy. Cancer Lett 322: 119-126. doi: 10.1016/j.canlet.2012.02.033
|
| [97] |
Koh M, Woo Y, Valiathan RR, et al. (2015) Discoidin domain receptor 1 is a novel transcriptional target of ZEB1 in breast epithelial cells undergoing H-Ras-induced epithelial to mesenchymal transition. Int J Cancer 136: E508-520. doi: 10.1002/ijc.29154
|
| [98] |
Wolf K, Wu YI, Liu Y, et al. (2007) Multi-step pericellular proteolysis controls the transition from individual to collective cancer cell invasion. Nat Cell Biol 9: 893-904. doi: 10.1038/ncb1616
|
| [99] |
Neuhaus B, Buhren S, Bock B, et al. (2011) Migration inhibition of mammary epithelial cells by Syk is blocked in the presence of DDR1 receptors. Cell Mol Life Sci 68: 3757-3770. doi: 10.1007/s00018-011-0676-8
|
| [100] | Jonsson M, Andersson T (2001) Repression of Wnt-5a impairs DDR1 phosphorylation and modifies adhesion and migration of mammary cells. J Cell Sci 114: 2043-2053. |
| [101] | Gavin BJ, McMahon AP (1992) Differential regulation of the Wnt gene family during pregnancy and lactation suggests a role in postnatal development of the mammary gland. Mol Cell Biol 12: 2418-2423. |
| [102] |
Dejmek J, Dib K, Jonsson M, et al. (2003) Wnt-5a and G-protein signaling are required for collagen-induced DDR1 receptor activation and normal mammary cell adhesion. Int J Cancer 103: 344-351. doi: 10.1002/ijc.10752
|
| [103] |
Roarty K, Serra R (2007) Wnt5a is required for proper mammary gland development and TGF-beta-mediated inhibition of ductal growth. Development 134: 3929-3939. doi: 10.1242/dev.008250
|
| [104] |
Ribatti D, Crivellato E (2012) “Sprouting angiogenesis”, a reappraisal. Dev Biol 372: 157-165. doi: 10.1016/j.ydbio.2012.09.018
|
| [105] |
Artavanis-Tsakonas S, Rand MD, Lake RJ (1999) Notch signaling: cell fate control and signal integration in development. Science 284: 770-776. doi: 10.1126/science.284.5415.770
|
| [106] |
Dong Y, Li A, Wang J, et al. (2010) Synthetic lethality through combined Notch-epidermal growth factor receptor pathway inhibition in basal-like breast cancer. Cancer Res 70: 5465-5474. doi: 10.1158/0008-5472.CAN-10-0173
|
| [107] |
Thairu N, Kiriakidis S, Dawson P, et al. (2011) Angiogenesis as a therapeutic target in arthritis in 2011: learning the lessons of the colorectal cancer experience. Angiogenesis 14: 223-234. doi: 10.1007/s10456-011-9208-2
|
| [108] |
Yang Y, Sun M, Wang L, et al. (2013) HIFs, angiogenesis, and cancer. J Cell Biochem 114: 967-974. doi: 10.1002/jcb.24438
|
| [109] |
Ren T, Zhang W, Liu X, et al. (2014) Discoidin domain receptor 2 (DDR2) promotes breast cancer cell metastasis and the mechanism implicates epithelial-mesenchymal transition programme under hypoxia. J Pathol 234: 526-537. doi: 10.1002/path.4415
|
| [110] |
Rubinstein E (2011) The complexity of tetraspanins. Biochem Soc Trans 39: 501-505. doi: 10.1042/BST0390501
|
| [111] | Berditchevski F (2001) Complexes of tetraspanins with integrins: more than meets the eye. J Cell Sci 114: 4143-4151. |
| [112] |
Powner D, Kopp PM, Monkley SJ, et al. (2011) Tetraspanin CD9 in cell migration. Biochem Soc Trans 39: 563-567. doi: 10.1042/BST0390563
|
| [113] | Miyake M, Nakano K, Ieki Y, et al. (1995) Motility related protein 1 (MRP-1/CD9) expression: inverse correlation with metastases in breast cancer. Cancer Res 55: 4127-4131. |
| [114] |
Castro-Sanchez L, Soto-Guzman A, Navarro-Tito N, et al. (2010) Native type IV collagen induces cell migration through a CD9 and DDR1-dependent pathway in MDA-MB-231 breast cancer cells. Eur J Cell Biol 89: 843-852. doi: 10.1016/j.ejcb.2010.07.004
|
| [115] |
Hansen RK, Bissell MJ (2000) Tissue architecture and breast cancer: the role of extracellular matrix and steroid hormones. Endocr Relat Cancer 7: 95-113. doi: 10.1677/erc.0.0070095
|
| 1. | Shabana Anwar, Muhammad Kamran Jamil, Amal S. Alali, Mehwish Zegham, Aisha Javed, Extremal values of the first reformulated Zagreb index for molecular trees with application to octane isomers, 2023, 9, 2473-6988, 289, 10.3934/math.2024017 | |
| 2. | Ali N. A. Koam, Ali Ahmad, Raed Qahiti, Muhammad Azeem, Waleed Hamali, Shonak Bansal, Enhanced Chemical Insights into Fullerene Structures via Modified Polynomials, 2024, 2024, 1076-2787, 10.1155/2024/9220686 | |
| 3. | Ali Ahmad, Ali N. A. Koam, Muhammad Azeem, Reverse-degree-based topological indices of fullerene cage networks, 2023, 121, 0026-8976, 10.1080/00268976.2023.2212533 | |
| 4. | Muhammad Waheed Rasheed, Abid Mahboob, Iqra Hanif, Uses of degree-based topological indices in QSPR analysis of alkaloids with poisonous and healthful nature, 2024, 12, 2296-424X, 10.3389/fphy.2024.1381887 | |
| 5. | Shriya Negi, Vijay Kumar Bhat, Face Index of Silicon Carbide Structures: An Alternative Approach, 2024, 16, 1876-990X, 5865, 10.1007/s12633-024-03119-0 | |
| 6. | Haseeb AHMAD, Muhammad AZEEM, Face-degree-based topological descriptors of germanium phosphide, 2024, 52, 18722040, 100429, 10.1016/j.cjac.2024.100429 | |
| 7. | Belman Gautham Shenoy, Raghavendra Ananthapadmanabha, Badekara Sooryanarayana, Prasanna Poojary, Vishu Kumar Mallappa, 2024, Rational Wiener Index and Rational Schultz Index of Graphs, 180, 10.3390/engproc2023059180 |
Figures(2)
Emmanuel Reyes-Uribe, Nathalia Serna-Marquez, Eduardo Perez Salazar. DDRs: receptors that mediate adhesion, migration and invasion in breast cancer cells[J]. AIMS Biophysics, 2015, 2(3): 303-317. doi: 10.3934/biophy.2015.3.303
| Dimension | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{12}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{15}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{36}}}{\bf{|}} $ |
| $ 1 $ | $ 24 $ | $ 48 $ | $ 7 $ |
| $ 2 $ | $ 32 $ | $ 94 $ | $ 14 $ |
| $ 3 $ | $ 40 $ | $ 152 $ | $ 23 $ |
| $ 4 $ | $ 48 $ | $ 222 $ | $ 34 $ |
| $ 5 $ | $ 56 $ | $ 304 $ | $ 47 $ |
| $ 6 $ | $ 64 $ | $ 398 $ | $ 62 $ |
| $ 7 $ | $ 72 $ | $ 504 $ | $ 79 $ |
| $ 8 $ | $ 80 $ | $ 622 $ | $ 98 $ |
| . | . | . | . |
| . | . | . | . |
| . | . | . | . |
| $ {n} $ | $ 8{n}+16 $ | $ 6{n}^{2}+28{n}+14 $ | $ {n}^{2}+4{n}+2 $ |
DownLoad:
CSV
| Dimension | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{12}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{14}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{17}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{18}}}{\bf{|}} $ |
| $ 1 $ | $ 6 $ | $ 0 $ | $ 0 $ | $ 0 $ |
| $ 2 $ | $ 6 $ | $ 12 $ | $ 12 $ | $ 12 $ |
| $ 3 $ | $ 6 $ | $ 24 $ | $ 24 $ | $ 60 $ |
| $ 4 $ | $ 6 $ | $ 36 $ | $ 36 $ | $ 144 $ |
| $ 5 $ | $ 6 $ | $ 48 $ | $ 48 $ | $ 264 $ |
| $ 6 $ | $ 6 $ | $ 60 $ | $ 60 $ | $ 420 $ |
| $ 7 $ | $ 6 $ | $ 72 $ | $ 72 $ | $ 612 $ |
| $ 8 $ | $ 6 $ | $ 84 $ | $ 84 $ | $ 840 $ |
| . | . | . | . | . |
| . | . | . | . | . |
| . | . | . | . | . |
| $ {k} $ | $ 6 $ | $ 12({k}-1) $ | $ 12({k}-1) $ | $ 18{k}^{2}-42{k}+24 $ |
DownLoad:
CSV
| Dimension $ {{\boldsymbol{m}}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{15}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{16}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{18}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}^{{\bf{\infty}}}{\bf{|}} $ |
| $ 2 $ | $ 3 $ | $ 2\left({n}-1\right) $ | $ {n}-1 $ | $ 20{n}+7 $ |
DownLoad:
CSV
| Dimension $ {{\boldsymbol{m}}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{15}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{16}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{17}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{18}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}^{{\bf{\infty}}}{\bf{|}} $ |
| $ 2 $ | $ 3 $ | $ 2\left({n}-1\right) $ | $ 0 $ | $ {n}-1 $ | $ 20{n}+7 $ |
| $ 3 $ | $ 2 $ | $ 2{n} $ | $ 1 $ | $ 3\left({n}-1\right) $ | $ 20{n}+17 $ |
| $ 4 $ | $ 2 $ | $ 2{n} $ | $ 3 $ | $ 5\left({n}-1\right) $ | $ 20{n}+27 $ |
| $ 5 $ | $ 2 $ | $ 2{n} $ | $ 5 $ | $ 7\left({n}-1\right) $ | $ 20{n}+37 $ |
| $ 6 $ | $ 2 $ | $ 2{n} $ | $ 7 $ | $ 9\left({n}-1\right) $ | $ 20{n}+47 $ |
| . | . | . | . | . | . |
| . | . | . | . | . | . |
| . | . | . | . | . | . |
| $ {m} $ | $ 2 $ | $ 2{n} $ | $ 2{m}-5 $ | $ 2{m}{n}-2{m}-3{n}+3 $ | $ 20{n}+10{m}-13 $ |
DownLoad:
CSV
| $ {{\boldsymbol{m}}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{14}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{15}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{16}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{17}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{18}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{20}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{35}}}{\bf{|}} $ |
| $ 1 $ | $ 2{n}+1 $ | $ 2 $ | $ 4{n}-2 $ | $ 0 $ | $ 0 $ | $ 2{n}-1 $ | $ 0 $ |
| $ 2 $ | $ 2{n}+2 $ | $ 2 $ | $ 8{n}-2 $ | $ 2 $ | $ 2{n}-2 $ | $ 4{n}-2 $ | $ 2{n}-1 $ |
| $ 3 $ | $ 2{n}+3 $ | $ 2 $ | $ 12{n}-2 $ | $ 4 $ | $ 4{n}-4 $ | $ 6{n}-3 $ | $ 4{n}-2 $ |
| . | . | . | . | . | . | . | . |
| . | . | . | . | . | . | . | . |
| . | . | . | . | . | . | . | . |
| $ {m} $ | $ 2{n}+{m} $ | $ 2 $ | $ 4{m}{n}-2 $ | $ 2{m}-2 $ | $ 2{m}{n}-2\left({m}+{n}\right)+2 $ | $ 2{m}{n}-{m} $ | $ 2{m}{n}-\left({m}+2{n}\right)+1 $ |
DownLoad:
CSV
| Dimension | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{12}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{15}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{36}}}{\bf{|}} $ |
| $ 1 $ | $ 24 $ | $ 48 $ | $ 7 $ |
| $ 2 $ | $ 32 $ | $ 94 $ | $ 14 $ |
| $ 3 $ | $ 40 $ | $ 152 $ | $ 23 $ |
| $ 4 $ | $ 48 $ | $ 222 $ | $ 34 $ |
| $ 5 $ | $ 56 $ | $ 304 $ | $ 47 $ |
| $ 6 $ | $ 64 $ | $ 398 $ | $ 62 $ |
| $ 7 $ | $ 72 $ | $ 504 $ | $ 79 $ |
| $ 8 $ | $ 80 $ | $ 622 $ | $ 98 $ |
| . | . | . | . |
| . | . | . | . |
| . | . | . | . |
| $ {n} $ | $ 8{n}+16 $ | $ 6{n}^{2}+28{n}+14 $ | $ {n}^{2}+4{n}+2 $ |
| Dimension | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{12}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{14}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{17}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{18}}}{\bf{|}} $ |
| $ 1 $ | $ 6 $ | $ 0 $ | $ 0 $ | $ 0 $ |
| $ 2 $ | $ 6 $ | $ 12 $ | $ 12 $ | $ 12 $ |
| $ 3 $ | $ 6 $ | $ 24 $ | $ 24 $ | $ 60 $ |
| $ 4 $ | $ 6 $ | $ 36 $ | $ 36 $ | $ 144 $ |
| $ 5 $ | $ 6 $ | $ 48 $ | $ 48 $ | $ 264 $ |
| $ 6 $ | $ 6 $ | $ 60 $ | $ 60 $ | $ 420 $ |
| $ 7 $ | $ 6 $ | $ 72 $ | $ 72 $ | $ 612 $ |
| $ 8 $ | $ 6 $ | $ 84 $ | $ 84 $ | $ 840 $ |
| . | . | . | . | . |
| . | . | . | . | . |
| . | . | . | . | . |
| $ {k} $ | $ 6 $ | $ 12({k}-1) $ | $ 12({k}-1) $ | $ 18{k}^{2}-42{k}+24 $ |
| Dimension $ {{\boldsymbol{m}}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{15}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{16}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{18}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}^{{\bf{\infty}}}{\bf{|}} $ |
| $ 2 $ | $ 3 $ | $ 2\left({n}-1\right) $ | $ {n}-1 $ | $ 20{n}+7 $ |
| Dimension $ {{\boldsymbol{m}}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{15}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{16}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{17}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{18}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}^{{\bf{\infty}}}{\bf{|}} $ |
| $ 2 $ | $ 3 $ | $ 2\left({n}-1\right) $ | $ 0 $ | $ {n}-1 $ | $ 20{n}+7 $ |
| $ 3 $ | $ 2 $ | $ 2{n} $ | $ 1 $ | $ 3\left({n}-1\right) $ | $ 20{n}+17 $ |
| $ 4 $ | $ 2 $ | $ 2{n} $ | $ 3 $ | $ 5\left({n}-1\right) $ | $ 20{n}+27 $ |
| $ 5 $ | $ 2 $ | $ 2{n} $ | $ 5 $ | $ 7\left({n}-1\right) $ | $ 20{n}+37 $ |
| $ 6 $ | $ 2 $ | $ 2{n} $ | $ 7 $ | $ 9\left({n}-1\right) $ | $ 20{n}+47 $ |
| . | . | . | . | . | . |
| . | . | . | . | . | . |
| . | . | . | . | . | . |
| $ {m} $ | $ 2 $ | $ 2{n} $ | $ 2{m}-5 $ | $ 2{m}{n}-2{m}-3{n}+3 $ | $ 20{n}+10{m}-13 $ |
| $ {{\boldsymbol{m}}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{14}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{15}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{16}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{17}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{18}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{20}}}{\bf{|}} $ | $ {\bf{|}}{\boldsymbol{f}}_{{\bf{35}}}{\bf{|}} $ |
| $ 1 $ | $ 2{n}+1 $ | $ 2 $ | $ 4{n}-2 $ | $ 0 $ | $ 0 $ | $ 2{n}-1 $ | $ 0 $ |
| $ 2 $ | $ 2{n}+2 $ | $ 2 $ | $ 8{n}-2 $ | $ 2 $ | $ 2{n}-2 $ | $ 4{n}-2 $ | $ 2{n}-1 $ |
| $ 3 $ | $ 2{n}+3 $ | $ 2 $ | $ 12{n}-2 $ | $ 4 $ | $ 4{n}-4 $ | $ 6{n}-3 $ | $ 4{n}-2 $ |
| . | . | . | . | . | . | . | . |
| . | . | . | . | . | . | . | . |
| . | . | . | . | . | . | . | . |
| $ {m} $ | $ 2{n}+{m} $ | $ 2 $ | $ 4{m}{n}-2 $ | $ 2{m}-2 $ | $ 2{m}{n}-2\left({m}+{n}\right)+2 $ | $ 2{m}{n}-{m} $ | $ 2{m}{n}-\left({m}+2{n}\right)+1 $ |
