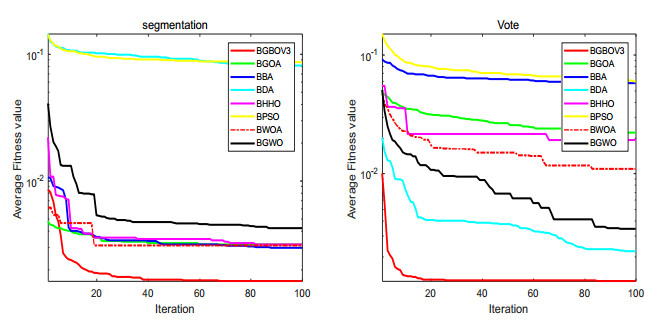
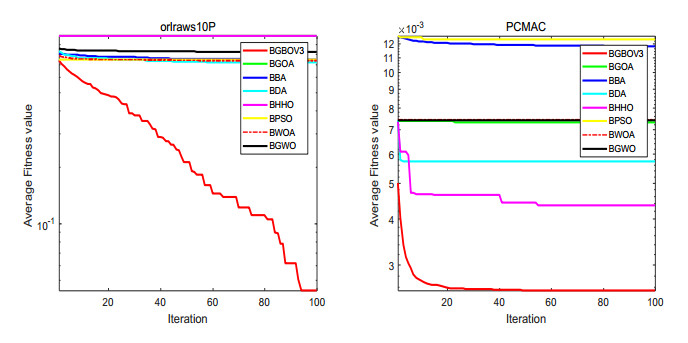
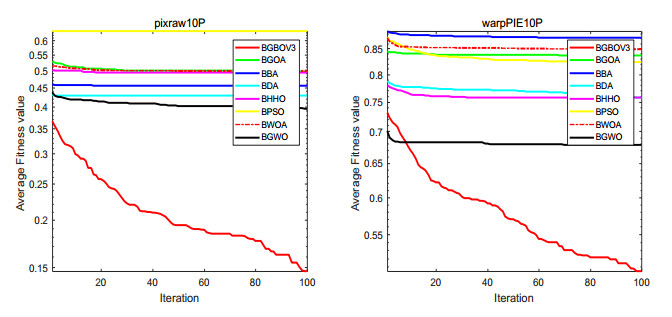
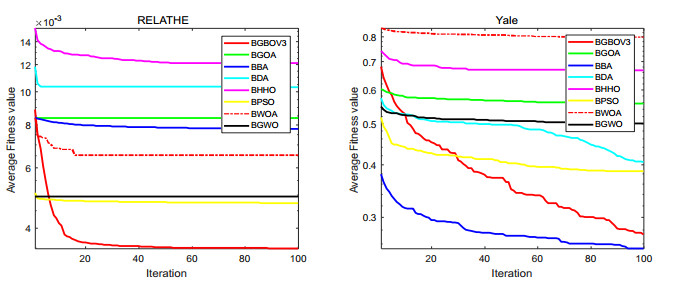
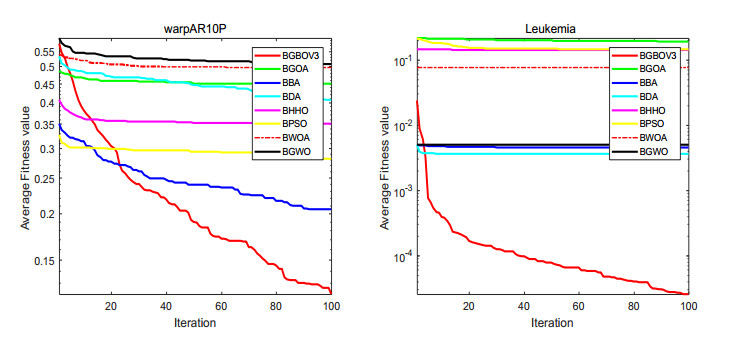
|
[1]
|
M. Chen, S. Mao, Y. Liu, Big data: A Survey, Mobile Netw. Appl., 19 (2014), 171-209. doi: 10.1007/s11036-013-0489-0

|
|
[2]
|
I. Guyon, A. Elisseeff, An introduction of variable and feature selection, J. Mach. Learn Res., 3 (2003), 1157-1182.
|
|
[3]
|
Y. Wan, M. Wang, Z. Ye, X. Lai, A feature selection method based on modified binary coded ant colony optimization algorithm, Appl. Soft Comput., 49 (2016), 248-258. doi: 10.1016/j.asoc.2016.08.011

|
|
[4]
|
H. Liu, H. Motoda, Feature selection for knowledge discovery and data mining, Kluwer Academic, 2012.
|
|
[5]
|
Z. Sun, G. Bebis, R. Miller, Object detection using feature subset selection, Pattern Recogn., 37 (2004), 2165-2176. doi: 10.1016/j.patcog.2004.03.013

|
|
[6]
|
H. Liu, H. Motoda, Feature Extraction, Construction and Selection: A Data Mining Perspective Springer Science & Business Media, Boston, MA, 1998.
|
|
[7]
|
Z. Zheng, X. Wu, R. K. Srihari, Feature selection for text categorization on imbalanced data, ACM Sigkdd Explor. Newsl., 6 (2004), 80-89. doi: 10.1145/1007730.1007741

|
|
[8]
|
H. Uguz, A two-stage feature selection method for text categorization by using information gain, principal component analysis and genetic algorithm, Knowl.-Based Syst., 24 (2011), 1024-1032. doi: 10.1016/j.knosys.2011.04.014

|
|
[9]
|
H. K. Ekenel, B. Sankur, Feature selection in the independent component subspace for face recognition, Pattern Recogn. Lett., 25 (2004), 1377-1388. doi: 10.1016/j.patrec.2004.05.013

|
|
[10]
|
H. R. Kanan, K. Faez, An improved feature selection method based on ant colony optimization (ACO) evaluated on face recognition system, Appl. Math. Comput., 205 (2008), 716-725.
|
|
[11]
|
F. Model, P. Adorjan, A. Olek, C. Piepenbrock, Feature selection for DNA methylation based cancer classification, Bioinformatics, 17 (2001), S157-S164. doi: 10.1093/bioinformatics/17.suppl_1.S157

|
|
[12]
|
N. Chuzhanova, A. J. Jones, S. Margetts, Feature selection for genetic sequence classification, Bioinformatics, 14 (1998), 139-143. doi: 10.1093/bioinformatics/14.2.139

|
|
[13]
|
S. Tabakhi, A. Najafi, R. Ranjbar, P. Moradi, Gene selection for microarray data classification using a novel ant colony optimization, Neurocomputing, 168 (2015), 1024-1036. doi: 10.1016/j.neucom.2015.05.022

|
|
[14]
|
D. Liang, C. F. Tsai, H. T. Wu, The effect of feature selection on financial distress prediction, Knowl.-Based Syst., 73 (2015), 289-297. doi: 10.1016/j.knosys.2014.10.010

|
|
[15]
|
M. Ramezani, P. Moradi, F. A. Tab, Improve performance of collaborative filtering systems using backward feature selection, in The 5th Conference on Information and Knowledge Technology, (2013), 225-230.
|
|
[16]
|
B. Tseng, Tzu Liang Bill, C. C. Huang, Rough set-based approach to feature selection in customer relationship management, Omega, 35 (2007), 365-383. doi: 10.1016/j.omega.2005.07.006

|
|
[17]
|
R. Sawhney, P. Mathur, R. Shankar, A firefly algorithm based wrapper-penalty feature selection method for cancer diagnosis, in International Conference on Computational Science and Its Applications, Springer, Cham, (2018), 438-449.
|
|
[18]
|
B. Guo, R. I. Damper, S. R. Gunn, J. D. B. Nelson, A fast separability-based feature-selection method for high-dimensional remotely sensed image classification, Pattern Recogn., 41 (2008), 1653-1662. doi: 10.1016/j.patcog.2007.11.007

|
|
[19]
|
R. Abraham, J. B. Simha, S. S. Iyengar, Medical datamining with a new algorithm for feature selection and naive bayesian classifier, in 10th International Conference on Information Technology (ICIT 2007), IEEE, 2007, 44-49.
|
|
[20]
|
L. Yu, H. Liu, Feature selection for high-dimensional data: A fast correlation-based filter solution, in Proceedings of the 20th international conference on machine learning (ICML-03), (2003), 856-863.
|
|
[21]
|
L. Cosmin, J. Taminau, S. Meganck, D. Steenhoff, A survey on filter techniques for feature selection in gene expression microarray analysis, IEEE/ACM Trans. Comput. Biol. Bioinf., 9 (2012), 1106-1119. doi: 10.1109/TCBB.2012.33

|
|
[22]
|
S. Maldonado, R. Weber, A wrapper method for feature selection using support vector machines, Inform. Sci., 179 (2009), 2208-2217. doi: 10.1016/j.ins.2009.02.014

|
|
[23]
|
J. Huang, Y. Cai, X. Xu, A hybrid genetic algorithm for feature selection wrapper based on mutual information, Pattern Recogn. Lett., 28 (2007), 1825-1844. doi: 10.1016/j.patrec.2007.05.011

|
|
[24]
|
C. Tang, X. Liu, X. Zhu, J. Xiong, M. Li, J. Xia, et al., Feature selective projection with low-rank embedding and dual laplacian regularization, IEEE Trans. Knowl. Data. Eng., 32 (2019), 1747-1760.
|
|
[25]
|
C. Tang, M. Bian, X. Liu, M. Li, H. Zhou, P. Wang, et al., Unsupervised feature selection via latent representation learning and manifold regularization, Neural Networks, 117 (2019), 163-178. doi: 10.1016/j.neunet.2019.04.015

|
|
[26]
|
S. Sharifzadeh, L. Clemmensen, C. Borggaard, S. Støier, B. K. Ersbøll, Supervised feature selection for linear and non-linear regression of L*a*b color from multispectral images of meat, Eng. Appl. Artif. Intel., 27 (2013), 211-227.
|
|
[27]
|
C. Tang, X. Zheng, X. Liu, L. Wang, Cross-view locality preserved diversity and consensus learning for multi-view unsupervised feature selection, IEEE Trans. Knowl. Data. Eng., 2021.
|
|
[28]
|
C. Tang, X. Zhu, X. Liu, L. Wang, Cross-View local structure preserved diversity and consensus learning for multi-view unsupervised feature selection, in Proceedings of the AAAI Conference on Artificial Intelligence, 33 (2019), 5101-5108.
|
|
[29]
|
M. Dorigo, Optimization, Learning and Natural Algorithms, Phd Thesis Politecnico Di Milano, 1992.
|
|
[30]
|
C. Lai, M. J. T. Reinders, L. Wessels, Random subspace method for multivariate feature selection, Pattern Recogn. Lett., 27 (2006), 1067-1076. doi: 10.1016/j.patrec.2005.12.018

|
|
[31]
|
B. Xue, M. Zhang, W. N. Browne, X. Yao, A survey on evolutionary computation approaches to feature selection, IEEE Trans. Evol. Comput., 20 (2016), 606-626. doi: 10.1109/TEVC.2015.2504420

|
|
[32]
|
J. J. Grefenstette, Optimization of control parameters for genetic algorithms, IEEE Trans. Syst. Man Cybern., 16 (1986), 122-128. doi: 10.1109/TSMC.1986.289288

|
|
[33]
|
B. G. Obaiahnahatti, J. Kennedy, A new optimizer using particle swarm theory, in MHS'95. Proceedings of the Sixth International Symposium on Micro Machine and Human Science, IEEE, (1995), 39-43.
|
|
[34]
|
K. Chen, F. Y. Zhou, X. F. Yuan, Hybrid particle swarm optimization with spiral-shaped mechanism for feature selection, Expert Syst. Appl., 128 (2019), 140-156. doi: 10.1016/j.eswa.2019.03.039

|
|
[35]
|
S. Mirjalili, A. Lewis, The whale optimization algorithm, Adv. Eng. Softw., 95 (2016), 51-67. doi: 10.1016/j.advengsoft.2016.01.008

|
|
[36]
|
S. Mirjalili, S.M. Mirjalili, A. Lewis, Grey wolf optimizer, Adv. Eng. Softw., 69 (2014), 46-61. doi: 10.1016/j.advengsoft.2013.12.007

|
|
[37]
|
S. Shahrzad, S. Mirjalili, A. Lewis, Grasshopper optimisation algorithm: Theory and application, Adv. Eng. Softw., 105 (2017), 30-47. doi: 10.1016/j.advengsoft.2017.01.004

|
|
[38]
|
E. Rashedi, H. Nezamabadi-Pour, S. Saryazdi, GSA: a gravitational search algorithm, Inform. Sci., 179 (2009), 2232-2248. doi: 10.1016/j.ins.2009.03.004

|
|
[39]
|
S. Li, H. Chen, M. Wang, A. A. Heidari, S. Mirjalili, Slime mould algorithm: A new method for stochastic optimization, Future Gener. Comput. Syst., 111 (2020), 300-323. doi: 10.1016/j.future.2020.03.055

|
|
[40]
|
Y. Yang, H. Chen, A. A. Heidari, A. H. Gandomi, Hunger games search: Visions, conception, implementation, deep analysis, perspectives, and towards performance shifts, Expert Syst. Appl., 171 (2021), 114864.
|
|
[41]
|
I. Ahmadianfar, O. Bozorg-Haddad, X. Chu, Gradient-based optimizer: A new metaheuristic optimization algorithm, Inform. Sci., 540 (2020), 131-159. doi: 10.1016/j.ins.2020.06.037

|
|
[42]
|
S. Mirjalili, Dragonfly algorithm: a new meta-heuristic optimization technique for solving single-objective, discrete, and multi-objective problems, Neural Comput. Appl., 27 (2016), 1053-1073. doi: 10.1007/s00521-015-1920-1

|
|
[43]
|
T. J. Ypma, Historical development of the Newton-Raphson method, SIAM Rev., 37 (1995), 531-551. doi: 10.1137/1037125

|
|
[44]
|
S. Mirjalili, A. Lewis, S-shaped versus V-shaped transfer functions for binary particle swarm optimization, Swarm Evol. Comput., 9 (2013), 1-14. doi: 10.1016/j.swevo.2012.09.002

|
|
[45]
|
H. Liu, J. Li, L. Wong, A comparative study on feature selection and classification methods using gene expression profiles and proteomic patterns, Genome Inform., 13 (2002), 51-60.
|
|
[46]
|
M. Dash, H. Liu, Feature selection for classification, Intell. Data Anal., 1 (1997), 131-156. doi: 10.3233/IDA-1997-1302

|
|
[47]
|
W. Siedlecki, J. Sklansky, A note on genetic algorithms for large-scale feature selection, Pattern Recogn. Lett., 10 (1989), 335-347. doi: 10.1016/0167-8655(89)90037-8

|
|
[48]
|
R. Leardi, R. Boggia, M. Terrile, Genetic algorithms as a strategy for feature selection, J. Chemom., 6 (1992), 267-281. doi: 10.1002/cem.1180060506

|
|
[49]
|
I. S. Oh, J. S. Lee, B. R. Moon, Hybrid genetic algorithms for feature selection, IEEE Trans. Pattern Anal., 26 (2004), 1424-1437. doi: 10.1109/TPAMI.2004.105

|
|
[50]
|
R. Storn, K. Price, Differential evolution-a simple and efficient heuristic for global optimization over continuous spaces, J. Global Optim., 11 (1997), 341-359. doi: 10.1023/A:1008202821328

|
|
[51]
|
B. Xue, W. Fu, M. Zhang, Differential evolution (DE) for multi-objective feature selection in classification, in Proceedings of the Companion Publication of the 2014 Annual Conference on Genetic and Evolutionary Computation, (2014), 83-84.
|
|
[52]
|
D. Karaboga, B. Akay, A comparative study of artificial bee colony algorithm, Appl. Math. Comput., 214 (2009), 108-132.
|
|
[53]
|
A. A. Heidari, S. Mirjalili, H. Faris, I. Aljarah, M. Mafarja, H. Chen, Harris hawks optimization: Algorithm and applications, Future Gener. Comput. Syst., 97 (2019), 849-872. doi: 10.1016/j.future.2019.02.028

|
|
[54]
|
A. Faramarzi, M. Heidarinejad, S. Mirjalili, A. H. Gandomi, Marine predators algorithm: A nature-inspired metaheuristic, Expert Syst. Appl., 152 (2020), 113377. doi: 10.1016/j.eswa.2020.113377

|
|
[55]
|
O. S. Qasim, Z. Algamal, Feature selection using particle swarm optimization-based logistic regression model, Chemom. Intell. Lab. Syst., 182 (2018), 41-46. doi: 10.1016/j.chemolab.2018.08.016

|
|
[56]
|
K. Chen, F. Y. Zhou, X. F. Yuan, Hybrid particle swarm optimization with spiral-shaped mechanism for feature selection, Expert Syst. Appl., 128 (2019), 140-156. doi: 10.1016/j.eswa.2019.03.039

|
|
[57]
|
B. Xue, M. Zhang, W. N. Browne, Particle swarm optimization for feature selection in classification: a multi-objective approach, IEEE Trans. Cybern., 43 (2013), 1656-1671. doi: 10.1109/TSMCB.2012.2227469

|
|
[58]
|
E. Emary, H. M. Zawbaa, A. E. Hassanien, Binary grey wolf optimization approaches for feature selection, Neurocomputing, 172 (2016), 371-381. doi: 10.1016/j.neucom.2015.06.083

|
|
[59]
|
P. Hu, J. S. Pan, S. C. Chu, Improved binary grey wolf optimizer and its application for feature selection, Knowl.-Based Syst., 195 (2020), 105746. doi: 10.1016/j.knosys.2020.105746

|
|
[60]
|
T. Qiang, X. Chen, X. Liu, Multi-strategy ensemble grey wolf optimizer and its application to feature selection, Appl. Soft Comput., 76 (2019), 16-30. doi: 10.1016/j.asoc.2018.11.047

|
|
[61]
|
M. M. Mafarja, S. Mirjalili, Whale optimization approaches for wrapper feature selection, Appl. Soft Comput., 62 (2018), 441-453. doi: 10.1016/j.asoc.2017.11.006

|
|
[62]
|
M. M. Mafarja, S. Mirjalili, Hybrid whale optimization algorithm with simulated annealing for feature selection, Neurocomputing, 260 (2017), 302-312. doi: 10.1016/j.neucom.2017.04.053

|
|
[63]
|
R. K. Agrawal, B. Kaur, S. Sharma, Quantum based whale optimization algorithm for wrapper feature selection, Appl. Soft Comput., 89 (2020), 106092. doi: 10.1016/j.asoc.2020.106092

|
|
[64]
|
C. R. Hwang, Simulated annealing: Theory and applications, Acta. Appl. Math., 12 (1988), 108-111.
|
|
[65]
|
H. Shareef, A. A. Ibrahim, A. H. Mutlag, Lightning search algorithm, Appl. Soft Comput., 36 (2015), 315-333. doi: 10.1016/j.asoc.2015.07.028

|
|
[66]
|
S. Mirjalili, S. M. Mirjalili, A. Hatamlou, Multi-verse optimizer: a nature-inspired algorithm for global optimization, Neural Comput. Appl., 27 (2016), 495-513. doi: 10.1007/s00521-015-1870-7

|
|
[67]
|
H. Abedinpourshotorban, S. M. Shamsuddin, Z. Beheshti, D. N. A. Jawawib, Electromagnetic field optimization: A physics-inspired metaheuristic optimization algorithm, Swarm Evol. Comput., 26 (2016), 8-22. doi: 10.1016/j.swevo.2015.07.002

|
|
[68]
|
A. Y. S. Lam, V. O. K. Li, Chemical-reaction-inspired metaheuristic for optimization, IEEE Trans. Evol. Comput., 14 (2009), 381-399.
|
|
[69]
|
F. A. Hashim, E. H. Houssein, M. S. Mabrouk, W. Al-Atabany, S. Mirjalili, Henry gas solubility optimization: A novel physics-based algorithm, Future Gener. Comp. Syst., 101 (2019), 646-667. doi: 10.1016/j.future.2019.07.015

|
|
[70]
|
R. Meiri, J. Zahavi, Using simulated annealing to optimize the feature selection problem in marketing applications, Eur. J. Oper. Res., 171 (2006), 842-858. doi: 10.1016/j.ejor.2004.09.010

|
|
[71]
|
S. W. Lin, Z. J. Lee, S. C. Chen, T. Y. Tseng, Parameter determination of support vector machine and feature selection using simulated annealing approach, Appl. Soft Comput., 8 (2008), 1505-1512. doi: 10.1016/j.asoc.2007.10.012

|
|
[72]
|
E. Rashedi, H. Nezamabadi-Pour, S. Saryazdi, BGSA: Binary gravitational search algorithm, Nat. Comput., 9 (2010), 727-745. doi: 10.1007/s11047-009-9175-3

|
|
[73]
|
S. Nagpal, S. Arora, S. Dey, Feature selection using gravitational search algorithm for biomedical data, Procedia Comput. Sci., 115 (2017), 258-265. doi: 10.1016/j.procs.2017.09.133

|
|
[74]
|
P. C. S. Rao, A. J. S. Kumar, Q. Niyaz, P. Sidike, V. K. Devabhaktuni, Binary chemical reaction optimization based feature selection techniques for machine learning classification problems, Expert Syst. Appl., 167 (2021), 114169. doi: 10.1016/j.eswa.2020.114169

|
|
[75]
|
N. Neggaz, E. H. Houssein, K. Hussain, An efficient henry gas solubility optimization for feature selection, Expert Syst. Appl., 152 (2020), 113364. doi: 10.1016/j.eswa.2020.113364

|
|
[76]
|
R. V. Rao, V. J. Savsani, D. P. Vakharia, Teaching-learning-based optimization: a novel method for constrained mechanical design optimization problems. Comput. Aided Design, 43 (2011), 303-315. doi: 10.1016/j.cad.2010.12.015

|
|
[77]
|
S. Hosseini, A. A. Khaled, A survey on the imperialist competitive algorithm metaheuristic: implementation in engineering domain and directions for future research, Appl. Soft Comput., 24 (2014), 1078-1094. doi: 10.1016/j.asoc.2014.08.024

|
|
[78]
|
R. Moghdani, K. Salimifard, Volleyball premier league algorithm, Appl. Soft Comput., 64 (2017), 161-185.
|
|
[79]
|
H. C. Kuo, C. H. Lin, Cultural evolution algorithm for global optimizations and its applications, J. Appl. Res. Technol., 11 (2013), 510-522. doi: 10.1016/S1665-6423(13)71558-X

|
|
[80]
|
M. Allam, M. Nandhini, Optimal feature selection using binary teaching learning based optimization algorithm, J. King Saud Univ.-Comput. Inform. Sci., 10 (2018).
|
|
[81]
|
S. J. Mousavirad, H. Ebrahimpour-Komleh, Feature selection using modified imperialist competitive algorithm, in ICCKE 2013, IEEE, (2013), 400-405.
|
|
[82]
|
A. Keramati, M. Hosseini, M. Darzi, A. A. Liaei, Cultural algorithm for feature selection, in The 3rd International Conference on Data Mining and Intelligent Information Technology Applications, IEEE, (2011), 71-76.
|
|
[83]
|
D. H. Wolpert, W. G. Macready, No free lunch theorems for optimization, IEEE Trans. Evol. Comput., 1 (1997), 67-82. doi: 10.1109/4235.585893

|
|
[84]
|
A. Fink, S. Vo, Solving the continuous flow-shop scheduling problem by metaheuristics, Eur. J. Oper. Res., 151 (2003), 400-414. doi: 10.1016/S0377-2217(02)00834-2

|
|
[85]
|
J. Kennedy, R. C. Eberhart, A discrete binary version of the particle swarm algorithm, in 1997 IEEE International conference on systems, man, and cybernetics. Computational cybernetics and simulation, IEEE, 5 (1997), 4104-4108.
|
|
[86]
|
E. Rashedi, H. Nezamabadi-Pour, S. Saryazdi, BGSA: binary gravitational search algorithm, Nat. Comput., 9 (2010), 727-745. doi: 10.1007/s11047-009-9175-3

|
|
[87]
|
N. S. Altman, An introduction to kernel and nearest-neighbor nonparametric regression, Am. Stat., 46 (1992), 175-185.
|
|
[88]
|
F. Pernkopf, Bayesian network classifiers versus selective k-NN classifier, Pattern Recogn., 38 (2005), 1-10. doi: 10.1016/j.patcog.2004.05.012

|
|
[89]
|
A. Asuncion, D. Newman, UCI Machine Learning Repository, University of California, 2007.
|
|
[90]
|
E. Emary, H. M. Zawbaa, A. E. Hassanien, Binary ant lion approaches for feature selection, Neurocomputing, 213 (2016), 54-65. doi: 10.1016/j.neucom.2016.03.101

|
|
[91]
|
Arizona State University's (ASU) repository, Available from: http://featureselection.asu.edu/datasets.php.
|
|
[92]
|
A. I. Hammouri, M. Mafarja, M. A. Al-Betar, M. A. Awadallah, I. Abu-Doush, An improved Dragonfly Algorithm for feature selection, Knowl.-Based Syst., 203 (2020), 106131. doi: 10.1016/j.knosys.2020.106131

|
|
[93]
|
H. Faris, M. M. Mafarja, A. A. Heidari, I. Aljarah, A. M. Al-Zoubi, S. Mirjalili, et al., An Efficient Binary Salp Swarm Algorithm with Crossover Scheme for Feature Selection Problems, Knowl.-Based Syst., 154 (2018), 43-67. doi: 10.1016/j.knosys.2018.05.009

|
|
[94]
|
M. Mafarja, S.Mirjalili, Whale optimization approaches for wrapper feature selection, Appl. Soft Comput., 62 (2018), 441-453. doi: 10.1016/j.asoc.2017.11.006

|
|
[95]
|
M. Mafarja, I. Aljarah, H. Faris, A. I. Hammouri, A. M. Al-Zoubi, S. Mirjalili, Binary grasshopper optimisation algorithm approaches for feature selection problems, Expert Syst. Appl., 117 (2019), 267-286. doi: 10.1016/j.eswa.2018.09.015

|
|
[96]
|
E. Emary, H. M. Zawbaa, A. E. Hassanien, Binary grey wolf optimization approaches for feature selection, Neurocomputing, 172 (2016), 371-381. doi: 10.1016/j.neucom.2015.06.083

|









 DownLoad:
DownLoad: