Effect of different divalent cations on the kinetics and fidelity of DNA polymerases

Ashwani Kumar Vashishtha; William H. Konigsberg; Ashwani Kumar Vashishtha; William H. Konigsberg

doi:10.3934/biophy.2018.4.272

AIMS Biophysics

2018, Volume 5, Issue 4: 272-289. doi: 10.3934/biophy.2018.4.272

Previous Article Next Article

Review

Effect of different divalent cations on the kinetics and fidelity of DNA polymerases

Department of Molecular Biophysics and Biochemistry, Yale University, New Haven, Connecticut 065208024, USA

Received: 17 September 2018 Accepted: 23 November 2018 Published: 11 December 2018

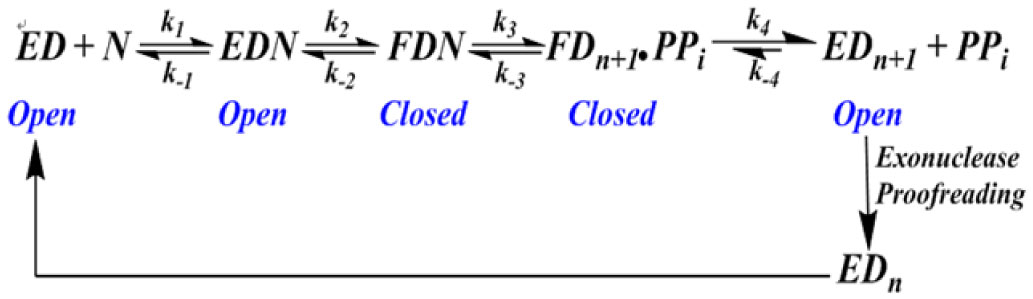
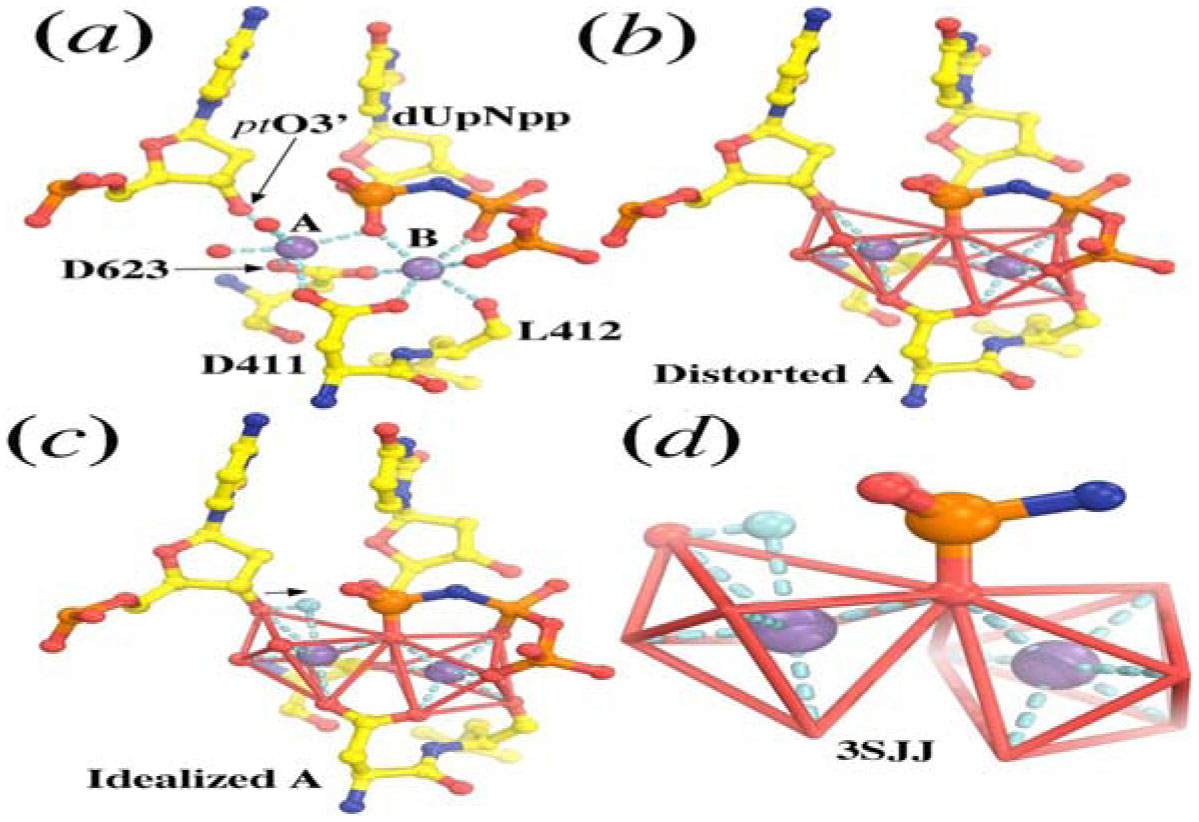
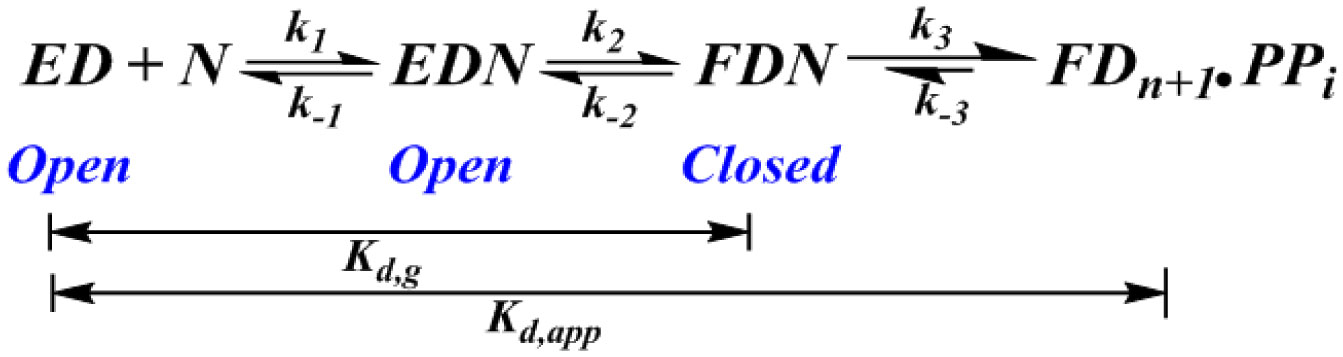
DNA polymerases (DNA pols) are essential for accurately copying genomes of all organisms. The polymerase and exonuclease activities associated with DNA pols require the presence of two divalent cations which occupy the A and B metal ion sites. Even though the two-metal ion mechanism is generally applicable for all DNA pols, a third metal ion was proposed to be essential for phosphoryl transfer reaction. The metal ion in the A site is coordinated by six ligands including the 3′ hydroxyl group of the primer, the carboxylates of two aspartic acid residues, as well as water molecules and the metal ion exhibits a distorted octahedral geometry. This metal ion plays a crucial role in lowering the pK_aof the 3′ hydroxyl group of the primer increasing its nucleophilicity for attack on α phosphorous atom of the incoming dNTP. The metal ion occupying the B site stabilizes the transition state and is coordinated to the non-bridging oxygen atoms of the incoming dNTP and carboxylates of aspartic acid residues along with carboxyl oxygen of an adjacent peptide bond. In a similar fashion, two divalent cations are required for the 3′–5′ exonuclease activity of DNA pols. Analogous to their role in the polymerase active site, one divalent cation lowers the pK_a of the water molecule making it a more potent nucleophile and the other cation helps to stabilize the transition state, assisting in excision of the 3′ terminal nucleotide. These divalent cations affect the various fidelity checkpoints along minimal kinetic scheme for DNA pols. The effect of different divalent cations on various steps in the kinetic scheme, including their influence on ground-state binding affinity, base selectivity, efficiency of extension past a mismatch and the exonuclease activity are discussed. We have also attempted to explain why only certain divalent cations act as cofactors for various DNA pols based on their properties including ionic radii, coordination geometry, and their ability to lower the pK_a of the 3′ hydroxyl group of primer strand.

Keywords:

Citation: Ashwani Kumar Vashishtha, William H. Konigsberg. Effect of different divalent cations on the kinetics and fidelity of DNA polymerases[J]. AIMS Biophysics, 2018, 5(4): 272-289. doi: 10.3934/biophy.2018.4.272

Related Papers:

[1]	Carlo Bianca . Differential equations frameworks and models for the physics of biological systems. AIMS Biophysics, 2024, 11(2): 234-238. doi: 10.3934/biophy.2024013
[2]	Carlo Bianca . Interplay and multiscale modeling of complex biological systems. AIMS Biophysics, 2022, 9(1): 56-60. doi: 10.3934/biophy.2022005
[3]	Carlo Bianca . Theoretical frameworks and models for biological systems. AIMS Biophysics, 2020, 7(3): 167-168. doi: 10.3934/biophy.2020013
[4]	Carlo Bianca . Mathematical and computational modeling of biological systems: advances and perspectives. AIMS Biophysics, 2021, 8(4): 318-321. doi: 10.3934/biophy.2021025
[5]	Bradley Vis, Jonathan J. Powell, Rachel E. Hewitt . Imaging flow cytometry methods for quantitative analysis of label-free crystalline silica particle interactions with immune cells. AIMS Biophysics, 2020, 7(3): 144-166. doi: 10.3934/biophy.2020012
[6]	Mario P. Carante, Francesca Ballarini . Modelling cell death for cancer hadrontherapy. AIMS Biophysics, 2017, 4(3): 465-490. doi: 10.3934/biophy.2017.3.465
[7]	Mehmet Yavuz, Fuat Usta . Importance of modelling and simulation in biophysical applications. AIMS Biophysics, 2023, 10(3): 258-262. doi: 10.3934/biophy.2023017
[8]	Domenico Lombardo, Pietro Calandra, Maria Teresa Caccamo, Salvatore Magazù, Luigi Pasqua, Mikhail A. Kiselev . Interdisciplinary approaches to the study of biological membranes. AIMS Biophysics, 2020, 7(4): 267-290. doi: 10.3934/biophy.2020020
[9]	Nor Afiqah Mohd Aris, Siti Suhana Jamaian . Dynamical analysis of fractional-order chemostat model. AIMS Biophysics, 2021, 8(2): 182-197. doi: 10.3934/biophy.2021014
[10]	Raghvendra P Singh, Guillaume Brysbaert, Marc F Lensink, Fabrizio Cleri, Ralf Blossey . Kinetic proofreading of chromatin remodeling: from gene activation to gene repression and back. AIMS Biophysics, 2015, 2(4): 398-411. doi: 10.3934/biophy.2015.4.398

Abstract

1. Introduction

In the last decades the interest towards the complex systems for the modeling and studying of biological systems has been ever growing (see among the others [1]–[11], and references therein).

More generally, the study of complex systems [12]–[15] is one of the main resaerch topics of the last years. However the description of such systems, with related quantities and parameters, depends on the particular approach that is chosen. Different mathematical frameworks have been proposed depending on the representation scale of the system. In the present paper, we turn our attention on the so-called “kinetic approach” which is a generalization of the model proposed by Boltzmann [16] to describe the statistical dynamics of gases, and is based on a suitable version of his equation. The interacting entities of the system are called active particles. The evolution of the system is described by a distribution function which depends on the time, on the mechanical variables (i.e. space and velocity) and on a scalar variable called “activity”, whose meaning depends on the current application [17]. In the kinetic theoretical description of a complex system, the evolution depends on an integral term that defines the interactions between the particles.

A new modeling framework has been recently proposed for the description of a complex system under the action of an external force field: the thermostatted kinetic theory [18], [19]. The action of an external force field moves the systems out of the equilibrium. In this framework, the complex system under investigation is divided into n functional subsystems such that particles belonging to the same functional subsystem share the same strategy (in a suitable sense, the same aim) [20]. The macroscopic state is described by specific pth-order moments (see Section 2). The introduction of a dissipative term, called thermostat, constrains the system to keep the 2nd-order moment of the system, which can be regarded as the physical global activation energy, costant in time. The evolution of the system is then described by a system of nonlinear integro-differential equations with quadratic nonlinearity. The thermostatted kinetic theory has been developed, for instance, in the study of Kac equation too [21].

A biological system is constituted by a large number of interacting entities (the active particles) whose microscopic state can be described by a real variable (activity) which represents the individual ability to express a specific strategy. The active particles of a biological systems have the ability to develop behaviour that cannot only be explained by the classical mechanics laws, and, in some cases, can generate proliferative and/or destructive processes. By using the functional subsystems, the complexity can be reduced by decomposing the biological systems into several interacting subsystems [22]. In fact, this approach can possibly be considered the first fundamental contribution to biological studies [23]. The decomposition method can be regarded as a tool to reduce complexity. In fact, the active particles in each module, which is described as a functional subsystem, are not of the same type, while they express the same strategy collectively. Thus the system can be studied regarding the evolution of each functional subsystem rather than the single active particle.

A biological system can be described at different representation scales, depending on the particular application. For instance, the microscopic scale in biology corresponds to cells, while the dynamics of cells depends on the dynamics at the lower molecular scale, namely the scale of molecules. In some cases, the complexity of the system induces the use of even a larger scale, the macroscopic scale, which corresponds to population dynamics [24]–[26]. By using the framework of kinetic theory, the microscopic scale models the interactive dynamics among active particles, and it is described by using the activity variable, while the macroscopic state of the whole system is described by the pth-order moments related to the distribution function. The description of the biological systems depends on the pairwise interactions among the active particles (microscopic scale) and the state of the overall system (macroscopic scale).

In the last years, a widely interesting biological application is the modeling of the dynamics of epidemics with virus variations (see [3]–[6], [27]–[30], and references therein). One of the most used model is SIR (Susceptible, Infectious, Recovered), with its variants (see [6], [31]–[36], and references therein). In this contest, the system may be decomposed into 3 functional subsystem of interacting individual. The first functional subsystems denotes the healthy individuals whose microscopic state models the susceptibility to contract the pathological state. The second functional subsystems represents denotes individuals healthy carriers of the virus, whose microscopic state models the infectivity of the virus. The thirs functional subsystem denotes the individuals affected by the virus at the first and subsequent stages, whose microscopic state models the progression of both the infectivity and the pathological state.

Beyond biological systems, the thermostatted framework is used to study, among other topics: socio-economic systems [37], [38], pedestrian dynamics [39], vehicular traffic [40], crowd dynamics [41], human feelings [42], [43] and opinion formation [44].

The present paper deals with the discrete thermostatted framework: the activity variable attains its value in a discrete subset of ℝ, so that in this case the evolution of the system is modeled by a system of nonlinear ordinary differential equations with quadratic nonlinearity. The existence and uniqueness of the solutions is assured for both the related Cauchy and nonequilibrium stationary problems [45], [46]. The analysis of the discrete framework is important in order to define the methods for the numerical simulations.

The use of a discrete variable is not only a technical choice. In some application, this is related to the description of the current biological systems [47], [48]. For instance in order to describe the state of a cell, three values are assigned to the activity variable: normal, infected, dead. Moreover, in socio-economic systems [37], [38], the discretization of the activity variable has conceptual reason. As a matter of fact, a continuous activity variable for the socio-economic quantities may not make any sense: e.g. the wealth-state.

The main aim of this paper is the study of stability in the Hadamard sense of the discrete themostatted kinetic framework. A model is stable in the Hadamard sense if the solution exists and is unique, and it depends continuously on the initial data. In the current paper, two Cauchy problems, related to the discrete thermostatted framework that differ for their initial data, are considered. The distance between the initial data is estimated by a δ > 0. Using typical analytical techniques, this paper shows that the distance between the corresponding solutions, in a suitable norm, is estimated continuously in function of δ (see [49] for the continuous case), i.e. if δ goes to 0, the two solutions collapse.

The importance of continuous dependence of the solutions on the initial data is related, among other things, to the numerical simulations that can be performed [7], [50]–[52]. As a matter of fact, since the initial data is in general derived from a statistical analysis, its value is affected by an error, then the stability of the model may assure a “slow and small propagation” of such an error during the evolution, at least for small time intervals. Among others, the application of the discrete thermostatted framework to the epidemic dynamics may be considered. In this case the initial data is obtained from a statistical study, then it may be affected by an error. The continuous dependence with respect to the initial data is an important issue such that the numerical simulations can be performed such that the solution represents the evolution of epidemic with sufficient accuracy.

This paper presents a first step towards the study of stability and dependence on the initial data for the discrete kinetic thermostatted framework.

The contents of this paper are divided into 5 more sections which follow this brief Introduction. In Section 2 the discrete thermostatted kinetic framework is presented, and the related state of art about existence and uniqueness of the solutions. In Section 3 the main Theorem about the dependence on the initial data is presented, after introducing suitable norms. Section 4 deals with the proof of Theorem, proving the stability and giving an explicit form to the constant in the final inequality which is obtained by performing some typical analytic arguments. Finally Section 5 deals with the future research perspective that may follow this paper, which is meant as a first step towards the dependence on the initial data for the discrete thermostatted kinetic framework.

2. The discrete thermostatted framework

Let us consider a complex system 𝒞 homogeneous with respect to the mechanical variables, i.e. space and velocity, which is divided into n (where n ∈ ℕ is fixed) functional subsystems such that particles belonging to the same functional subsystem share the same strategy [20]. Since some physical and life sciences models are characterized by discrete variables, in this paper the microscopic state of the system is described by a scalar variable u, called activity, which attains its values in a fixed discrete subset of ℝ, i.e.

$u \in I_{u} : = {u_{1}, u_{2}, \dots, u_{n}} \subseteq ℝ .$

The ith functional subsystem, for i ∈ {1, 2, …, n}, is described by:

$f_{i} (t) : [0, + \infty [\to ℝ^{+},$

which is the discrete distribution function, at time t > 0, of active particles with activity u_i. The distribution function of the whole system reads:

$f (t, u) = \sum_{i = 1}^{n} f_{i} (t) δ (u - u_{i}),$

where δ is the Delta function. The quantity f(t,u)du represents the number of active particles, at time t > 0, in the elementary volume [u, u + du].

The related vector distribution function of the system 𝒞 reads:

$f = f (t) : = (f_{1} (t), f_{2} (t), \dots, f_{n} (t)) .$

The macroscopic state of the system, at a time t > 0, is described by the pth-order moment, which is defined, for p ∈ ℕ, as:

$𝔼_{p} [f] (t) = \sum_{i = 1}^{n} u_{i}^{p} f_{i} (t) .$

The physical meaning of the moment depends on the value of p, e.g. the local density, the linear activity momentum and the global activation energy are obtained, respectively, for p = 0, p = 1 and p = 2.

The interaction among the active particles is described by the following parameters:

$\sum_{i = 1}^{n} B_{h k}^{i} = 1.$ (2.1)

Let F_i(t) : [0; +∞[→ ℝ⁺, for i ∈ {1,2, …, n}, be an external force acting on the ith functional subsystem, such that the external force field writes: $F (t) = (F_{1} (t), F_{2} (t), \dots, F_{n} (t))$ .

A discrete thermostat is included in order to keep constant the 2nd-order moment 𝔼₂[f](t). Let 𝔼₂ be the fixed value of the 2nd-order moment, then the evolution of the ith functional subsystem, for i ∈ {1,2, …, n}, is:

$\frac{d f_{i}}{d t} (t) = J_{i} [f] (t) + F_{i} (t) - \sum_{j = 1}^{n} (\frac{u_{j}^{2} (J_{j} [f] + F_{j})}{𝔼_{2}}) f_{i} (t),$ (2.2)

where the interaction operator J_i[f](t) can be split as follows:

$J_{i} [f] (t) = G_{i} [f] (t) - L_{i} [f] (t),$

where

$G_{i} [f] (t) = \sum_{h = 1}^{n} \sum_{k = 1}^{n} η_{h k} B_{h k}^{i} f_{h} (t) f_{k} (t)$

and

$L_{i} [f] (t) = f_{i} (t) \sum_{k = 1}^{n} η_{i k} f_{k} (t)$

are the gain term operator and the loss term operator (whose expression is related to the (2.1)), respectively. The equation (2.2) is obtained by balancing the time-derivative of the distribution function related to the ith functional subsystem, i.e f_i(t), and the net flux, J_i[f](t), related to the same functional subsystem, the external force field and the thermostat. Therefore the evolution of the system (2.2) is a system of nonlinear ordinary differential equations with quadratic nonlinearity.

Let $f^{0} : = (f_{1}^{0}, f_{2}^{0}, \dots, f_{n}^{0})$ be a suitable initial data. Then the Cauchy problem related to the discrete thermostatted framework (2.2) is defined as:

$(\begin{array}{l} (2.2) & i \in {1,2, \dots, n}, t \in [0, + \infty [ \\ f (0) = f^{0} . \end{array}$ (2.3)

Definition 2.1. Fixed 𝔼₂ > 0, the space function $ℛ_{f}^{2} = ℛ_{f}^{2} (ℝ^{+}, 𝔼_{2})$ is defined as:

${f (t) \in C ([0, + \infty [; {(ℝ^{+})}^{n}) : 𝔼_{2} [f] (t) = 𝔼_{2}, \forall t} .$

Henceforth, the following assumptions are taken into account:

$u_{i} \geq 1, \forall i \in {1,2, \dots, n};$

$𝔼_{2} [f^{0}] = 𝔼_{2};,$

H3 there exists η > 0 such that η_hk = η, for all h, k ∈ {1,2, …, n};

H4 there exists F > 0 such that F_i(t) = F, for all i ∈ {1,2, …, n} and for all t > 0.

Remark 2.2. The H1 is a technical assumption since the generalized framework has been treated in [53], where u_i ≥ 1 is not required.

In this framework, under the assumptions H1-H4, there exists one only function $f (t) \in C ([0, + \infty [; {(ℝ^{+})}^{n}) \cap ℛ_{f}^{2}$ which is solution of the Cauchy problem (2.3) [45].

Complex systems usually operate far from equilibrium since their evolution is related to an internal dynamics, due to the interaction between particles, and to an external dyanmics, due to the external force field. Then nonequilibrium stationary states are reached during the evolution.

The stationary problem related to (2.2) is, for i ∈ {1,2, …, n}:

$J_{i} [f] (t) + F_{i} - \sum_{j = 1}^{n} (\frac{u_{j}^{2} (J_{j} [f] + F_{j})}{𝔼_{2}}) f_{i} (t) = 0.$ (2.4)

In [46] the existence of a solution of (2.4), called non-equilibrium stationary solution, is gained, and the uniqueness is proved under some restrictions on the value of the external force F. Henceforth, without leading of generality, 𝔼₂[f] = 𝔼₀[f] = 1 is assumed.

If these further assumptions hold true:

$\sum_{i = 1}^{n} u_{i} B_{h k}^{i} = 0, \forall h, k \in {1,2, \dots, n},$

$\sum_{i = 1}^{n} u_{i}^{2} B_{h k}^{i} = u_{h}^{2}, \forall h, k \in {1,2, \dots, n},$

then the evolution equation of 𝔼₁[f](t) reads [46]:

$𝔼_{1} [f] (t) = 𝔼_{1} [f^{0}] e^{- ϕ (t)} + k,$

where

$ϕ (s) = \int_{0}^{s} (η + \sum_{j = 1}^{n} u_{j}^{2} F_{j}) d τ,$

and

$k = \frac{\sum_{i = 1}^{n} u_{i} F_{i}}{η + \sum_{i = 1}^{n} u_{i}^{2} F_{i}} .$

Moreover:

$𝔼_{1} [f] (t) \overset{t \to \infty}{\to} k .$

Remark 2.3. The assumptions H3-H4 are not restricted. Indeed the results of existence and, if possible, uniqueness can be proved if there exist F,η > 0 such that:

η_hk ≤ η, for all h, k ∈ {1,2, …, n};
F_i(t) ≤ F, for all i ∈ {1,2, …, n} and for all t > 0.

3. Dependence on the initial data

Under suitable assumptions the Cauchy problem (2.3) has a unique solution $f (t) \in C ([0, + \infty [; {(ℝ^{+})}^{n}) \cap ℛ_{f}^{2}$ [45].

Let us consider now two Cauchy problems related to the discrete thermostatted framework (2.2):

$(\begin{array}{l} (2.2) & t \in [0, + \infty [ \\ f (0) = f_{1}^{0} \end{array} (\begin{array}{l} (2.2) & t \in [0, + \infty [ \\ f (0) = f_{2}^{0} \end{array}$ (3.1)

where

$f_{1}^{0} = (f_{11}^{0}, f_{12}^{0}, \dots, f_{1 n}^{0})$ and

$f_{2}^{0} = (f_{21}^{0}, f_{22}^{0}, \dots, f_{2 n}^{0})$ are two different initial data.

If the assumptions H1-H6 hold true, there exist $f_{1} (t), f_{2} (t) \in C ([0, + \infty [; {(ℝ^{+})}^{n}) \cap ℛ_{f}^{2}$ solutions of the Cauchy problems (3.1) with initial data $f_{1}^{0}$ and $f_{2}^{0}$ , respectively. The main result of this paper estimates the distance between the two solutions for T > 0, i.e.

$\begin{array}{l} {‖ f_{1} (t) - f_{2} (t) ‖}_{C ([0, T]; {(ℝ^{+})}^{n})} & : = \underset{t \in [0, T]}{m a x} {‖ f_{1} (t) - f_{2} (t) ‖}_{1} \\ = \underset{t \in [0, T]}{m a x} \sum_{i = 1}^{n} | f_{1 i} (t) - f_{2 i} (t) |, \end{array}$

when the distance between the initial data is estimated, i.e. there exists δ > 0 such that

$‖ f_{1}^{0} - f_{2}^{0} ‖ : = \sum_{i = 1}^{n} | f_{1 i}^{0} - f_{2 i}^{0} | \leq δ .$

Theorem 3.1. Consider the Cauchy problems (3.1). Suppose that the assumptions H1-H6 hold true. If there exists δ > 0 such that:

${‖ f_{1}^{0} - f_{2}^{0} ‖}_{1} \leq δ,$ (3.2)

then for T > 0

${‖ f_{1} (t) - f_{2} (t) ‖}_{C ([0, T]; {(ℝ^{+})}^{n})} \leq δ e^{C T},$ (3.3)

where the constant C = C(η,F,I_u) depends on the parameters of the system.

Remark 3.2. As consequence of Theorem 3.1, the problem (2.3) is well-posed in the Hadamard sense.

4. Proof of main result

Proof of Theorem 3.1. The thermostatted equation (2.2), for i ∈ {1, 2, …, n}, writes:

$\begin{array}{l} \frac{d f_{i}}{d t} (t) & = \sum_{h = 1}^{n} \sum_{k = 1}^{n} η B_{h k}^{i} f_{h} (t) f_{k} (t) - f_{i} (t) \sum_{k = 1}^{n} η f_{k} (t) + F \\ - \sum_{j = 1}^{n} (u_{j}^{2} (J_{j} [f] + F_{j})) f_{i} (t) . \end{array}$ (4.1)

Integrating between 0 and t, the (4.1) reads:

$\begin{array}{l} \int_{0}^{t} \frac{d f_{i}}{d τ} (τ) d τ & = η \int_{0}^{t} \sum_{h = 1}^{n} \sum_{k = 1}^{n} B_{h k}^{i} f_{h} (τ) f_{k} (τ) d τ - η \int_{0}^{t} f_{i} (τ) d τ \\ + \int_{0}^{t} F d τ - \sum_{j = 1}^{n} (u_{j}^{2} (J_{j} [f] + F_{j})) \int_{0}^{t} f_{i} (τ) d τ . \end{array}$ (4.2)

By assumptions:

$\sum_{j = 1}^{n} (u_{j}^{2} (J_{j} [f] + F_{j})) = \sum_{j = 1}^{n} u_{j}^{2} F_{j},$

and then the (4.2) rewrites:

$\begin{array}{l} f_{i} (t) - f_{i} (0) & = η \int_{0}^{t} \sum_{h = 1}^{n} \sum_{k = 1}^{n} B_{h k}^{i} f_{h} (τ) f_{k} (τ) d τ - η \int_{0}^{t} f_{i} (τ) d τ \\ + F t - (\sum_{j = 1}^{n} u_{j}^{2} F_{j}) \int_{0}^{t} f_{i} (τ) d τ . \end{array}$ (4.3)

Finally, by (4.3), for i ∈ {1, 2, …, n}:

$\begin{array}{l} f_{i} (t) & = f_{i} (0) + η \int_{0}^{t} \sum_{h = 1}^{n} \sum_{k = 1}^{n} B_{h k}^{i} f_{h} (τ) f_{k} (τ) d τ - η \int_{0}^{t} f_{i} (τ) d τ \\ + F t - (\sum_{j = 1}^{n} u_{j}^{2} F_{j}) \int_{0}^{t} f_{i} (τ) d τ . \end{array}$ (4.4)

Using the (4.4) for $f_{1} (t), f_{2} (t) \in C ([0, + \infty [; {(ℝ^{+})}^{n}) \cap ℛ_{f}^{2}$ solutions of the Cauchy problems (3.1) with initial data $f_{1}^{0}$ and $f_{2}^{0}$ , respectively, one has, for i ∈ {1, 2, …, n}:

$\begin{array}{l} f_{1 i} (t) & = f_{1 i}^{0} + η \int_{0}^{t} \sum_{h = 1}^{n} \sum_{k = 1}^{n} B_{h k}^{i} f_{1 h} (τ) f_{1 k} (τ) d τ - η \int_{0}^{t} f_{1 i} (τ) d τ \\ + F t - (\sum_{j = 1}^{n} u_{j}^{2} F_{j}) \int_{0}^{t} f_{1 i} (τ) d τ \end{array}$ (4.5)

and

$\begin{array}{l} f_{2 i} (t) & = f_{2 i}^{0} + η \int_{0}^{t} \sum_{h = 1}^{n} \sum_{k = 1}^{n} B_{h k}^{i} f_{2 h} (τ) f_{2 k} (τ) d τ - η \int_{0}^{t} f_{2 i} (τ) d τ \\ + F t - (\sum_{j = 1}^{n} u_{j}^{2} F_{j}) \int_{0}^{t} f_{2 i} (τ) d τ . \end{array}$ (4.6)

Subtracting the (4.5) and (4.6), for i ∈ {1, 2, …, n}:

$\begin{array}{l} f_{1 i} (t) & - f_{2 i} (t) = (f_{1 i}^{0} - f_{2 i}^{0}) \\ + η \int_{0}^{t} \sum_{h = 1}^{n} \sum_{k = 1}^{n} B_{h k}^{i} (f_{1 h} (τ) f_{1 k} (τ) - f_{2 h} (τ) f_{2 k} (τ)) d τ \\ - η \int_{0}^{t} (f_{1 i} (τ) - f_{2 i} (τ)) d τ - (\sum_{j = 1}^{n} u_{j}^{2} F_{j}) \int_{0}^{t} (f_{1 i} (τ) - f_{2 i} (τ)) d τ . \end{array}$ (4.7)

By (4.7), for i ∈ {1, 2, …, n}:

$\begin{array}{l} | f_{1 i} (t) & - f_{2 i} (t) | \leq | f_{1 i}^{0} - f_{2 i}^{0} | \\ + η \int_{0}^{t} \sum_{h = 1}^{n} \sum_{k = 1}^{n} B_{h k}^{i} | f_{1 h} (τ) f_{1 k} (τ) - f_{2 h} (τ) f_{2 k} (τ) | d τ \\ + η \int_{0}^{t} | f_{1 i} (τ) - f_{2 i} (τ) | d τ + (\sum_{j = 1}^{n} u_{j}^{2} F_{j}) \int_{0}^{t} | f_{1 i} (τ) - f_{2 i} (τ) | d τ . \end{array}$ (4.8)

Moreover, for i ∈ {1, 2, …, n}:

$\begin{array}{l} | f_{1 i} (t) & - f_{2 i} (t) | \leq | f_{1 i}^{0} - f_{2 i}^{0} | \\ + η \int_{0}^{t} \sum_{h = 1}^{n} \sum_{k = 1}^{n} B_{h k}^{i} | f_{1 h} (τ) f_{1 k} (τ) - f_{2 h} (τ) f_{2 k} (τ) | d τ \\ + (η + \sum_{j = 1}^{n} u_{j}^{2} F_{j}) \int_{0}^{t} | f_{1 i} (τ) - f_{2 i} (τ) | d τ . \end{array}$ (4.9)

Summing the (4.9) on i:

$\begin{array}{l} \sum_{i = 1}^{n} | f_{1 i} (t) & - f_{2 i} (t) | \leq \sum_{i = 1}^{n} | f_{1 i}^{0} - f_{2 i}^{0} | \\ + η \int_{0}^{t} \sum_{i = 1}^{n} \sum_{h = 1}^{n} \sum_{k = 1}^{n} B_{h k}^{i} | f_{1 h} (τ) f_{1 k} (τ) - f_{2 h} (τ) f_{2 k} (τ) | d τ \\ + (η + \sum_{j = 1}^{n} u_{j}^{2} F_{j}) \int_{0}^{t} \sum_{i = 1}^{n} | f_{1 i} (τ) - f_{2 i} (τ) | d τ . \end{array}$ (4.10)

Then by (4.10):

$\begin{array}{l} ‖ f_{1} (t) & {- f_{2} (t) ‖}_{1} \leq {‖ f_{1}^{0} - f_{2}^{0} ‖}_{1} \\ + η \int_{0}^{t} \sum_{h = 1}^{n} \sum_{k = 1}^{n} | f_{1 h} (τ) f_{1 k} (τ) - f_{2 h} (τ) f_{2 k} (τ) | d τ \\ + (η + \sum_{j = 1}^{n} u_{j}^{2} F_{j}) \int_{0}^{t} {‖ f_{1} (τ) - f_{2} (τ) ‖}_{1} d τ . \end{array}$ (4.11)

Since, by straightforward calculations:

$\begin{array}{l} | f_{1 h} (τ) f_{1 k} (τ) - f_{2 h} (τ) f_{2 k} (τ) | \\ = | f_{1 h} (τ) f_{1 k} (τ) - f_{2 h} (τ) f_{1 k} (τ) + f_{2 h} (τ) f_{1 k} (τ) - f_{2 h} (τ) f_{2 k} (τ) | \\ \leq | f_{1 h} (τ) - f_{2 h} (τ) | | f_{1 k} (τ) | + | f_{1 k} (τ) - f_{2 k} (τ) | | f_{2 h} (τ) |, \end{array}$

then the second term of the right hand side of the (4.11) is estimated as follows:

$\begin{array}{l} \int_{0}^{t} \sum_{h = 1}^{n} \sum_{k = 1}^{n} | f_{1 h} (τ) f_{1 k} (τ) - f_{2 h} (τ) f_{2 k} (τ) | d τ \\ \leq \int_{0}^{t} \sum_{h = 1}^{n} \sum_{k = 1}^{n} (| f_{1 h} (τ) - f_{2 h} (τ) | | f_{1 k} (τ) | + | f_{1 k} (τ) - f_{2 k} (τ) | | f_{2 h} (τ) |) d τ \\ \leq \int_{0}^{t} \sum_{h = 1}^{n} | f_{1 h} (τ) - f_{2 h} (τ) | \sum_{k = 1}^{n} | f_{1 k} (τ) | d τ \\ + \int_{0}^{t} \sum_{k = 1}^{n} | f_{1 k} (τ) - f_{2 k} (τ) | \sum_{h = 1}^{n} | f_{2 h} (τ) | d τ \\ \leq 2 \int_{0}^{t} {‖ f_{1} (τ) - f_{2} (τ) ‖}_{1} d τ . \end{array}$ (4.12)

Using the (4.12) and (4.11) follows:

$\begin{array}{l} {‖ f_{1} (t) - f_{2} (t) ‖}_{1} & \leq {‖ f_{1}^{0} - f_{2}^{0} ‖}_{1} \\ + 2 η \int_{0}^{t} {‖ f_{1} (τ) - f_{2} (τ) ‖}_{1} d τ \\ + (η + \sum_{j = 1}^{n} u_{j}^{2} F_{j}) \int_{0}^{t} {‖ f_{1} (τ) - f_{2} (τ) ‖}_{1} d τ \\ \leq {‖ f_{1}^{0} - f_{2}^{0} ‖}_{1} + (3 η + \sum_{j = 1}^{n} u_{j}^{2} F_{j}) \int_{0}^{t} {‖ f_{1} (τ) - f_{2} (τ) ‖}_{1} d τ . \end{array}$ (4.13)

Let introduce the constant

$C (η, F, I_{u}) = C : = 3 η + \sum_{j = 1}^{n} u_{j}^{2} F_{j} = 3 η + F \sum_{j = 1}^{n} u_{j}^{2},$

that depends on the parameters of the system. Then, by the (3.2), the (4.13) rewrites:

${‖ f_{1} (t) - f_{2} (t) ‖}_{1} \leq δ + C \int_{0}^{t} {‖ f_{1} (τ) - f_{2} (τ) ‖}_{1} d τ .$ (4.14)

Applying the Gronwall Lemma [54] to the (4.14), one has:

${‖ f_{1} (t) - f_{2} (t) ‖}_{1} \leq δ e^{C t},$ (4.15)

for t ∈ (0, T).

Finally, by (4.15), the claim (3.3) is gained, since:

${‖ f_{1} (t) - f_{2} (t) ‖}_{C ([0, T]; {(ℝ^{+})}^{n})} = \underset{t \in [0, T]}{m a x} {‖ f_{1} (t) - f_{2} (t) ‖}_{1} \leq δ e^{C T} .$

5. Conclusion and research perspectives

The mathematical analysis performed in this paper has been addressed to the dependence on the initial data of the discrete thermostatted kinetic framework. Theorem 3.1 ensures the stability in the Hadarmd sense of the framework (2.2), for all T > 0. The constant C > 0, obtained after some technical computations, is explicit and is an important issue for future numerical simulations that may be performed for several applications (see among others [55], [56] and references therein).

Numerical simulations towards the framework (2.2) are a first future research prespective. Specifically, the parameters of the system, i.e. interaction rates η_hk and transition probability densities $B_{h k}^{i}$ , will have an explicit form and meaning related to the particular application taken into account. For instance, the modeling of the tumor-immune system competition may be considere. In this case the functional subsystems are: cells of immune system, normal cells and infected cells (see [57]–[59]). The initial distribution of cells, related to the three different functional subsystems, is gained by statistical analysis; then it may be affected by error. The solutions, related to the real and affected value of the initial data, differs of δ at time t = 0. By using the estimate (3.3), the maximal time time interval where the two solutions are as “close” as possible to each other is estimated.

For Theorem 3.1 the norm ${‖ f ‖}_{C ([0, T]; {(ℝ^{+})}^{n})}$ has been used in according to [18]. A research perspective is the estimate of the distance between the two solutions, during the time evolution, by using another suitable norm which may provide a sharper result. In this perspective, the estimate of distance between the time-derivatives of the two functions may be a next step.

Theorem 3.1 provides stability for the discrete framework when the 2nd-order moment is preserved. The general framework [45], when the generic pth-order moment is preserved, is not investigated in this paper. An interesting future research perspective is the analysis of the general case by using, in a first approach, the same norm of Theorem 3.1.

References

[1]	Echols H, Goodman MF (1991) Fidelity mechanisms in DNA replication. Annu Rev Biochem 60: 477–511. doi: 10.1146/annurev.bi.60.070191.002401
[2]	Johnson KA (1993) Conformational coupling in DNA polymerase fidelity. Annu Rev Biochem 62: 685–713. doi: 10.1146/annurev.bi.62.070193.003345
[3]	Joyce CM, Benkovic SJ (2004) DNA polymerase fidelity: kinetics, structure, and checkpoints. Biochemistry 43: 14317–14324. doi: 10.1021/bi048422z
[4]	Kunkel T, Bebenek K (1988) Recent studies of the fidelity of DNA synthesis. BBA-Gene Struct Expr 951: 1–15. doi: 10.1016/0167-4781(88)90020-6
[5]	Kunkel TA, Bebenek K (2000) DNA replication fidelity. Annu Rev Biochem 69: 497–529. doi: 10.1146/annurev.biochem.69.1.497
[6]	Drake JW (1969) Comparative rates of spontaneous mutation. Nature 221: 1132. doi: 10.1038/2211132a0
[7]	Goodman MF, Tippin B (2000) The expanding polymerase universe. Nat Rev Mol Cell Biol 1: 101–109.
[8]	Filee J, Forterre P, Sen-Lin T, et al. (2002) Evolution of DNA polymerase families: evidences for multiple gene exchange between cellular and viral proteins. J Mol Evol 54: 763–773. doi: 10.1007/s00239-001-0078-x
[9]	Vashishtha AK, Kuchta RD (2016) Effects of acyclovir, foscarnet, and ribonucleotides on Herpes Simplex Virus-1 DNA Polymerase: Mechanistic insights and a novel mechanism for preventing stable incorporation of ribonucleotides into DNA. Biochemistry 55: 1168–1177. doi: 10.1021/acs.biochem.6b00065
[10]	Vashishtha AK, Kuchta RD (2015) Polymerase and exonuclease activities in herpes simplex virus type 1 DNA polymerase are not highly coordinated. Biochemistry 54: 240–249. doi: 10.1021/bi500840v
[11]	Xia S, Wang M, Blaha G, et al. (2011) Structural insights into complete metal ion coordination from ternary complexes of B family RB69 DNA polymerase. Biochemistry 50: 9114–9124. doi: 10.1021/bi201260h
[12]	Snow ET, Xu LS, Kinney PL (1993) Effects of nickel ions on polymerase activity and fidelity during DNA replication in vitro. Chem Biol Interact 88: 155–173. doi: 10.1016/0009-2797(93)90089-H
[13]	Vaisman A, Ling H, Woodgate R, et al. (2005) Fidelity of Dpo4: effect of metal ions, nucleotide selection and pyrophosphorolysis. EMBO J 24: 2957–2967. doi: 10.1038/sj.emboj.7600786
[14]	Pelletier H, Sawaya MR, Wolfle W, et al. (1996) A structural basis for metal ion mutagenicity and nucleotide selectivity in human DNA polymerase beta. Biochemistry 35: 12762–12777. doi: 10.1021/bi9529566
[15]	Irimia A, Loukachevitch LV, Eoff RL, et al. (2010) Metal-ion dependence of the active-site conformation of the translesion DNA polymerase Dpo4 from Sulfolobus sulfataricus. Acta Crystallogr Sect F Struct Biol Commun 66: 1013–1018. doi: 10.1107/S1744309110029374
[16]	Sirover MA, Dube DK, Loeb LA (1979) On the fidelity of DNA replication. Metal activation of Escherichia coli DNA polymerase I. J Biol Chem 254: 107–111.
[17]	Sirover MA, Loeb LA (1977) On the fidelity of DNA replication. Effect of metal activators during synthesis with avian myeloblastosis virus DNA polymerase. J Biol Chem 252: 3605–3610.
[18]	Miyaki M, Murata I, Osabe M, et al. (1977) Effect of metal cations on misincorporation by E. coli DNA polymerases. Biochem Biophys Res Commun 77: 854–860. doi: 10.1016/S0006-291X(77)80056-9
[19]	Goodman MF, Keener S, Guidotti S, et al. (1983) On the enzymatic basis for mutagenesis by manganese. J Biol Chem 258: 3469–3475.
[20]	Sirover MA, Loeb LA (1976) Infidelity of DNA synthesis in vitro: screening for potential metal mutagens or carcinogens. Science 194: 1434–1436. doi: 10.1126/science.1006310
[21]	Lee HR, Wang M, Konigsberg W (2009) The reopening rate of the fingers domain is a determinant of base selectivity for RB69 DNA polymerase. Biochemistry 48: 2087–2098. doi: 10.1021/bi8016284
[22]	Villani G, Tanquy Le Gac N, Wasungu L, et al. (2002) Effect of manganese on in vitro replication of damaged DNA catalyzed by the herpes simplex virus type-1 DNA polymerase. Nucleic Acids Res 30: 3323–3332. doi: 10.1093/nar/gkf463
[23]	Xia S, Konigsberg WH (2014) RB69 DNA polymerase structure, kinetics, and fidelity. Biochemistry 53: 2752–2767. doi: 10.1021/bi4014215
[24]	Beard WA, Wilson SH (2014) Structure and mechanism of DNA polymerase β. Biochemistry 53: 2768–2780. doi: 10.1021/bi500139h
[25]	Sobol RW, Wilson SH (2001) Mammalian DNA beta-polymerase in base excision repair of alkylation damage. Prog Nucleic Acid Res Mol Biol 68: 57–74. doi: 10.1016/S0079-6603(01)68090-5
[26]	Beard WA, Wilson SH (2000) Structural design of a eukaryotic DNA repair polymerase: DNA polymerase beta. Mutat Res 460: 231–244. doi: 10.1016/S0921-8777(00)00029-X
[27]	Yang L, Arora K, Beard WA, et al. (2004) Critical role of magnesium ions in DNA polymerase beta's closing and active site assembly. J Am Chem Soc 126: 8441–8453. doi: 10.1021/ja049412o
[28]	Bakhtina M, Lee S, Wang Y, et al. (2005) Use of viscogens, dNTPalphaS, and rhodium(III) as probes in stopped-flow experiments to obtain new evidence for the mechanism of catalysis by DNA polymerase beta. Biochemistry 44: 5177–5187. doi: 10.1021/bi047664w
[29]	Nakamura T, Zhao Y, Yamagata Y, et al. (2012) Watching DNA polymerase eta make a phosphodiester bond. Nature 487: 196–201. doi: 10.1038/nature11181
[30]	Gao Y, Yang W (2016) Capture of a third Mg²⁺ is essential for catalyzing DNA synthesis. Science 352:1334–1337. doi: 10.1126/science.aad9633
[31]	Freudenthal BD, Beard WA, Shock DD, et al. (2013) Observing a DNA polymerase choose right from wrong. Cell 154: 157–168. doi: 10.1016/j.cell.2013.05.048
[32]	Xia S, Wang J, Konigsberg WH (2013) DNA mismatch synthesis complexes provide insights into base selectivity of a B family DNA polymerase. J Am Chem Soc 135: 193–202. doi: 10.1021/ja3079048
[33]	Doublie S, Tabor S, Long AM, et al. (1998) Crystal structure of a bacteriophage T7 DNA replication complex at 2.2 A resolution. Nature 391: 251–258.
[34]	Johnson SJ, Taylor JS, Beese LS (2003) Processive DNA synthesis observed in a polymerase crystal suggests a mechanism for the prevention of frameshift mutations. Proc Natl Acad Sci USA 100: 3895–3900. doi: 10.1073/pnas.0630532100
[35]	Johnson SJ, Beese LS (2004) Structures of mismatch replication errors observed in a DNA polymerase. Cell 116: 803–816. doi: 10.1016/S0092-8674(04)00252-1
[36]	Vashishtha AK, Konigsberg WH (2016) Effect of Different Divalent Cations on the Kinetics and Fidelity of RB69 DNA Polymerase. Biochemistry 55: 2661–2670. doi: 10.1021/acs.biochem.5b01350
[37]	Tabor S, Richardson CC (1989) Effect of manganese ions on the incorporation of dideoxynucleotides by bacteriophage T7 DNA polymerase and Escherichia coli DNA polymerase I. Proc Natl Acad Sci USA 86: 4076–4080. doi: 10.1073/pnas.86.11.4076
[38]	Hori K, Mark DF, Richardson CC (1979) Deoxyribonucleic acid polymerase of bacteriophage T7. Characterization of the exonuclease activities of the gene 5 protein and the reconstituted polymerase. J Biol Chem 254: 11598–11604.
[39]	Kuchta RD, Mizrahi V, Benkovic PA, et al. (1987) Kinetic mechanism of DNA polymerase I (Klenow). Biochemistry 26: 8410–8417. doi: 10.1021/bi00399a057
[40]	Venkitaraman AR (1989) Use of modified T7 DNA polymerase (sequenase version 2.0) for oligonucleotide site-directed mutagenesis. Nucleic Acids Res 17: 3314.
[41]	Joyce CM (1989) How DNA travels between the separate polymerase and 3'-5'-exonuclease sites of DNA polymerase I (Klenow fragment). J Biol Chem 264: 10858–10866.
[42]	Irimia A, Zang H, Loukachevitch LV, et al. (2006) Calcium is a cofactor of polymerization but inhibits pyrophosphorolysis by the Sulfolobus solfataricus DNA polymerase Dpo4. Biochemistry 45: 5949–5956. doi: 10.1021/bi052511+
[43]	Sirover MA, Loeb LA (1976) Metal-induced infidelity during DNA synthesis. Proc Natl Acad Sci USA 73: 2331–2335. doi: 10.1073/pnas.73.7.2331
[44]	Sirover MA, Loeb LA (1976) Metal activation of DNA synthesis. Biochem Biophys Res Commun 70: 812–817. doi: 10.1016/0006-291X(76)90664-1
[45]	Seal G, Shearman CW, Loeb LA (1979) On the fidelity of DNA replication. Studies with human placenta DNA polymerases. J Biol Chem 254: 5229–5237.
[46]	Vashishtha AK, Konigsberg WH (2018) The effect of different divalent cations on the kinetics and fidelity of Bacillus stearothermophilus DNA polymerase. AIMS Biophys 5: 125–143. doi: 10.3934/biophy.2018.2.125
[47]	Zhang H, Cao W, Zakharova E, et al. (2007) Fluorescence of 2-aminopurine reveals rapid conformational changes in the RB69 DNA polymerase-primer/template complexes upon binding incorporati of matched deoxynucleoside triphosphates. Nucleic Acids Res 35: 6052–6062. doi: 10.1093/nar/gkm587
[48]	Hariharan C, Bloom LB, Helquist SA, et al. (2006) Dynamics of nucleotide incorporation: snapshots revealed by 2-aminopurine fluorescence studies. Biochemistry 45: 2836–2844. doi: 10.1021/bi051644s
[49]	Frey MW, Sowers LC, Millar DP, et al. (1995) The nucleotide analog 2-aminopurine as a spectroscopic probe of nucleotide incorporation by the Klenow fragment of Escherichia coli polymerase I and bacteriophage T4 DNA polymerase. Biochemistry 34: 9185–9192. doi: 10.1021/bi00028a031
[50]	Chin YE, Snow ET, Cohen MD, et al. (1994) The effect of divalent nickel (Ni²⁺) on in vitro DNA replication by DNA polymerase alpha. Cancer Res 54: 2337–2341.
[51]	Bock CW, Katz AK, Markham GD, et al. (1999) Manganese as a replacement for magnesium and zinc: Functional comparison of the divalent ions. J Am Chem Soc 121: 7360–7372. doi: 10.1021/ja9906960
[52]	Dube DK, Loeb LA (1975) Manganese as a mutagenic agent during in vitro DNA synthesis. Biochem Biophys Res Commun 67: 1041–1046. doi: 10.1016/0006-291X(75)90779-2
[53]	Jin YH, Clark AB, Slebos RJC, et al. (2003) Cadmium is a mutagen that acts by inhibiting mismatch repair. Nat Genet 34: 326–329. doi: 10.1038/ng1172
[54]	Hays H, Berdis AJ (2002) Manganese substantially alters the dynamics of translesion DNA synthesis. Biochemistry 41: 4771–4778. doi: 10.1021/bi0120648
[55]	Beckman RA, Mildvan AS, Loeb LA (1985) On the fidelity of DNA replication: manganese mutagenesis in vitro. Biochemistry 24: 5810–5817. doi: 10.1021/bi00342a019
[56]	Doetsch PW, Chan GL, Haseltine WA (1985) T4 DNA polymerase (3'–5') exonuclease, an enzyme for the detection and quantitation of stable DNA lesions: the ultraviolet light example. Nucleic Acids Res 13: 3285–3304. doi: 10.1093/nar/13.9.3285
[57]	Kunkel TA, Soni A (1988) Exonucleolytic proofreading enhances the fidelity of DNA synthesis by chick embryo DNA polymerase-gamma. J Biol Chem 263: 4450–4459.
[58]	Wang W, Hellinga HW, Beese LS (2011) Structural evidence for the rare tautomer hypothesis of spontaneous mutagenesis. Proc Natl Acad Sci USA 108: 17644–17648. doi: 10.1073/pnas.1114496108
[59]	Bebenek K, Pedersen LC, Kunkel TA (2011) Replication infidelity via a mismatch with Watson-Crick geometry. Proc Natl Acad Sci USA 108: 1862–1867. doi: 10.1073/pnas.1012825108
[60]	Harris VH, Smith CL, Cummins WJ, et al. (2003) The effect of tautomeric constant on the specificity of nucleotide incorporation during DNA replication: support for the rare tautomer hypothesis of substitution mutagenesis. J Mol Biol 326: 1389–1401. doi: 10.1016/S0022-2836(03)00051-2
[61]	Topal MD, Fresco JR (1976) Complementary base pairing and the origin of substitution mutations. Nature 263: 285–289. doi: 10.1038/263285a0
[62]	Vashishtha AK, Wang J, Konigsberg WH (2016) Different Divalent Cations Alter the Kinetics and Fidelity of DNA Polymerases. J Biol Chem 291: 20869–20875. doi: 10.1074/jbc.R116.742494
[63]	Xia S, Vashishtha A, Bulkley D, et al. (2012) Contribution of partial charge interactions and base stacking to the efficiency of primer extension at and beyond abasic sites in DNA. Biochemistry 51: 4922–4931. doi: 10.1021/bi300296q
[64]	Zhang Y, Baranovskiy AG, Tahirov ET (2016) Divalent ions attenuate DNA synthesis by human DNA polymerase alpha by changing the structure of the template/primer or by perturbing the polymerase reaction. DNA Repair 43:24–33. doi: 10.1016/j.dnarep.2016.05.017
[65]	Schmitt MW, Kennedy SR, Salk JJ, et al. (2012) Detection of ultra-rare mutations by next-generation sequencing. Proc Natl Acad Sci USA 109: 14508–14513. doi: 10.1073/pnas.1208715109
[66]	Frederico LA, Kunkel TA, Shaw BR (1990) A sensitive genetic assay for the detection of cytosine deamination: determination of rate constants and the activation energy. Biochemistry 29: 2532–2537.
[67]	Bebenek K, Kunkel TA (1995) Analyzing fidelity of DNA polymerase. Methods Enz 262 217–232.
[68]	Yasukawa K, Iida K, Okano H, et al. (2017) Next-generation sequencing-based analysis of reverse transcriptase fidelity. Biochem Biophys Res Commun 492: 147–153. doi: 10.1016/j.bbrc.2017.07.169
[69]	Ellefson JW, Gollihar J, Shroff R, et al. (2016) Synthetic evolutionary origin of a proofreading reverse transcriptase. Science 352: 1590–1593. doi: 10.1126/science.aaf5409

This article has been cited by:

1.	Sana Abdulkream Alharbi, Azmin Sham Rambely, A New ODE-Based Model for Tumor Cells and Immune System Competition, 2020, 8, 2227-7390, 1285, 10.3390/math8081285
2.	Bruno Carbonaro, Marco Menale, Towards the Dependence on Parameters for the Solution of the Thermostatted Kinetic Framework, 2021, 10, 2075-1680, 59, 10.3390/axioms10020059
3.	Carlo Bianca, On the Modeling of Energy-Multisource Networks by the Thermostatted Kinetic Theory Approach: A Review with Research Perspectives, 2022, 15, 1996-1073, 7825, 10.3390/en15217825
4.	Carlo Bianca, Thermostatted Kinetic Theory Structures in Biophysics: Generalizations and Perspectives, 2024, 4, 2673-9909, 1278, 10.3390/appliedmath4040069
5.	Carlo Bianca, A decade of thermostatted kinetic theory models for complex active matter living systems, 2024, 50, 15710645, 72, 10.1016/j.plrev.2024.06.015
6.	Carlo Bianca, The Role of the Table of Games in the Discrete Thermostatted Kinetic Theory, 2024, 12, 2227-7390, 2356, 10.3390/math12152356
7.	Bruno Carbonaro, Marco Menale, A nonconservative kinetic framework under the action of an external force field: Theoretical results with application inspired to ecology, 2023, 34, 0956-7925, 1170, 10.1017/S0956792523000232
8.	Fengling Jia, Peiyan He, Lixin Yang, A Novel Coupled Memristive Izhikevich Neuron Model and Its Complex Dynamics, 2024, 12, 2227-7390, 2244, 10.3390/math12142244
9.	Bruno Carbonaro, Marco Menale, Markov Chains and Kinetic Theory: A Possible Application to Socio-Economic Problems, 2024, 12, 2227-7390, 1571, 10.3390/math12101571
10.	Marco Menale, Ezio Venturino, A kinetic theory approach to modeling prey–predator ecosystems with expertise levels: analysis, simulations and stability considerations, 2024, 43, 2238-3603, 10.1007/s40314-024-02726-2
11.	Muhammad Naeem Aslam, Nadeem Shaukat, Muhammad Sarmad Arshad, Muhammad Waheed Aslam, Javed Hussain, An intelligent approach for analyzing the effects of normal tumor immune unhealthy diet model through unsupervised physics informed neural-networks integrated with meta-heuristic algorithms, 2025, 18, 1864-5909, 10.1007/s12065-024-01007-5

Reader Comments

Your name:*

Email:*
© 2018 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Biophysics

1.1 2.4

Metrics

Article views(4877) PDF downloads(1733) Cited by(3)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

Figures and Tables

Figures(3) / Tables(2)

AIMS Biophysics

Effect of different divalent cations on the kinetics and fidelity of DNA polymerases

Related Papers:

Abstract

1. Introduction

2. The discrete thermostatted framework

3. Dependence on the initial data

4. Proof of main result

5. Conclusion and research perspectives

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Catalog

AIMS Biophysics

Effect of different divalent cations on the kinetics and fidelity of DNA polymerases

Related Papers:

Abstract

1. Introduction

2. The discrete thermostatted framework

3. Dependence on the initial data

4. Proof of main result

5. Conclusion and research perspectives

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog