1. Introduction
An adequate intrauterine growth process is not only of importance during prenatal phase but also reduces birth complications and postnatal morbidity and mortality [1,2]. Small for gestational age (SGA) newborns but also macrosomic or large for gestational age (LGA) newborns show an increased risk of birth complications, neonatal and childhood morbidity and mortality but also an increased risk of cardiovascular, type 2 diabetes and obesity in later life [1,2,3,4,5,6]. Especially macrosomia (> 4000 g) is associated with increased risk of intrauterine death, artificial induction of labor, prolonged birth, birth asphyxia, increased rates of caesarian section, postpartum hemorrhages and neonatal hypoglycemia and hyperbilirubinemia [7,8,9]. Consequently the analysis of potential risk factors of restricted or accelerated fetal growth is of special interest to gynaecologists, perinatologists, public health researchers [10] but also evolutionary anthropologists [11]. Fetal growth and consequently newborn size are the results of the complex interaction between genetic factors and the fetal environment [12]. An important factor is maternal weight status before and during pregnancy. Currently underweight but also obesity is widely found among women of reproductive age. This coexistence of underweight and obesity is commonly called the double burden of malnutrition, a phenomenon which can be found in most countries worldwide, although the prevalence of underweight is quite low in high income countries. Concerning reproductive success and pregnancy outcome maternal underweight as well as obesity are important risk factors. It is well known that all deviations from adequate and optimal nutritional status, including undernourishment und underweight but also over-nutrition and therefore obesity influence fetal growth, pregnancy outcome, childhood development but also adult health in an adverse manner [1,2,12,13,14]. While inadequate food supply or starvation before and during pregnancy may have fatal consequences such as fetal growth restriction, stunting, poor newborn health, maternal overnutrition and obesity represents a risk factor of perinatal complications but also macrosomia i.e. large for gestational age newborns. Macrosomic newborns show an increased risk of neonatal and childhood morbidity and mortality but also an increased risk of cardiovascular, type 2 diabetes and obesity in later life [1,2,3,4,5,6]. Additionally macrosomia represents a special risk factor of intrauterine death, artificial induction of labor, prolonged birth, birth asphyxia, increased rates of caesarian section, postpartum hemorrhages and neonatal hypoglycemia and hyperbilirubinemia [7,8,9,15,16]. These adverse effects of maternal obesity on pregnancy outcome is mainly due to the fact that maternal obesity is associated with adverse fetal growth patterns such as intrauterine growth restriction, but also fetal overgrowth resulting in large for gestational age (LGA) newborn [15,17,18]. Consequently maternal underweight as well as maternal obesity increase the risk of adverse pregnancy outcome. In the present study the impact of maternal pre-pregnancy weight status and gestational weight gain on newborn size and mode of delivery in a large sample of term births was analyzed.
2. Material and methods
2.1. Data set
This retrospective study is based on a data set of 9214 singleton births which took place at the University Clinic of Gynecology and Obstetrics in Vienna, Austria between 1995 and 2000. Although a total of 18425 births were collected, only 9214 met the strict inclusion and exclusion criteria. Following inclusion criterions have been defined:
term births which took place between the 38th and 41rst gestational week
primiparae women ageing between 19 and 40 years
all prenatal check-ups of the mother-child passport are completed
delivery of a single infant without congenital malformations
no registered maternal diseases before and during pregnancy
no hypertension (BP < 150/90 mmHg)
no proteinuria or glucosuria
no diabetes mellitus before pregnancy
no gestational diabetes
no preclampsia
no drug or alcohol abuse before and during pregnancy
no IVF
Gestational age was calculated in terms of the number of weeks from the beginning of the last menstrual bleeding to the date of delivery ( = duration of amenorrhoea). All subjects originated from Austrian or central Europe.
2.2. Maternal parameters
All women enrolled in the present study aged between 19 and 40 years (mean = 25.9 yrs SD = 5.1). The following maternal somatometric parameters were determined at the first prenatal visit: Stature height was measured to the nearest 0.5 cm. Body weight was measured to the nearest 0.1 kg on a balance beam scale [19]. Additionally maternal weight at the end of pregnancy (EPW) was measured before birth. The weight gain during pregnancy (PWG) was calculated by subtraction of pre-pregnancy weight from body weight at the end of pregnancy. A gestational weight gain below 7 kg was classified as low, while a gestational weight gain above 15 kg was defined as high gestational weight gain.
Maternal pre-pregnancy weight status was determined by the body mass index (BMI) kg/m2 using stature height and pre-pregnancy weight. To classify maternal weight status the cut-offs published by the WHO [20] were used.
Severe underweight = BMI < 16.00 kg/m2
Moderate underweight = BMI 16.00 kg/m2 to 16.99 kg/m2
Underweight = BMI 17.00 kg/m2 to 18.49 kg/m2
Normal weight = BMI 18.50 kg/m2 to 24.99 kg/m2
Overweight = BMI 25.00 kg/m2 to 29.99 kg/m2
Obesity = BMI 30.00 kg/m2 to 39.99 kg/m2
Morbid Obesity = BMI > 40.00 kg/m2
2.3. Newborn parameters
Birth weight, birth length, head circumference, diameter fronto-occipitalis and acromial circumference were taken directly from newborn immediately after birth. Newborn weight status was defined as follows: very low < 1500g, low 1500–2500g, normal 2500–4000g and high (macrosomia) > 4000g. Furthermore the one and the five minute APGAR scores [21] for the evaluation of the newborn were determined.
2.4. Mode of delivery
Spontaneous vaginal birth and caesarean section were recorded. Caesarean sections requested by the mother without any medical indication were not carried out at this hospital.
3. Statistical analyses
Statistical analyses were performed by means of SPSS for Windows program Version 22.0. After calculating descriptive statistics (means, SDs), group differences were tested regarding their statistical significance using Duncan analyses. Furthermore χ2 analyses and odds ratios were computed. Multiple regression analyses were performed to test the impact of maternal prepregnancy BMI, stature height, gestational weight gain on newborn size. Additionally binary logistic regressions were computed in order to test the association of maternal stature, prepregnancy body mass as well as newborn anthropometrics and caesarean section. Vaginal delivery was coded as 1, a caesarean section was coded as 2.
4. Results
4.1. Sample characteristics
Sample characteristics are presented in table 1. The incidence of underweight and obesity was quite low in the present sample. As demonstrated in figure 1 less than 10% of the mothers were classified as underweight, i.e. a BMI below 18.50 kg/m2 before pregnancy. Only 0.5% corresponded to the definition of severe underweight (BMI < 16.00 kg/m2) and only 1.1% exhibited a moderate underweight (BMI 16.00–17.00 kg/m2). 3.5% were classified as obese (BMI 30.00–39.99 kg/m2)and only 0.3% of the mothers were morbidly obese i.e. a BMI above 40.00 kg/m2, before pregnancy.
Table 1. Sample description Maternal and newborn characteristics (descriptive statistics mean, Sd, range, absolute and relative frequencies).
| Maternal parameters |
mean (SD) |
range |
n (%) |
| Maternal age (yrs) |
25.9 (5.1) |
19–40 |
|
| Menarcheal age (yrs) |
13.3 (1.5) |
8–18 |
|
| Gynecological age (yrs) |
12.6 (5.2) |
1–30 |
|
| Stature height (cm) |
163.3 (6.5) |
147–189 |
|
| Distanita spinarum (cm) |
24.9 (2.0) |
16–39 |
|
| Distantia cristarum (cm) |
28.1 (2.0) |
|
|
| Prepregnancy weight (kg) |
59.7 (10.3) |
41–130 |
|
| End of pregnancy weight (kg) |
73.3 (12.3) |
43–145 |
|
| Gestational weight gain (kg) |
12.9 (5) |
-2–38 |
|
| Gestational weight gain < 10kg |
|
|
2304 (25.0%) |
| Gestational weight gain 10–15kg |
|
|
4192 (45.5%) |
| Gestational weight gain > 15kg |
|
|
2718 (29.5%) |
| Prepregnancy body mass index (kg/m2) |
22.34 (3.59) |
14.94–52.78 |
|
| Prepregnancy weight status |
|
|
|
| Severe underweight BMI < 16.00 |
|
|
46 (0.5%) |
| Moderate underweight BMI 16.00–16.99 |
|
101 (1.1%) |
| Slight underweight BMI 17.00–18.49 |
|
|
653 (7.1%) |
| Normal weight BMI 18.50–24.99 |
|
|
6745 (73.3%) |
| Overweight BMI 25.00–29.99 |
|
|
1318 (14.3%) |
| Obese BMI 30.00–39.99 |
|
|
323 (3.5%) |
| Morbid obese > 40.00 |
|
|
28 (0.3%) |
| Newborn parameters |
|
|
|
| Sex |
|
|
|
| female |
|
|
4493 (48.8%) |
| male |
|
|
4721 (51.2%) |
| Birth weight (g) |
3371.1 (434.1) |
1550–5310 |
|
| Birth length (cm) |
49.9 (1.9) |
32–59 |
|
| Head circumference (cm) |
34.4 (1.4) |
30–40 |
|
| Diameter fronto-occipitalis (cm) |
11.3 (0.8) |
9–15 |
|
| Acromial circumference (cm) |
36.9 (2.3) |
24–48 |
|
| Newborn weight status |
|
|
|
| Very low birth weight < 1500g |
|
|
0 (0.0%) |
| Low birth weight 1500–2499g |
|
|
166 (1.8%) |
| Normal birth weight 2500–4000g |
|
|
8293 (90.0%) |
| Macrosome > 4000g |
|
|
755 (8.2%) |
| Apgar 1 |
8.6 (1.2) |
1–10 |
|
| Apgar 5 |
9.8 (0.8) |
1–10 |
|
4.2. Maternal characteristics and prepregnancy weight status
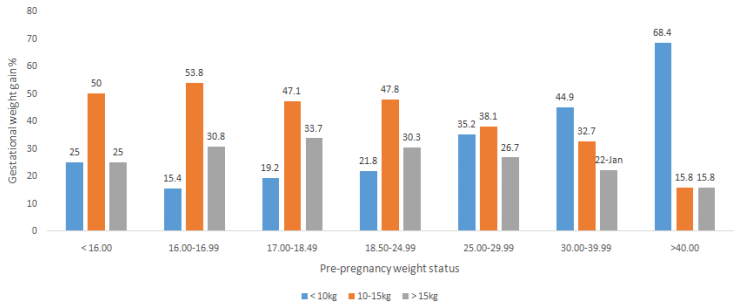
A profound impact of age on prepregnancy weight status was observed. Severe, moderate and slightly underweight women were significantly younger than normalweight, overweight and obese ones (see table 2). Gynecological age in contrast increased significantly with increasing prepregnancy weight status. Furthermore pelvic dimensions distantia cristarum and distantia spinosum increased significantly with increasing prepregnancy body mass index (see table 2). In contrast, overweight and obese mothers were significantly shorter than underweight and normal weight ones. The gestational weight gain decreased significantly with increasing prepregnancy weight status (see table 2). As demonstrated in figure 2, a high gestational weight gain, i.e. more than 15 kg was predominantly found among underweight, normal weight and overweight women, while among obese women a low gestational weight gain of less than 10 kg prevail. With increasing weight status the frequency of low weight gain (< 10 kg) increased significantly, while the frequency of high gestational weight gain (> 15 kg) decreased significantly.
Table 2. Maternal characteristics according to maternal pre-pregnancy weight status (Kruskall Wallis tests).
| Maternal characteristics |
Prepregnancy weight status BMI |
|
|
< 16.00 |
16.00–16.99 |
17.00–18.49 |
18.50–24.99 |
25.00–29.99 |
30.00–39.99 |
> 40.00 |
p |
|
mean (SD) |
mean (SD) |
mean (SD) |
mean (SD) |
mean (SD) |
mean (SD) |
mean (SD) |
|
| Age (years) |
23.7 (4.4) |
23.9 (4.2) |
24.4 (4.4) |
25.6 (4.9) |
26.8 (5.4) |
27.9 (5.4) |
28.9 (5.7) |
< 0.001 |
| Menarcheal age (years) |
13.5 (1.8) |
13.2 (1.5) |
13.4 (1.5) |
13.4 (1.4) |
13.1 (1.5) |
13.0 (1.5) |
12.6 (1.3) |
< 0.001 |
| Gynecological age (years) |
10.2 (4.5) |
10.6 (4.3) |
11.0 (4.6) |
12.3 (5.0) |
13.7 (5.4) |
14.8 (5.5) |
16.2 (5.5) |
< 0.001 |
| Distantia spinarum (cm) |
23.4 (1.8) |
23.8 (1.9) |
24.2 (1.9) |
24.8 (1.9) |
25.6 (2.1) |
26.2 (2.0) |
27.7 (3.2) |
< 0.001 |
| Distantia cristarum (cm) |
26.2 (1.4) |
26.4 (1.9) |
27.2 (1.9) |
27.9 (1.9) |
29.1 (1.9) |
30.0 (2.2) |
32.5 (1.9) |
< 0.001 |
| Body height (cm) |
165.7 (7.8) |
163.7 (6.5) |
164.5 (6.4) |
163.6 (6.4) |
162.8 (6.7) |
161.9 (6.9) |
160.1 (14.2) |
< 0.001 |
| Prepregnancy body weight (kg) |
42.0 (3.8) |
44.7 (3.7) |
48.5 (3.9) |
57.5 (6.2) |
71.2 (6.9) |
85.5 (9.2) |
109.5 (14.9) |
< 0.001 |
| End of pregnancy body weight (kg) |
53.3 (6.2) |
57.9 (6.7) |
61.9 (6.3) |
70.7 (8.4) |
83.9 (10.4) |
98.4 (12.0) |
119.4 (12.0) |
< 0.001 |
| Pregnancy weight gain (kg) |
11.9 (5.3) |
13.8 (5.0) |
13.6 (4.8) |
13.2 (5.2) |
12.2 (6.2) |
10.5 (6.4) |
7.5 (6.9) |
< 0.001 |
4.3. Maternal prepregnancy weight status and newborn size
As presented in table 3 birth weight, birth length, head circumference, diameter fronto occipitalis, and acromial circumference of the newborn increased significantly with increasing prepregnancy weight status. Concerning APGAR one minute and five s after birth the lowest values were observed among morbidly obese mothers. According to the results of the multiple regression analyses maternal age, body height, prepregnancy body mass index and gestational weight influenced independently significantly positively all newborn somatic parameters (see table 4). With increasing maternal age, maternal height, prepregnancy weight status and increasing weight gain birth weight, birth length, head circumference, fronto-occiptal diameter and acromial circumference
Table 3. Newborn size according to maternal pre-pregnancy weight status (Kruskall Wallis tests).
|
Prepregnancy bodx mass index |
|
| Newborn size |
< 16.00 |
16.00–16.99 |
17.00–18.49 |
18.50–24.99 |
25.00–29.99 |
35.00–39.99 |
> 40.00 |
Sig. |
|
mean (SD) |
mean (SD) |
mean (SD) |
mean (SD) |
mean (SD) |
Mean (SD) |
mean (SD) |
p-value |
| BW (g) |
3086.8 (494.6) |
3133.3 (424.8) |
3244.8 (406.3) |
3367.5 (422.3) |
3475.3 (455.0) |
3540.7 (445.1) |
3579.5 (540.1) |
< 0.001 |
| BL (cm) |
48.5 (2.2) |
49.1 (2.2) |
49.4 (1.9) |
49.9 (1.9) |
50.2 (1.9) |
50.4 (1.8) |
50.3 (1.3) |
< 0.001 |
| HC (cm) |
33.7 (1.6) |
33.7 (1.2) |
34.1 (1.3) |
34.4 (1.4) |
34.6 (1.4) |
34.7 (1.4) |
35.2 (1.6) |
< 0.001 |
| FOD (cm) |
11.0 (0.7) |
11.1 (0.8) |
11.2 (0.7) |
11.3 (0.8) |
11.4 (0.7) |
11.4 (0.8) |
11.5 (0.9) |
< 0.001 |
| AC (cm) |
35.7 (2.7) |
35.9 (2.1) |
36.4 (2.2) |
36.9 (2.3) |
37.4 (2.5) |
37.5 (2.4) |
38.1 (2.6) |
< 0.001 |
| APGAR 1 |
8.8 (1.0) |
8.5 (1.4) |
8.7 (1.1) |
8.7 (1.2) |
8.6 (1.4) |
8.4 (1.6) |
8.3 (1.3) |
0.060 |
| PGAR 5 |
9.7 (0.8) |
9.7 (0.7) |
9.8 (0.6) |
9.8 (0.7) |
9.7 (0.9) |
9.7 (1.1) |
9.6 (0.5) |
0.041 |
| Legend: BW = Birth weight; BL = Birth length; HC = Head circumference; FOD = Diameter Fronto-occipitalis; AC = Acromial circumference. |
Table 4. The impact of maternal age, body height pre-pregnancy weight status and gestational weight gain on newborn size (Multiple regression analyses).
| Dependent variables |
Multiple R |
Regression coefficient B |
Sig |
95% confidence interval |
| Birth weight |
|
| Maternal age |
0.36 |
5.88 |
< 0.001 |
3.24–8.49 |
| Body height |
|
12.41 |
< 0.001 |
10.39–14.42 |
| Prepregnancy weight status |
|
25.20 |
< 0.001 |
21.78–28.62 |
| Gestational weight gain |
|
16.39 |
< 0.001 |
13.95–18.83 |
| Birth length |
|
| Maternal age |
0.30 |
0.03 |
< 0.001 |
0.01–0.04 |
| Body height |
|
0.06 |
< 0.001 |
0.05–0.07 |
| Prepregnancy weight status |
|
0.08 |
< 0.001 |
0.06–0.09 |
| Gestational weight gain |
|
0.05 |
< 0.001 |
0.04–0.06 |
| Head circumference |
|
| Maternal age |
0.25 |
0.02 |
< 0.001 |
0.01–0.03 |
| Body height |
|
0.05 |
< 0.001 |
0.03–0.04 |
| Prepregnancy weight status |
|
0.06 |
< 0.001 |
0.05–0.07 |
| Gestational weight gain |
|
0.03 |
< 0.001 |
0.02–0.03 |
| Fronto-occiptial diameter |
|
| Maternal age |
0.12 |
0.01 |
0.042 |
0.00–0.01 |
| Body height |
|
0.01 |
< 0.001 |
0.00–0.01 |
| Prepregnancy weight status |
|
0.02 |
< 0.001 |
0.01–0.02 |
| Gestational weight gain |
|
0.01 |
0.006 |
0.00–0.01 |
| Acromial circumference |
|
| Maternal age |
0.28 |
0.04 |
< 0.001 |
0.03–0.06 |
| Body height |
|
0.04 |
< 0.001 |
0.03–0.06 |
| Prepregnancy weight status |
|
0.10 |
< 0.001 |
0.08–0.12 |
| Gestational weight gain |
|
0.08 |
< 0.001 |
0.06–0.09 |
4.4. Maternal prepregnancy weight status and mode of delivery
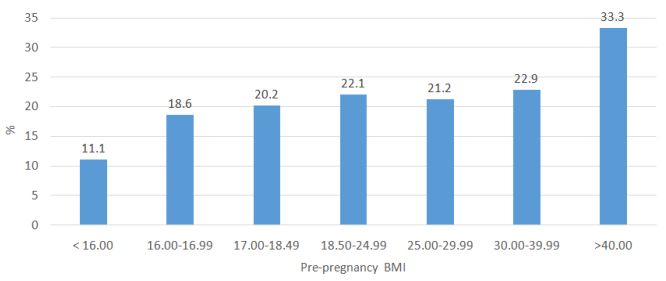
Caesarean section rate increased significantly with maternal prepregnancy weight status. The lowest rate of caesarean section occurred among severely underweight mothers (11.1%). Among morbidly obese women in contrast the caesarean section rate reached 33.3%, although the caesarean section rate of the whole sample was 21.8% (see figure 4).
According to the binary logistic regression analysis the mode of delivery was significantly influenced by maternal age, maternal body height, birth weight, newborn head circumference and newborn diameter fronto-occipitalis. Maternal prepregnancy BMI, however had no significant impact on the mode of delivery according to this analysis (see table 5).
Table 5. The impact of maternal and newborn somatometry on mode of delivery. Binary logistic regression analyses (spontaneous = 1, section = 2).
| Variable |
Coefficient B |
SE |
Sig |
95% confidence interval |
| Maternal age |
0.04 |
0.01 |
< 0.001 |
1.03–1.06 |
| Maternal height |
9.92 |
0.01 |
< 0.001 |
0.97–0.99 |
| Pre-pregnancy BMI |
–0.01 |
0.01 |
0.321 |
0.97–1.01 |
| Birth weight |
–0.01 |
0.00 |
< 0.001 |
0.99–0.99 |
| Birth length |
0.04 |
0.03 |
0.196 |
0.98–1.10 |
| Head circumferences |
0.21 |
0.04 |
< 0.001 |
1.15–1.33 |
| Diameter fronto-occipitalis |
0.16 |
0.06 |
0.003 |
1.06–1.31 |
| Acromial circumference |
0.05 |
0.03 |
0.076 |
0.99–1.10 |
5. Discussion
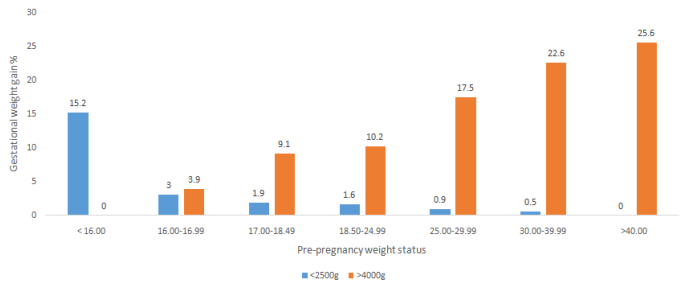
The present paper deals with the impact of maternal prepregnancy weight status on reproductive outcome based on a large data set containing data of 9214 singleton pregnancies. In particular the effects of maternal prepregnancy underweight (BMI < 18.50 kg/m2) but also obesity (BMI > 30.00 kg/m2) on fetal growth patterns estimated by newborn size were analyzed. In the present sample less than 4% of the participating mother corresponded to the definition of obesity i.e. a BMI above 30.00 kg/m2 before pregnancy and less the 2% corresponded to the definition of moderate or severe underweight i.e. BMI below 17.00 kg/m2. About 7% of the mothers were classified as slightly underweight (BMI between 17.00 to 18.50 kg/m2). About 14% of the mothers corresponded to the definition of overweight (BMI between 25.00 to 29.99 kg/m2). The low prevalence of moderate and severe underweight is typical of contemporary high income countries. Although during evolution and history of Homo sapiens undernourishment and underweight caused by starvation were frequent phenomenon [22,23], the prevalence of undernourishment and underweight was reduced markedly worldwide during the last decades [24]. Especially in high income countries undernourishment and underweight are rare conditions, as found in the present study. Nevertheless underweight during reproductive age is still a concern because it is well established that among females underweight and undernutrition have a clearly negative impact on reproductive outcome. In detail underweight and undernutrition increase the age at menarche, reduces the frequency of ovulatory cycles and increases the risk of giving birth to small for gestational age newborn [25]. In the present study severely underweight mothers showed the significantly highest frequency of giving birth to a small for gestational age newborn (SGA) (15.2%). Increased rates of SGA newborns are also found among moderate underweight mothers (3%) and even slightly underweight mothers (1.9%). With increasing maternal weight status the frequency of SGA newborns decreased markedly. In general newborn dimensions increased with increasing maternal prepregnancy weight status. The smaller size of newborn of underweight mothers may be on the one hand due to intrauterine conflicts over resources but may be also interpreted as an adaptation to the smaller pelvic dimensions of underweight mothers. In the present study the severely and moderate underweight women exhibited the significantly lowest dimensions of distantia spinarum and distantia cristarum.
But not only underweight has a negative impact on female reproduction and pregnancy outcome, on the other hand maternal obesity influences reproduction and pregnancy outcome in an adverse manner. The effects of maternal obesity on pregnancy are of special importance, because in contrast to prepregnancy underweight, maternal overweight as well as obesity are increasing dramatically among women of reproductive age [26,27]. In 2008 for the first time in the long history of Homo sapiens, the number of obese people on earth exceeded the number of people suffering from starvation and undernourishment [28]. Consequently overweight (BMI ≥ 25 kg/m2) and obesity (BMI ≥ 30 kg/m2) are frequently found conditions among women of reproductive age in recent times. Currently more than 1.9 billion adults, 18 years and older, are overweight. Of these over 600 million correspond to the definition of obesity [29]. 40% of women aged 18 years and older are overweight and 15% of women are obese. Consequently obesity and overnutrition represent important factors for reproductive health [30,31,32]. As pointed out above in the present sample the prevalence of obesity was quite low (less than 4%). Nevertheless maternal prepregnancy obesity is of major concern. It is well documented that maternal obesity increases the risk of miscarriage and stillbirth, gestational diabetes, gestational hypertension, pre-clampsia and delivery complication [33], but also the risk of congenital malformations, preterm birth and neonatal mortality [15,17,34]. An obesogenic fetal environment affects intrauterine growth patterns [35] and increases the risk of giving birth to small as well as large for gestational age newborns [18,32,36]. In the present study the prevalence of macrosome or large for gestational age (LGA) newborn was exceptionally high. More than 17% of the newborn of overweight mothers were classified as large for gestational age. Among obese mothers more than 20% of the newborn corresponded to the classification of LGA (>4000 g). These findings are in accordance with those of several previous studies [9,37]. In general it could be shown that newborn size increased significantly with increasing prepregnancy weight status of the mother. Although maternal height, gestational weight gain and maternal age influenced newborn size too. During pregnancy maternal weight status has a profound impact on fetal development, birth outcome and offspring growth and development during later life [37,38]. It is well documented that nutritional deficiency during pregnancy but also an obesogenic fetal environment are associated with many complications during pregnancy and birth such as small for gestational age (SGA) as well as large for gestational age (LGA) newborn, an increased the risk of spontaneous abortions and stillbirths [17,34], an increased prevalence of gestational diabetes and hypertensitive pregnancy disorders such as pre-eclampsia [39] but also with complications at the time of labor and delivery [32].
Maternal prepregnancy obesity is an important risk factor for the need of caesarean section. The present study yielded significantly increased rates of caesarean section among morbidly obese mothers (BMI > 40 kg/m2). Normalweight, overweight and obese mothers showed similar rates of caesarean section (about 22%). The lowest rates of caesarean section were found among underweight women. In contrast 33% of morbidly obese women experienced a caesarean section. This high prevalence of caesarean section among morbidly obese women is in accordance with the results of several other studies which yielded an increased risk of caesarean section among obese and so called super-obese parturients, i.e. a BMI above 50.00 kg/m2 [16,32,40,41]. An Australian study for example, demonstrated that super-obese mothers i.e. a body mass index above 50 kg/m2 have a significantly higher risk of obstetric complications during pregnancy and birth. 51.6% of these super-obese women gave birth via caesarean section [42]. In general the increasing prevalence of extreme obesity among women of reproductive age [26,27] and associated complications during pregnancy and labor increased caesarean section rates worldwide [43,44,45,46]. The association between maternal morbid obesity and caesarean section is mainly due to the fact to that an obesogenic fetal environment affects intrauterine growth patterns [35] and increases the risk of giving birth to large for gestational age newborns and preterm birth [47]. Both factors increase the likelihood of caesarean delivery [32]. Macrosomia, i.e. birthweight above 4000 g, is often associated with cephalopelvic disproportion or shoulder dystocia and increases therefore the risk of obstetric complications and caesarean section. In the present study 23% of the LGA newborn were born via caesarean section. This was true of 21% of normal weight newborn (2500–4000 g). Furthermore it is well documented that obese women progress more slowly through the first stage of labor [48]. The high rates of caesarean section among extremely obese women however are discussed critically because severe complications during and after caesarean section have been reported [46,49,50]. Caesarean section in obese women poses many surgical, anesthetic and logistical challenges such as increased infectious morbidity, thromboembolic events [51] but also postpartum haemorrhage [52] wound complications [53,54] prolonged hospitalization. Although these adverse consequences of caesarean section among obese women could not be proved in the present study, the high rates of caesarean section among obese women can be interpreted as a problematic consequence of maternal obesity.
6. Conclusion
From the results of the present study we can conclude that maternal malnutrition i.e. moderate and severe underweight or obesity affects fetal growth patterns and consequently newborn size. Maternal underweight is mainly associated with reduced fetal growth, and small for gestational age newborn, while obesity is mainly associated with enhanced fetal growth large for gestational age newborn and an increased fetal growth rate. Morbidly obese women however show a higher incidence of caesarean sections. Consequently maternal malnutrition has a profound impact on fetal growth and pregnancy outcome.









 DownLoad:
DownLoad: