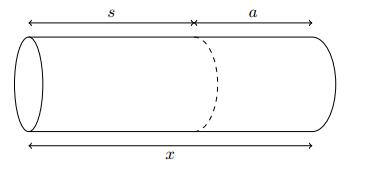
schematic representation of the variables on an E. coli bacterium
.We study the mathematical properties of a model of cell division structured by two variables – the size and the size increment – in the case of a linear growth rate and a self-similar fragmentation kernel. We first show that one can construct a solution to the related two dimensional eigenproblem associated to the eigenvalue $ 1 $ from a solution of a certain one dimensional fixed point problem. Then we prove the existence and uniqueness of this fixed point in the appropriate $ {\rm{L}} ^1 $ weighted space under general hypotheses on the division rate. Knowing such an eigenfunction proves useful as a first step in studying the long time asymptotic behaviour of the Cauchy problem.
Citation: Pierre Gabriel, Hugo Martin. Steady distribution of the incremental model for bacteria proliferation[J]. Networks and Heterogeneous Media, 2019, 14(1): 149-171. doi: 10.3934/nhm.2019008
| [1] | Pierre Gabriel, Hugo Martin . Steady distribution of the incremental model for bacteria proliferation. Networks and Heterogeneous Media, 2019, 14(1): 149-171. doi: 10.3934/nhm.2019008 |
| [2] | Fadia Bekkal-Brikci, Giovanna Chiorino, Khalid Boushaba . G1/S transition and cell population dynamics. Networks and Heterogeneous Media, 2009, 4(1): 67-90. doi: 10.3934/nhm.2009.4.67 |
| [3] | Pierre Degond, Sophie Hecht, Nicolas Vauchelet . Incompressible limit of a continuum model of tissue growth for two cell populations. Networks and Heterogeneous Media, 2020, 15(1): 57-85. doi: 10.3934/nhm.2020003 |
| [4] | Paulo Amorim, Alessandro Margheri, Carlota Rebelo . Modeling disease awareness and variable susceptibility with a structured epidemic model. Networks and Heterogeneous Media, 2024, 19(1): 262-290. doi: 10.3934/nhm.2024012 |
| [5] | Piotr Gwiazda, Karolina Kropielnicka, Anna Marciniak-Czochra . The Escalator Boxcar Train method for a system of age-structured equations. Networks and Heterogeneous Media, 2016, 11(1): 123-143. doi: 10.3934/nhm.2016.11.123 |
| [6] | Martino Bardi . Explicit solutions of some linear-quadratic mean field games. Networks and Heterogeneous Media, 2012, 7(2): 243-261. doi: 10.3934/nhm.2012.7.243 |
| [7] | Peter V. Gordon, Cyrill B. Muratov . Self-similarity and long-time behavior of solutions of the diffusion equation with nonlinear absorption and a boundary source. Networks and Heterogeneous Media, 2012, 7(4): 767-780. doi: 10.3934/nhm.2012.7.767 |
| [8] | Yacine Chitour, Guilherme Mazanti, Mario Sigalotti . Stability of non-autonomous difference equations with applications to transport and wave propagation on networks. Networks and Heterogeneous Media, 2016, 11(4): 563-601. doi: 10.3934/nhm.2016010 |
| [9] | Dieter Armbruster, Christian Ringhofer, Andrea Thatcher . A kinetic model for an agent based market simulation. Networks and Heterogeneous Media, 2015, 10(3): 527-542. doi: 10.3934/nhm.2015.10.527 |
| [10] | Andrea Tosin . Multiphase modeling and qualitative analysis of the growth of tumor cords. Networks and Heterogeneous Media, 2008, 3(1): 43-83. doi: 10.3934/nhm.2008.3.43 |
We study the mathematical properties of a model of cell division structured by two variables – the size and the size increment – in the case of a linear growth rate and a self-similar fragmentation kernel. We first show that one can construct a solution to the related two dimensional eigenproblem associated to the eigenvalue $ 1 $ from a solution of a certain one dimensional fixed point problem. Then we prove the existence and uniqueness of this fixed point in the appropriate $ {\rm{L}} ^1 $ weighted space under general hypotheses on the division rate. Knowing such an eigenfunction proves useful as a first step in studying the long time asymptotic behaviour of the Cauchy problem.
In structured population dynamics, finding the structuring variable(s) which best describes a phenomenon is a crucial question. For a population of proliferating cells or bacteria the variables usually considered are age, size (see [27,12,18]) or a combination of both (see [1,24,10] for modeling and [26,12,10,6] for mathematical analysis). Recent experimental work highlighted the limits of these models to describe bacteria, and a new variable to trigger division emerged: the size-increment, namely the size gained since the birth of the cell (see [22] and references therein for a review of the genesis of the related model). This so called 'adder principle ensures homeostasis with no feedback from the bacteria and explains many experimental data. In this model, bacteria are described by two parameters: their size-increment and their size, respectively denoted by
| $ \left\{ ∂tn(t,a,x)+∂a(g(x)n(t,a,x))+∂x(g(x)n(t,a,x))+B(a)g(x)n(t,a,x)=0,t≥0, x>a>0,g(x)n(t,0,x)=4g(2x)∫∞0B(a)n(t,a,2x)da,t≥0, x>0. \right. $ |
The function
| $ ∂tn(t,a,x)+∂a(g(x)n(t,a,x))+∂x(g(x)n(t,a,x))+B(a)g(x)n(t,a,x)=0,t≥0, x>a>0, $ | (1a) |
| $g(x)n(t,0,x)=∫10g(xz)∫∞0B(a)n(t,a,xz)da dμ(z)z,t≥0, x>0. $ | (1b) |
It appears that this model is a particular case of the one proposed in the pioneer work [10] for plants growing in a single dimension, mixing age and size control. Indeed, in this paper the authors noticed that in the case of a deterministic and positive growth rate, a size/age model is equivalent to a size/birth-size through the relation
First, we want the sum of the daughters sizes to be equal to the size of the mother. This rule, called mass conservation, prescribes
| $ ∫10zdμ(z)=1. $ | (2) |
We also assume that the division does not produce any arbitrarily small daughter by imposing that the support of
| $ θ:=infsuppμ>0and∃η∈(θ,1), suppμ⊂[θ,η]. $ | (3) |
In particular, these assumptions imply that the mean number of daughters
| $ ∃b≥0,suppB=[b,∞), $ | (4) |
see [8] for instance. It will be useful in our study to define the associated survivor function
| $ \Psi(a) = {\rm{e}} ^{-\int_0^a B(z)\, \, {\rm{d}} z}. $ |
For a given increment
| $ ∃k0>0,Ψ(a)=+∞O(a−k0). $ | (5) |
This assumption on the decay at infinity of the survivor function enables a wide variety of division rates. For instance, it is satisfied if there exists
| $ \forall\, a \geq A,\quad B(a)\geq \frac{k_0}{a}. $ |
The function
| $ Φ=BΨ=−Ψ′ $ | (6) |
which is the probability distribution that a cell divides at increment
| $ ∬xn(t,a,x)dadx=et∬xn0(a,x)dadx. $ | (7) |
This implies that if we look for a solution with separated variables
| $ ∂a(xN(a,x))+∂x(xN(a,x))+(1+xB(a))N(a,x)=0,x>a>0, $ | (8a) |
| $ N(0,x)=∫10∫∞0B(a)N(a,xz)da dμ(z)z2,x>0, $ | (8b) |
| $ N(a,x)≥0,x≥a≥0, $ | (8c) |
| $ ∫∞0∫x0N(a,x)dadx=1. $ | (8d) |
It is convenient to define the set
Theorem 1.1. Let
| $ N:(a,x)∈X↦Ψ(a)x2f(x−a) $ | (9) |
where
| $ f\in {\rm{L}} ^1( \mathbb{R} _+, x^l \, {\rm{d}} x) $ |
for all
| $ {\rm{supp}} \, f = [b_\theta,\infty) $ |
with
The fast decay of the function
Notice also that for the function
The article is organised as follows. In Section 2 we reduce the Perron eigenvalue problem with two variables to a fixed point problem for an integral operator in dimension one. Section 3 is dedicated to proving the existence and uniqueness of the fixed point by using functional analysis and Laplace transform methods. In Section 4 we go through the usefulness of knowing
Our study consists in constructing a solution to the eigenproblem (8) from the solution of a fixed point problem. First, we notice that the size
| $ M(a,s):=N(a,a+s). $ | (10) |
Thanks to this relation, it is equivalent to prove the existence of an eigenvector for the increment-size system or for the increment/birth-size system. To determine the equation verified by
| $ \partial_a((a+s)M(a,s))+\left(1 + (a+s)B(a)\right)M(a,s) = 0. $ |
Writing the non-local boundary condition (8b) with the new variables takes less calculation and more interpretation. In (8b) the number of cells born at size
| $ M(0,s) = \int_\theta^\eta \int_0^\frac{s}{z} B(a)M(a,\frac{s}{z}-a) \, {\rm{d}} a\ \frac{ {\rm{d}} \mu (z) }{z^2} $ |
since there is no mass for
| $ ∂a((a+s)M(a,s))+(1+(a+s)B(a))M(a,s)=0,a,s>0, $ | (11a) |
| $ M(0,s)=∫ηθ∫sz0B(a)M(a,sz−a)da dμ(z)z2,s>0, $ | (11b) |
| $ M(a,s)≥0,a,s≥0, $ | (11c) |
| $ ∫R2+M(a,s)dads=1. $ | (11d) |
Considering the variable
| $ M(a,s) = \frac{\Psi(a)}{(a+s)^2} s^2M(0,s). $ |
Having this expression in mind, we note that for any nonnegative function
| $ M_f : (a,s) \mapsto \frac{\Psi(a)}{(a+s)^2}f(s) $ |
is a solution of (11a) and satisfies (11c). Then it remains to choose the appropriate function
| $ Tf(s)=∫ηθ∫sz0Φ(sz−a)f(a)dadμ(z), $ | (12) |
where
Lemma 2.1. The function
Proof.
| $ Mf satisfies (11b)⟺f(s)s2=∫ηθ∫sz0B(a)Ψ(a)(sz)2f(sz−a)da dμ(z)z2⟺f(s)=∫ηθ∫sz0Φ(a)f(sz−a)da dμ(z)⟺f(s)=∫ηθ∫sz0Φ(sz−a)f(a)da dμ(z)⟺f(s)=Tf(s) $ |
The operator
Lemma 2.2. For all
Proof. We start with
| $ ∫βα|Tf(s)|slds≤∫ηθ∫βαsl∫sz0Φ(sz−a)|f(a)|dadsdμ(z)≤∫ηθ∫αz0|f(a)|∫βαΦ(sz−a)sldsdadμ(z)+∫ηθ∫βzαz|f(a)|∫βzaΦ(sz−a)sldsdadμ(z)≤∫ηθ∫αz0|f(a)|∫βz−aαz−aΦ(σ)(a+σ)lzl+1dσdadμ(z)+∫ηθ∫βzαz|f(a)|∫βz−a0Φ(σ)(a+σ)lzl+1dσdadμ(z)≤∫ηθzl+1∫αz0|f(a)|aldadμ(z)+∫ηθzl+1∫∞αz|f(a)|aldadμ(z)≤θl‖f‖L1(R+,slds), $ |
which gives the conclusion by passing to the limits
For the second part we begin with the proof that under condition (5), for any
| $ \int_0^\infty \Phi(a)a^k \, {\rm{d}} a < \infty. $ |
First, recall that
| $ \int_0^\beta \Phi(a)a^k \, {\rm{d}} a\leq \int_0^1 \Phi(a) \, {\rm{d}} a + \int_1^\beta \Phi(a)a^k \, {\rm{d}} a \leq 1 + k\int_1^\beta \Psi(a) a^{k-1} \, {\rm{d}} a $ |
and the last integral converges when
| $ ∫β0|Tf(s)|skds≤∫ηθ∫β0sk∫sz0Φ(sz−a)|f(a)|dadsdμ(z)=∫ηθ∫βz0|f(a)|∫βz−a0Φ(σ)(a+σ)kzk+1dσdadμ(z)≤C∫ηθzk+1∫βz0|f(a)|ak∫βz−a0Φ(σ)dσdadμ(z)+C∫ηθzk+1∫βz0|f(a)|∫βz−a0Φ(σ)σkdσdadμ(z)≤Cηk(‖f‖L1(R+,skds)+‖Φ‖L1(R+,skds)‖f‖L1(R+)). $ |
In this section we prove the existence of a unique nonnegative and normalized fixed point of the operator
Let us first recall some definitions from the Banach lattices theory (for more details, see [9,23]). Let
| $ f\geq 0\text{ if and only if } f(s)\geq 0\ \nu\text{-a.e. on }\Omega. $ |
Furthermore, endowed with its standard norm, the space
To prove the existence of an eigenvector associated to the eigenvalue
Theorem 3.1. Let
Due to a lack of compactness of the operator
| $ TΣf(s)=∫ηθ∫min(sz,Σ)bθΦ(sz−a)f(a)dadμ(z) $ | (13) |
| $ ={∫ηθ∫szbθΦ(sz−a)f(a)dadμ(z),bθ≤s<θΣ,∫sΣθ∫ΣbθΦ(sz−a)f(a)dadμ(z)+∫ηsΣ∫szbθΦ(sz−a)f(a)dadμ(z),θΣ≤s≤ηΣ,∫ηθ∫ΣbθΦ(sz−a)f(a)dadμ(z),ηΣ<s≤Σ. $ |
Defining the lower bound of the domain as
The following lemma ensures that the truncated operator
Lemma 3.2. If
| $ (Tf)_{|[b_\theta,\Sigma]} = T_\Sigma (f_{|[b_\theta,\Sigma]}). $ |
Lemma 3.2 is a straightforward consequence of the definition of operator
| $ {∀ϵ>0, ∀ω⊂⊂Ω, ∃δ∈(0,dist(ω,cΩ)) such that ‖τhf−f‖L1(ω,ν)<ϵ, ∀h∈(−δ,δ), ∀f∈F $ | (14) |
| $ {∀ϵ>0, ∃ω⊂⊂Ω, such that ‖f‖L1(Ω∖ω,ν)<ϵ, ∀f∈F $ | (15) |
where
Theorem 3.3 (from [3], corollary 4.27). If
Using Theorem 3.1, we prove the existence of an eigenpair
Proposition 1. Let
Applying Theorem 3.3, to
| $ \mathcal{F} = \{T_\Sigma f, f\in {\rm{L}} ^1((b_\theta,\Sigma),s^l \, {\rm{d}} s), \|f\|_{ {\rm{L}} ^1((b_\theta,\Sigma),s^l \, {\rm{d}} s)}\leq 1\}, $ |
which is bounded in
Lemma 3.4. Let
| $ \Sigma > \max(\frac{b}{1-\theta},1), $ |
the set
Proof of Lemma 3.4. The set
| $ ∫βα|TΣf(s+h)−TΣf(s)|slds≤∫θΣ−hα|TΣf(s+h)−TΣf(s)|slds=:(A)+∫θΣθΣ−h|TΣf(s+h)−TΣf(s)|slds=:(B)+∫ηΣ−hθΣ|TΣf(s+h)−TΣf(s)|slds=:(C)+∫ηΣηΣ−h|TΣf(s+h)−TΣf(s)|slds=:(D)+∫βηΣ|TΣf(s+h)−TΣf(s)|slds=:(E). $ |
since for
| $ (C)=∫ηΣ−hθΣ|TΣf(s+h)−TΣf(s)|slds≤∫ηΣ−hθΣ|∫s+hΣθ∫ΣbθΦ(s+hz−a)f(a)dadμ(z)−∫sΣθ∫ΣbθΦ(sz−a)f(a)dadμ(z)|slds+∫ηΣ−hθΣ|∫ηs+hΣ∫s+hzbθΦ(s+hz−a)f(a)dadμ(z)−∫ηsΣ∫szbθΦ(sz−a)f(a)dadμ(z)|slds≤∫ηΣ−hθΣsl∫sΣθ∫Σbθ|Φ(s+hz−a)−Φ(sz−a)||f(a)|dadμ(z)ds=:(C1)+∫ηΣ−hθΣsl∫s+hΣsΣ∫ΣbθΦ(s+hz−a)|f(a)|dadμ(z)ds=:(C2) $ |
| $ +∫ηΣ−hθΣsl∫ηs+hΣ∫szbθ|Φ(s+hz−a)−Φ(sz−a)||f(a)|dadμ(z)ds=:(C3)+∫ηΣ−hθΣsl∫ηs+hΣ∫s+hzszΦ(s+hz−a)|f(a)|dadμ(z)ds=:(C4)+∫ηΣ−hθΣsl∫s+hΣsΣ∫szbθΦ(s+hz−a)|f(a)|dadμ(z)ds=:(C5) $ |
The integrals
| $ (C1)=∫ηΣ−hθΣsl∫sΣθ∫Σbθ|Φ(s+hz−a)−Φ(sz−a)||f(a)|dadμ(z)ds=∫η−hΣθ∫Σbθ|f(a)|∫ηΣ−hzΣ|Φ(s+hz−a)−Φ(sz−a)|sldsdadμ(z)=∫η−hΣθzl+1∫Σbθ|f(a)|∫ηΣ−hz−aΣ−a|τhzΦ(σ)−Φ(σ)|(a+σ)ldσdadμ(z)=∫η−hΣθzl+1∫Σbθ|f(a)|al∫ηΣ−hz−aΣ−a|τhzΦ(σ)−Φ(σ)|dσdadμ(z)≤θlsupε∈[θ,η]‖τhεΦ−Φ‖L1(R+). $ |
These integrals are as small as needed when
| $ (C2)=∫ηΣ−hθΣsl∫s+hΣsΣ∫ΣbθΦ(s+hz−a)|f(a)|dadμ(z)ds=∫θ+hΣθ∫Σbθ|f(a)|∫zΣθΣΦ(s+hz−a)sldsdadμ(z)+∫η−hΣθ+hΣ∫Σbθ|f(a)|∫zΣzΣ−hΦ(s+hz−a)sldsdadμ(z)+∫ηη−hΣ∫Σbθ|f(a)|∫ηΣ−hzΣ−hΦ(s+hz−a)sldsdadμ(z)≤∫ηθ∫Σbθ|f(a)|∫zΣzΣ−hΦ(s+hz−a)sldsdadμ(z)=∫ηθzl+1∫Σbθ|f(a)|∫Σ−aΣ−hz−aΦ(σ+hz)(a+σ)ldσdadμ(z)≤∫ηθzl+1∫Σbθ|f(a)|al∫Σ−aΣ−hz−aΦ(σ+hz)dσdadμ(z)≤θlsup|I|=hθ∫IΦ(a)da $ |
which is small when
| $ (C4)=∫ηΣ−hθΣsl∫ηs+hΣ∫s+hzszΦ(s+hz−a)|f(a)|dadμ(z)ds=∫ηθ+hΣ∫zΣ−hθΣsl∫s+hzszΦ(s+hz−a)|f(a)|dadsdμ(z)=∫ηθ+hΣ∫zΣ−hθΣsl∫0−hzΦ(hz+a′)|f(sz−a′)|da′dsdμ(z)=∫ηθ+hΣ∫0−hzΦ(hz+a′)∫zΣ−hθΣ|f(sz−a′)|sldsda′dμ(z)=∫ηθ+hΣzl+1∫0−hzΦ(hz+a′)∫Σ−hz−a′θΣz−a′|f(σ)|(σ+a′)ldσda′dμ(z)≤∫ηθ+hΣzl+1∫0−hzΦ(hz+a′)da′dμ(z)≤θl(1−Ψ(hθ)), $ |
and the continuity of
| $ (B)=∫θΣθΣ−h|∫s+hΣθ∫ΣbθΦ(s+hz−a)f(a)dadμ(z)+∫ηs+hΣ∫s+hzbθΦ(s+hz−a)f(a)dadμ(z)−∫ηθ∫szbθΦ(sz−a)f(a)dadμ(z)|slds≤∫ηθ∫Σbθ|f(a)|∫θΣθΣ−h[Φ(s+hz−a)+Φ(sz−a)]sldsdadμ(z)≤∫ηθzl+1∫Σbθ|f(a)|al∫θΣz−aθΣ−hz−a[Φ(σ+hz)+Φ(σ)]dσdadμ(z)≤2θlsup|I|=hθ∫IΦ(a)da $ |
and again the last term vanishes as
| $ ∫αbθ|TΣf(s)|slds $ |
| $ ≤∫αbθsl∫ηθ∫szbθΦ(sz−a)|f(a)|dadμ(z)ds≤∫ηθ∫bθzbθ|f(a)|∫αbθΦ(sz−a)sldsdadμ(z)+∫ηθ∫αzbθz|f(a)|∫αzaΦ(sz−a)sldsdadμ(z)≤∫ηθzl+1∫bθzbθ(Ψ(bθz−a)−Ψ(αz−a))|f(a)|aldazdμ(z)+∫ηθ∫αzbθz(1−Ψ(αz−a))|f(a)|aldazdμ(z)≤θl(1−Ψ(α−bθθ)), $ | (16) |
since for
As done before, we choose a
| $ ∫Σβ|TΣf(s)|slds≤∫ηθ∫Σbθ|f(a)|∫ΣβΦ(sz−a)sldsdadμ(z)≤∫ηθzl+1∫Σbθ|f(a)|al∫Σz−aβz−aΦ(σ)dσdadμ(z)≤θlsup|I|=Σ−βθ∫IΦ(a)da, $ | (17) |
which is small when
We have checked the assumptions of Theorem 3.3 for the family
To prove the irreducibility of the operator
| $ T_\Sigma f(s) = \left\{ ∫sηbθf(a)∫ηθΦ(sz−a)dμ(z)da+∫sθsηf(a)∫saθΦ(sz−a)dμ(z)da,bθ≤s<θΣ,∫sηbθf(a)∫ηθΦ(sz−a)dμ(z)da+∫Σsηf(a)∫saθΦ(sz−a)dμ(z)da,θΣ≤s≤ηΣ,∫Σbθf(a)∫ηθΦ(sz−a)dμ(z)da,ηΣ<s≤Σ. \right. $ |
Lemma 3.5. Let
Proof. Let
| $ T_\Sigma f_\omega(s)\geq \int_{s_0}^{s_0+\zeta}\int_\theta^{\theta+\xi}\Phi(\frac{s}{z}-a) {\rm{d}} \mu (z) f_\omega(a) \, {\rm{d}} a. $ |
For
Proof of Proposition 1. Lemma 3.4 shows that the set
We now want to show that up to a subsequence,
Proposition 2. Under hypotheses (2)- (5) there exists a nonnegative and normalized fixed point
| $ f\in {\rm{L}} ^1( \mathbb{R} _+,(s^k+s^l) \, {\rm{d}} s) $ |
for all
First, we will show that the sequence
Lemma 3.6. If
| $ 1−Ψ((1η−1)Σ)≤ρΣ≤1−Ψ(Σθ−bθ). $ | (18) |
Proof. Integrating the equality
| $ ρΣ∫ΣbθfΣ(s)ds=∫θΣbθ∫ηθ∫szbθΦ(sz−a)f(a)dadμ(z)ds=:(A)+∫ηΣθΣ∫sΣθ∫ΣbθΦ(sz−a)f(a)dadμ(z)ds=:(B)+∫ηΣθΣ∫ηsΣ∫szbθΦ(sz−a)f(a)dadμ(z)ds=:(C)+∫ΣηΣ∫ηθ∫ΣbθΦ(sz−a)f(a)dadμ(z)ds=:(D) $ |
| $ (A)=∫ηθ∫bθzbθfΣ(a)∫θΣbθzΦ(sz−a)dsdadμ(z)+∫ηθ∫θΣzbθzfΣ(a)∫θΣzaΦ(sz−a)dsdadμ(z)=∫ηθz∫bθzbθfΣ(a)[Ψ(bθz−a)−Ψ(θΣz−a)]dadμ(z)+∫ηθz∫θΣzbθzfΣ(a)[1−Ψ(θΣz−a)]dadμ(z)(B)=∫ηθfΣ(a)∫Σbθ∫ηΣzΣΦ(sz−a)dsdadμ(z)=∫ηθz∫ΣbθfΣ(a)[Ψ(Σ−a)−Ψ(ηΣz−a)]dadμ(z) $ |
| $ (C)=∫ηθ∫θΣzbθfΣ(a)∫zΣθΣΦ(sz−a)dsdadμ(z)+∫ηθ∫ΣθΣzfΣ(a)∫zΣzaΦ(sz−a)dsdadμ(z)=∫ηθz∫θΣzbθfΣ(a)[Ψ(θΣz−a)−Ψ(Σ−a)]dadμ(z)+∫ηθz∫ΣθΣzfΣ(a)[1−Ψ(Σ−a)]dadμ(z)(D)=∫ηθ∫ΣbθfΣ(a)∫ΣηΣΦ(sz−a)dsdadμ(z)=∫ηθz∫ΣbθfΣ(a)[Ψ(ηΣz−a)−Ψ(Σz−a)]dadμ(z) $ |
Then notice that for
| $ \frac{b_\theta}{z}-a \leq \frac{b_\theta}{z}-b_\theta = b_\theta\left(\frac{1}{z}-1\right)\leq b_\theta\left(\frac{1}{\theta}-1\right) = b, $ |
so as in the computations leading to (16),
| $ ρΣ∫ΣbθfΣ(s)ds=∫ΣbθfΣ(s)ds−∫ηθz∫ΣbθΨ(Σz−a)fΣ(a)dadμ(z). $ | (19) |
Using the fact that the function
Now we show that up to a subsequence,
| $ \Sigma_0: = \inf\left\{\Sigma > \max(\frac{1}{1-\theta}b,1){\rm{\ such\ that\ }}\rho_\Sigma > \frac{1}{2}\right\}. $ |
Lemma 3.7. Under hypotheses (2), (3), (4) and (5), the set of eigenfunctions
Proof. Let
| $ ‖τhfΣ−fΣ‖L1(ω,(sk+sl)ds)≤2‖τhTΣfΣ−TΣfΣ‖L1(ω,(sk+sl)ds)≤2(βk+αl)‖τhTΣfΣ−TΣfΣ‖L1([α,β])≤2(βk+αl)‖τhTΣfΣ−TΣfΣ‖L1([α,Σ]). $ |
The last quantity is small when
| $ ‖fΣ‖L1((bθ,α),(sk+sl)ds)=1ρΣ∫αbθTΣfΣ(s)(sk+sl)ds≤2∫αbθTΣfΣ(s)slds+2αk∫αbθTΣfΣ(s)ds≤2θl(1−Ψ(α−bθθ))+2αk(1−Ψ(α−bθθ))≤2(θl+αk)(1−Ψ(α−bθθ)) $ |
which is again independent of
| $ \rho_\Sigma\int_\beta^\Sigma f_\Sigma(a) \, {\rm{d}} a = \int_\beta^{\theta\Sigma}T_\Sigma f_\Sigma(a) \, {\rm{d}} a + \int_{\theta\Sigma}^{\eta \Sigma}T_\Sigma f_\Sigma(a) \, {\rm{d}} a + \int_{\eta \Sigma}^\Sigma T_\Sigma f_\Sigma(a) \, {\rm{d}} a. $ |
For the first integral, we compute
| $ ∫θΣβTΣfΣ(a)da=∫ηθz∫βzbθ[Ψ(βz−a)−Ψ(θΣz−a)]fΣ(a)dadμ(z)+∫ηθz∫θΣzβz[1−Ψ(θΣz−a)]fΣ(a)dadμ(z). $ |
The two other integrals correspond to the integrals
| $ ρΣ∫ΣβfΣ(a)da=∫ηθz∫βzbθΨ(βz−a)fΣ(a)dadμ(z)+∫ηθz∫ΣβzfΣ(a)dadμ(z)−∫ηθz∫ΣbθΨ(Σz−a)fΣ(a)dadμ(z). $ |
We deal with the last integral using (19) and obtain after interverting integrals
| $ ρΣ∫ΣβfΣ(a)da=∫βηbθfΣ(a)∫ηθzΨ(βz−a)dμ(z)da+∫βθβηfΣ(a)∫βaθzΨ(βz−a)dμ(z)da+∫βθβηfΣ(a)∫ηβazdμ(z)da+∫ΣβθfΣ(a)da+ρΣ∫ΣbθfΣ(a)da−∫ΣbθfΣ(a)da⟺∫βηbθfΣ(a)da=∫βηbθfΣ(a)∫ηθzΨ(βz−a)dμ(z)da+∫βθβηfΣ(a)∫βaθzΨ(βz−a)dμ(z)da+ρΣ∫βbθfΣ(a)da−∫βθβηfΣ(a)∫βaθzdμ(z)da⟺∫βηβfΣ(a)∫ηθz[1−Ψ(βz−a)]dμ(z)da+(1−ρΣ)∫βbθfΣ(a)da+∫βθβηfΣ(a)∫βaθz[1−Ψ(βz−a)]dμ(z)da=∫βbθfΣ(a)∫ηθzΨ(βz−a)dμ(z)da $ |
Since
| $ \int_\beta^{r\beta} f_\Sigma(a)\int_\theta^\eta z\left[1- \Psi(\frac{\beta}{z}-a) \right] {\rm{d}} \mu (z) \, {\rm{d}} a \leq \int_{b_\theta}^\beta f_\Sigma(a)\int_\theta^\eta z\Psi(\frac{\beta}{z}-a) {\rm{d}} \mu (z) \, {\rm{d}} a, $ |
then
| $ \left(1-\Psi((\frac{1}{\eta}-r)\beta)\right)\int_\beta^{r\beta}f_\Sigma(a) \, {\rm{d}} a \leq \Psi((\frac{1}{\eta}-1)\beta), $ |
and finally
| $ \int_\beta^{r\beta}f_\Sigma(a)(a^l+a^k) \, {\rm{d}} a \leq (\beta^l+(r\beta)^k)\frac{\Psi((\frac{1}{\eta}-1)\beta)}{\left(1-\Psi((\frac{1}{\eta}-r)\beta)\right)} \leq 4(r\beta)^k\Psi((\frac{1}{\eta}-1)\beta) $ |
for
| $ ∫∞βfΣ(s)(sk+sl)ds=∞∑j=0∫rj+1βrjβfS(s)(sk+sl)ds≤4rk∞∑j=0(rjβ)kΨ((1η−1)rjβ) $ |
| $ ≤4Crk∞∑j=0(rjβ)k((1η−1)rjβ)−k0≤Ck,k0,η,rβk0−k $ |
due to hypothesis (5), for
We are now ready to prove the existence and uniqueness of a fixed point for the operator
Proof of Proposition 2. We have proved in Lemma 3.7 that the set of eigenfunctions
| $ ‖f−Tf‖L1(R+,(sk+sl)ds)≤‖f−fΣ‖L1(R+,(sk+sl)ds)+(1−ρΣ)+‖TΣfΣ−Tf‖L1(R+,(sk+sl)ds). $ |
The first term of the right-hand side tends to zero as
| $ ‖TΣfΣ−Tf‖L1(R+,(sk+sl)ds)≤‖TΣfΣ−TfΣ‖L1(R+,(sk+sl)ds)⏟=0+‖T(f−fΣ)‖L1(R+,(sk+sl)ds)≤‖f−fΣ‖L1(R+,(sk+sl)ds) $ |
due to Lemma 3.2 and to the continuity of
To prove uniqueness of the fixed point, we consider
| $ f(s)=∫ηθΦ∗f(sz)dμ(z). $ | (20) |
Since
| $ L[f−f1](y)=∫ηθL[f−f1](zy)L[Φ](zy)zdμ(z). $ | (21) |
The Laplace transform
| $ \mathcal{L}[f-f_1](0) = \int_0^\infty f(s) \, {\rm{d}} s - \int_0^\infty f_1(s) \, {\rm{d}} s = 0. $ |
We now define the functions
| $ \overline{{\rm{L}}}(y) = \sup\limits_{x\in [0,y]}\mathcal{L}[f-f_1](x)\quad {\rm{\ and\ }}\quad \underline{{\rm{L}}}(y) = \inf\limits_{x\in [0,y]}\mathcal{L}[f-f_1](x). $ |
By continuity in
| $ \forall y\geq 0,\qquad \overline{{\rm{L}}}(y)\geq 0,\ \underline{{\rm{L}}}(y)\leq 0. $ |
From (21), we obtain the inequality
| $ \mathcal{L}[f-f_1](y)\leq \overline{{\rm{L}}}(\eta y)\int_\theta^\eta\mathcal{L}[\Phi](zy)z {\rm{d}} \mu (z) \leq \overline{{\rm{L}}}(\eta y), $ |
since
| $ \mathcal{L}[f-f_1](x)\leq \overline{{\rm{L}}}(\eta x) \leq \overline{{\rm{L}}}(\eta y), $ |
from which we deduce
| $ ¯L(y)≤¯L(ηy). $ | (22) |
Iterating (22), we obtain for all
| $ \overline{{\rm{L}}}(y)\leq \overline{{\rm{L}}}(\eta^j y). $ |
Letting
It remains to prove that
We are now ready to prove the main theorem of the paper.
Proof of Theorem 1.1. Combining Lemma 2.1 and Proposition 2, we construct a solution to (11) using
| $ M(a,s): = \frac{\psi(a)}{(a+s)^2}f(s). $ |
It remains to prove uniqueness of the solution in the appropriate space. This solution belongs to
| $ ∫∞bθ∫∞0M(a,s)(1+s2)dads=∫∞bθ∫∞01(a+s)2f(s)Ψ(a)dads+∫∞bθ∫∞0s2(a+s)2f(s)Ψ(a)dads≤∫∞bθf(s)s−2∫∞0Ψ(a)dads+∫∞bθf(s)∫∞0Ψ(a)dads=‖f‖L1((bθ,∞),(1+s−2)ds)<∞ $ |
because
| $ M_1(a,s) = \frac{\Psi(a)}{(a+s)^2}f_1(s). $ |
For
| $ ∫∞0f1(s)ds=1β−α∫∞0∫βα(a+s)2Ψ(a)M1(a,s)dads≤2(β2+1)(β−α)Ψ(β)‖M1‖L1(R+,(1+s2)ds), $ |
and this ensures that
Now that we have solved the eigenvalue problem, we would like to characterize the asymptotic behaviour of a solution
| $ |n(t,a,x)|≤CetN(a,x),t≥0, x>a>0. $ | (23) |
It is usually ensured by the hypothesis
| $ \mathcal{H}[n] = \int_{b_\theta}^\infty\int_0^{x-b_\theta}xN(a,x)H\left(\frac{n(a,x)}{N(a,x)}\right) \, {\rm{d}} a \, {\rm{d}} x $ |
which satisfies the following entropy property.
Proposition 3. If
| $ ddtH[n(t,⋅,⋅)e−t]=−D[n(t,⋅,⋅)e−t], $ | (24) |
with
| $ D[n]=∫∞bθx2N(0,x)[∫ηθ∫xz−bθ0H(n(a,xz)N(a,xz))dνx(a,z)−H(∫ηθ∫xz−bθ0n(a,xz)N(a,xz)dνx(a,z))]dx $ |
where
Before proving this proposition, we make a remark about the conservative problem (i.e. when only one daughter out of two is kept after division). In this case, the dominant eigenvalue is
| $ ∂∂a(x2N)+∂∂x(x2N)=−x2BN, $ | (25) |
which might also be obtained multiplying (8a) by
Proof. Easy computations lead to
| $ \frac{\partial}{\partial t} \frac{n {\rm{e}} ^{-t}}{N} + x \frac{\partial}{\partial a} \frac{n {\rm{e}} ^{-t}}{N} + x \frac{\partial}{\partial x} \frac{n {\rm{e}} ^{-t}}{N} = 0, $ |
where
| $ ∂∂t(xNH(ne−tN))+∂∂a(x2NH(ne−tN))+∂∂x(x2NH(ne−tN))=−x2BNH(ne−tN), $ | (26) |
and integrating (26) over
| $ ddt∬(bθ,∞)×(0,x−bθ)xNH(ne−tN)dadx=∫∞bθx2N(0,x)H(n(t,0,x)e−tN(0,x))dx−∫∞bθx2N(x−bθ,x)H(n(t,x−bθ,x)e−tN(x−bθ,x))dx+∫∞0(a+bθ)2N(a,a+bθ)H(n(t,a,a+bθ)e−tN(a,a+bθ))da−∫∞bθ∫x−bθ0x2BNH(ne−tN)dadx=∫∞bθx2N(0,x)H(e−tN(0,x)∫ηθ∫xz−bθ0B(a)n(t,a,xz)dadμ(z)z2)dx−2∫ηθ∫∞bθ∫x−bθ0x2BNH(ne−tN)dadxzdμ(z)=∫∞bθx2N(0,x)H(∫ηθ∫xz−bθ0n(t,a,xz)e−tN(a,xz)dνx(a,z))dx−2∫ηθ∫bθzbθ∫xz−bθ0x2B(a)N(a,xz)H(n(t,a,xz)e−tN(a,xz))dadxdμ(z)z2−2∫ηθ∫∞bθ∫xz−bθ0x2B(a)N(a,xz)H(n(t,a,xz)e−tN(a,xz))dadxdμ(z)z2=∫∞bθx2N(0,x)[H(∫ηθ∫xz−bθ0n(t,a,xz)e−tN(a,xz)dνx(a,z))−∫ηθ∫xz−bθ0H(n(t,a,xz)e−tN(a,xz))dνx(a,z)]dx, $ |
since for
Appropriate choices of the function
| $ \lambda_j = 1+\frac{2ij\pi}{\log 2}, \qquad N_j(a,x) = x^{1-\lambda_j}N(a,x), \qquad \phi_j(a,x) = x^{\lambda_j}, $ |
so we expect a behavior as in [2], i.e. the convergence of
| $ \sum\limits_{j\in \mathbb{Z} } \langle n^0,\phi_j\rangle\, {\rm{e}} ^{\frac{2ij\pi t}{\log 2}}N_j(a,x), $ |
where
We have proved the existence and uniqueness of a solution of the eigenproblem (8) in the special yet biologically relevant case of linear growth rate with a self-similar fragmentation kernel. Hypotheses on both this kernel and the division rate are fairly general.
As possible future work we can imagine to extend the result to general growth rates. In this case the Perron eigenvalue is not explicit and it has to be determined in the same time as the eigenfunction, as in [26,12,6]. If we denote by
| $ P_\lambda(s) = \int_0^1 e^{-\lambda\int_s^{\frac sz}\frac{ \, {\rm{d}} u}{g(u)}}(\Phi*P_\lambda) (\frac{s}{z})\frac{ {\rm{d}} \mu (z) }{z} $ |
with
| $ N : (a,x) \mapsto \frac{\Psi(a)}{g(x)}e^{-\lambda\int_0^x \frac{ \, {\rm{d}} \alpha}{g(\alpha)}}P_\lambda(x-a). $ |
Additionally for nonlinear growth rates, the function
The other natural continuation of the present work is the proof of the well-posedness and the long-time behavior of the evolution equation, as in [26,12]. To do so one can take advantage of the General Relative Entropy as in [14,4,2] or use general spectral methods [28,16].
The authors are very grateful to Marie Doumic for having suggested them the problem treated in this paper, and for the many fruitful discussions.
| 1. | Bertrand Cloez, Benoîte de Saporta, Tristan Roget, Long-time behavior and Darwinian optimality for an asymmetric size-structured branching process, 2021, 83, 0303-6812, 10.1007/s00285-021-01695-y | |
| 2. | Pierre Gabriel, Hugo Martin, Periodic asymptotic dynamics of the measure solutions to an equal mitosis equation, 2022, 5, 2644-9463, 275, 10.5802/ahl.123 | |
| 3. | Katarzyna Pichór, Ryszard Rudnicki, Cell cycle length and long‐time behavior of an age‐size model, 2022, 45, 0170-4214, 5797, 10.1002/mma.8139 | |
| 4. | Mingtao Xia, Chris D. Greenman, Tom Chou, PDE Models of Adder Mechanisms in Cellular Proliferation, 2020, 80, 0036-1399, 1307, 10.1137/19M1246754 | |
| 5. | Ignacio Madrid, Exponential Ergodicity of a Degenerate Age-Size Piecewise Deterministic Process, 2023, 187, 0167-8019, 10.1007/s10440-023-00597-z |
schematic representation of the variables on an E. coli bacterium
Left: simulation of the function
Domain of the model, with respect to the choice of variables to describe the bacterium. Grey: domain where the bacteria densities may be positive. Arrows: transport. Left: size increment/size. Right: size increment/birth size. Dashed: location of cells of size $x_1$