1. Introduction
Sensory analysis of coffee is an important tool for monitoring coffee quality. Many techniques are available to suit the various objectives of a specific study, so it is necessary to carefully select the most appropriate technique to achieve the objectives with precision. Descriptive analysis has been used to monitor aroma formation during roasting [1]. A study of a particular attribute, such as texture, have also used descriptive analysis successfully [2]. Moreover, a sensory analysis with pre-determined attributes has been used to evaluate different ways of preparing beverages [3].
On the other hand, the evaluation of soluble coffee consumers' purchasing preferences demands another type of technique [4]. Specific techniques are also used for the commercial evaluations of coffee, such as those available from the American Special Coffee Association [5] and the Brazilian Speciality Coffee Association [6], which can be difficult to use in scientific studies.
Free choice profiling (FCP) is a descriptive sensory analysis based on the principle that people perceive the same characteristics in foods but express themselves differently to describe them [7].
FCP has been used to evaluate various types of food due to the many advantages that this technique presents. For example, Thamke et al. [8] used FCP to evaluate six formulations of dark chocolate, and the results showed a high similarity among the subjective evaluations by different tasters and the sensory evaluations, rendering FCP suitable for scientific investigations. Finally, a comparison of the results of the descriptive analysis and FCP to assess the quality of hop varieties showed good concordance [9].
This technique offers the advantage of being less time-consuming because there is no need for training individuals. Moreover, it is not necessary to develop a consensual terminology, and the number of descriptors employed may vary according to the experience of the panellists and their familiarity with the product. To accommodate different numbers and classes of attributes among panellists, FCP requires the use of generalized procrustes analysis (GPA) for data treatment. GPA transforms the data, avoiding variations or different interpretations of the attributes by the panellists while still allowing the detection of sensory differences [10]. Transformation of scale, rotation and translation are performed on the data. Transformation of scale corrects variations associated with the use of different scale amplitudes by panellists (individual settings). The second (rotation transformation) correction accounts for different interpretations of the terms and indicates panellists' agreement or disagreement in relation to the stimulus and denominations that were employed. Finally, the translation transformation corrects the intensity variations of the attributes appointed by the panellists [10,11].
To recommend a new coffee genotype, it is important to study the impact of factors such as genetic background, soil, climate and processing on coffee quality independently. In recent years, different genotypes (collection of IPRs) resulting from crosses among Coffea arabica, Villa Sarchi and the Timor Hybrid (Sarchimor) were registered and launched by the Paraná Agronomy Institute (IAPAR). These genotypes show not only high productivity but also resistance to coffee rust comparable with that of other coffee varieties [12,13,14,15].
Environmental conditions strongly influence coffee development and, consequently, its quality [16,17]. The effects of altitude and temperature are associated with the aroma precursors and flavours of the coffee beverage [18]. However, studies have shown that altitude has less influence on the quality of beverage in crops located in higher latitude [19].
The coffee region of Paraná, Brazil was defined as a function of temperature and precipitation, which are the major limiting factors in the cultivation of coffee. This region is located between the parallels 23-25 South latitude and between the meridians 49-53 West longitude [20]. The temperature increase and the decrease in altitude occur from east to west in this region [21]. Historically, it has been one of the major coffee producing regions, but coffee cultivation culture is currently being replaced by soybean and corn cultivation, which are highly mechanized.
The adaptability of cultivars to a production location is a basic condition for coffee farmers to choose and adopt new coffee cultivars. Usually, the agronomic characteristics of new cultivars are evaluated in different locations to identify their production potential. However, few studies have been conducted thus far assessing the sensory attributes of new cultivars in different locations. A large number of samples, lengthy training, and difficulty of maintaining the same team of tasters, along with scarce economic resources, are the main causes of the lack of evaluations of new cultivars.
The objective of this study was to apply free choice profiling sensory analysis to investigate the influence of genetic variability on the sensory characteristics of the following coffee genotypes: Iapar 59, IPR 97, IPR 98, IPR 99, IPR 100, IPR 101, IPR 102, IPR 103, IPR 104, IPR 105, IPR 106, IPR 107, IPR 108 and Catuaí grown in two locations in the Paraná state, Brazil (Londrina and Mandaguari).
2. Experimental design
2.1. Conditions of plantations
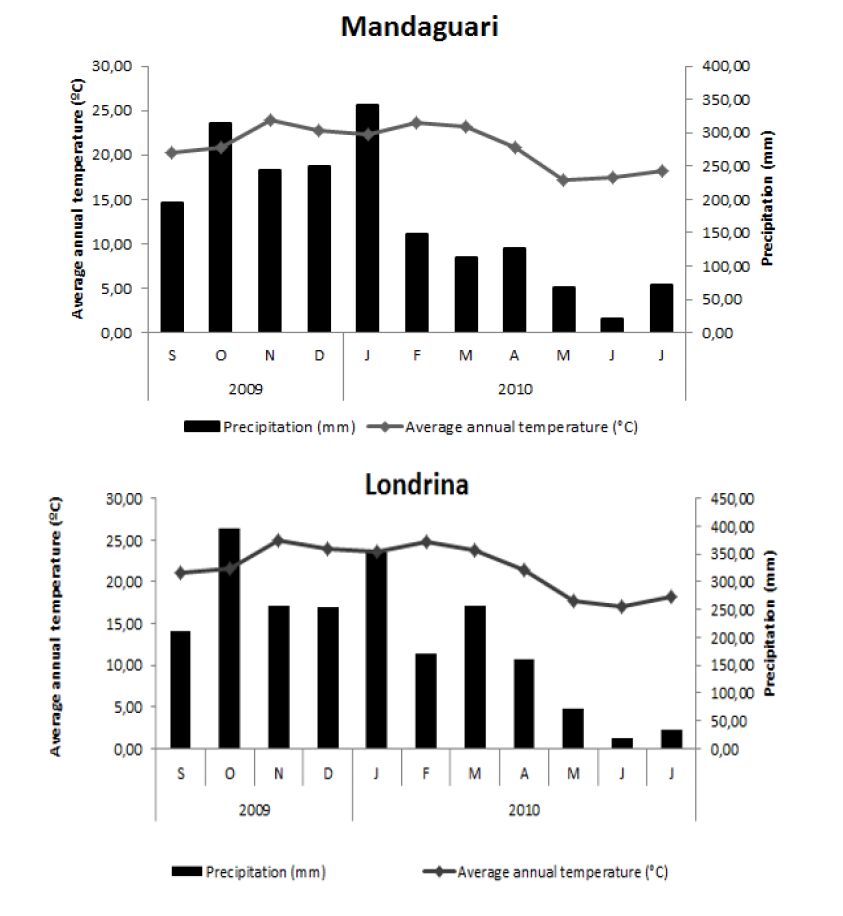
Coffee plants were cultivated in Mandaguari and Londrina, Paraná state, Brazil. The geographic locations and climatic conditions of the two locations are shown in Table 1. Figure 1 shows the climatic data for the development period of the coffee beans in each location.
Table 1. Geographic region, climatic variables and characterization of the Mandaguari and Londrina.
|
Local
|
|
Mandaguari |
Londrina
|
| Latitude |
23°32'52'' S |
23°18'36'' S
|
| Longitude |
51°40'15'' W |
51°09'56'' W
|
| Altitude |
650 m |
585 m
|
| Average annual temperature |
20-21 °C |
21-22 °C
|
In Mandaguari and Londrina regions, there were adequate conditions of pluviometric precipitation and temperature (September to January) these conditions at the period of development for the beans were favorable for the complete formation of the fruits (Figure 1 and Table 1). Lower pluviometric precipitation occurred when the fruits were already in the final phase of the cycle (May to July) and were dried, ripened fruit. This pluviometric precipitation in this last phase of the phenological cycle in the years of the experiment was suitable for fast drying of the fruit (Figure 1 and Table 1).
2.2. Coffee samples and coffee beverage
Cherry fruits from the following fourteen arabica coffee genotypes were harvested from May to July 2010, washed and sun-dried on a patio: Catuaí, Iapar 59, IPR 97, IPR 98, IPR 99, IPR 100, IPR 101, IPR 102, IPR 103, IPR 104, IPR 105, IPR 106, IPR 107 and IPR108. Samples were standardized in a sieve (6.5 mm), and defective beans were removed. Fruit ripening, post-harvesting and drying processes were standardized to avoid interference in the descriptions of the sensory attributes of coffee beverages [22]. The genetic origins of genotypes are described in Table 2.
Table 2. Genetic background of the arabica coffee genotypes.
| Arabica coffee genotypes |
Breeding
|
| Red Catuaí (IAC 81, register 02932)a |
Yellow Caturra (single mutation of Red Caturra) × Mundo Novo (hybridization of Red Bourbon and Sumatra)
|
| Iapar 59 (register 02324)a, IPR 97, IPR 98 (register 09950) a, IPR 99 (register 09949)a, IPR 104 |
Timor Hybrid × Villa Sarchi (Sarchimor)
|
| IPR 103 (register 09945)a |
Red Catuaí IAC 99 and Yellow IAC 66 × Icatu
|
| IPR 100, IPR 101, IPR 105 |
Catuaí Sh2, Sh3
|
| IPR 102 |
Catuaí and Icatu derived
|
| IPR 103 |
Icatu genetic background
|
| IPR 106 |
Icatu × Catuaí genetic background
|
| IPR 107 |
Sarchimor × Mundo Novo
|
| IPR 108 |
Sarchimor × Icatu × Catuaí
|
| a Registration number as Coffea arabica at the National Cultivar Registry (Registro Nacional de Cultivares—RNC) of the Ministry of Agriculture, Livestock and Supply (Ministério da Agricultura Pecuária e Abastecimento—MAPA), Brazil [13,15]. |
The coffee beans were subjected to a medium roasting process (Roaster Rod-bel, São Paulo), in which the beans were roasted for 8 to 11 minutes at 200 to 210 °C and achieved a mean weight loss of 14 ± 0.9%. The roasted coffee was then grounded (particles not exceeding 0.6 mm). A Minolta 400 colorimeter (Konica Minolta Sensing INC, Osaka, Japan) (CIE illuminant C, 10° angle and CIE standard observer) was used to assess colour characterization in the ground coffee. The coffees exhibited a lightness of 29.4 ± 1.5.
The beverages were prepared as described by Kitzberger et al. [23], with some modifications.To prepare the beverages, 1000 mL of boiling water (98-99 °C) was added to 70 g of roasted ground coffee. After 5 minutes of extraction, the mixture was filtered through filter paper.
The coffee was presented in plastic cups (50 mL) coded with three-digit numbers, at a temperature between 60 and 65 °C, without added sugar, in a day-light individual sensory booth in a tasting room. Mineral water and "cream cracker" biscuits were served to rinse the mouth and clean the palate between samples. Sample preparation, roasting and sensory analysis were conducted in the Physiology Laboratory of the IAPAR institute.
2.3. Recruitment and evaluation of coffee beverages
For the sensory analysis of coffees, we invited 13 and 16 participants/candidates to form the T1 and T2 teams, respectively. The coffee samples from Mandaguari and Londrina were analysed by T1 and T2, respectively. The candidates were between 21 and 47 years old; 60% had taken at least one college course, and all were regular consumers of coffee beverages (filtered, espresso or soluble).
The participants received information about the products and participated in testing procedures assessing their ability to recognize basic odours and tastes. The candidates with odour recognition scores above 70% who exhibited 100% accuracy in recognizing basic tastes were selected for the panel analysis [23]. The panellists were informed about the product and testing procedures and were presented the aims of the analysis and the free choice profiling technique, as described in the registered project in the Brazilian National Ethical Research System (Sistema Nacional de Ética em Pesquisa, Certificate n° 2054.0.000.268-11).
Because coffee has sensory complexity, and most of the panellists had no previous experience in sensory analysis, they were familiarized with the lifting technique attributes of the Network Method. In two preliminary sessions, the panellists were requested to cite terms to describe the appearance, aroma, taste and texture of food matrices (commercial orange juice and chocolate bars) to understand the analysis procedure and the definitions of the descriptors [23].
Subsequently, we performed a study of coffee beverage attributes by presenting a pair of coffee beverages and asked the panellists to describe the similarities and differences between these beverages. From this information, we prepared individual sensory sheets. After interviews between the team leader and each panellist, we developed an individual glossary with definitions for the specific attributes used by each panellist.
At each test session, four coffee samples were evaluated, and they were served once for each panellist. The repeatability test was performed with the IPR 99 and Iapar 59 coffees served in triplicate (over different sessions). The coffees were served in sequence in a randomized order of presentation for each panellist. At this time, the panellists received their sensory sheet and glossary. The sensory sheet was a range of 10 cm anchored at the ends with intensity expressions for the attributes [24]. Sensory evaluations were conducted in the Laboratory of Plant Physiology of Agronomic Institute of Paraná (Londrina, PR) in individual booths under white light. GPA was performed with XLStat version 2008.4.02 statistical software [25] to analyse the results.
3. Results and Discussion
3.1. Selection of panellists, development of the vocabulary of the attributes and evaluation of panel performance
From the candidates, we selected twelve panellists for the T1 team and fourteen panellists for the T2 team. The number of attributes raised by panellists in the two teams ranged from 11 to 21, and multiple panellists used some of the descriptive terms. For describing the appearances of the coffees, the most commonly used attributes were colour, turbidity and brightness, whereas for the texture, the most frequent descriptor used was full-bodied. Coffee, sweet, green and burnt aromas were cited by several panellists, as well as bitter, sweet, astringent, green and acid to describe the attributes of taste (Table 3).
Table 3. Attributes cited in the description of coffee beverages by T1 and T2 teams.
| Category |
Attribute |
N1 |
N2 |
Attribute |
N1 |
N2 |
Attribute |
N1 |
N2
|
| Appearance |
Coffee color |
12 |
14 |
Brightness |
7 |
9 |
Turbidity |
4 |
5
|
|
Transparence |
5 |
5 |
|
|
|
|
|
|
| Aroma |
Coffee |
11 |
13 |
Chocolate |
4 |
4 |
Honey |
1 |
1
|
|
Sweet |
10 |
13 |
Caramel |
2 |
1 |
Moldy |
1 |
−
|
|
Burnt/smoke |
10 |
11 |
Peanut |
2 |
2 |
Bitter |
1 |
1
|
|
Green |
11 |
13 |
Old |
2 |
2 |
|
|
|
|
Acid |
5 |
6 |
Fermented |
6 |
7 |
|
|
|
| Taste |
Bitter |
12 |
14 |
Fermented |
8 |
9 |
Peanut |
1 |
1
|
|
Sweet |
9 |
10 |
Burnt |
7 |
7 |
Coffee |
2 |
2
|
|
Astringent |
11 |
13 |
Chocolate |
3 |
1 |
Fruits |
1 |
1
|
|
Green |
11 |
13 |
Caramel |
4 |
4 |
|
|
|
|
Acid |
10 |
12 |
|
|
|
|
|
|
| Texture |
Full-bodied |
12 |
13 |
Watery |
3 |
3 |
Light |
1 |
1
|
| N1 = number of panelists of T1 team that used the attribute, N2 = number of panelists of T2 team that used the attributes. |
The data from the individual evaluations were used to calculate a GPA that is suitable for performing sensory analyses when the tasters have little or no training. By examining the analysis of variance from the GPA (PANOVA), we found that the greatest effects were due to translation (which corrects variations in the evaluation of attribute intensity) and scale transformation (which corrects variations associated with the use of different amplitudes), which was indicated and justified by the behaviour of the panellists who did not use the scale during training. The rotation effect correcting the different interpretations of the terms was not significant, indicating that the panellists agreed about the stimuli and the terms used for description. This behaviour was observed for both teams (T1 and T2) and was likely due to the orientation conducted to identify the attributes (Tables 4 and 5). These results allowed us to infer that there is no difference in the performance of T1 and T2; therefore, the differences between genotypes may be due to the cultivation area because the other factors were standardized.
Table 4. PANOVA for sensorial analysis of T1 team.
| Source |
DF |
SS |
MS |
F |
Pr > F
|
| Residuals after scaling transformation |
1606 |
4291 |
2.6 |
|
|
| Scaling transformation |
11 |
571 |
51.8 |
19.41 |
< 0.0001
|
| Residuals after rotation |
1617 |
4863 |
3 |
|
|
| Rotation |
2310 |
4014 |
1.7 |
0.65 |
1.000
|
| Residuals after translation |
3927 |
8877 |
2.3 |
|
|
| Translation |
231 |
18,065 |
78.2 |
29.26 |
< 0.0001
|
| Corrected total |
4158 |
26,943 |
6.5 |
|
|
| DF: Degrees of freedom, SS: Sum of squares, MS: Mean squares. |
Table 5. PANOVA for sensorial analysis of T2 team.
| Source |
DF |
SS |
MS |
F |
Pr > F
|
| Residuals after scaling transformation |
1898 |
8492,263 |
4474 |
|
|
| Scaling transformation |
13 |
989,275 |
76,098 |
17.01 |
< 0.0001
|
| Residuals after rotation |
1911 |
9481,538 |
4962 |
|
|
| Rotation |
2730 |
5716,598 |
2094 |
0.47 |
1.000
|
| Residuals after translation |
4641 |
15198,137 |
3275 |
|
|
| Translation |
273 |
17826,579 |
65,299 |
14.59 |
< 0.0001
|
| Corrected total |
4914 |
33024,716 |
6721 |
|
|
| DF: Degrees of freedom, SS: Sum of squares, MS: Mean squares. |
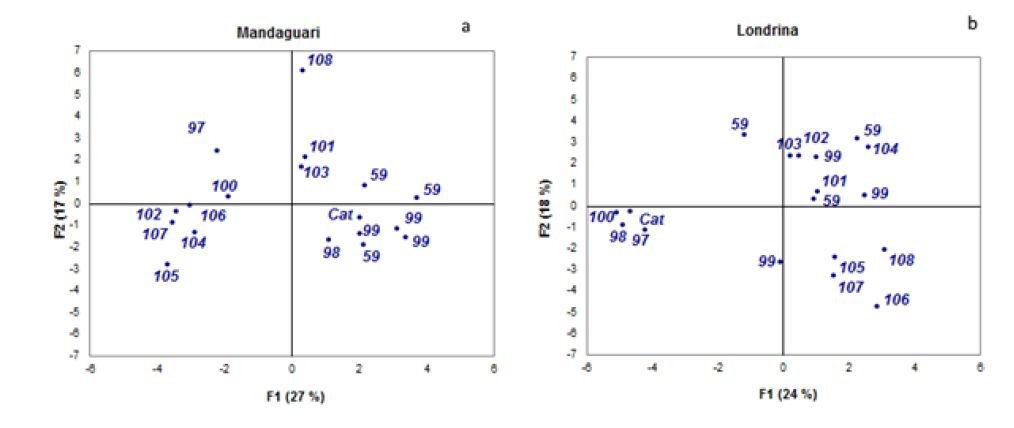
The triplicate repetitions of the IPR 99 and Iapar 59 coffees were used to evaluate the repeatability of the results and the teams' discrimination capabilities. The consensus configurations of these samples (Figure 2a and b) confirmed the consensus among the panellists of each team, which is shown by the proximity of the configurations of these samples.
3.2. Evaluation of coffee beverages
We observed good resolution in the dispersion of coffees in a two-dimensional solution (44 and 42% of Mandaguari and Londrina coffee explanations, respectively), as shown in Figure 2a and b. The remaining dimensions explained only a small proportion of the variance observed, which was very difficult to include in the interpretation of the results. The distribution of coffees in the consensus space (Figure 2a and b) is indicative of the differences among the coffees evaluated. The sensory description of coffee beverages located in each dimensions were interpreted associating the attributes closely correlated to dimension F1 and F2 (Table 6). For this association were considered only the attributes that presented correlation coefficient of greater than 0.30.
Table 6. Frequency of the attributes in the dimensions F1 and F2 and the signal corresponding to the dimension of the correlation.
|
T1 Mandaguari |
T2 Londrina
|
| Appearance |
F1+ |
F1− |
F2+ |
F2− |
F1+ |
F1− |
F2+ |
F2−
|
| Brightness |
0.34-0.76 (3) |
−0.32 to −0.48 (2) |
0.33 to 0.46 (3) |
−0.32 (1) |
0.67 to 0.68 (2) |
−0.34 to −0.70 (4) |
0.39 to 0.68 (4) |
−0.33 to −0.52 (3)
|
| Coffee color |
0.32 to 0.91 (5) |
−0.43 to −0.84 (4) |
0.33 to 0.55 (2) |
−0.34 to −0.77 (6) |
0.66 to 0.84 (3) |
−0.34 to −0.63 (7) |
0.43 to 0.72 (3) |
−0.47 (1)
|
| Turbidity |
0.30 to 0.40 (2) |
−0.39 to −0.42 (2) |
0.33 to 0.36 (2) |
−0.48 to −0.56 (2) |
0.75 (1) |
−0.47 (1) |
0.36 (1) |
−
|
| Transparence |
0.33 (1) |
−0.31 to −0.46 (2) |
0.48 to 0.82 (5) |
− |
0.33 (1) |
−0.35 (1) |
0.41 to 0.65 (2) |
−0.30 (2)
|
| Aroma |
|
|
|
|
|
|
|
|
| Acid |
0.39 to 0.63 (3) |
− |
− |
− |
0.69 (1) |
− |
− |
−0.39 to −0.40 (2)
|
| Coffee |
0.31 to 0.76 (4) |
−0.41 to −0.50 (3) |
0.49 (1) |
−0.48 to −0.60 (2) |
0.55 to 0.88 (3) |
−0.46 to −0.56 (2) |
0.31 to 0.69 (3) |
−0.38 to −0.52 (2)
|
| Chocolate |
0.35 to 0.59 (2) |
−0.55 (1) |
− |
−0.45 (1) |
0.64 (1) |
−0.47 (1) |
− |
−0.40 to −0.41 (2)
|
| Sweet |
0.32 to 0.66 (5) |
−0.33 to −0.44 (3) |
0.30 to 0.57 (4) |
− |
0.47 to 0.96 (3) |
−0.32 to −0.54 (3) |
0.39 to 0.71 (4) |
−0.40 to −0.48 (2)
|
| Fermented |
0.42 (1) |
−0.32 (1) |
0.66 to 0.74 (2) |
−0.36 to −0.41 (2) |
0.44 to 0.87 (2) |
−0.41 (1) |
0.42 (1) |
−0.37 to −0.39 (2)
|
| Smoke |
0.31 to 0.51 (2) |
−0.55 (1) |
0.46 (1) |
− |
0.54 (1) |
−0.40 to −0.58 (3) |
− |
−0.42 (1)
|
| Green |
0.80 (1) |
−0.31 to −0.60 (3) |
0.59 to 0.63 (2) |
−0.31 (1) |
0.32 to 0.66 (4) |
−0.36 to −0.48 (3) |
0.35 (1) |
−0.41 (1)
|
| Taste |
|
|
|
|
|
|
|
|
| Acid |
0.36 to 0.65 (2) |
−0.47 (1) |
0.49 to 0.76 (2) |
−0.49 (1) |
0.60 to 0.72 (3) |
−0.30 to −0.45 (2) |
0.30 to 0.73 (5) |
−0.32 to −0.51 (3)
|
| Astringence |
0.39 to 0.64 (2) |
−0.41 to −0.89 (2) |
0.39 to 0.57 (2) |
−0.33 to −0.70 (2) |
0.45 to 0.67 (3) |
−0.30 to −0.63 (5) |
0.33 to 0.46 (5) |
−0.32 to −0.62 (6)
|
| Bitter |
0.34 to 0.65 (4) |
−0.38 to −0.92 (3) |
0.41 to 0.44 (2) |
−0.38 to −0.86 (2) |
0.56 to 0.77 (3) |
−0.30 to −0.39 (4) |
0.31 to 0.76 (5) |
−0.49 (1)
|
| Sweet |
0.36 to 0.69 (7) |
−0.39 to 0.46 (3) |
0.41 to 0.65 (2) |
− |
0.46 to 0.78 (3) |
−0.31 to −0.33 (2) |
0.30 to 0.68 (4) |
−0.33 to −0.44 (2)
|
| Fermented |
− |
−0.40 to −0.51 (2) |
− |
−0.39 to −0.60 (2) |
0.40 (1) |
−0.30 to −0.45 (3) |
− |
−0.47 (1)
|
| Burnet |
0.31 to 0.61 (2) |
− |
− |
−0.67 (1) |
0.72 (1) |
−0.45 (1) |
0.34 to 0.41 (2) |
−
|
| Roasted |
0.55 (1) |
− |
− |
−0.41 to −0.44 (2) |
0.37 (1) |
−0.40 (1) |
− |
−
|
| Green |
0.44 (1) |
−031 to −0.68 (2) |
0.30 to 0.64 (4) |
−0.46 (1) |
0.48 to 0.50 (2) |
−0.31 to −0.69 (4) |
0.55 to 0.58 (2) |
−0.40 to −0.66 (2)
|
| Texture |
|
|
|
|
|
|
|
|
| Watery |
0.80 (1) |
−0.53 (1) |
0.30 to 0.57 (3) |
− |
− |
−0.63 (1) |
0.41 to 0.60 (2) |
−
|
| Body |
0.35 to 0.65 (3) |
− |
0.32 (1) |
−0.45 to −0.63 (3) |
0.34 to 0.73 (3) |
− |
0.42 to 0.45 (2) |
−0.30 (1)
|
| Full-bodied |
0.33 (1) |
− |
− |
−0.36 to −0.54 (3) |
0.58 (1) |
− |
0.40 (1) |
−0.37 (1)
|
| Sum of attributes |
53 |
36 |
38 |
31 |
43 |
49 |
46 |
34
|
The coffee colour, brightness and turbidity, which are appearance attributes dependent on the roasting process, were also perceived by the panellists and were associated in both F1+ and F1− (Table 6). This observation indicates that the conditions of roasting were similar for all coffees. These appearance attributes were easily evaluated by the panellists and were mentioned by a large number of panellists in the two dimensions, which indicates consensus among them (Table 6). In contrast, attributes such as an astringent and acid taste were also similarly correlated with F1+ and F1−. As these attributes are complex, making it difficult to reach a consensus among the panellists, these attributes were less efficient at discriminating coffees. The positive (F1+) and negative (F1−) dimensions were mainly responsible for the separation of coffees from Mandaguari. Coffees positioned in the F1+ dimension were mostly characterized by (1) the appearance attributes of colour and brightness; (2) the aroma attributes of acidity, coffee, sweetness and chocolate; (3) the taste attributes of acidity, bitterness, sweetness and a burnt flavour; and 4) the texture attribute of full-body.
Catuaí, Iapar 59, IPR 98, and IPR 99 formed this group and were described by the above attributes. With the exception of Catuaí and IPR 101 (Catuaí SH2SH3), all genotypes in F1+ had a C. canephora background (Sarchimor-Iapar 59, IPR 98 and IPR 99), Catuaí and Icatu backgrounds (IPR 103) and Sarchimor and Icatu × Catuaí backgrounds (IPR 108). Considering the genetic variability of these genotypes, we observed different sensory descriptions, promoting separation among genotypes. Interestingly, the same sensory characteristics grouped three coffees with highly similar genetic origins (Iapar 59, IPR 98 and IPR 99) with Catuaí, which is a cultivar with different genetic background.
Transparency, coffee colour, and a green aroma; a green, bitter, fermented and astringent taste; and a watery texture, were the principal attributes correlated with F1− (Figure 2a and Table 6). IPR 97, IPR 100, IPR 102, IPR 104, IPR 105, IPR 106 e IPR 107 showed that these attributes and were separated, forming a distinct group, regardless of their genetic background (Table 2).
In addition, the IPR 101, IPR 103 and IPR 108 genotypes with different genetic origins exhibited both F1+ and F1− sensory characteristics, suggesting that these attributes are not dependent on genetic background.
The same group (F1−) showed some attributes that are mainly associated with immature beans. Similar attributes were found in genotypes that were associated with growing conditions unfavourable to coffee plant physiological development. It is likely that the edaphoclimatic conditions in Mandaguari contributed to the occurrence of the desirable sensory attributes found in these genotypes in the F1+ group.
The attributes are negative to quality beverage when presented in high intensity causing disequilibrium in sensory quality. On the other hand, positive attributes are considered those that contribute to a greater appreciation of the beverage. In the present study, some genotypes (IPR 100, IPR 105 and IPR 106) showed negative and positive attributes (Figure 1a and Table 5). Similar behavior was also observed in the same genotypes evaluated in another study showed positive attributes, such as coffee colour, turbidity, and a sweet and chocolate aroma, as well as a sweet, bitter taste and a full-bodied texture [26]. Moreover, the IPR 102, IPR 104 and IPR 107 genotypes exhibited negative attributes (Figure 1a and Table 5) but were described by intermediate sensory characteristics, as mentioned in the previous studies [26].
The coffee genotypes undergo different physiological developments, reaching maturity at different times. IPR 97, IPR 104 and IPR 107 are semi-early ripening genotypes, IPR 100 is slow ripening, and IPR 102, IPR 105 and IPR 106 are very slow ripening genotypes [27]. The attributes that indicate incomplete maturation of these coffees may be due to improper harvesting times or the rapid development of fruit in semi-early maturing genotypes. Such climatic conditions were most likely unfavourable for the complete development of the sensory characteristics because of the low accumulation and lack of formation of the precursors of these sensory attributes.
Similar to Mandaguari, coffee grown in Londrina showed the formation of two groups, which were separated by dimension 1 positivity (F1+) and negativity (F1−). Catuaí, IPR 97, IPR 98 and IPR 100 genotypes formed the group on the left side of Figure 1b. The main attributes of this group were brightness, coffee colour, sweetness, green and a smoky aroma and astringency, acid, a fermented and green taste and a watery texture (Table 6). On the other hand, the attributes of turbidity; a green, coffee and sweet aroma; and an acid, astringent, bitter and sweet taste; and a full-bodied texture were used to describe the group formed by all of the remaining genotypes (F1+, Figure 2b).
3.3. Performance of genotypes at two locations
Agronomic and sensory performance in a specific location is an important factor for the coffee producer in choosing the plantation coffee genotype. In the present study, despite having similar local climatic and geographical characteristics (Table 1 and Figure 1), these parameters were sufficient to identify different attributes at each location. In both growing locations, genotypes with the same genetic origin (Iapar 59 and IPR 99) and different genetic backgrounds (IPR 101, IPR 103 and IPR 108) showed positive attributes, as shown in the F1+ dimension of Figure 2a and b. These results suggest that these genotypes presented good adaptability to climatic conditions in both locations. Interestingly, despite their different genetic origins, these genotypes are both an introgression of C. canephora, which is considered to be a more rustic coffee species than C. arabica, which may contribute to its improved adaptability.
The IPR 97 and IPR 100 genotypes presented negative attributes associated with immature beans in both locations. This most likely indicates that they are poorly adapted to these regions. Moreover, despite the fruits being visually mature (grain colour), they had not yet reached the degree of optimum ripeness for harvest. Therefore, coffee should be collected at the point of full maturation for the manifestation of positive attributes in the resulting brew.
In addition, the other genotypes in the F1 dimension showed different behaviours in both locations. The Catuaí and IPR 98 genotypes showed a greater number of positive attributes in Mandaguari than in Londrina. The IPR 102, IPR 104, IPR 105, IPR 106 and IPR 107 genotypes grown in Londrina showed a higher number of positive attributes than the same genotypes grown in Mandaguari.
The IPR 102, IPR 104 and IPR 108 genotypes were located within the F1 dimension but were differentiated in each location by the F2. In Mandaguari, IPR 102 and IPR 104 exhibited a coffee colour; turbidity; a coffee and fermented aroma; and an astringent, bitter, fermented and roasted taste, as well as full-bodied texture (F2−). In Londrina, these genotypes showed a coffee colour and brightness; a sweet, coffee and green aroma; and an acid, astringent, sweet, burnt and green taste, indicating more favourable attributes at this location (F2+). On the other hand, IPR 108 received better evaluations in Mandaguari than in Londrina.
It is also important to emphasize that the IPR 108 genotype grown in Mandaguari distanced itself from the group in the direction of F2+, mainly due to its brightness and transparency; its green and sweet aroma; its acid, astringent, bitter, sweet, and green taste; and its watery texture. Similar and different attributes were observed for this genotype when it was grown in different places of the coffee region in Paraná [28].
4. Conclusion
The standardization of the conditions of cultivation, harvesting, post-harvesting, roasting and beverage preparation allowed an association of the sensory attributes of individual coffee cultivars with the genetic origin of the genotypes evaluated.
Among the many attributes identified through the free choice profiling sensory technique, brightness, coffee colour and turbidity; a coffee, sweet and green aroma; an acid, astringent, bitter, sweet and green taste; and a full-bodied texture were the attributes that had greater contributions to descriptions of coffee.
In addition, we also found that a coffee plant containing various cultivars must take into account the optimal harvesting time for each cultivar so as not to affect coffee crop quality due to early or late maturation.
Sensory evaluation allowed us to not only identify the genotypes with positive or negative attributes in both locations but also to identify the genotypes that have different attributes in these two locations. These behaviours suggest a different adaptation for each genotype in each location. This information is important in helping breeders select the best-adapted genotypes in each region and in educating coffee producers on the best genotype for a specific region.
Finally, we note that edaphoclimatic growing conditions influence the sensory profiles of genotypes, independent of their genetic background. This fact shows the importance of performing adaptation studies of each genotype in each production location to obtain the maximum expression of the sensory potential of each new genotype. We also determined that some genotypes did not show good performance in both locations studied, suggesting that new genotypes should be evaluated in different edaphoclimatic conditions to meet the growing conditions for the best expression of their positive sensory attributes.
Conflict of Interest
All authors declare no conflicts of interest in this paper.









 DownLoad:
DownLoad: