1. Introduction
In the U.S. the major feedstocks for biofuel production come from agricultural commodity crops, which have been historically grown as food and animal feed products (corn-grain based ethanol and soybean oil based biodiesel) [1]. This has spurred a debate, known commonly as Food vs. Fuel, over the sustainability of using these crops for energy production. One option for bioenergy production that provides an alternative to use of these commodity crops are lignocellulosic sources, including dedicated biomass crops, residues, and waste products (both forestry and agricultural products). A revised set of renewable fuel standards was set in EISA 2007 [2] that call for the production of 102.21 billion liters per year (27 billion gallons per year) by 2022 with 60.56 billion liters per year (16 billion gallons per year) coming from lignocellulosic sources [3]. To date, the production of biofuels from lignocellulosic sources is constrained to a handful of second generation facilities utilizing corn stover as a feedstock in the Midwest.
Since limited data was available on a national scale to determine the possibility of reaching these lignocellulosic feedstock goals the 2011 Billion Ton Update was conducted, an update to the 2005 Billion Ton Study [4,3]. Under the baseline scenario using a $66.14 per dry tonne purchase price ($60 per dry ton), models found that by 2022 dedicated energy crops would account for 33.72% of the 546.13 million dry tonnes (602 million dry tons) of potential feedstock sources [3]. Using the high yield scenario this would increase to between 47.95% and 55.90% of potential resources, or 371.95 to 511.65 million dry tonnes nationally (410 to 564 million dry tons) [3]. Dedicated energy crop classifications are based on herbaceous or woody biomass materials grown under intensive management practices specifically to produce energy.
Conversion of lignocellulosic feedstocks to biofuels is an area still under development and optimization, and is listed as one of the seven board action areas by the Biomass Research and Development Board [5]. Due to the infancy of the technologies and economies of scale it is currently not feasible to consider conversion of lignocellulosic feedstocks directly to biofuels at farm scale, thus requiring logistical operations to accommodate production of material for an off-site biorefinery, a facility producing products from renewable biological sources through conversion processes. “Economies of scale” refers to the general idea that as total output of an industrial operation increases, the price per unit decreases [6]. As the size of a biorefinery, increases, the non-feedstock costs decrease while feedstock costs increase as a result of transportation and handling costs [7]. Using a ten percent cropland inclusion assumption for corn stover (Zea mays L.) as a feedstock, the U.S. National Renewable Energy Laboratory (NREL) found a corn stover biorefinery located in Iowa below 2000 dry tonnes per day of feedstock (1814.4 dry tons/day) (approx.: $0.36/liter or $1.38/gallon minimum ethanol sales price) is cost prohibitive primarily because of non-feedstock costs, yet a plant above 10,000 dry tonnes per day (approx.: $0.35/liter or $1.32/gallon) loses out on cost savings by expanded scale related to increased transportation costs [7]. A reduction in biomass logistics costs can potentially have major impacts on the minimum biofuel (e.g. ethanol) sales price, necessary to increase profitability of a biorefinery.
Biomass logistics encompasses operations from the harvest of lignocellulosic feedstocks to the point of feeding the material into the throat of the biorefinery. Additionally, logistics is listed by the Biomass Research and Development Board as an action area [5]. Some of the operations of biomass logistics include: harvest & collection, storage, transportation & handling, and pre-processing. Depending on the specific feedstock, biorefinery operations, and region among other site specific characteristics, different logistic operations may be warranted. This may mean specialized harvest operations, densification of feedstocks, pre-processing of the feedstock prior to arrival at the biorefinery, or an array of other options. The most important aspect of these systems is that a uniform feedstock is delivered on a regular schedule to the biorefinery at the lowest cost possible. In most cases the biomass logistics system will change most readily based on the feedstock being utilized by the biorefinery.
A wide range of parameters including feedstock types, transportation methods, modeling boundaries, and research scopes have been used to model biomass logistics chains. Resop et al. [8] used a geographic information system to set uniformly spaced storage sites to determine the least cost storage and transportation costs for baled perennial grasses. This was then improved by Judd et al. [9] using a solution to a traveling salesmen problem to optimally set storage location in an effort to optimize mobile processing and handling equipment. The Integrated Biomass Supply Analysis and Logistics (IBSAL) model was created to investigate the entire feedstock logistics chain from seed to biorefinery [10]. Less computationally intensive modeling of these logistics chains have been developed with focus on feedstock characteristics more than optimization of logistics chains, such as Worley & Cundiff [11]. The Uniform Format Bioenergy Feedstock Supply System was designed by the U.S. DOE to handle biomass feedstocks similarly to agricultural commodity crops [12], using existing logistical infrastructure and operations. Similar to the IBSAL model, researchers at the University of Illinois at Urbana-Champaign have developed the BioFeed model that is directly targeted for the state of Illinois [13,14]. Simulation models have been developed to treat biomass feedstocks similar to existing agricultural crops such as: cotton [15], sugarcane [16], and wood products [17]. Even multi-echelon modeling approaches have been implemented to model the complex biomass logistics chains for bioenergy production [18]. Each of these system models optimize their formats generally by cost and for a particular portion of the bioenergy production pathway rather than the entire system.
The objective of this analysis was to construct a model capable of preliminary siting a potential biorefinery, determining cropland conversion to biomass feedstocks, estimating required price to meet plant needs and comparing harvest system operations. A case study was completed for three potential biorefinery sites in North Carolina representing the three major regions of the state: Mountains, Piedmont, and Coastal Plain. While other studies on logistical operations focus on a particular feedstock and harvest operation(s) [8,9,10,11,12], the approach presented in this manuscript is unique in that it incorporates the combination of multiple feedstocks that are dedicated herbaceous crops (two) and multiple harvest systems that may be implemented concurrently, allowing for increased equipment utilization and adoption of biomass feedstocks into existing agricultural crop rotations in the Southeast. This work presents a model that describes the interrelationships of a biomass feedstock logistical network that incorporates the concept of supply-driven production.
2. Biomass logistics operations
2.1. Computational analysis
Model development for harvest systems was performed in the Matlab environment (Mathworks: Natick, MA) utilizing the Matlog logistical toolset developed at North Carolina State University by Kay [19]. Data from ArcGIS (ESRI: Redlands, CA) was transferred to Matlab for analysis as a comma delineated text file, which was converted to a structure for use in Matlab.
2.2. Heuristic biorefinery siting
Potential locations for biorefinery facilities were determined using a heuristic to minimize the number of sites for analysis in the developed model (Table 1). Since a biorefinery is an industrial operation requiring extensive infrastructure locating a facility near a medium sized city would be beneficial. The city would need to be large enough to provide infrastructure requirements of the facility but small enough to reduce nuisance issues related to logistical operations, such as increased tractor trailer traffic, loose biomass material appearing on roadsides, and twenty-four hour operations of the supply chain. Density of agricultural land is an important parameter to consider within a given radius of the facility, especially since the closer the feedstocks are produced the lower the logistical complexity and costs. This is related to both feedstock availability and community acceptance of agricultural operations. Finally it is important to consider characteristics of the potential area for available workforce and to place facilities within an economically struggling rural communities. Siting a facility in these areas can lead to additional government and community support of the potential biorefinery, as well as ensuring that adequate personnel trained in the required fields are available for operations. The simplified heuristic shown in Table 1 served as a preliminary method for biorefinery location selection. More detailed analysis of feedstock availability and logistical operations would be required for definitive siting decisions.
Table 1. Biorefinery siting heuristic parameters.
| Min | Max | Parameters | Source |
| City Size | 10k | 50k | Population | USDA (201) |
| Natural Land | 25% | 100% | Cultivated Crops, Hay/Pasture, Shrub/Scrub, Barren Land, Herbaceous | U.S. DOI (2014) (within 80.47 kg (50 mi)) |
| Regional Data | Multiple | Multiple | Employment Status, Occupation, Poverty | U.S. BOC (2014) (county specific) |
2.3. Feedstock & Harvest system selection
The feedstock supply chain for a biorefinery includes complex interconnected operations combining feedstock production, biomass logistics, and biorefinery facility operations. To reduce complexity of these systems a plantation style production system may be employed, where the biorefinery would operate large farms surrounding the biorefinery or specified storage locations. This would require large land and equipment investments, while diverting focus from conversion facility optimization. It is more likely that the conversion facility would contract local land managers to produce feedstocks, allowing individuals knowledgeable in field operations and owning agricultural equipment to focus on feedstock production. This shift in production system while advantageous to biorefinery operations would create a disaggregated set of fields that would complicate biomass logistics operations required.
Complexity of the logistics system would shift focus overly from conversion facility operations, which may necessitate the inclusion of a third entity to optimize logistical operations. Use of the term biomass logistics in this case would include harvest, storage, and transportation operations spanning from standing biomass feedstocks to delivery at the biorefinery. This scenario creates a three party system of land managers, biomass logistics operations, and the conversion facility allowing each to include experts in the individual areas that can optimize integration of system operations.
Examination of North Carolina biomass crops most suited for adoption by landowners found a combination of Switchgrass (Panicum virgatum L.) and Sorghum (Sorghum bicolor (L.) Moench) production to be profitable [20]. Switchgrass can be harvested and stored annually from the same field, while sorghum can be integrated with other annual cash crops on a multi-year crop rotation. As a winter annual with sorghum, rapeseed (Brassica napus L.) was included as a food and feed product, though other winter annuals are possible. Though currently no commercial scale conversion facility exists that can handle multiple feedstocks the current trend of biorefinery research is focused on use of multiple feedstocks to produce a range of value added products [21]. The inclusion of multiple feedstocks into this biomass logistics model supports the direction of creating a bio-based economy that will require a diverse biomass supply chain for sustainable operations.
Canola requires harvest with a combine and harvesting sorghum with a forage chopper was assumed to be a best management practice for reduced labor and time in the field as well as use of common agricultural equipment. Switchgrass on the other hand can be harvested in round bale, square bale, or a forage chopper with a pickup head either in the fall or winter. It is possible to use a multi-harvest system for switchgrass extending the harvest period, but it may increase cost significantly. Using average feedstock production values for Duplin County as a representative of the Coastal Plain region [20], a comparison of harvest systems can be made (Table 2). In-field transportation included in-field bale aggregation, loading, and short haul transportation of bales on secondary roads to a storage site. Bales were collected in-field with a telehandler capable of handling four bales simultaneously (4 bales collected and loaded every 15 minutes) then loaded onto a sixteen bale mover (maximum distance: 16.1 km (10 mi), maximum speed: 88.5 km/h (55 mph)) with sidewalls to facilitate rapid bale stabilization. The top speed and travel distance were used to calculate cost, with the assumption that shorter travel routes would account for lower travel speeds. Storage was modeled for outdoor crushed gravel pads covered with tarpaulins to reduce cost and dry matter losses, with tarpaulins and gravel pads found to be slightly higher in dry matter loss than indoor storage, but considerably lower than uncovered outdoor [22] (Outdoor Round Bale: 14% dry matter loss, Indoor Square Bale: 4.25% dry matter loss). Transportation combined loading of bales, travel for 80
.47 kilometers (50 miles), and unloading at the conversion facility, with a telehandler used for bales and a truck dump for forage chopped material.
Table 2. Comparison of Biomass Harvest Systems for Duplin County, NC (Coastal Plain).
| | Fixed | Harvest | Field Transport | Storage | Transportation | Final | Unit |
| $/ha | $/ha | $/ha | $/ha/yr | $/ha 80.47 km | $/ha | $/dry tonne |
| ($/ac) | ($/ac) | ($/ac) | ($/ac/yr) | ($/ac 50 mi) | ($/ac) | ($/dry?on) |
| Switchgrass | | | | | | | |
| Round Bale | $334 | $73.52 | $108.55 | $1.21 | $314.24 | $831.11 | $46.35 |
| ($135) | ($29.75) | ($43.93) | ($0.49) | ($127.17) | ($336.34) | ($42.05) |
| Square Bale | $334 | $81.12 | $90.05 | $40.45 | $226.18 | $771.39 | $38.44 |
| ($135) | ($32.83) | ($36.44) | ($16.37) | ($91.53) | ($312.17) | ($34.87) |
| Forage(fall) | $334 | $129.04 | | | $203.07 | $665.70 | $31.76 |
| ($135) | ($52.22) | | | ($82.18) | ($269.40) | ($28.81) |
| Forage (winter) | $334 | $129.04 | | | $147.08 | $608.87 | $29.09 |
| ($135) | ($52.22) | | | ($59.52) | ($246.40) | ($26.39) |
| Sorghum | $460 | $130.50 | | | $173.02 | $763.14 | $38.00 |
| ($186) | ($52.81) | | | ($70.02) | ($308.83) | ($34.47) |
Values shown in Table 2 were calculated using existing equipment cost information from Lazarus [23] and Lazarus [24], who used field capacity and equipment information from major commodity crop production in Minnesota, with the intent of modeling costs for the nth field. Since to date, North Carolina does not have a publically available field data set for equipment estimates representative of the spatial diversity of the region the Minnesota data was used. This data was considered adequate for the needed equipment cost estimations because of the thoroughness and public availability of the dataset and similarities in field efficiencies related to topographical variations in both states, allowing for replication and verification by others. The values presented in Table 2 are conservative because they do not account for additional harvest equipment wear and throughput related to the high dry matter yields of biomass crops, or the value of cropland, or the value of cropland. The traditional agricultural product enterprise budgets used for price comparisons did not incorporate land value, which would have raised per hectare costs by $207.57 annually for non-irrigated cropland in North Carolina ($84/acre) [25].
Though sorghum shows a higher production cost than some of the other systems the inclusion of a winter annual crop would decrease the unit price of sorghum to $30.91 per dry tonne ($28.04 per dry ton) [26], at a $302.77 per tonne canola price ($8.24 per bushel). Additionally this would allow the inclusion of an annual summer crop into other cash crop rotations, increasing land availability while reducing initial land manager investment. This demonstrates that the lowest cost system would include forage chopping switchgrass in the winter and sorghum in the fall, moving the supply chain closer to a “supply-driven production” system, and producing big square bales over the same time period for storage to allow year round supply availability. The term “supply-driven production” relates to a system dominated by high cost of carrying raw materials inventory compared to finished product inventory [27], which differs from “just-in-time” operations typically referring to a situation where the customer initiates or pulls production. When storage is removed from the values calculated in Table 2 square bales continue to be more profitable than round bales, and forage chopping operations were the most profitable. Similar findings were observed when transportation and the combination of both transportation and storage were excluded. Ensiled storage was not included because it would increase the cost of forage chopped switchgrass by 63.2% and 69.1% ($51.83 and $49.19 per dry tonne) for fall and winter harvests respectively, and sorghum by 55.1% ($58.95 per dry tonne), using transportation and filling/packing values from Benson et al. [28]. These additional costs may be worthwhile in conversion processing; however, the boundaries of the model ended at the biorefinery throat and do not capture the potential benefits of ensiled storage.
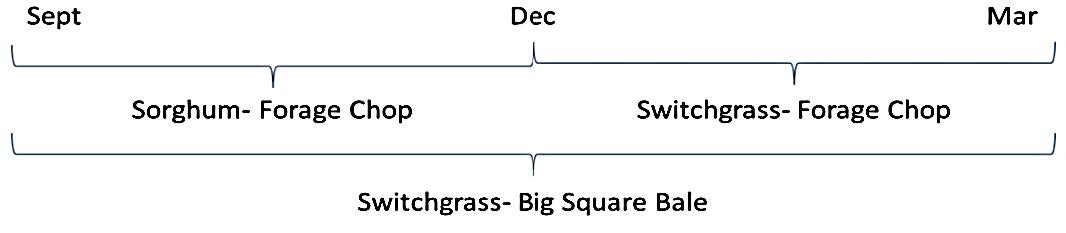
A set of harvest systems was constructed with several goals in mind: 1) reduce safety stocks, 2)increase utilization of capital intensive specialized equipment and 3) increase supply-driven production operations (Figure 1). The same fleet of self-propelled forage choppers could be used for both sorghum and forage chopped switchgrass, just changing the pickup head between crops to provide biomass for immediate use at the biorefinery. Baling specific fields within a given harvest window (Figure 1) will create a safety stock of bales during supply-driven production operations and create a stock of bales to be used for the following six months to ensure year round operation of the conversion facility. Generally, two weeks to one month maintenance down time is incorporated for similar large industrial facilities, which can be scheduled prior to the harvest period corresponding to the lowest quantity of stored biomass. The storage sites would be emptied on a first in, first out method, creating a uniform storage time of approximately six months for all bales and ensuring uniform removal operations at storage sites.
Though the harvest periods shown in Figure 1 may consist of multiple months the actual working days are significantly lower, depending on weather and soil moisture levels. Using a probabilistic method accounting for weather and soil moisture across representative Oklahoma counties, Hwang et al. [29] determined the number of working days for mowing and baling switchgrass (Table 3). Depending on moisture content of the standing crop, baling may occur directly after mowing without the need for any additional field curing. In North Carolina well drained sandy soils in the Coastal Plain may allow additional working days, while the poorly drained clay soils of the Piedmont may reducing working days. For this analysis temporal conditions of equipment use were not taken into account, though determination of this data for North Carolina could be used to determine the least number of pieces of equipment required for operations.
Table 3. Working Days for Mowing and Baling of Switchgrass across representative Oklahoma Counties [29] (95% probability level).
| | Total Days | Mowing | | Baling | |
| | Low | High | Low | High |
| Sept-Nov | 91 | 42 | 55 | 38 | 57 |
| | (46%) | (60%) | (42%) | (63%) |
| Dec-Feb | 901 | 23 | 31 | 31 | 55 |
| | (26%) | (34%) | (34%) | (61%) |
| 1Common year, non-leap year |
Inclusion of barley (Hordeum vulgare L.), in place of canola on sorghum fields, would allow utilization of the big square balers during barley harvest, May through June, for straw that can serve as an additional lignocellulosic feedstock. This may increase supply-driven production operations, further reducing storage requirements. As a result of the high cost of barley production [20] and low primary product value it was not included, though further analysis and system optimization may increase profitability and potential incorporation into this harvest system model with sorghum.
2.4. Prediction of cropland conversion to biomass feedstocks
A probabilistic profit based cropland conversion method was used to determine potential biomass feedstock production locations (see Caffrey et al. [20] for methodology). Yield data was used for the county where the potential conversion facilities were located, using North Carolina realistic yield expectations [30] for average and standard deviation values across soil types. Potential feedstock production fields were determined using a fifty mile radius from possible conversion facilities (based on the centroid of each field to facility location) using NASS cropland cover data[31] in ArcGIS. After using a normal random variable to determine yield variation in each field a uniform random variable was used to determine if a field converted to biomass feedstock production, after using the prescribed probabilistic equation in Caffrey et al. [20] for conversion probability.
Total feedstock requirements were determined for a 75.71 million liter cellulosic ethanol facility (20 million gallon) that uses a 354.68 liter per dry tonne conversion rate (85 gallon per dry ton) [3], or approximately 585.13 dry tonnes per day (645 dry tons). Safety stock included yield variability and storage losses, with other safety stock issues being alleviated by feedstock harvest schedule (Figure 1). A price of $33.07 per dry tonne ($30 per dry ton) was initially used, and if available feedstock did not equal the requirements for each of the categories then the price was raised by $1.10 per dry tonne ($1 per dry ton) until enough feedstock was available. This method accounted for profit above breakeven costs for both existing agricultural products and biomass feedstocks; though this did not represent the actual price that would most likely be paid by the conversion facility for the feedstock, which would need to go through contract agreements and localized production cost determination. These selected farm sites were used as the potential feedstock production locations.
Site selection criteria followed the highest cost transportation method first until feedstock requirements were filled, followed by the following crop and harvest group. This means that if forage chopped sorghum was the most expensive to transport on a $ per dry tonne-kilometer basis, feedstock production sites closest to the conversion facility would be allocated to sorghum production until annual tonnage requirements were fulfilled, then the next most expensive transportation options would be evaluated for remaining potential sites. Only a single year of cropland cover was used for this analysis to determine conversion related to profitability, so the multi-year nature of perennial grasses (i.e., switchgrass) would need to be evaluated over multiple years to determine if a given land parcel would convert to production. Use of a single year to determine profitability was deemed adequate for this analysis since the objective was to demonstrate use of the proposed logistical system and not to determine specific production locations. Since specific quantities of feedstock are required for each harvest and feedstock combination, a defined area was required. This method determined which potential feedstock and harvest combination would be most appropriate at each location, choosing feedstock order by transportation cost in $ per dry tonne-kilometer.
2.5. Biomass storage operations
Storage site location configurations can be split into three categories: centralized, dissipated, and decentralized. Centralized would require all material to be stored at a central storage location for use at the conversion facility, most likely near the facility. For the six months of feedstock required to operate outside the harvest range (Figure 1), excluding safety stock, approximately 130,000drytonnes of feedstock would be required (118,000 dry tons) for the assumed facility size. If each 0.9×0.9 × 2.3 m (3× 3 × 7.5 ft) big square bale of switchgrass weighed 340 kilograms (750 pounds) at 15% moisture content (wet basis) that would mean storage of around 370,000 bales, or almost 708 thousand m3 (25 million ft3). For a 9.1 meter (30 foot) tall stack (10 bales) this would require approximately 7.7 hectares (19 acres), or closer to 16.2 hectares (40 acres) accounting for lanes for use in handling and as fire breaks. The large requirement of contiguous land area for the centralized storage method makes it unreasonable. Multiple smaller storage yards would allow use of marginal or low value land areas and reduce the risk of a single localized catastrophic event impacting stored biomass material. Additionally a single storage location with one entry point would require increased handling requirements as stored material is removed or movement of the staging area which leads to additional travel distance across the storage yard.
A dissipated storage location method would require each individual field to have a co-located storage yard for harvested feedstocks. For a 4.05 hectare (10 acre) field at 17.93 tonnes per hectare yield (8 dry ton per acre) a storage area for 250 bales would be approximately 93 square meters (1000 square feet). Though this is a reasonable storage area, a proper site in each individual field may be difficult to locate, and annual payments to the land manager for use of that land may need to be made. If all fields were 4.05 hectares (10 acres) there would be around 1500 storage sites that would need to be managed and a proper transportation network maintained. This would greatly increase the complexity of the supply chain and require many partial loads to either be shipped to the conversion facility or taken to the next storage location to complete the load.
A combination of these methods would be the decentralized system where multiple storage sites are produced in a wheel and spoke system to store material from multiple farms. This would reduce the size of each of the storage sites and reduce the complexity of the logistics network. An alternative location allocation heuristic was utilized to determine the location of ten optimally located storage yards for the developed model. The use of ten decentralized storage locations (size: 1.64 hectares (4acres)) was assumed for the proposed decentralized wheel and spoke system to reduce risk and bale transportation from farms. It was assumed that these sites would use a storage method that involved crushed gravel to allow drainage and use of tarpaulins to reduce weathering and minimize total storage cost and dry matter loss [22].
2.6. Model limitations, assumptions and parameter sensitivity
Inherently computational models have certain limitations and results of analysis can be sensitive to values used for individual parameters. This specific model presented was constructed to demonstrate a novel approach for harvest, storage and logistical operations of biomass feedstock production for use at a centralized facility. As described, the model is intended to be a proof of concept of the biomass supply chain system and to demonstrate the feasibility of the modeling approach as a reasonable decision making tool for biomass production and delivery. Several assumptions were made in the model to highlight the functionality of the modeling approach. As such the model can effectively be used to evaluate logistic scenarios and should not be used in its current form as a means to determine specific price points or make absolute comparisons for system selection. More details concerning the assumptions, limitations and sensitivities of the model are described below:
Heuristic Biorefinery Siting: This simplified heuristic took two primary parameters, city population and natural land density. A secondary parameter that was taken into account was regional data, which could be used to determine available workforce and potential incentives to be garnered from a specific site. This heuristic limits potential biorefinery locations to those within the set parameter range, removing potential for construction of a facility in rural areas or in large industrial complexes. This method also doesn’t consider retrofitting an existing facility, such as a stalled fermentation operation or an aging coal power plant.
Feedstock & Harvest System Selection: An analysis of potential feedstocks and harvesting systems was used to determine the lowest cost production systems. This method used existing published equipment costs, standard feedstock production practices and field specific yield estimates. These cost calculations were used to determine which feedstocks and harvest systems were most economical, so any alteration in the production cost estimates would affect the selected systems. Only current production systems were considered in this analysis, without the inclusion of any assumptions for novel production practices or future improvements. The specific harvest windows related to each feedstock and harvest system assumed centralized planning of cultivars, planting dates, large equipment usage and harvest scheduling to ensure adequate supply annually.
Prediction of Cropland Conversion to Biomass Feedstocks: A detailed description of the modeling approach and parameter sensitivity of this predictive model is discussed in Caffrey et al.[20]. An assumed biorefinery capacity, conversion rate and safety stock requirements were established. Alteration of these parameters would necessitate additional feedstock production locations, possibly changing the optimized storage locations. An original feedstock cost was used to determine available low cost production sites, which was then increased until adequate feedstock was available for facility operation. Regardless of actual feedstock purchase price this method was able to determine the lowest cost feedstock production sites, using the production cost parameters used in this model.
Biomass Storage Operations: The assumed biorefinery facility size dictated the required number of bales for storage. Assumptions were also made as to the weight, moisture content, bale stack dimensions and handling/safety requirements, all having a direct effect on the storage space required. Both centralized and dissipated storage configurations were dismissed as unrealistic without any detailed analysis. An assumed ten decentralized storage sites, approximately 4 acres each, were assessed to create a wheel and spoke logistical operation. No optimization algorithm was performed to determine the optimal number and size of storage sites, which would likely not be uniformly sized.
3. Case study for regions of North Carolina
3.1. Biorefinery siting heuristic
For this analysis three cities were chosen across North Carolina to represent the three major regions of the state: Lenoir (Mountains), Sanford (Piedmont), and Kinston (Coastal Plain (Table 4). These regions represent different cropland densities and current agricultural crop production, both of which may influence selection of prospective fields for biomass feedstock production, and subsequently the supply chain configuration.
Table 4. Selected North Carolina Biorefinery Location Site Parameters [44,45].
| | Kinston (Coastal Plain) | Sanford (Piedmont) | Lenoir (Mountains) |
| City Size (population) | 21,677 | 28,094 | 18,228 |
| Natural Land Density | 45.6% | 34.1% | 25.9% |
| Economically Depressed County (DP3) |
| Employment Status | | | |
| In Labor Force (VC05) | 60% | 60.5% | 65.6% |
| Unemployed (VC13) | 9.1% | 10.5% | 9.8% |
| Occupation | | | |
| NatResources/Construction/Maintenance (VC44) | 13.9% | 12.3% | 11.5% |
| Production/Transport/Handling (VC45) | 19.3% | 27.4% | 22.9% |
| Industry | | | |
| Ag/Forestry/Fish&Hunt/Mining (VC50) | 3.5% | 1.1% | 1.5% |
| Construction (VC51) | 7.9% | 7.5% | 8.1% |
| Manufacturing (VC52) | 17.6% | 27.7% | 26.6% |
| Transport/Warehouse/Utilities (VC55) | 3.5% | 5.2% | 3.4% |
| Income and Benefits | | | |
| < $10,000 (VC75) | 13.6% | 9.3% | 8.9% |
| $10,000 to $14,999 (VC76) | 9.3% | 9.5% | 6.1% |
| $15,000 to $24,999 (VC77) | 14.5% | 14.6% | 12.7% |
| $25,000 to $34,999 (VC78) | 14.7% | 14% | 11.3% |
| $35,000 to $49,999 (VC79) | 15% | 14.5% | 16.5% |
| Families/People Below Poverty Line (VC156) | 18% | 12% | 11.6% |
3.2. Cropland conversion to biomass feedstocks & storage operations
All of the potential biorefinery locations had a large number of fields greater than one acre within the 80.47 kilometer transportation radius (50 mile), using great-circle distance (130 to 180 thousand fields for each of the selected sites). Great-circle distances were used in the model because they provide very good estimates of actual road distances in the type of agricultural regions where feedstocks are grown, namely, regions characterized by having the majority of distances greater than 50 miles and being a contiguous region without large restricted areas of travel [46]. From analysis in Caffrey et al. [20], it was decided to reduce the cropland cover areas of interest to those currently in corn, grain sorghum, winter wheat/soybeans, hay, fallow, and grasslands. Enterprise budgets for each of these were used to determine profitability [31,32,33,34,35,36,37,38,39,40,41], including average realistic yield expectations[29] for each county with a normal random variable to account for variability among soil types. Using a uniform random number generator, if the probability function [20] was less than the random number generated, the field was likely to convert to production of a biomass feedstock.
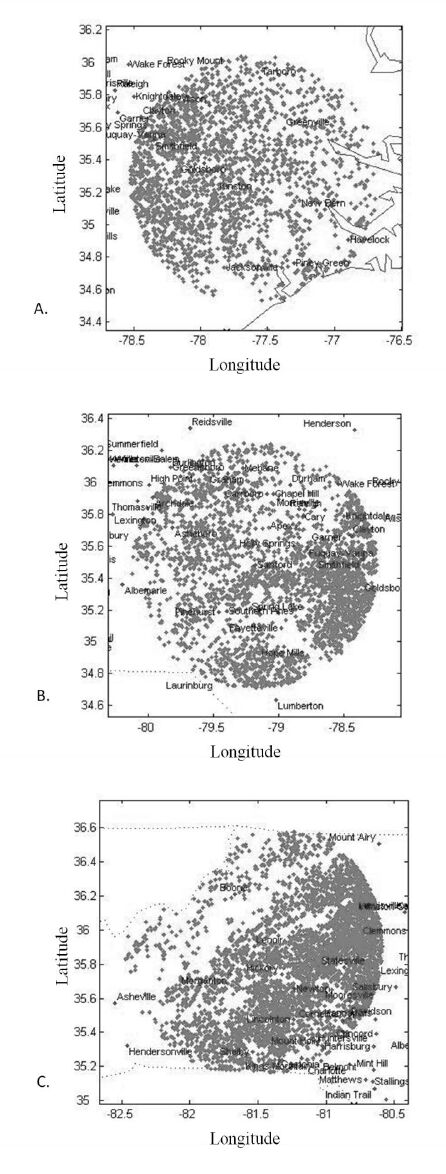
All of the potential sites showed adequate feedstock quantity at a purchase price of $33.07 per dry tonne ($30 per dry ton), using the probabilistic function developed by Caffrey et al. [20]. This value was an estimate of the breakeven production costs using a cropland conversion probability function and should not be considered an actual purchase price for biomass feedstocks. The estimate, for all intents and purposes, would be considered a minimal price for grower participation. For purposes of this model these selected fields were analyzed, assuming that regardless of purchase price these would be the most likely to convert to biomass production. This is partially because with the large number of fields, inclusion of a one percent inclusion rate for regardless of profitability (even if current crops are greater), and low value assigned for hay and grassland production, the model may be overestimating available field acreage at the given price point. What the value does offer is an idea of how the biomass production sites would be located and provides a method for simplifying logistical operations within the modeled supply-driven production system. This approach also reduced the number of potential sites from the initial number of sites in the 80.47 kilometer radius (50 mile) (which was over one hundred thousand for each potential biorefinery location) to between 2400 and 5100 (Figure 2). The coastal plain region (Kinston) showed the least number of potential sites, while the mountains (Lenoir) showed the highest. This observation may be related to the number of initial sites, size of each field, and profitability of the current agricultural products.
Converting feedstock transportation cost to $ per dry tonne-kilometer from $ per hectare (Figure 2), and removing loading and unloading, the total cost of sorghum, switchgrass forage, and switchgrass square bales was $0.10, $0.10, and $0.03 per dry tonne kilometer ($0.14, $0.14, and $0.05 per dry ton-mile), respectively. This lead to the model selecting a priority of feedstock production sites by radial distance from the conversion facility in order of: switchgrass square bales, sorghum forage chopped, and switchgrass forage chopped. Feedstock production sites were selected from the closest point moving radially in order of transportation cost priority and sites that were already selected were removed (Figure 3).
The farthest distance for all of the feedstock systems was for forage chopped switchgrass, which had a farthest location of 69.2, 18.4, 62 kilometers (43, 11.4, and 38.5 miles) for Sanford (Piedmont), Kinston (Coastal Plain), and Lenoir (Mountains), respectively. The allocation of feedstocks for Sanford (Figure 3B) was not as defined as Lenoir (Figure 3C) or Sanford (Figure 3A). This was related to many locations in the Piedmont showing favorable economics with switchgrass and not sorghum (as seen in Caffrey et al. [20]). All potential biorefinery locations located switchgrass bale operations close to the conversion facility, reducing transportation from storage yards and complexity of the supply chain. Kinston (Figure 3A) differed in spatial feedstock allocation compared to the other two regions, but this can be tied to the small feedstock production radius and high cropland cover in the area (Table 4).
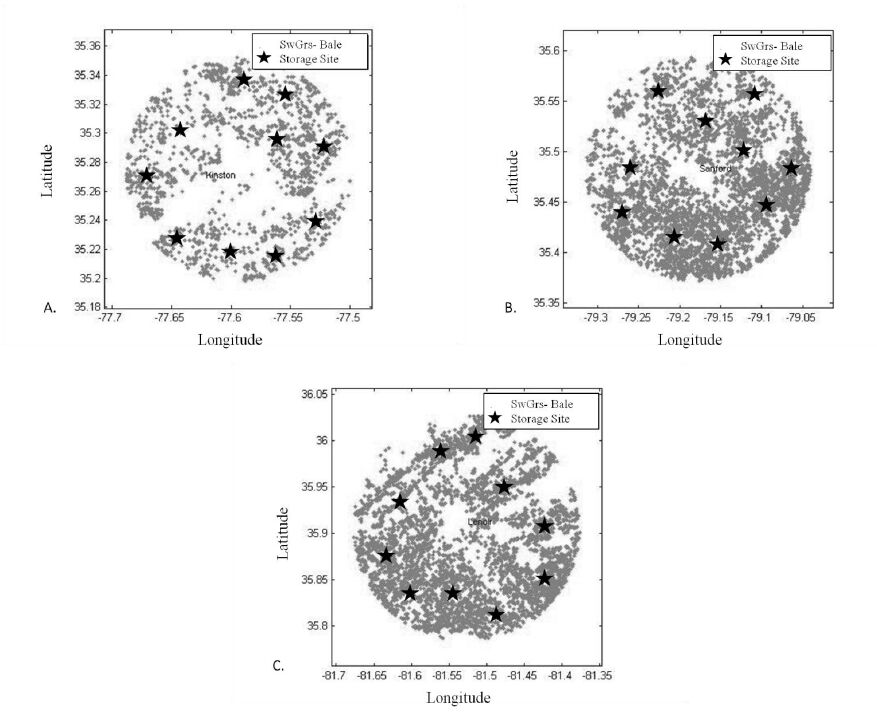
Maximum radial distance of the baled switchgrass was considerably shorter at 80.6%, 53.8%, and 78.2% (radial distance: 13.4, 8.5, and 13.5 kilometers) of all biomass crops for Sanford, Kinston, and Lenoir, respectively. This created a very tight radial area to determine optimal storage locations and made many combinations of storage yard locations close to optimal. Aside from the model results, it is still advisable to have multiple storage locations that are spread about the area of collection to reduce risk and allow for use of dissipated low value land area. If an alternative location-allocation procedure is used [42] a set number of storage locations can be set, with yield of each selected location used as a weighting factor. The single 16.2 hectare (40 acre) location determined seemed overly excessive but breaking this up into ten 1.6 hectare (4 acre) storage locations made the system simpler to optimize (Figure 4).
Siting storage as closely to these optimized sites as possible will reduce in-field transportation costs and help ensure that equal proportions of material will be taken to each storage site, while accounting for existing road networks. Average distance from conversion facility to storage site was 8.4, 6.4, and 9.5 kilometers (5.2, 4, and 5.9 miles) to facilities in Sanford, Kinston, and Lenoir, respectively. This modeled distance means that even with inclement weather, transportation may be possible, though a small storage facility would still be required at the conversion facility for day to day operations, further reducing the size and possibly the number of decentralized storage yards.
Results of this case study showed advantages in and benefits of siting a refinery in the Coastal Plain region of North Carolina because of the high proportion of agricultural land and high productivity of the region. For all modeled locations, siting perennial grass storage yards near the facility was observed as most optimal, while supply-driven production operations could be placed farther from the central location.
The simulation approach and developed model differ from others in that they include multiple feedstocks and multiple harvest systems, instead of assuming a single feedstock harvested in a single format to supply a conversion facility. This method allows selected feedstock production locations to be sited by transportation unit cost, which reduced the total area in which each of the feedstocks was produced. This compartmentalizes logistical optimization into set regions of the total area, allowing the potential for near optimal solutions to be realistic. The shift to supply-driven production supply chain management reduced storage requirements, corresponding storage losses and safety stock requirements, leading to reduced total feedstock requirements of the system. On a larger scale this modeling approach allows each individual entity or business unit to optimize operations within their own area of expertise (feedstock production, logistical operations, and conversion operations) and supports for full utilization of capital intensive specialized equipment.
4. Conclusion
Logistical operations of biomass supply chains can be incredibly complex with many different factors that need to be evaluated for optimal operations, and many involve a large number of disassociated production sites on defined harvest schedules which must feed a conversion facility operating continually year round (with the exception of a short maintenance period). The logistical model presented provides a method for determination of potential biorefinery locations, and proposes a multi-crop, multi-harvest system for increased supply-driven production operations, while also accounting for production costs to illustrate the relationships between the different model elements.
Optimization of the feedstock supply chain is an important aspect of biorefinery operations that can have a major impact on the overall economics of system operations. This is especially important for biomass since the material is low value, low bulk density, and required at a constant rate for year round operations. Though this specific model used data from North Carolina, the practical applications can be applied to produce a conceptual biomass supply system for any site with reasonable data available and assist the emerging biomass based industry evaluate different operations. Using the proposed multiple feedstock and harvest systems can facilitate increased supply-driven production operation, decrease storage requirements and increase equipment utilization.
· A simplified biorefinery siting heuristic can be used to target potential biorefinery locations, thus reducing computational complexity of a broad optimization procedure.
· Inclusion of multiple primary feedstocks (Sorghum & Switchgrass), winter cover crops where appropriate (Canola & Barley), and harvest methods (Forage & Bale) can shift the feedstock production system towards supply-driven production operations. This method has the potential to reduce total feedstock costs, reduce storage requirements, increase capital intensive harvest equipment utilization, and spread risk of catastrophic crop or storage losses.
· Use of a probabilistic profit based cropland conversion algorithm can assist with determination of potential cropland availability associated with a proposed biorefinery location. This can allow bio-based industry leaders to rapidly assess feedstock availability in a given area without the need for high cost ground trothing. This generated dataset can also be used to assess logistical options that may accurately reflect general locations of production sites, though they may represent the exact location.
· A decentralized storage system can provide benefits similar to both centralized (one site) or dissipated (site at each production field) storage systems, while limiting major disadvantages of each. This system will create a two stage wheel and spoke logistical formation for transportation between farms, storage sites, and the biorefinery.
· Analyzing the total feedstock logistical operations, from production to biorefinery delivery, allows for issues and benefits to be assessed much more than piece meal modeling approaches focusing on a single operational parameters (e.g. feedstock production). Production of multiple integrated tools for this analysis can greatly assist bio-based industry leaders in more efficiently assessing potential production sites across a range of system parameters.
· There were a number of limitations to this modeling approach, related to each of the modeling operations. These were primarily related to the use of current feedstock production practices, storage site configuration and biorefinery operations. Any alteration of these parameters could lead to major changes to the results of this model. While a sensitivity analysis of each parameter would be a logical next step in the model development, it was not included here to minimize the level of complexity tied to the model presented. This also maintained focus on the objectives of the work to highlight a novel supply chain system for biomass logistical operations and demonstrate the feasibility of this modeling approach as a decision making tool.
Acknowledgements
This research was partially supported by the USDA-NIFA Biomass Research and Development Initiative (grant#2011-10006-30363).
Conflict of interest
All authors declare no conflicts of interest in this paper.









 DownLoad:
DownLoad: