1. Introduction
Polluted sites, no matter if we are about talking the terrestrial or aquatic environment are omnipresent and significant efforts to disclose the sites and subsequently to clean up are made. However, remediation is not necessarily easy and not always straight forward. Thus, possibly a variety of remediation technologies may be brought into play, the different method having their advantages and disadvantages. Eventually, the choice of technology will be based on a weighing on pros and cons for the single options.
Typically, the choice cannot be based on a single indicator expressing the effectiveness of a given remediation approach. Thus, in order to rank the different possible remediation approaches in question requires several indicators as a proxy to an appropriate ranking. In other words, we are dealing with a so-called multi-indicator system (MIS) [1]. Based on such a set of indicators it is possible not only mutually to rank the single remediation approaches but also to obtain some ideas to what extent one approach is better, i.e., more efficient than another.
An alternative to handling the MIS with an appropriate mathematical model is, what is often seen a mathematical mapping of the single indicator values to get a one-dimensional scalar, eventually to be used as the ranking indicator [2]. However, this is not advisable as such a mapping process not only hides all the background information but also may have an unwanted effect of compensation [3].
There are many well-known methods to obtain from a multivariate data matrix a linear (or with respect to technical aspects also a weak, i.e., including ties) order like PROMETHEE [4], or the ELECTRE family [5, 6, 7, 8, 9]. However, all these methods require parameters beyond the data matrix to run them and may even be criticized from a theoretical point of view [3]. In the present paper, the concepts of partial order methodology are applied to the evaluation of remediation technologies. Partial ordering is, from a mathematical point of view simple, applying only the relation “≤” and appears as an advantageous way to look at MIS [10].
2. Methods
2.1. Partial ordering
Bruggemann and Carlsen (2012) recently argue that a partial order methodology is an alternative approach to study multi-indicator system (MIS). Partial ordering is a non-parametric method as, in contrast to standard multidimensional analyses, such as PROMETHEE [4] or the ELECTRE family [5, 6, 7, 8, 9] as no assumptions about linearity or distribution of the indicators neither are made and nor are necessary. ELECTRE III vs partial order based methodologies has been reported by Bruggemann and Carlsen(2015) [11].
Partial ordering has been described intensively in recent year [12, 13]. Hence, in the following only necessary basic information is given.
2.1.1. Basics of partial ordering
In Partial Ordering the only ma thematical relation among the objects, i.e., in the present case remediation options is “≤”. The “≤”-relation is the basis for a comparison of the different remediation options, ROs, and constitutes a graph. A given ROx is connected with ROy if and only if the relation y ≤ x holds. Thus, it needs to be defined what is meant by x ≤ y. If a system that can be described by a series of indicators rj, is considered, a ROx is characterized by a set of indicators rj(ROx), j = 1, . . ., m and can be compared to ROy, characterized by the indicators rj(ROy), when
| ri(ROy) ≤ ri(ROx) for all i = 1, …, m |
equation (1)
|
In the present study m equals 3 (cf. section 2.2).
Equation (1) is obviously a rather strict requirement for establishing a comparison demanding all indicators of ROx should be better (or at least equal) than the corresponding indicators of ROy. Note that in the present example (cf. the data section 2.2), the higher the indicator values are the better they are considered to be. Hence, if X is the group of ROs studied, i.e., X = {RO1, RO2, …, ROn}, ROx will be ordered higher (better) than ROy, i.e., ROx > ROy, if at least one of the indicator values for ROx is higher (better) than the corresponding indicator value for ROy and no indicator for ROx is lower (worse) than the corresponding indicator value for ROy. On the other hand, if for some indicator j rj(ROx) > rj(ROy) and for some other indicator i ri(ROx) < ri(ROy), ROx and ROy will be denoted incomparable (notation: ROx ǁ ROy). In mathematical term this means a contradiction due to conflicting indicator values prevails. In cases where all indicator values for two RO are equal for all j, the two options will be considered as equivalent, i.e., ROx ~ ROy, having the same order. Note that in other studies applying partial order methodology the term “order” is typically referred to as “rank” or “height”.
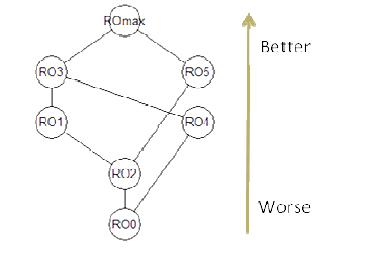
The graphical representation of the partial ordering is often given in a so-called Hasse diagram [12], which is a directed graphs, which is structured in levels and consist of chains and antichains (Figure 1).
· Chain: A subset X' ⊆ X, where all objects fulfill (1) is called a chain. A chain has a length, which is |X'|-1.For objects within a chain, e.g., from the bottom to the top of the chain, all indicators are simultaneously non-decreasing.
· Antichain: A subset X' ⊆ X, where no object fulfill (1), i.e., all objects in X' are mutually incomparable, is called an antichain. Thus, for any two objects within an antichain there is a conflict in indicator values.
· Level: The horizontal arrangement of objects within a Hasse diagram. Levels per definition constitute an antichain, whereas the reverse not necessarily is true.
2.1.2.Average orders
The level structure constitutes a first approximation to ordering. However, this will obviously give rise to many tied orders as all objects in a level automatically will be assigned identical orders. It is in general desirable that the degree of tiedness is as low as possible. Hence, ultimately a linear ordering of the single objects (here ROs) is desirable, which it is obviously not immediately obtainable when incomparable objects are included.
Partial order methodology provides a weak order, where tied orders are not excluded. This is obtained by calculating the average order of the single objects as described by Bruggemann and Annoni (2014) [14]. In cases comprising only a relatively low number of objects (typically < 25) the average orders may be calculated by an exact method based on lattice theory [15, 16, 17]. For larger systems, approximations have been presented [1].
The calculations, which in the present study the average orders are calculated based on the exact method will assign an average order to the individual objects [15, 16, 17]. With the indicator orientation chosen as in the present study, the average orders will be assigned from 1 (bottom) up to maximum n (top), n being the number of RO (here 6 (7), cf. section 2.2), which can be regarded as a scoring system, i.e., the higher the score the better the RO.
2.1.3.Perturbation of order characteristics
The single objects in the Hasse diagram are characterized by order characteristics, which are 4 values that state the number of equivalences, predecessors (number of upwards comparable objects), successors (number of downwards comparable objects) and incomparable elements, respectively [13]. Obviously, changes in one or more of the indicators for a given object may cause changes in the order characteristics for the object under investigation and thus be a tool to disclose where specific ROs advantageously could be improved [18].
2.1.4.PyHasse software
Partial order analyses were carried out applying the PyHasse software [19]. PyHasse is programmed using the interpreter language Python (version 2.6). The software package contains today more than 100 modules and is under continuous further development 1. The present study will apply only three central modules of the PyHasse suite, i.e., mHDCl2_8 (PyHasse main module), avrank5 (exact method to generate average orders) and the scanincomp4_4 (setting threshold limits for significance).
1 PyHasse is available upon request from the developer, Dr. R. Bruggemann (brg_home@web.de). An Internet version is currently under development and will contain a limited number of the more frequently used module. The internet version
that currently comprise a few central modules is available at www.PyHasse.org.
2.2.Datasets
To illustrate the principles of using partial order methodology as an analytical decision support tool for the selection of the most appropriate remediation technology for polluted sites a simple, but exemplary dataset has been created assuming 5 possible options for remediation and 3 polluting chemicals for which the remediation is supposed to remove to some extent. In Table 1 the dataset applied is shown. Note that in addition to the 5 remediation options a reference option, RO0, is introduced corresponding to the original concentrations, i.e., the remediation percentage for all 3 chemicals is 0.A further reference point, ROmax, is introduced referring to the optimal situation, i.e., all 3 chemicals being completely removed corresponding to a remediation percentage of 100%.
Table 1. Fraction of three chemicals being removed by different remediation options
| | Removed fraction |
| Remediation Option | Chemical 1 | Chemical 2 | Chemical 3 |
| ROmax | 1 | 1 | 1 |
| RO1 | 0.739 | 0.581 | 0.520 |
| RO2 | 0.417 | 0.240 | 0.141 |
| RO3 | 0.852 | 0.623 | 0.909 |
| RO4 | 0.035 | 0.011 | 0.603 |
| RO5 | 0.065 | 0.689 | 0.413 |
| RO0 | 0 | 0 | 0 |
Table 1 is the basic dataset for the present study; the single values correspond to the fraction of the 3 chemicals, respectively, being removed by the 5 remediation options.
To illustrate the effect of specific improvements of the remediation of a single option we assume in a first approach that the RO5 could be improved in a way so Chemical 1 could be 56.5% removed (Table 2).
Table 2. Fraction of three chemicals being removed by different remediation options improving RO5 to better remove Chemical 1
| | Removed fraction |
| Remediation Option | Chemical 1 | Chemical 2 | Chemical 3 |
| ROmax | 1 | 1 | 1 |
| RO1 | 0.739 | 0.581 | 0.520 |
| RO2 | 0.417 | 0.240 | 0.141 |
| RO3 | 0.852 | 0.623 | 0.909 |
| RO4 | 0.035 | 0.011 | 0.603 |
| RO5 | 0.565 | 0.689 | 0.413 |
| RO0 | 0 | 0 | 0 |
To further elucidate the effect of a virtually equal removal of the 3 chemicals we assume an approach where the 3 chemicals are removed virtually to the same extent by RO1 (Table 3).
Table 3. Fraction of three chemicals being removed by different remediation options following improvement of RO1 to removed Chemical 2 and 3 to an equal extent
| | Remedied fraction |
| Remediation Option | Chemical 1 | Chemical 2 | Chemical 3 |
| ROmax | 1 | 1 | 1 |
| RO1 | 0.739 | 0.781 | 0.720 |
| RO2 | 0.417 | 0.240 | 0.141 |
| RO3 | 0.852 | 0.623 | 0.909 |
| RO4 | 0.035 | 0.011 | 0.603 |
| RO5 | 0.065 | 0.689 | 0.413 |
| RO0 | 0 | 0 | 0 |
It should be noted that the above datasets are constructed in order to illustrate in the best possible way the advantageous use of partial order methodology to select the most appropriate remediation technology and as such the present study can be considered to be methodological.
3.Results and Discussion
An obvious way of ordering the different remediation options is by using an aggregated indicator that gives an indication on the overall remediation percentage. This may be done by a simple arithmetic average of the single indicator values for each remediation option. Hence, before exploring the partial order methodology it might be useful to answer the question: why partial ordering and not a simple aggregated indicator that immediately would allow an absolute ordering of the various options? The answer can be found by looking at a rather simple example.
As already mentioned in the introduction the use of aggregated indicator values in order to achieve an absolute ordering of the objects, here ROs, potentially is subject to compensation effects [3] whereby significant information may be lost. Thus, assume that ROx will clean for the 3 chemicals to 0, 100 and 100%, respectively. An aggregated indicator, based on a simple arithmetic mean will be (0 + 1 + 1)/3 = 0.667, corresponding to an overall remediation percentage of 67.6%. Alternatively we could apply a ROy where the removal of the 3 chemicals were 67.6%, thus by the same type of aggregated indicator, would lead to (0.676 + 0.676 + 0.676)/3 = 0.676, in other words to exactly the same overall remediation percentage, 67.6%, despite the fact that the two options obviously lead to significantly different remediation results, a difference, and the background for the difference that in no way can be explained based on the aggregated indicators. A possible introduction of weights for the single chemicals may further muddle the picture.
3.1.Partial ordering
Looking at the data given in Table 1 it is immediately clear that the 5 possible remediation options are quite different in their removal of the different chemicals ranging from 1.1% (RO4/Chemical 2) to 90.9% (RO3/Chemical 3). Further with a look at the data in Table 1 it is further clear that it is not evident which of the 5 remediation options that is actually the most optimal. However, this becomes much more obvious when looking at the partial ordering as displaying as a Hasse diagram in Figure 1.
First of all it is disclosed from Figure 1 as well as from the MIS in Table 1 that none of the ROs equals the optimal situation, as given by ROmax but, on the other hand, they are all better than the starting point, i.e., RO0.
Scrutinizing Figure 1, it is immediately seen that RO3 > RO4 and RO3 > RO1 > RO2, thus obviously fulfilling equation 1.On the other hand RO5 apparently is incomparable to all the other four ROs. Thus, we have basically no information about RO5 in relation to one or more of the other 4 ROs. It should be noted that the location of an object like RO5 in the Hasse diagram by convention will be placed as high as possible but in principle it could be placed where ever in the diagram within the limits RO0 and ROmax, respectively. Turning to RO4 it is seen from Figure 1 that it is better than RO0 and worse than RO3.However, if RO4 is better or worse than RO1 or RO2 cannot be deducted from the diagram. To obtain further information on the mutual ordering of the different options we turn to average orders (vide infra).
It is worthwhile to note that an apparent disadvantage of the partial ordering is that the ordering equation (equation 1) obviously may cause incomparabilities even due to very minor differences in indicator values. The software ELECTRE III takes care for such cases by a set of parameters beyond the data matrix and functions describing the degree of user preferences, which basically means that ELECTRE III can be seen as a fuzzy method [11]. A fuzzy concept is built in the partial order methodology [11]. However, this is outside the scope of the present study. Nevertheless, the possible effect of small differences in indicator values are addressed (vide infra).
3.2.Average orders
As evident from the discussion above, certain ROs, as RO4 and RO5 may possess a variety of orders without violating the overall ordering displayed in Figure 1. Using RO4 as an example the following orderings are possible ROmax > RO3 > RO4 > RO1 > RO2 > RO0, ROmax > RO3 > RO1 > RO4 > RO2 > RO0 and ROmax > RO3 > RO1 > RO2 > RO4 > RO0, respectively. Thus, the single ROs may in the partially ordered system be assigned a multitude of orders dependent on the actual location and environment in the partial order, as illustrated by the Hasse diagram (Figure 1). Consequently an absolute ordering of the ROs is not possible. However, it is possible to deduct a weak ordering based on average orders, formally obtained as averages of the probabilities for the single possible ranks [20, 21, 22]. In the present case calculations use the exact method based on lattice theory [15, 16, 17].
In Table 4 the average order, denoted Rkav, for the different ROs is given together with the [0, 1] normalized Rkav as well as the overall fraction being remedied, calculated as the arithmetic means of the indicator values given in Table 1.
Table 4. Ordering of the original dataset (Table 1)
| | Ordering |
| Remediation Option | Rkav | [0, 1]
normalized Rkav | Overall fraction remedied |
| ROmax | 7 | 1 | 1 |
| RO3 | 5.800 | 0.800 | 0.795 |
| RO1 | 4.200 | 0.533 | 0.613 |
| RO5 | 4.000 | 0.500 | 0.389 |
| RO4 | 3.400 | 0.400 | 0.216 |
| RO2 | 2.600 | 0.267 | 0.266 |
| RO0 | 1 | 0 | 0 |
The discrepancy between the ordering based on the Rkav and the arithmetic mean values is evident when looking at RO4 and RO2.
Admittedly the differences in the present rather simple study do not appear spectacular. It should in this connection be noted that in the present study the partial ordering as displayed in Figure 1 has very few incomparabilities. As a consequence of that each aggregation, which is order preserving (such as adding or multiplying indicator values) will reproduce the majority of order relations.
A further point that should be addressed is the potential problem concerning small differences in indicator values. Thus, looking at the data Table 1, looking at RO1 and RO4, it is seen that RO1 > RO4 for Chemicals 1 and 2, whereas RO4 > RO1 for Chemical 3, which leads to the incomparability between these two options (Figure 1). Based on the average orders (Table 4) it is concluded that RO1 > RO4.However, it may be argued that the difference in indicator values for Chemical 3 is so small that it could be neglected which would make RO1 and RO4 comparable and thus intuitively lead to the identical result, i.e., RO1 > RO4.The question of the possible effect of small differences can be mathematically dealt with applying a specialized module of the PyHasse software [19]. Hence, in the present case, i.e., RO1 vs RO4, it is disclosed that if absolute differences in the [0, 1] normalized indicator values, a threshold ˂ 0.09 is accepted as insignificant RO1 = RO4 with regard to Chemical 3 [19].
3.3.Improving a remediation technique
The above data and results may constitute an appropriate background for additional work in order to improve one or more of the remediation technologies in question. For illustrative purpose we will in the following focus on the two options RO1 and RO5, respectively. Figure 1 discloses that RO1 is “locked” between RO2 and RO3 whereas RO5 in principle is able to “float” to any position between the two extremes, RO0 and ROmax. It is further noted (Table 1) that RO1 is rather effective in removing Chemical 1 and medium effective for Chemicals 2 and 3.On the other hand it is clear (Table 1) that RO5 is rather poor in removing Chemical 1, medium effective for Chemical 3 and medium to high effective for Chemical 2, respectively. Despite these differences the average orders of the two options appear rather close (Table 4), the [0,1] normalized values being 0.532 and 0.500 for RO1 and RO5, respectively, whereas a significant discrepancy between the respective overall fractions being remedied are noted (Table 4), which, as explained above does not disclose any details about the two remediation options.
Assuming that RO5 for some reason, e. g., economic considerations, is favored over RO1, we can use the above described tools to elucidate what changes in the fractions being removed affects the average orders and thus possibly the decision process.
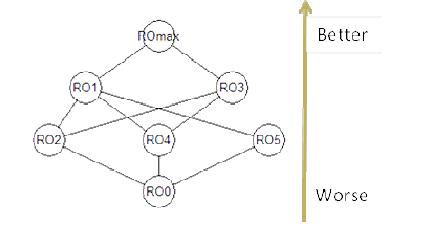
Obviously the very low removal of Chemical 1 constitutes the immediate problems in relation to RO5.Let us then assume that it is possible to adopt some strategies or methods from one of the other ROs that would improve the remediation percentage for Chemical 1 by RO5 from 6.5% to 56.5%, the resulting perturbed MIS is given in Table 2. This MIS lead to the Hasse diagram visualized in Figure 2.
The change in the diagram in Figure 2 compared to that based on the original MIS (Figure 1) is minor but important as RO5 is now comparable to RO2.Thus, the possible orders for RO5 have by this been somewhat limited. However, the two ROs to be compared, i.e., RO1 and RO5, are still incomparable.
Turning to the average orders (Table 5) it is immediately noted that the change in the remediation percentage for Chemical 1 by RO5 significantly changed the mutual relation between the options RO1 and RO5 the latter now being the more attractive choice.
Table 5. Ordering of the first perturbed MIS (Table 2)
| Ordering |
| Remediation Option | Rkav | [0, 1]
normalized Rkav | Overall fraction remedied |
| ROmax | 7 | 1 | 1 |
| RO3 | 5.727 | 0.788 | 0.795 |
| RO5 | 4.636 | 0.606 | 0.556 |
| RO1 | 4.000 | 0.500 | 0.613 |
| RO4 | 3.364 | 0.394 | 0.216 |
| RO2 | 2.273 | 0.212 | 0.266 |
| RO0 | 1 | 0 | 0 |
A final remark on the relation between RO1 and RO5, from Table 2 it can be seen that RO1 > RO5 for Chemical 1 and 3 whereas RO5 > RO1 for Chemical 2.Now it may be argued that the differences for indicator values Chemical 2 and 3 are − 0.108 and + 0.107, respectively and thus they “somehow” compensate for each other and leave only the indicator Chemical 1 as determining which would leave to the opposite ordering conclusion, i.e., RO1 > RO5.However, this is exactly a conclusion that cannot be drawn as there is no proof that what are good/bad for Chemical 2 can be compensated by what are bad/good for Chemical 3 [3].
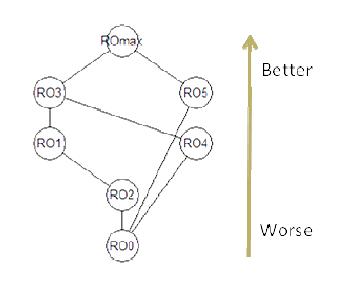
An alternative approach would obviously be to improve RO1 by increasing the remediation percentages of Chemicals 2 and 3 by, e.g., 20% thus have a remediation option that for all 3 chemicals would lead to roughly a 75% removal. The corresponding MIS is given in Table 3 which leads to the Hasse diagram displayed in Figure 3.
Comparing to the original Hasse diagram (Figure 1) it is obvious that significant changes have now appeared with an increased number of comparisons. Thus, it is immediately noted that now RO1 and RO5 are comparable and that RO1 > RO5. It can further be noted that now RO1 is no longer comparable to RO3 and both RO1 > RO4 and RO3 > RO4.
The fact that RO1 > RO5 is accordingly also shown when looking at the average orders (Table 6), where RO1 now constitutes as the most optimal remediation option. Hence, assuming all other equal RO1 should be chosen for the given remediation task.
Table 6. Ordering of the second perturbed MIS (Table 3)
| | Ordering |
| Remediation Option | Rkav | [0, 1]
normalized Rkav | Overall fraction remedied |
| ROmax | 7 | 1 | 1 |
| RO1 | 5.571 | 0.762 | 0.747 |
| RO3 | 5.286 | 0.714 | 0.795 |
| RO5 | 3.286 | 0.381 | 0.389 |
| RO2 | 2.929 | 0.322 | 0.266 |
| RO4 | 2.929 | 0.322 | 0.216 |
| RO0 | 1 | 0 | 0 |
It is at this stage necessary to comment on the term “all other equal”. In the present study we have considered the removal of 3 chemicals. The original as well as the residual “concentration” of the chemicals may be given as amounts (e. g., g/kg), toxicity (e. g., LD50 towards a selected organism), the carcinogenic potential etc. However, it is obvious that other factors typically are brought into play such as economic aspects, environmental aspects as destruction of areas worthy of preservation, destruction of habitats etc.
It is immediately possible to apply the above described methodology on these aspects as well and eventually combine the analyses applying hierarchical partial ordering [23] However, this is outside the scope of the present study.
4.Conclusion
In the present study a simple analytical tool for decision support in relation to selecting the more appropriate remediation technology/option for polluted sites. It has been demonstrated that applying partial order methodology a deeper insight in the factors governing the different options can be disclosed and as such form the background, not only for selecting the more appropriate option but also serving as an information source in relation to further improvements of one or more of the options under discussion.
The here presented example is based on the remediation of 3 chemicals. Obviously these chemicals, and thus the values in the MIS can be any kind of measure of “concentration”, i.e., amounts, persistence, bioaccumulation, toxicity etc. As such presented method does not have any imitations. Further, factors like economic and environmental considerations may be taken into account, possibly in a separate approach eventually combining the various analyses through a hierarchical approach [23].
Finally it should be emphasized that the ratio between the number of objects studied and the number of indicators may turn out as crucial [20]. Thus, if the number of indicators relative to the number of objects studied is too high the number of incomparisons unambiguously will increase, ultimately leading to a complete antichain obviously with a loss of predicting power. However, this apparent problem may be circumvented by applying hierarchical partial order ranking (HPOR) as previously described by the author [23]. Here the group of indicators is subdivided into appropriate groups. The objects, here remediation options are thus partially ordered according to the single subgroups of indicators. In a second partial ordering the results of the original analyses of the single subgroups are combined to the final ordering.
Conflict of Interest
Author declares no conflicts of interest in this paper.









 DownLoad:
DownLoad: