Practical generalized finite-time synchronization of duplex networks with quantized and delayed couplings via intermittent control

1.   Introduction

In the past, most of the research hotspots of complex networks theory focused on single-layer networks ^[1,2,3,4]. Considering that single-layer networks cannot describe the structure of two or more interconnected networks, many academics are now focusing on the structural model of multilayer networks ^[5,6,7,8], which is one of the most active topics in the field of complex networks research in recent years. Among them, the duplex networks (DNs) models can precisely depict the information exchange between two layers and accurately reflect the coupling relationship between layers. For example, Xu et al. ^[5] investigated the intralayer and interlayer quasi-synchronization of two-layer multi-weighted networks. The authors in ^[6] analyzed the interlayer synchronization for duplex networks by event-dependent intermittent control. At present, the research on DNs has made important progress and has been excellently applied in social networks analysis, transportation networks, and so on. And because the data information is transmitted over a network link, it is required to be quantized into a limited number of bits before transmission. This promotes us to study the stability and control of DNs with quantized couplings.

Many academics have assumed that all nodes between systems have exactly the same dynamics while studying the dynamical behaviors of complex networks. However, considering the complexity and changing topological nature of network structure, nodes in the networks usually have different dynamics. Thus, generalized synchronization has been proposed to study the synchronization of network systems with the identical or different dynamics, and some theoretical results have been investigated in ^[9,10,11,12]. Unlike other types of synchronization, generalized synchronization introduces a vector function between the systems, allowing the systems to exhibit richer dynamics. In particular, it can also be used to extend the coexistence of different synchronization types. Yang et al. ^[9] considered the generalized lag-synchronization of systems with uncertain parameters and unknown perturbations. Zheng et al. ^[10] investigated the generalized projective lag synchronization criteria of neural networks with delay. Nevertheless, most of the studies mentioned above have focused on the generalized synchronization of single-layer networks, while fewer studies have been conducted on multilayer networks. To our knowledge, in reality the states of systems usually converge to a neighborhood of the origin and not to the origin. From above, it can be seen that it is significant to explore the practical generalized finite-time synchronization (PGFETS) of DNs in this paper.

Obviously, a suitable control scheme is the key to achieve synchronization of the systems, which affects not only the settling time but also the synchronization performance. The control methods that are presented currently contain state feedback control ^[13], adaptive control ^[14], event-triggered control ^[15], intermittent control ^{[16,17,18,19,20]}, etc., where intermittent control is a typical discontinuous control strategy. It is the discontinuity in working time that makes intermittent control less expensive to control and more suitable for practical applications. Furthermore, we introduce a quantizer in the controller to further conserve communication resources and improve communication efficiency. For example, the finite-time control issue of a class of hybrid systems under quantized intermittent control is proposed in ^[18]. In ^[19], the authors discussed the finite-time synchronization of stochastic complex networks via quantized aperiodically intermittent control. However, chattering is inevitable in the controllers with sign function designed above. In conclusion, this paper designs intermittent control methods without sign function, which effectively mitigate chattering phenomenon.

Motivated by the points discussed above, this paper explores the PGFETS of DNs with quantized and delayed couplings via intermittent control, and the following are the main highlights:

(1) To better capture the characteristics of realistic multiplex networks, a class of DNs with quantized and delayed couplings is considered in this study.

(2) Consider that in reality the error system will not converge to the origin ideally, but rather in an interval of the origin. Then, the PGFETS is presented in this paper.

(3) The quantized intermittent control strategies without sign function are utilized in this paper, which save the channel resources and control costs.

The outline of the remaining parts is organized as: Section 2 presents the system model and preliminary works. In Section 3, the PGFETS issue of DNs is discussed, and sufficient conditions are obtained through the control schemes. To verify the validity of the results, numerical examples are shown in Section 4. Finally, Section 5 draws the conclusions.

Notations: In this paper, $\mathbb{R}, \mathbb{R}^{n}, \mathbb{R}^{n\times m}, \mathbb{N}$ denote the set of real numbers, the vector space with $n$-dimensional, the set of $n\times m$ matrices, and the set of natural numbers, respectively. $\mbox{diag}(\cdot)$ represents the diagonal matrix. $I_{n}$ is the $n$-dimensional unit matrix. $\|\cdot\|$ denotes the 2-norm of a vector or matrix. The superscript symbol $T$ and $-1$ indicate the transposition and inverse. $\lambda_{\max}(\cdot)$(or $\lambda_{\min}(\cdot)$) is the largest (or smallest) eigenvalue of a matrix. $\otimes$ represents the Kronecker product.

2.   Preliminaries

A class of DNs with quantized and delayed couplings under control is constructed below:

where $\imath, \jmath\in \mathcal{N} = \{1, 2, \ldots, N\}, \vartheta_{\imath}(t), \omega_{\imath}(t)\in \mathbb{R}^{n}$ denote the state vectors of the $\imath$th nodes in $\vartheta$-layer and $\omega$-layer, respectively. $\mathfrak{C} = \mbox{diag}(\mathfrak{c}_{1}, \mathfrak{c}_{2}, \ldots, \mathfrak{c}_{n})$, $\mathfrak{D}\in \mathbb{R}^{n\times m}.$ $\mathfrak{g}^{\vartheta(\omega)}(\cdot): \mathbb{R}^{m}\rightarrow \mathbb{R}^{m}$ is the activation function, where $\vartheta(\omega)$ denotes $\vartheta$-layer or $\omega$-layer. $\mathfrak{F}^{\vartheta(\omega)}(\cdot)$ is the quantizer defined below. $\mathfrak{P}^{\vartheta(\omega)} = [\mathfrak{p}^{\vartheta(\omega)}_{1}, \mathfrak{p}^{\vartheta(\omega)}_{2}, \ldots, \mathfrak{p}^{\vartheta(\omega)}_{m}]^{T},$ where $\mathfrak{p}^{\vartheta(\omega)}_{k}\in \mathbb{R}^{1\times n}, k = 1, 2, \ldots, m,$ $\mathfrak{P}^{\vartheta}\vartheta_{\imath}(t-\sigma^{\vartheta}_{1}(t)) = [\mathfrak{p}^{\vartheta}_{1}\vartheta_{\imath}(t-\sigma^{\vartheta}_{1}(t)), \mathfrak{p}^{\vartheta}_{2}\vartheta_{\imath}(t-\sigma^{\vartheta}_{1}(t)), \ldots, \mathfrak{p}^{\vartheta}_{m}\vartheta_{\imath}(t-\sigma^{\vartheta}_{1}(t))]^{T}$, $\mathfrak{P}^{\omega}\omega_{\imath}(t-\sigma^{\omega}_{1}(t)) = [\mathfrak{p}^{\omega}_{1}\omega_{\imath}(t-\sigma^{\omega}_{1}(t)), \mathfrak{p}^{\omega}_{2}\omega_{\imath}(t-\sigma^{\omega}_{1}(t)), \ldots, \mathfrak{p}^{\omega}_{m}\omega_{\imath}(t-\sigma^{\omega}_{1}(t))]^{T}$. $\mathfrak{g}^{\vartheta}(\mathfrak{P}^{\vartheta}\vartheta_{\imath}(t-\sigma^{\vartheta}_{1}(t))) = [\mathfrak{g}^{\vartheta}_{1}(\mathfrak{p}^{\vartheta}_{1}\vartheta_{\imath}(t-\sigma^{\vartheta}_{1}(t))), \mathfrak{g}^{\vartheta}_{2}(\mathfrak{p}^{\vartheta}_{2}\vartheta_{\imath}(t-\sigma^{\vartheta}_{1}(t))), \ldots, \mathfrak{g}^{\vartheta}_{m}(\mathfrak{p}^{\vartheta}_{m}\vartheta_{\imath}(t-\sigma^{\vartheta}_{1}(t)))]^{T}$, $\mathfrak{g}^{\omega}(\mathfrak{P}^{\omega}\omega_{\imath}(t-\sigma^{\omega}_{1}(t)))$ $= [\mathfrak{g}^{\omega}_{1}(\mathfrak{p}^{\omega}_{1}\omega_{\imath}(t-\sigma^{\omega}_{1}(t))), \mathfrak{g}^{\omega}_{2}(\mathfrak{p}^{\omega}_{2}\omega_{\imath}(t-\sigma^{\omega}_{1}(t))), \ldots, \mathfrak{g}^{\omega}_{m}(\mathfrak{p}^{\omega}_{m}\omega_{\imath}(t-\sigma^{\omega}_{1}(t)))]^{T}.$ $\rho^{\vartheta(\omega)}$ denotes the coupling strength, the outer coupling matrix of each layer represented by $\mathcal{A}^{\vartheta(\omega)} = (\alpha^{\vartheta(\omega)}_{\imath\jmath})\in \mathbb{R}^{N\times N}$ with $\alpha^{\vartheta(\omega)}_{\imath\jmath} > 0\; (\imath\neq \jmath)$ if node $\imath$ is related to node $\jmath$, otherwise, $\alpha^{\vartheta(\omega)}_{\imath\jmath} = 0$, and $\alpha^{\vartheta(\omega)}_{\imath\imath} = -\sum_{\imath = 1, \jmath\neq \imath}^{N}\alpha^{\vartheta(\omega)}_{\imath\jmath}$. $\Upsilon$ represents the inner coupling matrix of the nodes within two layers. $\sigma^{\vartheta(\omega)}_{1}(t)$ is time-varying delay satisfying $0\leq\sigma^{\vartheta(\omega)}_{1}(t)\leq\sigma^{\vartheta(\omega)}_{1}, \dot{\sigma}^{\vartheta(\omega)}_{1}(t)\leq\bar{\sigma}^{\vartheta(\omega)}_{1} < 1$. The coupling delay $\sigma^{\vartheta(\omega)}_{2}(t)$ satisfies $0\leq\sigma^{\vartheta(\omega)}_{2}(t)\leq\sigma^{\vartheta(\omega)}_{2},$ $\dot{\sigma}^{\vartheta(\omega)}_{2}(t)\leq \bar{\sigma}^{\vartheta(\omega)}_{2} < 1$. Let $\sigma^{\vartheta(\omega)}_{\max} = \max\{\sigma^{\vartheta(\omega)}_{1}, \sigma^{\vartheta(\omega)}_{2}\}.$ $\mathfrak{u}^{\vartheta(\omega)}_{\imath}(t)$ is the controller to be determined. The initial conditions of DNs (2.1) are $\vartheta_{\imath}(t) = \varphi^{\vartheta}_{\imath}(t)\in \mathcal{C}([-\sigma^{\vartheta}_{\max}, 0], \mathbb{R}^{n}), \omega_{\imath}(t) = \varphi^{\omega}_{\imath}(t)\in\mathcal{C}([-\sigma^{\omega}_{\max}, 0], \mathbb{R}^{n}).$ A quantizer $\mathcal{F}^{\vartheta(\omega)}(o):\mathbb{R}\rightarrow \Psi = \{\pm \gamma_{\tau}: \gamma_{\tau} = \ell^{\tau}\gamma_{0}, \tau = 0, \pm1, \pm2, \ldots, \}\cup \{0\}$ with $\gamma_{0} > 0.$ For $\forall o \in \mathbb{R},$ the quantizer $\mathcal{F}^{\vartheta(\omega)}(o)$ is designed as:

in which $\partial = \frac{1-\ell}{1+\ell}, 0 < \ell < 1.$ Refer to ^[21], where there is a Filippov solution $\Lambda\in [-\partial, \partial)$ that makes $\mathcal{F}^{\vartheta(\omega)}(o) = (1+\Lambda)o$. Let $\mathfrak{F}^{\vartheta(\omega)}(z_{\imath}) = (\mathcal{F}^{\vartheta(\omega)}(z_{\imath1}), \mathcal{F}^{\vartheta(\omega)}(z_{\imath2}), \ldots, \mathcal{F}^{\vartheta(\omega)}(z_{\imath n}))^{T}$, $|\mathfrak{F}^{\vartheta(\omega)}(z_{\imath})|^{\chi} = (|\mathcal{F}^{\vartheta(\omega)}(z_{\imath1})|^{\chi}, |\mathcal{F}^{\vartheta(\omega)}(z_{\imath2})|^{\chi}, \ldots, |\mathcal{F}^{\vartheta(\omega)}(z_{\imath n})|^{\chi})^{T}$ for $z_{\imath}\in \mathbb{R}^{n}, \chi > 0$.

It can be said that the networks (2.1) obtain synchronization when the states of nodes in $\vartheta$-layer converges to the identical state $\mathfrak{s}^{\vartheta}(t)$, while all states in $\omega$-layer reach the identical state $\mathfrak{s}^{\omega}(t)$. Hence, the target systems are modeled as

with the initial conditions $\mathfrak{s}^{\vartheta}(t) = \mathfrak{S}^{\vartheta}(t)\in\mathcal{C}([-\sigma^{\vartheta}_{1}, 0], \mathbb{R}^{n}), \mathfrak{s}^{\omega}(t) = \mathfrak{S}^{\omega}(t)\in\mathcal{C}([-\sigma^{\omega}_{1}, 0], \mathbb{R}^{n}),$ and other parameters remain the same as in systems (2.1).

Remark 1. Systems (2.1) consider the quantized couplings and the different connections of network systems. It is more general than the single-layer networks in ^[1,2] and two-layer networks without quantized couplings such as ^[5,6,7].

Below, we provide the assumptions, lemmas, and definitions needed for this article before getting the theoretical results.

Definition 1. The PGFETS between networks (2.1) and (2.2) with respect to the vector map $\psi(\cdot):\mathbb{R}^{n}\rightarrow \mathbb{R}^{n}$ is said to be realized, if there exist constants $\mathfrak{W} > 0$ and $\mathcal{T}_{f} > 0$ such that

where $\imath\in \mathcal{N}$, $\mathcal{T}_{f}$ is the settling time.

Remark 2. From Definition 1, it is clear that the finite-time synchronization in ^[3,4,5] is a special case of generalized finite-time synchronization when $\psi(\mathfrak{s}^{\vartheta(\omega)}(t)) = \mathfrak{s}^{\vartheta(\omega)}(t)$. However, it is not applicable for system nodes with different dynamic characteristics in reality. Consequently, the study of PGFETS is of more practical interest.

Assumption 1. For $\mathfrak{g}^{\vartheta(\omega)}(\cdot):\mathbb{R}^{n}\rightarrow \mathbb{R}^{n}$, there are nonnegative constants $\mathcal{L}^{\vartheta}$ and $\mathcal{L}^{\omega}$ that meet

Assumption 2. Let $0 < q < 1$ and $\varepsilon > 0$, then there is a continuous function $f:[0, \infty)\rightarrow [0, \infty)$ with $f(0)\geq0,$ for any $0\leq \mu\leq t,$ that satisfies

Lemma 1. ^[22] There is a positive definite matrix $\mathcal{Q}\in \mathbb{R}^{n\times n}$ such that

for any $\mathfrak{h}_{1}, \mathfrak{h}_{2}\in \mathbb{R}^{n}.$

Lemma 2. ^[23] Given that $\nu_{1}, \nu_{2}, \ldots, \nu_{N}\geq0, 0 < \zeta_{1}\leq1, \zeta_{2} > 1$, then

Lemma 3. ^[24] For any $\aleph\in\mathbb{R}, \beta\gg1$, there is

where $\flat = 0.2785$.

Lemma 4. ^[25] For $\mathfrak{y}_{1}, \mathfrak{y}_{2}, \ldots, \mathfrak{y}_{N} \in \mathbb{R}^{n}$, there exists $0 < p < 2$, which satisfies

Definition 2. ^[26] Function $V(x): \mathbb{R}^{n}\rightarrow \mathbb{R}$ is called C-regular if $V(x)$ is:

(ⅰ) regular in $\mathbb{R}^{n}$;

(ⅱ) positive definite, i.e., $V(x) > 0$ for $x\neq 0$ and $V(0) = 0$;

(ⅲ) radially unbounded, i.e., $V(x)\rightarrow +\infty$ as $\|x\|\rightarrow +\infty$.

Lemma 5. ^[27] Suppose that there exists a strict increasing time sequence $\{t_{k}\}_{k\in \mathbb{N}}$, which satisfies $t_{0} = 0, \lim\limits_{k \to +\infty}t_{k} = +\infty$. Let us take $£^{\downarrow}_{k} = [t_{2k}, t_{2k+1}), £^{\uparrow}_{k} = [t_{2k+1}, t_{2k+2}), \Im^{\downarrow}_{k} = t_{2k+1}-t_{2k}$, $\Im^{\uparrow}_{k} = t_{2k+2}-t_{2k+1},$ and $V(t): \mathbb{R}^{n}\rightarrow \mathbb{R}$ is C-regular, satisfying:

in which $t\in\big[0, +\infty)$, $\wp\in(0, 1)$, $\varrho_{0}$, $\varrho_{1}$, $\varrho_{2},$ and $\varrho_{3}$ are positive constants. Furthermore, if there exists a $k\in \mathbb{N}$ such that

then there exists a constant $\mathcal{T}_{f} > 0$ satisfying $V(t)\leq \big(\frac{\varrho_{0}}{\varrho_{2}(1-\delta)}\big)^{\frac{1}{\wp}}$ for $t > \mathcal{T}_{f}$, and

where $\phi_{1} = (1-\wp)\varrho_{1}$, $\phi_{2} = (1-\wp)\varrho_{2}\delta$, $\phi_{3} = (1-\wp)\varrho_{3}$, $\phi_{4} = (1-\wp)\varrho_{2}$, $\delta\in(0, 1)$, $\epsilon_{1} = \frac{\phi_{2}}{\phi_{1}}$, $\epsilon_{2} = \frac{\phi_{4}}{\phi_{3}}$, and $\epsilon_{1} > \epsilon_{2}$ with $\Delta \epsilon = \epsilon_{1}-\epsilon_{2}$, $\Xi(k-1) = (V^{1-\wp}(0)+\epsilon_{1}) \exp\big(\sum_{q = 0}^{k-1}(-\phi_{1}(t_{2q+1}-t_{2q})+\phi_{3} (t_{2q+2}-t_{2q+1}))\big)$ with $t_{-2} = t_{-1} = t_{0} = 0$, $k_{*} = \min\{k \in\mathbb{N}: \Gamma(k)\leq0\}$.

3.   Main results

Based on the Lyapunov stability theorem, the PGFETS criteria of DNs are established by utilizing intermittent controllers in this part.

Let $\varsigma^{\vartheta}_{\imath}(t) = (\varsigma^{\vartheta}_{\imath1}(t), \varsigma^{\vartheta}_{\imath2}(t), \ldots, \varsigma^{\vartheta}_{\imath n}(t))^{T} = \vartheta_{\imath}(t)-\psi(\mathfrak{s}^{\vartheta}(t))$, $\varsigma^{\omega}_{\imath}(t) = (\varsigma^{\omega}_{\imath1}(t), \varsigma^{\omega}_{\imath2}(t), \ldots, \varsigma^{\omega}_{\imath n}(t))^{T} = \omega_{\imath}(t)-\psi(\mathfrak{s}^{\omega}(t))\in \mathbb{R}^{n}$, and combine systems (2.1) and (2.2). The error systems are deduced as

where $\Pi^{\vartheta}_{\psi}, \Pi^{\omega}_{\psi}$ are the Jacobian matrices of the functions $\psi(\mathfrak{s}^{\vartheta}(t))$ and $\psi(\mathfrak{s}^{\omega}(t))$, respectively, which are defined as

The intermittent controllers are formulated as

where $\eta^{\vartheta(\omega)}_{1}$, $\eta^{\vartheta(\omega)}_{2}$, $\Re^{\vartheta(\omega)}_{1}, \Re^{\vartheta(\omega)}_{2}$ are positive constants, $\chi_{1} < \chi_{2},$ $\chi_{1}, \chi_{2}$ are positive odd constants, $\beta$ is defined as in Lemma 3, and $£^{\downarrow}_{k}$, $£^{\uparrow}_{k}$ are the same as that in Lemma $5$. Take $\Theta^{\vartheta}(t) = -\mathfrak{C}\psi(\mathfrak{s}^{\vartheta}(t)) +\mathfrak{D}\mathfrak{g}^{\vartheta}(\psi(\mathfrak{P}^{\vartheta}\mathfrak{s}^{\vartheta}(t-\sigma^{\vartheta}_{1}(t)))) +\Upsilon(\psi(\mathfrak{s}^{\omega}(t))-\psi(\mathfrak{s}^{\vartheta}(t))) -\Pi^{\vartheta}_{\psi}\big(-\mathfrak{C}\mathfrak{s}^{\vartheta}(t) +\mathfrak{D}\mathfrak{g}^{\vartheta}(\mathfrak{P}^{\vartheta}\mathfrak{s}^{\vartheta}(t-\sigma^{\vartheta}_{1}(t))) +\Upsilon(\mathfrak{s}^{\omega}(t)-\mathfrak{s}^{\vartheta}(t))\big)$, $\Theta^{\omega}(t) = -\mathfrak{C}\psi(\mathfrak{s}^{\omega}(t)) +\mathfrak{D}\mathfrak{g}^{\omega}(\psi(\mathfrak{P}^{\omega}\mathfrak{s}^{\omega}(t-\sigma^{\omega}_{1}(t)))) +\Upsilon(\psi(\mathfrak{s}^{\vartheta}(t))-\psi(\mathfrak{s}^{\omega}(t))) -\Pi^{\omega}_{\psi}\big(-\mathfrak{C}\mathfrak{s}^{\omega}(t) +\mathfrak{D}\mathfrak{g}^{\omega}(\mathfrak{P}^{\omega}\mathfrak{s}^{\omega}(t-\sigma^{\omega}_{1}(t))) +\Upsilon(\mathfrak{s}^{\vartheta}(t)-\mathfrak{s}^{\omega}(t))\big)$.

Remark 3. It is worth mentioning that compared with the intermittent control in ^[16,17], the above controllers (3.2) are without sign function, which effectively weakens the chattering phenomenon caused by the sign function. In addition, considering the quantization of information effectively reduces the control cost and alleviates the communication congestion.

Let $\bar{\mathfrak{g}}^{\vartheta}(\mathfrak{P}^{\vartheta}\varsigma^{\vartheta}_{\imath}(t-\sigma^{\vartheta}_{1}(t))) = \mathfrak{g}^{\vartheta}(\mathfrak{P}^{\vartheta}\vartheta_{\imath}(t-\sigma^{\vartheta}_{1}(t))) -\mathfrak{g}^{\vartheta}(\psi(\mathfrak{P}^{\vartheta}\mathfrak{s}^{\vartheta}(t-\sigma^{\vartheta}_{1}(t))))$, $\bar{\mathfrak{g}}^{\omega}(\mathfrak{P}^{\omega}\varsigma^{\omega}_{\imath}(t-\sigma^{\omega}_{1}(t))) = \mathfrak{g}^{\omega}(\mathfrak{P}^{\omega}\omega_{\imath}(t-\sigma^{\omega}_{1}(t))) -\mathfrak{g}^{\omega}(\psi(\mathfrak{P}^{\omega}\mathfrak{s}^{\omega}(t-\sigma^{\omega}_{1}(t))))$, $\bar{\mathfrak{F}}^{\vartheta}(\varsigma^{\vartheta}_{\jmath}(t-\sigma^{\vartheta}_{2}(t))) = \mathfrak{F}^{\vartheta}(\vartheta_{\jmath}(t-\sigma^{\vartheta}_{2}(t))) -\mathfrak{F}^{\vartheta}(\psi(\mathfrak{s}(t-\sigma^{\vartheta}_{2}(t))))$, $\bar{\mathfrak{F}}^{\omega}(\varsigma^{\omega}_{\jmath}(t-\sigma^{\omega}_{2}(t))) = \mathfrak{F}^{\omega}(\omega_{\jmath}(t-\sigma^{\omega}_{2}(t))) -\mathfrak{F}^{\omega}(\psi(\mathfrak{s}(t-\sigma^{\omega}_{2}(t)))),$ then systems (3.1) can be converted into

Theorem 1. Under Assumptions $1-2$, there exist positive constants $\varrho_{0}$, $\varrho_{1}$, $\varrho_{2}$, $\varrho_{3}$, $\varepsilon$, $\delta\in (0, 1)$, $\Re^{\vartheta(\omega)}_{1}$, $\Re^{\vartheta(\omega)}_{2}$, $r^{\vartheta(\omega)}_{i}$, $\bar{r}^{\vartheta(\omega)}_{i}(i = 1, 2)$, and a $k\in \mathbb{N}$, and the following factors are fulfilled:

where $\mathcal{G}^{\vartheta(\omega)} = (\mathcal{A}^{\vartheta(\omega)})^{T}\mathcal{A}^{\vartheta(\omega)}\otimes I_{n}$, $\varrho_{0} = \frac{nN\flat(\eta^{\vartheta}_{2}+\eta^{\omega}_{2})}{\beta}$, $\varrho_{2} = \min\{2^{\frac{\chi_{1}+\chi_{2}}{2\chi_{2}}}\eta^{\vartheta}_{1}(1-\partial)^{\frac{\chi_{1}}{\chi_{2}}}, 2^{\frac{\chi_{1}+\chi_{2}}{2\chi_{2}}}\eta^{\omega}_{1}(1-\partial)^{\frac{\chi_{1}}{\chi_{2}}}$, $\bar{r}^{\vartheta}_{1}2^{\frac{\chi_{1}+\chi_{2}}{2\chi_{2}}} (r^{\vartheta}_{1}\exp(\varrho_{1}\sigma^{\vartheta}_{1}))^{-\frac{\chi_{1}+\chi_{2}}{2\chi_{2}}}$, $\bar{r}^{\vartheta}_{2}2^{\frac{\chi_{1}+\chi_{2}}{2\chi_{2}}} (r^{\vartheta}_{2}\exp(\varrho_{1}\sigma^{\vartheta}_{2}))^{-\frac{\chi_{1}+\chi_{2}}{2\chi_{2}}}$, $\bar{r}^{\omega}_{1}2^{\frac{\chi_{1}+\chi_{2}}{2\chi_{2}}} (r^{\omega}_{1}\exp(\varrho_{1}\sigma^{\omega}_{1}))^{-\frac{\chi_{1}+\chi_{2}}{2\chi_{2}}}$, $\bar{r}^{\omega}_{2}2^{\frac{\chi_{1}+\chi_{2}}{2\chi_{2}}} (r^{\omega}_{2} \exp(\varrho_{1}\sigma^{\omega}_{2}))^{-\frac{\chi_{1}+\chi_{2}}{2\chi_{2}}}\}$. Moreover, $\Im^{\downarrow}_{k}$, $\Im^{\uparrow}_{k}$, $\phi_{1}$, $\phi_{2}$, $\phi_{3}$, $\phi_{4}$, $\epsilon_{1}$, $\epsilon_{2}$, $\Delta\epsilon$, $\Xi(k-1)$, $k_{*}$ are defined as those in Lemma 5 and $\wp = \frac{\chi_{1}+\chi_{2}}{2\chi_{2}}$. Then, networks (2.1) and networks (2.2) are able to achieve PGFETS through the controllers (3.2), and the settling time is $\mathcal{T}_{f} = t_{2k_{*}}+\frac{1}{\phi_{1}}\ln\big(\frac{1}{\Delta \epsilon} \Xi(k_{*}-1)+1\big)$.

Proof. Construct the Lyapunov functional as:

When $t\in £^{\downarrow}_{k}$, differentiating $V_{1}(t)$ yields

It's obvious to gain

By Assumption 1 and Lemma 1, it has

Moreover, it derives from Lemma 1 that

where $\varsigma(t) = (\varsigma^{T}_{1}(t), \varsigma^{T}_{2}(t), \ldots, \varsigma^{T}_{N}(t))^{T}$.

Same as above, a simple derivation yields

It's not hard to get

Employing Lemma 2 and $0 < \frac{\chi_{1}+\chi_{2}}{2\chi_{2}} < 1,$ it follows that

By simple calculation via Lemma 3, we get

The inequalities (3.13)–(3.19) guarantee that

Similar to $V_{1}(t)$,

Next, take the derivative of $V_{3}(t)$, which reaches that

Then, by Assumption 2 and Lemma 4, we get

Combining $(3.22)$ and $(3.23)$ yields

With the same analysis as above, we obtain

Refer to conditions (3.5)–(3.7), formulas (3.20)–(3.21) and (3.24)–(3.27), we have

With the same analytical procedure as above, for $t\in £^{\uparrow}_{k}$, in view of conditions (3.8)–(3.10), it is granted that

In view of Lemma 5, condition (3.4), and inequalities (3.28)–(3.29), one has $V(t)\leq \big(\frac{\varrho_{0}}{\varrho_{2}(1-\delta)}\big)^{\frac{2\chi_{2}}{\chi_{1}+\chi_{2}}},$ then $V_{1}(t)\leq \big(\frac{\varrho_{0}}{\varrho_{2}(1-\delta)}\big)^{\frac{2\chi_{2}}{\chi_{1}+\chi_{2}}}$ and $V_{2}(t)\leq \big(\frac{\varrho_{0}}{\varrho_{2}(1-\delta)}\big)^{\frac{2\chi_{2}}{\chi_{1}+\chi_{2}}},$ i.e., $\|\varsigma^{\vartheta}_{\imath}(t)\|\leq \sqrt{2}(\frac{\varrho_{0}}{\varrho_{2}(1-\delta)})^{\frac{\chi_{2}}{\chi_{1}+\chi_{2}}}$, $\|\varsigma^{\omega}_{\imath}(t)\|\leq \sqrt{2}(\frac{\varrho_{0}}{\varrho_{2}(1-\delta)})^{\frac{\chi_{2}}{\chi_{1}+\chi_{2}}}, \imath\in \mathcal{N}$ for $t > \mathcal{T}_{f}$. It follows from Definition 1 that there is a settling time $\mathcal{T}_{f}$ at which the PGFETS of systems (2.1) and (2.2) is ensured. This concludes the proof. □

Remark 4. Note that the two-layer networks have been studied in ^[6,7], and they belong to the problem of finite time synchronization for the error system, i.e., $\lim\limits_{t\rightarrow \mathcal{T}_{f}}V(t) = 0$, $\mathcal{T}_{f}$ is a settling time. Nevertheless, real systems cannot be strictly synchronized by various external factors, and the system states may oscillate in a certain region. Hence, the results in this paper are more consistent with real systems.

Corollary 1. Suppose Assumptions 1–2 hold if the mapping function $\psi(\mathfrak{s}^{\vartheta(\omega)}(t)) = \mathcal{H}\mathfrak{s}^{\vartheta(\omega)}(t),$ where $\mathcal{H}$ is a nonzero constant. Given positive constants $\varrho_{0}$, $\varrho_{1}$, $\varrho_{2}$, $\varrho_{3}$, $\varepsilon$, $\delta\in (0,1)$, $\Re^{\vartheta(\omega)}_{1}$, $\Re^{\vartheta(\omega)}_{2}$, $r^{\vartheta(\omega)}_{i}$, $\bar{r}^{\vartheta(\omega)}_{i}(i = 1,2)$, and a $k\in \mathbb{N}$ such that (3.4)–(3.10) hold, the parameters are similar to those in Theorem 3.1. Then, DNs (2.1) and (2.2) under controllers (3.2) can achieve finite-time projective synchronization. Moreover, it is a special case when $\mathcal{H} = -1$, and the finite-time anti-synchronization is reached.

4.   Numerical example

A numerical simulation is cited to corroborate the validity of Theorem 3.1. Take $n = m = 3, N = 5$, the time step is 0.001, and consider the systems (2.2) with $\mathfrak{s}^{\vartheta}(0) = (0.8,-0.5,0.4)^{T},$ $\mathfrak{s}^{\omega}(0) = (-0.2,-0.3,0.8)^{T}.$ $\sigma^{\vartheta}_{1}(t) = \sigma^{\omega}_{1}(t) = 0.01$. One has $\sigma^{\vartheta}_{1} = \sigma^{\omega}_{1} = 0.01, \bar{\sigma}^{\vartheta}_{1} = \bar{\sigma}^{\omega}_{1} = 0$. $\mathfrak{g}^{\vartheta}(\mathfrak{x}) = (\tanh(\mathfrak{x}_{1}),\sin(\mathfrak{x}_{2}),-1.5\tanh(\mathfrak{x}_{3}))^{T}$, while $\mathfrak{g}^{\omega}(\mathfrak{x}) = (\tanh(\mathfrak{x}_{1}),\sin(\mathfrak{x}_{2}),-2.5\tanh(\mathfrak{x}_{3}))^{T}$ for $\mathfrak{x} = (\mathfrak{x}_{1}, \mathfrak{x}_{2}, \mathfrak{x}_{3})^{T},$ thus Assumption 1 is satisfied with $\mathcal{L}^{\vartheta} = 1.5, \mathcal{L}^{\omega} = 2.5.$ $\mathfrak{C} = \mbox{diag}(1.9,1.5,1)$, $\Upsilon = \mbox{diag}(1,1,1)$, $\mathfrak{P}^{\vartheta} = \mbox{diag}(1,1,1)$,$\mathfrak{P}^{\omega} = \mbox{diag}(1,0,1),$ and

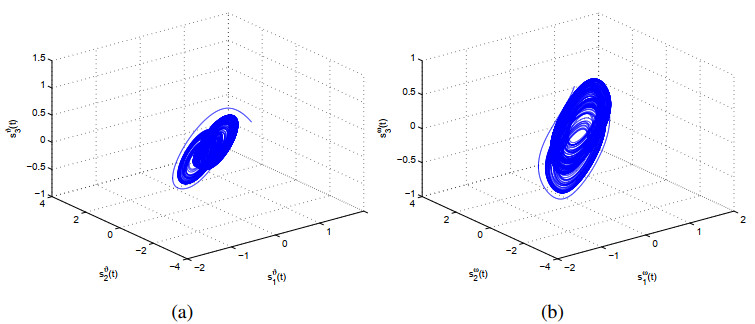
The trajectories of DNs (2.2) are demonstrated in Figure 1. Obviously, they are both chaotic.

In this paper, we consider DNs (2.1) with five nodes, the parameters are taken as $\rho^{\vartheta} = 5$, $\rho^{\omega} = 6$, $\sigma^{\vartheta}_{2}(t) = \sigma^{\omega}_{2}(t) = 0.02$ with $\sigma^{\vartheta}_{2} = \sigma^{\omega}_{2} = 0.02, \bar{\sigma}^{\vartheta}_{2} = \bar{\sigma}^{\omega}_{2} = 0$,

and others are consistent with systems (2.2). Then, we consider the PGFETS between DNs (2.1) and (2.2) with $\psi(\mathfrak{s}^{\vartheta(\omega)}(t)) = ((\mathfrak{s}_{1}^{\vartheta(\omega)}(t))^{2}, (\mathfrak{s}_{2}^{\vartheta(\omega)}(t))^{2}, (\mathfrak{s}_{3}^{\vartheta(\omega)}(t))^{2})^{T}.$ There is a sequence $\{t_{k}\}_{k\in \mathbb{N}}$ satisfying the first control width $t_{2k+1}-t_{2k} = 0.15$, and the second control width $t_{2k+2}-t_{2k+1} = 0.05$. The time sequence is depicted in Figure 2, where the different control intervals are presented alternately. The control parameters of DNs (2.1) are chosen as $\chi_{1} = 1, \chi_{2} = 3$, $\eta^{\vartheta}_{1} = \eta^{\omega}_{1} = \eta^{\vartheta}_{2} = \eta^{\omega}_{2} = 2$. Let $\gamma_{0} = 1$, $\ell = 0.6$, $\delta = 0.65$, $\varepsilon = 5$, $\beta = 4000$, then Assumption 2 is satisfied. In Theorem 3.1, we select $\varrho_{1} = 10$, $\varrho_{3} = 16$, and take $r^{\vartheta}_{1} = 51$, $r^{\omega}_{1} = 141$, $r^{\vartheta}_{2} = 1.5$, $r^{\omega}_{2} = 0.5$, $\bar{r}^{\vartheta}_{1} = 0.9$, $\bar{r}^{\omega}_{1} = 1$, $\bar{r}^{\vartheta}_{2} = 0.6$, $\bar{r}^{\omega}_{2} = 0.3$, $\Re^{\vartheta}_{1} = 48.6$, $\Re^{\omega}_{1} = 115$, $\Re^{\vartheta}_{2} = 32$, $\Re^{\omega}_{2} = 98$. Then, by a simple calculation, one has $\varrho_{0} = 0.0042$, $\varrho_{2} = 0.0548$, the left side of formula (3.5) is less than $0$, and the condition (3.5) is satisfied. Similarly, conditions (3.6)–(3.10) hold. From above, we can get $\phi_{1} = 3.3333, \phi_{2} = 0.0119, \phi_{3} = 5.3333, \phi_{4} = 0.0183$, then $\epsilon_{1} = 0.0036$, $\epsilon_{2} = 0.0034$, that is, $\epsilon_{1} > \epsilon_{2}$ is satisfied. In addition, one has $\Gamma(48) = -1.4269 \times10^{-6} < 0$, satisfying condition (3.4). Then, Figure 3 shows the trajectories of $\|\varsigma^{\vartheta}_{\imath}(t)\|$ and $\|\varsigma^{\omega}_{\imath}(t)\|\; (\imath = 1, 2, 3, 4, 5)$. It can be seen that unlike other results in previous literatures, Figure 3 shows that after a period of time, $\|\varsigma^{\vartheta}_{\imath}(t)\|$ and $\|\varsigma^{\omega}_{\imath}(t)\|$ will converge to a neighborhood near the origin instead of the origin. This means that the systems achieve PGFETS by Definition 1. Further, it concludes that DNs (2.1) with controllers (3.2) can be synchronized into networks (2.2) within $\mathcal{T}_{f} = 9.7497.$ By Lemma 5, it has that $V(t)\leq 0.1016$, then $V_{1}(t)\leq 0.1016$ and $V_{2}(t)\leq 0.1016$. As a result, $\|\varsigma^{\vartheta}_{\imath}(t)\|\leq \mathfrak{W} = 0.4509, \|\varsigma^{\omega}_{\imath}(t)\|\leq \mathfrak{W} = 0.4509$ for $t > \mathcal{T}_{f}$. In other words, the PGFETS of DNs (2.1) and (2.2) is achieved.

5.   Conclusions

To sum up, this brief considers the PGFETS for a class of DNs with quantized and delayed couplings. Via the finite-time stability theory, the PGFETS is proposed. Based on the Lyapunov functionals and 2-norm, some synchronization criteria have been established by adopting quantized intermittent control methods without sign function. Here, those criteria guarantee the DNs can be synchronized within a neighborhood of the origin in a finite time, which is different from some existing results. The introduction of quantizer effectively alleviates the communication burden and reduces control cost. Moreover, the designed controllers minimize the effects of chattering generated by the sign function. Finally, numerical simulations demonstrate the correctness and reasonableness of the new results. Note that DNs can be expanded to multiplex networks which can be used to describe more complex real systems. In the future, our research direction is to extend our proposed criteria to multiplex networks.

Author contributions

Ting Yang: Investigation, Writing-original draft, Writing-review & editing; Li Cao: Software, Validation; Wanli Zhang: Methodology, Writing-review & editing, Supervision. All authors have read and approved the final version of the manuscript for publication.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

This work was jointly supported by the National Natural Science Foundation of China (NSFC) (Grant No. 62003065), Natural Science Foundation of Chongqing, China(Grant No. CSTB2023NSCQ-MSX0771), the Science and Technology Research Program of Chongqing Municipal Education Commission of China (Grant No. KJZD-K202300502).

Conflict of interest

The authors declare no conflicts of interest in this paper.