Bivariate $\lambda$-Bernstein operators on triangular domain

1.   Introduction

Bernstein polynomials and their associated operators have been a cornerstone of the approximation theory, attracting significant research attention in recent years. Researchers have explored diverse generalizations and extensions of these operators, establishing their approximation properties ^{[1,2,3,4,5,6]}.

In 1963, Stancu ^[7] introduced a collection of bivariate Bernstein operators denoted as $B_m(f; x, y)$, designed for functions $f: S \rightarrow \mathbb{R}$:

where $S$ represents the triangular domain $\{(x, y) | x, y \geq 0, x+y\leq 1\}$, and $p_{m, i, j}(x, y)$ are the associated bivariate Bernstein basis functions:

Since then, researchers have actively explored various generalizations of Bernstein-type operators to the triangular domain.

In 2018, Cai et al. ^[8] introduced a novel generalization of Bernstein operators termed $\lambda$-Bernstein operators, defined as:

where $\tilde{b}_{n, k}(\lambda; x)$ represent Bézier basis functions with a shape parameter $\lambda \in [-1, 1]$ ^[9]:

where $b_{n, k}(x) = \binom{n}{k}x^k(1-x)^{n-k}, \ (k = 0, 1, \dots, n)$ are the Bernstein basis functions. This generalization reduces to the classical Bernstein operators, $B_{n}(f; x)$, when $\lambda = 0$. Recent years have witnessed a surge in interest surrounding $\lambda$-Bernstein operators $B_{n, \lambda}(f; x)$ ^{[10,11,12,13,14,15,16]}, attracting numerous researchers to explore their properties and applications.

The main purpose of this paper is to construct and study the approximation properties of bivariate $\lambda$-Bernstein operators on the triangular domain $S$. The paper is organized as follows: In Section 2, we present a novel class of bivariate Bézier basis functions on triangular domain $S$ with shape parameters $\lambda_1$ and $\lambda_2$, denoted by $p_{m, i, j}^{(\lambda_1, \lambda_2)}(x)$. These functions serve as the foundation for constructing a new kind of bivariate $\lambda$-Bernstein operators, $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$. In Section 3, we delve into the fundamental properties of $p_{m, i, j}^{(\lambda_1, \lambda_2)}(x)$, including nonnegativity, partition of unity, and endpoint properties. Furthermore, we present estimations for the moments and central moments of $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$. Section 4 establishes theoretical results pertaining to the approximation capabilities of $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$, including the Korovkin-type approximation theorem, the rate of convergence of $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$, the local approximation theorem, and a Voronovskaja type theorem. In Section 5, we provide empirical evidence for the convergence behavior through graphical representations and numerical examples, showcasing how $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$ approaches $f(x, y)$ under various parameter configurations. Finally, we summarize the key findings of this paper in Section 6.

2.   Construction of new bivariate $\lambda$-Bernstein operators on triangular domain

Building upon the construction of Bézier basis functions with a shape parameter $\lambda$ presented in ^[9] and the bivariate Bernstein basis functions on triangular domain defined in (1.2), we introduce a novel class of bivariate Bézier basis functions on the triangular domain $S$ with shape parameters $\lambda_1$ and $\lambda_2$ as follows:

where $\Lambda_{i, j}^1$ and $\Lambda_{i, j}^2$ are given by

with $|\lambda_1|\leq \frac12, \ |\lambda_2|\leq\frac12$. Motivated by the newly introduced class of bivariate Bézier basis functions $p_{m, i, j}^{(\lambda_1, \lambda_2)}(x, y)$, we formally define the associated bivariate $\lambda$-Bernstein operators on the triangular domain.

Definition 2.1. Let $f\in C[S]$ and $|\lambda_1|\leq \frac12, \ |\lambda_2|\leq\frac12$. The bivariate $\lambda$-Bernstein operators on the triangular domain $S$ are defined as:

where $p_{m, i, j}^{(\lambda_1, \lambda_2)}(x, y)$ are the new bivariate Bézier basis functions on triangular domain defined in (2.1).

Remark 2.1. It is noteworthy that when $\lambda_1 = \lambda_2 = 0$, the operators $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$ coincide with the classical bivariate Bernstein operators on triangular domain $B_m(f; x, y)$ defined in (1.1).

3.   Some preliminary results

Prior to delving into the properties of $p_{m, i, j}^{(\lambda_1, \lambda_2)}(x, y)$, it is beneficial to recall the key characteristics of their classical counterparts, the bivariate Bernstein basis functions $p_{m, i, j}(x, y)$.

● Nonnegativity:

● Partition of Unity:

● Endpoint properties:

We can demonstrate that $p_{m, i, j}^{(\lambda_1, \lambda_2)}(x, y)$ inherit these fundamental properties.

Theorem 3.1. The bivariate Bézier basis function on triangular domain with shape parameters $\lambda_1$ and $\lambda_2$, $p_{m, i, j}^{(\lambda_1, \lambda_2)}(x, y)$, as defined in (2.1), have the following properties:

● Nonnegativity:

● Partition of Unity:

● Endpoint properties:

Proof. (Nonnegativity) Within the triangular domain $S$, we can leverage (1.2) and (2.1) to express $p_{m, i, j}^{(\lambda_1, \lambda_2)}(x, y)$ as:

For $i\geq 1, \ j\geq 1$, and $i+j\leq m-1$ cases, since $|\lambda_1| \leq \frac12, |\lambda_2|\leq \frac12,$ we have

For $i = 0$ or $j = 0$, and $i+j\leq m-1$ cases, we have

For $i+j = m$ cases, we have

Therefore, $p_{m, i, j}^{(\lambda_1, \lambda_2)}(x, y) \geq 0$ for all $(x, y)\in S$ and $0\leq i+j\leq m$, proving the nonnegativity.

(Partition of Unity)

We can rearrange with common indices, letting $i' = i+1, \ j' = j+1$.

Therefore, we obtain the partition of unity property of $p_{m, i, j}^{(\lambda_1, \lambda_2)}(x, y)$.

(Endpoint properties) The endpoint properties of $p_{m, i, j}^{(\lambda_1, \lambda_2)}(x, y)$ can be directly inherited from the corresponding properties of $p_{m, i, j}(x, y)$ due to their construction and relationship.

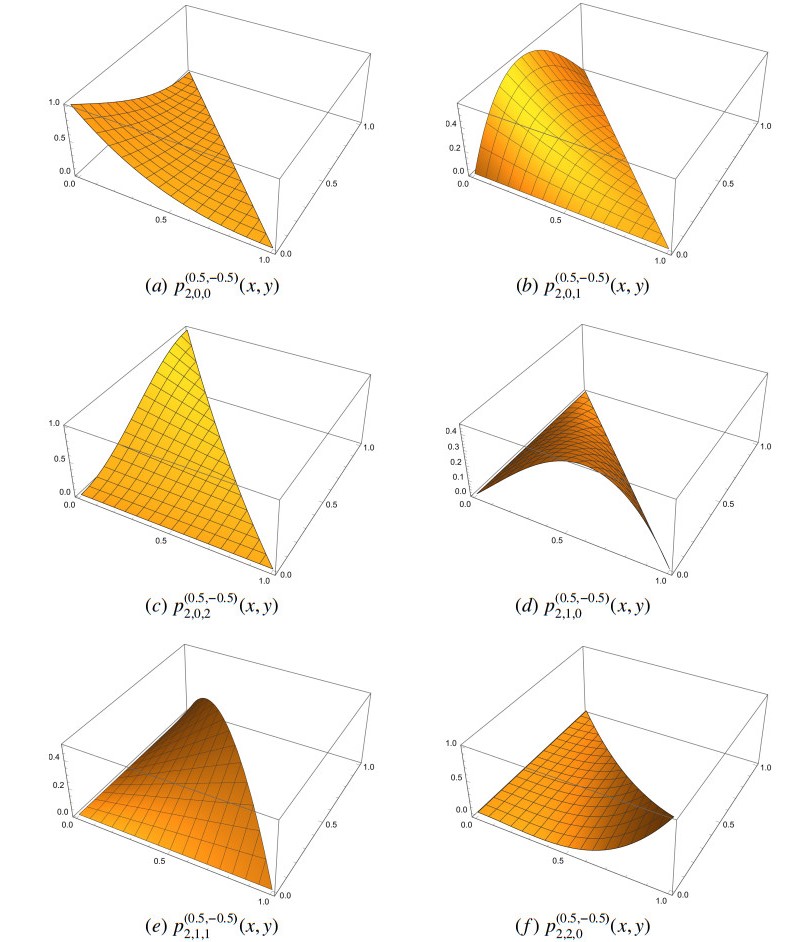
Figure 1 presents the graphs of $p_{m, i, j}^{(\lambda_1, \lambda_2)}(x, y)$ for $m = 2, \ \lambda_1 = 0.5$, and $\lambda_2 = -0.5$. These visualizations effectively illustrate the nonnegativity and endpoint properties of $p_{m, i, j}^{(\lambda_1, \lambda_2)}(x, y)$.

Remark 3.1. Building upon the nonnegativity and partition of unity of $p_{m, i, j}^{(\lambda_1, \lambda_2)}(x, y)$, we can further deduce that the associated operators $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$ possess the characteristic of linear positivity.

Lemma 3.1. Let $f(s, t) = s^k t^l = e_{kl}, \; (s, t)\in S$, where $k, l\in \mathbb{N} ~~ \mathit{\text{and}}~~ 0\leq k+l \leq 4$, then for the bivariate $\lambda$-Bernstein operators on triangular domain $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$, we have the following equalities:

The conclusions of Lemma 3.1 can be derived via more intuitive calculations, hence we omit the proof process here. Based on Lemma 3.1, we present the following Lemma 3.2, established through direct calculations.

Lemma 3.2. Let $f(s, t) = (s-x)^k(t-y)^l = h_{kl}$, where $k, l \in \mathbb{N} ~~ \mathit{\text{and}}~~ 1\leq k+l \leq 2$, then for the bivariate $\lambda$-Bernstein operators on triangular domain $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$, we have the following equalities:

Corollary 3.1. Consider a fixed point $(x, y)\in S$. Under $|\lambda_1|\leq \frac12$ and $|\lambda_2|\leq \frac12$, we have

Since

when $(x, y)\in S$, combining (3.11), (3.13), and $|\lambda_1| \leq \frac12$, we obtain the upper bounds for $B_{m}^{\lambda_1, \lambda_2}((s-x); x, y)$ and $B_{m}^{\lambda_1, \lambda_2}((s-x)^2; x, y)$.

Similar steps yield the bounds for $B_{m}^{\lambda_1, \lambda_2}((t-y); x, y)$ and $B_{m}^{\lambda_1, \lambda_2}((t-y)^2; x, y)$.

Remark 3.2. By leveraging the endpoint properties of $p_{m, i, j}^{(\lambda_1, \lambda_2)}(x, y)$, we can readily deduce the endpoint interpolation properties of $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$.

4.   Convergence properties

We begin by presenting a Korovkin-type approximation result for the bivariate $\lambda$-Bernstein operators on triangular domain $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$.

Theorem 4.1. For any $f\in C[S]$ and $|\lambda_1|\leq \frac12, \ |\lambda_2|\leq \frac12$, the bivariate $\lambda$-Bernstein operators on triangular domain $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$ converge uniformly to $f$ on $S$.

Proof. Due to the linearity and positivity of $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$, Lemma 3.1 guarantees that for all $(x, y)\in S$,

all holding uniformly. Applying the Korovkin-Type theorem from ^[17], it follows that $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$ converges uniformly to $f$ on $S$ for any $f\in C[S]$.

Next, we analyze the convergence rate of $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$ approaching to $f(x, y)$. We begin by introducing several smoothness moduli for bivariate functions from ^[18]. For functions $f\in C[S]$,

● Complete modulus of continuity:

● Modulus of continuity:

● Partial moduli of continuity:

where $\alpha > 0, \beta > 0, \delta > 0$. The following inequalities hold for the aforementioned smoothness moduli (cf. ^[18]):

when $|x_1 - x_2|\leq \alpha, |y_1-y_2| \leq \beta$.

Theorem 4.2. For any $f\in C[S]$ and $|\lambda_1|\leq \frac12, \ |\lambda_2|\leq \frac12$, we have

Proof. Consider the nonnegativity of $p_{m, i, j}^{(\lambda_1, \lambda_2)}(x, y)$ and $B_{m}^{\lambda_1, \lambda_2}(1; x, y) = 1$. This implies

By the inequalities (4.2) and (4.4),

for $\alpha, \ \beta > 0$. Thus,

Applying Cauchy-Schwarz inequalities, we get

Hence,

Setting $\alpha = \sqrt{\psi_m(x, y)}, \beta = \sqrt{\psi_m(y, x)}$ yields the desired result

Theorem 4.3. For any $f\in C[S]$ and $|\lambda_1|\leq \frac12, \ |\lambda_2|\leq \frac12$, we have

Proof. To begin, we have

Utilizing the inequalities (4.1), (4.3), and (4.4), we get

According to (4.6) and (4.7), we obtain

Setting $\alpha = \sqrt{\psi_m(x, y)}, \beta = \sqrt{\psi_m(y, x)}$ yields the desired result

For $f\in C[S]$, the Peetre's K-functional measures smoothness and is defined as:

where

● $C^2[S] : = \{f\in C[S]: f^{(i, j)}\in C[S], 0\leq i+j\leq 2\}$, $f^{(i, j)}$ is $(i, j)$th-order partial derivative with respect to $x, y$ of $f$.

● $\|f\| = \sup_{(x, y)\in S}|f(x, y)|$.

● $\|f\|_{C^2[S]} = \|f\| + \sum_{i = 1}^2\left(\left\|\frac{\partial^i f}{\partial x^i}\right\| + \left\|\frac{\partial^i f}{\partial y^i}\right\| \right)$.

It is known (^[19], page 192) that a constant $C > 0$ exists such that

for all $f\in C[S]$, where $\omega_2(f; \sqrt{\delta})$ is the second order modulus of continuity. This property lays the groundwork for a local approximation theorem for $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$.

Theorem 4.4. For any $f\in C[S]$ and $|\lambda_1|\leq \frac12, \ |\lambda_2|\leq \frac12$, we have

where $\Theta_m(x, y) = \psi_m(x, y) + \phi_m^2(x, y) + \psi_m(y, x) + \phi_m^2(y, x)$.

Proof. Let

Define the auxiliary operators

From Lemma 3.1, we have

For $g\in C^2[S]$ and $(s, t)\in S$, Taylor's expansion yields

Applying operator $\bar{B}_{m}^{\lambda_1, \lambda_2}$ to both sides of (4.12), we get

Applying the triangle inequality and (3.23)–(3.26), we obtain

On the other hand, we have

Therefore,

Taking the infimum on the righthand side over all $g\in C^2[S]$, we obtain

Therefore, Theorem 4.4 is proved.

Theorem 4.5. For any $f\in C^1[S]$, we have

Proof. For any fixed point $(x, y)\in S$, we have

Applying the operator $B_{m}^{\lambda_1, \lambda_2}$ to both sides of (4.14), we get

Since

we obtain

Therefore, Theorem 4.5 is proved.

Theorem 4.6. For any $f\in C^2[S]$, we have the following Voronovskaja-type asymptotic formula:

uniformly in $S$.

Proof. Let $(x, y)\in S$ be fixed. Utilizing the Taylor formula, we get

where $r(s, t;x, y)$ is the Peano form of the remainder satisfying

Applying operators $B_{m}^{\lambda_1, \lambda_2}$ to both sides of (4.16), we obtain

By the Cauchy-Schwarz inequality, we get

Hence, combining (3.21), (3.22), and (4.17), we obtain

uniformly in $S$. By using Corollary 3.1 and (4.18), we obtain

uniformly in $S$. This concludes the proof.

5.   Graphical and numerical analysis

This section numerically illustrates the convergence behavior of the bivariate $\lambda$-Bernstein operators on triangular domain $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$.

Let $f(x, y) = x^2 - \sqrt{7}(1-x-y)^2 - 10xy$. Figure 2 displays graphs of $f(x, y)$ (yellow) and its approximations by $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$ (blue) for varying values of $m$ with $\lambda_1 = 0.5, \lambda_2 = -0.5$. Table 1 shows the corresponding approximation errors, clearly decreasing with increasing $m$. This confirms the convergence of the operators.

We investigate the function $f(x, y) = \sin(4x) + \cos(7y)$. Figure 3 displays graphs of $f(x, y)$ (yellow) and its approximations by $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$ (blue) with $m = 10$ and different values of $\lambda_1$ and $\lambda_2$. Table 2 shows the approximation errors of $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$ to $f(x, y)$ for $m = 10$ and various parameter combinations $(\lambda_1, \lambda_2)$. Similarly, Table 3 presents the errors for the function $f(x, y) = \sin(4(1-x-y)) + \cos(7xy)$ under identical conditions.

Table 3 reveals that the bivariate $\lambda$-Bernstein operators on triangular domain $B_{10}^{\lambda_1, \lambda_2}(f; x, y)$ outperform the standard bivariate Bernstein operators on triangular domain $B_{10}^{0, 0}(f; x, y)$ in certain cases. For example, when $\lambda_1 = \lambda_2 = -0.25 \text{ or } -0.5$, the $L^{\infty}$ error norm $\|B_{10}^{\lambda_1, \lambda_2}(f; x, y)-f(x, y)\|_{\infty}$ is smaller than that of $B_{10}^{0, 0}(f; x, y)$. This observation highlights the potential of $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$ to achieve better approximation accuracy in specific scenarios.

6.   Conclusions

This paper presents a novel construction of bivariate Bézier basis functions with shape parameters $\lambda_1$ and $\lambda_2$ on a triangular domain. These functions exhibit key properties like nonnegativity, partition of unity and endpoint properties. Building upon them, we introduce the new bivariate $\lambda$-Bernstein operators on triangular domain $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$, encompassing the classical bivariate Bernstein operators on triangular domain as a special case ($\lambda_1 = \lambda_2 = 0$).

We derive explicit estimates for the moments and central moments of $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$ through direct calculations. Furthermore, we establish the Korovkin-type approximation theorem for these operators. Additionally, we estimate the rate of convergence of $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$ toward $f(x, y)$, and rigorously prove both the local approximation theorem and the Voronovskaja-type asymptotic formula for this family of operators.

Numerical examples and diverse graphical representations showcase the convergence behavior of $B_{m}^{\lambda_1, \lambda_2}(f; x, y)$ under various parameter configurations. Notably, in specific scenarios, these operators achieve superior approximation accuracy compared to the standard bivariate Bernstein operators on triangular domain $B_{m}(f; x, y)$, highlighting their potential for tailored applications.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

This work is supported by the Natural Science Foundation of Fujian Province of China (Grant No. 2021J011192) and the Fujian Provincial Office of Education Project (Grant No. JAT200494).

Conflict of interest

All authors declare no conflicts of interest in this paper.