Approximation properties of generalized Baskakov operators

1.   Introduction

Chen et al. ^[14] introduced a generalization of Bernstein polynomials by means of a parameter $\alpha,$ where $0\leq\alpha \leq 1,$ and studied some approximation properties. Subsequently, the modifications and the generalizations of the operators introduced in ^[14] were extensively studied in many papers ^{[3,4,5,8,19,20,21,22,24]}. Inspired by these studies, Aral and Erbay ^[1] proposed $\alpha-$ Baskakov operators as follows:

For $\gamma > 0$ and $\varphi\in C_\gamma(S): = \bigg\{\varphi\in C(S): |\varphi(r)|\leq M(1+r^\gamma), \; for\; some\; M > 0 \bigg\},$ where $S: = [0, \infty),$ the parametric generalization of Baskakov operators is given by

where $m\geq 1, \; x\in S$ and

with $\binom{m-3}{-2} = \binom{m-2}{-1} = 0.$ The authors ^[1] studied some approximation theorems in weighted spaces and a Voronovskaya type asymptotic theorem. Further, they established a representation of these operators in terms of the divided differences.

We observe that for $\alpha = 1,$ the operators (1.1) reduce to the classical Baskakov operators.

The purpose of the present paper is to investigate the degree of approximation for the operators given by (1.1) by means of the Peetre's K-functional and the Ditzian Totik modulus of smoothness. Also, we construct a bivariate case of these operators and determine the order of convergence by means of the moduli of continuity and the Peetre's K-functional. The Voronovskaya type asymptotic theorem is also established. Further, we propose the associated GBS operators and consider their rate of convergence with the aid of the mixed modulus of smoothness. We also add some numerical examples to validate the theoretical findings and compare the convergence of the operators (1.1) with the corresponding GBS operators.

2.   Preliminaries

Lemma 1. ^[1] For $m\in \mathbb{N},$ the $\alpha-$Baskakov operators $\mathcal{J}_{m, \alpha}(\cdot; x)$ verify the following identities:

(a) $\mathcal{J}_{m, \alpha}(1;x) = 1;$

(b) $\mathcal{J}_{m, \alpha}(r; x) = x+x(\alpha-1)\frac{2}{m};$

(c) $\mathcal{J}_{m, \alpha}(r^2;x) = x^2+x^2(4\alpha-3)\frac{1}{m}+x(m+4\alpha-4)\frac{1}{m^2}.$

By a simple computation, it follows that

(d) $\mathcal{J}_{m, \alpha}(r^3;x) = x^3+x^3(6m\alpha-3m+6\alpha-4)\frac{1}{m^2}+x^2(18\alpha+3m-15)\frac{1}{m^2}+x(8\alpha+m-8)\frac{1}{m^3};$

(e) $\mathcal{J}_{m, \alpha}(r^4;x) = x^4+\{(8\alpha-2)m^2+x^4(24\alpha-13)m+(16\alpha-10)\}\frac{1}{m^3}+x^3\{6m^2+(48\alpha-30)m+(48\alpha-36)\}\frac{1}{m^3}+x^2\{(4\alpha+3)m+60\alpha-53\}\frac{1}{m^3}+x(16\alpha+m-16)\frac{1}{m^4}.$

Consequently, we obtain the following result:

Lemma 2. ^[1] The operators $\mathcal{J}_{m, \alpha}(\cdot; x)$ given by (1.1) satisfy the following equalities:

(a) $\mathcal{J}_{m, \alpha}((r-x); x) = \frac{2}{m}(\alpha-1)x;$

(b) $\mathcal{J}_{m, \alpha}((r-x)^2;x) = \frac{1}{m}(1+x)x+\frac{4}{m^2}(\alpha-1)x;$

(c) $\mathcal{J}_{m, \alpha}((r-x)^4;x) = \frac{3}{m^2}(1+x)^2 x^2-\frac{1}{m^3}(10x^4+36x^2+25x-1)x+\frac{1}{m^3}\alpha(16x^2+48x+32)+\frac{1}{m^4}16(\alpha-1)x.$

Remark 1. For the operators $\mathcal{J}_{m, \alpha}(\cdot; x)$ defined by (1.1), we have

(a) $\underset{m\to \infty}{\lim}\; m\mathcal{J}_{m, \alpha}((r-x); x) = 2(\alpha-1)x;$

(b) $\underset{m\to \infty}{\lim}\; m\mathcal{J}_{m, \alpha}((r-x)^2;x) = (1+x)x;$

(c)$\underset{m\to \infty}{\lim}\; m^2\mathcal{J}_{m, \alpha}((r-x)^4;x) = 3x^2(1+x)^2.$

Remark 2. From Lemma 2, it follows that for every $x\in S$ and $m\in \mathbb{N},$

Further, for each $x\in S$ and sufficiently large $m,$

where $C$ is a positive constant independent of $m.$

Let $C_{2}^{*}(S)$ be the subspace of $C_\gamma(S)$, for $\gamma = 2$, defined as follows:

3.   Approximation properties of the operators $\mathcal{J}_{m, \alpha}$

Theorem 1. Let $\varphi\in C_{2}^{*}(S)$. Then $\underset{m\to \infty}{\lim}\mathcal{J}_{m, \alpha}(\varphi; x) = \varphi(x),$ uniformly on each compact subset of $S$.

Proof. From Lemma $1$, $\underset{m\to \infty}{\lim}\mathcal{J}_{m, \alpha}(r^i; x) = x^i, \; i = 0, 1, 2,$ uniformly on each compact subset of $S.$ Applying the Korovkin type theorem (^[2], Thm. 4.1.4 (vi), p.199), the required result is immediate. Let $C_B(S)$ be the space of all real valued bounded continuous functions $\varphi$ on $S,$ endowed with the norm

For $\varphi\in \overline{C}_B(S): = \{\varphi\in C_B(S): \varphi \; {\rm{is\; uniformly\; continuous\; on}}\; S\},$ the usual modulus of continuity of $\varphi$ is defined as

and the Peetre's K-functional is defined by

where $C^2_B(S) = \{f\in C_B(S): f', f''\in C_B(S)\}.$ For each $\varphi\in \overline{C}_B(S),$ by ^[15] there exists an absolute constant $M$ such that

where $\omega_2(\varphi, \sqrt{\eta}) = \underset{0 < |h|\leq \sqrt{\eta}}\sup\; \underset{x, x+2h\in S}\sup|\varphi(x+2h)-2\varphi(x+h)+\varphi(x)|$ is the second-order modulus of continuity of $\varphi$ on $S.$

Theorem 2. Let $\varphi\in \overline{C}_B(S).$ Then for every $x\in S,$ the following inequality holds:

where $M$ is an absolute positive constant and

Proof. First, we define an auxiliary operator

Applying Lemma 1, it is seen that

Let $f\in C^2_B(S).$ From Taylor's formula, we may write

Applying the operators $\mathcal{L}_{m, \alpha}(\cdot; x)$ on the above equation and using (3.3), we get

or,

which implies that

Now, using the fact that

from Remark 2, it follows that

and

Hence,

From (3.2), for every $\varphi\in \overline{C}_B(S)$ we have

Hence from (3.2), (3.5) and (3.6), for $\varphi\in \overline{C}_B(S)$ and any $f\in C^2_B(S),$ we obtain

Now, taking the infimum on the right hand side over all $f\in C^2_B(S),$ we get

Hence, in view of (3.1), we obtain

which completes the proof.

Lipschitz type space: For $\mu\in (0, 2],$ we consider the following Lipschitz type space ^[25]:

where M is any positive constant depending only on $\varphi$.

Theorem 3. For $0 < \mu\leq 2,$ let $\varphi\in Lip_M^{(0, 2]}(\mu).$ Then for all $x\in (0, \infty),$ we have

where $M$ is any positive constant depending only on $\varphi$.

Proof. First, we prove the theorem for the case $\mu = 2.$ Then, for $f\in Lip_M^{(0, 2]}(\mu),$ we have

Using the fact that $\frac{1}{\frac{j}{m}+x}\leq \frac{1}{x}, \; \forall\; j = 0, 1, 2, ...$ and Remark 2, we have

Now, let us prove the theorem for the case $0 < \mu < 2.$ Applying the Hölder inequality

with $(p, q) = (\frac{2}{\mu}, \frac{2}{2-\mu}),$ in view of Lemma 1, we find that

which leads us to the desired result.

Next, we study a local direct estimate for the operators defined in (1.1) by applying the Lipschitz-type maximal function of order $\xi,$ given by Lenze ^[23] as

Theorem 4. Let $\varphi\in \overline{C}_B(S)$ and $0 < \xi \leq 1.$ Then, for all $x\in S,$ we have

Proof. In view of (3.7), we have

and hence

Now, applying the Hölder's inequality with $p = \frac{2}{\xi}$ and $q = \frac{2}{2-\xi},$ in view of Lemma 1 and Remark 2, we obtain

Thus, the proof is completed.

Let $\phi(x) = \sqrt{x(1+x)}$ and $\varphi\in \overline{C}_B(S),$ then for any $\delta > 0,$ the unified Ditzian-Totik modulus $\omega_{\phi^\tau}(\varphi, \delta), 0\leq\tau \leq 1$ is defined as

and the appropriate $K-$functional is given by

where $W_{\tau} = \{f: f\in AC_{loc}(S), \|\phi^{\tau} f' \| < \infty \}$ and $AC_{loc}(S)$ denotes the space of locally absolutely continuous functions on $S.$

From ^[16], it is known that $\omega_{\phi^{\tau}}(\varphi, \delta)\sim K_{\phi^{\tau}}(\varphi, \delta),$ i.e. there exists a constant $M > 0$ such that

Theorem 5. Let $\varphi\in \overline{C}_B(S).$ Then for each $x\in S$ and sufficiently large $m,$ there holds the inequality

where $M_4$ is some constant independent of $\varphi$ and $m.$

Proof. From the definition of $K_{\phi^{\tau}}(\varphi, \delta),$ for a fixed $\tau, \; m$ and $x\in S$ we can choose $f = f_{m, x, \tau}\in W_{\tau}$ such that

From the representation

it follows that

Now, applying the Hölder's inequality

But,

hence using the inequality $|a+b|^s\leq |a|^s+|b|^s, \; \; 0\leq s\leq 1,$ we have

Thus,

Applying Cauchy-Schwarz inequality, Lemma 1 and Remark 2, we get

Now, since $\mathcal{J}_{m, \alpha}(\varphi; x)\to \varphi(x),$ as $m\to \infty,$ for sufficiently large $m$, we have

for some constant $M_1 > 0.$ Hence,

where $M_2 = 2^\tau(1+M_1).$

Thus for $\varphi\in \overline{C}_B(S)$ and any $f\in W_\tau,$ we get

Now, taking the infimum on the right hand side of the above inequality over all $f\in W_\tau$ and using the relation (3.8), we have

This completes the proof.

Now, we present some numerical results to show the convergence of $\mathcal{J}_{m, \alpha}(\varphi; x)$ to $\varphi(x)$ with different values of $\alpha$ by using Matlab.

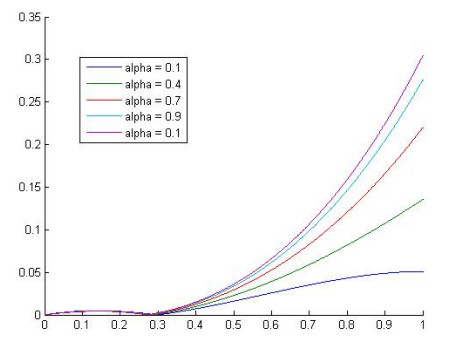
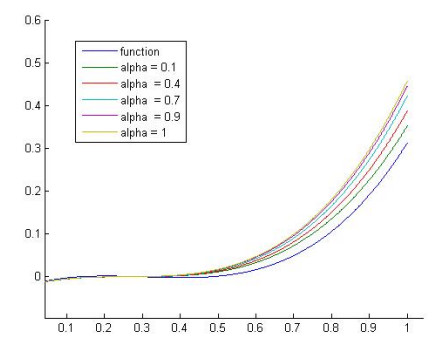
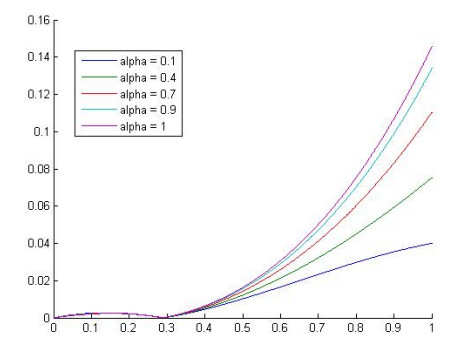
Example 1. Let $\varphi(x) = (x-\frac{1}{2})(x-\frac{1}{4})(x-\frac{1}{6}),$ $m = 15, 30$ and $\alpha \in \{0.1, 0.4, 0.7, 0.9, 1\}.$

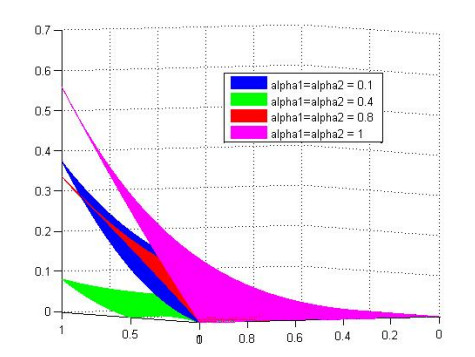
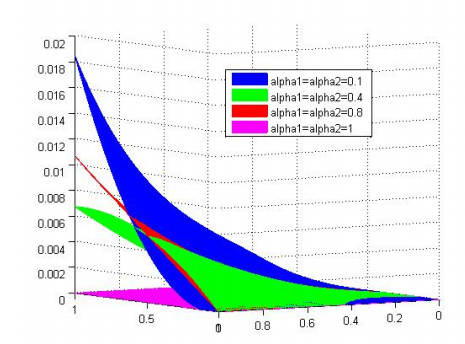
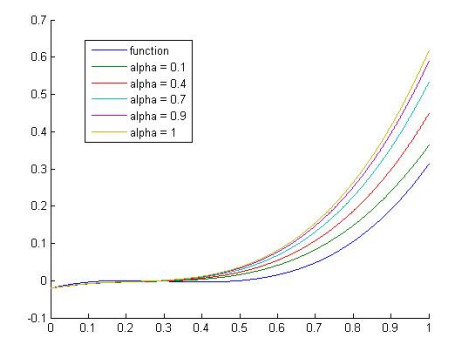
Denote $\mathcal{E}_{m}^{\alpha}(\varphi; x) = |\mathcal{J}_{m, \alpha}(\varphi; x)-\varphi(x)|,$ the error function of approximation by $\mathcal{J}_{m, \alpha}(\varphi; x)$ operators. The convergence of the operators $\mathcal{J}_{m, \alpha}(\varphi; x)$ to the function $\varphi$ with different values of $\alpha$ on the interval $[0, 1]$ and $m = 15, 30$ is illustrated in Figures 1 and 3. We can see from Figures 1 and 3 that for $\alpha = 0.1,$ the operator $\mathcal{J}_{m, \alpha}(\varphi; x)$ gives the best approximation to the function $\varphi$ in comparison with the other values of $\alpha.$ Further, the Tables 1 and 2 and the Figures 2 and 4 clearly show that the error in the approximation $\mathcal{E}_m^\alpha(\varphi; x)$ for $\alpha = 0.1$ is the smallest in comparison with the error corresponding to the other values of $\alpha.$ From the Tables 1 and 2, we also observe that the error becomes smaller as the value of $m$ increases from 15 to 30, for all values of $\alpha.$

4.   Construction of bivariate operators

For $\gamma_1, \gamma_2 > 0$ and $\varphi\in C_{\gamma_1, \gamma_2}(S^2): = \{\varphi\in C(S^2):\; |\varphi(r_1, r_2)|\leq M(1+r_1^{\gamma_1})(1+r_2^{\gamma_2}), \; \forall \; (r_1, r_2)\in S^2\},$ where $S^2: = S\times S$ and $M$ is some positive constant dependent on $\varphi$, we introduce a bivariate extension of the operators $\mathcal{J}_{m, \alpha}(\cdot; x)$ defined by (1.1) as follows:

where $m_1, m_2 \in\mathbb{N}, \; (x_1, x_2)\in S^2$ and $P_{m_1, m_2, j_1, j_2}^{(\alpha_1, \alpha_2)}(x_1, x_2) = P_{m_1, j_1}^{(\alpha_1)}(x_1)\; P_{m_2, j_2}^{(\alpha_2)}(x_2),$

with $\binom{m_1-3}{-2} = \binom{m_1-2}{-1} = 0$ and $\binom{m_2-3}{-2} = \binom{m_2-2}{-1} = 0.$

Let

As a consequence of Lemma 1 and the definition (4.1), we easily obtain:

Lemma 3. For $m_1, m_2\in \mathbb{N},$ the bivariate operators $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(\cdot; x_1, x_2)$ verify the following identities:

(a) $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(e_{00};x_1, x_2) = 1;$

(b) $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(e_{10};x_1, x_2) = x_1+(\alpha_1-1)x_1\frac{2}{m_1};$

(c) $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(e_{01};x_1, x_2) = x_2+(\alpha_2-1)x_2\frac{2}{m_2};$

(d) $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(e_{20};x_1, x_2) = x_1^2+x_1^2(4\alpha_1-3)\frac{1}{m_1}+x_1(m_1+4\alpha_1-4)\frac{1}{m_1^2}.$

(e) $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(e_{02};x_1, x_2) = x_2^2+x_2^2(4\alpha_2-3)\frac{1}{m_2}+x_2(m_2+4\alpha_2-4)\frac{1}{m_2^2}.$

(f) $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(e_{30};x_1, x_2) = x_1^3+x_1^3(6m_1\alpha_1-3m_1+6\alpha_1-4)\frac{1}{m_1^2} +x_1^2(18\alpha_1+3m_1-15)\frac{1}{m_1^2} +x_1(8\alpha_1+m_1-8)\frac{1}{m_1^3};$

(g) $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(e_{03};x_1, x_2) = x_2^3+x_2^3(6m_2\alpha_2-3m_2+6\alpha_2-4)\frac{1}{m_2^2} +x_2^2(18\alpha_2+3m_2-15)\frac{1}{m_2^2} +x_2(8\alpha_2+m_2-8)\frac{1}{m_2^3};$

(h) $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(e_{40};x_1, x_2) = x_1^4+\{(8\alpha_1-2)m_1^2+x_1^4(24\alpha_1-13)m_1+(16\alpha_1-10)\}\frac{1}{m_1^3} +x_1^3\{6m_1^2+(48\alpha_1-30)m_1+(48\alpha_1-36)\}\frac{1}{m_1^3}+x_1^2\{(4\alpha_1+3)m_1+60\alpha_1-53\}\frac{1}{m_1^3}+x_1(16\alpha_1+m_1-16)\frac{1}{m_1^4}$

(i) $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(e_{04};x_1, x_2) = x_2^4+\{(8\alpha_2-2)m_2^2+x_2^4(24\alpha_2-13)m_2+(16\alpha_2-10)\}\frac{1}{m_2^3} +x_2^3\{6m_2^2+(48\alpha_2-30)m_2+(48\alpha_2-36)\}\frac{1}{m_2^3}+x_2^2\{(4\alpha_2+3)m_2+60\alpha_2-53\}\frac{1}{m_2^3}+x_2(16\alpha_2+m_2-16)\frac{1}{m_2^4}.$

Consequently, by a simple computation, we obtain the following result:

Lemma 4. For the operators $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(\cdot; x_1, x_2)$, we have

(a) $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}((r_1-x_1);x_1, x_2) = \frac{2}{m_1}(\alpha_1-1)x_1;$

(b) $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}((r_2-x_2);x_1, x_2) = \frac{2}{m_2}(\alpha_2-1)x_2;$

(c) $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}((r_1-x_1)^2;x_1, x_2) = \frac{1}{m_1}(1+x_1)x_1+\frac{4}{m_1^2}(\alpha_1-1)x_1;$

(d) $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}((r_2-x_2)^2;x_1, x_2) = \frac{1}{m_2}(1+x_2)x_2+\frac{4}{m_2^2}(\alpha_2-1)x_2;$

(e) $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}((r_1-x_1)^4;x_1, x_2) = \frac{3}{m_1^2}(1+x_1)^2 x_1^2-\frac{1}{m_1^3}(10x_1^4+36x_1^2+25x_1-1)x_1+\frac{1}{m_1^3}\alpha_1(16x_1^2+48x_1+32)+\frac{16}{m_1^4}(\alpha_1-1)x_1;$

(f) $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}((r_2-x_2)^4;x_1, x_2) = \frac{3}{m_2^2}(1+x_2)^2x_2^2-\frac{1}{m_2^3}(10x_2^4+36x_2^2+25x_2-1)x_2 +\frac{1}{m_2^3}\alpha_2(16x_2^2+48x_2+32)+\frac{16}{m_2^4}(\alpha_2-1)x_2.$

Remark 3. From Lemma 4, the operators $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(\cdot; x_1, x_2)$, verify the following equalities:

(a) $\underset{m_1\to \infty}{\lim}\; m_1\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}((r_1-x_1);x_1, x_2) = 2(\alpha_1-1)x_1;$

(b) $\underset{m_2\to \infty}{\lim}\; m_2\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}((r_2-x_2);x_1, x_2) = 2(\alpha_2-1)x_2;$

(c) $\underset{m_1\to \infty}{\lim}\; m_1\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}((r_1-x_1)^2;x_1, x_2) = (1+x_1)x_1;$

(d) $\underset{m_2\to \infty}{\lim}\; m_2\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}((r_2-x_2)^2;x_1, x_2) = (1+x_2)x_2;$

(e)$\underset{m_1\to \infty}{\lim}\; m_1^2\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}((r_1-x_1)^4;x_1, x_2) = 3x_1^2(1+x_1)^2;$

(f)$\underset{m_2\to \infty}{\lim}\; m_2^2\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}((r_2-x_2)^4;x_1, x_2) = 3x_2^2(1+x_2)^2.$

Let $C_B(S^2)$ be the space of all bounded and continuous functions on $S^2$ and $S_{cd} = [0, c]\times [0, d]$ be a compact subset of the set $S^2$. Further, let $\|\varphi\| = \underset{(x_1, x_2)\in S^2}{\sup}|\varphi(x_1, x_2)|, \; \; \varphi\in C_B(S^2).$

Theorem 6. If $\varphi\in C_B(S^2),$ then $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi; x_1, x_2)$ converges uniformly to $\varphi$, as $m_1, m_2\to \infty,$ on $S_{cd}.$

Proof. From Lemma 3, we have

and

uniformly on $S_{cd}.$ The proof, now follows on applying Theorem 2.1 of Volkov given in ^[9].

In what follows, let $\delta_{m_i, \alpha_i}(x_i) = \mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}((r_i-x_i)^2;x_1, x_2), \; i = 1, 2.$ Now, we consider the degree of approximation of the operators $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(\cdot; x_1, x_2)$ in the space of bounded and uniformly continuous functions on $S^2.$

For $\varphi\in \overline{C}_B(S^2): = \{\varphi\in C_B(S^2): \varphi\; is\; uniformly\; continuous \}$ and $\delta > 0,$ the total modulus of continuity for the bivariate case is defined as follows:

Further, the partial moduli of continuity with respect to $x_1$ and $x_2$ is defined as

and

The properties of the modulus of continuity for the bivariate case can be seen in ^[15]. Now, we give the estimate of the convergence of the bivariate operators defined by (4.1) in terms of the total modulus of continuity.

Theorem 7. Let $\varphi\in \overline{C}_B(S^2),$ then for all $(x_1, x_2)\in S^2,$ we have

Proof. From the definition of the complete modulus of continuity (4.2) of $\varphi$, we may write

$|\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi; x_1, x_2)-\varphi(x_1, x_2)|$

for any $\delta > 0.$

Now, applying the Cauchy-Schwarz inequality and Lemma 3, we get

Choosing $\delta: = (\delta_{m_1, \alpha_1}(x_1)+\delta_{m_2, \alpha_2}(x_2))^{1/2},$ we reach the required result. Next, we obtain the degree of approximation of the operators $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(\cdot; x_1, x_2)$ by means of the partial moduli continuity.

Theorem 8. If $\varphi\in \overline{C}_B(S^2),$ then for all $(x_1, x_2)\in S^2,$ we have

Proof. Using the definitions of partial moduli of continuity given by (4.3) and (4.4) for $\varphi\in \overline{C}_B(S^2),$ we can write

$|\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi; x_1, x_2)-\varphi(x_1, x_2)|$

Applying the Cauchy-Schwarz inequality and Lemma 3 for any $\delta_1, \delta_2 > 0,$ we have

Hence choosing $\delta_1: = \sqrt{\delta_{m_1, \alpha_1}(x_1)}$ and $\delta_2: = \sqrt{\delta_{m_2, \alpha_2}(x_2)},$ we get the desired result.

Let

In particular for $k = 2,$ let the norm on the space $C_B^2(S^2)$ be given by

The appropriate Peetre's K-functional for the function $\varphi\in \overline{C}_B(S^2)$ is defined as

From ^[13], for $\varphi\in \overline{C}_B(S^2),$ it is known that

where $\overline{\omega}_2(\varphi; \sqrt{\delta})$ is the second order modulus of continuity for the bivariate case and $M$ is a constant independent of $\delta$ and $\varphi$.

Theorem 9. For the function $\varphi\in \overline{C}_B(S^2)$ and for all $(x_1, x_2)\in S^2,$ we have the following inequality $|\mathcal{J}_{m_1, m_1, \alpha_1, \alpha_2}(\varphi; x_1, x_2)-\varphi(x_1, x_2)|\leq M \overline{\omega}_2\bigg(\varphi; \frac{\sqrt{\mathcal{A}_{m_1, m_2, \alpha_1, \alpha_2}(x_1, x_2)}}{2}\bigg)$

where

Proof. We define an auxiliary operator $\mathcal{L}_{m_1, m_2, \alpha_1, \alpha_2}(\cdot; x_1, x_2)$ as follows:

In view of Lemma 3, we have

Let $f\in C^2_B(S^2),$ and $(r_1, r_2), \; (x_1, x_2)\in S^2$ be arbitrary. By using the Taylor's expansion, we can write

Now, applying $\mathcal{L}_{m_1, m_2, \alpha_1, \alpha_2}(\cdot; x_1, x_2)$ on the above equation and using (4.7), we obtain

Let us note that

and

Similarly,

Hence, we conclude that

$|\mathcal{L}_{m_1, m_2, \alpha_1, \alpha_2}(f(r_1, r_2);x_1, x_2)-f(x_1, x_2)|$

From (4.6), using Lemma 3, we have

$|\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi; x_1, x_2)|\leq |\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi; x_1, x_2)|+|\varphi(x_1, x_2)|+\bigg|\varphi\bigg(\frac{x_1(2\alpha_1+m_1-2)}{m_1}, \frac{x_2(2\alpha_2+m_2-2)}{m_2}\bigg)\bigg|$ $\leq 3\|\varphi\|.$

Hence, for all $\varphi\in {\overline{C}_B(S^2)}$ and $f\in C^2_B(S^2),$ from (4.6) we get

Now, taking the infimum on the right hand side over all $f\in C^2_B(S^2)$ and using the relation (4.5) we get

This completes the proof of the theorem.

Next, we establish the degree of approximation by the bivariate operators $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(\cdot; x_1, x_2)$ for the Lipschitz class functions in the bivariate case.

For $0 < \mu_1, \mu_2\leq 1$, the Lipschitz class $Lip_M(\mu_1, \mu_2)$ for the bivariate case is defined as follows:

where M is any positive constant and $(r_1, r_2), (x_1, x_2)\in S^2$ are arbitrary.

Theorem 10. Let $\varphi\in Lip_M(\mu_1, \mu_2),$ then, we have

Proof. For $\varphi\in Lip_M(\mu_1, \mu_2),$ we may write

Now, using Hölder's inequality with $(p_1, q_1) = (\frac{2}{\mu_1}, \frac{2}{2-\mu_1})$ and $(p_2, q_2) = (\frac{2}{\mu_2}, \frac{2}{2-\mu_2})$ and Lemma 3, we get $|\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi; x_1, x_2)-\varphi(x_1, x_2)|$

which is the required result.

Theorem 11. For $\varphi\in C_B^1(S^2)$ and each $(x_1, x_2)\in S^2,$ we have

Proof. Let $(x_1, x_2)\in S^2$ be a fixed point and $\varphi\in C_B^1(S^2).$ Then by our hypothesis, we can write

Now, applying the operator $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(\cdot; x_1, x_2)$ on both sides of the above equation, we are led to

Now,

and

hence on combining (4.8) and the above inequalities, we get

Applying the Cauchy-Schwarz inequality and Lemma 3, we are led to the desired result.

In the following result, we establish a Voronovskaya type asymptotic theorem.

Theorem 12. Let $\varphi\in C_B^2(S^2),$ then

uniformly on $S_{cd}.$

Proof. Let $(x_1, x_2)\in S_{cd}$ be arbitrary but fixed. By the Taylor's expansion, we get

where $\epsilon(r_1, r_2;x_1, x_2)\in C_B(S^2)$ and $\epsilon(r_1, r_2;x_1, x_2)\to 0,$ as $(r_1, r_2) \to (x_1, x_2)$.

Applying the operators $\mathcal{J}_{m, m, \alpha_1, \alpha_2} (\cdot; x_1, x_2)$ on both sides of (4.9), we get

Hence, using Lemma 4, we obtain

uniformly on $S_{cd}.$

Now, using the Cauchy-Schwarz inequality, we obtain

$\bigg|\mathcal{J}_{m, m, \alpha_1, \alpha_2}\bigg(\epsilon(r_1, r_2;x_1, x_2)\sqrt{(r_1-x_1)^4+(r_2-x_2)^4};x_1, x_2\bigg)\bigg|$

From Theorem 6, $\mathcal{J}_{m, m, \alpha_1, \alpha_2}(\epsilon^2(r_1, r_2;x_1, x_2);x_1, x_2) \to 0,$ as $m \to \infty,$ uniformly on $S_{cd}$ and from Remark 3, we have

$\mathcal{J}_{m, m, \alpha_1, \alpha_2}((r_1-x_1)^4;x_1, x_2) = O\bigg(\frac{1}{m^2}\bigg)$, $\mathcal{J}_{m, m, \alpha_1, \alpha_2}((r_2-x_2)^4;x_1, x_2) = O\bigg(\frac{1}{m^2}\bigg),$ uniformly on $S_{cd}$.

Hence

uniformly on $S_{cd}$.

The required result now, follows from (4.10) and (4.11).

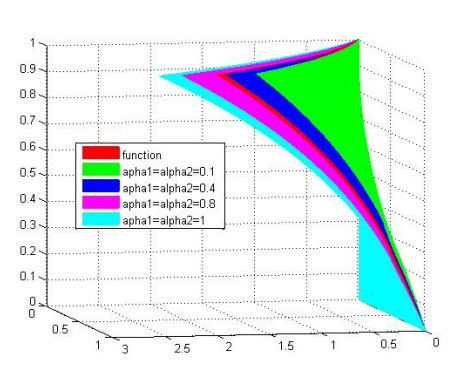
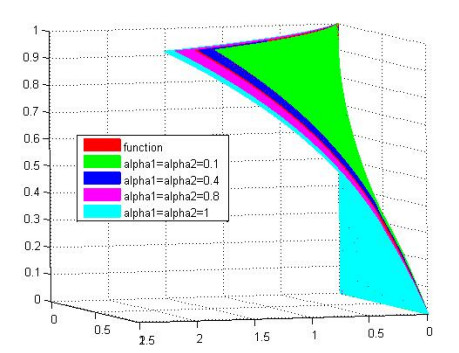
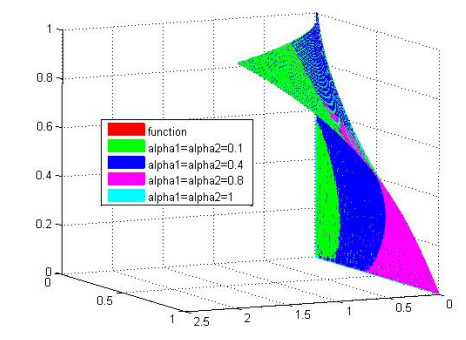
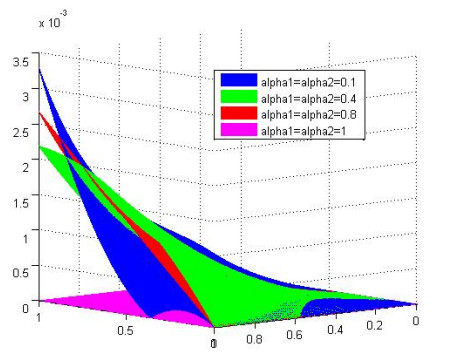
Example 2. Consider the function $\varphi(x_1, x_2) = 5 x_1 x_2^3+x_1^3x_2$, and $(\alpha_1, \alpha_2) \in \{(0.1, 0.1), (0.4, 0.4), (0.8, \\0.8), (1, 1)\}$, $m_i = 15, 30$ for $i = 1, 2$. Denote $\mathcal{E}_{m_{1}, m_{2}}^{\alpha_{1}, \alpha_{2}} = |\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi; x_1, x_2)-\varphi(x_1, x_2)|,$ the error function of approximation by operators. For different values of $(\alpha_1, \alpha_2),$ the convergence of the operators $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi; x_1, x_2)$ for $m_1 = m_2 = 15$ and $m_1 = m_2 = 30$ to the function $\varphi$ is as shown in Figures 5 and 7. From Figures 6 and 8 and Tables 3 and 4 we note that when $\alpha_1 = \alpha_2 = 0.4$, the bivariate approximation by $\alpha$-Baskakov operators outperforms others.

5.   Construction of GBS (Generalized Boolean Sum) operators

Bögel ^[10,11] defined the concepts of Bögel continuous and Bögel differentiable functions. For details on these notions, we refer the reader to ^[12]. Dobrescu and Matei ^[17] established the uniform convergence of GBS of bivariate Bernstein polynomials to the Bögel continuous functions (B-continuous functions). Badea and Cottin ^[7] gave Korovkin type theorems for GBS operators. Badea et al. ^[6] gave a quantitative variant of the Korovkin type theorem for the B-continuous functions and applied the results to certain operators. Bǎrbosu and Muraru ^[9] constructed a GBS operator of Bernstein-Schurer-Stancu type to study the approximation of B-continuous functions. In this section, we introduce the GBS case of the operators $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi; x_1, x_2)$ and study its approximation properties. First, we give some definitions and notations, the details can be found in the book ^[18].

Let $Y_1$ and $Y_2$ be any subsets of $\mathbb{R}.$ A function $\varphi:Y_1\times Y_2 \to \mathbb{R}$ is called B-continuous at a point $(x_1, x_2)\in Y_1\times Y_2$ if

where $\Delta_{(r_1, r_2)}\varphi(x_1, x_2) = \varphi(x_1, x_2)-\varphi(x_1, r_2)-\varphi(r_1, x_2)+\varphi(r_1, r_2)$ denotes the mixed difference.

A function $\varphi$ is said to be B-continuous in $Y_1\times Y_2$ if it is B-continuous at each point of $Y_1\times Y_2.$ The space of B-continuous functions is denoted by $C_b(Y_1\times Y_2).$

A function $\varphi:Y_1\times Y_2 \to \mathbb{R}$ is said to be B-bounded in $Y_1\times Y_2$ if there exists $M > 0$ such that

for every $(x_1, x_2), (r_1, r_2)\in Y_1\times Y_2.$

Let $B_b(Y_1\times Y_2)$ be the space of B-bounded functions on $Y_1\times Y_2\to \mathbb{R},$ with the norm

Let $B(Y_1\times Y_2): = \{\varphi:Y_1\times Y_2 \to \mathbb{R}|\; \varphi\; is\; bounded\}$ endowed with the sup-norm $\|.\|_\infty$ and $C(Y_1\times Y_2): = \{\varphi:Y_1\times Y_2 \to \mathbb{R}|\; \varphi\; is\; continuous\}$. It is easily seen that $C(Y_1\times Y_2)\subset C_b(Y_1\times Y_2)$ ^[12].

A function $\varphi:Y_1\times Y_2\to \mathbb{R}$ is said to be uniformly B-continuous in $Y_1 \times Y_2$ if for any $\varepsilon > 0$ there exists a $\delta = \delta(\varepsilon) > 0$ such that $|\Delta_{(r_1, r_2)}\varphi(x_1, x_2)| < \varepsilon,$ whenever $\max\{|r_1-x_1|, |r_2-x_2|\} < \delta$ and $(r_1, r_2), (x_1, x_2)\in Y_1\times Y_2.$

Let $\overline{C}_b(Y_1\times Y_2)$ denote the space of all uniformly B-continuous functions on $Y_1\times Y_2.$

A real valued function $\varphi$ on $Y_1\times Y_2$ is called B-differentiable (Bögel differentiable) at a point $(x_1, x_2)\in Y_1\times Y_2$ if the limit

exists and is finite.

The limit is called the B-differential of $\varphi$ at the point $(x_1, x_2)$ and is denoted by $D_B(\varphi; x_1, x_2).$

Let

For $\varphi\in B_b(Y_1\times Y_2),$ the mixed modulus of smoothness is given by

for all $(x_1, x_2), (r_1, r_2)\in Y_1\times Y_2$ and for any $\delta_1, \delta_2 > 0.$ It is known (cf. ^[6] and ^[7]) that $\omega_B(\varphi; \delta_1, \delta_2)\to 0,$ as $\delta_1, \delta_2 \to 0,$ if and only if $\varphi$ is uniformly B-continuous on $Y_1\times Y_2$. Further, for any $\lambda_1, \lambda_2 > 0$

For every $\varphi\in C_b(S^2),$ the GBS operator associated with the operator $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi; x_1, x_2)$ is defined by:

where $P_{m_1, m_2, j_1, j_2}^{(\alpha_1, \alpha_2)}(x_1, x_2)$ is defined by Eq (4.1). It is evident that $\mathcal{T}_{m_1, m_2, \alpha_1, \alpha_2}$ is a linear operator. The following result yields us an error estimate in the approximation of a B-continuous function by the operators (5.2).

Theorem 13. For every $\varphi\in \overline{C}_b(S^2)$ and each $(x_1, x_2)\in S^2,$ there holds the following inequality

Proof. Using the definition of $\omega_B(\varphi; \delta_1, \delta_2)$ and the property (5.1), we have

for every $(r_1, r_2), (x_1, x_2)\in S^2$ and any $\delta_1, \delta_2 > 0.$

From (5.2), by using the definition of mixed difference $\Delta_{(r_1, r_2)}\varphi(x_1, x_2)$ and inequality (5.3), we get $|\mathcal{T}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi; x_1, x_2)-\varphi(x_1, x_2)|$

Now, applying Lemma 3 and Cauchy-Schwarz inequality, we get

$|\mathcal{T}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi; x_1, x_2)-\varphi(x_1, x_2)|$

which leads us to the required result on choosing $\delta_1: = \sqrt{\delta_{m_1, \alpha_1}(x_1)}$ and $\delta_2: = \sqrt{\delta_{m_2, \alpha_2}(x_2)}.$

Lipschitz class of B-continuous functions: For $0 < \mu_1\leq 1, \; 0 < \mu_2\leq 1$ and $\varphi\in C_b(S^2),$ the Lipschitz class $Lip^{\ast}_M(\mu_1, \mu_2)$ of $\varphi$ is defined as follows:

for all $(r_1, r_2), \; (x_1, x_2)\in S^2$ and $M$ is a positive constant.

In the following theorem, we obtain the approximation degree for the operators $\mathcal{T}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi),$

if $\varphi\in Lip_M^\ast(\mu_1, \mu_2).$

Theorem 14. For $\varphi\in Lip^{\ast}_M(\mu_1, \mu_2)$ and $\mu_1, \mu_2\in(0, 1],$ we have

Proof. From the definition of the mixed difference $\Delta_{(r_1, r_2)}\varphi(x_1, x_2),$ (5.2) and by our hypothesis, we may write

Now, applying the Hölder inequality with $(p_1, q_1) = \bigg(\frac{2}{\mu_1}, \frac{2}{2-\mu_1}\bigg)$ and $(p_2, q_2) = \bigg(\frac{2}{\mu_2}, \frac{2}{2-\mu_2}\bigg)$, in view of Lemma 3, we are led to

from which the desired result is immediate.

The following result provides us the rate of convergence of the GBS operators $\mathcal{T}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi)$ in terms of the mixed modulus of smoothness of the B-derivative of $\varphi.$

Theorem 15. Let $\varphi\in D_b(S^2)$ such that $D_B \varphi\in \overline{C}_b(S^2)\cap B(S^2).$ Then for each $(x_1, x_2)\in S^2,$ we have

Proof. Since $\varphi\in D_b(S^2),$ by the mean value theorem we can write

where $x_1 < \xi_1 < r_1$ and $x_2 < \xi_2 < r_2.$

From the definition of the mixed difference, we obtain

Since $D_B \varphi\in \overline{C}_b(S^2)\cap B(S^2),$ we have

Hence taking into account

for any $\delta_1, \delta_2 > 0$ and applying the Cauchy-Schwarz inequality, we obtain

Since $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}((r_1-x_1)^{2i}(r_2-x_2)^{2j}; x_1, x_2) = \mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}((r_1-x_1)^{2i}; x_1, x_2)\; \mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}((r_2-x_2)^{2j}; x_1, x_2), \\ for\; \; j = 1, 2,$ using Lemma 4 and choosing $\delta_1 = \frac{1}{\sqrt{m_1}}$ and $\; \delta_2 = \frac{1}{\sqrt{m_2}},$ we reach the required result.

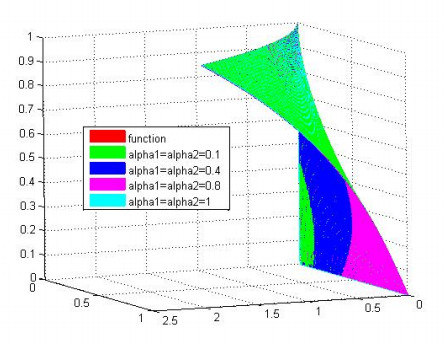
Example 3. Let $\varphi(x_1, x_2) = x_1^2x_2+x_1^3x_2,$ and $(\alpha_1, \alpha_2) \in \{(0.1, 0.1), (0.4, 0.4), (0.8, 0.8), (1, 1)\}.$ The convergence of the GBS operators $\mathcal{T}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi; x_1, x_2)$ for $m_1 = m_2 = 15$ and $m_1 = m_2 = 30,$ to the function $\varphi$ for different values of $(\alpha_1, \alpha_2)$ is illustrated in Figure 9 and Figure 11. In Table 3 and Table 4, we have computed the error function of approximation by operators $\mathcal{E^*}_{m_{1}, m_{2}}^{\alpha_{1}, \alpha_{2}} = |\mathcal{T}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi; x_1, x_2)-\varphi(x_1, x_2)|$ for $m_1 = m_2 = 15$ and $m_1 = m_2 = 30.$ When we examine the error approximation in Table 5 and Table 6, we observe that the best approximation is achieved when $\alpha_1 = \alpha_2 = 1.$ This aspect is also illustrated graphically by Figures 10 and 12 respectively.

Further, we note that the error in the approximation of $\varphi$ by $\mathcal{T}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi; x_1, x_2)$ is much smaller than the errors in the approximation of $\varphi$ by $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi; x_1, x_2).$ As seen from the Table 3-6, our GBS operator $\mathcal{T}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi; x_1, x_2)$ gives us a better rate of convergence than the bivariate $\alpha$-Baskakov operators $\mathcal{J}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi; x_1, x_2)$

6.   Conclusions

The $\alpha$-Baskakov operators yield a much better approximation for a function in comparison to the classical Baskakov operators. As seen from the Tables 1 and 2, the advantage of using a non-negative real parameter is that it provides flexibility to the operators, and the results presented here show that depending on the value of the parameter $\alpha,$ an approximation to a function improves when we compare with classical Baskakov operators (see Example 1). Also, the GBS operators presented in this paper provide a better error estimation of convergence than the bivariate $\alpha$-Baskakov operators with a non-negative real parameter. It is observed that the convergence rate of GBS operator $\mathcal{T}_{m_1, m_2, \alpha_1, \alpha_2}(\varphi)$ to the function $\varphi(x_1, x_2)$ is much better than $\mathcal{J}_{m_1, m_1, \alpha_1, \alpha_2}(\varphi)$ operator.

Acknowledgments

The authors are extremely thankful to the learned reviewers for a critical reading of our paper and making valuable comments leading to an improvement in the presentation of the paper. The third author is thankful to the Ministry of Human Resource and Development, Govt. of India for providing financial support to carry out the above work.

Conflict of interest

The authors declare no conflict of interest.