1. Introduction
While it has been long acknowledged that volatility tends to be higher when (market) excess returns are negative, recent years have been characterized by a rollercoaster-type variation in stock prices and volatility that has greatly emphasized this well-known fact: while most indicators of volatility skyrocketed to unprecedented levels between October 2007 and March 2009, the US market dropped by around 50%, amid frenzied trading and panic waves. Under these circumstances, rising risk aversion prompted investors to look for alternative asset classes able to limit their losses by hedging negative stock (and real estate) returns in bear states. Naturally, given its dynamic properties volatility itself has gradually emerged as such an asset class (see Alexander et al., 2015; Grant et al., 2007; Hafner and Wallmeier, 2007).1
1 Such a search for better diversifiers of market returns was made even more desperate by the fact that many classical hedging strategies previously fashionable with asset managers did break down during the Great Financial Crisis. For example, the negative correlation that has historically characterized the relationship between equities and commodities became positive by 2009 (see Szado, 2009). Moreover, in 2008 the HFRX Global Hedge Fund lost about 25% while the loss on the S & P GSCI spot commodity index was also 70%; yet, historically, the two asset classes show negative correlation. The HFRX Global Hedge Fund index includes all eligible strategies and hedge funds, such as convertible arbitrage, distressed securities, equity speculation, neutral equity strategies (long-short), event-and macroeconomic-driven, arbitrage mergers and arbitrage of relative value.
Among the volatility indices used to assess the value of volatility as an asset class, the VIX—publicly reported by the Chicago Board of Exchange (CBOE)—has naturally emerged as a primary reference. The VIX index reflects volatility expectations at a 30-day horizon on the S & P 500 index implicit in option prices. For instance, DeLisle et al. (2011) have studied the relationship between the S & P 500 and VIX, to demonstrate an asymmetric relationship by which their correlation turns higher in bear market states.2 However, VIX and therefore the literature that has made a reference to it, faces one key limitation: albeit it has become one of the most useful indices available in academic as well as in practitioners' research (see Whaley, 2000, 2009), VIX is not an investable index. Although, it may be possible to replicate the VIX by investing in the underlying basket of options, it appears either technically hard or costly to continuously replicate VIX index returns this way, as such a strategy would entail investing in a large number of options and rebalancing the position at least on a daily basis (see the discussion in Whaley, 2009).
2 Whaley (2013) has also shown that VIX is an interesting investable asset in itself: investors may try and exploit the mean-reverting patterns in VIX created by the existence of a cyclical process that defines a trend in volatility movement reverting to its mean.
The obvious reaction to such impediments has been to argue (and to invest accordingly) that even though VIX is not tradable, derivatives (exchange traded products, ETPs) exist that allow us to trade exposures on the VIX (see, e.g., Alexander and Korovilas, 2013). ETPs—such as exchange-traded funds, notes, covered warrants, and investment certificates—represent a key financial innovation of the last few decades. Their success is due to their versatility and liquidity that derives from being traded on regulated exchanges, so that they are now routinely used both in strategic and tactical portfolio applications (see Amenc et al., 2010). In this paper, we use data on one specific Exchange Traded Fund (ETF) with a low commission profile, listed on a stock exchange as ordinary share, which aims at passively replicating the performance of the VIX. This is usually achieved synthetically, often using appropriately structured, over-the-counter swap contracts. When compared to futures on the VIX, ETFs and ETNs are often advertised as cheaper alternatives, as they are characterized by low transaction costs and are not subject to the mispricing of futures contracts close to roll-over date (see Madhavan et al., 2014).3 To test whether this is the case or not in an empirical perspective, represents one of our goals.
3 A recent literature has also found that ETFs may incorporate and discount new information faster than the underlying spot or futures markets (see Bollen, O'Neill, and Whaley, 2017, for an application to VIX futures markets). For instance, when changes in the price of futures contracts on the S & P 500, and the price of ETFs written on the same index (SPDR) are compared, there is some evidence in favor of this hypothesis. Therefore, besides being simple and relatively cost-effective, trading ETNs may also represent an informationally efficient strategy.
In this paper, we use weekly data for the period January 2009–February 2016 to assess realized portfolio outcomes from taking long-only positions in the VIX either directly or through what is arguably the most popular exchange traded note written on the VIX, Barclays' iShares S & P 500 VIX ST Futures (identified by the VXX ticker) exchange traded note (ETN). VXX provides passive replication of the S & P VIX Short Term Futures Index Total Return (SPVXSTR): changes in the index level are supposed (intended to be) highly correlated with changes in market volatility. We select VXX over alternative ETNs (such as VIIX, issued by Velocity Share and XVZ also issued by iShares which however tracks an index that can take long or short positions on futures contracts on the VIX depending implied changes in forward VIX) because it allows us to access the longest time series among all similar ETPs. However, to show that the exact choice of VXX does not represent the key choice, we also test the robustness of our results to directly trading short-term VIX futures.
Specifically, we solve recursive asset allocation problems at a monthly frequency with references to an expanding, out-of-sample (OOS) February 2010–February 2016 window, analyzing alternative asset menus—without volatility-trading, with VIX added, with VXX instead, or with strategies based on trading only the closest-to-maturity VIX futures. The other assets in the selection menu are represented by ETFs that allow us to invest in equities, real estate, and bonds. The portfolio outcomes that we track and comment are, besides optimal portfolio composition, the realized Sharpe and Sortino ratios, the information ratio, portfolio turnover, and average certainty equivalent returns (CERs). Because the portfolio problems are solved under three alternative types of preferences—mean-variance, power, and negative exponential utility functions—the CER measures are computed under each of the three metrics. Although shorting volatility had become an extremely popular strategy with a sub-class of investors during the 2006–2007 bubble years leading to the spectacular crash during the Great Financial Crisis, in our paper we limit ourselves to long-only strategies that make more sense for a variety of institutional investors that are restricted from (or strongly advised against) shorting volatility or be contractually restricted from futures roll-over strategies.
Our key result is that while going long in the VIX remains a good idea—thus confirming earlier results in Black (2006) and Dash and Moran (2007)—the ETPs that are commonly used (or at least, one of the key ETPs that have become popular) to actually trade volatility give very different results and have structural difficulties in creating risk-adjusted economic value, especially after transaction costs are netted out. This holds in a range of experiments and under a variety of preferences. This confirms a result in Hancock (2013) and Whaley (2013) who has analyzed a fixed buy-and-hold position in volatility ETNs and obtained evidence of poor performance mostly caused by the tendency of ETN prices to decline over time due to a "bleeding" effect caused by the need to roll over expiring VIX futures contracts with longer-maturity but more expensive ones, due to the typical (and yet sensible, as more distant events are more uncertain) downward sloping term structure of the VIX futures curve. Interestingly, his result obtains even though VXX is strongly negatively correlated with other asset classes. Differently from Whaley (2013) however, in this paper we perform such tests in a fully optimized framework in which an investor is recursively allowed to select her commitment to VXX based on the maximization of standard (expected) utility criteria popular in the literature. This means that the hedging power of VIX or VXX are taken into account, and the reported risk-adjusted performance metrics are the most appropriate.4
4 One referee has correctly pointed out that many practitioners are likely to interpret ETPs such as the VXX as tools to hedge left-tail risk of a given portfolio more than as an asset class by itself in a fully optimized portfolio strategy. On the one hand, our approach is instead to ask whether and how VXX may surrogate VIX as an alternative asset class; we find that while VIX may represent such a new asset class, VXX faces severe difficulties in that respect, and that rolling over futures strategies directly leads to similar results. On the other hand, even though VXX might remain quite effective as a tool to control and trade left-tail risk only (see Bhansali, 2008), one of our utility functions, power utility, fully reflects the co-skewness and co-kurtosis properties of returns, in the sense that an investor is allowed to strongly dislike left-tail excessive risk.
The paper is organized as follows: section 2 reviews the literature on volatility trading and volatility in asset management, when assessed as an additional asset class. Section 3 describes the framework of analysis and our methodology and Section 4 reports summary statistics concerning the data. Section 5 presents the recursive results on optimal asset allocation and portfolio weights. Section 6 performs a back-test OOS evaluation of the risk adjusted value created by VIX vs. VXX. Section 7 deals with the case in which we simulate a strategy that directly trades VIX futures contracts to tease out from our result what portion of the poor performance of ETNs is due to their high costs and low efficiency in "riding" the futures curve. Section 8 concludes.
2. Our Research Question: A Review of the Literature
Volatility plays a decisive role in the dynamics of financial markets and volatility is considered a key driver in Merton's, intertemporal CAPM-style analyses. Also to favor the development of trading strategies concerning volatility, in 1993, the Chicago Board Options Exchange (CBOE) introduced the first version of its volatility index known as VXO, based on the implied volatility of eight at the money options written on the S & P 100. A revised version of the index, since then known as VIX, was introduced in 2003 (see CBOE, 2003). VIX uses a formula that extracts implied volatilities from far more extensive basket of options on the S & P 500.
After the VIX had been introduced, a literature has developed that studies volatility as an asset class and that proposed a range of strategies to exploit volatility trading in its fullest. Whaley (2009) explains the structure and role of the index, emphasizing the inability to directly invest and assume positions in it. In particular, although it is generally possible to replicate the payoff of any non-traded index by investing in the underlying basket of securities, it would be almost impossible to replicate the performance of the VIX index through the same process. This is directly caused by the nature of the VIX formula, which uses only out-of-the money (OTM) call and put market prices at one month to expiration: therefore, creating a portfolio that replicates VIX on any given day, would mean investing in numerous options and rebalancing such positions on a daily basis, thus incurring in elevated transaction costs.
Although VIX may represent an asset class in which it is difficult to invest in and that implies considerable transaction costs, its investment benefits have immediately attracted attention. For instance, the relationship between the underlying stock index and the VIX displays an asymmetric profile depending on whether the index is rising or falling (see Stanton, 2011). Whaley (2009) explains what happens during market downturns: in this scenario, the demand for OTM and at the money (ATM) defensive puts increases, driving up their price and implied volatilities; such an increase in the price of options causes an increase, albeit not proportional, in the VIX, that therefore provides a natural hedge against sudden and sharp bear market phases. On the contrary, during a market expansion, few investors resort to the implicit leverage offered by the purchase of call options, while contrarians may also engage in writing calls: as a result the corresponding implicit volatilities tend to be constant or even to slowly decline over time, and therefore the VIX tends to display low volatility and drift lower, during bull markets. Hafner and Wallmeier (2008) link such negative correlation between equity indices and the VIX to the absence of arbitrage opportunities in the options market, when it is characterized by a marked skew of volatility, i.e., the tendency of OTM puts to quote higher than they should, given ATM prices. In the literature, since Bekaert and Wu (2000), such a pattern has been dubbed the volatility feedback effect: assuming that volatility is one of the drivers of equity prices, a positive change in perceived variance increases future performance while at the same time it reduces the stock price today.
Given such an inverse relationship between key equity indices and the VIX, a small portfolio management literature has investigated the benefits of investing in the VIX and the possibility of replicating its payoffs via strategies that simply trade options and futures. Black (2006) and Dash and Moran (2007) have shown that by adding VIX futures to a passive portfolio strategy may help reduce the volatility of realized returns. However, VIX futures contracts yield performances that are inferior to directly investing in the VIX or even the options themselves. The lower benefits are caused by the roll-over costs of the VIX future, that is, the cost incurred to close the position on the expiring contract and to open a new one on contracts with a later expiration date. Even though Black and Dash and Moran ask the same research question as we do, their effort does not directly quantify the risk-adjusted benefits of the VIX vs. rolling futures contracts on the VIX, when interpreted as an additional asset class in portfolio construction.5
5 Most of these papers either impose long, defensive positions in the VIX and the replicating strategies or obtain endogenously such a defensive position. On the contrary, Fallon, Park and Yun (2015) estimate the contribution that the exposure to volatility may generate in terms of strategic portfolio allocation and—because their distinctive objective is to analyze the role of volatility within the portfolio of institutional investors—they demonstrate that taking short positions in volatility turns out to be extremely profitable, boosting long-term investment performance. In particular, they estimate an increase of about 12% in Sharpe's index against an increase in short-term risk.
DeLisle et al. (2010) demonstrate that taking a direct position in the VIX is an effective hedge during market downturns that, at the same time, does not penalize returns in the upswing. The alternative represented by futures contracts leads instead to a mean differential return of -3.12% on a monthly basis for the period April 2004–August 2009, against a VIX average contribution to total portfolio returns of + 1.8%. Doran and Krieger (2010) have also studied the possibility of replicating the VIX index through the construction of a synthetic portfolio in order to obtain the best hedge against S & P 500 losses, but obtain mixed results. Brenner et al. (2006) build a straddle option as the hedging instrument from the volatility of the S & P500 and observe that it may lead to performances that approximate those from the VIX.
Another strand of literature has analyzed not the power of VIX to hedge portfolio losses during market busts, but has instead considered volatility investing as an alternative investment strategy. For instance, Kuenzi and Xu (2007) define volatility as an alternative source of beta, for example, compared to hedge funds, namely an asset class whose yield is linked to exposure to a specific and novel systematic risk factor. In this context, Brière et al. (2010) assess volatility in the perspective of a long-term investor. They analyze two alternatives: a direct position in the VIX vs. investing in variance swaps that allow them to take a position on the difference between realized volatility and implied volatility.6 The strategy that invests in the VIX has the effect of reducing extreme risks and the result is a less risky strategic allocation vis-à-vis traditional investments in a balanced portfolio that includes bond positions. In addition, the combination of the two volatility strategies improves performance. However, variance swaps alone cannot replace the VIX.
6 A variance swap is a contract that allows an investor to take a position on volatility through the exchange of flows linked to the realized volatility and implied volatility: a leg of the swap will pay an amount based on the realized variance of the underlying, while the other leg will pay a flow-maturity equal to the implied variance from option prices.
3. Methodology
3.1. Baseline mean-variance preferences
We start by using standard mean-variance theory and look for a constrained, optimal combination of the assets in the selection menu that minimizes risk and maximizes (i.e., for a fixed level of) expected returns
under the constraints ∑Ni=1ωi=1, ωi∈[0,1], and μμ′ω=μP, where ω is the N x 1 vector of portfolio weights, Σ is the covariance matrix of asset returns, μ is the vector of expected returns, and μP is some pre-fixed target for expected portfolio returns. The two constraints ∑Ni=1ωi=1ωi∈[0,1] jointly imply that it is impossible to sell assets/securities short.7 In practice, we model a risk-averse investor who selects the portfolio that maximizes her expected utility from portfolio returns, given an assumed level of risk aversion, λ:
|
V(RPt+1)=Et[RPt+1]−12λVart[RPt+1].
|
(2)
|
7 We could have extended the analysis to also include a risk-free asset, but, as it is well known from the separation theorem, the same risky portfolio would then be selected independently of the risk-aversion parameter, λ.
Because it can be shown that maximization of (2) subject to a choice of a mean-variance efficient portfolio, is equivalent to the maximization of the Sharpe ratio, defined as SR(RPt+1)≡(E[RPt+1]−rf)/√Var[RPt+1], ex-post, after appropriate recursive back-tests are performed, the appropriate metric to assess the effective, risk-adjusted performance is therefore represented by the realized Sharpe ratio:
|
^SRT≡T−1∑T−1t=1(RPt+1−rft)√T−1∑Tt=1[(RPt+1−rft)−T−1∑T−1t=1(RPt+1−rft)]2,
|
(3)
|
where rft is the risk-free rate between time t and t+1, and ^SRT denotes the realized Sharpe ratio over a back-testing sample of length T. The RPt+1 are realized portfolio returns that depend on the optimal portfolio weights determined in the course of the exercise, RPt+1=RPt+1(ˆωt,t+1)), where ˆωt,t+1 is the vector of optimal portfolio weights. For the time being, we ignore transaction costs and taxes.
3.2. Additional preferences
Although mean-variance analysis finds widespread application because of its simplicity and intuitive appeal, as it is well known, the type of preferences (utility function of terminal wealth) that provides micro-foundations to mean-variance—essentially, quadratic utility, unless further restrictive assumptions are imposed on the joint distribution of asset returns—may imply the existence of a bliss point for wealth beyond which any additional increase in wealth causes a reduction in realized utility, i.e., preferences may be plagued by satiation issues to the point of allowing arbitrage. Moreover, in empirical applications, mean-variance analysis is well known to often deliver extreme and erratice portfolio weights (see e.g., Guidolin, 2013).
Because of these limitations of standard mean-variance analysis, a literature has developed that assumes instead a power utility function of portfolio returns (or terminal wealth, which is identical for one-period horizons):
|
V(RPt+1)={(1+RPt+1)1−γ1−γγ≠1,γ>0ln(1+RPt+1)γ=1.
|
(4)
|
The preferences in (4) imply constant relative risk aversion (CRRA) and practically that investment and consumption decisions will not be affected by the scale of the problem, i.e., the optimization will be framed in terms of relative quantities, such as portfolio weights and the consumption-wealth ratio. Since the seminal work by Scott and Horvath (1980) (see also the review in Guidolin, 2013), it is well known that under appropriate conditions (such as continuity and differentiability) a simple Taylor expansion of the expected utility in (4) around expected portfolio return,
|
Et[V(RPt+1)]=∞∑k=01k!∂kV(Et[RPt+1])∂(RPt+1)kEt[(RPt+1−Et[RPt+1])k]
|
(5)
|
allows us to establish a precise functional relationship between the classical mean-variance objective in (2) and power utility in (4). Because when a truncation is applied at k =4 and Et[(RPt+1−Et[RPt+1])]=0 (when k =1), we have
|
Et[V(RPt+1)]≅V(Et[RPt+1])+12V″(Et[RPt+1])Vart[RPt+1]+16V‴(Et[RPt+1])SPt,t+1+124V⁗(Et[RPt+1])KPt,t+1,
|
(6)
|
(SPt,t+1 and KPt,t+1 indicate the third and fourth moments of portfolio returns), for k =2
|
VMV(RPt+1)≡Et[V(RPt+1)]Et[RPt+1]V(Et[RPt+1])≅Et[RPt+1]+12V″(Et[RPt+1])Et[RPt+1]V(Et[RPt+1])Vart[RPt+1]=Et[RPt+1]−12λVart[RPt+1].
|
(7)
|
gives mean variance preferences in which the coefficient of risk aversion is proportional, apart from a positive constant E[RPt+1]/V(Et[RPt+1]), to the second derivative of the utility function V(RPt+1) to portfolio returns. In the case of risk aversion, we therefore expect V″(Et[RPt+1])<0 and therefore λ≡−V″(Et[RPt+1])Et[RPt+1]/V(Et[RPt+1]) When k > 2, (5) and (6) allow us to consider preferences that assign a weight to moments greater than the second, especially skewness and kurtosis, therefore incorporating both asymmetries and fat tails in portfolio choice. Note that even though in principle (5) features an infinite sum while (6) stops the Taylor expansion at the fourth order, Guidolin and Timmermann (2005) and Jondeau and Rockinger (2006) have shown that for many often-used utility functions, the four-moment functional in (6) provides a close approximation to the convergent series in (5).
Applying now the result in (6) to the case of power utility function, we have (for the case γ≠1):
|
Et[V(RPt+1)]≅(1+Et[RPt+1])(1−γ)1−γ−γ2(1+Et[RPt+1])(γ−1)σ2p,t+1+γ(γ+1)6(1+Et[RPt+1])(γ−2)SPt,t+1−γ(γ+1)(γ+2)24(1+Et[RPt+1])(γ−2)KPt,t+1,
|
(8)
|
Note that when ∧ > 0, then ∧/2 > 0 so that the investor dislikes variance, ∧(∧ + 1)/6 > 0 and the investor shall prefer to have a larger exposure to SPt,t+1, and ∧(∧ + 1)(∧ + 2)/24 > 0 so that the approximate expected utility functional declines in KPt,t+1. In what follows, we shall use (8) to approximate power utility-driven asset allocation results. Under approximate power utility, different strategies or portfolios can be ranked either directly on the basis of their OOS average realized utility,
|
ˉV(RPt+1)=1TT−1∑t=1(1+RPt+1(ˆωt,t+1))(1−γ)1−γ,
|
(9)
|
or through the corresponding certainty equivalent return (CER):
|
(1+E[RPt+1])(1−γ)1−γ=ˉV(RPt+1)⇒CER=[(1−γ)ˉV(RPt+1)]11−γ−1
|
(10)
|
Because it is a standardized, relative measure, we prefer the latter. Finally, we also consider the case of an investor whose preferences are described through a negative exponential, CARA (Constant Absolute Risk Aversion) utility function,
|
V(RPt+1)=−exp[−θ(1+RPt+1)],
|
(11)
|
where θ is the coefficient of absolute risk aversion. Again we consider a Taylor expansion of the fourth order to determine the optimal allocation:
|
Et[V(RPt+1)]≅exp[−θ(1+Et[RPt+1])](−1−θ22σ2p,t+1+θ36Sp,t+1−θ424Kp,t+1)
|
(12)
|
Also in this case, the realized risk-adjusted performances in back-testing OOS exercises are either computed as ˉV(RPt+1)=1TT−1∑t=1exp[−θ(1+RPt+1(ˆωt,t+1))] or
|
exp[−θ(1+E[RPt+1])]=ˉV(RPt+1)⇒CER=−1θ[lnˉV(RPt+1)]−1
|
(13)
|
3.3. Backtesting design
Under each of the three utility functions in (2), (4), and (11)—always approximated as a fourth-order Taylor expansion (but in the case of mean-variance preferences, k =2 and the formula becomes exact)—we compute optimal portfolio weights and realized, risk-adjusted performances, in three scenarios:
▪ A baselines case where investment in volatility is not available, and the asset menu is limited to three US risky assets, that is, equities, government and corporate bonds, and real estate.
▪ Next, we expand this asset menu by introducing volatility as an asset class, in this case proxied by the non-investable VIX index.
▪ Finally, in the asset menu we replace VIX with an exchange traded note that in principle replicates the movements in the VIX (see Section 4 for details).
Under each of the three scenarios and for each week in the back-testing period t =1, 2, …, T, we choose the optimal portfolio, subject to no short-sale constraints, by solving (see Sharpe, 2006):
|
maxωt,t+1V(Et[RPt+1(ωt,t+1)])+12V″(Et[RPt+1(ωt,t+1)])Vart[RPt+1(ωt,t+1)]+16V‴(Et[RPt+1(ωt,t+1)])SPt,t+1(ωt,t+1)+124V⁗(Et[RPt+1(ωt,t+1)])KPt,t+1(ωt,t+1),
|
(14)
|
where V(⋅) is the function in (2), (4), or (11), and the conditional central moments (Et[RPt+1(ˆωt,t+1)], Vart[RPt+1(ˆωt,t+1)], and SPt,t+1(ˆωt,t+1)) are computed on the basis of the sample up to time t. In other words, we use expanding window sample estimators of mean, variance, asymmetry, and tail-thickness as a function of the portfolio weights. In particular, for the quadratic utility case, we consider λ =0.1, 0.2, 0.5, or 1; for the power utility function we entertain the values λ =2, 4, 7, and 10 (see also Hafner and Wallmeier, 2008); finally, for the negative exponential utility function, we use alternative values θ =0.5, 1, 1.25, and 2.
The back-testing sample is built in the following way. The first 56 weekly observations provide a starting estimation sample for all the exercises subsequently performed. We then recursively updated and expand the corresponding sample at a monthly frequency, i.e., at each step we increase the estimation sample by 4 observations.8 Therefore, for each choice of utility function and risk aversion parameters (λ,γ, and θ, respectively), we obtain 86 monthly portfolios. Each time series of portfolios generates 86 realized monthly returns of which we compute and compare both basic summary statistics and risk-adjusted performances, in the form of Sharpe ratios and appropriate CER estimates.
8 Expanding the estimation window on every single week would imply rebalancing the optimal portfolio on a weekly basis. This would be unusual because in most real-life situations, this would imply very high transaction costs and fail to reflect the practice of the asset management industry.
4. The Data
4.1. Asset classes and their ETP proxies
Our empirical analysis is based on weekly (Wednesday-to-Wednesday, whenever possible) series of discretely compounded (dividend-adjusted) return data from January 2009 to February 2016. The investment universe includes four asset classes, stocks, bonds, real estate, and—in two exercises out of three—volatility. To be consistent with the analysis performed in the case in which volatility is tradable, we have a preference for ETF returns data also in the case of asset classes different from volatility. In particular, we opt for ETFs that engage in the physical replica of the underlying indices. Equities are represented by the SPDR S & P 500 ETF (ISIN: US78462F1030, ticker SPY) that replicates the S & P 500 index. Bond performance is reflected by returns on the iShares Core US Aggregate Bond ETF (ISIN: US4642872265, ticker AGG). The iShares US Real Estate ETF is used as a proxy for the performance of real estate investments (ISIN: US4642877397, ticker IYR). Subsequently, this baseline asset menu is extended to include alternately the VIX index (see http://www.cboe.com/products/vix-index-volatility/vix-options-and-futures/vix-index) or the iPath S & P 500 VIX ST Futures ETN (ISIN: US06740Q2527, ticker VXX). The latter ETN reflects the returns on a strategy that continuously owns a rolling portfolio of one-and two-month VIX futures contracts to target a constant weighted average futures maturity of 1 month. All these ETPs are chosen among a few similar alternatives because of their high liquidity, trading volumes, and size: in fact, amongst all listed ETFs and ETNs, these report the largest daily volume and largest number of outstanding shares.9 This reduces possible data biases due to poor liquidity. Of course, we must accept that our empirical conclusions will be affected by our specific selection of the ETPs analyzed, even though these four ETPs are based on a physical replica of the underlying indices that should somewhat minimize the margins for discretionary choices. However, should we have considered other EPTs that are not subject to physical replica or that over time may have switched on and off to synthetic replication strategies (e.g., based on OTC swaps), then the corresponding returns may have significantly differed in spite of an identical underlying index/asset. The data on VXX prices are taken from Bloomberg and, given the good traded volume of this ETN, these represent Wednesday, last-traded market prices gross of fund expenses and fees. No data cleaning were applied to purge spikes or "winsorize" the data, as these are weekly return data that correspond to market transactions.
9 Two obvious alternatives would have been VIIX and the VXZ ETNs. VIIX, issued by Credit Suisse/Velocity Shares, has been launched in 2010 and as of mid-2017 had a size of approximately 12.5 million USD vs. approximately 1 billion for VIX; XVZ, issued by Barclays iShare, was launched in 2011 and has AUM of approximately 16 million USD, and with positions in medium-term VIX futures. However, the trading volumes of VIIX and XVZ over October 2016 – August 2017 have been 70 miilion and approximately half a million USD vs. 11 billion USD in the case of VIX.
On the one hand, our choice of the sample period—starting right in the middle of the US Great Financial Crisis—is entirely due to data availability, without any subjective meddling or discretion. Although the VIX has been computed and reported by the CBOE since 1992, the first ETN that tracks VIX performance was indeed listed in January 2009, and it is indeed VXX, the iPath S & P 500 VIX ST Futures ETN. On the other hand, a weekly frequency ensures a sample of 400 observations for each asset class, which guarantees a sufficient size for expanding window sample moments to be estimated with some precision. In what follows and in the tables and figures, the different asset classes will be often identified by their acronyms/tickers (SPY, AGG, IYR, VIX or VXX, respectively) to save space.
4.2. Summary statistics
Table 1 presents summary statistics. The first column shows the average performance for each asset class. As expected, bonds are the asset class with the lowest mean return and risk, 0.01% and 0.25% (standard deviation), respectively. Equities and real estate show instead similar average returns and risk, 0.14% and 1.15% per week in the case of SPY and 0.15% and 1.74% in the case of IYR. Interestingly, in spite of the financial crisis, real estate still gave an annualized mean return of almost 8% over our sample (that does not include 2008); however, the effects of the crisis emerge in the relatively high standard deviation, 12.5% in annualized terms. Both equities and real estate imply rather striking Sharpe ratios of 0.11 and 0.08 per week. However, IYR returns imply large excess kurtosis, to indicate that risks not completely captured by classical variance may be present.
Table 1. Summary statistics.
QFE-01-00334-g0T1.jpg
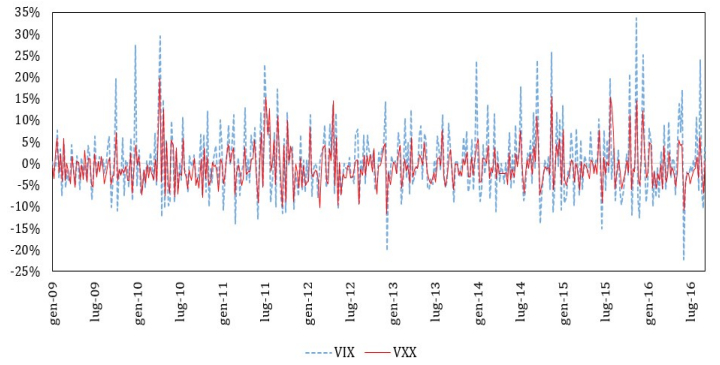
It is most interesting to analyze the properties of volatility as an asset class. In Table 1, it emerges that—on the wake of its surge during 2009–2010, in the midst of the financial crisis—VIX is characterized by a large and positive weekly mean return of 0.46% per week, but also by negative and large median, of -0.70%. This is indeed an indication of massive right-skewness (1.97) and kurtosis (9.14): the VIX spiked up in a few weeks only in our sample, but slowly declined most of the time, which explains the massive difference between mean and median. As a result, also the weekly standard deviation of VIX exceeds 8%, i.e., it is almost 5 times that of IYR and 7 times that of stocks. Of course, while a simple mean-variance framework will be unfit to capture these differences, other types of utility function may succeed in capturing the interaction between the estimated joint density of the data and optimal portfolio weights. Interestingly, the ETN returns on VXX have radically different properties: both mean and median are negative in this case, even though positive skewness remains (1.06). This is due to the well-known tendency of ETPs written on the VIX to "bleed" funds due to the massive roll costs when the term structure of VIX futures is downward sloping (in contango, which happens most of the time, see Alexander and Korovilas, 2013) and expiring futures have to be replaced by more expensive, longer-term futures, see e.g., the analysis in Whaley (2013). Moreover, the asset management firms in charge of managing ETNs do charge annual fees, for instance a 0.89% annual expense ratio in the case of VXX.
As a result, a mean return of -0.67% per week is hard to ignore. However, rolling over VIX futures seems at least to have stabilizing effects in terms of realized standard deviation of VXX returns, with a 4.52% per week that halves the volatility of the underlying VIX index. Interestingly, this occurs mainly because VXX reduces portfolio exposure to extremely negative relative changes in the VIX: the minimum of VIX is -22.2% in a single week vs.
-11.6% only for VXX. Figure 1 shows that VIX and VXX display a similar return dynamics over time, but that the amplitude of the two series turns out to be rather different, with VIX relative percentage changes covering a weekly range that is visually approximately half the one shown by VIX. In fact, the pairwise correlation between VIX and VXX is 0.85.
A Reader may feel hurried to conclude that while VIX is a value-enhancing but very risky asset class, to entertain VXX exposures turns out to be utterly pointless. However, the lower panel of Table 1 that displays pairwise correlations complicates the picture somewhat. VIX and VXX display essentially identical correlations with respect to stocks (negative and large) and bonds (small, yet allowing considerable hedging); however, when it comes to real estate, VXX returns a correlation that is even more negative vs. the underlying VIX. Therefore volatility is clearly useful for hedging purposes and, if any, VXX is a bit more valuable than VIX itself.
5. Empirical Results
5.1. Recursive optimal portfolio weights
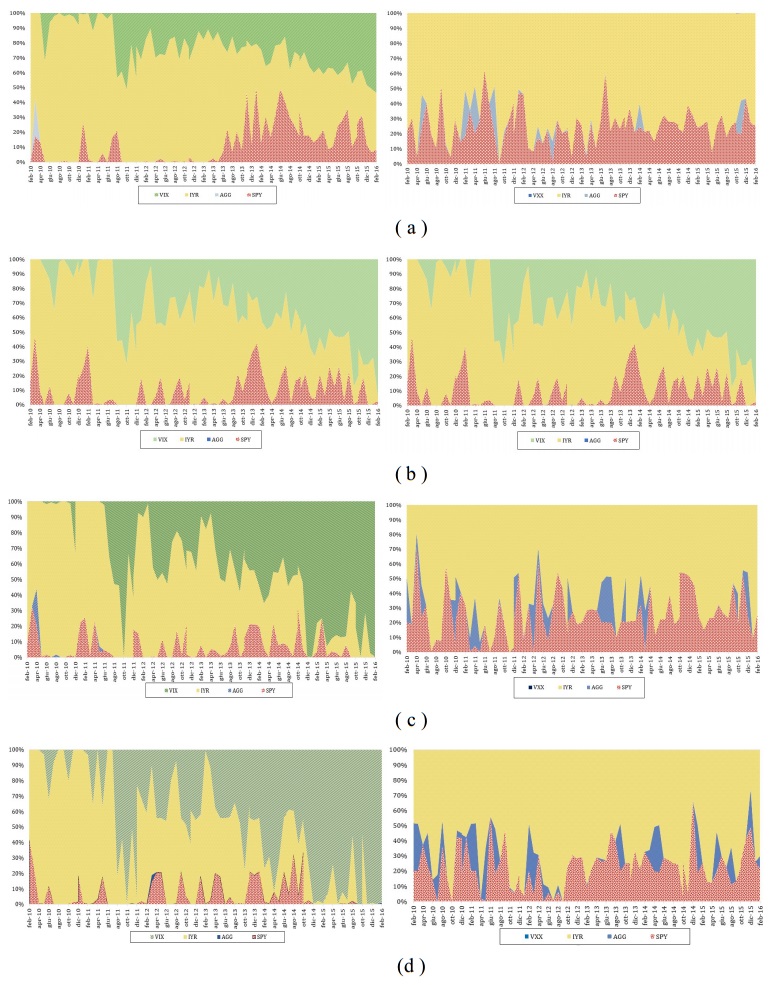
In this section, we analyze the results from recursive portfolio choice under the different preference frameworks introduced in Section 3. Starting from the mean-variance case, Figure 2 shows the composition of optimal portfolios between February 2010 and February 2016. The four plots on the left, for different choices of the risk aversion coefficient λ, show weights when VIX is part of the asset menu; the corresponding plots to the right display comparable figures when VXX replaces VIX in the asset menu. On the left, VIX carries high weights, especially during the Fall 2010 European sovereign debt crisis and starting in late 2014. In the case of a highly risk averse investor (λ =1), in fact VIX comes to completely dominate the portfolio. On the contrary, VXX only finds a limited role—in the order of 10-15% at most and only episodically—for highly risk-averse investors. In fact, the role of moderating the total amount of risk taken up as a result of portfolio optimization is instead played by bonds, which practically denies a role to the de-correlation properties of volatility-related ETPs. Equivalently, while if the VIX were directly available, we would expect to see a modest demand for bonds at best and the level of portfolio risk would be controlled by varying over time the commitment to VIX, thanks to its low or negative correlations, when an investor can at most trade VXX the result is that the most effective way to limit risk exposure is to reduce the overall weight of real estate and equities in favor of relatively riskless bonds, a rather traditional strategy.
Figure 3 shows the composition of the optimal portfolios for an investor who maximizes expected CRRA utility when the risk aversion parameter takes the alternative values of 2, 4, 7, or 10. As explained in Section 3, by contrasting these weights with those in Figure 2, one may derive a visual summary of the relevance of higher-order moments for the composition of the optimal portfolio. The optimal portfolio shares—also because the riskless asset is excluded from the menu—do not seem to strongly depend on the assumed value for ∧ even though the role played by the investment in volatility and in bonds increases slightly as we move down the plots. The fact that the percentage invested in bonds does not strongly increase may seem surprising at first, even though from Table 1 one should note that under CRRA preferences—because of their large negative skewness and positive excessive kurtosis—also bonds are quite risky in spite of their modest volatility. However, the percentage committed to bonds appears to be structurally high, ranging between an average 40% in the case of ∧ =2 to 80% in the case of a highly risk-averse individual characterized by ∧ =10. At low risk aversion levels, a substantial weight also goes to real estate, while equities always enter modestly.
As far as volatility is concerned, we obtain indications that are very different from Figure 2: the optimal weight assigned to long volatility positions is always positive but modest over time; it is slightly higher when VXX is used (15-20%) than under VIX (less than 15% but with a spike in excess of 30% in late 2015, in correspondence to the so-called "taper tantrum"). Moreover, the dynamics over time of the two positions appear to be similar. These results are interesting besides the case under consideration: there is persistent, albeit modest, demand of an asset with a large, negative mean and median return; this occurs because the asset has large and negative correlation with the asset class in the highest demand, real estate. In fact, even though VXX has an average return that is considerably inferior to VIX, it is in higher demand because it brings positive skewness and low (negative) excess kurtosis to the portfolio. In other words, while the standard VIX is a good hedge only in the classical portfolio mean-variance dimension, VXX acts as a hedge also in additional dimensions that a power utility function is able to pick up, skewness and kurtosis. The full importance of higher-order moments in portfolio choice (see also Guidolin, 2013, and Guidolin and Timmermann, 2008) can be easily appreciated by contrasting Figures 2 and 3, that give rather different portfolio allocations based on identical asset classes and data: contrary to what one sometimes reads, mean-variance can be—and in this case is—a very poor approximation to CRRA-implied portfolio allocation. Interestingly, but consistently with what has been already observed in earlier research (see Guidolin, 2013), power utility-driven portfolio decisions turn out to be more stable than typical mean-variance selections.
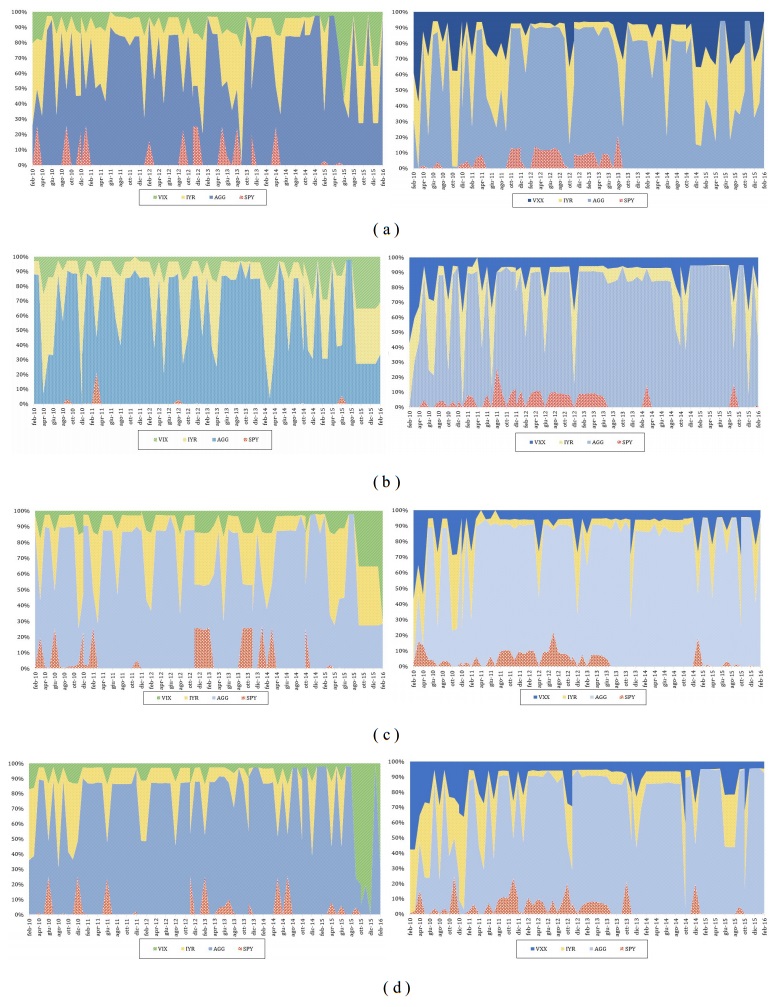
An unreported series of charts similar to Figure 2 (and available upon request) examine the optimal allocation under negative exponential, CARA preferences. It is well known that when the joint, multivariate distribution of the N asset returns is normal, the overall portfolio return distribution will be normal and CARA preferences give the same optimal portfolio allocation as the mean-variance case. However, as emphasized by Table 1, this is not exactly the case for our asset menu. Therefore the CARA case may lead to different portfolio decisions vs. the mean-variance framework. While CARA preferences with Σ ≥ 1 yield rather cautious portfolio decisions in which only 60% at most of the portfolio is invested in real estate and stocks, the way in which such risk mitigation is obtained depends on whether the VIX is (counter-factually) assumed to be an investable index or not. In the former case, the portfolios include a substantial proportion, up to an average 35% and increasing over time in the case of Σ =2, with the rest of the portfolio largely invested in real estate; in the latter case, the optimal portfolios use little or no VXX and instead exploit the low risk exposure of bonds, as noted in Figure 2. Therefore, the CARA utility is indeed the case in which the differences in the attractiveness of VXX vs. VIX are the largest, as the former vehicle is tradable but essentially never part of the optimal investment decision of an investor. Implicitly, it is then clear that maximizing the approximate expected utility in (12) must lead in this case to a strong aversion to the negative mean returns of VXX manifested during the latter part of our sample that prevails over the liking for its positive skewness and negative excess kurtosis, contrary to what had occurred in Figure 3. Figure 4 is then helpful to drive home one of the key implications of our analysis: in general, the attitude of an optimizing investor with regards to a negative mean (and median) asset strictly depends on her specific preferences; in particular, whether or not popular ETPs used to trade US aggregate market volatility may be beneficial to portfolio decisions, strongly depends on the specific preferences of an investor. In short, optimal portfolio management ought to be strictly tailor-made, even in the face of an identical representation of the set of efficient investment opportunities.
6. Backtesting Risk-Adjusted Economic Value
On the basis of the optimal, recursive estimates of portfolio shares obtained through the recursive process implemented in Section 5, in this section we focus on indicators that allow to quantify the costs and benefits of investing in volatility and whether the specific tradable vehicle through which this is performed carries any importance. In practice, we compute, average, and report a range of performance measures across the 86 monthly portfolio allocations, each updated at a monthly frequency, already visualized in Figures 2-4. In Section 6.1, we focus on a small set of general-interest and commonly reported measures in money management, such as Sharpe ratios. In Section 6.2, we focus instead on CERs, utility-based measures that perform the risk adjustment in a way that is fully consistent with the structure of the assumed preferences. In all cases, we compute the measures as means of 5-year moving averages to simulate the perspective of an investor with a plausible 5-year horizon.10
10 Results turned out to be robust to using the full sample or different rolling window lengths.
6.1. Classical performance measures
Table 2 reports Sharpe ratio indices as in (3) for each of the three preferences frameworks investigated in Section 5. The risk-free rate is proxied by the 1-month T-bill rate. Although the Sharpe index tends to be publicized by asset managers irrespectively of the preferences of their investors more as a description of the relationship of their selected portfolio to the MVF than anything else, from Section 3, we already know that (3) represents the correct performance measure to rank portfolios only in a mean-variance perspective.
Table 2. Realized out-of-sample sharpe ratios.
|
Baseline |
Portfolio with VIX |
Portfolio with VXX |
| Panel A – Quadratic utility function |
|
|
|
| 5 yrs RW |
λ = 0.1 |
0.0477 |
0.0509 |
0.0128 |
| λ = 0.2 |
0.0273 |
0.0233 |
0.0166 |
| λ = 0.5 |
0.0219 |
0.0439 |
0.0310 |
| λ = 1 |
0.0415 |
0.0381 |
0.0174 |
| Panel B – Power utility function |
|
|
| 5 yrs RW |
γ = 2 |
0.1308 |
0.0537 |
0.0256 |
| γ = 4 |
0.0154 |
0.0614 |
0.0056 |
| γ = 7 |
0.0154 |
0.0278 |
0.0197 |
| γ = 10 |
0.0077 |
0.0454 |
0.0058 |
| Panel C – Negative exponential utility function |
| 5 yrs RW |
θ = 0.5 |
0.0395 |
0.0254 |
0.0104 |
| θ = 1 |
0.0480 |
0.0266 |
-0.0146 |
| θ = 1.5 |
0.0400 |
0.0943 |
0.0327 |
| θ = 2 |
0.0229 |
0.0851 |
0.0734 |
| Note: This table reports the ex-post, realized Sharpe ratios under alternative preferences and asset menus. Each panel shows results for different assumptions on the risk aversion coefficients. |
We start with panel B of Table 2, i.e., under power utility preferences, the case in which in Section 5 we have obtained the biggest quantitative role for VXX. In spite of this, it is clear that while for ∧ =4 and higher (i.e., intermediate and high risk aversion), the availability of VIX would increase the realized Sharpe ratio (e.g., from 0.015 to 0.028 in the case of ∧ =7), VXX fails to do that uniformly and it always implies a visible decline in Sharpe ratio vs. VIX. However, VXX does better than dropping volatility as an asset class altogether in the case of ∧ =7. Interestingly, this result weakens in panel A, for the case of simpler, mean-variance preferences: VIX sometimes improves the realized OOS Sharpe ratio (it happens for λ =0.1 and 0.5, therefore when risk aversion is low to intermediate), but VXX does that only one case. Under mean-variance preferences the result is hard to interpret because it seems to suffer from being based on a back-test sample that is not long enough to reach a firm conclusion. Panel C, devoted to the negative exponential utility case, confirms these conclusions: while VIX may create economic value under some risk aversion assumptions, this is not likely when VXX is used.
Another indicator often reported to evaluate performance is the index proposed by Sortino, a version of (3) where the denominator is just downside standard deviation, i.e.,
|
^SortinoT≡T−1∑T−1t=1(RPt+1−rft)√T−1∑Tt=1I{RPt+1−rft<T−1∑T−1t=1(RPt+1−rft)}[(RPt+1−rft)−T−1∑T−1t=1(RPt+1−rft)]2,
|
(15)
|
The idea of (15) is that only under-performance vs. the mean represents genuine risk. Table 3 shows the realized, OOS Sortino ratios under alternative preferences. The comments and conclusions drawn from the analysis of the realized Sharpe ratios are confirmed and strengthened here. VIX often improves realized performance, although in panel B this happens to a larger extent only for low-risk averse investors. Possibly, the presence of VIX in the portfolio during 2010 would have simply helped to avoid some substantial losses that were otherwise incurred. In general, an analysis of the realized downside volatility of portfolios including VIX/VXX vs. the benchmark, shows that although including volatility always reduces it, in the case of VXX the effect of mean reduction prevails, leading to an overall decline of the Sortino index. In panel B, for highly risk averse investors, in fact we obtain a few negative Sortino ratios when VXX investing occurs, indicating that the benchmark would be superior.
Table 3. Realized out-of-sample sortino ratios.
|
Baseline |
Portfolio with VIX |
Portfolio with VXX |
| Panel A – Quadratic utility function |
|
|
|
| 5 yrs RW |
λ = 0.1 |
0.0975 |
0.0890 |
0.0235 |
| λ = 0.2 |
0.0822 |
0.0756 |
0.0226 |
| λ = 0.5 |
0.0791 |
0.0567 |
-0.0475 |
| λ = 1 |
0.0469 |
0.0991 |
0.0213 |
| Panel B – Power utility function |
| 5 yrs RW |
γ = 2 |
0.0978 |
0.1227 |
0.0152 |
| γ = 4 |
0.0277 |
0.0980 |
0.0079 |
| γ = 7 |
0.0412 |
0.0644 |
-0.0058 |
| γ = 10 |
0.0289 |
0.0512 |
-0.0081 |
| Panel C – Negative exponential utility function |
| 5 yrs RW |
θ = 0.5 |
0.0395 |
0.0254 |
0.0104 |
| θ = 1 |
0.0480 |
0.0266 |
-0.0146 |
| θ = 1.25 |
0.0400 |
0.0943 |
0.0327 |
| θ = 2 |
0.0229 |
0.0851 |
0.0734 |
| Note: This table reports the ex-post, realized Sortino ratios under alternative preferences and asset menus. Each panel shows results for different assumptions on the risk aversion coefficients. |
Finally, in Table 4, we consider the realized OOS information ratios. This indicator is defined as the ratio between the average return in excess of the market portfolio (here proxied by the S & P 500) and the realized sample standard deviation of this excess, also known as tracking error volatility:
|
InformationRatio=T−1∑T−1t=1(RPt+1−RMktt+1)√T−1∑Tt=1[(RPt+1−RMktt+1)−T−1∑T−1t=1(RPt+1−RMktt+1)]2.
|
(16)
|
Table 4. Realized out-of-sample information ratios.
|
Baseline |
Portfolio with VIX |
Portfolio with VXX |
| Panel A – Quadratic utility function |
|
|
|
| 5 yrs RW |
λ = 0.1 |
0.0250 |
0.0470 |
0.0030 |
| λ = 0.2 |
0.0237 |
0.0193 |
-0.0009 |
| λ = 0.5 |
0.0559 |
0.1153 |
0.0553 |
| λ = 1 |
0.0073 |
0.0923 |
-0.021 |
| Panel B – Power utility function |
| 5 yrs RW |
γ = 2 |
0.0978 |
0.1227 |
0.0152 |
| γ = 4 |
0.0277 |
0.0980 |
0.0079 |
| γ = 7 |
0.0412 |
0.0644 |
-0.0058 |
| γ = 10 |
0.0289 |
0.0512 |
-0.0081 |
| Panel C – Negative exponential utility function |
| 5 yrs RW |
θ = 0.5 |
0.0395 |
0.0254 |
0.0104 |
| θ = 1 |
0.0480 |
0.0266 |
-0.0146 |
| θ = 1.25 |
0.0400 |
0.0943 |
0.0327 |
| θ = 2 |
0.0229 |
0.0851 |
0.0734 |
| Note: This table reports the ex-post, realized information ratios under alternative preferences and asset menus. Each panel shows results for different assumptions on the risk aversion coefficients. The S & P 500 index has been used as benchmark. |
The point of this indicator is to measure abnormal performance generated by a portfolio strategy vs. a benchmark. In this sense, in the asset management industry, (16) is often perceived to be more practical and relevant than the Sharpe and Sortino ratios, that instead implicitly adopt riskless cash investments as their benchmarks. In a CAPM perspective, it is natural to center the IR index on the market portfolio, even though the subsequen identification of the latter with a broad market index is somewhat arbitrary. Moreover, on a closer inspection, in our study, producing IR rankings may be felt as natural because—when it comes to assess the use VIX/VXX as an asset class—we are considering an investor willing to take on a certain level of risk which suggests that her natural benchmark will not be represented by 1-month T-bills. The evidence provided by the realized OOS information ratios in Table 4 is in line with what is shown by the Sharpe and Sortino ratios: including the VIX in the asset menu tends to produce positive and often relevant economic value; with very few exceptions, replacing VIX with tradable VXX considerably lowers the information ratios; occasionally, the asset menus that include VXX give negative information ratios, i.e., trading volatility through the ETN gives realized OOS information ratios below a simple CAPM-like market portfolio buy-and-hold strategy.
To complete the analysis, we also compute and report one measure of portfolio turnover to quantify the needed, implied average rebalancing in each period to keep the portfolio structure at the optimal weights, for each strategy. The formula used follows, for instance, DeMiguel, Garlappi and Uppal (2009): for each asset allocation program (as defined by preferences and asset menu), portfolio turnover is defined as the sum of the changes in the absolute value of weights to the N assets between time t and t + 1 (in our application, on a monthly basis), for t =1, 2, …, T -1:
|
Turnover=1T−1T−1∑t=1N∑j=1(|wj,t+1−wj,t|).
|
(17)
|
In our case, N =3 and 4, and T =86 months. Usually, the calculation of a measure like (17) is a prelude or a proxy for full consideration of transaction costs: the more a portfolio is turned over in time, the higher total transaction costs are, whatever is their measure per unit trade.
Table 5 shows the portfolio turnover rate for the case of power utility. We just deal with this case because this is the one in which both the addition of VIX and of VXX to the asset menu (occasionally) led to an improvement in risk-adjusted performance, according to a Sharpe ratio metric. As one would expect, turnover declines as the coefficient ∧ increases: investors who are less and less aggressive in their portfolio approach, trade less and less. Moreover, while the asset menu that includes VIX ought to be approximately traded with the same intensity as the benchmark portfolio, we note that VXX-augmented portfolio are considerably more stable, i.e., inserting VXX has a stabilizing effect and does not lead to potentially higher transaction costs that would otherwise go to compound the negative mean and median returns from the asset class.
Table 5. Portfolio turnover under power utility, CRAA preferences.
|
γ |
2 |
4 |
7 |
10 |
| Benchmark Case |
|
|
|
|
|
| Turnover |
|
64.93% |
55.04% |
50.24% |
48.13% |
| Portfolio with VIX |
|
|
| Turnover |
|
62.52% |
53.22% |
54.20% |
46.83% |
| Portfolio with VXX |
|
|
| Turnover |
|
50.73% |
47.96% |
47.86% |
44.62% |
6.2. Utility-based, risk-adjusted performance
In this Section, we proceed to comment on the most appropriate measures of risk-adjusted performance, the OOS realized CER estimates that take into account the specific type of preferences that were used to optimize and obtain portfolio weights. In fact, what we report in Table 6 are the CER losses/gains from switching between different portfolio rules and asset menus: VIX vs. the baseline which gives a virtual indication of the CER gains from adding volatility to the menu of choice; VXX vs. the baseline, which gives a practical estimate of the percentage gain/loss from adding to the asset menu a tradable ETN that should track the VIX over time; VIX vs. VXX, which measures what is the additional risk-adjusted performance that an investor should be able to obtain by using ETPs of better quality—with lower tracking error vs. the VIX—than the specific ETN used in our analysis. In the light of the literature reviewed in Section 2, we expect the CER difference from VIX vs. the baseline to be positive and potentially large; we have uncertain priors as to the difference of VXX vs. the baseline in the light of the evidence from Sections 3 through 5; we certainly anticipate the CER difference of VIX vs. VXX to be positive, even though it would be good news to practitioner were such differences to be small. It is important to emphasize that a positive CER gain when going from the benchmark to a VIX-augmented asset menu is not a logical necessity, according to the reasoning that when the asset menu is expanded, an investor can only gain, also after applying appropriate risk-adjustments. In fact, in this paper we are considering realized OOS changes in CER and even though ex-ante, based on in-sample estimates these cannot be but positive, out-of-sample any sign can be obtained. Note that the same applies to the comparison VIX vs. VXX.
Starting from panel B of Table 6, concerning CRRA preferences, we find mixed results. In OOS back-testing exercises, we obtain robust evidence that all in all, VIX does not convincingly create economic value to power utility investors, even the very risk-averse ones. For instance, for ∧ =7, the weekly difference in CERs is 7 basis points (bps), however small, but one may argue to build up to a total in excess of 3% per year. However, as ∧ increases, the standard deviation of the sample mean CER difference becomes very large, an indication that in a formal test, it would be unlikely that a null hypothesis of no difference could be rejected. When we compare VXX with the benchmark, because of its negative mean and median returns (and in spite of positive skewness and negative excess kurtosis), VXX does even worse: for instance, assuming ∧ =7, the weekly difference in CERs is almost 9 bps. Therefore going from VIX to VXX makes CERs worse and by substantial percentage amounts; for instance, when ∧ =7, an investor would pay a fee up to 2 and half bps per week to access direct VIX trading and avoid the VXX. However, also in this case, these sample means are estimated very imprecisely. All in all, panel B of Table 6 shows that in ex-ante terms, VXX would be demanded by a risk-averse investor with power utility, but that—at least in our back-testing OOS period, Feb. 2010 – Feb. 2016—it would have betrayed this very investor in ex-post terms, by inflicting risk-adjusted performance losses. However, this does not derive entirely from an ineffectiveness of the ETN examined in this paper: the VIX itself would suffer from the same limitations.
Table 6. Annualized certainty equivalent return loss.
|
λ |
|
0.1 |
0.2 |
0.5 |
1 |
| Panel A – Quadratic utility function |
|
|
|
|
|
|
| Portfolio with VIX vs Baseline |
|
|
|
|
|
|
| Sample Mean |
|
|
0.013% |
0.010% |
-0.001% |
-0.018% |
| Std. Dev. of Mean |
|
|
0.285% |
0.216% |
0.017% |
0.389% |
| Portfolio with VXX vs Baseline |
|
|
|
|
|
|
| Sample Mean |
|
|
-0.000% |
0.000% |
0.000% |
0.000% |
| Std. Dev. of Mean |
|
|
0.024% |
0.016% |
0.001% |
0.018% |
| Portfolio with VIX vs Portfolio with VXX |
|
|
|
|
|
|
| Sample Mean |
|
|
0.013% |
0.010% |
-0.001% |
-0.018% |
| Std. Dev. of Mean |
|
|
0.285% |
0.216% |
0.017% |
0.389% |
| Panel B – Power utility function |
|
|
|
|
|
|
| Portfolio with VIX vs Baseline |
|
|
|
|
|
|
| Sample Mean |
|
|
-0.001% |
-0.023% |
-0.061% |
-0.399% |
| Std. Dev. of Mean |
|
|
0.152% |
1.617% |
5.833% |
22.208% |
| Portfolio with VXX vs Baseline |
|
|
|
|
|
|
| Sample Mean |
|
|
-0.002% |
-0.014% |
-0.087% |
-0.455% |
| Std. Dev. of Mean |
|
|
0.152% |
1.525% |
7.609% |
25.386% |
| Portfolio with VIX vs Portfolio with VXX |
|
|
|
|
|
|
| Sample Mean |
|
|
0.002% |
-0.009% |
0.026% |
0.056% |
| Std. Dev. of Mean |
|
|
0.074% |
1.166% |
1.660% |
10.207% |
|
Panel C – Negative exponential utility function
|
|
|
|
|
|
|
| Portfolio with VIX vs Baseline |
|
|
|
|
|
|
| Sample Mean |
|
|
0.000% |
0.001% |
0.002% |
0.002% |
| Std. Dev. of Mean |
|
|
0.152% |
0.187% |
0.264% |
0.264% |
| Portfolio with VXX vs Baseline |
|
|
|
|
|
|
| Sample Mean |
|
|
0.000% |
0.000% |
0.000% |
0.000% |
| Std. Dev. of Mean |
|
|
0.016% |
0.015% |
0.013% |
0.016% |
| Portfolio with VIX vs Portfolio with VXX |
|
|
|
|
|
|
| Sample Mean |
|
|
0.000% |
0.001% |
0.002% |
0.002% |
| Std. Dev. of Mean |
|
|
1.414% |
1.732% |
2.236% |
2.449% |
| Note: The certainty equivalent return (CER) loss is calculated as a difference between the certainty-equivalent returns obtained from two alternative portfolio strategies and can be interpreted as the maximum, yearly percentage fee that an investor should be ready to pay in order to switch between two alternative portfolio strategies. |
Panel A of Table 6 deals instead with the case of mean-variance preferences. Here, we obtain perfect consistence between in-sample and OOS results. First, whether or not volatility as an asset class helps in portfolio decisions depends on the risk-aversion coefficient, λ. Aggressive investors (with modest λ) tend to benefit from the availability of VIX in the order of about 1 bp per week, which any way amounts to more than 0.5% a year; however, more risk averse investors derive small benefits because they prefer to moderate their risk exposures by investing in bonds. Second, VXX yields a positive but always marginal risk-adjusted benefit, just a fraction of 1 bp. However, for the most risk-averse investors, interestingly this means that in our OOS tests, the ETN outperforms the underlying VIX. For an investor characterized by λ =1, the difference is almost 2 bp per week and amounts to a round 2% per year. Even though this was not obvious from Figure 2, it turns out that VIX would be in too high a demand because of its past positive performance so to end up hurting performance in OOS terms. This does not occur with VXX, that has never given high realized mean performances: paradoxically, worse in-sample properties would help a mean-variance investor in a OOS perspective. Finally, panel C of Figure 6 gives a picture that is unsurprisingly favorable to a modest contribution to economic value by VIX paired with a zero or modestly negative differential between VXX and VIX.
6.3. The effects of transaction costs
So far, the OOS picture turns out to be generally but mildly favorable to considering volatility—as proxied by VIX—a novel asset class, but starkly against (with the minor exception of a few specific mean-variance configurations) the possibility that VXX may represent a viable tool to harvest the risk-adjusted gains deriving from volatility trading in asset management. There is however one logical possibility that needs to explored: VXX may turn out to be competitive and hence a useful tool to implement volatility trading in the case in which VXX implies lower trading volume and hence lower transaction costs than VIX does. This is in line with the evidence in Table 5.1. In principle, it is even possible that VXX may yield negative mean and median return asset and yet allow an investor to hedge herself so efficiently that the transaction cost savings end up more than compensating the "damage" to performance deriving from a negative mean.
Because a fair comparison based on transaction costs requires that VIX and VXX be demanded in similar proportions, in this Section we focus on the case of optimal allocations obtained under power utility. The limitation of transaction-cost based analyses is that we are compelled to introduce assumption on the size and "structure" of the trading costs. Define CPt as the proportional component at time t and CF the fixed component, both expressed in basis points. As usual, CPt is computed as a proportion of the sum of portfolio weight changes across all asset classes between t and t + 1:
|
CPt+1=αN∑i=1|wi,t+1−wi,t|
|
(18)
|
Therefore the net-of-trading costs performance can be computed as [1−(CPt+1+CF)] multiplied by the gross-of-trading costs portfolio return, RPt+1. In particular, we assume α=9 bps and CF=5 bps. We have also experimented with alternative selections in a neighborhood of these values (i.e., 7-8 or 10-11 bps for proportional costs and 3 and 7 bps for fixed costs) finding qualitatively similar results.
Table 7 reports the key findings on average realized OOS excess returns. For added visibility, we have boldfaced all positive values. Interestingly, for the least risk-averse investors who trade the most, transaction costs lead to negative realized mean returns already in the benchmark case. As it sometimes happens in back-testing experiments, trading a lot ends up hurting. More risk averse investors (L =7 and 10) achieve mean realized excess returns that tend to be small (1-3 bps per week, at most 1.56% per year). Things worsen drastically when volatility—an asset class that creates additional trading opportunities—is added. Interestingly, VXX leads to higher mean returns than VIX does in the case of ∧ equal to or in excess of 4, but once transaction costs are modeled, such a differential in performance fades.
Table 7. Effects of including transaction costs: power utility case.
| γ |
2 |
4 |
7 |
10 |
| Panel A -Baseline |
|
|
|
|
| No transaction cost |
0.183% |
0.164% |
0.191% |
0.177% |
| Including transaction cost |
-0.043% |
-0.035% |
0.001% |
0.003% |
| Panel B – Portfolio with VIX |
|
|
|
|
| No transaction cost |
0.109% |
-0.093% |
-0.238% |
-0.078% |
| Including transaction cost |
-0.369% |
-0.526% |
-0.677% |
-0.479% |
| Panel C – Portfolio with VXX |
|
|
|
|
| No transaction cost |
-0.053% |
0.030% |
0.029% |
0.033% |
| Including transaction cost |
-0.466% |
-0.369% |
-0.365% |
-0.344% |
| Note: The table shows the mean excess returns for portfolio under power utility function for different alternative choices of ∧ and compares then the cases of no transaction costs vs. the inclusion of transaction costs. In the table, we have boldfaced positive values for additional clarity. |
7. Discussion: Poor ETPs vs. Poor Portfolio Strategies
What is the economics of the failure of volatility investment, as a part of an optimizing, risk-averse portfolio strategy, to deliver the benefits it is alleged to yield when one considers the underlying VIX index? The facts are in plain sight: its practical implementation, as achieved through the most popular (at least in the sense of having being traded for the longest time) ETNs written on the VIX leads to disappointing risk-adjusted results, with the exceptions of a handful of special cases. One therefore wonders whether the existence of such a differential is a reflection of the ineffectiveness of the ETN (or of its expensiveness due to massive losses that ETNs incur to roll over short-term futures on the VIX, as they expire, see the evidence in Alexander, Kapraun, and Korovilas, 2015) or of the fact that treating volatility as an asset class in long-only portfolios may be practically questionable. To further investigate this issue, we proceed to perform afresh a portion of the previous tests when an investor is assumed to be capable to trade and roll on short-term VIX futures, to try and approximate the virtual returns one would obtain going long in the VIX index directly.11
11 A referee correctly reminds us that many institutional investors are likely to or be contractually restricted from futures roll-over strategies. Comparing a strategy that rolls VIX futures and ETNs that mostly invest in futures is informative because we need to recall that because volatility indices are not tradable, there is no unique closed-form, arbitrage free, cost-of-carry relationship connecting them with the price of futures contracts. As a result, a sizeable difference between the index and futures prices may appear. Yet, the futures price represents the risk-neutral expectation of the corresponding volatility index at maturity, and as such, these futures offer a volatility exposure that should be still highly correlated with the volatility index.
To this end, we use weekly returns computed from the continuous series of the closest-to-expiry VIX from CBOE. In principle, such a strategy is what a long-VIX ought to implement, but here we abstract from transaction costs and margination constraints that are instead important and do worsen the realized performance of ETNs in reality. The sample period is identical to the one used above. Such a futures strategy implies a mean return of 0.16% per week but a median of -0.16% per week and therefore a high and positive skewness (0.99). Interestingly, these properties are similar, but less extreme, vs. what we have reported for the VIX. However, the futures roll over strategy (henceforth, FROS) implies slightly negative excess kurtosis. Such differences are emphasized by a pairwise correlation of 0.84 with VIX. Interestingly though, the correlation between FROS and VXX is slightly larger, 0.86. FROS has also correlations with SPY, IYR, and AGG similar to what we have reported above.
Figure 4, displays optimal recursive portfolio weights between February 2010 and February 2016, computed on an expanding window of data, at a monthly frequency. The plot needs to be compared to Figure 3 for each of the assumed levels of the CRRA coefficient L. Interestingly, all power utility investors place a higher but more stable fraction of their wealth in the FROS (on average 20-25%) vs. both VIX and VXX. This is not surprising because—even though FROS has similar correlations and skewness when compared to VIX and VXX—its mean and variance are different and more favorable. Correspondingly, when investors can go long in FROS, less bonds need to be used to moderate risk exposure, especially in the second half of the example. However, the real, deep difference between Figures 4 and 3 is that in the former a much higher weight is invested on average in equities, that seem to benefit much more from the hedge provided by FROS than by VIX or VXX. All in all, such differences are not major and yet they are important enough to motivate us to compute and report realized OOS risk-adjusted performances compared to VIX and VXX long-only portfolios.
The deficiencies and limitations of ETNs fully emerge comparing the last two columns of Table 8, concerning the OOS Sharpe ratios of VXX vs. FROS: for all Ls but one, the increase in Sharpe ratios is stunning. Aggressive investors benefit from FROS because it provides hedging without inflicting the mean losses of VXX, presumably due to the fees charged on the asset under management and roll costs (see Dash and Liu, 2012); very risk-averse investors benefit from FROS because it is a better hedge than VXX is. However, FROS is generally inferior to VIX itself. For instance, for ∧ =7, FROS leads to a realized Sharpe ratio of 0.015, similar to the benchmark, but inferior to the VIX Sharpe ratio of 0.028. Table 8 leads us to conclude that even though it is not easy for practical long-only volatility strategies to outperform VIX, a large portion of the poor performance of VXX derived from its institutional features and the costs that the ETN structure implies. In fact, we emphasize that the FROS is implemented here disregarding the related costs, while our data on ETN returns do discount such costs.12 Table 9 performs a similar set of calculations in terms of differences in CERs deriving from switching among alternative ways to invest in volatility. Clearly, trading VIX represents the first best in risk-adjusted terms and a FROS cannot represent a threat. However, FROS also turns out to be better than VXX, at least for ∧ =4 and higher. In our view, this represents an important indication that while treating VIX as a separate asset class may be sensible as represented in earlier literature (see Section 2), in practice this may be subject to important limitations deriving from the non-tradability of the VIX.
Table 8. Realized out-of-sample sharpe ratios from an asset allocation based on rolling VIX futures over in Time.
|
Baseline |
Portfolio with VIX |
Portfolio with VXX |
Portfolio based on Futures |
| 5 yrs RW |
γ = 2 |
0.1308 |
0.0537 |
0.0256 |
0.1149 |
| γ = 4 |
0.0154 |
0.0614 |
0.0056 |
0.0106 |
| γ = 7 |
0.0154 |
0.0278 |
0.0197 |
0.0151 |
| γ = 10 |
0.0077 |
0.0454 |
0.0058 |
0.0736 |
| Note: This table reports the ex-post, realized Sharpe ratios under power utility function and for alternative asset menus. Each panel shows results for different assumptions on the risk aversion coefficients. |
Table 9. Annualized Certainty Equivalent Return Loss from an Asset Allocation Based on Rolling VIX Futures Over in Time.
| γ |
2 |
4 |
7 |
10 |
| Portfolio with VIX vs Portfolio with VXX |
|
|
|
|
| Mean |
0.002% |
-0.009% |
0.026% |
0.056% |
| Std. Dev. of Mean |
0.074% |
1.166% |
1.660% |
10.207% |
| Portfolio with VXX vs Portfolio with Future-Based Strategies |
|
|
|
|
| Mean |
0.010% |
-0.112% |
-0.065% |
-0.189% |
| Std. Dev. of Mean |
0.010% |
0.016% |
0.041% |
0.038% |
| Portfolio with VIX vs Portfolio with Future-Based Strategies |
|
|
|
|
| Mean |
0.012% |
0.116% |
0.091% |
0.256% |
| Std. Dev. of Mean |
0.003% |
0.016% |
0.023% |
0.069% |
| Note: The certainty equivalent return (CER) loss is calculated as a difference between the certainty-equivalent returns obtained from two alternative portfolio strategies and can be interpreted as the maximum, yearly percentage fee that an investor should be ready to pay in order to switch between two alternative portfolio strategies. |
12 Dash and Moran (2007) report that the performance of a volatility strategy based on investing in futures contracts is lower by about 40% vs. the performance recorded by the VIX index. This derives from the cost of rolling -over the various contracts, i.e. both by the "bleeding effect" due to the typical slope of the term structure of VIX futures and by the costs of trading the positions. Also note that futures imply no credit risk because settlement is generally guaranteed by a clearing house, while ETPs (ETNs) generally imply substantial credit risk, as documented by Alexander et al. (2015) for the VXX note.
8. Conclusion
This paper aims at assessing the role of Exchange Traded Products in (long-only) portfolio strategies, focusing on volatility investing. In this sense, it differs from the previous literature that has assessed the role played by volatility as an asset class through the use of derivative instruments and futures contracts to hedge existing portfolio positions; in our analysis, instead we focus on the use of a ETN whose underlying is the VIX index, a weighted average of the implied volatility on the S & P 500. The exercise consists of the recursive calculation of optimal portfolio weights assigned to stocks, real estate, bonds, and volatility, when short positions are (realistically) ruled out, to understand how optimal asset allocation—with special emphasis on volatility trading—may vary as a function of alternative preference assumptions typical of the applied finance literature, i.e., mean-variance, power, and negative exponential utility functions.
The recursive estimation of optimal weights and the back-testing of their performance over the period January 2009–February 2016 shows that the VIX index generally belongs to the optimal allocation of investors for most preferences and risk aversion coefficients considered, leading to the—by now routinely encountered—claim that volatility is indeed an important asset class. However, such a conclusion is weakened and survives only for special preferences and assumptions when volatility is represented by one of the most popular ETNs written on volatility (iPath S & P 500 VIX Short-Term Futures, with VXX ticker). The weight of the ETN tends to be smaller than the one of VIX and appears to be substantial only under power utility.
Next, such weights are used to recursively construct portfolios that are then assessed on a OOS basis to measure their realized, risk-adjusted performance using a range of indicators, from classical Sharpe and Sortino ratios to theoretically appealing CERs. We find that while VIX investments tend to create important and often precisely estimated risk-adjusted economic value, the same does not apply—at least not generally—when volatility is traded using the ETN. In fact, we find a fraction of experiments in which trading VXX leads to a net reduction of risk-adjusted performance. This is also due to the large and negative mean returns of the ETN investigated in our paper. Interestingly these conclusions are qualitatively similar—although volatility trading tends to generate stronger value—when ETN is replaced by a strategy that trades the closest-to-maturity VIX futures, even though in this case we have ignored transaction costs and all frictions an investor would come to face in reality. The implication is that although ETNs may successfully track volatility indices in terms of their correlations with them, this is just a necessary condition for a successful ETP to support portfolio decision; other statistical features—such as the mean and median—may render the passive index-tracking portfolio sufficiently "costly" in terms of risk-adjusted performance, that the high correlation condition fails to be sufficient. In fact, a recent applied portfolio management literature has shown that simpler target volatility strategies that perform systematic rebalancing between a risky asset and cash with dynamic weights so that ex-ante risk is kept constant, may deliver high (er) risk-return trade-offs with lower costs (see, e.g., Füss et al., 2014).
Importantly, such empirical findings in no way represent evidence that ETPs are not useful: they may remain valuable tools to implement market timing strategies that exploit the predicted power of volatility for subsequent excess stock returns and to hedge left-tail risk.13 In fact, it would be interesting to pursue dynamic extensions (as in Adams et al., 2017 or Carroll et al., 2017 for recent, critical perspectives) to investigate how volatility and covariance timing may be improved by the availability of ETPs that allow to trade volatility directly. Our goal in this paper was simply to show that even in the starkest, simplest setting, VXX cannot even remotely deliver the average realized economic gains that in principle VIX can create. Yet, it is clear that the standard utility models we have assumed and the simple static framework in which investment opportunities are constant cannot do justice to these two additional ways in which volatility ETPs may create value, even though the related research questions are of great importance.
13 For instance, Rakowski, Shirley and Stark (2017) have recently shown that in general US mutual funds select ETN investments that underperform but that a number of these ETNs offer return patterns that fund managers value in specific situations (e.g., to implement short sales that are otherwise difficult or costly) related to tail risk reduction, to bypass constraints on holding derivatives, and ensuring yield flows.
Of course, different results might have been also obtained by the use of different and better optimized ETNs to track the dynamics of VIX, although such alternative ETPs did tend to report shorter time series. Alexander and Korovilas (2013) have studied the portfolio benefits that may be obtained by combining several ETNs that track the VIX. Finally, it would be interesting—even though it is true that many institutional investors that are restricted from (or strongly advised against) shorting volatility (through options and futures, with all the rolling issues and costs that this implies)—to explore the OOS economic value to portfolio decisions of ETPs that allow investors to short asset classes, including volatility, with or without market timing opportunities. Preliminary evidence indicates that, because the mean excess returns on traditional asset classes turns negative and their correlation structure increases particularly during periods of high volatility, in large portions of our sample, volatility should have been shorted. We leave these extensions to future research.
Acknowledgements
We would like to thank Gianluca Giudice for his help and Francesco Ianniello for his support. The editors and two anonymous referees provided insightful comments that have allowed us to improve the paper.
Conflict of Interest
All authors declare no conflicts of interest in this paper.









 DownLoad:
DownLoad: