Existence and global behavior of the solution to a parabolic equation with nonlocal diffusion

1.   Introduction

In this paper, we consider the following parabolic problem with nonlocal nonlinearity:

where $\Omega\subseteq R^N$ ($N\geq1$) is a sufficiently regular domain, $\gamma\in[1, +\infty)$, $0 < T\leq +\infty$, $a\in C^1([0, +\infty), [0, +\infty))$, $u_0\in C^{2+\alpha}(\overline{\Omega})$, $f\in C^1(R, R)$.

This type of problem was studied initially by Chipot and Lovat in ^[8], where they proposed the equation

for modelling the density of a population, for example, of bacteria, subject to spreading and presented the existence and uniqueness of a weak solution to this equation. Another interesting result was obtained in ^[3] where Almeida, Antontsev and Duque considered the following problem

and proved the existence, uniqueness and asymptotic behaviour of the weak solutions. Note that $f(x, t)$ is independent on $u$ in problem (1.2) and if $a(t) = t^{\gamma}$, problem (1.1) can be changed into problem (1.3). Therefore, problem (1.1) is a generalization of problem (1.2) and (1.3).

For $f$ depending on the state $u$ such as $f(u) = ru(k-u)$ or $f(u) = ru/(k+u)$, Ackleh and Ke ^[1] studied the problem

proved the existence-uniqueness of a solution to this problem and gave some conditions for the extinction in finite time and the persistence respectively. If the coefficient $1/a$ is an unbounded function around the origin (e.g., $a(u(\cdot, t)) = \int_{\Omega}u(x, t)dx$), then a diffusion of this type could model a population that is anxious to move quickly out of zones experiencing a sharp decrease in population densities. For example, consider a population attempting to leave a spatial region due to a sudden dangerous situation. The individuals in the population move randomly (due to lack of information) in an attempt to leave the area. In this case, diffusion out of the region will increase as population decreases due to a decrease in the interaction between individuals that hinders their movement out. One can imagine such an occurrence related to an epidemic. The asymptotic behaviour of the solutions as time tends to infinity was studied for nonlinear parabolic equations with two classes of nonlocal terms or a non-autonomous sublinear terms also (see ^[6,30]).

We point out that there is only one unknown function in (1.1). In fact, there are many types of species in some areas and then it is interesting to discuss nonlocal coupled systems. For examples, in ^[4], Duque et al. presented some results on the existence, uniqueness of weak and strong global in time solutions, polynomial and exponential decay and vanishing of the solutions in finite time. In ^[19], Raposo et al.· discussed the existence, uniqueness and asymptotic behavior of the solutions for a nonlinear coupled system.

In this work, we present some conditions for reaction term $f$ and diffusion coefficient $a$ different from most previous papers: (1) reaction term $f$ may grow superlinearly or exponentially at $+\infty$ or be lack of local Lipschiz continuity at $0$; (2) diffusion coefficient $a$ is unbounded and even grows exponentially at $+\infty$. This work is concerned with the proof of the existence, uniqueness and asymptotic behavior of the solutions and extend some results in previous literature (see ^[1,3,6,8,30]).

The paper is organized as follows. In Section 2, according to the proof in ^[1], we consider a generalized problem (2.1) of (1.1), transform (2.1) into (2.2) and show that the existence of solution to (2.1) is equivalent to the existence of solution to (2.2). In Section 3, we define the sub-supersolution pair for (3.1) which generalizes (2.2) and present the existence of solutions between the subsolution and supersolution. Section 4 is devoted to the proof of the existence, uniqueness and long-time behavior of solutions to (1.1) by using the method of sub-supersolution in Section 3. In Section 5, motivated by the idea in ^[1], we develop a finite difference scheme to approximate the solution of some reaction-diffusion equations. This scheme is then used to numerically study the long time behavior of the some models. Some ideas in our paper come from ^{[5,7,9,11,12,13,14,15,16,20,21,23,24,25,26,27,28,29]} also.

2.   Equivalence of some generalized system to (1.1)

Now we generalize (1.1) to the following problem

where $\Omega\subseteq R^N$ is a bounded domain with $\partial\Omega\in C^{2+\alpha}$, $u_0\in C^{2+\alpha}(\overline{\Omega})$, $\alpha\in(0, 1)$, $T\leq +\infty$, $\gamma\in[1, +\infty)$.

In order to consider (2.1), we consider the following problem

where $a$ satisfies

Theorem 2.1 Suppose that $u_0\in C^{2+\alpha}(\overline{\Omega})$, with $u_0\not = 0$ and (2.3) holds. Then (2.1) has a solution $u(x, t)$ on $[0, \overline{T}_{\max})$ if and only if that (2.2) has a solution $v(x, t)$ on $[0, T_{\max})$, where the relation between $\overline{T}_{\max}$ and ${T}_{\max}$ is

Proof. Sufficiency. Suppose that $v$ is a solution of (2.2). Let $\tau(t)$ be a solution to the following ordinary differential equation

Separating variables and integrating in $t$ we get the following equation:

Set

It can be easily shown that $G$ is a $C^1$ diffeomorphism from $[0, T_{\max})$ onto $[0, \overline{T}_{\max})$ and (2.3) implies that (2.4) has a unique solution given by $\tau(t) = G^{-1}(t)$ on $[0, \overline{T}_{\max})$.

Now let

Then clearly $u$ satisfies the following: $u(x, 0) = v(x, \tau(0)) = u_0(x)$ and $u(x, t)$ is continuous for $t\geq 0$, continuously differentiable for $t > 0$. Furthermore, we have that

Hence, $u$ is a local solution of Eq (2.1).

Necessity. Let $u(x, t)$ be a solution to (2.1) and let $G$ be the solution to the differential equation

Separating variables and integrating in $t$ we get the following equation:

Set

It can be easily shown that $\tau$ is a $C^1$ diffeomorphism from $[0, \overline{T}_{\max})$ onto $[0, T_{\max})$ and (2.3) implies that (2.5) has a unique solution given by $G(t) = \tau^{-1}(t)$ on $[0, T_{\max})$.

Set

We have that $v(x, 0) = u(x, 0) = u_0(x)$, $v(x, t)$ is continuous for $t\geq 0$, continuously differentiable for $t > 0$. Moreover,

The proof is complete.

Remark 2.1 Under the uniform Lipschitz continuity requirement on the functions $F$, condition (2.2) on $a$ and $\gamma\geq 1$, Eq (2.2) has a unique mild solution (see ^[17,22]).

Remark 2.2 The idea of our theorem comes from Theorem 2.1 in ^[1]. But in our proof, it is not necessary that $F$ satisfies uniform Lipschitz continuity condition.

3.   Sub-supersolution method

Let $k\geq 0$ be an integer, $C^{k}(\overline{\Omega}) = \{u:\overline{\Omega}\to\mathbb{R}|D^lu\in C(\overline{\Omega}), \; \forall\; |l|\leq k\}$ with the norm

$C^{k+\alpha}(\overline{\Omega}) = \{u:\overline{\Omega}\to\mathbb{R}|u\in C^{(k)}(\overline{\Omega}), H_{\alpha}(D^su) < +\infty \; \forall\; |s| = k\}$ with the norm

where

$C(\overline{Q}_T) = \{u:\overline{Q}_T\to\mathbb{R}|u$ is continuous on $\overline{Q}_T\}$ with the norm

$C^{\alpha, \frac{\alpha}{2}}(\overline{Q}_T) = \{u:\overline{Q}_T\to\mathbb{R}|u\in C(\overline{Q}_T), H_{\alpha, \frac{\alpha}{2}}(u) < +\infty\}$ with the norm

and $C^{2k+\alpha, k+\frac{\alpha}{2}}(\overline{Q}_T) = \{u:\overline{Q}_T\to\mathbb{R}|u\in C^{2k, k}(\overline{Q}_T), H_{\alpha, \frac{\alpha}{2}}(D^r_tD^s_xu) < +\infty \ \ \forall 2r+|s| = 2k\}$ with the norm

where

For $p > 1$, let $L_p(\Omega) = \{u:\Omega\to\mathbb{R}|u$ is measurable on $\Omega$ and $\int_{Q}|u|^pdx < +\infty\}$ with norm

$W_p^k(\Omega) = \{u:\Omega\to\mathbb{R}|u\in L_p(\Omega), D^lu\in L_p(\Omega), |l|\leq k\}$ with the norm

Let $Q_T = \Omega\times(0, T]$ and $L_p(Q_T) = \{u:Q\to\mathbb{R}|u$ is measurable on $Q_T$ and $\int_{Q_T}|u|^pdxdt < +\infty\}$ with the norm

$W_p^{2k, k}(Q_T) = \{u:Q_T\to\mathbb{R}|u\in L_p(Q_T), D^s_tD^l_xu\in L_p(Q_T), 2s+|l|\leq 2k\}$ with the norm

In this section, we generalize (2.2) to the following problem:

where $\Omega\subseteq R^N$ is a bounded domain with $\partial\Omega\in C^{2+\alpha}$, $u_0\in C^{2+\alpha}(\overline{\Omega})$, $\alpha\in(0, 1)$, $T < +\infty$, $Q_T = \Omega\times(0, T]$, $\gamma\in(0, +\infty)$ and $a:[0, +\infty)\to(0, +\infty)$ is continuous with

Definition 3.1 The pair functions $\alpha$ and $\beta$ with $\alpha$, $\beta\in C(\overline{Q}_T)\cap C^2(Q_T)$ are subsolution and supersolution of (3.1) if $\alpha(x, t)\leq \beta(x, t)$ for $(x, t)\in \overline{Q}_T$ and

and

where $a_0 = a(0)$ and $b_0(t) = \sup\limits_{s\in[0, \int_{\Omega}\max\{|\alpha(x, t)|, |\beta(x, t)|\}^{\gamma}dx]}a(s)$.

For fixed $\lambda > 0$, we list the following problem

where $\Omega\subseteq R^N$ is a smooth bounded domain and give the deformation of Agmon-Douglas-Nirenberg theorem for (3.3).

Lemma 3.1 (see ^[2], Agmon-Douglas-Nirenberg) If $p > 1$ and $h\in L^{p}(Q_T)$, $\phi\in W^2_p(\Omega)$, then (3.3) has a unique solution $u\in W^{2, 1}_{p}(Q_T)$ such that

where $C_1$, $C_2$ are independent from $u$, $h$.

We define the unique solution $u = ({ }\frac{\partial}{\partial t}-\Delta+\lambda)^{-1}h$ of (3.3) and obviously $({ }\frac{\partial}{\partial t}-\Delta+\lambda)^{-1}$ is a linear operator. In order to prove our theorem, we list the Embedding theorem.

Lemma 3.2 (See ^[10,18])Suppose $Q_T\subseteq R^{N+1}$ is a bounded domain with smooth boundary and $2p > N+2$. Then there exists a $C(N+2, p, Q_T) > 0$ such that

where $0 < \alpha < 1-\frac{N+2}{2p}$.

Then we have the following main theorem.

Theorem 3.1 Suppose that $F:\overline{Q}_T\times R\to R$ is a continuous function. Assume $\alpha$ and $\beta$ are the subsolution and supersolution of (3.1) respectively. Then problem (3.1) has at least one solution $u\in C^2(Q_T)\cap C(\overline{Q}_T)$ such that, for all $(x, t)\in\overline{Q}_T$,

Proof. For $u\in C(\overline{Q}_T)$, define

We will study the modified problem ($\lambda > 0$)

Step 1. Every solution $u(x, t)$ of (3.4) satisfies $\alpha(x, t)\leq u(x, t)\leq\beta(x, t)$, $x\in\overline{Q}_T$.

We prove that $\alpha(x, t)\leq u(x, t)$ on $\overline{Q}_T$. Obviously, $|\chi(x, t, u(x, t))|\leq\max\{|\alpha(x, t)|, |\beta(x, t)|\}$, which implies that

By contradiction, assume that $\alpha(x_0, t_0)-u(x_0, t_0) = \max_{x\in\bar{Q}_T}(\alpha(x, t)-u(x, t)) = M > 0$. Note that: $\alpha(x, 0)-u(x, 0)\leq 0$ on $\Omega$, $\alpha(x, t)-u(x, t)\leq 0$, $(x, t)\in \partial\overline{\Omega}\times(0, T]$, there are two cases: (1) $(x_0, t_0)\in \Omega\times (0, T)$; (2) $x_0\in\Omega$, $t_0 = T$.

For the case (1), we have $0\leq -\Delta(\alpha(x_0, t_0)-u(x_0, t_0))$ and $\frac{\partial(\alpha(x_0, t)-u(x_0, t))}{\partial t}|_{t = t_0} = 0$, which together with the definition of subsolution $\alpha(x, t)$ implies

This is a contradiction.

For the case (2), we have $0\leq -\Delta(\alpha(x_0, t_0)-u(x_0, t_0))$ and $\frac{\partial(\alpha(x_0, t)-u(x_0, t))}{\partial t}|_{t = t_0}\geq0$, which together with the definition of subsolution $\alpha(x, t)$ implies that

This is a contradiction also.

Consequently, $\alpha(x, t)\leq u(x, t)$ for $(t, x)\in \overline{Q}_T$.

A similar argument shows that $\beta(x, t)\geq u(x, t)$ for $(t, x)\in \overline{Q}_T$ and we omit the proof.

Hence,

Step 2. Every solution of (3.4) is a solution of (3.1). From step 1, every solution of (3.4) is such that: $\alpha(x, t)\leq u(x, t)\leq \beta(x, t)$, which implies that

and $u(x, t)$ is a solution of (3.1).

Step 3. The problem (3.4) has at least one solution.

Choose $2p > N+2$ and $0 < \alpha < 1-\frac{N+2}{2p}$ and define an operator

Since $F$ is continuous, $\overline{N}: C(\overline{Q}_T)\to C(\overline{Q}_T)$ is well defined, continuous and maps bounded sets to bounded sets. Since (3.2) is true, $a$ is continuous and ${ }\frac{1}{a(\int_{\Omega}|\chi(x, t, u(x, t))| ^{\gamma}dx)}\leq\frac{1}{a_0}$, the operator $\overline{N}_1u = \frac{1}{a(\int_{\Omega}|\chi(x, t, u(x, t))|^{\gamma}dx)}\overline{N}u$ is continuous, and maps bounded sets of $C(\overline{Q}_T)$ into bounded sets of $C(\overline{Q}_T)\subseteq L_p(\overline{Q}_T)$.

Now, for $u\in C(\overline{Q}_T)$, we define an operator $\overline{A}: C(\overline{Q}_T)\to C(\overline{Q}_T)$ by

Now we show that $\overline{A}: C(\overline{Q}_T)\to C(\overline{Q}_T)$ is completely continuous.

(1) By the construction of $\chi$, we have, for every $u\in C(\overline{Q}_T)$,

which guarantees that there exists a $K > 0$ big enough such that $N_1u+\lambda\chi(\cdot, \cdot, u)\in B_{L^p}(0, K)$ for all $u\in C(\overline{Q}_T)$, where

By Lemma 3.1, we have

which implies that $\overline{A}(C(\overline{Q}_T))$ is bounded in $W^{2, 1}_p(Q_T)$. Now Lemma 3.2 guarantees that $\overline{A}(C(\overline{Q}_T))$ is bounded in $C^{\alpha, \frac{\alpha}{2}}(\overline{Q}_T)$. Therefore, $\overline{A}(C(\overline{Q}_T))$ is relatively compact in $C(\overline{Q}_T)$.

(2) For $u_1$, $u_2\in C(\overline{Q}_T)$, by Lemma 3.1, one has

which together the continuity of the operator $N_1+\lambda\chi$ guarantees that $A:C(\overline{Q}_T)\to C(\overline{Q}_T)$ is continuous.

Consequently, $A:C(\overline{Q}_T)\to C(\overline{Q}_T)$ is completely continuous.

By (3.5) and Lemma 3.2, there exists a $K_1 > 0$ big enough such that

where $B_{C}(0, K_1) = \{u\in C(\overline{Q}_T)|\|u\|\leq K_1\}$, which implies that

The Schauder fixed point theorem guarantees that there exists a $u\in B_{C}(0, K_1)$ such that

i.e., $u$ is a solution of (3.4).

Consequently, the step 1 and step 2 guarantees that $u$ in the step 3 is a solution of (3.1).

The proof is complete.

Corollary 3.1 Assume that the conditions of Theorem 3.1 hold and $F$ satisfies local lipschitz condition. Then (3.1) has a unique solution $u$ with

Proof. Assume that there is another solution $u_1$ with $u_1(x, t)\not\equiv u(x, t)$. Then there is a $t_1 > 0$ with $u_1(x, t_1)\not = u(x, t_1)$. Let $t_* = \inf\{t < t_1|u_1(x, s)\not = u(x, s)$ for all $s\in [t, t_1)\}$. Since $u(x, 0) = u_1(x, 0) = u_0(x)$, we have $t_*\geq 0$, $u_1(x, t_*) = u(x, t_*)$ and $u_1(x, t)\not = u(x, t)$ for all $t \in(t_*, t_1]$. Since $\frac{1}{a(\int_{\Omega}|u(x, t)|^{\gamma}dx)}F(x, t, u)$ is locally Lipschitz continuous,

has a unique solution. This is a contradiction. The proof is complete.

4.   Results

In this section, using the method of sub-supersolution in above section, we consider the existence, uniqueness and long time behavior of the solution for (1.1).

Now we list some results for a parabolic equation (see ^[18]) which will be used later. Assume that $\overline{u}$ and $\underline{u}$ are the super-subsolutions to the following equation

If $\underline{u}(x)\leq \phi(x)\leq \overline{u}(x)$, $x\in\overline{\Omega}$, then $u_{\overline{u}}$ and $u_{\underline{u}}$ are the super-subsolutions to the following equation

If $f\in C^1(\overline{\Omega}\times R)$, then (4.1) has a unique solution $u(x, t)$ with

where $V(x, t) = u_{\underline{u}}(x, t)$ satisfies

and $U(x, t) = u_{\overline{u}}(x, t)$ satisfies

Lemma 4.1 (see, ^[27]) $V(x, t)$ is nondecreasing on $[0, +\infty)$ and $U(x, t)$ is nonincreasing on $[0, +\infty)$.

Lemma 4.2 (see, ^{[18, 27]}) Suppose $u$, $v\in C^{2, 1}(Q_T)\cap C(\overline{Q}_T)$ satisfying

If $(x, t)\in \overline{Q}_T$, $u$, $v\in [m, M]$ and $\frac{\partial F}{\partial u}\in C(\overline{\Omega}\times[m, M])$, then

Moreover, if $u(x, 0)\not\equiv v(x, 0)$, $x\in \Omega$, we have

Let $\Phi_1$ be the eigenfunction corresponding to the principle eigenvalue $\lambda_1$ of

It is found that $\lambda_1 > 0$,

According to Theorem 2.1, in order to study system (1.1), we only consider the following problem

where $a$ satisfies (2.3) and $u_0\in C^{2+\alpha}(\overline{\Omega})$ with $u_0\not = 0$.

Theorem 4.1 Suppose $f\in C^1(R, R)$ with $f(0) = f(1) = 0$ and $f(u) > 0$ for all $u\in(0, 1)$ and $f(u) < 0$ for $u > 1$. Then, for any $u_0(x)\geq\not\equiv0$ with $u_0\in C^{2+\alpha}(\overline{\Omega})$, (1.1) has a unique nonnegative solution $u\in C^2(Q_{+\infty})\cap C(\overline{Q}_{+\infty})$.

Proof. Since $f\in C^1(R, R)$, (4.4) has a unique local solution $v$.

Step 1. We show that for any $l > 0$, $z_0 > 0$, the ordinary differential equation

has a unique positive solution $z(t, z_0)$ with

In fact, since $f\in C^1$, (4.5) has a unique solution $z(t, z_0)$. Since $f(1) = 0$ and $f\in C^1$, $z(t)\equiv1$ is the unique solution of $\frac{dz}{dt} = \frac{1}{l}f(z)$ across any $(t_0, 1)$. If $1 > z_0 > 0$, since $f(u) > 0$ for all $u\in(0, 1)$ and $f(u) < 0$ for all $u > 1$, then $z(t, z_0)$ is increasing and $z(t, z_0) < 1$, which implies that there is a $1\geq c > 0$ such that

Therefore, there is a $\{t_n\}$ with ${ }\lim_{n\to+\infty}t_n = +\infty$ such that

By $z'(t_n, z_0) = f(z(t_n, z_0))$, one has

Hence, $c = 1$, i.e.

which guarantees that (4.6) is true. By a same proof, we get if $z_0\geq 1$,

also.

Step 2. We establish the sub-supersolution pair for (4.4).

Choose $M = \max\{2, { }\max\limits_{x\in\overline{\Omega}}u_0(x)\}$. (4.5) and (4.6) imply that

has a unique positive nonincreasing solution $z(t, M)$ with

Let $\beta(x, t) = z(t, M)$ and $\alpha(x, t) = 0$. Set

which together with $f(\beta(x, t)) < 0$ implies that

By (4.7)-(4.9) and the definitions of $\alpha(x, t)$ and $\beta(x, t)$, we have

(1) $\alpha(x, t) < \beta(x, t)$, $\forall (x, t)\in \Omega\times (0, +\infty)$;

(2)

and

which imply that $\alpha$ and $\beta$ are subsolution and supersolution to (4.4).

Hence, Theorem 3.1 together with $f\in C^1$ implies that (4.4) has a unique positive solution $v$ such that

Step 3. We show that (1.1) has a unique solution $u$, $(x, t)\in\overline{\Omega}\times[0, +\infty)$.

Since $0\leq v(x, t)\leq M$, one has $a_0\leq a(\int_{\Omega}|v(x, t)|^{\gamma}dx)\leq a(M^{\gamma}|\Omega|)$. Since $T_{\max} = +\infty$ in Theorem 2.1, one has

Let

and $\tau(t) = G^{-1}(t)$, $t\in[0, +\infty)$. Then $u(x, t) = v(x, \tau(t))$ is a unique nonnegative solution to (1.1) on $[0, +\infty)$.

The proof is complete.

Corollary 4.1 Suppose the conditions of Theorem 4.1 hold. If there is a $\varepsilon_0 > 0$ with $u_0(x)\geq \varepsilon_0\phi_1(x)$ for all $x\in\overline{\Omega}$ and $f'(0) > a(M^{\gamma}|\Omega|)\lambda_1$($M = \max\{1, { }\max\limits_{x\in\overline{\Omega}}u_0(x)\}$), then the unique solution $u\in C^2(Q_{+\infty})\cap C(\overline{Q}_{+\infty})$ of (1.1) satisfies

Proof. Choose a $\varepsilon: 0 < \varepsilon < \min\{1, \varepsilon_0\}$ small enough such that

which implies

Let $\alpha_1(x) = \varepsilon\phi_1(x)$. Then

Hence,

Step 1. Problem

has a unique solution $V(x, t)$ such that

Let $\alpha_1(x, t) = \alpha_1(x)$ and $\beta_1(x, t) = 1$, $\forall (x, t)\in\overline{\Omega}\times[0, +\infty)$. Then

(1) $\alpha_1(x)\leq \beta_1(x, t)$, $\forall (x, t)\in\overline{\Omega}\times[0, +\infty)$;

(2)

and

which imply that $\alpha_1$ and $\beta_1$ are subsolution and supersolution to (4.10) also. Thus, (4.10) has a unique positive solution $V(x, t)$ such that

Choose an arbitrary $x_0\in\Omega$. Then there exists a $\overline{B}(x_0, \delta) = \{x\in \Omega||x-x_0|\leq\delta\}\subseteq \Omega$ and $t_1 > 0$ such that

Set $\delta_0 = \min_{x\in\overline{B}(x_0, \delta)}V(x, t_1)$. Lemma 4.1 implies that $V(x, t)$ in nondecreasing on $[0, +\infty)$, which guarantees that that $V(x, t)\geq \delta_0$ for all $(x, t)\in\partial B(x_0, \delta)\times[t_1, +\infty)$.

Let $z_0 = \delta_0$ and $z(t, \delta_0)$ is the unique solution of the ordinary differential equation

(4.5) and (4.6) guarantee that

Let $V_1(x, t) = V(x, t+t_1)$, $t\geq 0$. We have

Lemma 4.2 implies that

which together with $V(x, t)\leq 1$ guarantees that

Step 2. The unique solution $v(x, t)$ of problem (4.4) satisfies that

Let $\alpha_2(x, t) = V(x, t)$ and $\beta_2(x, t) = \beta(x, t)$, $(x, t)\in\overline{\Omega}\times[0, +\infty)$, where $\beta(x, t)$ is the unique positive solution of (4.7). Let

Then

(1) $\alpha_2(x, t) < \beta_2(x, t)$, $\forall (x, t)\in \Omega\times (0, +\infty)$;

(2)

and

which imply that $\alpha$ and $\beta$ are subsolution and supersolution to (4.4) also. The corollary 3.1 implies that

And so

Step 3. We show that

According to the step 3 in the proof of Theorem 4.1, one knows that $\tau(t) = G^{-1}(t)$ exists on $[0, +\infty)$ and

Hence

The proof is complete.

Theorem 4.2 Suppose $f\in C^1(R, R)$ with $f(0) = 0$, $f'(0) < a_0\lambda_1$ and $f(u)\geq 0$ for $u\in[0, +\infty)$. Then, there exists a $\varepsilon > 0$ such that for all $0\leq u_0\leq \varepsilon\phi_1(x)$ with $u_0\in C^{2+\alpha}(\overline{\Omega})$, (1.1) has a unique nonnegative solution $u\in C^2(Q_{+\infty})\cap C(\overline{Q}_{+\infty})$ such that

Proof. Since $f(0) = 0$ and $f'(0) < a_0\lambda_1$, there is a $\lambda_1 > r > 0$ and $\varepsilon > 0$ such that

which guarantees that

Let $0\leq u_0(x)\leq \varepsilon \phi_1(x)$, $\alpha(x, t) = 0$, $\beta(x, t) = \varepsilon e^{-rt}\phi_1(x)$ and

Then, from (4.11), one has

(1) $\alpha(x, t) < \beta(x, t)$, $\forall (x, t)\in \Omega\times (0, +\infty)$;

(2)

and

which imply that $\alpha$ and $\beta$ are subsolution and supersolution to (4.5).

Now Theorem 3.1 guarantees that (4.5) has a unique solution $v(x, t)$ such that

It is easy to see that

Since $0\leq v(x, t)\leq \varepsilon$, one has $a_0\leq a(\int_{\Omega}|v(x, t)|^{\gamma}dx)\leq a(|\Omega|\varepsilon^{\gamma})$. Since $T_{\max} = +\infty$ in Theorem 2.1, one has

Let

and $\tau(t) = G^{-1}(t)$, $t\in[0, +\infty)$. Then $u(x, t) = v(x, \tau(t))$ is a unique nonnegative solution to (1.1) on $[0, +\infty)$ and

The proof is complete.

Next we consider another the special case of (1.1) for reaction function $f = (\lambda u^s-u^p)$, $s\in(0, 1)$ and $p > 1$. According to theorem 2.1, we only consider the following system

where $s\in(0, 1)$ and $p > 1$.

Theorem 4.3 Suppose there exists a $\varepsilon_0 > 0$ such that $u_0(x)\geq \varepsilon_0\phi_1(x)$. Then, for any $\lambda > 0$, (1.1) has at least one positive solution $u\in C^2(Q_{+\infty})\cap C(\overline{Q}_{+\infty})$.

Proof. For $\lambda > 0$, choose $M > 1+\frac{1}{\lambda}+{ }\max_{x\in\overline{\Omega}}u_0(x)$ such that

Since $s\in(0, 1)$ and $p > 1$, we choose $\varepsilon_0 > \varepsilon > 0$ small enough such that

Let $\underline u(x, t) = \varepsilon\phi_1(x)$ and $\overline u(x, t) = M$ for all $(x, t)\in\overline{\Omega}\times[0, +\infty)$. Set

which together (4.13) implies that

From (4.14), one has

(1) $\underline u(x, t) < \overline u(x, t) = M$ for all $(x, t)\in\overline{\Omega}\times[0, +\infty)$;

(2)

and

which imply that $\underline{u}(x, t)$ and $\overline{u}(x, t)$ are sub-super solutions to (4.12).

Theorem 3.1 guarantees that (4.12) has at least one positive solution $v(x, t)$ such that

Since $0\leq v(x, t)\leq M$, one has $a_0\leq a(\int_{\Omega}|v(x, t)|^{\gamma}dx)\leq a(M^{\gamma}|\Omega|)$. Since $T_{\max} = +\infty$ in Theorem 2.1, one has

Let

and $\tau(t) = G^{-1}(t)$, $t\in[0, +\infty)$. Then $u(x, t) = v(x, \tau(t))$ is a nonnegative solution to (1.1) on $[0, +\infty)$. The proof is complete.

Remark. The function $f$ in the above theorem does not satisfy $Lipschitz$ condition.

5.   Some one-dimensional numerical experiments

In this section we consider the following case of Eq (1.1):

To numerically solve this equation the following implicit backward finite difference approximation was employed:

where $\Delta t = \overline{T}_{\max}/K = 0.0002$, $\Delta x = 1/N = 0.02$, $x_j = j\Delta x$, $j = 0$, $1$, $\cdots$, $N$, and $t_i = i\Delta t$, $i = 0$, $1$, $\cdots$, $M$. In (5.1)$^\prime$, $u^i_j$ denotes the difference approximations of $u(t_i, x_j)$. Using above scheme, we simulate the solution of (5.1) under different $f$, $a$ and $\lambda$.

According to section 4, it is necessary to analyse the first eigenvalue and corresponding eigenfunction of

Obviously, $\lambda_1 = \pi^2$ and $\phi_1(x) = \sin(\pi x)$, $x\in[0, 1]$.

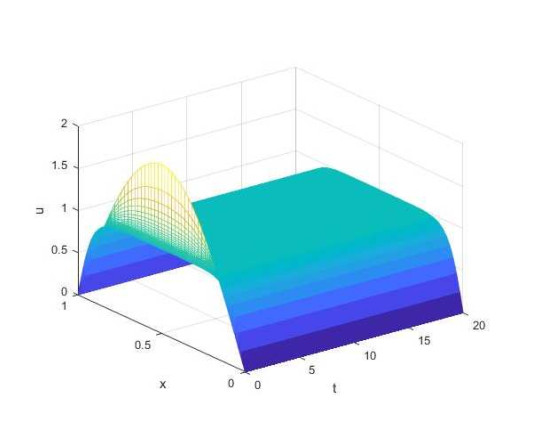
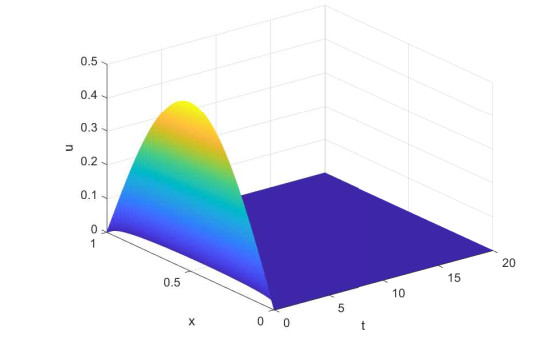
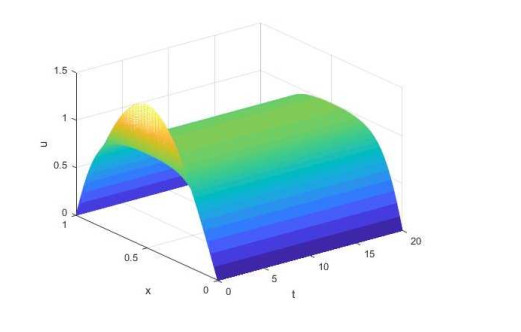
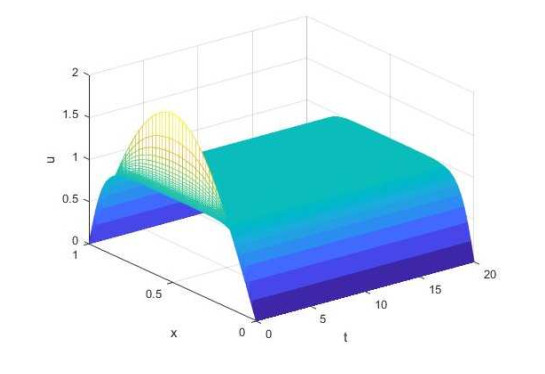
First, for Eq (5.1), let $a(t) = 1+t$, $\gamma = 1$, $\lambda = 500$, $f(u) = u-u^3$. If the initial condition is given by $u_0(x) = 2\sin(\pi x)$, which satisfies that ${ }\max_{x\in[0, 1]}u_0(x) = 2 > 1$ and $\frac{1}{a(M^{\gamma}|\Omega|)}f'(0) = \frac{500}{1+4/\pi} > \pi^2$, in our numerical simulations, we present the result in Figure 1. If $u_0(x) = 0.5\sin(\pi x)$ which satisfies that ${ }\max_{x\in[0, 1]}u_0(x) = 0.5 < 1$ and $\frac{1}{a(M^{\gamma}|\Omega|)}f'(0) = \frac{500}{1+1/\pi} > \pi^2$, in our numerical simulations, we present the result in Figure 2. The simulations indicate Corollary 4.1 is in agreement with the numerical results presented in Figure 1. Note $f$ is independent on $u$ in problem (1.2) and (1.3) and $f(u) = u-u^3$ in this example, which illustrates that our results improve these ones in ^[3,8].

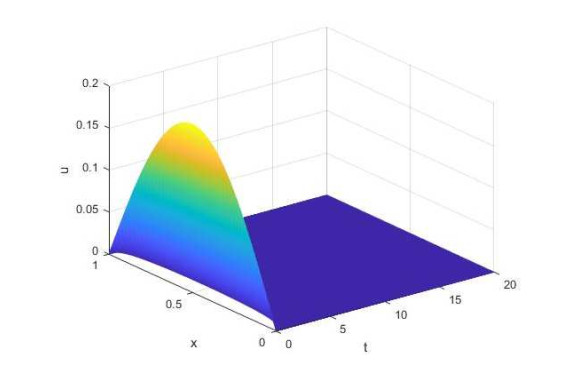
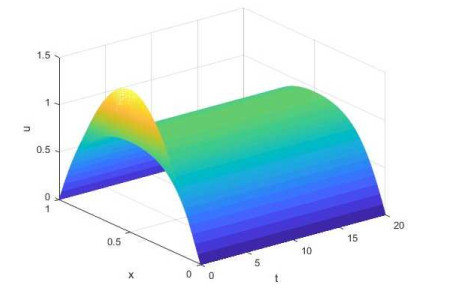
Second, for Eq (5.1), let $a(t) = 1+t$, $\gamma = 1$, $\lambda = 7$. If $f(u) = e^u-1$ and the initial condition is given by $u_0(x) = 0.5\sin(\pi x)$, which satisfies that $\frac{1}{a_0}f'(0) = 7 < \pi^2$, in our numerical simulations, we present the result in Figure 3. If $f(u) = u+u^3$ and $u_0(x) = 0.2\sin(\pi x)$ which satisfies that $\frac{1}{a_0}f'(0) = 7 < \pi^2$, in our numerical simulations, we present the result in Figure 4.

The simulations indicate Theorem 4.2 is in agreement with the numerical results presented in Figure 2. In these examples, $f(u) = e^u-1$ or $f(u) = u+u^3$, which are different from $f(u) = ru(k-u)$ or $f(u) = ru/(k+u)$ in ^[1].

Finally, for Eq (5.1), let $a(t) = 1+t$, $\gamma = 1$, $s = \frac{1}{2}$, $p = 3$, $f(u) = u^s-u^p$ and $u_0(x) = 1.5\sin(\pi x)$. If $\lambda_1 = 50$, in our numerical simulations, we present the results in Figure 5. If $\lambda_1 = 100$, in our numerical simulations, we present the results in Figure 6. The simulations indicate Theorem 4.5 is in agreement with the numerical results presented in Figure 3.

6.   Conclusions

This paper studies on a parabolic equation with nonlocal diffusion. Using the sub-supersolution method, we prove the existence, uniqueness and long-time behavior of positive solutions. Under the suitable cases for one-dimensional equations, we plotted 3D simulations of the the solutions. From these Figures 1-6, it may be observed that solutions to the studied nonlinear model show the estimated solution propagations.

Acknowledgments

We would like to thank the referees for their suggestions. This work is supported by the National Natural Science Foundation of China (62073203) and the Fund of Natural Science of Shandong Province (ZR2018MA022).

Conflict of interest

No potential conflict of interest was reported by the authors.