FEM-DBEM approach to analyse crack scenarios in a baffle cooling pipe undergoing heat flux from the plasma

R. Citarella; V. Giannella; M. A. Lepore; J. Fellinger; R. Citarella; V. Giannella; M. A. Lepore; J. Fellinger

doi:10.3934/matersci.2017.2.391

AIMS Materials Science

2017, Volume 4, Issue 2: 391-412. doi: 10.3934/matersci.2017.2.391

Previous Article Next Article

Research article

FEM-DBEM approach to analyse crack scenarios in a baffle cooling pipe undergoing heat flux from the plasma

1.
Department of Industrial Engineering, via Giovanni Paolo II, 132, University of Salerno, Fisciano (SA), Italy
2.
Max-Planck-Institut Für Plasmaphysik, Teilinstitut Greifswald, Greifswald, Germany

Received: 09 December 2016 Accepted: 12 February 2017 Published: 22 February 2017

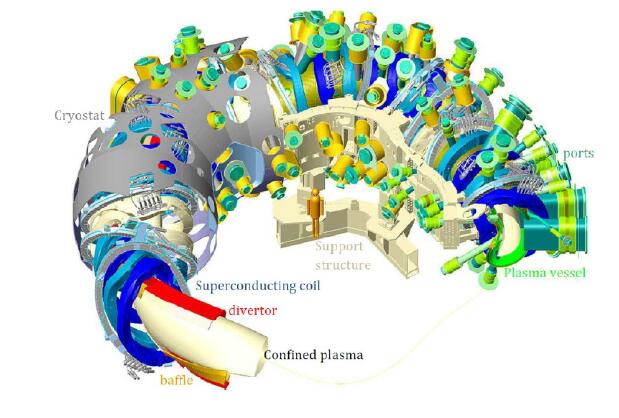
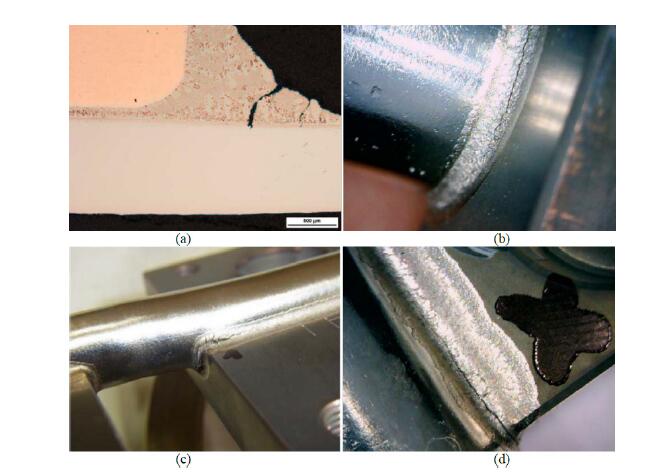
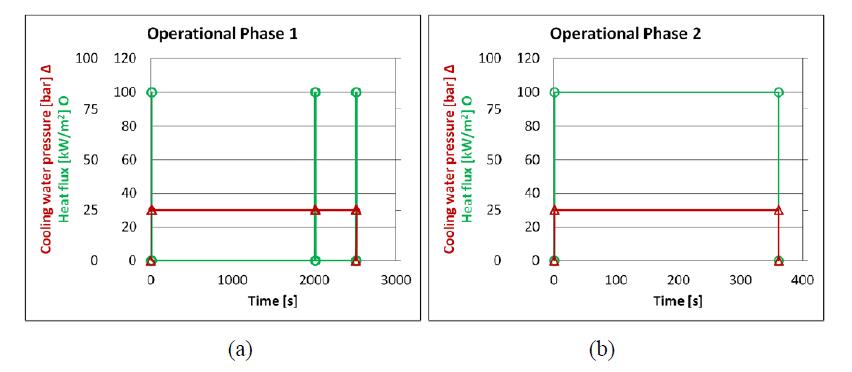
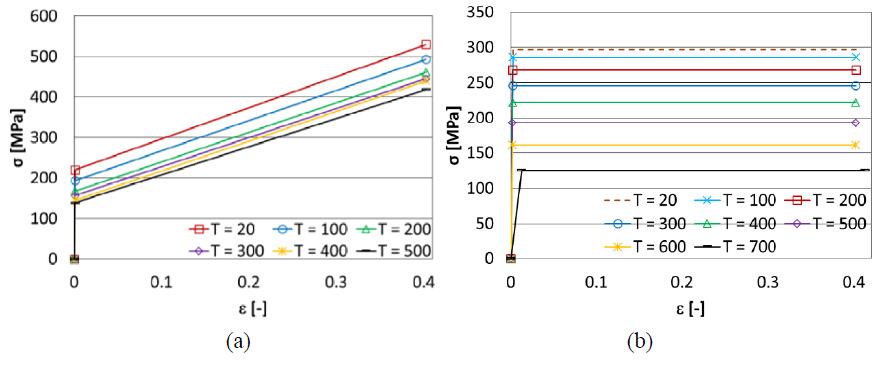
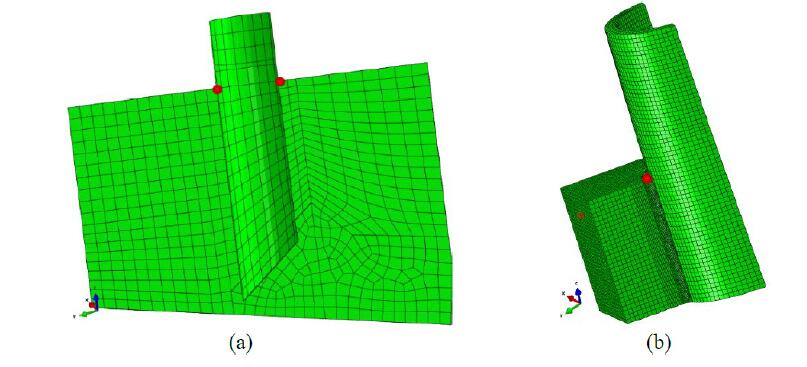
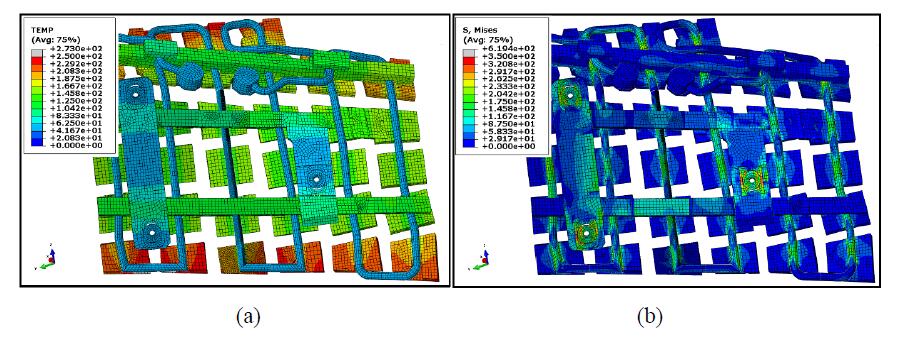
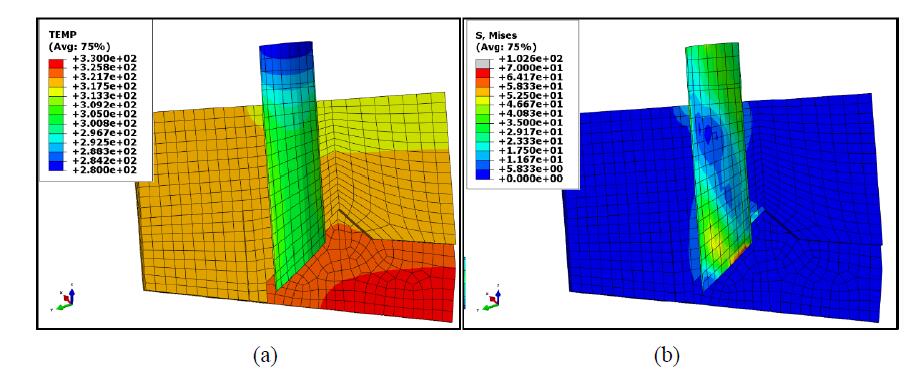
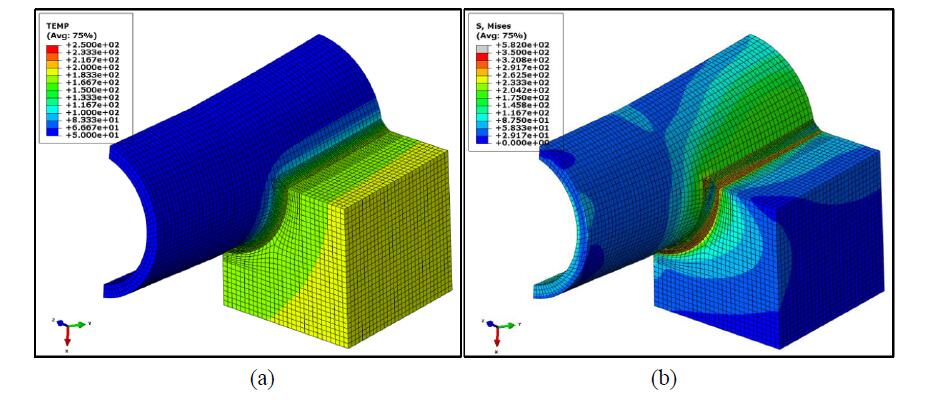
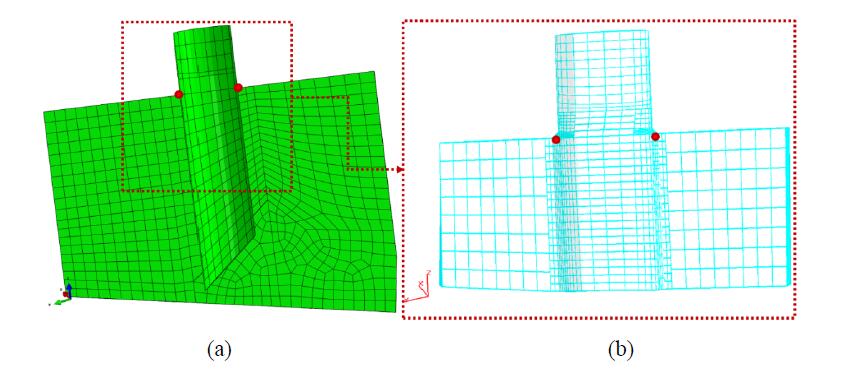
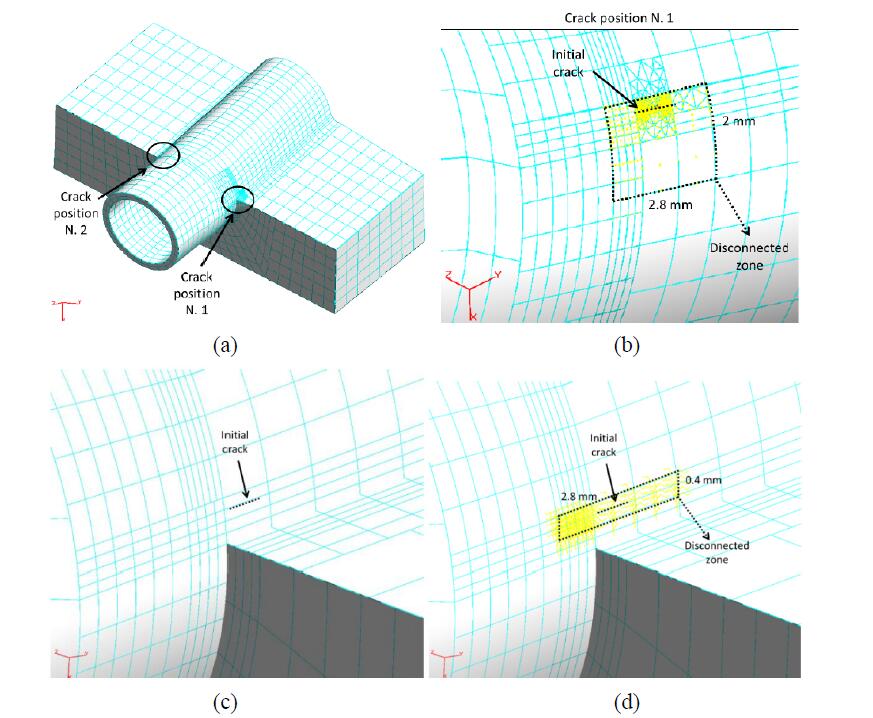
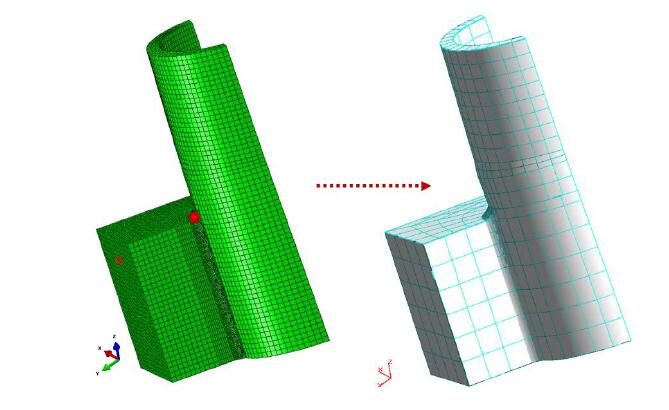
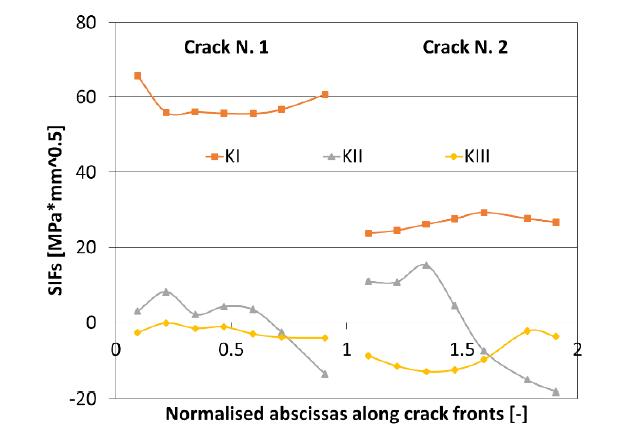
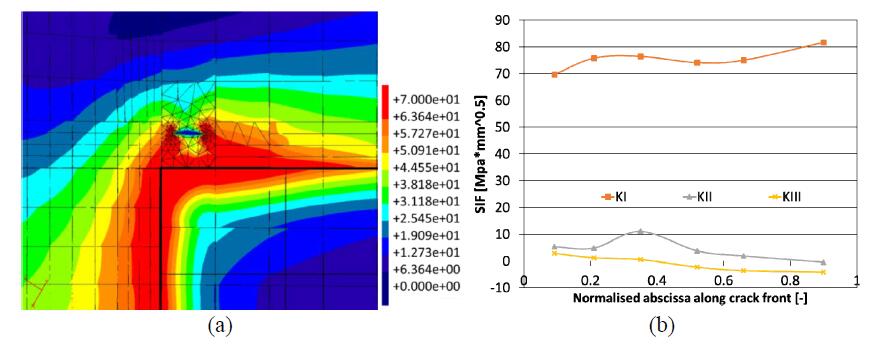
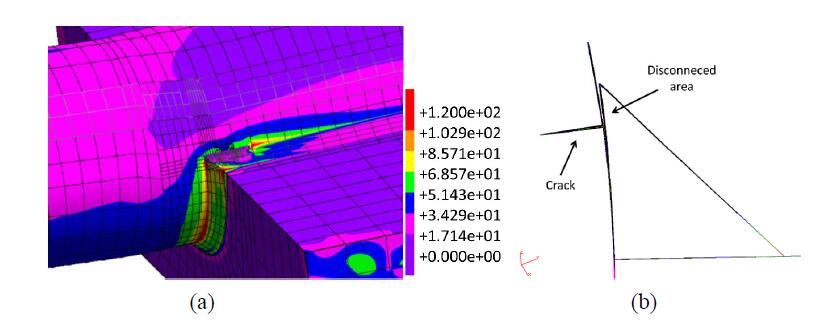
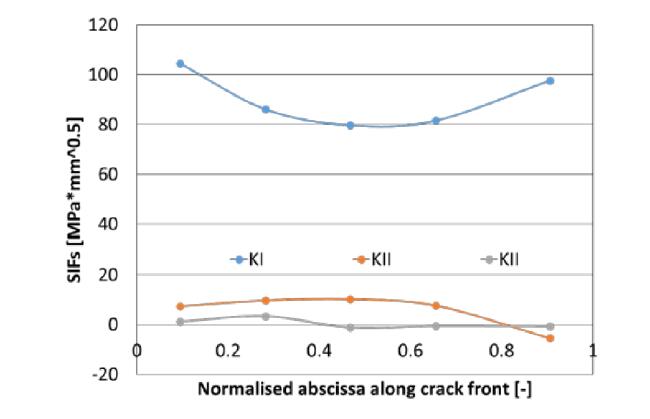
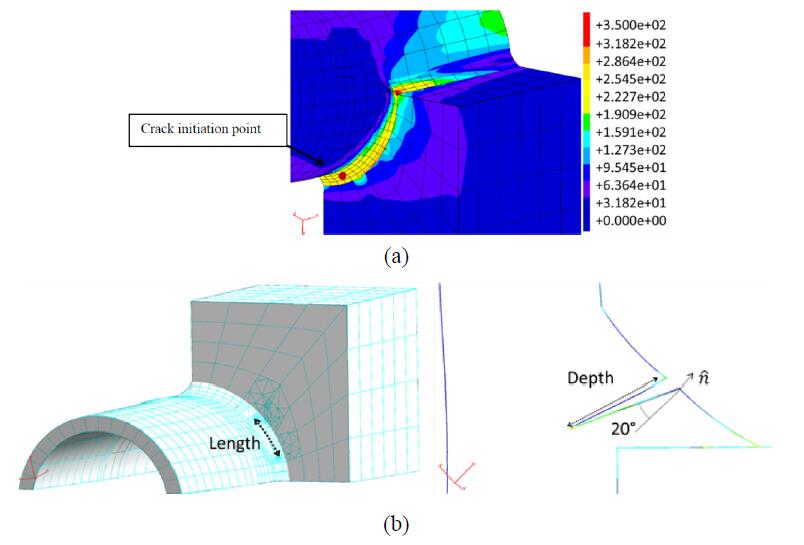
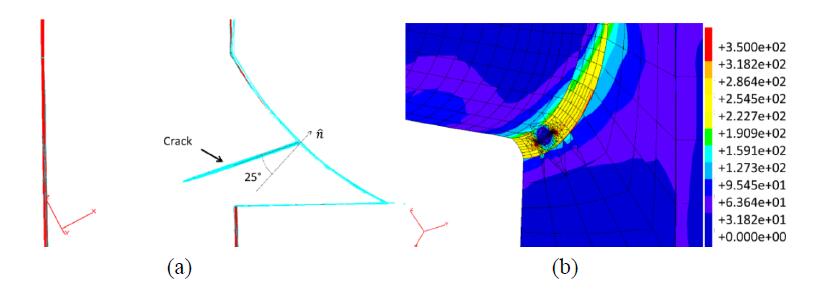
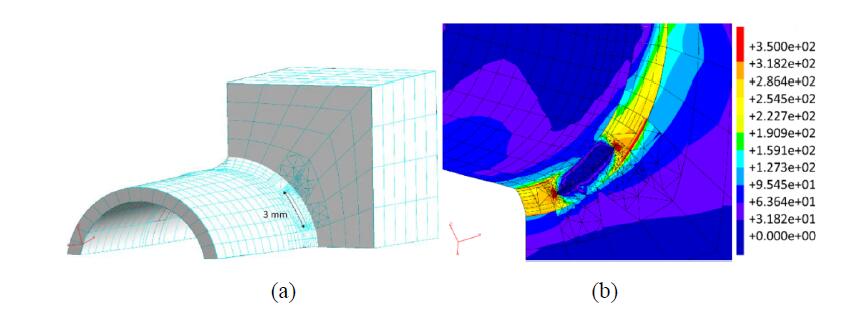
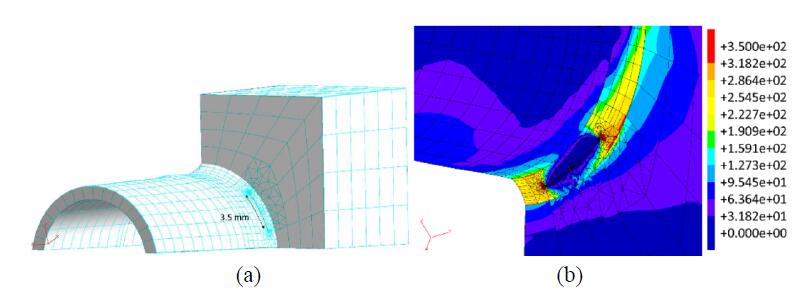
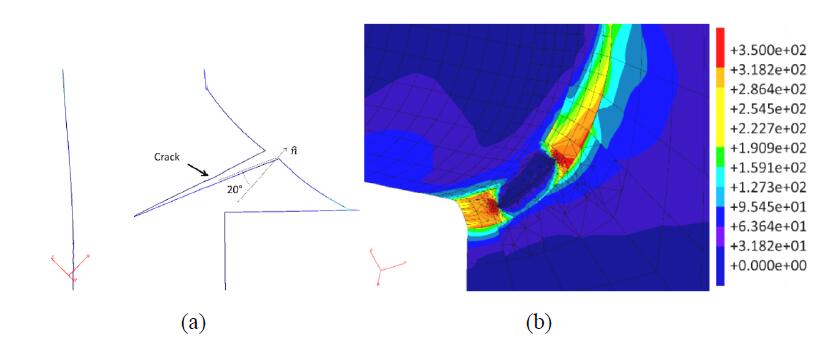
Wendelstein 7-X is the world’s largest nuclear fusion experiment of stellarator type, in which a hydrogen plasma is confined by a magnet field generated with external superconducting coils, allowing the plasma to be heated up to the fusion temperature. The water-cooled Plasma Facing Components (PFC) protect the Plasma Vessel (PV) against radiative and convective heat from the plasma. After the assembly process of heat shields and baffles, several cracks were found in the braze and cooling pipes. Due to heat load cycles occurring during each Operational Phase (OP), thermal stresses are generated in the heat sinks, braze root and cooling pipes, capable to drive fatigue crack-growth and, possibly, a water leak through the pipe thickness. The aim of this study is to assess the most dangerous initial crack configurations in one of the most critical baffles by using numerical models based on a FEM-DBEM approach.

Keywords:

Citation: R. Citarella, V. Giannella, M. A. Lepore, J. Fellinger. FEM-DBEM approach to analyse crack scenarios in a baffle cooling pipe undergoing heat flux from the plasma[J]. AIMS Materials Science, 2017, 4(2): 391-412. doi: 10.3934/matersci.2017.2.391

Related Papers:

[1]	Ana Isabel Muñoz, J. Ignacio Tello . On a mathematical model of bone marrow metastatic niche. Mathematical Biosciences and Engineering, 2017, 14(1): 289-304. doi: 10.3934/mbe.2017019
[2]	Tianyuan Xu, Shanming Ji, Chunhua Jin, Ming Mei, Jingxue Yin . EARLY AND LATE STAGE PROFILES FOR A CHEMOTAXIS MODEL WITH DENSITY-DEPENDENT JUMP PROBABILITY. Mathematical Biosciences and Engineering, 2018, 15(6): 1345-1385. doi: 10.3934/mbe.2018062
[3]	Dawid Czapla, Sander C. Hille, Katarzyna Horbacz, Hanna Wojewódka-Ściążko . Continuous dependence of an invariant measure on the jump rate of a piecewise-deterministic Markov process. Mathematical Biosciences and Engineering, 2020, 17(2): 1059-1073. doi: 10.3934/mbe.2020056
[4]	Abdulhamed Alsisi, Raluca Eftimie, Dumitru Trucu . Non-local multiscale approach for the impact of go or grow hypothesis on tumour-viruses interactions. Mathematical Biosciences and Engineering, 2021, 18(5): 5252-5284. doi: 10.3934/mbe.2021267
[5]	Katarzyna Pichór, Ryszard Rudnicki . Stochastic models of population growth. Mathematical Biosciences and Engineering, 2025, 22(1): 1-22. doi: 10.3934/mbe.2025001
[6]	Luis F. Gordillo . Optimal sterile insect release for area-wide integrated pest management in a density regulated pest population. Mathematical Biosciences and Engineering, 2014, 11(3): 511-521. doi: 10.3934/mbe.2014.11.511
[7]	Xuan Zhang, Huiqin Jin, Zhuoqin Yang, Jinzhi Lei . Effects of elongation delay in transcription dynamics. Mathematical Biosciences and Engineering, 2014, 11(6): 1431-1448. doi: 10.3934/mbe.2014.11.1431
[8]	Virginia González-Vélez, Amparo Gil, Iván Quesada . Minimal state models for ionic channels involved in glucagon secretion. Mathematical Biosciences and Engineering, 2010, 7(4): 793-807. doi: 10.3934/mbe.2010.7.793
[9]	Nikolai Leonenko, Enrica Pirozzi . The time-changed stochastic approach and fractionally integrated processes to model the actin-myosin interaction and dwell times. Mathematical Biosciences and Engineering, 2025, 22(4): 1019-1054. doi: 10.3934/mbe.2025037
[10]	Blessing O. Emerenini, Stefanie Sonner, Hermann J. Eberl . Mathematical analysis of a quorum sensing induced biofilm dispersal model and numerical simulation of hollowing effects. Mathematical Biosciences and Engineering, 2017, 14(3): 625-653. doi: 10.3934/mbe.2017036

Abstract

References

[1]	Bykov V (2009) Structural analysis of W7-X: Overview. Fusion Eng Des 84: 215–219. doi: 10.1016/j.fusengdes.2008.12.113
[2]	Corato V, Affinito L, Anemona A, et al. (2015) Detailed design of the large-bore 8T superconducting magnet for the NAFASSY test facility. Supercond Sci Tech 28: 034005. doi: 10.1088/0953-2048/28/3/034005
[3]	Citarella R, Lepore M, Fellinger J, et al. (2013) Coupled FEM-DBEM method to assess crack growth in magnet system of Wendelstein 7-X. Frattura ed Integrità Strutturale 26: 92–103.
[4]	Citarella R, Lepore M, Perrella M, et al. (2016) Coupled FEM-DBEM approach on multiple crack growth in cryogenic magnet system of nuclear fusion experiment 'Wendelstein 7-X'. Fatigue Fract Eng M 39: 1488–1502. doi: 10.1111/ffe.12466
[5]	Citarella R, Cricrì G, Lepore M, et al. (2014) Assessment of Crack Growth from a Cold Worked Hole by Coupled FEM-DBEM Approach. Key Eng Mater 577-578: 669–672.
[6]	Citarella R, Cricrì G, Lepore M, et al. (2014) Thermo-Mechanical Crack Propagation in Aircraft Engine Vane by Coupled FEM-DBEM Approach. Adv Eng Softw 67: 57–69. doi: 10.1016/j.advengsoft.2013.07.006
[7]	Citarella R, Cricrì G (2009) A two-parameter model for crack growth simulation by combined FEM-DBEM approach. Adv Eng Softw 40: 363–377. doi: 10.1016/j.advengsoft.2008.05.001
[8]	Carlone P, Citarella R, Lepore M, et al. (2015) A FEM-DBEM investigation of the influence of process parameters on crack growth in aluminum friction stir welded butt joints. Int J Mater Form 8: 591–599. doi: 10.1007/s12289-014-1186-7
[9]	Citarella R, Cricrì G (2010) Comparison of DBEM and FEM Crack Path Predictions in a notched Shaft under Torsion. Eng Fract Mech 77: 1730–1749. doi: 10.1016/j.engfracmech.2010.03.012
[10]	Citarella R, Cricrì G, Lepore M, et al. (2010) DBEM and FEM Analysis of an Extrusion Press Fatigue Failure. In: Öchsner A, Da Silva LFM, Altenbach H, Materials with Complex Behaviour-Advanced Structured Materials, Springer-Verlag, Berlin, Germany, 3: 181–191.
[11]	Citarella R, Buchholz FG (2008) Comparison of crack growth simulation by DBEM and FEM for SEN-specimens undergoing torsion or bending loading. Eng Fract Mech 75: 489–509. doi: 10.1016/j.engfracmech.2007.03.039
[12]	Dassault Systèmes Simulia Corp. (2011) Abaqus Analysis User's Manual, Version 6.12.1, Providence, RI, USA.
[13]	BEASY (2011) BEASY V10r14 Documentation, C.M. BEASY Ltd.
[14]	Rigby RH, Aliabadi MH (1993) Mixed-mode J-integral method for analysis of 3D fracture problems using BEM. Eng Anal Bound Elem 11: 239–256. doi: 10.1016/0955-7997(93)90026-H
[15]	Rigby RH, Aliabadi MH (1998) Decomposition of the mixed-mode J-integral-revisited. Int J Solids Struct 35: 2073–2099. doi: 10.1016/S0020-7683(97)00171-6
[16]	Portela A, Aliabadi MH, Rooke DP (1991) The dual boundary element method: effective implementation for crack problems. Int J Numer Meth Eng 33: 1269–1287.
[17]	EI Haddad MH, Smith KN, Topper T (1979) Fatigue crack propagation of short cracks. J Eng Mater Techn-T ASME 101: 42–46. doi: 10.1115/1.3443647
[18]	Simonovski I, Nilsson KF, Cizelj L (2007) Crack tip displacements of microstructurally small cracks in 316L steel and their dependence on crystallographic orientations of grains. Fatigue Fract Eng M 30: 1–10. doi: 10.1111/j.1460-2695.2006.01092.x
[19]	Benedetti I, Aliabadi MH, Davì G (2008) A fast 3D dual boundary element method based on hierarchical matrices. Int J Solids Struct 45: 2355–2376. doi: 10.1016/j.ijsolstr.2007.11.018

This article has been cited by:

1.	Christian Engwer, Thomas Hillen, Markus Knappitsch, Christina Surulescu, Glioma follow white matter tracts: a multiscale DTI-based model, 2015, 71, 0303-6812, 551, 10.1007/s00285-014-0822-7
2.	Thomas Lorenz, Christina Surulescu, On a class of multiscale cancer cell migration models: Well-posedness in less regular function spaces, 2014, 24, 0218-2025, 2383, 10.1142/S0218202514500249
3.	Gülnihal Meral, Christian Stinner, Christina Surulescu, On a multiscale model involving cell contractivity and its effects on tumor invasion, 2015, 20, 1553-524X, 189, 10.3934/dcdsb.2015.20.189
4.	Christina Surulescu, Nicolae Surulescu, 2013, Chapter 9, 978-3-319-03079-1, 269, 10.1007/978-3-319-03080-7_9
5.	Sandesh Hiremath, Christina Surulescu, A stochastic multiscale model for acid mediated cancer invasion, 2015, 22, 14681218, 176, 10.1016/j.nonrwa.2014.08.008
6.	JAN KELKEL, CHRISTINA SURULESCU, A MULTISCALE APPROACH TO CELL MIGRATION IN TISSUE NETWORKS, 2012, 22, 0218-2025, 1150017, 10.1142/S0218202511500175

Reader Comments

Your name:*

Email:*
© 2017 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)