|
[1]
|
Bhargava AK (2004) Engineering Materials: Polymers, Ceramics and Composites, New Delhi: Prentice-Hall of India Pvt. Ltd.
|
|
[2]
|
Morgan P (2005) Carbon fibers and their composites, Boca Raton: CRC Press.
|
|
[3]
|
Askeland D, Fulay P, Wright W (2010) The Science and Engineering of Materials, Boston: Cengage Learning.
|
|
[4]
|
CEN (European Committee for Standardization) (2005) EN 1993-1-11: Design of Steel Structures–Part 1–11: Design of structures with tension components, In: Eurocode 3, Brussels.
|
|
[5]
|
Schlaich M, Liu Y, Zwingmann B (2015) Carbon Fibre Reinforced Polymer for Orthogonally Loaded Cable Net Structures. Struct Eng Int 25: 34–42. doi: 10.2749/101686614X14043795570534

|
|
[6]
|
Quilter A (2001) Composites in aerospace applications. IHS White Paper 444: 1–5.
|
|
[7]
|
Meier U (1992) Carbon Fiber Reinforced Polymer: Modern Materials in Bridge Engineering. Struct Eng Int 2: 7–12. doi: 10.2749/101686692780617020

|
|
[8]
|
Andrä H, Maier M, Poorbiazar M (2004) Carbon fiber composites for a new generation of tendons, In: Proc., 1st Conf. on Application of FRP Composites in Construction and Rehabilitation of Structures, Tehran.
|
|
[9]
|
Winistoerfer AU, Mottram T (2001) The future of pin-loaded straps in civil engineering applications, In: Proc., 2001 US-Canada-Europe Workshop on Bridge Engineering, Zurich.
|
|
[10]
|
Taerwe L (1995) Non-Metallic (FRP) Reinforcement for Concrete Structures: Proceedings of the Second International RILEM Symposium, Boca Raton: CRC Press.
|
|
[11]
|
Santoh N (1993) CFCC (Carbon FRP Cable). Developments in Civil Engineering 42: 223–223.
|
|
[12]
|
Schober K, Rautenstrauch K (2005) Experimental investigation on flexural strengthening of timber structures with CFRP, In: Proc., 2005 International Symposium on Bond Behavior of FRP in Structures, Hong Kong.
|
|
[13]
|
Winistoefer AU (1999) Ph.D. thesis: development of non-laminated advanced composite straps for civil engineering applications, Warwick: University of Warwick.
|
|
[14]
|
Grace NF, Enomoto T, Abdel-Sayed G, et al. (2003) Experimental study and analysis of a full-scale CFRP/CFCC double-tee bridge beam. Pci J 48: 120–139. doi: 10.15554/pcij.07012003.120.139

|
|
[15]
|
Grace NF, Enomoto T, Yagi K (2002) Behavior of CFCC and CFRP leadline prestressing systems in bridge construction. Pci J 47: 90–103.
|
|
[16]
|
Pfeifer (2011) Pfeifer Tension Members, Memmingen: Pfeifer Seil- und Hebetechik GmbH.
|
|
[17]
|
Schlaich M, Zwingmann B, Liu Y, et al. (2012) Zugelemente aus CFK und ihre Verankerungen. Bautechnik 89: 841–849. doi: 10.1002/bate.201200057

|
|
[18]
|
Meier U (2012) Carbon Fiber Reinforced Polymer Cables: Why? Why Not? What If? Arabian J Sci Eng 37: 399–411.
|
|
[19]
|
Meier U (1987) Proposal for a carbon fiber reinforced composite bridge across the Strait of Gibraltar at its narrowest site. P I Mech Eng B-J Eng 201: 73–78.
|
|
[20]
|
Karbhari VM (1998) Use of Composite Materials in Civil Infrastructure in Japan. Baltimore: International Technology Research Institute.
|
|
[21]
|
Liu Y (2015) Ph.D. thesis: Carbon Fiber Reinforced Polymer (CFRP) Cables for Orthogonally Loaded Cable Structures: Advantages and Feasibility, Berlin: Technische Universität Berlin.
|
|
[22]
|
Serdjuks D, Rocens K, Pakrastinsh L (2000) Utilization of composite materials in saddle-shaped cable roofs. Mech Compos Mater 36: 385–388. doi: 10.1023/A:1026647117370

|
|
[23]
|
Feng P, Ye LP, Teng JG (2007) Large-span woven web structure made of fiber-reinforced polymer. J Compos Constr 11: 110–119. doi: 10.1061/(ASCE)1090-0268(2007)11:2(110)

|
|
[24]
|
Palkowski S (2003) Ausgewählte Probleme bei statischen Berechnungen von Seilkonstruktionen. Stahlbau 72: 708–714. doi: 10.1002/stab.200302470

|
|
[25]
|
Palkowski S (2013) Statik der Seilkonstruktionen: Theorie und Zahlenbeispiele, Berlin: Springer-Verlag (in German).
|
|
[26]
|
Noesgen J (1974) Vorgespannte Seilnetztragwerke - zum Tragverhalten des quadratischen Netzes mit starrem Rand, Cologne: Werner-Verlag.
|
|
[27]
|
Belytschko T, Liu WK, Moran B, et al. (2013) Nonlinear finite elements for continua and structures, Hoboken: John Wiley & Sons.
|
|
[28]
|
De Borst R, Crisfield MA, Remmers JJ, et al. (2012) Nonlinear finite element analysis of solids and structures, Hoboken: John Wiley & Sons.
|
|
[29]
|
SOFiSTiK (2014) SOFiSTiK user’s manual, version 2014, Oberschleißheim: SOFiSTiK AG.
|
|
[30]
|
CEN (European Committee for Standardization) (2005) EN 1991-1-4: Actions on structures–Part 1–4: General actions. In: Eurocode 1, Brussels.
|
|
[31]
|
Goris A, Schneider K (2006) Bautabellen für Ingenieure. Cologne: Werner-Verlag (in German).
|
|
[32]
|
CEN (European Committee for Standardization) (2002) EN 1990: Basis of structural design, In: Eurocode 0, Brussels.
|
|
[33]
|
CEN (European Committee for Standardization) (2005) EN 1993-1-1: Design of Steel Structures–Part 1-1: General rules and rules for buildings, In: Eurocode 3, Brussels.
|











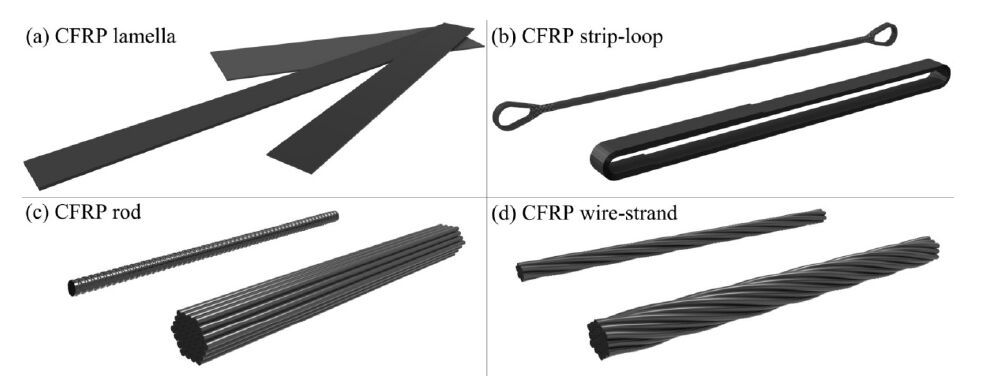

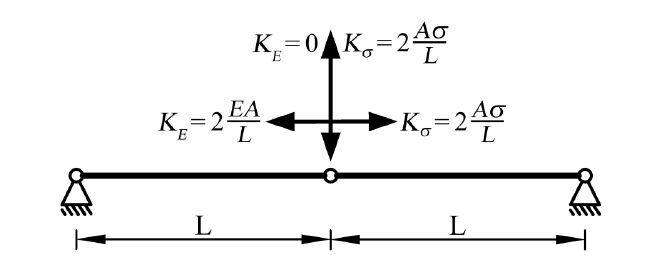
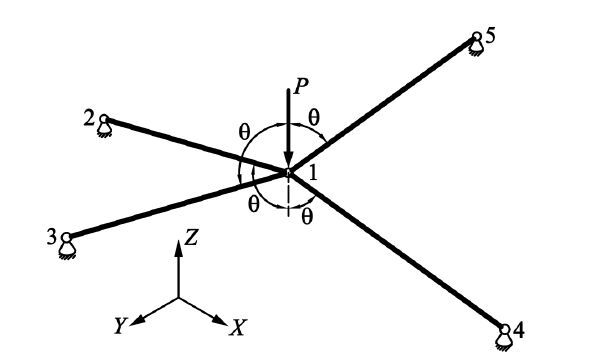
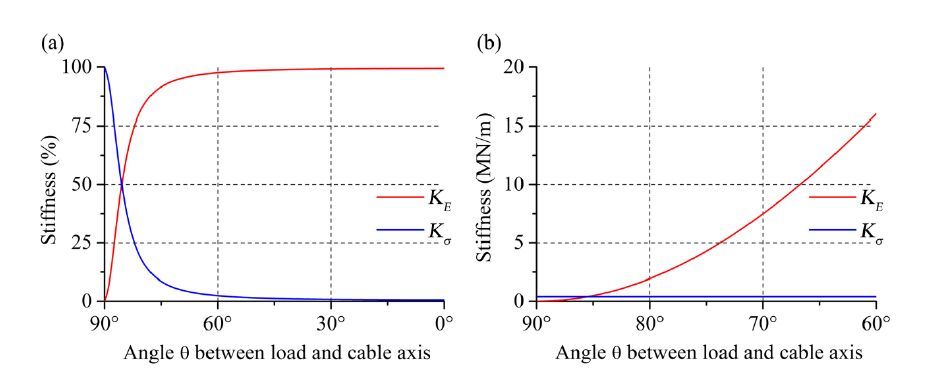









 DownLoad:
DownLoad: