Contribution of a power multivector to distorting load identification

Nomenclature: u(t): Voltage time function [V]; i(t): Current time function [A]; U: Voltage rms value [V]; I: Current rms value [A]; U_i: Rms value of i-th voltage harmonic [V]; I_i: Rms value of i-th current harmonic [A]; α_i: Phase angle of i-th voltage harmonic; β_i: Phase angle of i-th current harmonic; φ_i: Phase difference of i-th harmonic voltage and current; u: Voltage vector [V]; i: Current vector [A]; S: Apparent power [VA]; P: Active power [W]; Q: Reactive power [Var]; D_B: Budeanu's distortion power [VA]; Q_B: Budeanu's reactive power [VA]; Q_F: Fryze's reactive power [VA]; S_Q: Sharon's quadrature reactive power [VA]; S_I: Fundamental apparent power [VA]; D_I: Current distortion power [VA]; D_V: Voltage distortion power [VA]; S_H: Harmonic apparent power [VA]; S: Power multivector [VA]; P_ii: Active power of i-th harmonic [W]; Q_ii: Reactive power of i-th harmonic [Var]; Q: Reactive power bivector [Var]; Q_ii: Reactive power bivector of i-th harmonic [Var]; P_M: Nonactive power bivector associated with in-phase current components [VA]; Q_M: Nonactive power bivector associated with quadrature current components [VA]; P_Mij: Nonactive power bivector associated with i-th harmonic voltage and j-th harmonic current component in phase with its respective voltage harmonic [VA]; P_Mij: Magnitude of P_Mij [VA]; Q_Mij: Nonactive power bivector associated with i-th harmonic voltage and j-th harmonic current component in quadrature with its respective voltage harmonic [VA]; Q_Mij: Magnitude of Q_Mij [VA]; B_M: Generalized Mutual Coupling (GMC) bivector [VA]; B_Mh: Component of B_M associated with harmonics up to the 50^th order [VA]; B_Msh: Component of B_M associated with supraharmonics [VA]; u_x(t): Voltage of load x time function [V]; i_x(t): Current of load x time function [A]; α_x,i: Phase angle of i-th voltage harmonic of load x; β_x,i: Phase angle of i-th current harmonic of load x; φ_x,i: Phase difference of i-th harmonic voltage and current of load x; θ_x,i: Phase difference between the voltage at a common point of connection and the voltage of load x; U_x,i: Rms value of i-th voltage harmonic of load x [V]; I_x,i: Rms value of i-th current harmonic of load x [A]; u_x: Voltage vector of load x [V]; i_x: Current vector of load x [A]; S_x: Power multivector of load x [VA]; P_x,ii: Active power of i-th harmonic of load x [W]; Q_x,ii: Reactive power of i-th harmonic of load x [Var]; P_Mx,ij: Magnitude of nonactive power bivector of load x associated with i-th harmonic voltage and j-th harmonic current component in phase with its respective voltage harmonic [W]; Q_Mx,ij: Magnitude of nonactive power bivector of load x associated with i-th harmonic voltage and j-th harmonic current component in quadrature with its respective voltage harmonic [Var]; B_Mx: GMC bivector of load x [VA]; B_Mxp: Component of B_Mx parallel to the GMC bivector B_M at a common point of connection [VA]; B_Mxr: Component of B_Mx orthogonal to the GMC bivector B_M at a common point of connection [VA]; b_Mx, b_Mhx, b_Mshx: Coefficients of contribution of load x to B_M, B_Mh and B_Msh respectively

1.   Introduction

1.1.   Motivation and incitement

Power quality is one of the most prominent topics in modern power engineering literature. Among the numerous problems classified as power quality disturbances, power system harmonics are a serious concern for utilities and consumers alike. The growing awareness regarding the environmental impact of fossil fuel consumption as well as their impending depletion has given rise to a quest for more energy-efficient electronic equipment in industrial, commercial and residential installations. Nonlinear loads such as LED lamps, industrial converters, electronic power supplies and battery chargers are becoming increasingly common. The integration of renewable energy systems into utility grids is also rapidly growing, with photovoltaic (PV) systems emerging as major contributors to energy generation. In addition to large scale photovoltaic power plants, small scale PV installations on rooftops connected to the low voltage utility grid are practically commonplace. Furthermore, new kinds of equipment incorporating power electronic converters, such as electric vehicle (EV) battery chargers are expected to proliferate in the future. All these devices tend to inject harmonics into the grid at the point of their connection. The harmonic currents generated by these devices cause a corresponding voltage drop on the distribution line impedance resulting in a distorted voltage supply for all loads.

Waveform distortion in power systems can result in increased losses and heating in transformers and motors. It can also cause interference with communication circuits and malfunction of electronic equipment. The identification of harmonic generating customers is an important first step in controlling harmonic levels in the grid. By identifying harmonic emitting loads, appropriate mitigation solutions can be considered ^[1,2,3].

1.2.   Literature review

Various approaches for distorting load identification have been presented in the literature ^{[4,5,6,7,8,9,10,11]}. They are usually classified into multipoint and single-point methods. The former involve multiple measurement points and synchronous distributed measurements. Their superior accuracy is offset by the complexity of the required equipment and the amount of data involved. The latter involve a single measurement point at the cross-section between the grid and the load under consideration. They are less accurate but more easily applied.

Some of the single-point methods that have been proposed involve the construction of a Thevenin or Norton equivalent circuit in order to determine the dominant harmonic source. An important requirement is the accurate estimation of the utility side and customer side equivalent impedances or admittances. A traditional single-point method is based on the determination of the direction of the harmonic active power flow with respect to the fundamental active power at the point of connection of a load. By measuring harmonic voltage and current rms values and phase difference angles at the point of connection, the signs of individual harmonic active powers are derived. A bidirectional active power flow indicates that the load generates harmonics. However, it has been demonstrated that in certain situations a bidirectional flow will not occur even though the load is nonlinear ^[12]. This approach may also fail to reveal a nonlinear load simply because the values of harmonic active (and reactive) components are very low, as it is often the case in real scenarios. Due to these considerations, more recent methods focus on non-active components of previously defined apparent power equations ^[8,9,10,11]. More specifically, the proposed distorting load identification criteria are based on some kind of distortion power. This distortion power is defined as one of the components of an apparent power equation. A common characteristic of such expressions is the fact that the conservation principle does not apply to them.

In ^[8], Budeanu's distortion power is used in order to determine whether a load is nonlinear. This power quantity is defined as

where S is the apparent power and P is the active power. Also Q_B is defined as

where U_i, I_i are the rms values of the i-th harmonic voltage and current respectively and φ_i their phase difference.

Power quantities defined in IEEE Std. 1459 ^[13] have also been considered for the identification of distorting loads ^[9,10]. The apparent power equation suggested by IEEE Std. 1459 is the following.

where S_I = U₁I₁ is the fundamental apparent power, D_I = U₁I_H is the current distortion power, D_V = U_HI₁ is the voltage distortion power and S_H = U_HI_H is the harmonic apparent power. Furthermore, if U, I are the rms values of the voltage and current, then

and

In ^[11], an approach was presented based on the comparison of three different nonactive powers found in the literature. The three quantities are the fundamental reactive power, i.e.,

Fryze's reactive power, defined as

and Sharon's quadrature reactive power, defined as

The fundamental reactive power Q₁₁ is considered as a minimum reference value, since it will usually be nonzero, even in the absence of harmonics. Q_F is the nonactive power written as a single entity and equals Q₁₁ in the sinusoidal case. S_Q assumes an intermediate value between Q₁₁ and Q_F. If the value of S_Q is closer to Q₁₁ than to Q_F, then the source of harmonic pollution is assumed to be upstream from the metering point, otherwise the source of harmonic pollution is assumed to be downstream from the metering point.

1.3.   Contribution and paper organization

Distorting load identification based on power components calculated at the load terminals is one of the incentives for the development of a power theory for circuits with periodic, nonsinusoidal waveforms. For decades, the proposed approaches were mainly focused on providing a decomposition of the apparent power based on various criteria and this is still the norm ^[13]. However, any apparent power equation can only provide limited information, due to the fact that its terms are squared and often aggregated ^[14]. This can be an issue even for the sinusoidal case. The apparent power equation S² = P² + Q² needs to be accompanied by an expression such as capacitive/inductive for the reactive power. On the other hand, the complex power S = P + jQ fully describes power related phenomena at a cross-section between a source and a linear time invariant passive load of any complexity. Its magnitude is equal to the apparent power and its direction and sense are defined by its components on the two axes of the complex plane.

The multidimensionality of voltages and currents under nonsinusoidal conditions does not permit the representation of power by means of complex algebra. Even though current and voltage calculations can be performed using phasor representations for each individual frequency based on the superposition principle, the same principle cannot be applied in power calculations. In ^[14,15] a novel energy flow model to describe all power components at a cross-section between a source and a load under nonsinusoidal conditions was introduced. An interpretation of the power components in association with an equivalent circuit was presented. For the representation of power, the proposed model was based on the Geometric Algebra (GA) framework ^[16]. More specifically, a power multivector was introduced, which is fully capable of providing all the necessary information to determine the power components. The apparent power S can be derived as the magnitude of the multivector. The aim of this paper is to investigate the information that can be derived from the components of this power multivector regarding the impact of a load on the distortion at a point of interest.

This paper is organized as follows. The multivectorial representation of power that will be utilized in the proposed approach is briefly outlined in Section 2 and an index for distorting load identification based on this representation is introduced. The determination of the role of a specific load in the distortion at a common point of connection based on this index is presented in Section 3. Simulations using the proposed approach are presented in Section 4. The results are discussed in Section 5. The conclusions are presented in Section 6.

2.   Geometric Algebra representation of power

2.1.   Formulation of a power multivector

For the following analysis it will be assumed that the voltage and the current contain the same harmonic orders. However, the approach proposed in ^[14,15] can handle any situation, including the case of harmonics only present in the voltage or the current.

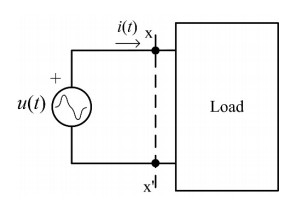
Let us consider the circuit in Figure 1, where a load is supplied by a periodic, nonsinusoidal voltage source. The voltage at the cross-section x-x΄ is

where U₁, U_k are the rms values of the fundamental and the k-th harmonic voltage component respectively and α₁, α_k are the phase angles.

The load current will consist of harmonic components of the same order, with rms values I₁, I_k and phase angles β₁, β_k respectively. If φ_i = α_i – β_i, for i = 1, k then the current can be decomposed into subcomponents in phase and in quadrature with the corresponding harmonic voltage component, as follows.

Functions u(t) and i(t) are expressed as linear combinations of the four orthonormal basis functions $\left\{ {\sqrt 2 \cos \left({\omega t + {\alpha _1}} \right)} \right.$, $\sqrt 2 \sin \left({\omega t + {\alpha _1}} \right)$, $\sqrt 2 \cos \left({k\omega t + {\alpha _k}} \right)$, $\left. {\sqrt 2 \sin \left({k\omega t + {\alpha _k}} \right)} \right\}$. They can also be represented by vectors u and i in a 4-dimensional vector space V₄ spanned by 4 orthonormal basis vectors {e₁, e₂, e₃, e₄}, which have a one-to-one correspondence with the basis functions, as follows.

and

The vectors of V₄ generate a larger linear space, the geometric algebra G₄ of V₄ which is spanned by {1, e₁, e₂, e₃, e₄, e₁₂, e₁₃, e₁₄, e₂₃, e₂₄, e₃₄, e₁₂₃, e₁₂₄, e₁₃₄, e₂₃₄, e₁₂₃₄}. In this basis, 1 is a scalar, e₁, e₂, e₃, e₄ are unit vectors, e₁₂, e₁₃, e₁₄, e₂₃, e₂₄, e₃₄ are unit bivectors, e₁₂₃, e₁₂₄, e₁₃₄, e₂₃₄ are unit 3-vectors and e₁₂₃₄ is a unit 4-vector.

Power can be expressed as a multivector S in G₄ generated by the geometric product of the voltage and current vectors, i.e.,

The power multivector S can be calculated by using (11), (12) and (13), along with the following rules:

1) The geometric product of two basis vectors generates a basis bivector, for example e₁e₂ = e₁₂.

2) A basis vector squares to +1, for example e₁e₁ = 1.

The power multivector S at the cross-section x-x′ can be written as follows:

where P_ii = U_iI_icosφ_i, Q_ii = U_iI_isinφ_i, P_Mij = U_iI_jcosφ_j, Q_Mij = U_iI_jsinφ_j, i = 1, k and j = 1, k.

By grouping together terms of the same nature it can be written that

Power is therefore represented by a multivector that consists of a scalar part corresponding to average power and a bivector part corresponding to nonactive power. The bivector part is expressed as a linear combination of basis bivectors. All components are defined through their magnitude, direction and sense. More specifically, there are scalar terms P_ii associated with active power in each harmonic. Also, there are bivectorial, nonactive power terms, uselessly contributing to the increase of the apparent power. Bivectorial terms are associated with current components that are either in phase or in quadrature with a voltage component. The symbol P is used to designate the former and the symbol Q is used to designate the latter. Bivectorial terms with magnitude Q_ii correspond to the reactive power in each harmonic. The remaining terms with magnitudes of the form P_Mij and Q_Mij can be associated with a mutual coupling effect attributed to the difference in frequency between the currents i₁(t) and i_k(t) ^[15]. It should be noted that at the terminals of purely reactive elements P_Mij terms are zero. A predominantly capacitive element, such as a compensation capacitor, can thus readily be identified.

It was shown in ^[14] that the power multivector in its analytic form (14) can successfully demonstrate the bidirectional active power flow that occurs when a nonlinear/time-varying load is supplied by a sinusoidal voltage source with internal impedance. When a bidirectional active power flow is detected, then the presence of a harmonic source in the load side is confirmed. However, as it was demonstrated in ^[12], when the source voltage is non-sinusoidal, then, in the case of harmonics produced in the load side that are also present in the source voltage, the direction of active power is affected by the relative phase angle between the two harmonic sources. This means that even when harmonic active powers at the metering point turn out to be positive it cannot be safely assumed that there is no harmonic source in the load side. Therefore, in this paper, another part of the power multivector will be utilized in the identification of distorting loads.

By using bold notation to denote individual bivectorial terms, it can be written that

or, more concisely,

where

Furthermore, it can be observed that terms P_M1k = P_M1ke₁₃ and P_Mk1 = P_Mk1e₃₁ have the same direction and opposite sense, due to the fact that e₁₃ = –e₃₁. In the case of i = 1, k and j = 1, k, the power multivector S can thus be rewritten in the compact form

In a more general situation including multiple harmonics, the terms of P_M can be grouped into pairs of identical direction but opposite sense, as follows.

In ^[14], it was demonstrated that the total apparent power S is the magnitude of the multivector S. Two apparent power equations can thus be derived. From the analytic form (14) it can be derived that

or, in more general notation,

From the compact form (22) it can be derived that

or, in more general notation,

where

2.2.   The generalized mutual coupling bivector

It was demonstrated in ^[14] and ^[15] that apparent power equations proposed by other methods dealing with power theory can be extracted from (25) or (27). Components of these equations have been utilized in harmonic load identification ^[8,9,10,11]. However, apparent power equations do not include all the necessary information to describe the power components, which may lead to erroneous conclusions.

In (17), the reactive power Q₁₁ is not associated with the distortion. Harmonic reactive power components of the form Q_ii, $i \ne 1$, are usually relatively small, so they will not be considered either. The remaining bivectorial components will be grouped into a bivector B_M, as follows:

Bivector B_M will be henceforth referred to as the generalized mutual coupling (GMC) bivector due to the nature of the terms it comprises. The formulation of this bivector requires knowledge of the rms values of voltage and current and their phase difference, at every harmonic, at the load terminals.

In a more realistic scenario, with multiple harmonics present, all possible harmonic pairs have to be taken into account in the formulation of bivector B_M. More specifically, if there are H harmonics present in the circuit, including the fundamental, and N possible pairs of harmonics, then for harmonics of orders l and m ($l \ne m$), forming pair z, it can be written that

where e_a, e_b and e_c, e_d are pairs of basis vectors uniquely associated with harmonics l and m respectively, with $a \in \left\{ {1, 3, 5, ..., 2H - 1} \right\}$, b = a + 1, $c \in \left\{ {1, 3, 5, ..., 2H - 1} \right\}$, $c \ne a$ and d = c + 1. Bivector B_M can then be calculated as the sum of the N bivectors resulting from the N pairs of harmonics.

Another grouping of bivectorial terms may also be useful, depending on the profile of harmonic pollution. More specifically, bivector B_M can be decomposed into subcomponents involving different groups of harmonics. For example, B_Mh may contain terms that only involve harmonics of order lower than 50 and B_Msh may contain terms that involve at least one harmonic of order over 50. Then

Harmonics of orders beyond the 50^th are called supraharmonics. They have lately gained the attention of researchers due to the impact of supraharmonic sources on neighbouring loads and the fact that, despite their rising levels, there are no regulations in place for their mitigation ^[17,18,19]. Indeed, existing standards regarding harmonic limits consider harmonics up to the 50^th (or 40^th) order. Equipment manufacturers usually produce devices that comply with imposed limitations, but this is often accompanied by a simultaneous increase of the distortion at harmonic orders well beyond the 50^th. As a result, significant supraharmonic content can be detected at the point of connection of PV inverters, EV battery chargers etc. Furthermore, even if the bivector B_Msh at the common point of connection of a distribution line turns out to be low, interactions among individual loads may be significant enough to generate noticeable disturbances. The analysis proposed in this paper can provide valuable insight in this case. On the other hand, if enforcing compliance to existing limits is the only objective, then components beyond the 50^th don't have to be taken into account at all, and a single bivector including only harmonics up to the 50^th order can be considered.

3.   Assessment of a distorting load impact

3.1.   GMC bivector at the terminals of a load

In order to find the multivector S_x of a specific load x, the current at the cross-section between the load and the rest of the network has to be analysed into a component parallel to its respective voltage and a component orthogonal to it. This is also true for the multivector at any point along the network, such as the source terminals. However, in order to correlate the two multivectors, they need to be expressed using the same basis.

The cross-section between the source and the rest of the network will be used as a reference, with a voltage given by (9) and a multivector given by (17). All functions referring to the load will then be expressed using the function basis chosen for the source. This means that every phase angle has to be expressed in terms of the phase angle of the respective harmonic of the reference voltage. Equivalently, all vector and multivector expressions corresponding to load x must be expressed using the corresponding geometric algebra basis.

Let us assume that the voltage u_x(t) of load x is of the form

where U_x,1, U_x,k are the rms values of the fundamental and the k-th harmonic voltage component respectively and α_x,1, α_x,k are the phase angles. Let θ_x,i = α_i – α_x,i, , for i = 1, k, then

The load current will consist of two harmonic components with rms values I_x,1, I_x,k and phase angles β_x,1, β_x,k respectively. If φ_x,i = α_x,i – β_x,i = α_i – θ_x,i – β_x,i, for i = 1, k, then the current can be decomposed into subcomponents in phase and in quadrature with the corresponding harmonic voltage component of (36), as follows.

Any function of the form

can be represented by a vector that results from a rotation of the vector e₁, which lies on the plain defined by the bivector e₁₂, by an angle y₁. This vector can be written as

where R_y1 = cos(y₁/2) + e₁₂sin(y₁/2) represents the rotation and ${{\bf{R}}}_{y1}^\dagger$ its reverse. Similarly, a function of the form $\sqrt 2 \sin (\omega t + {a_1} - {y_1})$ corresponds to

Such representations can be derived for components of any harmonic order. Voltage and current vectors at the load terminals can thus be expressed as follows.

and

where

The power multivector at the load terminals can be calculated as the geometric product of (41) and (42), i.e.,

This geometric product will contain the following terms.

where

Similarly

due to e₃₄₁₂ = e₁₂₃₄.

Therefore, the power multivector of the load in the new basis can be written as

where P_x,ii = U_x,iI_x,icosφ_x,i, Q_x,ii = U_x,iI_x,isinφ_x,i, P_Mx,ij = U_x,iI_x,jcosφ_x,j, Q_Mx,ij = U_x,iI_x,jsinφ_x,j, i = 1, k and j = 1, k.

It can thus be deduced that active and reactive power components of the form P_x,ii and Q_x,ii are not affected by the basis change. The remaining terms are grouped into the GMC bivector of the load B_Mx. The magnitude of this bivector is preserved in the new basis, but individual subcomponents are transformed as follows.

The multivector R_x is associated with the phase angle difference between the voltage at the reference terminals and the voltage at the terminals of load x. When there are multiple harmonics present, then more of these multivectors have to be derived, one for each possible harmonics pair. More specifically, for harmonics of orders l and m ($l \ne m$), forming pair z, it can be written that

where

Then the GMC bivector of element x can be calculated as the sum of the N bivectors resulting from the N pairs of harmonics. It should be noted here that a different approach is possible. All voltage and current functions could have been expressed in terms of the orthonormal basis functions {$\sqrt 2 \cos \omega t$, $\sqrt 2 \sin \omega t$, $\sqrt 2 \cos k\omega t$, $\sqrt 2 \sin k\omega t$}. Components P_ii, Q_ii would not be affected, but the remaining bivectorial components would have no distinguishing characteristic. This could be counterintuitive when mitigation of such components with passive elements is an objective.

3.2.   Conservation of power multivector components

According to Tellegen's theorem the sum of the power of all circuit components is zero, or, equivalently, the power delivered to the network is equal to the power that is received. Every power quantity that results as a product of quantities that represent voltage and current and are subject to KVL and KCL constraints complies with this theorem. Voltage and current vectors are such quantities, and thus the power multivector S is conservative.

For a network with various loads the multivector S at the cross-section between the source and the rest of the network can be written as

where S_n is the power multivector of load n. This subscript will be used to denote all quantities referring to this load.

The scalar parts of the power multivectors represent active power components and their sum equals the active power of the source. More specifically, from (22) and (54) it can be deduced that

Reactive power bivectors of the form Q_ii are independent from the rest of the multivector terms, as indicated by their respective basis bivectors in (22) and (54). Therefore, for every harmonic order, the sum of the reactive power bivectors of the loads equals the reactive power bivector of the source. More specifically, for the fundamental frequency it is

and for the k-th harmonic order it is

The remaining power multivector components constitute B_M. From (22), (54), (59)–(61), it can be deduced that

This equation is valid at the cross-section between the source and the rest of the network, regardless of the way the loads are connected. By associating B_Mx with B_M the impact of load x on B_M can be assessed.

3.3.   Contribution of a load to the GMC bivector at the supply terminals

According to (62), the GMC bivector at the supply terminals equals the sum of the GMC bivectors of the loads. Each of the load bivectors in (62) has its own magnitude, direction and sense. They counteract each other in every other direction but the direction of B_M. Each of them can thus be decomposed into a component parallel to B_M and a component orthogonal to it.

If bivector B_Mx of load x is decomposed into a parallel component B_Mxp and an orthogonal component B_Mxr, then the former will represent the part of B_Mx that participates in B_M and the latter the remaining part of B_Mx. The sum of the parallel components of all load bivectors equals B_M, whereas the sum of the orthogonal components equals zero. The parallel component can be associated with an energy exchange between the load and the source, whereas the orthogonal component with energy interactions of the loads, as perceived by the source.

The parallel component B_Mxp can be expressed as

where b_Mx is a signed scalar coefficient. Component B_Mxp has the same direction as B_M and magnitude and sense given by b_MxB_M, where B_M is the magnitude of B_M. It can be calculated as a projection in GA, i.e.,

where B_M^-1 is the inverse of B_M and ${{{\bf{B}}}_{Mx}} \cdot {{{\bf{B}}}_M}$ the inner product of the two bivectors. Furthermore,

where ${B}_M^\dagger$ is the reverse of B_M. Due to the fact that B_M is a bivector, the inner product can be calculated as the scalar part ${\left\langle {{{{\bf{B}}}_{Mx}}{{{\bf{B}}}_M}} \right\rangle _0}$ of the geometric product B_MxB_M. Furthermore, the reverse can be calculated as follows.

Therefore,

Coefficient b_Mx can thus be calculated as follows:

When all the loads are taken into account, then

Furthermore, if supraharmonics and lower order harmonics are examined separately and B_M is decomposed into B_Mh and B_Msh, then two distinct coefficients can be derived, i.e., b_Mhx and b_Mshx.

4.   Examples

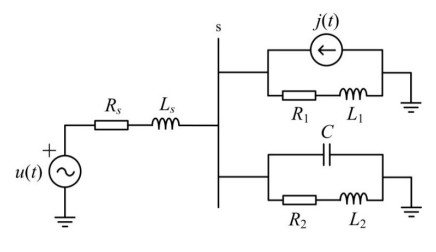
Let us consider the circuit in Figure 2, where a nonideal source is supplying two loads, one of which is nonlinear.

For the source: R_S = 0.01 Ω, L_S = 2 mH, for the loads: R₁ = 9 Ω, L₁ = 12 mH, R₂ = 7 Ω, L₂ = 30 mH. The capacitor has C = 125 μF and is considered separately. Furthermore,

The GMC bivectors of the circuit components are

The GMC bivector at point s, which is considered to be the common point of connection of all loads, is

A summation of individual circuit element bivectors results in B_M, as expected. However, from the viewpoint of s, the loads are responsible for the following:

The remaining components, indicating load interactions, are

The magnitudes of the bivectors of the loads are B_M1 = 458.2 VA, B_M2 = 67.7 VA, B_MC = 531.0 VA. At first glance, the capacitor behaviour seems to be similar to that of the nonlinear load, or even more disruptive. However, the bivector components of the capacitor happen to counteract the respective components of the nonlinear load, i.e., when one circuit element delivers power the other receives. This observation would not be possible just by inspecting bivector magnitudes, or any index based on apparent power components. For example, the harmonic apparent power S_H according to IEEE Std. 1459 ^[13] is 0.1 VA for the linear load, 7.4 VA for the nonlinear load and 8.8 VA for the capacitor.

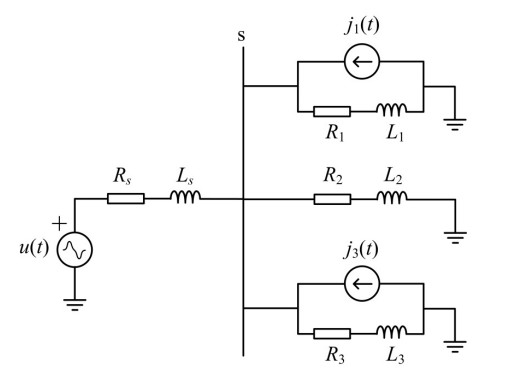
Let us consider the circuit in Figure 3, where a nonideal source is supplying three loads, two of which are nonlinear.

For the source: R_S = 0.01 Ω, L_S = 2 mH, for the loads: R₁ = R₃ = 9 Ω, L₁ = L₃ = 12 mH, R₂ = 7 Ω, L₂ = 30 mH. Furthermore,

Various indices that have been proposed for load identification have been calculated and are presented in Table 1 for γ = 0° and in Table 2 for γ = 180°. The GMC bivector magnitudes and b_M coefficients have also been included.

In the case of Table 1, the Total Harmonic Distortion indices for the current and the voltage at the common point of connection s are ITHD = 12.2% and VTHD = 11.8% respectively. The two identical nonlinear loads equally contribute to the GMC bivector magnitude at point s. More specifically, they are each responsible for 0.451·2404.7 = 1084.5 VA of the total 2404.7 VA at point s.

In the case of Table 2 the distortion at the supply terminals is radically reduced. More specifically, ITHD = 1.5% and VTHD = 3.6%. This is evident in the GMC bivector magnitude, as well as the rest of the indices calculated at point s. However, the participation of each harmonic source has to be determined. According to the harmonic apparent power S_H defined in IEEE Std. 1459 the two sources seem almost equally disruptive. The triplet of Q₁₁, S_Q and Q_F does not differentiate the two loads either. According to Budeanu's distortion power D_B and the GMC bivector magnitude the two sources are somewhat differentiated, but important information is still missing. It should also be noted that there is no bidirectional active power flow at the point of connection of load 3. More specifically, the fundamental active power is 4093.5 W, the 5^th harmonic active power is 38.1 W and they both have positive signs. Therefore, the harmonic active power flow direction does not provide helpful information. On the other hand, by examining the b_M coefficients for the two loads it can be deduced that load 1 contributes 2.281·413.9 = 944.1 VA, whereas load 3 mitigates the disturbance by 1.583·413.9 = 655.2 VA, as indicated by the sign of its b_M coefficient.

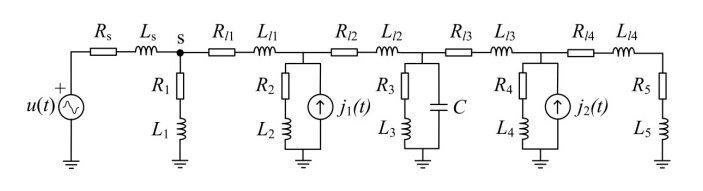
Let us now consider the circuit in Figure 4.

For the source: R_S = 0.0002 Ω, L_S = 0.06 mH, for the loads: R₁ = 14 Ω, L₁ = 16 mH, R₂ = 12 Ω, L₂ = 20 mH, R₃ = 8 Ω, L₃ = 25 mH, R₄ = 15 Ω, L₄ = 15 mH, R₅ = 10 Ω, L₅ = 14 mH, for the connecting line segments: R_l1 = 0.005 Ω, L_l1 = 0.05 mH, R_l2 = 0.01 Ω, L_l2 = 0.1 mH, R_l3 = 0.02 Ω, L_l3 = 0.2 mH, R_l4 = 0.01 Ω, L_l4 = 0.1 mH. The capacitor has C = 120 μF and is considered separately. Furthermore,

In this case, a one-by-one comparison of bivector terms is neither practical nor helpful. B_Mh, B_Msh of all loads and b_Mh, b_Msh coefficients are shown in Table 3.

Table 3 indicates that the GMC bivector at point s is mainly affected by loads 2 and 4, as well as the capacitor. However, as indicated by b_Mhx, the actual impact of the capacitor on the bivector at point s is less critical. Furthermore, by examining the distortion associated with the supraharmonic (h = 100) in the current, it can be deduced that the capacitor has a mitigating effect. This could be detrimental to the capacitor, but that is beyond the scope of this paper. It should be noted that, in this case, there is no bidirectional active power flow at the point of connection of load 2, so the harmonic active power flow direction does not provide helpful information.

5.   Discussion

In the examples of Section 4, the GMC bivector was used in order to assess the impact of a load on the distortion at a common point of connection. Its unique feature is the fact that it contains information regarding not only magnitude, but also direction and sense. The magnitude can serve as an indicator of a distorting load just like other indices proposed by methods based on components of an apparent power equation, but the additional information included in the bivector can also reveal whether the load adds to the overall distortion or acts in a mitigating manner. Furthermore, its conservative nature permits an association between the distortion at the load terminals and a common point of connection where the distortion has to be assessed and regulated. This particular feature is missing from the apparent power, as well as any components derived from possible decompositions of that quantity.

According to the proposed approach all loads can be viewed as black boxes. As long as the voltage and current phasors at every harmonic are available at the load terminals, either through measurements or calculations, then the proposed bivector can be derived. However, a common concern with single-point methods is the fact that load current harmonics flowing through the supply system impedance cause a corresponding voltage drop that affects the voltage supply of every load in the circuit. As a result, nonlinear loads contribute to the harmonic pollution in the supply voltage, interfering with the harmonic current emissions of other loads and even causing harmonic emissions from linear loads. A severely distorted voltage can cause large linear loads to produce unexpectedly high harmonic currents. In such cases, linear loads can be mistaken for nonlinear ones, even though they are not to blame for the distortion. On the other hand, a moderate voltage distortion will also result in harmonic current injection from linear loads, but the associated B_M magnitude will be low. Therefore, the presented approach is based on the assumption that voltage distortion is moderate. This assumption is common among single-point methods and is based on the observation that the control of the supply voltage harmonics is the responsibility of the utility and that normally the necessary measures to ensure compliance with standard limits will be taken.

In the examples presented in Section 4 the circuits are assumed to be supplied by voltage sources with nonzero impedance. In addition, a distorted source voltage waveform was considered in the second circuit. The harmonic distortion of the load voltages is considerable. Due to this fact, the linear loads participate in the distortion at point s. However, their contribution is not comparable to that of nonlinear loads. On the other hand, in the circuit of Figure 4 the contribution of load 5 to the supraharmonic distortion at point s ended up being unexpectedly high, even though no supraharmonic was included in the source voltage. This is due to the supraharmonic currents injected by the nonlinear loads, which, at first glance, could be considered insignificant. However, these currents combined with the high impedance of the line in this frequency resulted in a considerable supraharmonic component in the voltage supply of the loads. The necessity for regulations dealing with consumers generating supraharmonics is evident.

In the examples of Figure 2 and Figure 4 of Section 4 capacitors are included in the circuits. Depending on the order of the harmonics present in the system, capacitors appear to either enhance or mitigate the distortion. If the former is true, then the presence of the capacitor is causing a disturbance that needs to be addressed. Nevertheless, the impact of a capacitor should be differentiated from that of a nonlinear load. This information is inherent in the bivector, since bivector component P_M results from components of the form P_Mij, which are all zero at the terminals of a capacitor. Therefore, a capacitor cannot be mistaken for a nonlinear load, even if its B_M bivector magnitude turns out to be comparable to that of a nonlinear load.

However, it should be noted that if the capacitor is considered to be part of a larger installation viewed as a black box and available measurements are strictly limited to the installation terminals, then the overall impact of this installation as a single entity can only be assessed. The proposed method cannot offer information regarding the exact nature of individual devices inside the black box and their interconnections. If the installation turns out to be exacerbating harmonic distortion, then the necessity for corrective measures will have to be examined. A more detailed study of the installation and its specific components utilizing optimization techniques can reveal the most effective measures for reducing the impact of this installation on harmonic distortion.

6.   Conclusions

In this paper, components of a previously introduced power multivector were utilized in the identification of distorting loads. These components were grouped into a single quantity, the generalized mutual coupling (GMC) bivector B_M, with magnitude, direction and sense. In situations where the magnitude provides ambiguous information, an inspection of its other two attributes can provide useful guidance. When the distortion at a specific point of a network comprising various loads is examined, then the impact of individual loads can be evaluated by means of b_M coefficients. These coefficients assign a part of the GMC bivector at the point of interest to each load. The remaining part of each load bivector is associated with load interactions and has no bearing on the bivector at the point of interest.

Conflict of interest

All authors declare no conflicts of interest in this paper.