The aim of this paper is to consider a non-autonomous predator-prey-like system, with a Gompertz growth law for the prey.By introducing random variations in both prey birth and predator death rates,a stochastic model for the predator-prey-like system in a random environment is proposed and investigated.The corresponding Fokker-Planck equation is solved to obtain the joint probability density for the prey and predator populations and the marginal probability densities.The asymptotic behavior of the predator-prey stochastic model is also analyzed.
1.
Introduction
For centuries, Asian people have utilized soybean (Glycine max L., Fabaceae) as a part of their staple diet. Soybean-based products, both fermented and non-fermented, have traditionally been widely consumed in East and Southeast Asia [1]. Besides their exceptional nutritional qualities and functional properties, the consumption of soy products worldwide is due to their affordability and sustainability [2]. Among products available in the market, fermented soybean products gained more attention in health-conscious societies, owing to their health benefits and the fermentation process that contributes to the functionality of products [3]. Previous research reported that during the fermentation of soybean, several biochemical changes occur in the soybean, which are catalyzed naturally by microorganisms [4]. These modifications increase nutrient bioavailability, degrade anti-nutrients, improve the antioxidant activity, release flavor, improve product stability and even create a new product form [5]. Soy products are typically fermented by either fungi or bacteria, resulting in a variety of product characteristics [6]. Tempeh, a mold-based fermentation of soybean products that has gained worldwide attention, is one of the most popular soy foods.
Tempeh (also referred to as tempeh) is a traditional fermented soybean product originating from Indonesia and is widely consumed as a staple, inexpensive protein source [7]. Besides high amounts of protein, tempeh contains high amounts of fiber, low amounts of saturated fat and low amounts of sodium [2]. Tempeh has been regarded as a source of functional properties such as antioxidants [8,9] and bioactive peptides [10]. Moreover, the intake of tempeh has been linked to preventing degenerative diseases such as hypertension, atherosclerosis, cardiovascular diseases, diabetes and cancer [10]. The flavonoid compounds in soybean, such as isoflavones, have been reported as the major antioxidants in tempeh [11]. During fermentation, isoflavones in conjugated form are broken by the enzyme beta-glucosidase secreted by the molds, resulting in the free form of isoflavones. Thus, this phenomenon increases its bioavailability and increases antioxidant capacities [12]. Apart from isoflavones, several compounds responsible for the antioxidant activities of tempeh have been described, such as free amino acids, tocopherols and 3-hydroxyanthranilic acid [13,14].
The design of fermentation conditions could affect the antioxidant capacities of tempeh. Previous research reported that fermentation conditions could affect the antioxidant activity of tempeh, such as incubation under aerobic and anaerobic conditions [15] and fermentation time [16]. However, comprehensive studies reporting the addition of acid during fermentation and its effects on physicochemical characteristics of tempeh are limited. In this study, lactic acid was used to reduce the pH of soybean to replace the function of Lactobacillus during soaking step. In addition, the effects between fermentation time, incubation temperature and different lactic acid concentrations on antioxidant capacities of tempeh remains unknown. Therefore, this research focused on evaluating various fermentation conditions (lactic acid addition, fermentation time, fermentation temperature) on antioxidant capacities and isoflavones contents of tempeh.
2.
Materials and method
2.1. Tempeh preparation
About 200 g of soybean was soaked for 4 h and dehulled. After removing the hull, soybean was added into boiling water containing 0.8%, 0.9% and 1.0% lactic acid and cooked for 30 mins. The soybean was cooled down and added with a tempeh starter containing 106 CFU/gram Rhizopus oligosporus and 2 grams of starter was added into 1 kg of soybean. Afterward, the soybean that had been mixed with starter was packaged into a perforated plastic bag and incubated at 25 ℃, 30 ℃ and 35 ℃ for 2, 3 and 4 days. Obtained tempeh was freeze-dried and ground into powders using a grinder machine and the powders were stored at −20 ℃ for further analysis. Color value (L*, a* and b*) was measured using a chromameter (TC-1500 DX, Densoku, Tokyo, Japan).
2.2. ABTS radical scavenging activity
ABTS radical scavenging activity was measured following the previous report [17]. ABTS was dissolved in water to a 7 mM concentration. The ABTS radical cation (ABTS•+) was produced by reacting ABTS stock solution with 2.45 mM potassium persulfate (final concentration), allowing the mixture to stand in the dark at room temperature for 12-16 hours before use. For the study of co-inoculated soy tempeh extract, the ABTS•+ solution was diluted with ethanol to an absorbance of 0.70 (± 0.02) at 734 nm and equilibrated at 30 ℃. After the addition of 1.0 mL of diluted ABTS•+ solution to 10 µL of freeze-dried co-inoculated soy tempeh or Trolox standards (final concentration 0-15 µM) in methanol, the absorbance reading was taken at 734 nm using a spectrophotometer after 6 mins incubation. The EC50 value represents the effective concentration to reach 50% of radical scavenging activity. Radical scavenging activity (%) was calculated using the following equation:
2.3. Ferrous ion chelating activity
The Fe2+-chelating ability was determined according to the method of Huang et al. [18]. The Fe2+ was monitored by measuring the formation of the ferrous iron-ferrozine complex at 562 nm. The sample was mixed with 2 mM FeCl2 and 5 mM ferrozine at a ratio of 10:1:2. The mixture was shaken and left to stand at room temperature for 10 min. The absorbance of the resulting solution at 562 nm was measured using a spectrophotometer. The lower the absorbance of the reaction mixture is, the higher the Fe2+-chelating ability is. The capability of the sample to chelate the ferrous iron was calculated using the following equation, and the EC50 value represents the effective concentration to reach 50% of chelating activity.
2.4. Total phenolic content
Total phenolic content was determined based on a method described by Strycharz and Shetty [19]. Briefly, 1 mL of sample was added to 1 mL of 95% ethanol, 5 mL of distilled deionized water and 0.5 mL of 50% (v/v) Folin-Ciocalteau reagent and reacted for 5 min. Afterward, 1 mL of 5% (w/v) Na2CO3 was added and reacted for 1 hour. The color generated was measured by a spectrophotometer at 725 nm, and the amount was calculated based on a gallic acid (0-100 µg/mL) standard curve.
2.5. Isoflavone compounds determination by HPLC
The contents and compositions of isoflavones were determined quantitatively by HPLC, as previously described [20]. The HPLC system (Hitachi, Tokyo, Japan) consisted of a Chromaster 5110 pump, a Chromaster 5210 autosampler, and a Chromaster 5430 diode array detector. A Mightysil RP-18 GP column (4.6 × 250 mm) (Kanto Chemical Co. Inc., Tokyo, Japan) was set at 40 ℃. The mobile phase for HPLC consisted of solvent (A), i.e., 0.1% (v/v) acetic acid in filtered MilliQ water, and (B) solvent, i.e., 0.1% (v/v) acetic acid in acetonitrile. The following gradient for solvent B was applied: 15-25% over 35 mins, 25-26.5% over 12 min, and 26.5-50% over 30 s, followed by isocratic elution for 14.5 mins. The flow rate was 1.0 mL/min, the sample amount was 20 µL, and the absorbance was measured at 254 nm. The isoflavone content of the samples was calculated by interpolation of the calibration curves prepared by various concentrations of the isoflavone standards (daidzin, genistin, daidzein and genistein).
2.6. Statistical analysis
All measurements were carried out in three replications. Values were expressed as mean ± standard deviation (SD). Statistical analysis was done by correlation analysis, and one-way analysis of variance (ANOVA) followed by post hoc testing (Duncan's test) was used for statistical analysis at p < 0.05 by using SPSS (Statistical Package for Social Sciences) 21.
3.
Results and discussion
3.1. Characteristics of tempeh with different fermentation conditions
Tempeh production usually involves several fermentation processes, such as lactic acid fermentation during soaking and mold fermentation during incubation of tempeh. Traditionally, the soaking process is usually conducted for 24 hours to allow hydration and lactic acid fermentation, thus decreasing the pH of the soybean from 7 to around 4 [21]. This condition helps to reduce or inhibit the growth of pathogenic and spoilage-causing microorganisms and makes suitable conditions for the growth of tempeh starters [22]. However, natural acidification takes a longer time to decrease the pH. Some modifications in tempeh fermentation were conducted, including adding organic acid and co-inoculation using lactic acid bacteria during soaking and boiling [23]. In this research, lactic acid at three different concentrations (0.8−1.0 %) was added during the cooking of the soybean, incubated at three different temperatures (25−35 ℃) and stored for three different times (2, 3 and 4 days).
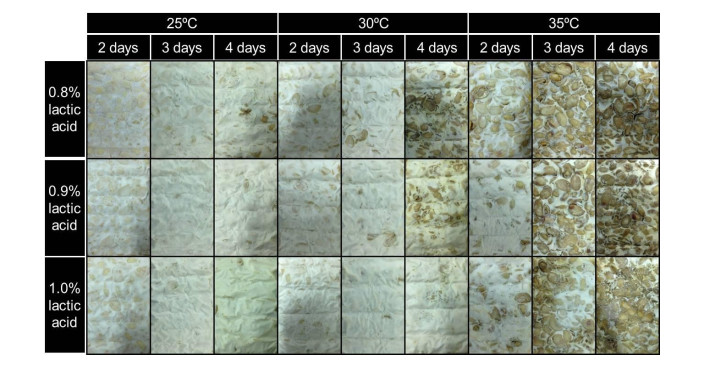
Figure 1 shows the physical appearance of the tempeh under different fermentation parameters. Tempeh fermented at 25 ℃ for 3 days was fully fermented, indicated by white mycelium covering the outer part of the product, while tempeh fermented at 25 ℃ for 2 days showed mold growth, but the soybean was not fully covered. However, tempeh fermented at 35 ℃ for 3 days or more showed overripe conditions. The overripe conditions were also in line with the increase in incubation temperature, where the higher temperature could intensify the fermentation speed. Comparing different acid concentrations, tempeh made by cooking soybean at 0.8% lactic acid solution needs a shorter time to become overripe tempeh than with 1% lactic acid solution. Overripe tempeh also exhibited a slight ammonia smell. Overripe tempeh is tempeh produced over the optimum fermentation time, in which the mold growth is overcome by bacterial growth [24]. It is characterized by producing a pungent odor and a dark appearance [25].
Table 1 shows the results of the color analysis of tempeh. It is shown that there was a significant decrease in the L* value and increases in the a* and b* values of the tempeh stored at longer incubation times at various storage temperatures and with various lactic acid concentrations (p < 0.05). At 35 ℃, the tempeh was significantly darker than tempeh incubated at lower temperatures under all fermentation conditions (p < 0.05). The decrease of lightness is associated with the mold entering the stationery and death phases. In this condition, the mold becomes matured and produces a lower value of proteolytic enzymes responsible for the growth, thus causing the color changes of tempeh from white to darkish-brown [26]. The color values of the fermented soybean developed from light to dark, showing that the mold growth had a noticeable effect on the L* values and chromaticity coordinates (a* and b*). When the ratio of mature mold to immature mold increased, the development of a glimmer began gradually [27].
3.2. Antioxidant capacities of tempeh produced with different fermentation conditions
Table 2 shows the results for the EC50 value of chelating ability, ABTS radical scavenging ability and total phenolic compounds of tempeh with different fermentation conditions. In general, tempeh incubated at higher temperatures and longer incubation times exhibited significantly lower EC50 value (p < 0.05). Compared to the pre-fermented soybean, all tempeh showed a significant decrease in EC50 value (p < 0.05). The ferrous iron chelating ability of tempeh incubated at 25 ℃ showed a decreased EC50 value, ranging from 50.31 to 25.11 mg/mL. Tempeh produced with the 0.8% lactic acid addition and incubated at 30 and 35 ℃ showed a significant decrease in EC50 value (p < 0.05), with ranges of 79.63-22.25 mg/mL and 54.19-10.61 mg/mL for 2-4 days, respectively. A similar occurrence was also observed in tempeh made with 0.9% and 1% lactic acid additions. The higher fermentation temperature and longer incubation time decreased the EC50 value of the ferrous iron chelating ability. This result indicated that the longer fermentation time could induce a higher chelating effect.
Similar results were observed, when tempeh fermented at higher temperatures and longer incubation time showed a lower EC50 value of ABTS scavenging activity (p < 0.05). The significant results of fermented tempeh were also observed compared to the pre-fermented soybean (p < 0.05). The EC50 of tempeh fermented at 25 ℃ and 0.8% lactic acid addition showed a decrease of EC50 over the incubation time up to 4 days, from 58.36 to 34.04 mg/mL. A lower EC50 value was observed for tempeh fermented at 30 ℃ and 35 ℃, where the EC50 value decreased over the incubation time, with ranges of 46.11-28.24 mg/mL and 31.19-17.96 mg/mL, respectively (p < 0.05). Intriguingly, a highly similar pattern was shown in tempeh treated with 0.9% and 1% lactic acid additions (p < 0.05). The findings of this research are in line with the previous findings that revealed the antioxidant activity of tempeh [7,8,9]. It has long been reported that the fermentation process could enhance antioxidant activity. During fermentation, the proteolysis activity of mold increases the bioavailability of several bioactive compounds responsible for antioxidant activity, such as oligopeptides and phenolic compounds [28]. Moreover, a longer fermentation time has been reported to result in a higher antioxidant activity of tempeh than the control [29], and the findings are in accordance with the antioxidant activity of tempeh measured in this study.
It is known that phenolic compounds contribute to the antioxidant activity of tempeh. In this research, there was a significant difference in total phenolic compounds of tempeh fermented at various conditions compared to the pre-fermented soybean (p < 0.05). The results of this research showed that fermentation time contributed to the increase in total phenolic activity of tempeh for up to 4 days. The increment of total phenolic compounds was significant for tempeh incubated at 25 ℃ at different lactic acid concentrations (p < 0.05). A similar condition was observed when the incubation temperature increased. The previous study also reported the highest total phenolic compounds of tempeh incubated for 3 days, but they slightly declined afterward [29]. It was also shown that the tempeh produced with higher lactic acid concentration showed a reduction of total phenolics, probably due to the faster fermentation condition. The increase of phenolic compounds is probably due to the enzymatic activity of mold that cleaved the nutrient compounds and therefore released water-soluble phenolic compounds [29,30].
3.3. Isoflavones profile of tempeh fermented at different conditions
The isoflavones (daidzin, daidzein, genistin and genistein) profile of the tempeh is shown in Table 3. The obtained results showed that there is a significant difference in isoflavones content at different fermentation times (p < 0.05). Fermentation reduced the daidzin and genistin content in all fermentation conditions, while at the same time, the daidzein and genistein contents increased. The most significant reduction in the contents of daidzin and genistin was observed on the fourth day of fermentation. A similar pattern was also observed in the increment of daidzein and genistein. In general, fermentation could increase the total isoflavones content of tempeh when fermented at 30 ℃ and 35 ℃. These results are in line with the previous study that reported the increase of aglycone isoflavones in tempeh compared to the pre-fermented soybean [10,29,31]. It is assumed that higher fermentation temperatures and higher lactic acid concentrations contribute to the faster fermentation time that speeds up the conversion of daidzin and genistin, the most abundant isoflavones, to aglycone forms. During tempeh fermentation, the chemical bond that forms the conjugated isoflavones was broken down by the enzyme beta-glucosidase produced by molds to make a free form of isoflavones (daidzein and genistein) [7,10]. The presence of isoflavones has a strong correlation with the antioxidant activity of tempeh (0.987 at p < 0.001). Previously, the antioxidant activity of isoflavones has been described by several researchers. Research exploring the antioxidant activity of genistein and daidzein isolated from soybean has been reported [32]. Moreover, isoflavones extracted from tempeh have better radical scavenging activity than soybean isoflavones extract [33]. The report mentioned above could support the antioxidant activity exhibited by tempeh isoflavones.
4.
Conclusions
Tempeh obtained from different fermentation conditions results in different physical appearances and antioxidant capacities. The longer fermentation time of up to 4 days and higher incubation temperature up to 35 ℃ changed the appearance of tempeh to be darker, increased the antioxidant activity, increased total phenolic contents, decreased the conjugated isoflavones and increased the aglycone isoflavones of tempeh. Tempeh cooked with 1% lactic acid had the highest chelating capacity, while 0.8% lactic acid-tempeh had the highest total phenolic content and aglycone isoflavones. Therefore, overripe tempeh has higher antioxidant capacity, total phenolic content and total aglycone isoflavones than ordinary tempeh. Moreover, tempeh that is soaked for a longer time to reach a lower pH will have a higher chelating ability.
Acknowledgments
This research was funded by the Research Technology and Transfer Office, Bina Nusantara University, through International Research Grant BINUS University with contract number 017/VR.RTT/III/2021.
Conflict of interest
All authors declare no conflicts of interest in this paper.









 DownLoad:
DownLoad:


