T model of growth and its application in systems of tumor-immunedynamics

1.   Introduction

Rice bran is an agricultural by-product obtained after rice milling process, which accumulates more than 180, 000 cubic tons per year. There are many products from rice bran such as rice bran oil, animal feed, ingredient for cosmetics, etc. Rice bran has high nutritious chemical compositions with protein, lipid, carbohydrate, fiber, and vitamin B as detailed in previous reports ^[1,2]. Including many bioactive compounds as gamma-oryzanol, tocopherol, tocotrienol, adenosine, and ferulic acid which played an important role as a functional food. Moreover, there are many investigations of rice bran with health benefits including blood cholesterol reduction, cardio protective, and anticancer effects, etc. ^[3,4,5]. Consequently, rice bran becomes more interesting agricultural residue for the food and pharmaceutical industry applications.

Lactic acid bacteria (LAB) also known as probiotic that live in digestive systems, such as Lactobacillus and Bifidobacterium. LAB has an important role in benefitting human and animal health that well documented with the effect of gastrointestinal pathogen inhibition, the production of antimicrobial metabolites, the immune response enhancement, the improvement of lactose metabolism and the colon cancer prevention ^[6].

Solid state fermentation (SSF) is a biochemical process of biomass conversion by microorganism on solid material with limited free liquid. SSF has more advantages over submerged fermentation especially with higher fermentation productivity and better product functionalities as bioactivity and bioavailability during the fermentation process. Many SSF applications have been developed the agricultural by products as substrates in order to adding the value to products such as rice bran, sugar cane bagasse, coffee grounds, cassava waste, soybean cake, etc. ^[7].

By the reason of rice bran is an inexpensive which highly available natural source and comprises of many bioactive compounds. This study aims to evaluate the rice bran extract from SSF by LAB with different moisture contents following determine total polysaccharide, total phenolic content, and the antioxidant activity then further investigate the composition of phenolic compounds by HPLC analysis.

2.   Materials and methods

2.1.   Materials

Two rice bran strains for this study were Khao Bahn Nah and Thai jasmine from Prachin Buri province, Thailand. Probiotic strains: L. casei TISTR 1463 and L. plantarum TISTR 1465 were cultivated in Man Rogosa Sharpe medium (MRS) from Himedia (Mumbai, India). All other chemicals used were analytical grade and purchased from Sigma-Aldrich (Saint Luis, USA) and Carlo ERBA (Val de Reuil, France).

2.2.   Rice bran solid state fermentation by lactic acid bacteria

Rice bran was dried in an oven at 105 ℃ for 24 h and prepared 30 g in each flask, then autoclaved at 121 ℃ for 15 minutes. After that, the rice bran was adjusted the moisture content into 50 and 75% w/v by sterilized water. L. casei and L. plantarum were grown at 37 ℃ for 48 h under anaerobic condition in MRS broth and determined the optical cell density with a spectrophotometer at 600 nm, then equal to 1.0 (previously estimated about 10⁸ CFU/mL by Trabelsi et al. ^[8]) for applying as an inoculum. LAB was separately inoculated by adding into the prepared rice bran in a ratio of 10% w/v then subjected to incubation at 37 ℃ for 72 h. Five grams of each sample was taken every 24 h then extracted by adding 50 mL of 70% v/v ethanol further incubated in a shaker at room temperature, 200 ×g for 24 h. All extracted samples were centrifuged at 16, 000 ×g for 20 minutes at room temperature, after that evaporated by a rotary evaporator at 50 ℃, 100 ×g, then stored the samples at 4 ℃ for further determination with triplicate analysis.

2.3.   Total polysaccharide determination

The total polysaccharide content of the rice bran extracts were determined by the phenol sulfuric acid according Dubois method ^[9]. Prepared an appropriate diluted sample 0.25 mL in a test tube, then immediately added 1.25 mL of concentrated sulfuric acid (95% v/v) and 0.25 mL of 5% v/v phenol. A mixture was heated at 90 ℃ for 5 minutes then cooled until it reached to room temperature. A colorimetric of rice bran sample performed the total polysaccharide content by monitoring an absorbance at 490 nm. The standard and blank were prepared in the same way as the analyzed sample, except for adding 0.25 mL glucose standard and 0.25 mL distilled water instead of the sample, respectively.

2.4.   Total phenolic content determination

The rice bran extracts were determined total phenolic compound by Folin-Ciocalteu assay from modified method of Yee et al. ^[10]. After the extraction process, an aliquot (0.04 mL) of extracted rice bran was mixed with 0.2 mL Folin-Ciocalteu reagent and 0.26 mL distilled water, left in a room temperature for 8 minutes. After that 0.6 M sodium bicarbonate (0.5 mL) was added into the reaction mixture and incubated in a dark room temperature for 2 h, then determined the absorbance at 725 nm. Gallic acid was prepared and analyzed in the same way as the rice bran extracts which was used as a standard for phenolic content calculation.

2.5.   Antioxidant activity determination

The antioxidant activity was examined by DPPH assay, which described from the previous protocol ^[11]. The rice bran extract (0.12 mL) was mixed with freshly prepared 0.5 mM DPPH reagent in methanol (1.08 mL), then left in a darkness room for 30 minutes and measured the absorbance at 517 nm. DPPH in methanol was used as a blank for calculation the percentage of antioxidant activity (% DPPH inhibition) by following equation:

2.6.   High performance liquid chromatography (HPLC)

The quantifying composition of the rice bran extracts were analyzed by HPLC that is described elsewhere in Brum et al. ^[12]. HPLC system (Thermo Fisher Scientific, USA) coupled UV/visible detector were used with a column of Thermo Hypersil Gold (4.6 mm × 150 mm, 3 µm) at 40 ℃ with 2% v/v of acetic acid in methanol solution as a mobile phase at a constant flow rate of 0.7 mL/min. The samples were analyzed with differential refractometer. All standard compounds as tocopherol, gamma-oryzanol, coumaric acid, and ferulic acid were used for peak identification.

2.7.   Statistical analysis

One-way Analysis of Variance (ANOVA) test was performed with SPSS program for Windows, V.21 (SPSS, Chicago, IL, USA). Differences with a probability value of < 0.05 were considered significant and all data were reported as mean ± SD.

3.   Results and discussion

After the rice brans were SSF by different LAB and moisture contents, the fermented samples were collected and extracted by ethanol, then stored at 4 ℃ for further analytical studies as shown below.

3.1.   Total polysaccharide determination

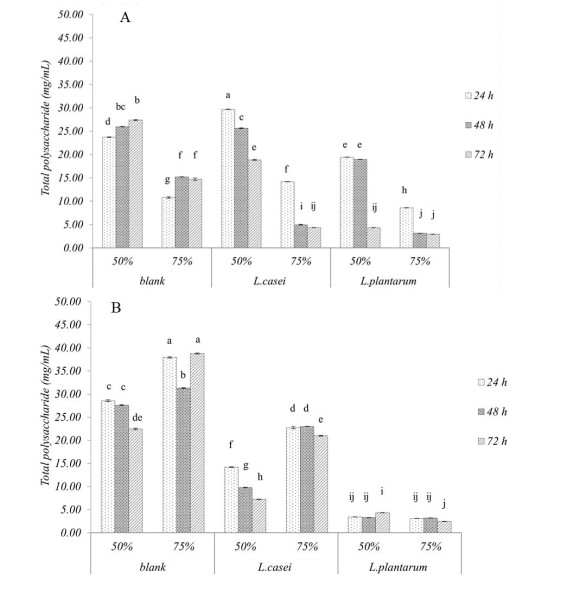
The total polysaccharide of the rice bran extracts from SSF by different LAB and moisture contents showed in Fig. 1. In the condition without SSF (blank) presented a high total polysaccharide along the fermentation period when compared with the rice bran extracts from SSF by both LAB significantly (p < 0.05). Thai jasmine without SSF at 75% w/v moisture exhibited the highest total polysaccharide as 38.77 mg/mL after 72 h, while the rice bran extracts with SSF showed a lower total polysaccharide with a longer incubation period. The rice bran extracts from Khao Bahn Nah with SSF by L. casei at 50% w/v moisture (Figure 1A) presented a high total polysaccharide as 29.69 mg/mL at 24 h then total polysaccharide decreased after 48 and 72 h (25.63 and 18.85 mg/mL), which has the same tendency as the rice bran extracts from Thai jasmine with SSF by both LAB (Figure 1B). Noticeably, both strains of rice extracts by L. plantarum displayed a lower total polysaccharide than L. casei. The lowest total polysaccharide was in the condition of Thai jasmine with L. plantarum at 75% w/v moisture after 72 h (2.44 mg/mL). The reduction of total polysaccharide along the SSF period can be explained that LAB was using carbohydrate during the fermentation for their growth lead to lower total polysaccharide in the rice extract samples with SSF. These results are consistent with those of Saman et al. ^[13] who found the reducing sugar was decreased after 48 hours when inoculated L. plantarum NCIMB8826 with whole grain brown rice and rice bran, while L. plantarum grew well and reached to a maximum value at 30 h.

Figure 1.  Total polysaccharide (mg/mL) of the rice bran extracts with different moisture contents, LAB, and incubation times of (A) Khao Bahn Nah and (B) Thai jasmine. Error bars illustrate the standard deviation (±SD) among the replications. Data points indicated with different letters are significantly different from each other at p < 0.05.

DownLoad: Full-Size Img PowerPoint

3.2.   Total phenolic content determination

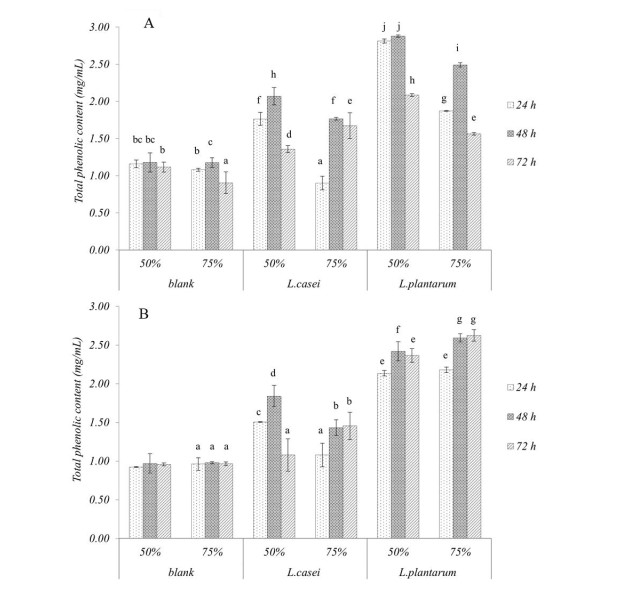
The rice bran extracts from SSF by both LAB with different moisture contents (Figure 2) displayed a significant increase amount of phenolic content when compared with both strains of the rice bran extracts without SSF (p < 0.05). Khao Bahn Nah extract with SSF by L. plantarum (Figure 2A) at 50% w/v moisture for 48 hours presented the highest total phenolic content (2.88 mg/mL). Observable, the rice bran extracts of all SSF conditions showed higher total phenolic content at 48 hours of incubation than the other periods. This may explain by the maximum LAB growth at 48 hours from the previous report of Nisa et al. ^[14] that also discovered the maximum amount of total phenolic content at 48 h as well in the fermented rice bran with L. plantarum (FNCC 0027) and L. lactic (FNCC 0080). Furthermore, SSF technique has successfully performed to increase phenolic compounds by microorganism fermented rice bran with Rhizopus oryzae (CT1217) which provided a high quality of rice bran extract and biological activity compared to unfermented rice bran following the description by Schmidt et al. ^[15].

Figure 2.  Total phenolic content (mg/mL) of the rice bran extracts with different moisture contents, LAB, and incubation times of (A) Khao Bahn Nah and (B) Thai jasmine. Error bars illustrate the standard deviation (±SD) among the replications. Data points indicated with different letters are significantly different from each other at p < 0.05.

DownLoad: Full-Size Img PowerPoint

3.3.   Antioxidant activity determination

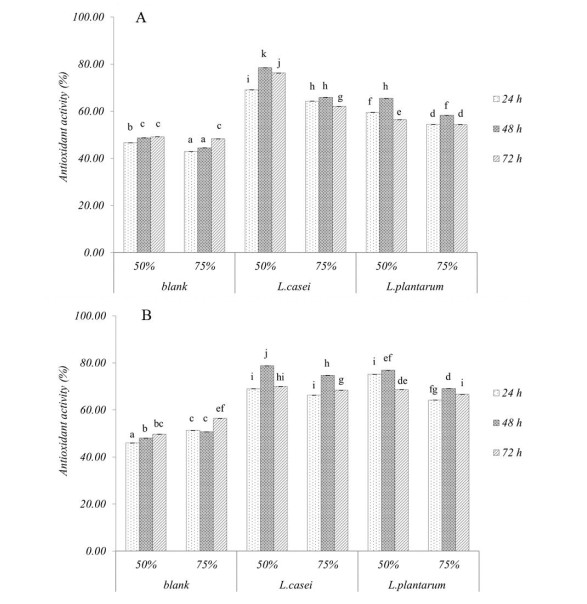
The antioxidant activity results in Figure 3 exposed both strains of rice bran extracts without SSF (blank) had a significant lower antioxidant activity (about 45%-56%) than the rice bran extracts with SSF (p > 0.05). Whereas the rice bran extracts from Thai jasmine and Khao Bahn Nah with SSF by L. casei at 50% w/v moisture for 48 hours displayed the highest percentage of antioxidant activities as 78.79% and 78.49%. A similar study was conducted from Zubaidah et al. ^[16] that investigated the antioxidant activity from the fermented rice bran and skim milk by L. plantarum J2 and L. casei. From the results, the rice bran extracts from the fermentation by LAB displayed a high antioxidant activity of 88.86%, while the antioxidant activity from fermented skim milk was at only 30.13%. This could be interpreted as during the SSF process, biocatalyst from microorganism would cleave the bonds between phenolic compounds and other substances then subjected to enhance the antioxidant activity. As in the experiment of Cheng et al. ^[17] which inspected the high value of total phenolic content, total flavonoid content conversion, and their antioxidant activity during the fermentation process of the rice bran with Monascus pilosus KCCM60084.

Figure 3.  Antioxidant activity (%) of the rice bran extracts with different moisture contents, LAB, and incubation times of (A) Khao Bahn Nah and (B) Thai jasmine. Error bars illustrate the standard deviation (±SD) among the replications. Data points indicated with different letters are significantly different from each other at p < 0.05.

DownLoad: Full-Size Img PowerPoint

In this study, the fermented rice bran extracts by both LAB disclosed lower total polysaccharide, even though the phenolic content and antioxidant activity revealed higher value than rice bran extracts without SSF. These results may differ from some research reports ^[18,19] that described the polysaccharide from natural sources considering the antioxidant effectiveness. By the way, there is a possibility of no correlation between the increasing of polysaccharide content and the high performance of antioxidant activity, since LAB growth is one of the main factors affecting the reduction of polysaccharide concentration. Besides, the polysaccharide is subjected to transform into other bioactive compounds along the SSF process and contribute to the improvement of antioxidant properties as observed by Magro et al. ^[20] and Ayyash et al. ^[21].

3.4.   HPLC

According to the analytical results of this study, the rice bran extracts with 50% w/v moisture at 48 hours presented high phenolic content along with very effective antioxidant activity. Under these conditions, six rice bran extract samples were selected for HPLC analysis: A = Khao Bahn Nah without SSF (blank), B = Khao Bahn Nah with SSF by L. casei, C = Khao Bahn Nah with SSF by L. plantarum, D = Thai jasmine without SSF (blank), E = Thai jasmine with SSF by L. casei, and F = Thai jasmine with SSF by L. plantarum. The standard compounds as tocopherol, gamma-oryzanol, coumaric acid, and ferulic acid, were used for peak identification in order to compare the quantity of the rice bran extract samples. From Table 1 tocopherol, gamma-oryzanol, and ferulic acid were found in all rice bran extract samples in their compositions. Only in the sample E displayed coumaric acid at 14.47 mg/L with high level of ferulic acid (35.23 mg/L). Increasing of ferulic acid during the fermentation had been investigated in the previous research of Huang and Lai ^[22] that analyzed the bioactive compounds from four strains of Taiwan rice bran and two strains of Thai rice bran, after the fermentation process, all rice brans demonstrated high concentration of ferulic acid. As well to that documented by Rashid et al. ^[23] who evaluated the composition of bioactive compounds which revealed many phenolic compounds and organic acids (such as alpha-tocopherol, gamma-oryzanol, ferulic acid, ascorbic acid, etc.) of the fermented rice bran with LAB by HPLC analysis.

Table 1.  HPLC analysis of the phenolic compounds (mg/L) in the rice bran extract samples with 50% w/v moisture at 48 h.

Sample	Tocopherol	Gamma-oryzanol	Coumaric acid	Ferulic acid
A	3.69 ± 0.29c	1.55 ± 0.74c	ND	18.91 ± 0.60d
B	8.75 ± 1.11a	2.57 ± 0.56ab	ND	30.93 ± 0.81b
C	4.09 ± 0.17c	1.44 ± 0.36c	ND	19.39 ± 0.56d
D	3.35 ± 0.97c	1.69 ± 0.35ab	ND	18.86 ± 1.05d
E	4.51 ± 0.38c	3.16 ± 0.15a	14.47 ± 1.20	35.23 ± 0.82a
F	7.10 ± 0.23b	2.31 ± 0.65abc	ND	21.61 ± 0.66c
ND = not detected. Data are expressed as mean ± SD and the values in the same column with different letters are significantly different at p < 0.05.

---

 | Show Table

DownLoad: CSV

4.   Conclusions

From this study, two rice bran strains (Khao Bahn Nah and Thai jasmine) were SSF by LAB (L. casei and L. plantarum) with different moisture contents (50% and 75% w/v) for 72 h. The rice bran extracts were evaluated total polysaccharide, total phenolic content, antioxidant activity, and their composition by HPLC analysis. Total polysaccharide in the fermented rice bran extract was lower than those without SSF since LAB growth during the fermentation causes the polysaccharide decreased in the fermented rice bran extract. However, considering of phenolic content and antioxidant activity, all fermented rice bran extracts exposed with higher antioxidant property aspect than the samples without SSF. Specifically, the fermented Khao Bahn Nah extract by L. plantarum with 50% w/v moisture demonstrated a great amount of total phenolic content after 48 h. As the same SSF incubation time and moisture content, both fermented rice bran extract strains by L. casei performed high efficiency of antioxidant activity. Tocopherol, gamma-oryzanol, and ferulic acid were found in all rice bran extract samples, mostly in the fermented Thai jasmine extract by L. casei with 50% w/v moisture expressed with high quantity of coumaric acid, and ferulic acid compared with the other rice bran extract samples. Overall, the results from this study indicate that the rice bran extract from SSF by LAB can establish the varieties of phenolic compounds and enhance the potential of antioxidant activity which could be applied further as functional food products and value added to the agricultural residues in Thailand.

Acknowledgments

The authors would like to thank Miss Duangkamon Sangiamdee from the Chemistry Department, Ramkhamhaeng University for HPLC analysis. Financial support from Ramkhamhaeng University is gratefully acknowledged.

Conflict of interest

The authors declare no conflict of interest.