1. Introduction
Since the introduction of the German Renewable Energy Act (EEG) in 2004, Germany has experienced a considerable increase in biogas production using renewable raw materials. A significant share of the electricity in the German energy mix is now produced in nearly 8, 000 biogas plants with an annual electricity generation of nearly 25 TWh [1]. The fact that renewable raw materials of plant origin make up more than 50% of the substrate mix [2] causes the important factors involved in biogas production to face a number of ecological challenges [3,4,5,6,7,8,9]. In regions with a high availability of biogas, water protection is one of these challenges. The production of biogas from agricultural activities results in large amounts of spatially concentrated nutrients, especially nitrogen[10], which are primarily spread over surrounding agricultural areas.
In order to meet the aim of reducing nutrient contamination caused by agriculture, central environmental management policies exist in EU member states. In Germany, this is the German Fertilizer Ordinance (DüV) [11]. The DüV focuses on implementing the European Nitrates Directive 91/676/EEC (ND) [12] into German law. It defines the “good professional practice” of fertilization and aims to use this approach towards the achievement of various environmental targets. Furthermore, it regulates factors such as the maximum application amounts of nitrogen from organic fertilizers in order to avoid the excessive pollution of groundwater and surface water. The limit stipulated is currently 170 kg of organic nitrogen per hectare of agricultural land. In exceptional cases, the limit may be higher if higher nitrogen requirements for intensively used grassland and agricultural grass areas can be verified. The upper limit for nitrogen spreading currently exclusively refers to manure of animal origin. Digestates 1 (1 Digestate and manure are used as synonyms in the following, as there are no differences in the transportation, procurement and remuneration costs.) of plant origin from the biogas production outlined above have therefore not yet been considered. At the time of the most recent adoption of these regulations, biogas production did not play an important role in these terms. Its rapid development was clearly not sufficiently anticipated with regard to environmental law, and, as a result, there is now a gap in the regulations. Digestates of plant origin could therefore theoretically be spread to an unlimited extent without penalties. They are often spread in close proximity to biogas plants due to their relatively high transport costs, even if the cultivated crops do not have corresponding higher nutrient requirements. Although these actions taken by a multitude of biogas producers do not conform to the principles of “good professional fertilization practice”, they occur frequently. Such actions therefore represent a typical negative externality that needs a corresponding regulation. Nevertheless, all biogas plants have one thing in common, namely, the fact that they are preferentially constructed in regions with high manure production because of intensive livestock farming. The good fermentation properties of the admixture of manure, its good substrate properties and the favorable availability of manure [13,14,15,16,17,18] have led to this regional focus on biogas production. The pricing of electricity generated from biogas in the EEG, which is oriented towards the use of manure, also forced this development. Biogas plants that were put into operation in or before 2012 were able to receive a significant additional reward for a mass use of slurry of at least 30% [19]. As a result, biogas plants were preferentially constructed in livestock-intensive areas in the north-west of Germany, namely in the German Federal States of Lower Saxony and North Rhine-Westphalia. Table 1 illustrates the additional nutrients from digestates of plant origin that are produced in strong spatial concentrations, due to the considerable size of biogas plants and their spreading on the surrounding agricultural land.
Table 1. Total amount of nitrogen resulting from biogas production and excessive amounts of nitrogen, including nitrogen from animal production, according to the federal states of Germany and Germany as a whole in 2011.
| Nitrogen of digestates of plant origin resulting from biogas production | Excess nitrogen from digestates of plant origin at the municipal level | Excess nitrogen of animal origin and digestates of plant origin at the municipal level | Minimum area required for the legal application of surplus nitrogen (animal origin + digestate of plant origin) at 170 kg N/ha | Utilized agricultural area of the individual federal states resp. of Germany (UAA) | Share of minimum area required of the total UAA for the application of excessive quantities |
| column | I | II | III | IV | V | VI |
| kg N | kg N | kg N | ha | ha | % |
| Lower Saxony | 62, 252, 479 | 14, 115, 171 | 22, 841, 130 | 134, 360 | 2, 548, 047 | 5.3 |
| North Rhine-Westphalia | 21, 247, 467 | 4, 891, 126 | 6, 694, 284 | 39, 378 | 1, 449, 860 | 2.7 |
| Germany total | 240, 159, 633 | 36, 813, 597 | 50, 756, 022 | 298, 565 | 16, 667, 300 | 1.8 |
| Source: own calculations, based on Federal Bureau of Statistics and Transmission System Operator. |
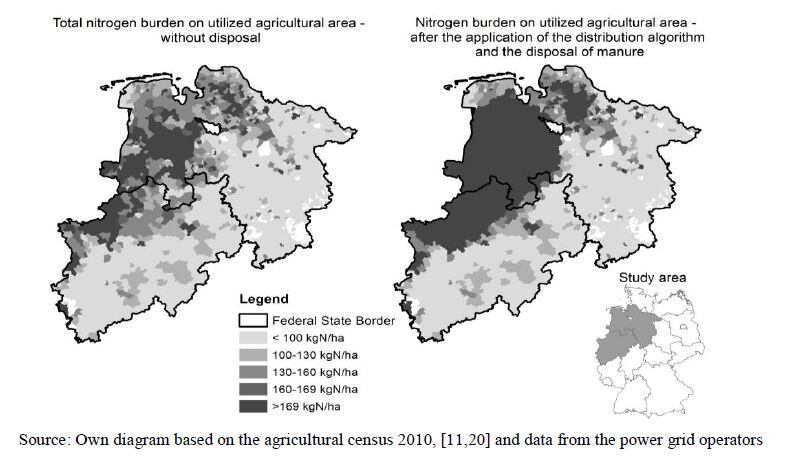
This table shows that approx. 35% of the nitrogen produced from digestates of plant origin that additionally need to be transported are produced in the two federal states specified above (column I), but these states only represent approx. 24% of utilized agricultural land (UAA) in Germany (column V). There is, however, another spatial concentration within the federal states. This can be illustrated on a local level by the nitrogen spreading limit of 170 kg N per hectare presented in Figure 2 (left map) 2 (2 The nitrogen produced from animal manure was calculated and recorded based on the coefficients of nitrogen excretion (appendix 5 DüV; [20]) and the different chargeable nitrogen losses in the stall and during storage depending on the type of farming (appendix 6 DüV). In the case of cattle farming, the assignment of animal excretions to slurry/solid manure systems and grazing is based on sample testing of the farming system and/or type of housing carried out within the framework of the UAA. For other species, the calculation is based on [21]. In the case of pig and poultry farming, an equal apportionment between standard feed and feeding methods with reduced N/P was assumed in accordance with [27].). According to this limit, every hectare of UAA in a municipality contains 170 kg of nitrogen from digestates of plant origin and from manure of animal origin from the municipality in question. In many municipalities, these results in excessive quantities that need to be disposed of and must therefore be spread on land located outside of the regions with excessive quantities. If excessive quantities of these municipalities are aggregated on a state level (column III), the problem of insufficient equal distribution of nitrogen becomes very clear. For ecological reasons, this leads to a need to determine the minimum areas outside of municipalities additionally required to transport these excessive quantities of nitrogen. In Lower Saxony, this additional area already represents more than 5% of the entire UAA in the federal state (column 6 as a quotient of columns IV and V). It also becomes clear that the additional digestates of plant origin in particular contribute towards a massive increase in the total amount of excessive nitrogen. Biogas production therefore leads to a significant increase in transport pressure on nutrients in these regions, which are defined as the study area below, in order to exemplify regional and enterprise specific impacts of the amendment of the German Fertilizer Ordinance.
At present, draft regulations are being discussed in order to also adequately factor in digestates of plant origin. This discussion aims to explore this matter from the perspective of the upper limit for nitrogen spreading of 170 kg per hectare and to highlight the matter on an economic level from both spatial and enterprise perspectives. In addition to answering the question of how much the disposal costs of manure and digestate substrates will increase with new regulation, we will test the following hypothesis:
· Inclusion of manure of plant origin in the calculation of the upper limit for nitrogen spreading will lead to differing competitiveness among biogas producers and pig farms in terms of manure disposal costs.
Thus, this study aims to quantify impacts at the regional and farm level. A spatial model approach is integrated to estimate spatial distribution, transport amounts of manure as well as transport distances with respect to an amended DüV. The second part of the analysis addresses the motivation of enterprises to pay higher land fees as a result of increased manure export costs. As far as we know, such surveys do not yet exist, although a large number of research projects also explore the economic implications of biogas production (see [22,23,24,25], for example). This analysis should also provide evidence for other regions that may have increased biogas production in the future in order to avoid negative ecological and economic impacts.
2. Research background, scope of study and methods
2.1. Research background and scope of study
The diagrams and calculations in this study are based on the necessary and sensible integration of digestates of plant origin into the upper limit for nitrogen spreading of 170 kg per hectare, which, to date, has only applied to manure of animal origin.
The focus of the following study solely concerns the upper limit for the spreading of nitrogen produced from manure, although phosphorus (P) can also represent an ecologically and economically important factor for the spreading of manure, especially in livestock farming regions in Germany. Nevertheless, at present, it is a change in the minimum percent of excretions of total nitrogen for pig manure that is being discussed. This requirement would result in the upper limit for nitrogen spreading having maximum authority in the future with regard to maximum manure application rates. The focus on the nutrient of nitrogen chosen in this study therefore seems to be suitable. Furthermore, an additional focus on P would ultimately lead to similar general economic results. A further limitation of the use of P would increase disposal costs and thus land costs as well. Nevertheless, the regional impact would, in some cases, be different in comparison with nitrogen.
The emphasis on economics is placed on the regions of north-west Germany that are characterized by a high occurrence of manure as a result of intensive animal husbandry and/or biogas production. The economic impacts presented below mainly concern farms that rely on the disposal of manure to other farms. Economic impacts often involve farms that have strongly grown in the areas of animal husbandry and/or biogas production in the past. In the case of increasing purchase and lease prices of agricultural land, however, they also refer to farms in the observed regions that are able to secure the disposal of manure on the land that they cultivate themselves. Within this context, the study focuses on the federal states of Lower Saxony and North Rhine-Westphalia (study area). These regions are characterized by an extremely high concentration of livestock [26,27]. In addition, our own calculations based on regional data from the German Energy Agency [28] and the four power grid operators 3 (3 Transmission system operators are obliged to publish details on the location and installed power of the biogas plants connected to their grids in accordance with §48 EEG. The data used in this study are based on biogas plants operated in accordance with the EEG up to 31.12.2012.) reveal that a considerable occurrence of manure from digestates 4 (4 A nitrogen quantity of 76.7 kg in digestates of plant origin was used for each 1 kW of installed electrical power. 313 kg of nitrogen in digestates of plant origin was used as a basis for each 1 m3/h of bio-methane injection capacity of bio-methane plants. Each of these processes involved a process loss of 10%, which can be seen as normal on an international level [4,6].) of plant origin can also be recorded in these livestock-rich regions. Exporting excess manure to regions that still have free capacities is conceivable as a possible adaptation strategy. Therefore, the economic impacts of an amended DüV are focused on manure disposal costs to receptive farms, which are mostly located outside nutrient-burdened areas. Thus, in the case of leasing land nearby the biogas facility, the economic advantages of lower transport distances (e.g., silage maize transport) are not considered. In this context, however, it should be assumed that the disposal costs for liquid manure, which is characterized by its limited transportability, are significantly greater. This development would also subsequently have an impact on farms that do not currently use any manure of plant origin themselves but do depend on the use of manure across farms. This particularly concerns pig farmers in the regions observed. In the case of increasing the lease and purchase prices of agricultural land, this development would also affect farms that are able to guarantee manure is fully used on their own land. For this reason, the economic impact of increasing manure transport costs on biogas production and pig farming in north-west Germany are the main focuses of observation within the context of the structural developments.
2.2. Methods
2.2.1. Model for the quantification of transported amounts of manure and their transport distances
In order to quantify transported amounts of manure and transport distances of an amended DüV, spatial data on nitrogen production from livestock farming [21] and biogas production were analyzed within the study area. With this type of modelling, the additional and average transportation cost due to an amended DüV could be derived. These costs are the basis for the disposal costs per region and per farm.
The total status quo nitrogen production at the municipality level was referred to the UAA and mapped. Furthermore, a distribution algorithm was developed, enabling the distribution of nitrogen amounts between municipalities by using nearest neighbor relationships based on linear distances. The algorithm first checks if a municipality is above the 170 kg per hectare limit, identifying the municipality with the highest nitrogen burden as well as its nearest neighbor. The amount of transferable nitrogen is calculated depending on the nearest neighbor’s nitrogen burden, which has to be below the 170 kg per hectare limit. Otherwise, the second nearest neighbor is identified. Finally, nitrogen is virtually transported until the issuing municipality is below or the receiving municipality is at the 170 kg per hectare limit. The algorithm stops if no municipality is above the stated limit (see Figure 1) 5 (5 A limitation of this normative approach has to be considered, that real distribution differs from our results because of farmers‘ land tenure in different municipalities.).
2.2.2. Survey of manure brokerage services in north-west Germany
In order to estimate the economic impacts of the increasing costs of disposing manure between farms from a practical point of view, 6 experts were consulted. The surveyed experts were employees of institutions that broker manure between farms within the study area. Given the low number of such manure brokerage services in existence, this survey can indeed be considered to be representative of the target area. The aim of this survey was to gain a comprehensive overview of the current situation of manure transport and of future developments in the ‘nutrient-intensive’regions of North Rhine-Westphalia and Lower Saxony. The experts were therefore asked to specify the minimum, maximum and a weighted average of the disposal costs per cubic meter (m3) that the farmers had to pay in the year 2013 as well as the total turnover of m3 within their institution 6 (6 Prices for manure disposal differ over the year. In spring when fertilizer demand is high prices are lower by trend. We focused within this study on the weighted average value in order to discuss general trends in price developments within the context of the amendment of the German Fertilizer Ordinance.). The disposal costs per cubic meter must be paid to the broker of the manure by the supplier. They include the transport costs incurred when collecting liquid manure (e.g., slurry) from the supplier's farm, the procurement fee paid to the broker of the manure and the remuneration for the farm accepting the manure. These parts of the total disposal costs were asked as one single question. The buyer's own costs are not incurred in this scenario. The spreading costs are normally paid by the recipient. The survey participants were also asked to estimate the level that the corresponding figures in their area of responsibility would, in their opinion, reach if digestates of plant origin from biogas production had to be included in the future.
2.2.3. Full cost modelling of biogas production and pig farming
Full cost pricing of pig fattening, pig breeding and biogas production was used to make the calculations required for affordable manure disposal costs and changes in willingness to pay for agricultural land. The calculation bases used for the production processes involved in pig farming are based on [29] with regard to investment costs, and on regional and national statistics according to[30,31,32] with regard to biological performance and other process figures. The calculation of biogas production is based on [33,34,35] with regard to investment costs and process figures. The calculation of the payable disposal costs of the production process of biogas was determined on the basis of a biogas plant that uses renewable raw materials, is subject to the general conditions of the EEG 2009 and uses 70% maize silage and 30% slurry as substrates. This aims to accommodate the frequent occurrence of this biogas plant category in the regions observed. Table 2 shows the most important figures of modeled production processes. In accordance with national statistics, it distinguishes between an average and above-average (25% ‘best’) performance level in pig fattening, pig breeding and biogas production. A wage projection of 15 €/h and a required rate of return of 4% were assumed for the full-cost accounting.
Table 2. Assumed production figures of the production processes.
| Pig fattening | | Ø | 25% | Pig breeding | | | Ø | 25% |
| Investment costs | €/FP* | 420 | 400 | Investment costs | | €/BS*** | 3, 540 | 3, 340 |
| Fattening pig start weight | kg | 28 | 28 | Number of litters per year | | Units/BS*** | 2.31 | 2.35 |
| Fattening pig end weight | kg | 120 | 120 | Piglet loss total | | % | 14.7 | 13.1 |
| Feed conversion ratio | | 1:2.85 | 1:2.74 | Piglets sold | | Units/BS*** | 25.9 | 27.9 |
| Daily weight gain | g/day | 802 | 826 | Feed consumption | | 100 kg/BS*** | 23.7 | 23.7 |
| Profit quotation | c/kg SW** | 0 | +2 | Stock replacement rate | | % | 40 | 40 |
| Losses | % | 2.7 | 2.0 | Veterinary expenses | | €/BS*** | 166 | 144 |
| Working hours required | h/FP* | 0.85 | 0.70 | Working hours required | | h/BS*** | 17 | 14 |
| Manure | m3/FP* | 2.0 | 2.0 | Manure | | m3/BS*** | 6.6 | 6.6 |
| Biogas production | | Ø | 25% | Biogas production | | | Ø | 25% |
| Investment costs | €/kWel | 4, 402 | 4, 302 | Electricity sales | | million kWhel | 4.0 | 4.0 |
| Substrate requirement | % | 100 | 95 | Revenue from electricity sales | | €/kWel | 1, 690 | 1, 706 |
| Electricity revenue per kWhel | c/kWhel | 21.1 | 21.3 | Revenue from heat sales | | €/kWel | 68 | 140 |
| Heat revenue per kWhth | c/kWhth | 2.0 | 3.5 | Total costs**** | | €/kWel | 904 | 883 |
| Working hours required | €/kWel | 4.0 | 3.2 | Digestate | | m3/kWel | 21 | 21 |
| *FP = fattening place; **SW= slaughter weight; ***BS= breeding sow; ****without substrate costs and manure transport costs; all price and cost assumptions in this table are net prices. Source: own diagram based on [29,32,34]. |
2.2.4. Monte Carlo simulation for the depiction of the distribution of disposal costs
Static full-cost calculations are disadvantageous in that they only show one possible result without factoring in uncertainty from the behavior of certain price and cost assumptions (e.g., fattened pig prices, feed prices and substrate prices). A Monte Carlo simulation was therefore integrated into the full-cost calculation as a risk analysis tool [36,37], especially to depict the change in competitiveness between biogas producers and pig farmers. Such simulations have already been used in economic evaluations of renewable energies and/or animal production processes on many occasions [38,39,40]. The first stage of this simulation was to define a decision model that contributes to the identification of stochastic factors that are important for the target value (= input variables). In the case of the production process of pig fattening (PF), these factors are the slaughter pig price, the piglet price and the feed price. In the case of the production process of pig breeding (PB), the piglet price and feed price were considered to be important influencing factors [39] and for the production process of biogas production, the important factor was the substrate price for maize silage 7 (7 In the case of slurry, the assumption was made that no costs are involved in the provision of liquid manure to operators of biogas plants in the relevant regions of north-west Germany.) up to the fermenter. The Kolmogorov-Smirnov test (see Appendix II) and graphical analyses of the histograms were then used to define the best possible probability density functions of the risky variables, which were assumed to be normal distributions (see Table 3).
Table 3. Capped normal distributions of risk factors.
| Risk Factor | Unit | μ | Ϭ | Min. | Max. |
| Basic piglet price | €/piglet | 44.52 | 7.93 | 30.50 | 58.00 |
| Slaughter pig price | €/kg slaughter weight | 1.52 | 0.16 | 1.12 | 1.03 |
| Feed price for pig fattening | €/100 kg | 26.19 | 4.46 | 18.61 | 33.74 |
| Feed price for pig breeding | €/100 kg | 28.91 | 3.88 | 21.64 | 36.18 |
| Maize silage price up to fermenter | €/100 kg fresh weight | 42.03 | 8.79 | 27.32 | 55.75 |
All of the prices listed in the table are net prices.
Source: own calculations based on [41,42,43]. | | | | | |
This involved the consultation of historical price data series 8 (8 The period from January 2007 to April 2014 was selected as the period of observation.) from the past as samples, namely the VEZG 9 (9 German producer union of cattle and meat) slaughter pig quote for the north-west slaughter pig price, the north-west quotation for piglets [41] for the piglet prices and the monthly price fixings of the animal feed prices 10 (10 Study area price information was used, but due to breaks in the selected time period, was adjusted by Bavarian adopted price information (LFL), which was available for the entire time period.) [42,43]. Given that silage maize is not a cash crop for which reliable price data series from the past are available, the following methodology was used to define the density function of these variables: first, the assumption was made that silage maize and winter wheat have a comparable profit margin as competing field crops. In consideration of additional process-specific calculation bases [31], this assumption can be used to determine a corresponding indifference price for silage maize ex field. In consideration of harvest and transport costs of 7 €/100kg and ensilage and storage losses of 12%[34], this price can be used to deduce the price of maize silage up to the fermenter. Historical price data series for wheat were then used as a basis for the determination of the best possible density function for maize silage up to the fermenter. On the basis of this approach, a normally distributed density function was assumed for all risk factors, and the ends of this function were capped according to the observed min/max values of the price data series.
The input variables were then simulated and transferred to the target function via a pseudo-random number generator in Microsoft Excel and in consideration of the distribution functions of the risk factors observed. This enabled a multitude of calculation procedures (number: 10, 000; [36,37]) to be used to determine the probability distribution of the target function. The correlations between the individual variables had to be factored into these procedures. If the prices for piglets and slaughter pigs were independently simulated, it is conceivable that the result would show that, for example, extremely high slaughter pig prices involve extremely low piglet prices. This is not realistic because high slaughter pig prices tend to increase the demand for piglets and thus cause the price of piglets to increase [39]. Table 4 shows the determined correlation matrix.
Table 4. Correlation matrix for the period January 2007 to April 2014.
| Piglet price | Pig price | Feed price for PF* | Feed price for PB** | Maize silage price |
| Piglet price | 1.00 | 0.53 | 0.30 | 0.28 | -0.09 |
| Pig price | | 1.00 | 0.65 | 0.68 | 0.34 |
| Feed price for PF* | | | 1.00 | 0.96 | 0.79 |
| Feed price for PB** | | | | 1.00 | 0.83 |
| Maize silage price | | | | | 1.00 |
*PF = pig fattening, **PB= pig breeding
Source: own calculations based on [41,42,43]. |
3. Results
The following section presents the results of the study. A distinction must be drawn between the results in terms of the study area (3.1 and 3.2) as well as the results on the farm or biogas plant levels (3.3 and 3.4).
3.1. Spatial impacts of an amended DüV within the study area in terms of shipped amounts of nitrogen and estimated transport distances as well as estimated transport costs
Within the study area, total nitrogen burden (animal and biogas plant origin) ranges in between 1 and 620kgN per hectareUAA (Average 99) on municipal area. 192 of 1373 considered municipalities show more than the 170 kg per hectareN limit (see Figure 2, left map), whereas in the case of exclusively considering nitrogen from animal origin, 73 municipalities violate the stated limit (not shown). Mapping the nitrogen pressure on a municipality level shows that spatial distribution is heterogeneous within the study area. Very high nitrogen concentrations are detectable in the western part of the study area. Those with very low concentrations are in the eastern part, which allows the disposal of manure.
Applying the distribution algorithm to the study area results in a maximum of 170 kg N per hectare UAA, if linear distances of each municipality are calculated within a 100 km radius. The area covered with maximum manure content per ha is ca. 240 km in north-south direction and 150 km in west-east direction and represents 419 municipalities out of 1,373 (see Figure 2, right map).
In total, 29.6 Mio kg N are shipped. Assuming average nitrogen content of ca. 4 kg N m−3 manure (see Table 5), 7.4 Mio m3 manure have to be transported per year. Considering linear transport distances, the total transport amount equals 223.7 Mio m3 km. With average transport costs of 0.07 Euro m−3 km (truck transport 11 (11 Assumption: Costs for the truck including fuel and driver: 100 Euro/h, transport capacity 25 m3, transport distance: 60 km/h)), overall transport costs result in 14.9 Mio Euro per year or 2 Euro m−3. Manure transport costs equal 1.1 Euro m−3 on average or 2.9 Mio Euro per year in total if only nitrogen from animal origin is distributed with the developed algorithm. Hence, the increase in transportation costs is 82% in comparison to the status quo situation based on linear distances.
Table 5. Amount of manure per hectare.
| | Pig fattening | Pig breeding | Biogas production |
| Net* nutrient occurrence** | kg N per FP, BS, kWel*** | 8.4 | 24 | 97 |
| Units per hectare for 170 kg N | FP, BS, kWel** per ha | 30.2 | 7.1 | 1.8 |
| Amount of manure per unit | m3 per FP, BS, kWel** | 2.0 | 6.6 | 21 |
| NPK content* | kg N; P2O5; K2O per m3 | 4.2; 2.5; 2.5 | 3.6; 2.9; 2.6 | 4.6; 2.3; 5.0 |
| Max. amount of manure per ha | m3 per ha | 40 | 47 | 37 |
* After deduction of stall and storage losses in accordance with appendix 6 DüV; a loss coefficient of 10% was assumed for biogas production; ** Nutrient production in pig farming in the case of feeding methods with reduced N/P; ***FP = fattening place, BS= breeding sow.
Source: own diagram based [20,30] and own calculations |
3.2. Survey results regarding transport costs according to the study region
Figure 3 shows that according to those surveyed, the inclusion of manure of plant origin in the calculation of the 170 kg per hectare N limit in all six observed districts, which are located within the study area, would lead to an increase in the cost of disposal between farms via manure brokerage services. The survey participants stated that this would be the result of higher transport distances due to higher land requirements for the transportation of the manure and/or digestates and increasing claims for remuneration by the farms accepting the manure. The highest disposal costs are currently incurred by farmers in the north-west German districts of Vechta, Cloppenburg and Borken. In comparison with the disposal costs in 2013 (situation a), the inclusion of the digestates of plant origin (situation b) would involve an average cost increase of approx. 47% or from approx. 9 €/m3 to 13 €/m3 (excluding value added tax (VAT)).
3.3. Affordable disposal costs in pig fattening, pig breeding and biogas production
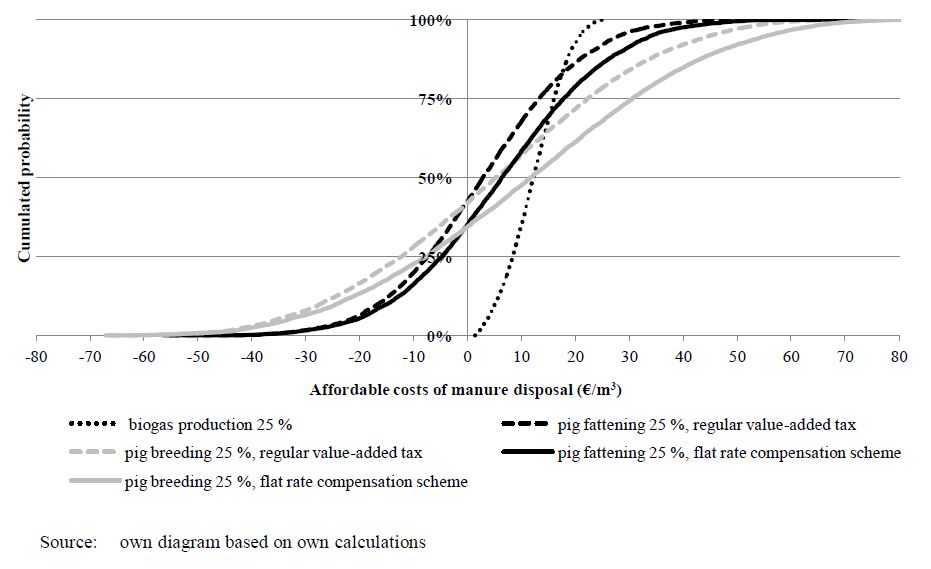
A Monte Carlo simulation was used as an aid to generate the distribution functions of affordable manure transport costs and to depict the change with regard to the relative competitiveness between biogas producers and pig farmers 12 (12 The limitation of this study is the inability to integrate adjustments or responses to regulatory change because it does not include a time dimension. Further, this study does not address enterprise specific analyses, such as the willingness of biogas producers to pay for land aside from manure disposal costs, that would also reduce transport distances, e.g. silage corn. For this purpose, farm or biogas plant specific infrastructure would have to be considered. Further research is needed to assess impacts on enterprises individually. The conducted study aims to identify general economic impacts, which are solely caused by manure disposal.). Although pig farms normally make use of the value-added tax flat rate compensation scheme, some farms are subject to standard taxation 13 (13 To compare regular value-added tax (VAT) with the flat rate compensation scheme and its economic impact see [44].). Therefore, in the following explanations, a distinction is made between the value-added tax flat rate compensation scheme (= “flat rate compensation farms”) and regular value-added tax (“VAT farms”). Figure 4 shows the distribution function of the manure disposal costs that are affordable for the observed production sectors in north-west Germany.
On the basis of Figure 4, and in consideration of the production figures assumed in Table 2, biogas production has the highest expected value 14 (14 Intersection of simulated curves and 50% horizontal line because of the symmetry of the distribution function.) (approx. 5 €/m3) with regard to the maximum affordable manure disposal costs.
Although the expected values of pig farms are predominantly negative, Figure 5 shows that in comparison to successful biogas producers, above-average animal performance in pig farming can facilitate almost the same ability to pay the additional cost because their expected value is closer to that of the biogas producers.
Under these assumptions, the expected value is approx. 3 €/m3 (VAT farms) or 7 €/m3 (flat rate compensation farms) for the production sector of pig fattening, approx. 5 €/m3 (VAT farms) or 11.5 €/m3 (flat rate compensation farms) for the production sector of pig breeding and 12.5 €/m3 for the production sector of biogas production.
Comparing Figures 3 and 4, the current average cost of manure disposal between farms in the observed regions already exceed the cost that pig farmers and, to lesser extent, biogas producers, can pay in most of the simulated cases. The possibility that the cost increase associated with the inclusion of manure of plant origin would even cause pig fattening farmers with above-average success in some districts to reach their economic limits is high (see Figures 3 and 5). The farms concerned would be forced to compensate for these high disposal costs by, for example, going without wage payments, return on capital and/or depreciation. Nevertheless, a sustainable ability to evolve and grow and a competitive ability are not possible without suitable remuneration for the factors used. Under the assumptions made, only above-average pig breeders using the value-added tax flat rate compensation scheme and above-average biogas producers would be able to generate the required expected values and/or ability to pay the additional costs due to the comparatively high value creation per m³ of manure produced. The current increased requirements for reducing the emissions of these types of production in the north-west federal states of Germany have not yet been factored into this equation. In this context, however, it is important to consider the fact that increasing disposal costs would mean that farms that are unable to incur the increased costs would have to take suitable adaptation measures that may help to relieve the nutrient problem in the regions concerned (see section 4). Given that, in many cases, these adaptation measures (e.g., abandoning production or reorganization) only take place when reinvestments and/or new leases are impending, it can nevertheless be assumed that there will be no relief to the nutrient situation.
The trend of the distribution functions in Figure 5 can be used to infer that the expected values of pig breeding are connected to a higher variation coefficient than the expected value of biogas production. In connection with these values, a higher probability of an inability to pay the additional costs must be demonstrated. In comparison, successful biogas producers are always able to pay the additional costs. Another important finding is the changing relative competitiveness, especially between pig fattening and pig breeding. The latter will be relatively more competitive because there is a higher value added per nutrient unit. As the next chapter shows, this could also have an impact on land markets.
3.4. Potential impact on regional motivation to pay additional costs for agricultural land
Farms with a limited amount of land can alternatively choose to lease or purchase more land to avoid manure disposal between farms. This results in an indirect connection between the motivation (not necessarily the ability) to pay the additional manure disposal costs and the motivation to pay the additional costs for agricultural land. Given that manure disposal within the study area is a widespread spatial problem, no farm-specific circumstances (e.g., reduction of silage corn transport distance with fields closer to the biogas plant) are considered below. In order to make a statement on the impacts of increasing costs of manure disposal to other farms may have on the development of the lease price level, the maximum amounts of manure that can be spread per hectare were initially determined by means of the respective occurrence of manure per animal place (fattening place/breeding sow) and/or the installed electrical output (kWel) and the nitrogen content in manure, as well as in consideration of the upper limit for nitrogen spreading (see Table 5).
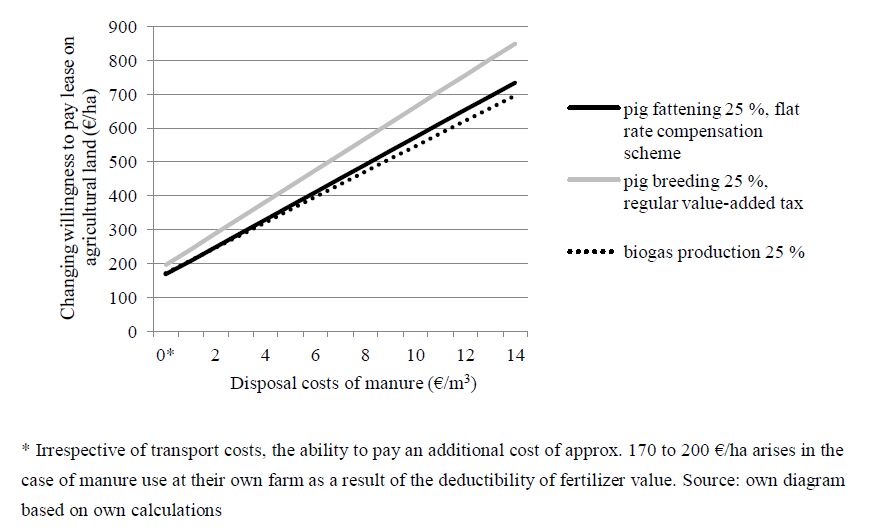
If manure values of 6 €/m3 for pig slurry and 7.5 €/m3 for liquid digestates (because of their fertilizer value) and spreading costs of 3 €/m3 in the case of manure use at one single farm are assumed [45], the connection between the cost of disposal between farms and the change in willingness to pay a lease for agricultural land 15 (15 The willingness to pay an additional lease generated by means of crop farming and land-dependent direct payments remains unconsidered.) (shown in Figure 6) can be identified based on the manure quantities shown in Table 5.
The diagrams are based on the assumption that the motivation to pay additional costs to lease agricultural land corresponds to the overall costs arising from manure disposal between farms, plus the fertilizer value and minus the costs of spreading on their own agricultural land. If the average increase in disposal costs is assumed, from approx. 9 to 13 €/m3 (determined in section 3.2), an increase in transport costs of approx. 200 € for a manure quantity of approx. 47 m3 occurs for the case of, for example, pig breeders. This concerns the amount of manure that could alternatively be spread on a maximum of 1 ha of leased agricultural land in consideration of the nitrogen content (see Table 5). The increase in disposal costs of approx. 4 €/m3 derived from the conducted survey therefore leads to an increase in the motivation to pay the additional cost for 1 hectare of leased land of approx. 200 € or from approx. 600 € to 800 € (see Figure 6).
4. Discussion and conclusions
Within the study area of north-west Germany, which is now already characterized by its high manure disposal costs and high lease price of agricultural land in particular, the inclusion of manure of plant origin in the calculation of the upper limit of 170 kg per hectare will cause the manure disposal and land costs to further increase and, as a result, a multitude of farms would no longer be able to suitably pay for the factors used. The spatial dimension of additional manure transportation is outstanding. As the modelling shows, nutrients would have to be shipped much further than before. Both the spatial modelling and the conducted survey of experts show higher expected transportation and disposal costs of 1 and 4 Euro/m³ on average, respectively. However, net manure exports to north-west Germany from the Netherlands and Belgium, which also show high nutrient burdens and border west to the study area, were not taken into consideration. Furthermore distribution algorithm is based on linear distances. This implies that the theoretical increase in manure disposal costs was underestimated. This is another reason why the survey results are different from the modelled results.
The full cost analysis, together with a Monte Carlo simulation as a risk analysis tool, depicts the changing relative competitiveness, especially between piglet producing and pig fattening. Therefore, pig breeding could succeed more in north-west Germany with the new circumstances of the DüV than could pig fattening because of its comparatively low value added per manure unit produced. This confirms the hypothesis that the competitiveness between biogas producers and especially between piglet production and pig fattening will change. Only above-average pig breeders using the value-added tax flat rate compensation scheme, and biogas plant operators would be able to compensate for increasing disposal costs in some of the regions assessed.
Nevertheless, the developments highlighted above would not only affect the production processes of pig fattening, pig breeding and biogas production observed in this study. Changing manure disposal costs and lease and purchase prices for agricultural land in a region would also influence the competitiveness of other animal production processes such as cattle farming. Higher disposal and land costs may also limit their competitiveness. However, the impact on cattle farms still needs to be investigated further. Poultry farming would probably be less affected because manure from poultry farming (e.g., dry chicken manure or chicken droppings) has a comparatively high transportability and therefore hardly rivals liquid manure in terms of the spreading area.
In order to keep the economic impacts of a revised DüV as low as possible for the affected farms, adaptation strategies need to be promptly developed and established. A variety of different measures will emerge alongside the higher investment costs caused by the procurement of new spreading technology and additional storage capacities that can be expected as a result of the revised DüV and were not factored into this study.
Furthermore, the increasing manure disposal costs would also mean that farms would, for example, in the case of impending reinvestments, suspend their production activities and, where necessary, make new investments in and/or relocate their facilities to regions that are characterized by a low occurrence of manure and therefore gain relative excellence. Where this matter is concerned, successful pig farmers in the stock farming regions should not underestimate the impact of stall units available for lease, which does not lead to a reduction in the occurrence of manure. Nevertheless, production sectors such as pig farming, which do not depend on amount of land, at least in terms of feed supply, will experience a forced structural change as a result of a revised DüV. These developments may help to lessen the economic and/or structural effects specified above, but will not enable them to be completely avoided. From an economic perspective alone, it therefore seems that the further growth of stock farming in the observed regions of north-west Germany will be even more difficult in the future than at present.
For farms, it is now more important than ever to exhaust all options that result in a reduction in the operational occurrence of nutrients (e.g., feeding methods with reduced N/P) but also increase the transportability of the manure produced (e.g., separation of slurry/digestates or using 'twin trailers') and improve the nutrient efficiency of manure use (e.g., optimized spreading techniques) in order to accommodate both the water protection requirements and the sustainable ability to evolve and grow. In the case of the inclusion of digestates of plant origin in the calculation of the upper limits for nitrogen spreading, these measures are even more applicable. This development in Germany should serve as a warning to other countries both in and outside of Europe, indicating that they should not permit any uninhibited biogas production, especially in regions with intensive livestock keeping. Although this actually lends itself to biogas production due to the high occurrence of manure of animal origin, an additionally higher proportion of farmland-based substrates should definitely not occur. The nutrient concentrations resulting from such substrates would lead to unacceptable ecological and economic consequences.
Conflict of interest
The authors certify that they have no affiliations with or involvement in any organization or entity with any financial interest (such as honoraria; educational grants; participation in speakers’ bureaus; membership, employment, consultancies, stock ownership, or other equity interest; and expert testimony or patent-licensing arrangements), or non-financial interest (such as personal or professional relationships, affiliations, knowledge or beliefs) in the subject matter or materials discussed in this manuscript.
Appendix:
I. Assumptions for Monte Carlo simulation as a risk analysis tool:
1. Definition of a decision model to identify the stochastic factors for pig fattening, pig breeding and biogas production
a. Stochastic factors for pig fattening: slaughter price, piglet price and feed price
b. Stochastic factors for pig breeding: piglet price and feed price
c. Stochastic factors for biogas production: silage maize price
2. Definition of probability density function for risk variables by using historical price data series
a. Pig production: direct use of above mentioned stochastic factors
b. Biogas production: determination of an indifference price for silage maize using price data for winter wheat (Assumption: equal profit margin of silage maize and winter wheat)
3. Assumption for all risk variables: normally distributed density function
4. Simulation of input variables via a pseudo-random generator and transferring the result to the target function
5. Drawing the distribution functions of the manure disposal costs, which are affordable for average and above-average performance levels in pig fattening, pig breeding and biogas production
II. Results of Kolmogorov Smirnov Test:
| | Slaughter pig price | Basic piglet price | Feed price for pig fattening | Feed price for pig breeding | Maize silage price up to fermenter |
| N | | 88 | 88 | 88 | 88 | 88 |
| Normal Parameters a, b | Mean | 1.5245 | 44.5167 | 26.1874 | 28.9119 | 42.0307 |
| Std. Deviation | 0.16065 | 7.92595 | 4.45562 | 3.88335 | 8.79167 |
| Most Extreme Differences | Absolute | 0.063 | 0.096 | 0.100 | 0.086 | 0.107 |
| Positive | 0.063 | 0.052 | 0.083 | 0.086 | 0.106 |
| Negative | -0.050 | -0.096 | -0.100 | -0.084 | -0.107 |
| Kolmogorov-Smirnov Z | | 0.063 | 0.096 | 0.100 | 0.086 | 0.107 |
| Asymp. Sig. (2-tailed) | | 0.200c | 0.044c | 0.030c | 0.111c | 0.014c |
a. Test distribution is Normal.
b. Calculated from data.
c. Significance correction according Lilliefors. |
Annotation: Critical value of most extreme differences (N = 88 and α = 0.01): 0.173; Asymp. Sig. (2-tailed) ≥ α; Normal distribution of considered prices can be assumed.









 DownLoad:
DownLoad: