The purpose of this review is to acknowledge the current state-of-the-art and the progress of in situ Raman spectro-electrochemistry, which has been made on all the elements in lithium-ion batteries: positive (cathode) and negative (anode) electrode materials. This technique allows the studies of structural change at the short-range scale, the electrode degradation and the formation of solid electrolyte interphase (SEI) layer. We also discuss the results in the perspective of spatial and real-time investigations by in situ Raman imaging (mapping) during charge/discharge cycling.
1.
Introduction
The formation of shear bands in elasticity is described by a degenerate operator of elliptic-hyperbolic type [1, 23, 4]. The shear bands that are mathematically obtained in this model are infinitesimally thin. To overcome this non-physical description, it is customary to penalize the elasticity operator by a fourth-order singular perturbation [18]. Subsequently, it was suggested that the penalization should be followed by a homogenization procedure, which results in different regimes depending on the order of penalization as compared to the length-scale of the periodic heterogeneities. This was first carried out in [6, 18]. Similar problems in the framework of Γ-convergence have been studied in [32, 8, 39]. A quantitative analysis of this problem appeared in [27, 26, 29]. The aim of the present work is to revisit this problem by employing the homogenization framework developed by Conca and Vanninathan [13]. Bloch wave method in homogenization is a spectral method of homogenization. It also offers an alternative approximation scheme in the form of Bloch approximation [11, 12] which provides sharp convergence estimates in homogenization under minimal regularity requirements. In this paper, we shall establish new characterizations of homogenized tensor for the singularly perturbed problem in terms of the first Bloch eigenvalue and use Bloch wave method to recover the homogenization result for singularly perturbed periodic homogenization problem.
1.1. Notation and definitions
We will study the simultaneous homogenization and singular perturbation limits of the following operator
where 0<κ,ε≪1, and A(y)=(ajk)dj,k=1 is a matrix whose entries are real, bounded, measurable functions of y∈Rd. Further, the matrix A satisfies the following hypotheses:
A1. A is elliptic, i.e., there exists α>0 such that for all ξ∈Rd and a.e. y∈Rd, A(y)ξ⋅ξ≥α|ξ|2.
A2. A is Y-periodic, i.e., A(y+2πp)=A(y) for all p∈Zd, a.e. y∈Rd. The set Y:=[0,2π)d is called a basic periodicity cell and may also be interpreted as a parametrization of the d-dimensional torus Td.
A3. The matrix A is symmetric.
We mention briefly the function spaces that make an appearance in this problem. The solutions of the cell problem associated with homogenization of (1.1), as well as Bloch eigenfunctions, are sought in the space H2♯(Y), which is the space of all periodic distributions u for which the norm ||u||H2=(∑n∈Zd(1+|n|2)2|ˆu(n)|2)12 is finite. The space H2♯(Y) may be identified with H2(Td). The spaces Hs♯(Y) for s∈R are similarly defined. For s>0, Hs♯(Y) forms a subspace of the space of all periodic L2 functions in Rd, denoted by L2♯(Y) or L2(Td). We shall denote the mean value or average of a periodic function u on the basic periodicity cell Y by MY(u):=1|Y|∫Yu(y)dy. Averaged integrals such as 1|Y|∫Yu(y)dy are sometimes denoted as 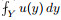 .
.
1.2. The method of Bloch wave homogenization
The method of Bloch waves rests on decomposition of a periodic operator in terms of Bloch waves which may be thought of as a periodic analogue of plane waves. As plane waves decompose a linear operator with constant coefficients by means of the Fourier transform, Bloch waves diagonalize a linear operator with periodic coefficients. This decomposition begins with a direct integral decomposition of a periodic operator A in Rd.
The fiber operator A(η) has compact resolvent for each fixed η∈Td. The eigenfunctions viewed as functions of η are called Bloch waves. Finally, the operator A(η) is diagonalized by means of Bloch waves.
The homogenization limits for a highly oscillating scalar periodic operator are obtained from its first Bloch mode. The rest of the Bloch modes do not contribute to the homogenization limit. This is a consequence of the separation of the first Bloch eigenvalue from the rest of the spectrum. Such an interpretation of homogenization is also called spectral threshold effect [7]. Moreover, the homogenized tensor is obtained from Hessian of the first Bloch eigenvalue. Therefore, the second-order nature of the differential operator is reflected in the quadratic nature of the first Bloch eigenvalue near the bottom of the spectrum. Indeed, homogenization of higher-even-order periodic operators can also be obtained by the Bloch wave method, where the first Bloch eigenvalue behaves like a polynomial of the corresponding order near the bottom of the spectrum [37, 36].
The homogenization result in Theorem 8.1 exhibits three different regimes depending on the ratio of κ and ε, where κ is to be interpreted as a function of ε satisfying limε→0κ=0. Define ρ:=κε. The three different regimes correspond to
● limε→0ρ=0,
● 0<limε→0ρ<∞, and
● limε→0ρ=∞.
In the first regime, the subcritical case, that is, limε→0ρ=0, homogenization dominates over the singular perturbation. Hence, it may be thought of as taking the homogenization limit followed by the singular perturbation limit. In the critical regime, that is, 0<limε→0ρ<∞, we can see the combined effects of singular perturbation and homogenization. The cell functions are solutions to a fourth order operator. On the other hand, in the supercritical regime, that is, limε→0ρ=∞, singular perturbation dominates the homogenization limit and homogenized coefficients are given by the average of the periodic coefficients.
The approach is to treat ρ as a fixed number and obtain Bloch wave decomposition for the unscaled operator Aρ=ρ2Δ2−∇⋅A(y)∇. This decomposition is employed to obtain the homogenization limit as ε,κ→0. While the qualitative homogenization result is not new, the presentation is original. Some novel features of the proof are characterization of homogenized tensor and cell functions in terms of derivatives of the first Bloch eigenvalue and eigenfunctions. This requires us to prove analyticity of the first Bloch eigenvalue and eigenfunction in a neighbourhood of zero in the dual parameter. Interestingly, the region of analyticity does not depend on the singular perturbation, which plays an important role in the simultaneous passage to 0 of κ and ε. In order to study the stability of the homogenized tensor in the different regimes, we obtain uniform in ρ estimates for Bloch eigenvalues, eigenfunctions and their derivatives of all orders in the dual parameter. We also prove that only the first Bloch mode contributes to homogenization and the higher modes are negligible. The analysis of three separate regimes provides considerable challenges in the Bloch wave method, particularly in obtaining uniform estimates in these regimes.
While the motivation for the problem (1.1) comes from the theory of elasticity, it is for the sake of simplicity that we only study the scalar operator. However, it must be noted that Bloch wave homogenization of systems carries some unique difficulties, such as the presence of multiplicity at the bottom of the spectrum. Indeed, these challenges have been surmounted by the use of directional analyticity of Bloch eigenvalues in [35, 7, 3]. Further, the assumption of symmetry, while customary in elasticity, is made for a simplified presentation. A Bloch wave analysis of homogenization of non-selfadjoint operators may be found in [19].
In a forthcoming work, we will obtain quantitative estimates for the combined effects of singular perturbation and homogenization through the notion of Bloch approximation, which was introduced in [11]. Higher order estimates in homogenization have been obtained by these methods, particularly for the dispersive wave equation [14, 15, 2, 22].
1.3. Plan of the paper
The plan of the paper is as follows: In Section 2, we obtain Bloch waves for the singularly perturbed operator Aρ. In Section 3, we prove that the first Bloch eigenpair are analytic functions of the dual parameter in a neighbourhood of 0. In Section 4, we prove that the neighbourhood of analyticity is independent of ρ. In Section 5, we recall the cell problem for the operator Aρ and the estimates associated with it. In Section 6, we characterize the homogenized tensor in the three regimes by way of Bloch method. In Section 7, we shall define the first Bloch transform and analyze its asymptotic properties. In Section 8, we obtain the qualitative homogenization theorem by means of the first Bloch transform, which is the periodic analogue of Fourier transform. Finally, in Section 9, we quantify the contribution of the higher Bloch transforms towards the homogenization limit.
2.
Bloch waves for the singularly perturbed operator
In this section, we will prove the existence of Bloch waves for the singular operator given by
Recall that ρ was earlier set as κε, however in this section, ρ will be assumed to be a fixed positive number. Bloch waves for (2.1) refers to eigenfunctions of (2.1) satisfying the so-called (η−Y)-periodicity condition, that is, we look for functions ψ satisfying the following eigenvalue problem:
The above problem is invariant under Zd-shifts of η, hence it suffices to restrict η to Y′:=[−12,12)d. Now, if we set ψ(y)=eiy⋅ηϕ(y) where ϕ is a Y-periodic function, then the above eigenvalue problem is transformed into:
The operator Aρ(η) is often called the shifted operator associated with Aρ where the shift iη appears as a magnetic potential. In order to prove the existence of eigenvalues for (2.2), we shall begin by proving that a zeroth-order perturbation of Aρ(η) is elliptic on H2♯(Y). This amounts to a Gå rding type inequality for the operator Aρ(η). Combined with Rellich compactness theorem, this will allow us to prove compactness of the inverse in L2♯(Y). Then, a standard application of the spectral theorem for compact self-adjoint operators will guarantee the existence of eigenvalues for each fixed ρ and η.
The bilinear form aρ[η](⋅,⋅) defined on H2♯(Y)×H2♯(Y) by
is associated to the operator Aρ(η). We shall prove the following Gå rding-type inequality for aρ[η].
Lemma 2.1. There exists a positive real number C∗ not depending on η but depending on ρ such that for all u∈H2♯(Y) and all η∈Y′, we have
Proof. We have
We shall estimate the two summands separately. For the first summand, observe that
where Re denotes the real part. Now we shall estimate each term on the RHS above. The first term of I is estimated as follows:
For the second term of I, observe that
Finally, the third term of I is dominated by L2♯(Y) norm of u as follows:
Now, we may choose C2 so that C1C2=α2, then
For the second summand, observe that
We estimate the last three terms as follows.
The previous threee estimate use Cauchy-Schwarz inequality for the second step and Young's inequality for the third step. Substituting the inequalities (2.12), (2.13) and (2.14) into (2.11), we get
Since |η|≤12, we get
Now, notice that
Substituting (2.16) in (2.15), we get
Combining (2.10) and (2.17), we obtain (2.4) with
Remark 2.2. In the Gå rding type inequality for the operator Aρ(η), the perturbation C∗I in zeroth term depends on the parameter ρ. It is possible to avoid the dependence of C∗ on ρ by forgoing the shift iη in the biharmonic term. This simplification was employed in [34].
Now that we have the coercivity estimate (2.4), we can prove the existence of Bloch eigenvalues and eigenfunctions for the operator Aρ.
Theorem 2.3. For each η∈Y′ and ρ>0, the singularly perturbed Bloch eigenvalue problem (2.2) admits a countable sequence of eigenvalues and corresponding eigenfunctions in the space H2♯(Q).
Proof. Lemma 2.1 shows that for every η∈Y′ the operator Aρ(η)+C∗I is elliptic on H2♯(Y). Hence, for f∈L2♯(Y), this shows that Aρ(η)u+C∗u=f is solvable and the solution is in H2♯(Y). As a result, the solution operator Sρ(η) is continuous from L2♯(Y) to H2♯(Y). Since the space H2♯(Y) is compactly embedded in L2♯(Y), Sρ(η) is a self-adjoint compact operator on L2♯(Y). Therefore, by an application of the spectral theorem for self-adjoint compact operators, for every η∈Y′ we obtain an increasing sequence of eigenvalues of Aρ(η)+C∗I and the corresponding eigenfunctions form an orthonormal basis of L2♯(Y). However, note that both the operators Aρ(η) and Aρ(η)+C∗I have the same eigenfunctions but each eigenvalue of the two operators differ by C∗. We shall denote the eigenvalues and eigenfunctions of the operator Aρ(η) by η→(λρm(η),ϕρm(⋅,η)).
Remark 2.4. We can prove the existence of Bloch eigenvalues and eigenfunctions for the case ρ=0 by a similar method. This corresponds to the standard Bloch eigenvalue problem considered in [13].
2.1. Bloch decomposition of L2(Rd)
Now that we have proved the existence of Bloch eigenvalues and eigenfunctions, we can state the Bloch Decomposition Theorem which offers a partial diagonalization of the operator Aρ in terms of its Bloch eigenvalues. This is facilitated by the Bloch transform which is a mapping from L2(Rd) to ℓ2(N;L2(Y′)). The proof is similar to the one in [6] and is therefore omitted. Note that the proof relies on a measurable selection of the Bloch eigenfunctions with respect to η. A measurable selection of Bloch eigenfunctions for the Schrödinger operator was first demonstrated in [38]. In contrast, the Bloch eigenvalues are Lipschitz continuous in the dual parameter η. We will prove this fact in Section 4.
Theorem 2.5. Let ρ>0. Let g∈L2(Rd). Define the mth Bloch coefficient of g as
1. The following inverse formula holds
2. Parseval's identity
3. Plancherel formula For f,g∈L2(Rd), we have
4. Bloch Decomposition in H−1(Rd) For an element F=u0(y)+∑Nj=1∂uj(y)∂yj of H−1(Rd), the following limit exists in L2(Y′):
The definition above is independent of the particular representative of F.
5. Finally, for g∈D(Aρ),
3.
Regularity of the ground state
The Bloch wave method of homogenization requires differentiability of the Bloch eigenvalues and eigenfunctions in a neighbourhood of η=0. In this section, we will prove the following theorem. As before, ρ>0 will be treated as a fixed number.
Theorem 3.1. For every ρ>0, there exists δρ>0 and a ball Uρ:=Bδρ(0):={η∈Y′:|η|<δρ} such that
1. The first Bloch eigenvalue η→λρ(η) of Aρ is analytic for η∈Uρ.
2. There is a choice of corresponding eigenfunctions ϕρ1(⋅,η) such that η∈Uρ→ϕρ1(⋅,η)∈H2♯(Y) is analytic.
For the proof, we will make use of Kato-Rellich theorem which establishes the existence of a sequence of eigenvalues and eigenfunctions associated with a selfadjoint holomorphic family of type (B). The definition of selfadjoint holomorphic family of type (B) and other related notions may be found in Kato [21]. Nevertheless, they are stated below for completeness. We begin with the definition of a holomorphic family of forms of type (a).
Definition 3.2.
1. The numerical range of a form a is defined as
where D(a) denotes the domain of the form a. Here, D(a) is a subspace of a Hilbert space H.
2. The form a is called sectorial if there are numbers c∈R and θ∈[0,π/2) such that
3. A sectorial form a is said to be closed if given a sequence un∈D(a) with un→u in H and a(un−um)→0 as n,m→∞, we have u∈D(a) and a(un−u)→0 as n→∞.
Definition 3.3. [Kato] A family of forms a(z),z∈D0⊆CM is called a holomorphic family of type (a) if
1. each a(z) is sectorial and closed with domain D⊆H independent of z and dense in H,
2. a(z)[u,u] is holomorphic for z∈D0⊆CM for each u∈D.
A family of operators is called a holomorphic family of type (B) if it generates a holomorphic family of forms of type (a).
In [21, 31], Kato-Rellich theorem is stated only for a single parameter family. In [5], one can find the proof of Kato-Rellich theorem for multiple parameters with the added assumption of simplicity for the eigenvalue at η=0.
Theorem 3.4. (Kato-Rellich) Let D(˜η) be a self-adjoint holomorphic family of type (B) defined for ˜η in an open set in CM. Further let λ0=0 be an isolated eigenvalue of D(0) that is algebraically simple. Then there exists a neighborhood R0⊆CM containing 0 such that for ˜η∈R0, the following holds:
1. There is exactly one point λ(˜η) of σ(D(˜η)) near λ0=0. Also, λ(˜η) is isolated and algebraically simple. Moreover, λ(˜η) is an analytic function of ˜η.
2. There is an associated eigenfunction ϕ(˜η) depending analytically on ˜η with values in H.
The proof of Theorem 3.1 proceeds by complexifying the shifted operator Aρ(η) before verifying the hypothesis of Kato-Rellich Theorem.
Proof. (Proof of Theorem 3.1)
(i) Complexification of Aρ(η): The form aρ[η](⋅,⋅) is associated with the operator Aρ(η). We define its complexification as
for ˜η∈R where
(ii) the form t(˜η) is sectorial: We have
From above, it is easy to write separately the real and imaginary parts of the form t(˜η).
The following coercivity estimate can be easily found for the real part:
Let us define the new form ˜t(˜η) by ˜t(˜η)[u,v]=t(˜η)[u,v]+(C5+C6)(u,v)L2♯(Y). Then it holds that
Also, the imaginary part of ˜t(˜η) can be estimated as follows:
This shows that ˜t(˜η) is sectorial. However, sectoriality is invariant under translations by scalar multiple of identity operator in L2♯(Y), therefore the form t(˜η) is also sectorial.
(iii) The form t(˜η) is closed: Suppose that unt→u. This means that un→u in L2♯(Q) and t(˜η)[un−um]→0. As a consequence, ℜt(˜η)[un−um]→0. By (3.1), ||un−um||H2♯(Y)→0, i.e., (un) is Cauchy in H2♯(Y). Therefore, there exists v∈H2♯(Y) such that un→v in H2♯(Y). Due to uniqueness of limit in L2♯(Y), v=u. Therefore, the form is closed.
(iv) The form t(˜η) is holomorphic: The holomorphy of t follows as a consequence of t being a quartic polynomial in η.
(v) 0 is an isolated eigenvalue: Zero is an eigenvalue because constants are eigenfunctions of Aρ(0)=−∇⋅A∇+ρ2Δ2. As a result, C∗ is an eigenvalue of Aρ(0)+C∗I. We proved using Lemma 2.1 that Aρ(0)+C∗I has compact resolvent. Therefore, C−1∗ is an eigenvalue of (Aρ(0)+C∗I)−1 and C−1∗ is isolated. Hence, zero is an isolated point of the spectrum of Aρ(0).
(vi) 0 is a geometrically simple eigenvalue: Denote by kerAρ(0) the kernel of operator Aρ(0). Let v∈kerAρ(0), then ∫YA∇v⋅∇vdy+ρ2∫Y|Δv|2=0. Due to the coercivity of the matrix A, we obtain ||∇v||L2♯(Y)=0. Hence, v is a constant. This shows that the eigenspace corresponding to eigenvalue 0 is spanned by constants, therefore, it is one-dimensional.
(vii) 0 is an algebraically simple eigenvalue: Suppose that v∈H2♯(Y) such that Aρ(0)2v=0, i.e., Aρ(0)v∈kerAρ(0). This implies that Aρ(0)v=C for some generic constant C. However, by the compatibility condition for the solvability of this equation, we obtain C=0. Therefore, v∈kerAρ(0). This shows that the eigenvalue 0 is algebraically simple.
4.
Neighbourhood of analyticity
In Section 3, we have proved that the first Bloch eigenvalue and eigenfunction is analytic in a neighbourhood of η=0. However, a priori, this neighbourhood depends on the parameter ρ. In this section, we will prove that the neighbourhood is, in fact, independent of ρ. This requirement is essential for problems where simultaneous limits with respect to two parameters are studied, such as [28, 16]; as well as for quantitative estimates, such as [10].
We begin by proving that Bloch eigenvalues are Lipschitz continuous in the dual parameter.
Lemma 4.1. For all m∈N and ρ>0, λρm is a Lipschitz continuous function of η∈Y′.
Proof. The following form is associated with Aρ(η):
Hence, for η,η′∈Y′, we have
where C and C′ are generic constants independent of ρ. By the Courant-Fischer minmax characterization of eigenvalues, we obtain
where μm is the mth eigenvalue of the following spectral problem:
and νm is the mth eigenvalue of the following spectral problem:
By interchanging the role of η and η′, we obtain
Here, C is a generic constant independent of ρ.
Now, we will prove a spectral gap result, viz. the second Bloch eigenvalue is bounded below.
Lemma 4.2. For all m≥2 and for all η∈Y′, we have
where λN2 is the second Neumann eigenvalue of the operator −Δ in Y.
Proof. Notice that λρm(η)≥λρ2(η) for all m≥2 and for all η∈Y′. Next, observe that
The bound obtained in Lemma 4.2 will be useful for the small ρ regime, however, for the large ρ regime, that is, for ρ→∞, we need a different lower bound.
Lemma 4.3. For all m≥2 and for all η∈Y′, we have
where κ2 is the 2nd eigenvalue of periodic bilaplacian on Y, where C and C′ are generic constants independent of ρ and η.
Proof. Recall the following Gå rding type estimate (2.4) for the form aρ[η] associated with the operator Aρ(η):
The inequality in Lemma 4.3 follows readily from above by applying the minmax characterization.
Remark 4.4. In Lemma 4.2 and Lemma 4.3, we have avoided estimating the second Bloch eigenvalue by using the spectral problem associated with Neumann bilaplacian as it is known to be ill-posed [30]. Moreover, polyharmonic Neumann eigenvalue problems on polygonal domains (such as Y) are less well understood [20, 17]. However, suitable natural boundary conditions associated with the operator Δ2−τΔ are obtained in [9].
We are finally in a position to prove that the neighbourhood of analyticity of the first Bloch eigenvalue does not depend on the parameter ρ.
Theorem 4.5. There exists a neighbourhood U=Bδ(0) of η=0 , not depending on ρ, such that λρ1(η) is analytic on Bδ(0).
Proof. It was proved in Theorem 3.1 that the first Bloch eigenvalue is analytic in a neighbourhood of η=0. However, a priori it is not clear whether this neighbourhood is independent of ρ. To prove this, it suffices to prove that the first Bloch eigenvalue is simple in a neighbourhood of η=0 independently of ρ. Observe that
where C is a generic constant independent of ρ and η.
● For sufficiently large ρ,
for |η|<C‴2. Here, C,C′,C″ and C‴ are all generic constants independent of ρ and η.
● For remaining values of ρ,
for |η|<αλN22C.
Remark 4.6. In the papers [33, 34], an additional artificial parameter is introduced in the Bloch eigenvalue problem to facilitate the homogenization method. Unlike (1.1), these papers employ successive limits of the two parameters instead of simultaneous limits. Therefore, the non-dependence of the neighbourhood of analyticity on the second parameter is not required in [33, 34].
5.
Cell problem and estimates
In this section, we will consider the classical cell problem associated with (1.1) and the estimates for the corrector field. This section will allow us to characterize the homogenized tensor for (1.1) and the corrector field in terms of Bloch eigenvalues and eigenfunctions.
For 1≤j≤d, consider the following cell problem associated with the operator (1.1):
By a simple application of Lax-Milgram lemma on H2♯(Y), we obtain solution to above for every ρ>0 (For ρ=0, Lax-Milgram lemma is applied for H1♯(Y)). Further, since the equation is also satisfied in the sense of distributions, we conclude that χρj∈H3♯(Y) for ρ>0. By using χρj as a test function, we obtain the following bound:
If we use Δχρj as a test function, we obtain
We also collect below a few estimates which will be required later. Similar estimates have been proved in [26] to which we refer for more details.
Lemma 5.1. Let ρ1,ρ2>0. Let χρ1,χρ2∈H2♯(Y) be solutions to (5.1) for ρ=ρ1 and ρ=ρ2 respectively, then the following estimate holds.
Proof. Define z=χρ1j−χρ2j, then z satisfies the following equation
Now, the quoted estimate readily follows by taking z as the test function, applying uniform ellipticity of A, (5.3) and an application of Poincaré inequality.
Lemma 5.2. Let ρ>0. Let χρ∈H2♯(Y) be the solution to (5.1) and let χ0∈H1♯(Y) solve
Then, there is q∈(1,∞) such that for every ϰ>0 there exists a matrix B with entries in C∞♯(Y) such that ||A−B||Lq♯(Y)≤ϰ and the following estimates hold.
where χB∈H1♯(Y) solve
Proof. Observe that given any q∈(1,∞) and ϰ>0, we can find a smooth periodic matrix B with the same ellipticity constant and upper bound as A such that
For example, this can be achieved by a standard smoothing by convolution. Now, by regularity theory, χBj∈H2♯(Y). Define z=χρj−χBj, then z satisfies the following equation
We test this equation against z to obtain:
This leads to
By Young's inequality,
On the last term, we apply a form of Meyers estimate for the Lp integrability of ∇χρj proved in [26, Page 7, Theorem 2.3]. This fixes the choice of q. This finishes the proof of the estimate (5.8). The proof of (5.7) is similar and simpler and hence omitted.
For every fixed 0≤ρ<∞, the homogenized tensor for the operator Aρ,ε=ρ2Δ2−divA(xε)∇ is given by
Definition 5.3 (Homogenized Tensor for Aκ,ε)
6.
Bloch characterization of homogenized tensor
In this section, we will give a new characterization of the homogenized tensor (see Definition 5.3), and corrector field (5.1) in terms of the first Bloch eigenvalue and eigenfunction. These characterizations are obtained by differentiating the Bloch spectral problem (2.2) with respect to the dual parameter η. Indeed, this is possible since we proved the analyticity of the first Bloch eigenvalue and eigenfunction with respect to η in a neighbourhood of η=0 in Theorem 3.1. Moreover we will use the properties of the first Bloch eigenvalue to prove the stability of the homogenized tensor with respect to the limits ρ→0 and ρ→∞.
6.1. Derivatives of Bloch eigenvalues and eigenfunctions
We recall the Bloch eigenvalue problem for the operator Aρ here:
We know that λρ1(0)=0. For η∈Y′, recall that Aρ(η)=ρ2(∇+iη)4−(∇+iη)⋅A(y)(∇+iη). In this section, for notational convenience we will hide the dependence on y.
We shall normalize the average value of the first Bloch eigenfunction ϕρ1(⋅;η) to be (2π)−d/2, that is,
for all η in the neighbourhood of analyticity. We shall use β to denote a multiindex, such as β=(β1,β2,…,βd)∈Nd∪{0} and |β|:=|β1|+|β2|+⋯+|βd|. We will use the shorthand ∂βη to denote ∂βη:=∂β1∂ηβ11⋯∂βd∂ηηdd. For simplicity, we will also use the notation ∂β0u=∂β1u∂ηβ11⋯∂βdu∂ηηdd|η=0. Differentiating (6.2), we obtain
for all |β|>0. Later on, we will see this as the compatibility condition associated with the equation satisfied by ∂β0ϕρ1. Denote by ∂β0Aρ:=∂βA∂ηβ|η=0. Then, it holds true that
since Aρ(η) is a fourth order polynomial in η. Direct calculation shows that
where ej denotes the standard Euclidean unit vector with 1 in the jth place and 0 elsewhere. Now, we are in a position to write down the differential equations satisfied by the derivatives of ϕρ1 of all orders. To this end, we recall the Leibniz's formula for the derivatives of product of functions, viz.,
where (βγ)=(β1γ1)⋯(βdγd) and the sum is always finite since (βjγj)=0 whenever βj<γj.
Cell problems for ∂β0ϕρ1_ On differentiating (6.1) with respect to η and applying (6.6) we obtain
Substituting (6.5) in (6.7), we obtain
Expression for ∂β0λρ1_ It is easy to see that λρ1(0)=0 since 0 is the first eigenvalue of the operator Aρ(0). On the other hand, λρ1 is an even function of η since aρ[−η](ϕ,ϕ)=aρ[η](¯ϕ,¯ϕ) and ¯ϕ∈H2♯(Y) if and only if ϕ∈H2♯(Y) (see also [15, Page 41, Lemma 4.4]).
Rearranging (6.8), we get
Integrating (6.9) over Y, using (6.2) and (6.3) and the fact that integrals over Y of derivatives of periodic functions vanish due to Green's identity, we obtain the following formula for the derivatives of the first Bloch eigenvalue at η=0:
We specialize to β=el in (6.10) to get ∂λρ1∂ηl(0)=0 for all l=1,2,…,d.
On the other hand, if we set β=el in (6.8), we obtain
Comparing with (5.1), we conclude that χρl−1iϕρ1(0)∂ϕρ1∂ηl(0) is a constant.
We also specialize to β=el+ek in (6.10) to get
On comparing (6.11) with (5.11), we obtain the following theorem:
Theorem 6.1. The first Bloch eigenvalue and eigenfunction satisfy:
1. λρ1(0)=0.
2. The eigenvalue λρ1(η) has a critical point at η=0, i.e.,
3. For l=1,2,…,d, the derivative of the eigenvector (∂ϕρ1/∂ηl)(0) satisfies:
(∂ϕρ1/∂ηl)(y;0)−iϕρ1(y;0)χρl(y) is a constant in y where χρl solves the cell problem (5.1).
4. The Hessian of the first Bloch eigenvalue at η=0 is twice the homogenized matrix Aρ,hom as defined in (5.11), i.e.,
6.2. Stability of homogenized tensor
Now, we will prove the stability of homogenized tensor in the limits ρ→0 and ρ→∞.
Lemma 6.2. Let 0≤θ<∞ such that ρ↓θ then λρ1(η)→λθ1(η) uniformly on compact sets K contained in their common domain of definition.
Proof. The fact that the first Bloch eigenvalues {λρ1(η)}ρ≥0 are analytic on a common domain in Y′ has been proved in Theorem 4.5. Since ρ↓θ where θ is finite, the sequence ρ is bounded, that is, 0<ρ<ρ0 for some 0<ρ0<∞. We will prove that the family {λρ1(η)}0≤ρ<ρ0 is locally uniformly bounded, that is, for every compact set K,
where C′ is independent of ρ and η. It is an easy consequence of Montel's Theorem [25, Page 9, Prop. 7] that pointwise convergence implies uniform convergence for locally uniformly bounded sequences. We will show that λρ1(η) converges pointwise to λθ1(η). Applying minmax characterization to the form below
we obtain the following inequality:
where ϑ1(η) is the first Bloch eigenvalue of the bilaplacian and λθ1(η) is the first Bloch eigenvalue of the operator −div(A∇)+θ2Δ2 for θ∈[0,∞). On the other hand, we also have
so that an application of minmax characterization yields:
Thus, we obtain
As a consequence, for each η∈Y′, λρ1(η)→λθ1(η) as ρ↓θ.
Theorem 6.3. Let 0≤θ≤∞ such that ρ→θ then Aρ,hom→Ahom, with Ahom as in Definition 5.3.
Proof.
Case 1. θ∈[0,∞): By the characterization in Theorem 6.1, Aρ,hom=12∇2ηλρ1(0). In Lemma 6.2, we have proved that λρ1 conveges uniformly to λθ1 when ρ↓θ for θ∈[0,∞). By Theorem of Weierstrass [25, Page 7, Prop. 5], derivatives of all orders of λρ1(η) converge uniformly on compact sets to corresponding derivatives of λθ1(η). In particular, ∇2ηλρ1 converges uniformly on compact sets to ∇2λθ1. As a consequence,
Case 2. θ=∞: Observe that
where the last inequality follows from Poincaré inequality and (5.3). Therefore, as ρ→∞,
Remark 6.4. As a consequence of Lemma 6.2 and the discussion in Case 1 of Theorem 6.3, for any R>0, and 0≤ρ<R, the first Bloch eigenvalue and all its derivatives are bounded uniformly in their domain of analyticity independent of ρ. We may also conclude from the same that the corresponding (suitably normalized) Bloch eigenfunction and all their derivatives with respect to dual parameter are bounded in H1♯ independent of ρ. For the first Bloch eigenfunction, the boundedness is a consequence of the following calculation, which is a consequence of (2.4):
Recall that the number C∗ is explicitly given in (2.18). The boundedness in H1♯(Y) of the derivatives of the first Bloch eigenfunction in the dual parameter is a consequence of its analyticity [25, Page 5, Prop. 3]. This precludes the case ρ→∞ which is covered in Theorem 6.5.
We end this section by finding boundedness estimates for higher order derivatives of the first Bloch eigenvalue and eigenfunction in the dual parameter in the regime ρ→∞.
Theorem 6.5. For 1≤ρ<∞,
1. ||∂ej0ϕρ1||H1≲, \partial^{e_j}_0\lambda^\rho_1 = 0 .
2. ||\partial_0^{e_j+e_k}\phi^\rho_1||_{H^1}\lesssim\frac{1}{\rho^2} , \left|\partial^{e_j+e_k}_0\lambda^\rho_1-\mathcal{M}_Y(e_j\cdot Ae_k)\right|\lesssim\frac{1}{\rho^2} .
3. ||\partial_0^{e_j+e_k+e_l}\phi^\rho_1||_{H^1}\lesssim\frac{1}{\rho^2} , \partial^{e_j+e_k+e_l}_0\lambda^\rho_1 = 0 .
4. ||\partial_0^{\beta}\phi^\rho_1||_{H^1}\lesssim 1 for all |\beta|\geq 4 .
5. |\partial^{\beta}_0\lambda^\rho_1|\lesssim{\begin{cases} &\rho^2\;\mathit{for}\;|\beta| = 4.\\ &1\;\mathit{for}\;|\beta|>4.\\ \end{cases} } .
Proof. The estimates are computed in tandem from the equations (6.8) and (6.10). One begins by proving the estimate on the derivative of the first Bloch eigenfunction at \eta = 0 , followed by the estimate on the corresponding derivative of the first Bloch eigenvalue. The solvability of (6.8) in H^2_\sharp(Y) follows from a standard application of Lax-Milgram lemma. On the other hand, one can also read off from (6.8) that the solution \partial^\beta_0\phi^\rho_1\in H^3_\sharp(Y) . As a consequence, the estimate on the derivatives of the first Bloch eigenfunction are obtained by employing the test function \Delta_y\partial^\beta_0\phi^\rho_1 in (6.8) and repeated applications of Poincaré inequality. The computations are standard and therefore, omitted.
7.
Bloch transform and its properties
In this section, we will relate the Bloch spectral problem (2.2) to the Bloch spectral problem at the \varepsilon -scale:
Comparing to (2.2), by homothety and \kappa = \rho \varepsilon , we conclude that
Now, we can state the Bloch decomposition theorem of L^2(\mathbb{R}^d) at \varepsilon -scale. We shall normalize \phi^\rho_1(y;0) to be (2\pi)^{-d/2} .
Theorem 7.1. Let \rho >0 . Let g\in L^2(\mathbb{R}^d) . Define the m^{th} Bloch coefficient of g at \varepsilon -scale as
1. The following inverse formula holds
2. Parseval's identity
3. Plancherel formula For f,g\in L^2(\mathbb{R}^d) , we have
4. Bloch Decomposition in H^{-1}(\mathbb{R}^d) For an element F = u_0(x)+\sum_{j = 1}^N\frac{\partial u_j(x)}{\partial x_j} of H^{-1}(\mathbb{R}^d) , the following limit exists in L^2\left(\frac{Y^{'}}{ \varepsilon}\right) :
The definition above is independent of the particular representative of F .
5. Finally, for g\in D(\mathcal{A}^{\kappa, \varepsilon}) ,
7.1. First Bloch transform goes to Fourier transform
In order to compute the homogenization limit, we need to know the limit of Bloch Transform of a sequence of functions. The following theorem proves that for a sequence of functions convergent in a suitable way, the first Bloch transform converges to the Fourier transform of the limit.
Theorem 7.2. Let K\subseteq\mathbb{R}^d be a compact set and (g^ \varepsilon) be a sequence of functions in L^2(\mathbb{R}^d) such that g^ \varepsilon = 0 outside K . Suppose that g^ \varepsilon\rightharpoonup g in L^2(\mathbb{R}^d) -weak for some function g\in L^2(\mathbb{R}^d) . Then it holds that
in L^2_{\;\mathit{loc}\;}(\mathbb{R}^d_\xi) -weak, where \widehat{g} denotes the Fourier transform of g and 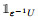 denotes the characteristic function of the set { \varepsilon^{-1}U} .
denotes the characteristic function of the set { \varepsilon^{-1}U} .
Proof. In Theorem 4.5, the existence of the set U indepedent of \rho was proved. The function \mathcal{B}_1^{\kappa, \varepsilon} g^ \varepsilon is defined for \xi\in \varepsilon^{-1}Y^{'} . However, we shall treat it as a function on \mathbb{R}^d by extending it outside \varepsilon^{-1}U by zero. We can write
Now, we need to distinguish between the regimes:
Case 1. \theta\in [0,\infty) : The first term above converges to the Fourier transform of g on account of the normalization of \phi_1(y;0) whereas the second term goes to zero since it is O( \varepsilon\xi) due to the Lipschitz continuity of the first regularized Bloch eigenfunction which follows from (4.3).
Case 2. \theta = \infty : In this case, again, the first term converges to the Fourier transform of g on account of the normalization of \phi_1(y;0) . However, for the second term, we make use of the analyticity of \phi_1^{\kappa, \varepsilon} in \varepsilon^{-1}U and the estimates in Theorem 6.5 to conclude that the second term is O( \varepsilon\xi) independent of \rho .
8.
Qualitative homogenization
In this section, we will prove the qualitative homogenization result for the singularly perturbed homogenization problem. There are three regimes according to convergence of \rho = \frac{\kappa}{ \varepsilon} , viz., \rho\to 0 , \rho\to\theta\in(0,\infty) and \rho\to\infty .
Theorem 8.1. Let \Omega be an arbitrary domain in \mathbb{R}^d and f\in L^2(\Omega) . Let u^ \varepsilon\in H^2(\Omega) be such that u^ \varepsilon converges weakly to u^* in H^1(\Omega) , \kappa\Delta u^ \varepsilon is uniformly bounded in L^2(\Omega) , and
where \kappa\to 0 as \varepsilon\to 0 and \lim_{ \varepsilon\to 0}\frac{\kappa}{ \varepsilon} = \theta\in [0,\infty] . Let A^{hom} = (a^*_{kl})_{k,l = 1}^d be as defined in Definition 5.3. Then
1. For all k = 1,2,\ldots,d , we have the following convergence of fluxes:
2. The limit u^* satisfies the homogenized equation:
Remark 8.2. In the spirit of H-convergence [24], we do not impose any boundary condition on the equation. The H-convergence compactness theorem concerns convergence of sequences on which certain differential constraints have been imposed. In homogenization, the weak convergence of solutions is a consequence of uniform bounds on them, which follow from boundary conditions imposed on the equation. In the theorem quoted above, the uniform boundedness on \kappa\Delta u^ \varepsilon would have followed if appropriate boundary conditions were imposed.
The proof of Theorem 8.1 is divided into the following steps. We begin by localizing the equation (8.1) which is posed on \Omega , so that it is posed on \mathbb{R}^d . We take the first Bloch transform \mathcal{B}^{\kappa, \varepsilon}_1 of this equation and pass to the limit \kappa, \varepsilon\to 0 . The proof relies on the analyticity of the first Bloch eigenvalue and eigenfunction in a neighborhood of 0\in Y^{'} . The limiting equation is an equation in Fourier space. The homogenized equation is obtained by taking the inverse Fourier transform. We will use the notation a_{kl}^ \varepsilon(x) to denote a_{kl}\left(\frac{x}{ \varepsilon}\right) . Further, we will assume the Einstein convention of summing over repeated indices. The proof has been divided into separate cases for the regimes \rho\to\theta\in(0,\infty) , \rho\to\infty , and \rho = 0 .
8.1. Localization
Let \psi_0 be a fixed smooth function supported in a compact set K\subset\mathbb{R}^d . Since u^ \varepsilon satisfies \mathcal{A}^{\kappa, \varepsilon} u^ \varepsilon = f , \psi_0 u^ \varepsilon satisfies
where
While the sequence g^ \varepsilon is bounded in L^2(\mathbb{R}^d) , the sequence h^{ \varepsilon} is bounded in H^{-1}(\mathbb{R}^d) . Taking the first Bloch transform of both sides of the equation (8.4), we obtain for \xi\in \varepsilon^{-1}U a.e.
We shall now pass to the limit \kappa, \varepsilon\to 0 in the equation (8.11).
8.2. \underline{{\rm{Case}} 1 : \rho\to\theta\in(0,\infty)}
8.2.1. Limit of \lambda^{\kappa, \varepsilon}_1(\xi)\mathcal{B}^{\kappa, \varepsilon}_1(\psi_0 u^ \varepsilon)
We expand the first Bloch eigenvalue about \eta = 0 in \lambda^{\kappa, \varepsilon}_1(\xi)\mathcal{B}^{\kappa, \varepsilon}_1(\psi_0 u^ \varepsilon) to write
The higher order derivatives of \lambda^\rho_1(\eta) are bounded uniformly in \rho (see Remark 6.4). Hence, their contribution is O( \varepsilon^2) . Now, we can pass to the limit \kappa, \varepsilon\to 0 in L^2_{\rm{loc}}(\mathbb{R}^d_\xi) -weak by applying Lemma 7.2 to obtain:
8.2.2. Limit of \mathcal{B}^{\kappa, \varepsilon}_1(\psi_0 f)
An application of Lemma 7.2 yields the convergence of \mathcal{B}^{\kappa, \varepsilon}_1(\psi_0 f) to (\psi_0 f)^{\bf\widehat{}} in L^2_{\rm{loc}}(\mathbb{R}^d_\xi) -weak.
8.2.3. Limit of \mathcal{B}^{\kappa, \varepsilon}_1g^ \varepsilon
The sequence g^ \varepsilon as defined in (8.5) is bounded in L^2(\mathbb{R}^d) and hence has a weakly convergent subsequence with limit g^*\in L^2(\mathbb{R}^d) . This sequence is supported in a fixed set K . Also, note that the sequence \sigma_k^ \varepsilon(x):= a^ \varepsilon_{kl}(x)\frac{\partial u^ \varepsilon}{\partial x_l}(x) is bounded in L^2(\Omega) , hence has a weakly convergent subsequence whose limit is denoted by \sigma^*_k for k = 1,2,\ldots,d . Extend \sigma^*_k by zero outside \Omega and continue to denote the extension by \sigma^*_k . Thus, g^* is given by -\frac{\partial \psi_0}{\partial x_k}\sigma^*_k . Therefore, by Lemma 7.2, we obtain the following convergence in L^2_{\rm{loc}}(\mathbb{R}^d_\xi) -weak:
8.2.4. Limit of \mathcal{B}_1^{\kappa, \varepsilon}h^{ \varepsilon}
We have the following weak convergence in L^2_{\rm{loc}}(\mathbb{R}^d_{\xi}) .
We shall prove this in the following steps.
Step 1. By the definition of the Bloch transform (7.7) for elements of H^{-1}(\mathbb{R}^d) , we have
Step 2. The first term on RHS of (8.15) is the Bloch transform of the expression -i\xi_k\frac{\partial \psi_0}{\partial x_l}(x)a^{ \varepsilon}_{kl}(x)u^ \varepsilon(x) which converges weakly to -i\xi_k\mathcal{M}_Y(a_{kl})\left(\frac{\partial \psi_0}{\partial x_l}(x)u^*(x)\right) .
Step 3. Now, we analyze the second term on RHS of (8.15). To this end, we make use of analyticity of first Bloch eigenfunction with respect to the dual parameter \eta near 0 . We have the following power series expansion in H^1_\sharp(Y) for \phi_1^\rho(\eta) about \eta = 0 :
We know that \gamma^\rho(y;0) = 0 and (\partial \gamma^\rho/\partial \eta_s)(y;0) = 0 , therefore, \gamma^\rho(\cdot;\eta) = O(|\eta|^2) in L^\infty(U;H^1_\sharp(Y)) . We also have (\partial\gamma^\rho/\partial y_k)(\cdot;\eta) = O(|\eta|^2) in L^\infty(U;L^2_\sharp(Y)) . These orders are uniform in \rho by Remark 6.4. Now,
Differentiating the last equation with respect to x_k , we obtain
For \xi belonging to the set \{\xi: \varepsilon\xi\in U{\rm{ and }}|\xi|\leq M\} , we have
As a consequence,
The second term on the RHS of (8.15) is given by
Substituting (8.18) in (8.21), we obtain
In the last expression, the term involving \gamma^\rho goes to zero as \varepsilon\to 0 in view of (8.19), whereas the other term has the following limit as \rho\to\theta\in(0,\infty) :
To see this, we write the second term as
The first term in parantheses goes to (8.23) due to strong convergence of u^ \varepsilon in L^2(K) and weak convergence of a_{kl}\frac{\partial \chi^{\theta}_s}{\partial y_k}\left(\frac{x}{ \varepsilon}\right) , whereas the expression in the square brackets goes to zero due to Lemma 5.1.
Step 4. By Theorem 6.1 and Remark 6.2, it follows that
Therefore, we have the following convergence in L^2_{\rm{loc}}(\mathbb{R}^d_\xi) -weak:
8.2.5. Limit of \mathcal{B}_1^{\kappa, \varepsilon}l_1^{\kappa, \varepsilon}
We shall prove that
Observe that
The integral is the Bloch transform of \frac{\partial^4\psi^0}{\partial x_k^4}(x)u^ \varepsilon(x) which converges to the Fourier transform of \frac{\partial^4\psi^0}{\partial x_k^4}(x)u^*(x) . However, since \kappa\to 0 , the whole expression goes to zero.
8.2.6. Limit of \mathcal{B}_1^{\kappa, \varepsilon}l_2^{\kappa, \varepsilon}
We shall prove that
Observe that
The integral is the Bloch transform of \frac{\partial^3\psi^0}{\partial x_k^3}(x)\frac{\partial u^ \varepsilon}{\partial x_k}(x) which converges to the Fourier transform of \frac{\partial^3\psi^0}{\partial x_k^3}(x)\frac{\partial u^*}{\partial x_k}(x) . However, since \kappa\to 0 , the whole expression goes to zero.
8.2.7. Limit of \mathcal{B}_1^{\kappa, \varepsilon}l_3^{\kappa, \varepsilon}
We shall prove that
Observe that
The integral is the Bloch transform of \frac{\partial^2\psi^0}{\partial x_k^2}(x)\kappa\frac{\partial^2 u^ \varepsilon}{\partial x_k^2}(x) which converges for a subsequence since \kappa\frac{\partial^2 u^ \varepsilon}{\partial x_k^2}(x) is bounded in L^2(\Omega) (and hence converges weakly in L^2(\Omega) for a subsequence). However, since \kappa\to 0 , the whole expression goes to zero.
8.2.8. Limit of \mathcal{B}_1^{\kappa, \varepsilon}l_4^{\kappa, \varepsilon}
We shall prove that
Observe that
belongs to H^{-1}(\mathbb{R}^d) , hence the Bloch trasform for H^{-1}(\mathbb{R}^d) , that is, (7.7) applies. Hence,
The analysis of the first term is the same as that of \mathcal{B}_1^{\kappa, \varepsilon}l_3^{\kappa, \varepsilon} . For the second term, observe that \kappa\frac{\partial^2 u^ \varepsilon}{\partial x_k^2}(x) is bounded in L^2(\Omega) and \frac{\partial{\phi^{\rho}_1}}{\partial x_k}\left(\frac{x}{ \varepsilon}; \varepsilon\xi\right) is bounded in L^2_\sharp( \varepsilon Y) uniformly in \rho (see Remark 6.4). Hence, their product is bounded in L^1(K) . As a result, the integral is a Fourier transform of a sequence bounded in \rho and \varepsilon . However, the presence of \kappa in front of the integral causes the expression to go to zero.
Finally, passing to the limit in (8.11) as \varepsilon\to 0 by applying equations (8.12), (8.13), (8.14), (8.26), (8.28), (8.30), and (8.32), we get:
8.3. \underline{{\rm{Case}} 2 : \rho\to\infty }
In this regime, the convergence proofs for \mathcal{B}_1^{\kappa, \varepsilon}l_1^{\kappa, \varepsilon} , \mathcal{B}_1^{\kappa, \varepsilon}l_2^{\kappa, \varepsilon} , \mathcal{B}_1^{\kappa, \varepsilon}l_3^{\kappa, \varepsilon} , \mathcal{B}_1^{\kappa, \varepsilon}g^{ \varepsilon} , \mathcal{B}_1^{\kappa, \varepsilon}(\psi_0 f) are the same as in the earlier regime. Therefore, we will only look at the remaining convergences.
8.3.1. Limit of \lambda^{\kappa, \varepsilon}_1(\xi)\mathcal{B}^{\kappa, \varepsilon}_1(\psi_0 u^ \varepsilon)
We expand the first Bloch eigenvalue about \eta = 0 in \lambda^{\kappa, \varepsilon}_1(\xi)\mathcal{B}^{\kappa, \varepsilon}_1(\psi_0 u^ \varepsilon) to write
The fourth order derivative is of order \rho^2 by Theorem 6.5. The derivatives of \lambda^\rho_1(\eta) of order greater than 4 are bounded uniformly in \rho (see Theorem 6.5). Hence, their contribution is O( \varepsilon^4) . Hence, we can write the above as
Now, we can pass to the limit \kappa, \varepsilon\to 0 in L^2_{\rm{loc}}(\mathbb{R}^d_\xi) -weak by applying Lemma 7.2 to obtain:
8.3.2. Limit of \mathcal{B}_1^{\kappa, \varepsilon}h^{ \varepsilon}
We shall prove this in the following steps.
Step 1. As before, we have
Step 2. As before, the first term on RHS of (8.35) is the Bloch transform of the expression -i\xi_k\frac{\partial \psi_0}{\partial x_l}(x)a^{ \varepsilon}_{kl}(x)u^ \varepsilon(x) which converges weakly to
where the last equality is due to Definition 5.3.
Step 3. Now we shall prove that the second term on RHS of (8.35) goes to zero. As before, we make use of analyticity of first Bloch eigenfunction with respect to the dual parameter \eta near 0 . We have the following power series expansion in H^1_\sharp(Y) for \phi_1^\rho(\eta) about \eta = 0 :
We know that \gamma^\rho(y;0) = 0 and (\partial \gamma^\rho/\partial \eta_s)(y;0) = 0 , therefore, \gamma^\rho(\cdot;\eta) = O(|\eta|^2) in L^\infty(U;H^1_\sharp(Y)) . We also have (\partial\gamma^\rho/\partial y_k)(\cdot;\eta) = O(|\eta|^2) in L^\infty(U;L^2_\sharp(Y)) . These orders are uniform in \rho by Theorem 6.5. Now,
Differentiating the last equation with respect to x_k , we obtain
For \xi belonging to the set \{\xi: \varepsilon\xi\in U{\rm{ and }}|\xi|\leq M\} , we have
As a consequence,
The second term on the RHS of (8.35) is given by
Substituting (8.36) in (8.38), we obtain
In the last expression, the term involving \gamma^\rho goes to zero as \varepsilon\to 0 in view of (8.37).
The other term also goes to zero as \rho\to\infty due to strong convergence of u^ \varepsilon and the fact that
as shown in Theorem 6.5.
8.3.3. Limit of \mathcal{B}_1^{\kappa, \varepsilon}l_4^{\kappa, \varepsilon}
The analysis is the same as before, however the uniform-in- \rho boundedness of \frac{\partial{\phi^{\rho}_1}}{\partial x_k}\left(\frac{x}{ \varepsilon}; \varepsilon\xi\right) in L^2_\sharp( \varepsilon Y) is due to Theorem 6.5.
Hence, for the regime \rho\to\infty , we also recover (8.34).
8.4. \underline{{\rm{Case}} 3 : \rho\to 0 }
In this regime, all the convergence proofs are the same as in the regime \rho\to\theta\in(0,\infty) except for the convergence of \mathcal{B}_1^{\kappa, \varepsilon}h^{ \varepsilon} , which we prove below.
8.4.1. Limit of \mathcal{B}_1^{\kappa, \varepsilon}h^{ \varepsilon}
For this limit, all steps except the third are the same, hence we only explain the part of Step 3 which differs from the regime \rho\to\theta\in(0,\infty) . We begin with the following equation which was earlier labelled as (8.22).
In the last expression, the term involving \gamma^\rho goes to zero as \varepsilon\to 0 in view of (8.19), whereas the other term has the following limit as \rho\to 0 :
To see this, we write
The first term I is responsible for (8.41) due to strong convergence of u^ \varepsilon in L^2(K) and weak convergence of a_{kl}\frac{\partial \chi^0_s}{\partial y_k}\left(\frac{x}{ \varepsilon}\right) .
For the expressions II and III , we make use of Lemma 5.2. Indeed, we obtain II = O(\rho)+O(\varkappa) and III = O(\varkappa) . Hence, their contribution to the limit in (8.40) as \rho\to 0 is O(\varkappa) .
This completes the modification required for the regime \rho\to 0 . Instead of (8.34), we obtain
However, since \varkappa>0 is an arbitrary positive number in Lemma 5.2, we also recover (8.34) for the regime \rho\to 0 .
8.5. Proof of the homogenization result
Taking the inverse Fourier transform in the equation (8.34), we obtain the following:
where the operator \mathcal{A}^{hom} is defined in (8.3). At the same time, calculating using Leibniz rule, we have:
Using equations (8.42) and (8.43), we obtain
Let \omega be a unit vector in \mathbb{R}^d , then \psi_0(x)e^{ix\cdot\omega}\in\mathcal{D}(\Omega) . On substituting in the above equation, we get, for all k = 1,2,\ldots,d and for all \psi_0\in\mathcal{D}(\Omega) ,
Let x_0 be an arbitrary point in \Omega and let \psi_0(x) be equal to 1 near x_0 , then for a small neighbourhood of x_0 :
However, x_0\in\Omega is arbitrary, so that
Thus, we have obtained the limit equation in the physical space. This finishes the proof of Theorem 8.1.
9.
Contribution of higher modes
The proof of the qualitative homogenization theorem only requires the first Bloch transform. It is not clear whether the higher Bloch modes make any contribution to the homogenization limit. In this section, we show that they do not. We know that Bloch decomposition is the isomorphism L^2(\mathbb{R}^d)\cong L^2(Y^{'}/ \varepsilon;\ell^2(\mathbb{N})) which is reflected in the inverse identity (7.4). For simplicity, take \Omega = \mathbb{R}^d and consider the equation \mathcal{A}^{\kappa, \varepsilon} u^ \varepsilon = f in \mathbb{R}^d which is equivalent to
We claim that one can neglect all the equations corresponding to m\geq 2 .
Proposition 9.1. Let
then ||v^{\kappa, \varepsilon}||_{L^2(\mathbb{R}^d)}\leq c \varepsilon , where c does not depend on \rho and \varepsilon .
Proof. Due to boundedness of the sequence (u^ \varepsilon) in H^2(\mathbb{R}^d) , we have
However, by Plancherel Theorem (7.6), we have
Using (7.8), we have
Now, by Lemma 4.2
where \lambda^N_2 is the second eigenvalue of Laplacian on Y with Neumann boundary condition on \partial Y . Since \lambda^{\kappa, \varepsilon}_m(\xi) = \varepsilon^{-2}\lambda^{\rho}_m( \varepsilon\xi) , we obtain
By Parseval's identity (7.5), the LHS equals ||v^{\kappa, \varepsilon}||^2_{L^2(\mathbb{R}^d)} . This completes the proof.
Acknowledgments
The author gratefully acknowledges the careful examination of the editor and the anonymous referees. The author would also like to thank Ayan Roychowdhury for a discussion about formation of shear bands.









 .
.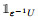 denotes the characteristic function of the set
denotes the characteristic function of the set 



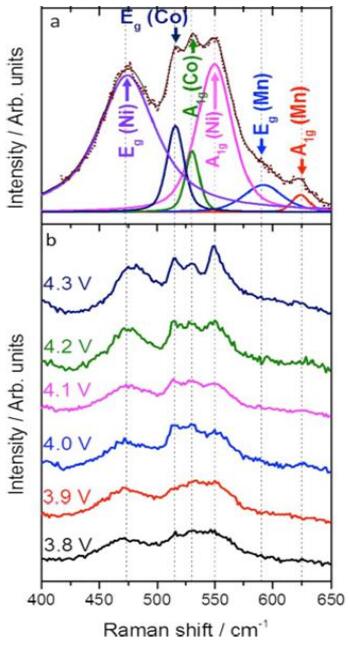

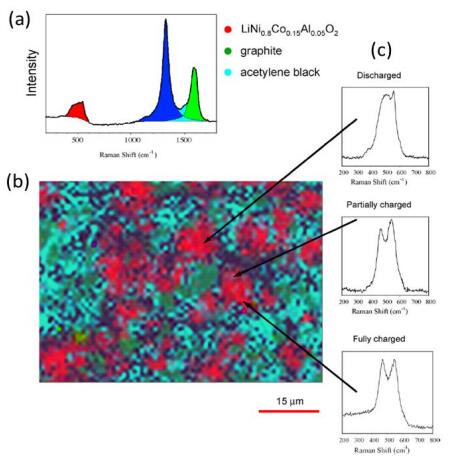
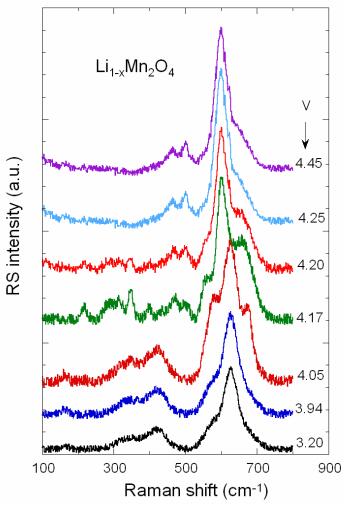
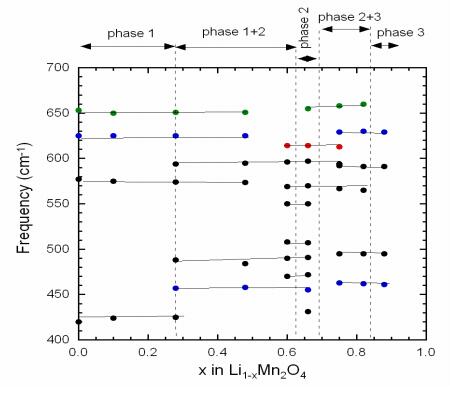


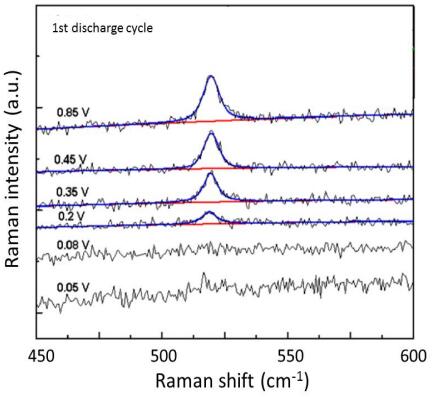

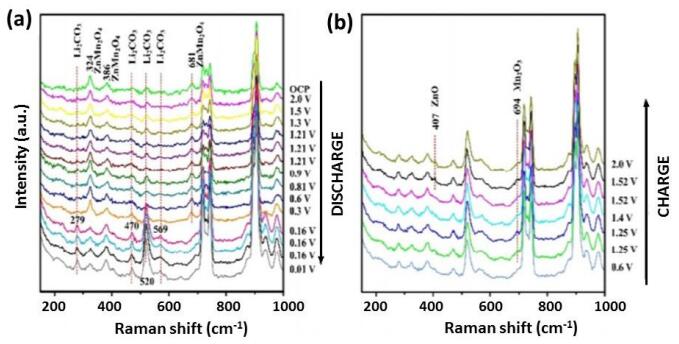


 DownLoad:
DownLoad: