Application of intelligent X-ray image analysis in risk assessment of osteoporotic fracture of femoral neck in the elderly

1.   Introduction

As of April 2021, the coronavirus disease (COVID-19) continues to spread in Japan. On June 19, 2020, the Japanese government released the COVID-19 Contact-Confirming Application (COCOA), a smartphone app ^[1] that was developed to let COCOA users know whether they had come into contact with any COVID-19 infectors. The contact notification process comprises the following steps:

1. An individual with a pre-existing severe acute respiratory distress syndrome coronavirus 2 (COVID-19) infection and an uninfected individual both install COCOA on their smartphones (the infector is unaware of the infection because he/she has not undergone the reverse transcription polymerase chain reaction (RT-PCR test) for COVID-19).

2. The uninfected individual is in the proximity (<1 m) of the individual with a pre-existing COVID-19 infection for 15 minutes or longer. Through this process, the uninfected person becomes a new infector.

3. At a later date, the individual with the pre-existing COVID-19 infection who is the source of infection undergoes an RT-PCR test.

4. In the case of a positive result for COVID-19, the individual with a pre-existing COVID-19 infection who is the source of infection registers this result in the COCOA.

5. Then, individuals who came into contact with the individual with a pre-existing COVID-19 infection who is the source of infection within less than 2 weeks from the date of the positive result get a contact notification via COCOA.

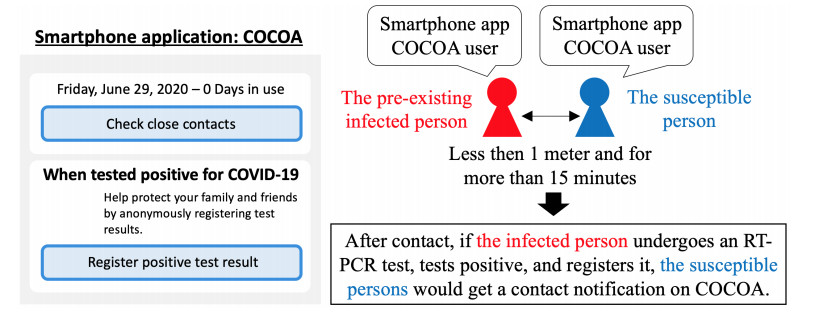
The display of the COCOA is shown in Figure 1. If COCOA users click on the "check close contacts" button to the top-left, they receive information on whether they had any contact with the infectors. Infectors who become aware of a positive RT-PCR test result can register this result by using the lower "Register positive test result" button. Through these features, COCOA users can become aware of the possibility of infection through close contact with infectors with a pre-existing COVID-19 infection. If these exposed individuals decrease venturing outside and initiate self-quarantine, the number of total infectors in the community will decrease.

Worldwide, apps that are similar to COCOA have been developed (e.g., England ^[2], China, India, and Israel ^[3]) as various countries consider that the contact notification function is important for overcoming COVID-19. There are some important parameters that can decrease the number of new infectors as a function of COCOA. It is essential that the pre-existing infector and the close contact both use COCOA; therefore, the usage rate of COCOA is an important parameter (the term "close contact" refers to a person who has been in close contact with a pre-existing COVID-19 infector). Moreover, because the condition by which COCOA ascertains a positive contact with a pre-existing infector is contact for 15 minutes or longer at a distance of less than 1 m, the rate of new infectors who meet the abovementioned condition is important. Individuals who have undergone an RT-PCR test and have received positive results only could register their result in the COCOA. Therefore, the rate of PCR testing and the false-negative rate of PCR tests are important parameters. Furthermore, the duration of delay from the time of infection to undergoing an RT-PCR test is important. If the delay is too long, the close contact would not receive the "infector contact" notification from COCOA for a long duration. If the close contact becomes an infector through contact with pre-existing infectors, he/she may infect another individual. Moreover, COCOA cannot send the contact notification to a close contact if the infector who is the source of infection does not register a positive result in COCOA, even if he/she has received a positive result on the RT-PCR test and is aware of the infection. Therefore, the rate of registration of positive results is an important parameter. If the pre-existing infector who was the source of infection had maintained appropriate correspondence (using COCOA, getting the RT-PCR test done, registering positive results, and so on), the close contact can receive contact notification from COCOA. However, if a person in close contact does not undertake self-quarantine, the effectiveness of COCOA in decreasing the number of COVID-19 infectors does not occur. Therefore, the rate of self-quarantine of individuals who receive contact notifications from COCOA is another important parameter. Thus, there are some important parameters to estimate the effectiveness of COCOA.

Simulation is a desirable approach to clarify the effectiveness of COCOA. Many studies on infection have used simulation (e.g., ^[4,5,6,7]). Recently, COVID-19 infection simulations have been reported from target countries, such as China ^[8,9,10], India ^[11], The Netherlands ^[12], Korea, Italy, France ^[13], Nigeria ^[14], Arizona ^[15], Australia ^[16], South Carolina ^[17], Scandinavia ^[18], and Brazil ^[19,20]. Moreover, Niwa et al. reported the effectiveness of airport quarantine ^[21]. Kurahashi et al. reported the effectiveness of remote working and staggered commuting ^[22]. Vasconcelos et al. ^[23] and Macêdo et al. ^[24] analyzed the fatality curve of COVID-19 infectors by the simulation approach.

Recently, simulation studies have been conducted to estimate the effectiveness of contact-tracing apps. Various approaches ^{[25,26,27,28,29]} have been evaluated; however, the target of these researches were not COCOA. As an exception, Omae et al. ^[30] verified the effectiveness of COCOA using multi-agent simulation (MAS). However, because the calculation cost of MAS is high, this simulation scale is small (approximately 1000 agents), and undertaking trial and error in the various scenarios is difficult. Moreover, Omae et al. ^[30] did not consider the rate of RT-PCR test results and false negative rates. Kurita et al. ^[31] reported the effectiveness of COCOA in reducing the number of infectors; however, they did not consider the rate of registration of positive results and the false negative rate of RT-PCR tests.

Against this background, we developed a Susceptible-Infected-Removed (COCOA-SIR) model to estimate the effectiveness of COCOA in decreasing infectors to simulate infection spread in Japan. The COCOA-SIR model has the following important parameters for estimating the effect of COCOA.

1. the usage rate of COCOA

2. the rate of new infectors who are infected by contact with other infectors of less than 1 m and for 15 minutes or longer.

3. the rate of undergoing RT-PCR test

4. the delay from infection timing to undergoing an RT-PCR test

5. the false negative rate of RT-PCR test

6. the rate of registration of a COVID-19 positive result

7. the rate of self-quarantine of individuals who receive the contact notification from COCOA

The SIR model is used for infection epidemic simulation of COVID-19 (e.g., ^{[32,33,34,35]}) and, therefore, we adopted the SIR model as our research approach.

2.   Basic SIR model

The basic SIR model ^[36] is represented as,

where $S(t), I(t), R(t)$ are the number of susceptible (not infected), infected, and removed individuals at time $t$, respectively. "Removed persons" means individuals who are recovering from a COVID-19 infection or those who died of the infection. $\beta(t)$ is the infectivity of time $t$, and $\gamma$ is the recovery rate. If people in the community are careful about preventing infection, then the infectivity is low. However, if individuals in the community are not careful about infection, then the infectivity is high. The degree of care differs based on various situations. Therefore, $\beta(t)$ is the function of time $t$.

Based on the above-described differential equations of Equations (2.1)-(2.3), we can understand the infection spread dynamics continuously. However, the number of new COVID-19 infectors is reported once a day in Japan. Therefore, it is desirable to express this parameter through a discrete-time equation rather than via a differential equation. Thus, we use,

as discrete-time equations for simulating infection spread. Where $S_t$, $I_t$, and $R_t$ are the numbers of susceptible (not infected), infected, and removed persons on day $t$, respectively. $\beta'_t$ is the infectivity on day $t$, and $\gamma'$ is the recovery rate per day. Assuming that the infection period follows exponential distribution, the recovery rate $\gamma'$ is the reciprocal of the average infection period ^[37,38]. For example, if the average infection period is 7 days, $\gamma'$ is $1/7$. Moreover, it is necessary to set $S_0$, $I_0$, and $R_0$.

In Equation (2.4), the first term is the number of susceptible individuals on day $t$, and the second term represents the number of individuals who are susceptible to virus infection from day $t$ to day $t+1$. In Equation (2.5), the first term is the number of infected persons on day $t$, the second term is the number of newly infected persons from day $t$ to day $t+1$, and the third term represents the number of infected persons who are excluded due to recovery following virus infection (or death) from day $t$ to day $t+1$. In Equation (2.6), the first term is the number of recovered individuals on day $t$, and the second term represents the number of newly recovered persons based on recovery from COVID-19 infection (or death) from day $t$ to day $t+1$.

An effective reproduction number is an important index for the virus-spread simulation. The effective reproduction number refers to the number of additional persons infected by an infected person during the entire infection period ^[39]. In Equation (2.5), an infector infects $\beta'_tS_t$ persons per day, and the average infection period is $(\gamma')^{-1}$. Therefore, we can define the effective reproduction number $r_t$ on day $t$ as

If the effective reproduction number $r_t > 1$, then $\beta'_t S_t I_t - \gamma'I_t > 0$. Therefore, $I_{t+1}$ is larger than $I_t$. In contrast, if the effective reproduction number is $r_t < 1$, $I_{t+1}$ is less than $I_t$. For decreasing $r_t$, it is required to decrease $\beta'_t$. To decrease $\beta'_t$, it is important to wear a mask, disinfect fingers, decrease going out, and so on.

3.   COCOA-SIR model

With regard to the originality of our research, we propose the COCOA-SIR model, which can represent the effectiveness of the COCOA developed by Japan. After this section, we use two variables $i, t$ to represent "day" $i$ represents the timing of the occurrence of a new infector. $t$ is a variable that represents the current day.

3.1.   The effectiveness of COCOA on decreasing infectors

By the second term of Equation (2.5) of the basic SIR model, the number of new infectors occurring from day $t$ to day $t+1$ is defined by the value obtained by multiplying the number of infectors $I_t$ on day $t$ and $\beta'_t S_t$. Thus, the infector $I_t$ on day $t$ generates new infectors. If COCOA is present in the community, we consider that a percentage of individuals who receive notification of contact with infectors from COCOA would undertake self-quarantine. Here, the number of infectors who receive notifications of contact with infectors through COCOA and undertake self-quarantine is defined as $I^\mathrm{notice}_t$. Then, the number of infectors that infect other persons on day $t$ is $I_t - I^\mathrm{notice}_t$ instead of $I_t$. Therefore, in the case of existing COCOA in society, the number of infectors on day $t+1$ is defined by

This means that the infectors $I_t - I^\mathrm{notice}_t$ who did not receive a COCOA notification of contact with infectors (or did not take self-quarantine in spite of receiving it) would generate new infectors.

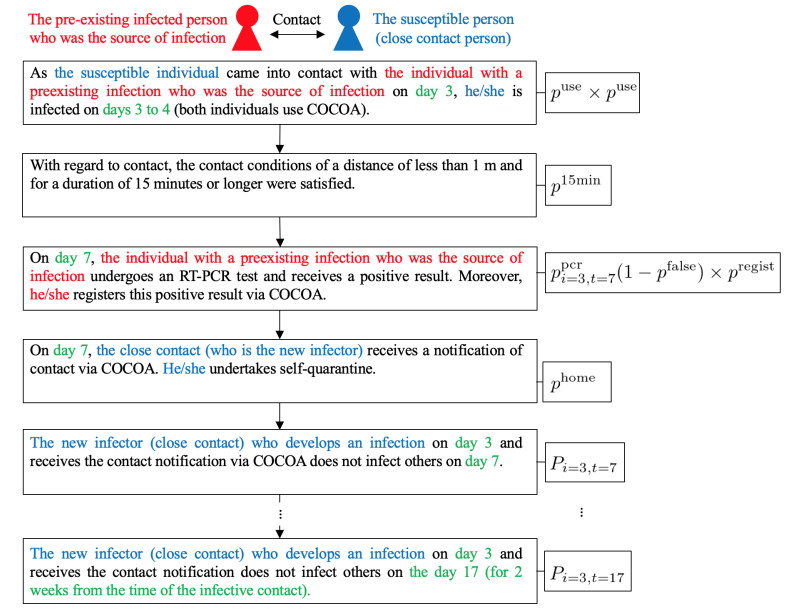
Herein, we consider the details of $I^\mathrm{notice}_t$. We explain the process flow until the new infectors receive a COCOA notification of contact, based on Figure 2. This is an example of how a newly infected person reproduces the infection by contact with an individual with a preexisting infection from days 3 to 4. In this case, to send the contact notification via COCOA, both individuals need to use COCOA and satisfy the condition of contact of COCOA ( < m and $\geq$ minutes). Moreover, an individual with a preexisting infection needs to undergo an RT-PCR test and to obtain a positive result, as well as input the fact into COCOA. In the case shown in Figure 2, on day 7, an individual with the preexisting infection fulfills these conditions. At this time, an individual who is newly infected from days 3 to 4 can receive a contact notification via COCOA. If a newly infected individual who receives the contact notification appropriately undertakes self-quarantine, he/she will not infect other individuals after day 7. With regard to the function of COCOA ^[1], the app retains the notification-related information for 14 days after the contact with an individual with a preexisting infection. Therefore, an individual who is newly infected from days 3 to 4 receives the notification via COCOA until day 17.

Based on the abovementioned discussions, it is necessary to fulfill various conditions to ensure the effectiveness of COCOA in decreasing new infectors. Furthermore, the timing when the infected individual receives the contact notification through COCOA is the time at which the individual with the preexisting infection undergoes the RT-PCR test, receives a positive test result, and inputs this result into COCOA. Therefore, the probability that a newly infected individual who appears on day $i$ will receive a contact notification on day $t$, and will not infect others from day $t$ to day $t+1$ is defined by

Where, $p^\mathrm{use}$ is the usage rate of COCOA, $p^\mathrm{15 min}$ is the rate of satisfying the conditions of infective contact of more than 15 minutes at a distance less than 1 m, $p^\mathrm{false}$ is the false-negative rate of the RT-PCR test. $p^\mathrm{regist}$ is the rate of registration of a COVID-19-positive result, and $p^\mathrm{home}$ is the rate of self-quarantine of a person who receives a contact notification via COCOA (shown in Table 1). There are two $p^\mathrm{use}$ in Equation (3.2) because both an individual with a preexisting infection and a close contact use COCOA. The period of sending the contact notification was 14 days from the contact and time of infection ^[1]. Therefore, if the current day $t$ is more than 14 days from day $i$, $P_{i, t}$ is zero.

Next, we explain $p^\mathrm{pcr}_{i, t}$ in Equation (3.2). As a function of COCOA, the timing of sending the contact notification to the close contact is not the timing of contact with the infected person who is the source of infection (it is not on day $i$). The timing when the close contact can receive the contact notification is when the infected person who is the source of infection registers a positive result on the RT-PCR test in COCOA. To represent the described delay time, we define the probability of the individual with the preexisting infection obtaining an RT-PCR test on day $t$ (who is the source of a new infected person occurring on day $i$) as

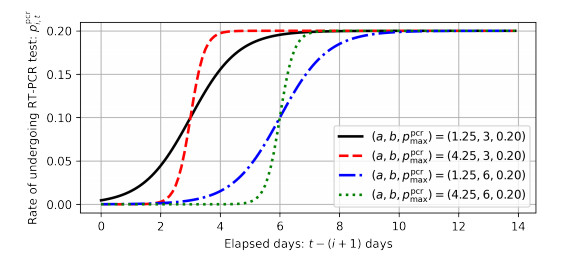
where, $p^\mathrm{pcr}_\mathrm{max}$ is the maximum probability of the individual undergoing an RT-PCR test. As it is a sigmoid function, $p^\mathrm{pcr}_{i, t} \in (0, p^\mathrm{pcr}_\mathrm{max})$. where the parameter $a$ is the gradient of the sigmoid function and the parameter $b$ is the number of days that half of the infected individuals of $p^\mathrm{pcr}_\mathrm{max}$ undergo the RT-PCR test. For example, we show the results for $p^\mathrm{pcr}_\mathrm{max} = 0.2$ and $(a, b) = (1.25, 3), (4.25, 3), (1.25, 6), (4.25, 6)$ in Figure 3. The horizontal axis $t-(i+1)$ represents the number of days elapsed from the infection timing (day $i$) of individuals in close contact. Because $t = i+1$ is the next day of the infection timing by contact with infectors, $p^\mathrm{pcr}_{i, t = i+1}$ is a small value. The reason is that we assume the rate of undergoing RT-PCR test gradually increases depending on the elapsed time. We can understand that the larger the $a$ is, the larger is the gradient of $p^\mathrm{pcr}_{i, t}$. Moreover, $b$ is the number of days that half of the infected persons of $p^\mathrm{pcr}_\mathrm{max}$ undergo the RT-PCR test. As the parameters $a, b$ represent the delay time from infection timing to the RT-PCR test, we call $a, b$ the delaying parameters. For your information, $p^\mathrm{pcr}_{i, t}$ is not more than $p^\mathrm{pcr}_\mathrm{max}$. Therefore, in the case of Figure 3 ($p^\mathrm{pcr}_\mathrm{max} = 0.2$), 80% of individuals with preexisting infection who were the source of infection did not undergo an RT-PCR test regardless of how much time had elapsed. Moreover, there is a problem in that the RT-PCR test is negative even if the individual is infected (false negative). In this case, because infected persons cannot be aware of infection, they cannot register positive results into COCOA even if they are infected. As the false negative rate is $p^\mathrm{false}$, the $1-p^\mathrm{false}$ in Equation (3.2) is the correct positive rate.

We consider $I^\mathrm{notice}_t$, which is the number of infectors who receive contact notifications on day $t$ based on the above-described discussions. We define the number of new infectors occurring from day $i$ to day $i+1$ as $I^{+}_i$. As this is the second term of Equation (3.1), $I^{+}_i$ is

Here, $I^{+}_i$, which is the infectors generated from day $i$ to day $i+1$, and is recovered by the rate $\gamma'$ per day. Therefore, the number of unrecovered infected persons on day $t$ is,

In the case of COCOA existing in the community, some of $I^{+}_i (1-\gamma')^{t-(i+1)}$ will receive a contact notification on COOCA. As it is the rate of satisfying the event, as shown in Figure 2, the number of infectors who have received contact notifications on COCOA is

In other words, the number of persons who were infected on day $i$ and have not recovered from infection by day $t$ and have received the contact notification is $P_{i, t} I^{+}_i (1-\gamma')^{t-(i+1)}$.

These abovementioned discussions indicate only new infectors who are generated from day $i$ to day $i+1$. As of day $t$, these new infectors exist for $t-1$ days. Therefore, as of day $t$, the number of new infectors who have not recovered from infection and have received the contact notification in COCOA and will not infect others by undertaking self-quarantine is

Note that, by using Equation (3.2), the summation range of Equations (3.7) and (3.8) can also be from $t-14$ to $t-1$ instead of from $0$ to $t-1$ when satisfying $t \geq14$. In Equation (3.8), $I^\mathrm{notice}_ t$ includes $I^\mathrm{notice}_ {\{0, \; \cdots, \; t-1\}}$. Therefore, we cannot calculate $I^\mathrm{notice}_0$. As $I^\mathrm{notice}_0$ denotes the number of infectors who have received contact notification on COCOA on day 0, we set

In other words, we can set $I^\mathrm{notice}_0$ to any number on the simulation starting time. If there is no particular reason, set $I^\mathrm{notice}_0$ to 0. This means that there are no individuals who have received contact notifications at the simulation starting time. By substituting $I^\mathrm{notice}_t$ calculated by Equation (3.8) for Equation (3.1), we can calculate the infectors $I_{t+1}$ on day $t+1$ by considering the effect of COCOA on decreasing the number of infectors.

Thus, we arrange Equation (3.1) as,

Comparing Equation (2.5) of the basic SIR model and Equation (3.10) of the proposed SIR model, the first, second, and third terms are the same, and the fourth term is then added. In other words, we consider the fourth term $\beta'_t S_t I^\mathrm{notice}_t$ of Equation (3.10) as the effect of COCOA on decreasing the number of new infectors. Therefore, we define the effect of COCOA in decreasing the number of new infectors on day $t$ to day $t+1$ as

This indicates that the effect of COCOA is affected not only by $P_{i, t}$ but also by various variables.

3.2.   Proposed SIR model

Based on the above discussion, to estimate the effect of COCOA in decreasing the number of infectors, we propose the COCOA-SIR model, defined as

Equation (3.14) to estimate the number of infectors $I_{t+1}$ is obtained by substituting $E^\mathrm{app}_t$ defined by Equation (3.11) for Equation (3.10). Moreover, because the number of new infectors decreases by $E^\mathrm{app}_t$, Equation (3.13) to estimate the number of susceptible persons $S_{t+1}$ has the term $E^\mathrm{app}_t$. The number of recovered persons $R_{t+1}$ is the same as in the basic SIR model. Here, when we set the coefficient $P_{i, t}, \forall i, t$ affects the effect of COCOA as 0, $E^\mathrm{app}_t = 0$ by Equation (3.12). In this case, the COCOA-SIR model defined by Equations (3.13), (3.14), and (3.15) are in the same form as the basic SIR model defined by Equations (2.4), (2.5), and (2.6). Therefore, we consider the COCOA-SIR model an extension of the basic SIR model.

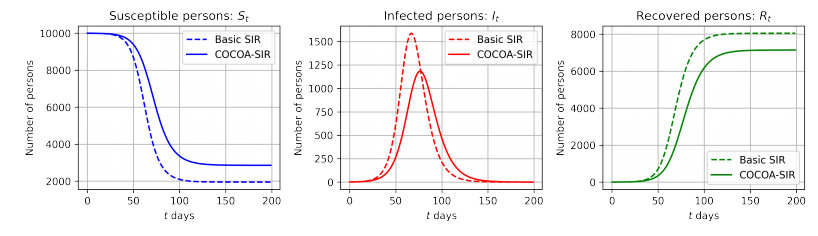
The simulation results of the basic SIR and COCOA-SIR models are shown in Figure 4. The simulation parameters are $r_t = 2$ as the effective reproduction number, $S_0 = 9999, I_0 = 1, R_0 = 0$ as the number of initial susceptible, infected, and recovered individuals, $\gamma' = 1/7$ as the recovery rate (the average recovery period is 7 days), and the simulation period is 200 days. We set $p^\mathrm{use} = 1$, $p^\mathrm{15min} = 0.5$, $p^\mathrm{pcr}_\mathrm{max} = 0.5$, $p^\mathrm{false} = 0.1$, $p^\mathrm{regist} = 1$ and $p^\mathrm{home} = 1$ for parameters of COCOA. Moreover, the delaying parameters were adopted as $(a, b) = (4, 3)$. From Figure 4, it was confirmed that the number of infectors decreased.

4.   Simulations in Japan

4.1.   Objective and outline

In this section, we compare the simulations in the case of the presence and absence of COCOA using the COCOA-SIR model and actual infector-open data in Japan ^[40]. COCOA was released on June 19, 2020 ^[41] in Japan. Open data ^[40] obtained after June 19, 2020 cannot be used because it is impossible to assume a Japan where the COCOA does not exist. Moreover, there are some days in which new infectors were reported to be zero, before February 12, 2020. This means that fluctuations in the number of newly infected people are unstable. Therefore, we use the open data ^[40] of reported new infectors in Japan from February 12, 2020 to June 18, 2020 for simulations (the maximum simulation period: $t^\mathrm{max} = 128$ days).

4.2.   Simulations parameters

4.2.1.   New infectors considering infectors who are unaware of infection

The open data ^[40] of the number of new infectors reported by Japan is only the number of infectors who were aware of infection based on the results of the RT-PCR test. There will be many infectors who are unaware of the infection. Therefore, we define the number of new infectors on day $t$, considering the amount of both individuals who are unaware and aware of infection as

where $I^\mathrm{+, find}_t$ is the number of new infectors on day $t$ reported in Japan, and $C$ is a non-finding coefficient. Tsuchiya estimated that the number of new infectors, considering the amount of being unaware and aware of infection, was 23 times larger than that reported by Japan ^[39]. Therefore, we adopted $C = 23$.

4.2.2.   Recover rate $\gamma'$

Kobayashi et al.^[32] reported that $\gamma'$ ranges from 0.13 to 0.17 as depicting the spreading of COVID-19 infection in Japan; therefore, we adopted $\gamma' = 0.15$, which is the median. In this study, because we assume that the infection period follows an exponential distribution according to reports from previous research ^[37] of the SIR model, we set $\gamma'^{-1} = 1/0.15 \simeq 6.67$ days as the average infection period. The function of the exponential distribution in the case of setting $t$ as the infective period is

By integrating the exponential distribution, the probability of recovering from 0 to $x$ days is:

For example, when adopting $\gamma' = 0.15$, we obtain $\mathrm{Pr}(x = 20) \simeq 0.950$. This means that approximately 95% of COVID-19 infectors recover within 20 days.

4.2.3.   The numbers of initial susceptible, infected and recovered persons $S_0$, $I_0$, $R_0$

To perform simulations using the SIR model, it is necessary to set the numbers of initial susceptible, infected, and recovered individuals ($S_0$, $I_0$, and $R_0$). As mentioned above, the starting time of the simulation was February 12, 2020, and the average infection period was 6.67 days. Therefore, we calculated the total number of new infectors reported in Japan for 7 days (from February 5 to February 11) prior to February 12, 2020. Subsequently, we multiplied the non-finding coefficient $C$ to the total new infectors, and we adopted it as $I_0$. As a result, the initial infectors $I_0$ were 46 individuals in Japan. Moreover, because the total population of Japan is approximately $1.2 \times 10^8$ persons, we adopted the value of subtracting $I_0$ from $1.2 \times 10^8$ as the initial number of susceptible (non-infected) individuals $S_0$. Because of the starting time of the simulation, we assumed the initial number of recovered individuals to be zero (i.e., $R_0 = 0$). In conclusion, we set $S_0+I_0+R_0 = 1.2 \times 10^8$, which is the total population of Japan.

4.2.4.   infectivity $\beta'_t$

To perform the simulation using the COCOA-SIR model, it is necessary to set the infectivity $\beta'_t$. As mentioned above, we used the open data of periods without COCOA. Therefore, we searched for infectivity $\beta'_t$ that minimizes the absolute error between the new infectors $\beta'_t S_t I_t$ using the basic SIR model shown in Equation (2.5) and the new infectors $I^\mathrm{+, all}_t$ reported by Japan. Thus, we adopted the optimal infectivity ${\beta'}^\mathrm{opt}_t$ as,

Moreover, by using Equation (2.7), we calculated the optimal effective reproduction number ${r}^\mathrm{opt}_t$ as

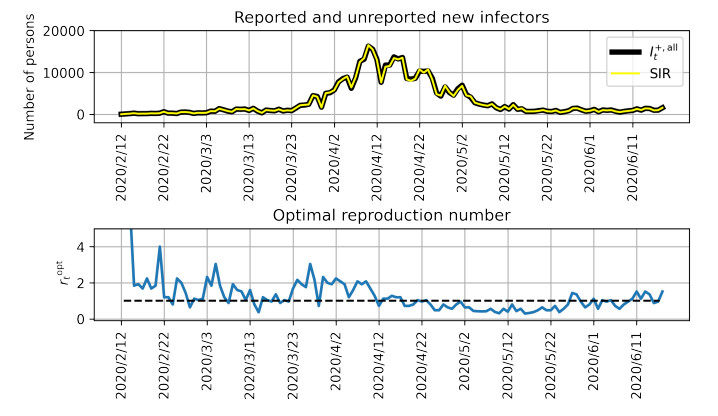
The line charts of new infectors in Japan $I^\mathrm{+, all}_t$ and new infectors reproduced by the SIR model using the optimal reproduction number ${r}^\mathrm{opt}_t$ are shown in Figure 5. The black line on the upper side is $I^\mathrm{+, all}_t$, and the yellow line on the upper side represents new infectors reproduced by the SIR model. As they are very similar, we adopted ${\beta'}^\mathrm{opt}_t$ to simulate the spread of infection and to verify the effect of COCOA in reducing infectors in Japan. The blue line at the bottom of Figure 5 indicates the optimal reproduction number ${r}^\mathrm{opt}_t$ solved by Equation (4.5).

4.2.5.   The usage rate of COCOA

According to the Japanese government, the number of COCOA users was 23.1 million on January 8, 2021, in Japan^[41]. This means that the usage rate of COCOA in Japan is 0.1925 because the total population in Japan is $1.2 \times 10^8$. Therefore, we adopted $p^\mathrm{use} = 0.1925$. Moreover, we adopted $p^\mathrm{use} = 0.6, 0.9$ to perform simulations in the case of the successful promotion of COCOA for the Japanese population.

4.2.6.   The rate of undergoing RT-PCR test

Tsuchiya ^[39] reported that the estimated number of total infectors in Japan may be 23 times the number of infected individuals. In other words, the rate of RT-PCR testing in Japan may be approximately $1/23 \simeq 0.0435$ for all infectors. Therefore, we adopted $p^\mathrm{pcr}_\mathrm{max} = 0.0435$ as the maximum rate of the PCR test. Next, we consider the delaying parameters of undergoing the RT-PCR test. It is difficult to determine the evidence-based values for the delaying parameters $a, b$ in Equation (3.3). Therefore, we adopted $(a, b) = (1.25, 3)$ as the optimistic scenario and $(a, b) = (1.25, 6)$ as the pessimistic scenario, respectively (these forms are shown in Figure 3). This means that half of $p^\mathrm{pcr}_\mathrm{max}$ underwent the RT-PCR test by day 3 or 6. Moreover, we adopted $p^\mathrm{pcr}_\mathrm{max} = 0.3, 0.6, 0.9$ to perform simulations in the case of successful PCR tests in the Japanese population.

4.2.7.   The registration rate of positive result to COCOA

The total number of registrations of positive results on the RT-PCR test to COCOA is 5297 individuals on December 25, 2020, and 6929 individuals on January 8, 2021, ^[41]. Therefore, the number of registrations of positive results for these 2 weeks is $6929-5297 = 1632$ individuals. Moreover, the number of new infectors for 2 weeks from December 25, 2020, to January 8, 2021, was 63383 individuals ^[40]. As the usage rate of COCOA $p^\mathrm{use}$ is 0.1925, the estimated total number of infectors who use COCOA may be approximately $63383 \times 0.1925 \simeq 12201$ individuals for these 2 weeks. In this case, we can estimate $p^\mathrm{regist} = 1632/12201 \simeq 0.1337$ as the registration rate of the positive result inputted to COCOA. Moreover, we adopted $p^\mathrm{regist} = 0.3, 0.6, 0.9$ to perform simulations in the case of successful promotion of the registration of RT-PCR positive results to the Japanese population.

4.2.8.   False negative rate of PCR test

Kucirka et al. ^[42] reported a false-negative rate of the COVID-19 RT-PCR test. These authors indicated that the false negative rate depended on the days elapsed from the time of infection, and the median value was 0.38. Therefore, we adopted $p^\mathrm{false} = 0.38$ as the false negative rate.

4.2.9.   The rate of satisfying the contact condition and self-quarantine

The conclusive condition of the COCOA of contact with infectors is that individuals are in proximity to the infectors by less than 1 m and for more than 15 minutes. However, it is difficult to know what percentage of infected people have been infected by satisfying these conditions. In other words, it is difficult to set the evidence-based value to $p^\mathrm{15 min}$. Therefore, we adopted $p^\mathrm{15 min} = 0.6$ as the optimistic scenario and $p^\mathrm{15 min} = 0.3$ as the pessimistic scenario. Moreover, it is difficult to know what percentage of close contacts who receive a contact notification from COCOA undertake self-quarantine appropriately. Therefore, we adopted $p^\mathrm{home} = 0.3, 0.6, 0.9$ to obtain the results for various scenarios.

4.3.   Results and discussions

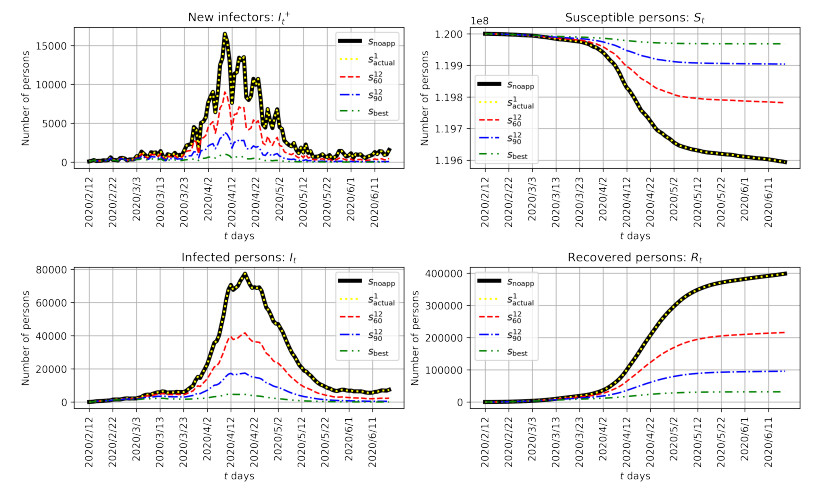
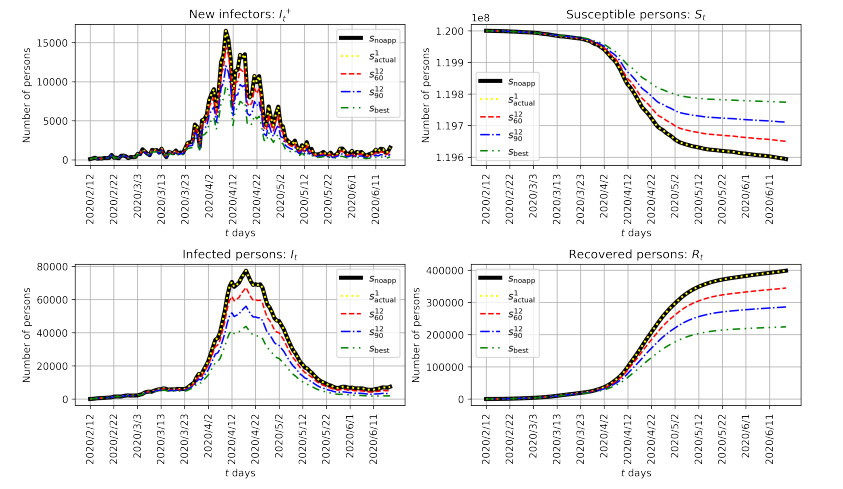
We calculated the COCOA-SIR model, which consists of Equations (3.13), (3.14) and (3.15), using Python 3.7.3 ^[43], Numpy 1.18.5 ^[44]. The simulation results of the optimistic and pessimistic scenarios are presented in Tables 2 and 3, respectively. The optimistic and pessimistic scenarios indicate that the simulations adopted $(a, b, p^\mathrm{15 min}) = (1.25, 3, 0.6)$ and $(a, b, p^\mathrm{15 min}) = (1.25, 6, 0.3)$, respectively. Column 1 means scenario ID. $s_\mathrm{noapp}$ represents Japan, which is the absence of COCOA, which means that the actual number of infectors are considered to be infectors who were unaware of the infection. $s^{1}_\mathrm{actual}, \cdots, s^{3}_\mathrm{actual}$ are the scenarios wherein parameters close to reality are adopted in the case of COCOA in Japan. $s_{60, 90}^{1}, \cdots, s_{60, 90}^{12}$ are the scenarios for the successful promotion of COCOA ($p^\mathrm{use} = 0.6, 0.9$). Thus, $s_{60, 90}^{1}, \cdots, s_{60, 90}^{3}$ are the scenarios wherein parameters close to reality are adopted, with the exception of the usage rate of COCOA, and $s_{60, 90}^{4}, \cdots, s_{60, 90}^{12}$ are the scenarios in which all parameters improved. Moreover, $s_\mathrm{best}$ is the best scenario for decreasing infectors. "total infectors" in Table 2 and 3 is the total number of infectors at the ending time of simulation ($t = t^\mathrm{max}$). "reductions" in Table 2 and 3 means that the number of decreasing infectors in comparison with the scenario $s_\mathrm{noapp}$ and the "reduction rate" represents that rate.

First, we compare $s_\mathrm{noapp}$ and $s^{1}_\mathrm{actual}, \cdots, s^{3}_\mathrm{actual}$. In both the optimistic and pessimistic scenarios, the rate of the number of decreasing infectors was only 0.00 % to 0.04 %. Therefore, we consider that the effect of COCOA on decreasing infectors is small in the actual conditions on January 2021 in Japan. Next, we check the cases of successful increase in the usage rate of COCOA to 60% ($s_{60}^{1}, \cdots, s_{60}^{12}$). In these cases, where $p^\mathrm{pcr}_\mathrm{max}, p^\mathrm{regist}, p^\mathrm{home}$ are high rates and the optimistic scenarios, the total infectors decrease by 46.23% (e.g., the scenario of $s_{60}^{12}$). However, even if the usage rate of COCOA is 60%, in these cases, $p^\mathrm{pcr}_\mathrm{max}, p^\mathrm{regist}, p^\mathrm{home}$ are low (e.g., scenarios $s_{60}^{1, 2, 3}$), the effect of COCOA on decreasing infectors is insufficient. In the case of a usage rate of 90% ($s_{90}^{1, \cdots, 12}$), there are similar trends. In previous studies ^[30], the objective usage rate of the contact tracing app was 60% or higher. However, we found that $p^\mathrm{pcr}_\mathrm{max}, p^\mathrm{regist}, p^\mathrm{home}$ are also important parameters. The reasons for the different results from the previous studies are discussed. Omae et al.^[30] verified the effect of COCOA on decreasing infectors using a multi-agent simulation. However, there were unrealistic assumptions, such as one assumption that all infectors underwent PCR tests and the false negative was zero ^[30].

We consider the results described in this report to reflect the specifications of COCOA as much as possible, and the conditions were set to be realistic. Therefore, the results shown in Tables 2 and 3 appear to be reliable. As for which is right, the optimistic or pessimistic scenario, it is difficult to decide this conclusively. However, it is important to promote not only the usage rate of COCOA, but also the rate of RT-PCR testing, the registration of positive results, and of self-quarantine to improve the effect of COCOA on decreasing the total number of infectors.

The line charts of some scenarios are shown in Figure 6 and 7. Figure 6 and 7 mean the optimistic and pessimistic scenarios, respectively. These indicate that $s_\mathrm{noapp}$ is very similar to $s^1_\mathrm{actual}$. Therefore, we consider that there will be little effect of COCOA on decreasing the number of infectors in the case of a small usage rate of COCOA. However, in the case of success in improving the rate of usage of COCOA, usage of RT-PCR tests, registration of positive results, and undertaking of self-quarantine (the scenarios: $s_{60}^{12}$, $s_{90}^{12}$, $s_\mathrm{best}$), Figure 6, and 7 indicate that COCOA has a significant effect on decreasing the number of infectors. We consider the COCOA developed by Japan to be very important in overcoming the spread of COVID-19.

Caution must be exercised when explaining the explained simulations. The SIR model implies complete mixing of the population. However, this premise is not a realistic assumption because Japan is a sufficiently large country. For this point, we consider that this is not a problem because the COVID-19 outbreak in different locations in Japan started at approximately the same time (Postnikov ^[45] discussed the relationship between estimating infectors of the country as a whole and local disjoint sub-populations by the SIR model).

5.   Conclusion

In this research, we proposed a COCOA-SIR model to verify the effect of the contact tracing app COCOA that was developed by Japan. The important parameters $p^\mathrm{use}, p^\mathrm{15 min}, p^\mathrm{pcr}_{i, t}, p^\mathrm{false}, p^\mathrm{regist}, p^\mathrm{home}$ shown in Table 1 were included in the COCOA-SIR model. Moreover, we performed simulations of various scenarios assumed for Japan. The results improved an understanding of the effect of COCOA on decreasing the number of infectors.

We show the future directions for research below. We have used the assumed numbers (e.g., $a, b, p^\mathrm{15 min}$) to perform the simulation and, therefore, we will analyze and find more desirable numbers. In this research, we used an SIR model. However, we consider the SEIR model, which can represent the incubation period, may be a more desirable model for such a simulation. Therefore, we plan to propose and develop an SEIR model-based simulation to clarify the effect of COCOA on decreasing the number of infectors.

Acknowledgments

This work was supported in part by the Telecommunications Advancement Foundation (Grant No. 20203002 to Y. Omae) and a JSPS Grant-in-Aid for Scientific Research (C) (Grant No. 21K04535 to J. Toyotani].

Conflict of interest

The authors have no conflict of interest.