Figure 1.
Map of Balochistan Province Pakistan.
Recently, T. Masson, J. Francois, S. Lazzarini, C. Fournel and J. Attard have introduced a new method of the reduction of gauge symmetries called the dressing field method. In this paper we analyse this method from the fiber bundle point of view and we show the geometric implications for a principal bundle underlying a given gauge theory.We show how the existence of a dressing field satisfying certain conditions naturally leads to the reduction of the principal bundle and, as a consequence, to the reduction of the configuration and phase bundle of the system.
Citation: Marcin Zając. The dressing field method in gauge theories - geometric approach[J]. Journal of Geometric Mechanics, 2023, 15(1): 128-146. doi: 10.3934/jgm.2023007
| [1] | Titin Siswantining, Alhadi Bustamam, Devvi Sarwinda, Saskya Mary Soemartojo, Moh. Abdul Latief, Elke Annisa Octaria, Anggrainy Togi Marito Siregar, Oon Septa, Herley Shaori Al-Ash, Noval Saputra . Correction: Triclustering method for finding biomarkers in human immunodeficiency virus-1 gene expression data. Mathematical Biosciences and Engineering, 2023, 20(4): 7298-7301. doi: 10.3934/mbe.2023316 |
| [2] | Saskya Mary Soemartojo, Titin Siswantining, Yoel Fernando, Devvi Sarwinda, Herley Shaori Al-Ash, Sarah Syarofina, Noval Saputra . Iterative bicluster-based Bayesian principal component analysis and least squares for missing-value imputation in microarray and RNA-sequencing data. Mathematical Biosciences and Engineering, 2022, 19(9): 8741-8759. doi: 10.3934/mbe.2022405 |
| [3] | Wei Kong, Feifan Xu, Shuaiqun Wang, Kai Wei, Gen Wen, Yaling Yu . Application of orthogonal sparse joint non-negative matrix factorization based on connectivity in Alzheimer's disease research. Mathematical Biosciences and Engineering, 2023, 20(6): 9923-9947. doi: 10.3934/mbe.2023435 |
| [4] | Ahmed Hammad, Mohamed Elshaer, Xiuwen Tang . Identification of potential biomarkers with colorectal cancer based on bioinformatics analysis and machine learning. Mathematical Biosciences and Engineering, 2021, 18(6): 8997-9015. doi: 10.3934/mbe.2021443 |
| [5] | Fang Niu, Zongwei Liu, Peidong Liu, Hongrui Pan, Jiaxue Bi, Peng Li, Guangze Luo, Yonghui Chen, Xiaoxing Zhang, Xiangchen Dai . Identification of novel genetic biomarkers and treatment targets for arteriosclerosis-related abdominal aortic aneurysm using bioinformatic tools. Mathematical Biosciences and Engineering, 2021, 18(6): 9761-9774. doi: 10.3934/mbe.2021478 |
| [6] | Sijie Lu, Juan Xie, Yang Li, Bin Yu, Qin Ma, Bingqiang Liu . Identification of lncRNAs-gene interactions in transcription regulation based on co-expression analysis of RNA-seq data. Mathematical Biosciences and Engineering, 2019, 16(6): 7112-7125. doi: 10.3934/mbe.2019357 |
| [7] | Yu Jin, Zhe Ren, Wenjie Wang, Yulei Zhang, Liang Zhou, Xufeng Yao, Tao Wu . Classification of Alzheimer's disease using robust TabNet neural networks on genetic data. Mathematical Biosciences and Engineering, 2023, 20(5): 8358-8374. doi: 10.3934/mbe.2023366 |
| [8] | Youlong Lv, Jie Zhang . A genetic regulatory network based method for multi-objective sequencing problem in mixed-model assembly lines. Mathematical Biosciences and Engineering, 2019, 16(3): 1228-1243. doi: 10.3934/mbe.2019059 |
| [9] | Ming-Xi Zhu, Tian-Yang Zhao, Yan Li . Insight into the mechanism of DNA methylation and miRNA-mRNA regulatory network in ischemic stroke. Mathematical Biosciences and Engineering, 2023, 20(6): 10264-10283. doi: 10.3934/mbe.2023450 |
| [10] | Shuaiqun Wang, Tianshun Zhang, Wei Kong, Gen Wen, Yaling Yu . An improved MOPSO approach with adaptive strategy for identifying biomarkers from gene expression dataset. Mathematical Biosciences and Engineering, 2023, 20(2): 1580-1598. doi: 10.3934/mbe.2023072 |
Recently, T. Masson, J. Francois, S. Lazzarini, C. Fournel and J. Attard have introduced a new method of the reduction of gauge symmetries called the dressing field method. In this paper we analyse this method from the fiber bundle point of view and we show the geometric implications for a principal bundle underlying a given gauge theory.We show how the existence of a dressing field satisfying certain conditions naturally leads to the reduction of the principal bundle and, as a consequence, to the reduction of the configuration and phase bundle of the system.
Drought has been observed to occur every 4 out of 10 years in Pakistan having a devastating effect on the agriculture, livestock, economy and, consequently, on people's livelihoods. The German watch Climate Risk Index ranks Pakistan 5th in the list of countries most effected by the adverse effects of climate in 2020. Out of total 79.6 million hectares area of Pakistan, 88% consist of arid and semi-arid lands. According to the facts provided, only 9% of Pakistan's land receive more than 508 mm of rain, 22% receive between 254–508 mm of rain, and 69% less than 254 mm [1]. In the recent past there has been an increase in the incidence, frequency, and intensity of extreme climatic events, about 40% of the people of Pakistan are highly prone to frequent multiple disasters that also includes droughts [2,3].
Three elements make Pakistan especially susceptible to climate change and its probable effects on agriculture. The first is its geographical location. Temperature increase within the country is predicted to be higher than the global average [4]. Second, demographic dynamics in Pakistan threaten to compound climate risks. At over 2.2 billion, the country not only has sixth largest population, but this population remains growing fast at a rate of 2.4% between the 1998 and 2017 censuses and also urbanizing soon. These strategies are exerting excellent strain on the natural assets needed to maintain agriculture protection. Third, the country is economically at risk; because of its heavy reliance on agriculture. According to the World Bank, the second largest sector of the economy agriculture, which includes (plants, cattle, fisheries and forestry), contributes approximately 25% of the gross home product (GDP) [5]. This was also consistent with the Economic Survey of Pakistan [6], which reports that, major plants (wheat, rice, maize, sugarcane and cotton) account for approximately 23.55% of agricultural output and 4.67% of GDP. Moreover, agriculture employs more than 42% of the labor force and generates over 75% of export sales through agri-based textiles (cotton) and agri-food products [7].
Among other provinces, Balochistan is the most drought prone region. Majority of population lives in rural areas of the province and 85% population depends upon agriculture and livestock as their main source of income [8].The provincial economy of Balochistan also depends mainly on agriculture and livestock sectors, which accounts for 67% of the labor force [9]. In connection to that, drought in the study area has severely affected the agriculture growth and has suffered an intense setback that most of the farmers have quit farming and switched over to other off-farm activities for their sustenance. The drought in the province has been severe in magnitude as annual precipitation (60–150 mm) have been well below than the average annual rainfall (200–250 mm). This has also negatively affected the study area in particular.
Results of long-term study show that the annual range production has declined from 150–250 kg/ha to 30–45 kg/ha because of continuous drought in most parts of the province. The limited precipitation and availability of surface water drastically restricted the cultivated land to around 2.1 million hectares (Mha) during the drastic drought of 2002–2001, which was around 6% of the province's geographical area. Osbahr et al. (2008) argue that high dependency of agriculture on natural resources results in higher vulnerability to drought impacts and it becomes difficult for agriculture dependent groups to regain normal livelihood even after drought [10]. In severe cases, it has increased the chance of seasonal food crises. These prolonged droughts destroyed nearly 80% of fruit orchards specially apples, which is the most water-consuming fruit in the region. Repeated crop failures and declining yields leads to falling farm income and associated problems of food shortage, malnutrition and general impoverishment of local inhabitants [8,9,10,11]. The study conducted by Ashraf & Routray [8] regarding farmers' perception was the first, whereas, Rehman et al. (2019) were the second to seek farmers' perception towards drought in other different districts in the context of Balochistan [12]. It is therefore, this study would be the third of its type and different from other studies conducted as to understand farmer's risk perception of upland area of the province Balochistan and to explore farmers' vulnerability to socio-economic drought in the study area.
A number of studies have indicated that drought stands first among all natural hazards [13,14,15]. Most of the people have been affected directly or indirectly in different parts of the world [16]. In comparison to other natural disasters such as floods and hurricanes, it affects a much larger spatial area. Not only, drought is considered a great disaster in economic, social and environmental terms as stated by Mniki [17], rather it will become worse in future with sever impacts to rural communities of the developing countries [18]. Increasing frequency and intensity of droughts in many parts of Asia are attributed largely to a rise in temperature, particularly during the summer and normally drier months, and during ENSO events [19]. The lack of precipitation and droughts in most delta regions of Pakistan, Bangladesh, India and China have resulted in the drying up of wetlands and severe degradation of ecosystems.
The arid climate and rough topography of Balochistan, coupled with high rainfall variability, contributes to the province's vulnerability to socio-economic droughts. Severe and extreme early winter droughts are frequent in the north of the province [9]. The northern area of the province also includes the study area. The province's agricultural sector has suffered severe losses in the past due to summer droughts. The summer droughts occur due to scarcity of monsoon rainfall and have a severe effect on local populations and ecosystems, especially on agriculture-dependent populations in the region [20]. During the severe drought occurred from 1998–2002 the annual rainfall in upland Balochistan ranged from 200–250 mm [21,22]. It was the worst hit period in the last 75 years in Balochistan, which badly affected all spheres of lives and resulted in the loss of life. Out of 22 million livestock, almost 36.59% were affected [23]. These droughts resulted in the loss of 50–80% of the mountainous regions of Balochistan that were rather more prone to frequent droughts [18].
In their study, Naz et al. (2020) used Standardized Precipitation Index (SPI) to characterize the meteorological droughts of 37 years that occurred from 1980–2017 in different parts of Balochistan [24]. The researchers found that the province had experienced summer and winter droughts throughout the period of 37 years. Drought severity in Balochistan was also analyzed by Ashraf and Routray [25] using standardized precipitation index (SPI) to examine spatiotemporal variability of dry periods in Balochistan in which they used precipitation data of 36 years (1975–2010). They found the existence of negative trends in precipitation in more than 70% of climatic stations at both annual and seasonal scale, however, the period selected for their study did not provide complete picture of the evolution of droughts throughout the 20th century. Furthermore, SPI is appropriate merely for short-term meteorological dry events; therefore, this index does not reflect the changes in evaporative demand occurring due to warming. It is therefore, other researchers used more advanced and reliable indices such as Standardized Precipitation Evapotranspiration Index (SPEI) to explore the evolution of drought characteristics in Balochistan over the years from 1902–2015 [26]. They concluded that the drought in Balochistan will increase dryness which indicates that there would be extreme drought events in future and will further worsen the aridity and desertification.
This study intends to examine the understanding of farmers' perception and awareness to drought vulnerability. As perception refers to a range of judgments, beliefs and attitudes therefore understanding people's perception can help identifying barriers for the effective formulation and implementation of policies [27,28]. The specific objective of this study are (1) exploring farmers' perception to drought vulnerability in the study area (2) farmers' level of awareness regarding extended drought and (3) analyzing the factors affecting the farmer's risk attitude and perception.
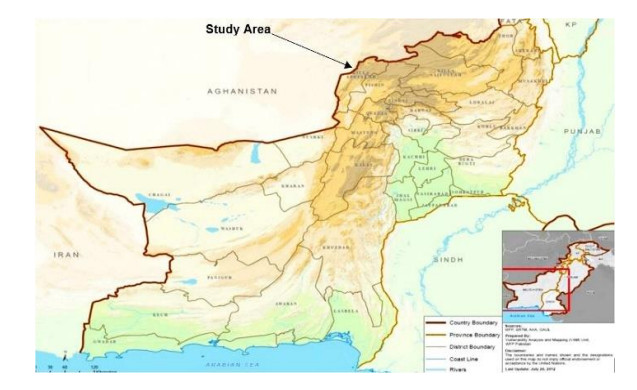
The study area, district Killa-Abdullah (Figure 1) lies in the upland area (1500–2500 meters above sea level) of Balochistan province. Balochistan is known as fruit garden of the country and more than 31 crops grow in the province. The province mainly depends on agriculture and livestock sectors, which accounts for over 50% of the GDP and employ about 67% of the labor force [29]. Within the province, this district exists in agro-climatic zones of Balochistan and has been severely affected by extremely high droughts [9,30]. The district lies in the north of the province and in the west of Pakistan. It stretches between 66°14′23″–67°15′43″ East longitudes and 30°05′7″–31°18′46″ North latitudes in the foothills of the Shela Bagh Mountain range. The geographical area of the district is 5263 km2 [31]. It is administratively divided into 4 Tehsils (Gulistan, Killa Abdullah, Deobandi & Chaman) which includes 25 Union Councils called UCs (the lowest unit of administration), while headquarter of the district is in "Chaman" city, close to Afghan border [32].
The weather of the study area remains dry in both winter and summer with scanty rainfall. The annual average rainfall ranges from 200–250 mm [33]. Out of total (15122 hectares) cultivated area 11550 hectares area is irrigated by 10743 tube wells and 126 wells. The overall population of the district is 757,578 as compare to that of 1998 census, which was 360,724. The rural population consists of (80.29%) with 4.02% of annual growth rate. The Female population of the district is 47.52%, whereas, the male population is around 52.48%. In 1998, almost 112 people resided per square kilometer area, whereas in 2010 only about 190 persons lived per square kilometer, which means 78 persons increased per square kilometer in the past 12 years. The main reason for this increase was the high growth rate (4.02%).
Approximately 80% of the population lives in rural areas of the district and 68% of the population is employed in agriculture. Agriculture sector uses around 80% of fresh water in Balochistan [32]. Currently in the study area, the only water resource left for the farmers is the availability of groundwater. The groundwater level is declining at an alarming rate of 3 to 4 meters annually (Field survey, 2018), hence tube wells drying is a common phenomenon [34]. There are numerous crops grown in the district. The potential Rabi1 crops are wheat, barley, vegetables, tobacco and cumin, whereas, fruits, onion, melons, fodder and potato are the important Kharif2 crops. The main fruits in the districts are apple, grapes, apricot, pomegranate and peach. Amongst them, the most typical fruit that farmers grow from previous generations is Apple. Women's involvement in the agriculture/ horticulture sector is negligible due to the cultural and tribal set-up.
1 Rabi is a cropping season that begins in October-December and ends in April-May.
2 Kharif is a cropping season that begins in April-June and harvesting during October-December.
We aim to understand not only farmers' perception of drought vulnerability, but also their behavior and awareness regarding extended drought in the drought prone district of Balochistan province. We used questionnaire survey, which is one of the effective instruments of data collection. Three focus group discussions were held with respondents in three different Tehsils (sub-districts) of the district that helped in framing the questionnaire. Primary data was collected from 265 small-scale farmers in face-to-face interview. We selected small-scale farmers for this study, which were more vulnerable to the severity of drought impacts and formed 97% of the sample. The state bank of Pakistan defines small-scale farmers as holding the land size of 32 acres and Agriculture Census (2010) reports that 84% of the farmers have 25 acres of farmland in the province of Balochistan [35]. However, as per field data almost 80% of small-scale farmers in the study area had less than 25 acres of land holding size in which the average farm land-holding size is 14 acres. Contrary to that, there are some larger commercial farms as well that are also subject to the drought impacts but they are not the focus of our study.
The interviews were conducted by the first author himself including two other enumerators who were MS students and properly trained. Verbal consent was sought from the respondents to fill in the questionnaire. Those who volunteered to participate in the study were included. The entire target respondents were male and were explained the objectives of the study. Any identification of the participants was not recorded and confidentiality was assured during data collection. We conducted survey in the local language (Pashto) for better understanding as all the interviewers and respondents could speak and understand the same language. Prior to the survey, the questionnaire was pre-tested with sub-sets of the targeted population to check the redundancy, missing information, relevancy as well as validity of the questions. The questionnaire was then modified based on pre-test results. The individuals included in pre-test were omitted from the sample considered in this study.
For the selection of sample villages and farmers, multistage sampling technique was used, as such sort of techniques were also adopted by Ashraf and Routray as well as Iqbal et al. [8,36] for such type of studies. The study district Killa-Abdullah consists of 4 Tehsils (sub-districts). We first selected three Tehsils as the fourth Tehsil Chaman did not fall in the agriculture zone. Then after consultation with the agriculture officer, 14 union councils out of 25 were selected for our survey research. The 11 UCs (lowest administrative units) which were not the focus of our study were that of Chaman Tehsil. The sample size (265) was drawn from the total number of households in the study area that was 77,919. All of these Tehsils and UCs were scattered. There are several approaches to determining the sample size. These include using a census for small populations, imitating a sample size of similar studies, using published tables, and applying formulas to calculate a sample size. However, we wanted to determine the sample size in order to have true representation of the population, so that we could draw statistically significant conclusions about the population through an analysis of our sample. For this, the method of Arkin and Colton [37] was employed to determine sample size for household survey. The sample size determination formula is given as:
| n=nz2×p×(1−p)Nd2+Z2×p×(1−p) | (1) |
Where,
n = sample size (265)
N = total number of households (77919)
Z = confidence level (95% level Z = 1.96)
p = estimated population proportion (0.5)
d = margin of error 5% (0.05)
In the later stage, villages from within the Union Councils were purposively selected and finally individual households were selected using the random walk sampling technique. The questionnaire survey administered for this study was structured into three parts: (i) farmers' perception and awareness to drought vulnerability (ii) socio-economic vulnerability assessment (iii) farmers' coping strategies and adaptation measures. The data were processed and statistically analyzed in to interpret the results. Descriptive and inferential statistics (tables and figures) were used to understand farmers' level of perceptions and awareness to drought vulnerability.
In the study conducted, 265 farm households were interviewed, in which all of the respondents were male. The women in the study area do not work in the field due to cultural norms. The average age of respondent was 52 years, which implies that majority were in the productive age group. The age of the participants ranged between 25 to 82 years. The household size (10) of the sampled respondents was higher than the national average household size (7.79) of the district, which indicated the presence of high fertility rate. Data on education indicated that 57% respondents had no education, and 43% were literate in which 24.5% had primary and 18.5% had secondary and higher education. Almost 93% of the respondents were engaged in agriculture while nearly 7% were employed in government organizations (service holders), and farming was the secondary occupation of the latter group. A significant proportion of the respondents (56%) had no secondary source of income. The average farming experience is about 31 years, whereas, the average farm and non-farm land-holding size was 14 and 9 acres respectively. The average annual income of the sampled respondents is 5129 US dollars 3 annually. The major source of the income (66%) in the study area was fruit orchards; whereas, the secondary source of income for most of the respondents was government employment, off-farm business activities, labor work etc.
3 The exchange rate of 1 US dollar was PKR 110 during the data collection period Jan-Feb 2018.
Different people perceive drought differently, it includes certain levels of conceptualization such as education, age, location and different livelihood activities [38,39]. It is evident from Table 1. that drought was well perceived by the respondents and that how prevailing drought has affected the study area over the years.
| Variables | Increased | Decreased | No change | No idea | ||||
| No | % | No | % | No | % | No | % | |
| Rainfall Intensity | 0 | 0 | 242 | 91.3 | 7 | 2.6 | 16 | 6 |
| Temperature | 218 | 82.3 | 0 | 0 | 19 | 7.2 | 28 | 10.6 |
| Frequency of drought | 213 | 80.4 | 0 | 0 | 34 | 12.8 | 18 | 6.8 |
| Availability of ground water | 0 | 0 | 251 | 94.7 | 0 | 0 | 14 | 5.3 |
| Length of winter period | 9 | 3.4 | 202 | 76.2 | 17 | 6.4 | 37 | 14 |
| Length of summer period | 208 | 78.5 | 19 | 7.2 | 8 | 3 | 30 | 11.3 |
| Source: Field survey, 2018. | ||||||||
DownLoad:
CSV
According to the respondents, drought in the region has been frequent in the last 20 years and more specifically, it prevails from 3–4 years out of 10 years in the study area. This statement is also in line with the results of Farooqi and co-authors [40]. They analyzed that frequency of this creeping disaster in Pakistan is 2–3 years in every 10 years, whereas, Anjum et al. [1] recorded 4 out of 10 years. The most recent spell of drought extended from 2011–2014. Majority of the respondents (91.3%) reported that decreasing of rainfall intensity is rather increasing the severity in current drought situation and according to them (82.3%), the temperature in the region has increased with the passage of time. Various researchers in different regions of the world reported similar types of results for example, Maddison [41] mentions that a significant number of farmers in eleven African countries believe that temperature has increased and that precipitation has declined, moreover, Habiba et al. (2012) also mentioned in her research findings that decreasing rainfall is responsible for drought in Bangladesh [42]. In addition to that, Chaudhry et al. (2009) stated in his research that Pakistan experienced 0.76 ℃ rise in temperature during the last 40 years [43]. This means, it will enhance heat waves and drought severity in the region.
In addition to that, the longer summer period and shorter winter period indicate as observed climate change in the area. As many as (76.2%) and (78.5%) respondents reported decrease and increase in winter and summer periods respectively. Similar findings were revealed by some of the researchers that cold periods have become shorter and hot periods longer [41,43]. Not only this, but also most of the respondents (94.7%) stated that ground water level has reached to an alarming situation. This finding is also true in the context of Bangladesh [42] and with reference to the Balochistan province Ashraf and Routray [8] found somewhat similar situation regarding ground water depletion. Ground water is the only source left for irrigation and domestic purposes. Almost 97% of groundwater is used by agriculture sector in Balochistan province. In some UCs, for example (Habibzai Ⅰ-Ⅱ, Mazai, Masezai, Kulazai, Badwan and Lajwar) the depth of water table has reached from 244–305 meters as compared to the mountainous area such as Jilga, Kandanai & Tabeen Alizai where almost the aquifer level is at 122–152 meters. It is therefore; households frequently buy drinking water at a high cost of Rs. 1000–1500, which is unaffordable especially when farmers face heavy losses. According to the respondents, the decline in groundwater level occurs almost 3–4 meters a year due to excessive pumping and this causes enhancing of drought [42,45].
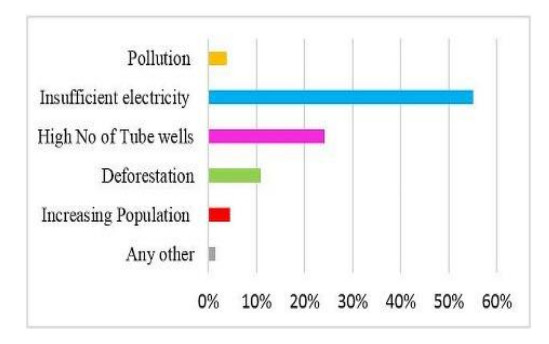
Amongst the most highlighted non-climatic factors, as shown in Figure 2. insufficient supply of electricity was the one that stood responsible for the severity of drought in the district. According to the respondents (55%), their farms dried up due to groundwater through lack of electricity supply. Over recent decades, groundwater use has grown exponentially in scale and intensity in many places, leading to aquifer depletion and groundwater pollution [46,47]. Since there are no other water resources available in the study area for irrigation purposes except groundwater that is why farmers fetch groundwater through electric power tube wells using submersible pumps. If for example, there is insufficient electricity, (which is less than 6 hours per day) farmers fail to water their orchards and crops properly, consequently farms dry up. There is high demand for the availability and consumption of enough electricity in the study area; however, due to non-availability of documented data, it is difficult to estimate that how much electricity is required per unit of land.
The water and power department authority (WAPDA) which is a federal government power company and responsible for the provision of electricity in the entire country, provided electricity with 90% cost of subsidy to the farmers in the study area. Tube well owners with a legal connection paid a flat rate of as little as PKR 6000 per month (USD 55 per month). The WAPDA was supposed to provide electricity officially for 6 hours on daily basis. However, the shortfall of electricity in some villages even increased and respondents would get electricity for 2–3 hours daily. The low voltage and disturbance in the flow of electricity further deteriorated the situation and caused the tube wells out of order to fetch ground water for their farms. Ashraf and Routray [8] revealed similar findings that, load shedding and electricity fluctuation severely affected orchards and other means connected to electricity. The study conducted by Iqbal et al. (2018) in Afghanistan also reveals that lack of electricity supply increases drought vulnerability [36], whereas, Sebastian Jülich [48] took electricity as proxy indicator and outlined in his study conducted in East India that electricity supply has no direct causal connection to drought vulnerability, rather both are connected by one or more background variable(s).
During the failure of electricity in the study area, farmers in compulsion operated their tube wells through rented or owned generators. The factual ground reality is that farmers also need to cope with electricity power deficit. In order to cope with power deficit, they used rented or owned generators that consume 12–13 liters diesel per hour approximately. These generators are the alternative of electricity, which run the submersibles that of the tube wells in order to raise groundwater for the farms. It is however, consumption of much diesel upon these generators, especially on the rented ones, give farmers a financial loss as well as cause air pollution. Such an alternative of the electricity is the final resort for the farmers to protect their farms by getting dry. Somehow, similar experiences and complaints were also reported by the owners of cold storages, who were interviewed as key informants, that during the absence of electricity, they run 300 kv generators that consume 25–30 liters diesel/hour to maintain the temperature of cold storages and that stock of apples could be stored there for a longer period.
Apart from deforestation and increase in population, the high number of tube wells was considered as the second biggest non-climatic factor that increased drought vulnerability in the study area. This is because, the uncontrolled installation of numerous public and private tube wells was the real source of groundwater depletion and combined with prevailing drought, it worsened the situation. On average, a tube well served 6–8 ha of land. The worsened electricity supply further dampened orchard expansion. It also paradoxically encouraged additional tube well development by those farmers that could afford it. The idea of the additional drilling was that several wells would operate at the same time, when there was no power outage [49]. This however, resulted the decline in water table that continued with several tube wells going dry or having severely reduced discharge.
The results of other researchers [8,42] are also in line with our findings that high number of tube wells further declines the water table. The tube wells in the entire province were electrified in 1970s as a substitute of Karez/Manat (natural water resource) by the government of that time to provide more reliable source of irrigation and utilize water more efficiently. The Balochistan Groundwater Rights Ordinance first announced in 1978 and then amended in 2001 was one of the first 'enabling' groundwater laws, however, this law was never implemented in its true spirit. Later locally agreed rules were made, a standard minimum distance rule for the installation of tube well was adopted in an amendment of the Ordinance which does not carry much importance. It is however, in their study, Halcrow and Cameos [50], also reported that there is no authority existing to regulate the pumping of groundwater in Balochistan and speedy extraction of groundwater may soon cross the aquifer level.
The respondents (68.3%) in Table No.2 rated the impact of drought as very high for decline in production and (70.9%) farmers rated for livestock losses to perceive the level of drought impact. The results are in line with the findings of other researchers that, drought has severed impacts on damaging the livestock and crops production, as well as on reduction of fruit in other parts of the world [36,45].
The extended drought and lack of monsoon rain in the region severely affected the main source of income (fruit orchards) in the study area. The farmers were not ready switching from the present crops to the new ones particularly Apples. Some of the farmers were hesitant to take any risks, whereas, other believed that the area may not be suitable for the plantation of new crops and verities due to different environment. However, farmers demanded the plantation of new crops on demonstration plots to ensure if they can be grown with good production and environmentally suitable. Contrary to that, government of Balochistan until now does not have any such plans to introduce crops or varieties on such patterns. The farmers were highly likely to go for other cropping patterns such as drought resistant and high yield corps but due to lack of knowledge and limited access to extension services, farmers were unable to identify and purchase high yield crops. They were also ignorant of the crops and verities that were more drought resistant. It is therefore, farmers did not have knowledge about high yield verities and drought resistant crops.
Farmers lacked empirical knowledge about certain drought resistant crops that could be used in the region during the extended drought. They had never tried new crops and varieties because they were not ready to leave the years' long crops and inherited practices. For example, most of the farmers confined themselves to growing apples only. According to them, this is what their ancestors used to do. Apart from this, farmers were not ready to take any risk regarding plantation of other crops, nor they tried for other varieties told by the extension workers such as almond, pistachio, cashew net, saffron, olive etc. Such crops require less water, are more productive, moneymaking and may highly decrease the drought loss.
Farmers grew different varieties of apples in the study area such as Tor kulu, Shin Kulu, Gaja and Black Ambrey. Among them, Tor Kulu and Shin Kulu, which are famous apples for its taste and color in the region, required optimum level of water for their maturity. Farmers, therefore, based on their experience, are growing more Gaja apples now as they are drought resistant to some extent and consume less water as compare to other varieties of apples. Besides apples, farmers also grew grapes, pomegranate and apricot in some villages to reduce the economic loss that farmers are facing due to extended drought in the study area.
The respondents planted apple trees with 25–30 feet distance between them. Apricot, which is an early maturing crop, was grown in-between apple trees to generate some income. Farmers, however, faced heavy losses of fruit production due to depletion of groundwater, lack of precipitation, limited supply of electricity and extended drought in the region. The statement of Nasreen [51] also supports our findings that drought affects the crops, water resources and leads to loss of production. In fact, (43.8%) respondents reported that, there has been high level of spread of diseases due to prolonged drought in the study area, which has badly affected agriculture, livestock and people's health in farming communities. The drought caused numerous diseases such as, asthma, allergy, skin diseases, malaria, diarrhea, intestinal parasites, upper respiratory infections, and tuberculosis, however, the most common disease among them was pneumonia. In their findings, Habiba et al. [42] have also mentioned such type of diseases that instigated during the dry seasons.
The impact of drought on livestock was very high (71%) due to prevailing drought, lack of water, non-availability of grass, fodder and hay. Certain diseases, relevant to animals had emerged during the dry spells such as swelling of lungs and sudden death of animals called (Kavaiy) and (Tuck) respectively in local language. Concerns over animal and crop diseases were also reported by other researchers including Muhammad Abid [52] and Sarah Jane [53]. According to the respondents in the field, they would call a medical technician for injecting medicines into animals, as there was no Vet/veterinary hospital in the entire district. In the worst case, they would treat animals themselves, however, such method of self-medication had revers repercussions upon animals. Poor health of animals during the dry spells was also reported by some researchers in a study conducted in Ethiopia [54].
In the orchard farms, diseases such as Shaftha and Magas (local names) that made the trees oily and the fruit sprinkled respectively were common in the study area, however, the disease Khara (spider mite) was of high concern. The dust on the fruit and leaves provide an opportunity for mites to develop its net around them, thus the apples become weaker and fall of the tree. Consequently, farmers cut off the trees as they fail to bloom up and bear fruits. Almost 42% respondents rated "high" the level of drought impact on selling of assets. This indicates, that a significant portion of respondents were compelled to sell their household assets during drought to purchase agriculture inputs, invest in the installation of new tube wells and purchase other livelihood items. Farmers, were however, found least interested in terms of selling land. In a study conducted by Iqbal et al. [36] in Afghanistan, reveals that farmers were forced to sell their land in order to meet their short-term food needs. Contrary to that, selling of land was rated very low in our study by majority of the respondents (76.2%), despite the fact that there has been great intensity of drought and low production in the region. This is because land is considered very important for any villager in the district and they hope that it may turn productive at any time in future. Even though, seasonal migration has been observed in the study area, yet people do not sell the land and return to their villages every now and then to share their sorrows and happiness.
In connection to that, some of the respondents (47.5%) mentioned that, there has been low migration from the study area; however, (25.3%) respondents rated migration as high level of drought impacts. In order to lessen the impacts of drought and cope with drought situation farmers seek other ways such as migration [45,55]. According to Jülich, [48] the main push factors of migration are drought and low wages in the villages. Architesh Panda [56] considers the role of migration as a livelihood strategy. Moreover, migration is considered an ex-ante risk management strategy by Osawe [57]. The remittance sent back to households increases their assets, which in turn reduces their vulnerability [58]. Migration in our study area occurred due to unemployment, low production, livestock losses and decrease in household income which was recorded as very high by (50.2%) of respondents. It is therefore, vulnerable farmers and household members were compelled to migrate towards cities in order to meet the resource deficit. Table.2, reveals that about (70%), (47%) and (40%) respondents rated the impacts as very high, high, and moderate respectively for unemployment, increase in crime rate and drop out of school-going children in the study area.
| Factors affected | Very Low | Low | Moderate | High | Very High | |||||
| No | % | No | % | No | % | No | % | No | % | |
| Decline in production | 0 | 0 | 9 | 3.4 | 29 | 10.9 | 46 | 17.4 | 181 | 68.3 |
| Livestock losses | 6 | 2.3 | 12 | 4.5 | 22 | 8.3 | 37 | 14 | 188 | 70.9 |
| Sale of household assets | 37 | 14 | 71 | 26.8 | 32 | 12.1 | 110 | 41.5 | 15 | 5.7 |
| Sale of land | 202 | 76.2 | 37 | 14 | 17 | 6.4 | 7 | 2.6 | 2 | 0.8 |
| Migration | 15 | 5.7 | 126 | 47.5 | 39 | 14.7 | 67 | 25.3 | 18 | 6.8 |
| Increase in crime rate | 4 | 1.5 | 14 | 5.3 | 29 | 10.9 | 160 | 60.4 | 58 | 21.9 |
| Depletion of water | 7 | 2.6 | 20 | 7.5 | 60 | 22.6 | 139 | 52.5 | 39 | 14.7 |
| Spread of diseases | 2 | 0.8 | 30 | 11.3 | 97 | 36.6 | 116 | 43.8 | 20 | 7.5 |
| Decrease in household income | 6 | 2.3 | 13 | 4.9 | 36 | 13.6 | 77 | 29.1 | 133 | 50.2 |
| Drop out of school going children | 14 | 5.3 | 47 | 17.7 | 115 | 43.4 | 70 | 26.4 | 19 | 7.2 |
| Farmers' Vulnerability | 6 | 2.3 | 18 | 6.8 | 50 | 18.9 | 71 | 26.8 | 120 | 45.3 |
| Source: Field survey, 2018. | ||||||||||
DownLoad:
CSV
In the same table, the variable farmers' vulnerability depicts the level of drought vulnerability faced by the farmers in the study area. Farmers' vulnerability level was rated very high by (45.3%) the respondents and some of them (26.8%) rated it high, because farmers had received heavy losses on the part of agriculture and livestock. However, some of the respondents (18.9%) considered it as moderate and only (6.8%) perceived farmers' vulnerability level to drought as low. The respondents confirmed that there was no campaign or trainings conducted for drought vulnerability to reduce farmers' vulnerability against the extended drought.
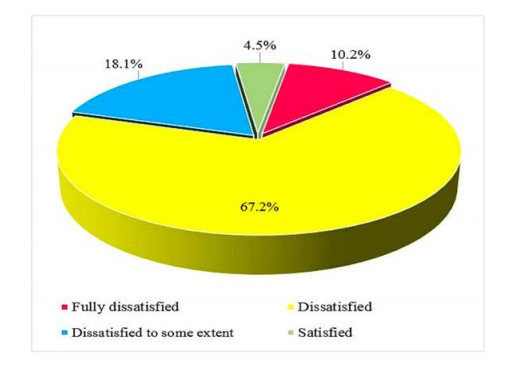
Satisfaction rating of the respondents regarding government support to the agricultural services was very low, as shown in Figure 3 almost (67%) respondents reported that they were dissatisfied and only (18.1%) were satisfied to some extent. This is because respondents did not receive any financial and technical support in the form of new technologies, irrigation facilities, agriculture inputs or trainings from the provincial or federal government except for subsidized electricity.
The respondents had also registered their complaints against extension workers, agriculture and research officers, that they hardly pay any visits to address their issues. They expressed their disappointment during interviews and reported that both government and NGOs' representatives visit them for data collection only and do not keep their promises later on. In other studies, Bahta et al. [59], also communicated farmers' dissatisfaction about inadequate support and that government promises were not fulfilled. Similarly, Jordaan [60] and Ngaka [61] in their previous studies also confirm farmers' level of dissatisfaction regarding government assistance during the dry spells of droughts.
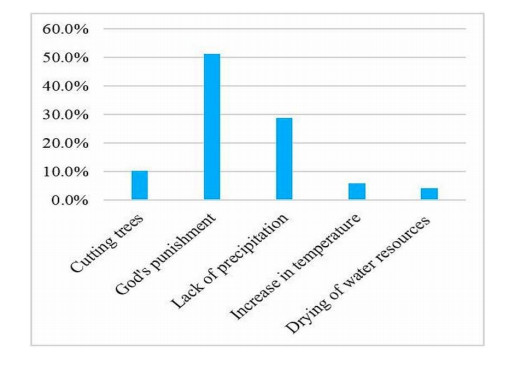
When farmers' perception was asked about the occurrence of drought in the region, some respondents (51.3%) religiously connected drought with human actions, as shown in Figure 4. According to them, Allah is un-happy from our bad deeds and actions. They further explained that the study area remained in tribal feuds for almost two decades and each tribe has enmity with other. Thus, killing, robbing, snatching and other evil activities became very common in the district and they therefore, regarded drought as the form of punishment in return. Similar findings were revealed by Ashraf & Routray [8] and Iqbal [36] where certain elements were connected with religious beliefs. According to Mitchell [62] religious belief is considered as an important element in understanding and responding to natural hazards. Others argue that traditionally natural hazards have been seen as "act of God" or as exoteric forces against which humanity had no defense and that in strictly religious communities, faith and culture can influence perception more often than experience [63,64]. On the other hand, (28.7%) respondents perceived lack of precipitation as the second main cause of drought. This finding is closely in line with the findings of other researchers who believe that not only precipitation is the cause of drought rather it also increases farmers' vulnerability to climate change and variability [65,67]. However, only (10.2%) reported that cutting of trees caused drought. This was also seen in the study of Ashraf & Routray [8] that farmers practiced extra pruning of trees to mitigate the negative impact of drought.
The Figure 5 provides details about farmers' training on early drought preparedness. Majority of the respondents (63.4%) in the study area reported that neither had they ever received any training on drought preparedness, its impacts and early warning system nor any coping methods or adaptation strategies were introduced to them by any of the organizations. It was also reported that there was no drought awareness or drought early warning issued to the farmers until the drought was already in progress. Such complaints were also reported in the study conducted by Fumiso Muyambo [68] in South Africa.
Among other respondents (20.4%) had received drought awareness trainings from INGO/NGOs and only (11.3%) had received training from agriculture department on extension services. According to Bon'sile [69] and Nani Maiya [70] regular training and capacity building to upgrade farmers' skills and knowledge is a pre-requisite to cope with the adverse impact of drought. In the case of Nguyen Thi [71] households who did not have any trainings were more vulnerable to drought, whereas, in the case of Sarah Jane [53] an increase in the income was seen through trainings from the extension services. It is however worth mentioning that, there was only one extension office in the entire district and majority of the respondents (73.6%) did not have access to the office as they lived in far-flung areas. Moreover, local government with (5%) and Provincial Disaster Management Authority (PDMA) with (2%) had very little contribution regarding provision of trainings and awareness sessions on drought and early warning system.
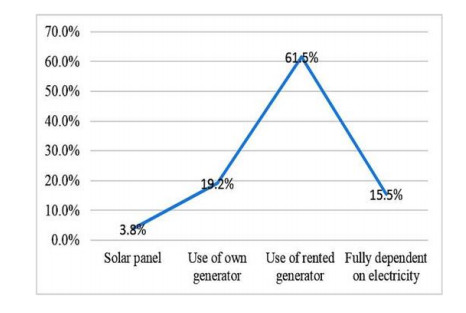
Electricity has remained the main source of power for irrigation purposes. Electricity allows farmers to store their production in cold storage for times of scarcity. It is also essential for production processes, adding value to smallholder farming [72]. At the same time, electricity allows access to information through TV media and telephone. Farmers heavily depended upon electricity in the study area. According to the farmers, limited access to electricity would bring destruction to the farms. Our findings reveal that 15.5% farmers fully depended on electricity.
During the failure of electricity in the field, farmers were compelled to use other means of power to run their tube wells and fetch ground water for their orchard farms. Besides electricity, respondents (61.5%) reported that they rented a generator whereas (19.2%) had their own generators. However, the farmers who were fully dependent on electricity were in a miserable condition because during the failure of electricity, they had no other means of power and their farms were highly likely to go dry. Nevertheless, some farmers also used solar panel as a means of power generation but they are very few in numbers as installation of solar panel is very expensive and quite a few could afford it.
Farmers' perception regarding the land holding size in the field was disappointing. Majority of the farmers reported that due to lack of precipitation and extended drought they faced heavy losses in terms of production. Depletion of water on the other hand further deteriorated the drought situation in the study area and many of the trees in each farm went dry. The farmers therefore, started cutting the dry trees and either would sell them in the market or used them for their domestic needs. This was also considered a kind of coping strategy and a source of income. Such type of trend ultimately resulted into reduction of farm size. The table 3 depicts that the land holding size which was (51.4%) before drought, reduced to (22%) only after the extended drought in the study district, which implies the loss of production on (29.4%) land holding, ranging from 21–30 acres and above. This also indicates that production loss is negatively associated with income and farmers were badly crippled economically. It was also observed in the field, that majority of the farmers who had larger land holding size in past would also have larger farms in the field. However, due to continuous and intense drought, size of the farms shrink to few of the acres only. Some of the farmers completely cut off the trees and quit farming. They tilted towards off-farm activities and migrated to other cities for jobs.
| Before Drought | After Drought | |
| Land holding size (acres) | (%) | (%) |
| 0–10 | 18.6 | 32.7 |
| 11–20 | 30 | 45.3 |
| ≥21–30 | 51.4 | 22 |
| Annual income (PKR) | ||
| 100,000–500,000 | 16.3 | 37.9 |
| 500,001–100,000 | 35.1 | 55 |
| ≥100,000, 1–150,000, 0 | 48.6 | 7.1 |
| Source: Field survey, 2018. | ||
DownLoad:
CSV
It is also of paramount importance to note farmer's annual income i.e. 48.6% farmers used to earn their annual income between PKR 100,000, 0–150,000, 0 (USD 9090–13,636) and above, however, after the drought situation only (7.1%) of the farmers were able to earn the same amount per year. The highest decrease in per annual income can be associated to those farmers who had the larger land holding size. Even though, agriculture production is an important source of income as investigated by Bhandari et al. [73], farmers in the study area had very low production. Overall, an intense reduction has been observed both in land holding size and annual income.
Figure. 7 expresses farmers' perception on means of financial support. The main source of getting loan as per (63%) respondents was the cooperative society called (Kotti-Wala4) in local language. Cooperative societies or social networks always supported farmers largely when they were economically crippled. Such social networks serve as a channel for economic and social support [75]. It was also true in the case of Kuhlicke et al. [75] that community networks are important factors in reducing vulnerability to drought and the findings of Susan Sam [55] also depict that social networking may help households in coping with the negative impacts of drought. However, farmers do not always have access to such networks as in the case of Fumiso Muyambo [68] and Xi Jiao & Hasan Moinuddin [76]. The findings further revealed that (15%) respondents reported that selling of household assets was a secondary source of loan.
4 A cooperative farming society that helps the agriculture community by moneylending and ask farmers sell their production through them whereby they earn commission from both buyers and the sellers.
The selling of household assets had been a kind of support to the small-scale farmers whereby they manage in the face of hardship as a means of resistance or capacity to cope. According to Mishra [77], to overcome the difficult drought situation many people in India are forced to mortgage their land and other household assets like ornaments, utensils, etc. Similar findings were revealed by Iqbal [36] that farmers sell their livestock and other household assets in Afghanistan to encounter the adverse effects of drought. However, Alemayehu and Bewket [78], revealed in their findings that selling of household assets will increase their vulnerability to future shocks.
In addition to that, Asfaw [79], points out that farmers might not be able to recover from and finally will be trapped by a vicious circle of poverty whenever they sell their household assets. It is however, (10%) respondents reported that during the dry spell they borrowed money either from their close relatives or from friends.
Neither were there any such financial schemes run by the bank for the agricultural community nor was there any aid by the INGOs/NGOs, or any civil society organization. The micro-credit program also remained un-successful in the entire region due to interest rate which is strongly discouraged and is taboo in Islamic societies and that is why the villagers also refrained from bank loans, which might have been given for other purposes. The study conducted by Asfaw et al. [79] also reveals that borrowing money from friends/relatives was a means of financial support to the farmers to cope with drought situation. In addition to that, Ashraf & Routray [8] found in their study that borrowing money from friends and relatives was a good source of income for the farmers whereby they would spend that money for agriculture purposes such as digging of well/tube wells and to buy inputs to minimize the production loss incurred due to drought. In extreme drought conditions, farmers in Bangladesh also tilted towards borrowing money from their closed ones as in the case of Habiba et al. [42].
Despite all these, majority of the respondents in the study area were in debt to Kotti-wala i.e. agriculture cooperative society or dealers who lend money upon the condition that farmer will sell their entire production by them. This is because they get a certain amount as a commission from both seller and the buyer. The farmers always remain under compulsion to keep their products with Kotti-wala. However, almost (60%) farmers reported that such type of financial support helped them to some extent.
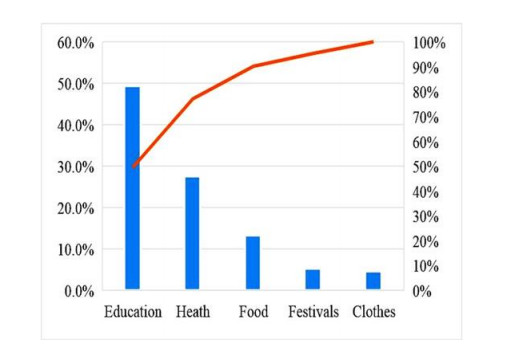
According to the respondents in Figure 8. The extended drought caused heavy losses in the study area. The drought loss has affected health, education, food and farmers' expenditures upon different livelihood items. The economic situation of the farmers crippled badly that many of the farmers did not have access to credit and loans, therefore, their expenditures shrink to a great level. As many as (50%) and (27.5%) respondents reported that due to financial constraints caused by the continuous drought in the region they have reduced expenditure on education and health respectively.
There has been empirical evidence that indicates that consumption smoothing is a known practice among small-scale farmers [80,81]. For example, Bhandari et al. [73], reports that low income households have reduced expenses on health, education and festivals in India during severe droughts.
According to Parmeshwar [45] drought mostly affected the income of low-income farmers forcing them to reduce their expenses on festivals, which has a negative impact on social life and mental health. The reports of other researchers also support our findings that in severe cases of drought, households reduced their expenditures on food, clothes and festivals and some of them see this as important strategies to cope up with drought situation [36,77].
This study has specifically focused on understanding of farmers' perception and attitude towards drought in the drought prone district of Balochistan, province of Pakistan. Drought in Balochistan prevails 3–4 years out of 10 years that increases farmers' vulnerability to drought. Farmers' perception about recurrent drought and climatic variability are in conformity with the results obtained through analyzing climatic data over a period of 36 years, 37 years and that of 113 years by different researchers. The results of this study indicate that farmers were aware of drought and its severity. Majority of the farmers connected drought with religion and making evil actions responsible for its occurrence. Socio-economic and environmental factors were determined regarding farmers' perceptions towards numerous threats and challenges faced by them. The findings of this study show that farmers perceived that prevailing drought severely affected agriculture production, livestock, household income and groundwater usage. In addition to that, in-sufficient electricity, mushroom growth of tube wells and deforestation increased their drought vulnerability.
Farmers in the study area perceived that expenditure's reduction on health, education, festivals and food lead them to poor health, limited access to education, lack of social activities and malnutrition respectively. Moreover, outbreak of certain diseases, lack of training for drought preparedness, abolishment of natural resources, in-sufficient means of electricity, lack of access to credit and prevalence of crime in the study area further increased their vulnerability to the drought. Farmers also perceived lack of adequate support by the government and majority of the farmers were dissatisfied from government support done for sustainable agriculture or irrigation purposes. Existing government or non-government institutions and groups were not functioning effectively to reduce farmers' vulnerability to drought. Some of the farmers relinquished farming completely and switched over to other off-farm activities or migrated to other places for livelihood opportunities, whereas, rest of the farmers were at great risk because of the repeated droughts in the study area which caused substantial loss of productivity and income during the last two decades.
This study provides some recommendations/suggestions with aim to determine and design effective policies by incorporating farmers' perception that may help farmers cope with drought vulnerability.
● PDMA should be well prepared for any forthcoming dry spells and establish early warning system in the district as the founders meant to establish this department only to encounter all natural disasters. In addition to that, District Disaster Management Authority (DDMA) should be activated to deliver services at gross root level.
● Establish strong connection and interaction between farmers and agriculture department to ensure that the farmers benefit from the extension workers and research officer.
● There should be close coordination and collaboration among all stakeholders such as WAPDA, WASA, PDMA, NDMA, NGOs, Agriculture department and private sectors. This will in turn reduce farmers' vulnerability to drought impacts.
● Policy makers and research institutes should provide risk management tools, accurate information on climate variability, access to adequate credit and more off-farm income opportunities, which are needed for farmers to overcome farming management deficiencies regarding risks at the farm level.
● Nonstop use of solar panels for irrigation purposes will overexploit and speedup extraction of groundwater, which will cross the aquifer level before time. Thus, this study recommends, that policy makers should develop new policies regarding efficient use of solar panels and extraction of groundwater with an adequate supply of subsidized electricity.
● Creation of employment and off-farm business activities will provide farmers with an alternative source of income that will help farmers financially under extreme spell of droughts.
● Effective water harvesting techniques contribute substantially to meeting irrigation requirements. Therefore, the policy makers should prioritize providing financial and technical support to farmers for construction and maintenance of water-harvesting structures as well as construction of small dams/reservoirs for water storage as large dams are difficult to construct due to high cost.
● Introduction of drought resistant crops and verities will help farmers in agriculture production and protect them from financial losses.
The orchard farms, livestock and livelihood in study area has been severely affected by the extended drought for the last couple of decades. This study has limitations as only understanding farmers' risk perception and their attitude towards drought vulnerability will not contribute much for developing any plans. In order to understand drought in a holistic manner, it is of paramount importance to employ interdisciplinary approach where each discipline such as meteorological, agricultural, hydrological and socio-economic perspectives will equally and collectively contribute to devise effective policies. Such a direction will be our future course of action.
All authors declare no conflict of interest in this paper.
| [1] | J. Attard, Conformal Gauge Theories, Cartan Geometry and Transitive Lie Algebroids, PhD thesis, Université d'Aix-Marseille, 2018. |
| [2] |
J. Attard, J. Fran |
| [3] |
C. Campos, M. de León, D.M. de Diego, M. Vaquero, Hamilton-Jacobi theory in Cauchy data space, Rep. Math. Phys., 76 (2015), 359–387. https://doi.org/10.1016/S0034-4877(15)30038-0 doi: 10.1016/S0034-4877(15)30038-0
|
| [4] |
J. F. Cariñena, M. Crampin, L. A. Ibort, On the multisymplectic formalism for first order theories, Differ. Geom. Appl., 1 (1991), 354–374. https://doi.org/10.1016/0926-2245(91)90013-Y doi: 10.1016/0926-2245(91)90013-Y
|
| [5] | S. S. Chern, W. H. Chen, K. S. Lam, Lectures on differential geometry, World Scientific Publishing Co., 1999. |
| [6] |
O. Esen, H. Gümral, Lifts, jets and reduced dynamics, Int. J. Geom. Methods Mod. Phys., 8 (2011), 331–344. https://doi.org/10.1142/S0219887811005166 doi: 10.1142/S0219887811005166
|
| [7] |
T. Eguchi, P. B. Gilkey, A. J. Hanson, Gravitation, gauge theories and differential geometry, Phys. Rep., 66 (1980), 213–393. https://doi.org/10.1016/0370-1573(80)90130-1 doi: 10.1016/0370-1573(80)90130-1
|
| [8] | J. M. Figueroa-O'Farrill, Gauge theory, Lecture notes, Available from: https://empg.maths.ed.ac.uk/Activities/GT/. |
| [9] |
C. Fournel, J. Fran
|
| [10] |
J. Fran |
| [11] |
J. Fran
|
| [12] |
K. Grabowska, A Tulczyjew triple for classical fields, J. Phys. A: Math. Theor., 45 (2012), 145207. https://doi.org/10.1088/1751-8113/45/14/145207 doi: 10.1088/1751-8113/45/14/145207
|
| [13] |
K. Grabowska, L. Vitagliano, Tulczyjew triples in higher derivative field theory, J. Geom. Mech., 7 (2015), 1–33. https://doi.org/10.3934/jgm.2015.7.1 doi: 10.3934/jgm.2015.7.1
|
| [14] |
V. N. Gribov, Quantization of non-abelian gauge theories, Nucl. Phys. B, 139 (1978), 1–19. https://doi.org/10.1016/0550-3213(78)90175-X doi: 10.1016/0550-3213(78)90175-X
|
| [15] |
G. S. Guralnik, C. R. Hagen, T. W. B. Kibble, Global conservation laws and massless particles, Phys. Rev. Lett., 13 (1964), 585–587. https://doi.org/10.1103/PhysRevLett.13.585 doi: 10.1103/PhysRevLett.13.585
|
| [16] |
P. W. Higgs, Broken symmetry and the mass of gauge bosons, Phys. Rev. Lett., 13 (1964), 508–509. https://doi.org/10.1103/PhysRevLett.13.508 doi: 10.1103/PhysRevLett.13.508
|
| [17] |
J. Kijowski, W. Szczyrba, A canonical structure for classical field theories, Commun. Math. Phys., 46 (1976), 183–206. https://doi.org/10.1007/BF01608496 doi: 10.1007/BF01608496
|
| [18] |
M. de León, P. D. Prieto-Martínez, N. Román-Roy, S. Vilarino, Hamilton-Jacobi theory in multisymplectic classical field theories, J. Math. Phys., 58 (2017), 092901. https://doi.org/10.1063/1.5004260 doi: 10.1063/1.5004260
|
| [19] | M. de León, S. Vilarino, Methods of Differential Geometry in Classical Field Theories: k-Symplectic and k-Cosymplectic Approaches, World Scientific Publishing Co. Pte. Ltd., Hackensack, NJ, 2016. |
| [20] |
M. de León, M. Zając, Hamilton-Jacobi theory for gauge field theories, J. Geom. Phys. 152 (2020), 103636. https://doi.org/10.1016/j.geomphys.2020.103636 doi: 10.1016/j.geomphys.2020.103636
|
| [21] | T. Masson, J. C. Wallet, A Remark on the Spontaneous Symmetry Breaking Mechanism in the Standard Model, arXiv : 1001.1176., 2011. |
| [22] | K. Nomizu, Lie groups and differential geometry, Publications of the Mathematical Society of Japan, 1956. |
| [23] |
N. Roman-Roy, A. M. Rey, M. Salgado, S. Vilariño, On the k-symplectic, k-cosymplectic and multisymplectic formalism of classical field theories, J. Geom. Mech., 3 (2011), 113–137. https://doi.org/10.3934/jgm.2011.3.113 doi: 10.3934/jgm.2011.3.113
|
| [24] | G. Sardanashvily, Gauge theory in jet manifolds, Hadronic Press Monographs in Applied Mathematics, Hadronic Press, Inc., Palm Harbor, FL, 1993. |
| [25] | G. Sardanashvily, Generalized Hamiltonian formalism for field theory. Constraint systems, World Scientific Publishing Co., Inc., River Edge, NJ, 1995. |
| [26] | D. Saunders, The Geometry of Jet Bundles, Cambridge Univ. Press, Cambridge, 1989. |
| [27] |
I. M. Singer, Some remark on the gribov ambiguity, Comm. Math. Phys., 60 (1978), 712. https://doi.org/10.1007/BF01609471 doi: 10.1007/BF01609471
|
| [28] | S. Sternberg, Group Theory and Physics, Cambridge University Press, 1994. |
| [29] |
J. Śniatycki, O. Esen, De Donder form for second order gravity, J. Geom. Mech., 12 (2020), 85–106. https://doi.org/10.3934/jgm.2020005 doi: 10.3934/jgm.2020005
|
| [30] | A. Trautman, Fiber Bundles, Gauge Field and Gravitation, Gen. Relativ. Gravit., 1 (1980), 287–308. New York, (1979). |
| [31] | E. Witten, The problem of gauge theory. Geometry and analysis, Adv. Lect. Math., 18 (2011), 371–382. |
| [32] | C. Yang, Selected Papers (1945-1980), with Commentary, World Scientific Publishing Company, 2005. |
| 1. | Wilhelmina A.N. Daeng, Titin Siswantining, Alhadi Bustamam, Prasnurzaki Anki, 2022, δ-TRIMAX Method with Silhouette Coefficient on Microarray Gene Expression Data for Early Detection of Heart Failure, 978-1-6654-5140-6, 412, 10.1109/ICOIACT55506.2022.9971865 | |
| 2. | David Gutierrez-Aviles, Jose F. Torres, Francisco Martinez-Alvarez, Jairo Cugliari, 2024, An evolutionary triclustering approach to discover electricity consumption patterns in France, 9798400702433, 386, 10.1145/3605098.3636034 | |
| 3. | Marcin Michalak, Searching for Continuous n-Clusters with Boolean Reasoning, 2024, 16, 2073-8994, 1286, 10.3390/sym16101286 | |
| 4. | Marcin Michalak, Triclustering based on Boolean reasoning — a proof–of–concept, 2024, 246, 18770509, 2351, 10.1016/j.procs.2024.09.538 |
Marcin Zając. The dressing field method in gauge theories - geometric approach[J]. Journal of Geometric Mechanics, 2023, 15(1): 128-146. doi: 10.3934/jgm.2023007
| Variables | Increased | Decreased | No change | No idea | ||||
| No | % | No | % | No | % | No | % | |
| Rainfall Intensity | 0 | 0 | 242 | 91.3 | 7 | 2.6 | 16 | 6 |
| Temperature | 218 | 82.3 | 0 | 0 | 19 | 7.2 | 28 | 10.6 |
| Frequency of drought | 213 | 80.4 | 0 | 0 | 34 | 12.8 | 18 | 6.8 |
| Availability of ground water | 0 | 0 | 251 | 94.7 | 0 | 0 | 14 | 5.3 |
| Length of winter period | 9 | 3.4 | 202 | 76.2 | 17 | 6.4 | 37 | 14 |
| Length of summer period | 208 | 78.5 | 19 | 7.2 | 8 | 3 | 30 | 11.3 |
| Source: Field survey, 2018. | ||||||||
DownLoad:
CSV
| Factors affected | Very Low | Low | Moderate | High | Very High | |||||
| No | % | No | % | No | % | No | % | No | % | |
| Decline in production | 0 | 0 | 9 | 3.4 | 29 | 10.9 | 46 | 17.4 | 181 | 68.3 |
| Livestock losses | 6 | 2.3 | 12 | 4.5 | 22 | 8.3 | 37 | 14 | 188 | 70.9 |
| Sale of household assets | 37 | 14 | 71 | 26.8 | 32 | 12.1 | 110 | 41.5 | 15 | 5.7 |
| Sale of land | 202 | 76.2 | 37 | 14 | 17 | 6.4 | 7 | 2.6 | 2 | 0.8 |
| Migration | 15 | 5.7 | 126 | 47.5 | 39 | 14.7 | 67 | 25.3 | 18 | 6.8 |
| Increase in crime rate | 4 | 1.5 | 14 | 5.3 | 29 | 10.9 | 160 | 60.4 | 58 | 21.9 |
| Depletion of water | 7 | 2.6 | 20 | 7.5 | 60 | 22.6 | 139 | 52.5 | 39 | 14.7 |
| Spread of diseases | 2 | 0.8 | 30 | 11.3 | 97 | 36.6 | 116 | 43.8 | 20 | 7.5 |
| Decrease in household income | 6 | 2.3 | 13 | 4.9 | 36 | 13.6 | 77 | 29.1 | 133 | 50.2 |
| Drop out of school going children | 14 | 5.3 | 47 | 17.7 | 115 | 43.4 | 70 | 26.4 | 19 | 7.2 |
| Farmers' Vulnerability | 6 | 2.3 | 18 | 6.8 | 50 | 18.9 | 71 | 26.8 | 120 | 45.3 |
| Source: Field survey, 2018. | ||||||||||
DownLoad:
CSV
| Before Drought | After Drought | |
| Land holding size (acres) | (%) | (%) |
| 0–10 | 18.6 | 32.7 |
| 11–20 | 30 | 45.3 |
| ≥21–30 | 51.4 | 22 |
| Annual income (PKR) | ||
| 100,000–500,000 | 16.3 | 37.9 |
| 500,001–100,000 | 35.1 | 55 |
| ≥100,000, 1–150,000, 0 | 48.6 | 7.1 |
| Source: Field survey, 2018. | ||
DownLoad:
CSV
| Variables | Increased | Decreased | No change | No idea | ||||
| No | % | No | % | No | % | No | % | |
| Rainfall Intensity | 0 | 0 | 242 | 91.3 | 7 | 2.6 | 16 | 6 |
| Temperature | 218 | 82.3 | 0 | 0 | 19 | 7.2 | 28 | 10.6 |
| Frequency of drought | 213 | 80.4 | 0 | 0 | 34 | 12.8 | 18 | 6.8 |
| Availability of ground water | 0 | 0 | 251 | 94.7 | 0 | 0 | 14 | 5.3 |
| Length of winter period | 9 | 3.4 | 202 | 76.2 | 17 | 6.4 | 37 | 14 |
| Length of summer period | 208 | 78.5 | 19 | 7.2 | 8 | 3 | 30 | 11.3 |
| Source: Field survey, 2018. | ||||||||
| Factors affected | Very Low | Low | Moderate | High | Very High | |||||
| No | % | No | % | No | % | No | % | No | % | |
| Decline in production | 0 | 0 | 9 | 3.4 | 29 | 10.9 | 46 | 17.4 | 181 | 68.3 |
| Livestock losses | 6 | 2.3 | 12 | 4.5 | 22 | 8.3 | 37 | 14 | 188 | 70.9 |
| Sale of household assets | 37 | 14 | 71 | 26.8 | 32 | 12.1 | 110 | 41.5 | 15 | 5.7 |
| Sale of land | 202 | 76.2 | 37 | 14 | 17 | 6.4 | 7 | 2.6 | 2 | 0.8 |
| Migration | 15 | 5.7 | 126 | 47.5 | 39 | 14.7 | 67 | 25.3 | 18 | 6.8 |
| Increase in crime rate | 4 | 1.5 | 14 | 5.3 | 29 | 10.9 | 160 | 60.4 | 58 | 21.9 |
| Depletion of water | 7 | 2.6 | 20 | 7.5 | 60 | 22.6 | 139 | 52.5 | 39 | 14.7 |
| Spread of diseases | 2 | 0.8 | 30 | 11.3 | 97 | 36.6 | 116 | 43.8 | 20 | 7.5 |
| Decrease in household income | 6 | 2.3 | 13 | 4.9 | 36 | 13.6 | 77 | 29.1 | 133 | 50.2 |
| Drop out of school going children | 14 | 5.3 | 47 | 17.7 | 115 | 43.4 | 70 | 26.4 | 19 | 7.2 |
| Farmers' Vulnerability | 6 | 2.3 | 18 | 6.8 | 50 | 18.9 | 71 | 26.8 | 120 | 45.3 |
| Source: Field survey, 2018. | ||||||||||
| Before Drought | After Drought | |
| Land holding size (acres) | (%) | (%) |
| 0–10 | 18.6 | 32.7 |
| 11–20 | 30 | 45.3 |
| ≥21–30 | 51.4 | 22 |
| Annual income (PKR) | ||
| 100,000–500,000 | 16.3 | 37.9 |
| 500,001–100,000 | 35.1 | 55 |
| ≥100,000, 1–150,000, 0 | 48.6 | 7.1 |
| Source: Field survey, 2018. | ||
