Geostatistical modelling of soil contamination with arsenic, cadmium, lead, and nickel: the Silesian voivodeship, Poland case study

Jolanta Kwiatkowska-Malina; Andrzej Szymon Borkowski; Jolanta Kwiatkowska-Malina; Andrzej Szymon Borkowski

doi:10.3934/geosci.2020009

AIMS Geosciences

2020, Volume 6, Issue 2: 135-148. doi: 10.3934/geosci.2020009

Previous Article Next Article

Research article

Geostatistical modelling of soil contamination with arsenic, cadmium, lead, and nickel: the Silesian voivodeship, Poland case study

Department of Spatial Planning and Environmental Sciences, Faculty of Geodesy and Cartography, Warsaw University of Technology, Politechniki Sq. 1, 00-661 Warsaw, Poland

Received: 22 January 2020 Accepted: 23 March 2020 Published: 01 April 2020

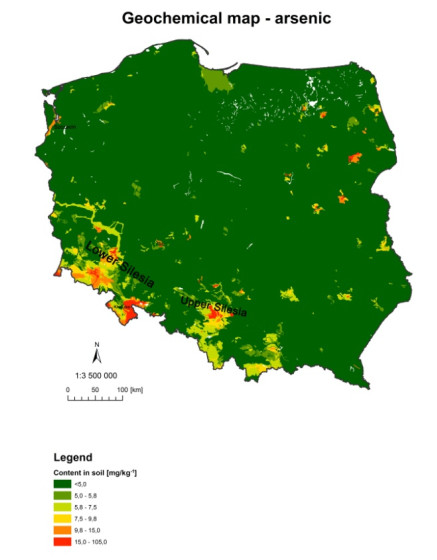
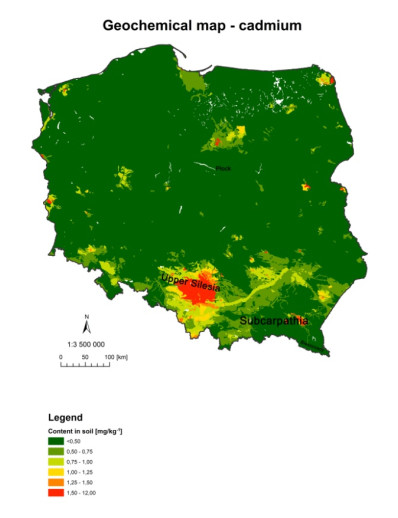
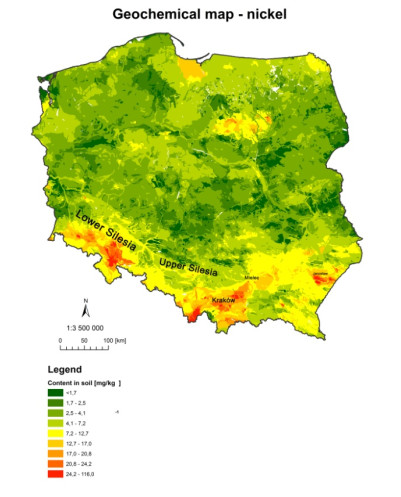
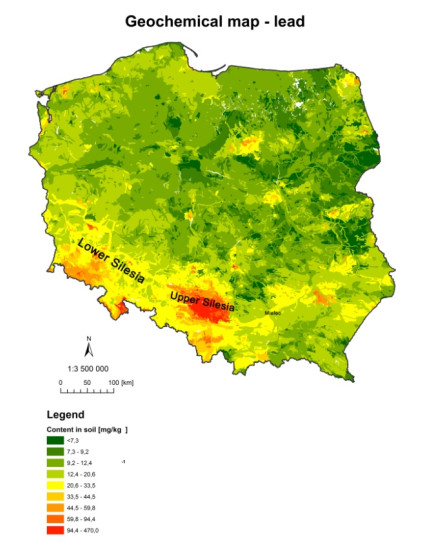
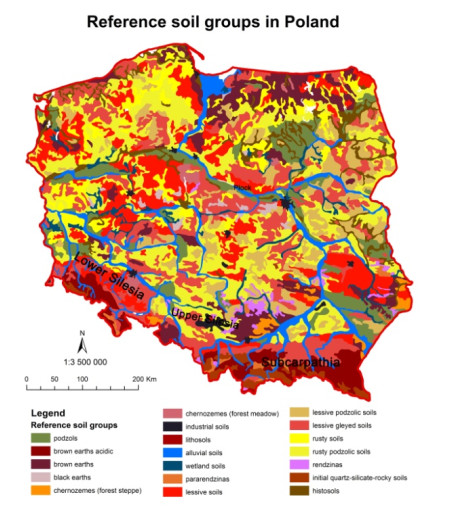
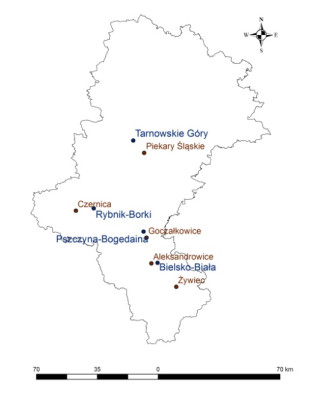
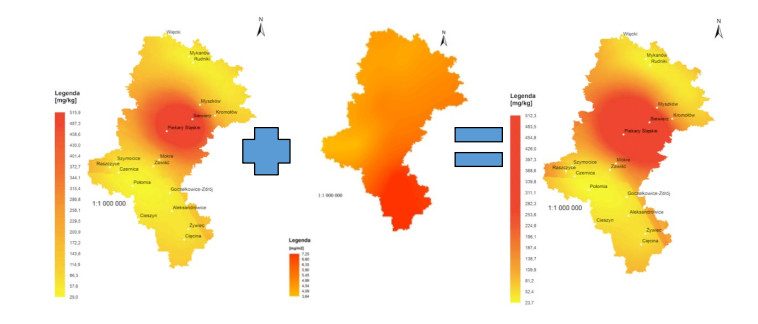
The increasing number of spatial data sets permits their application for minimising the duration and cost of such research. An example of such application is geostatistical modelling. Data on the quality of atmospheric air can be used for the assessment of the quality of soil in a given area. The objective of the study was an attempt to apply geostatistical methods in the estimation of the degree of soil contamination with selected heavy metals resulting from deposition from atmospheric air. This paper uses data obtained from the State Environmental Monitoring (data on the quality of atmospheric air) and other collections of publicly available spatial data for the purpose of analysis of the state of quality of soils in the province of Silesia in Poland. Conducted analyses revealing that contamination with lead in atmospheric air in the Silesian voivodeship considerably exceeds acceptable values, and the load of lead deposition is largely transferred to the soil. The paper also presents geochemical maps necessary to understand sources of soil contamination, including their natural content.

Keywords:

Citation: Jolanta Kwiatkowska-Malina, Andrzej Szymon Borkowski. Geostatistical modelling of soil contamination with arsenic, cadmium, lead, and nickel: the Silesian voivodeship, Poland case study[J]. AIMS Geosciences, 2020, 6(2): 135-148. doi: 10.3934/geosci.2020009

Related Papers:

[1]	Yinwan Cheng, Chao Yang, Bing Yao, Yaqin Luo . Neighbor full sum distinguishing total coloring of Halin graphs. AIMS Mathematics, 2022, 7(4): 6959-6970. doi: 10.3934/math.2022386
[2]	Baolin Ma, Chao Yang . Distinguishing colorings of graphs and their subgraphs. AIMS Mathematics, 2023, 8(11): 26561-26573. doi: 10.3934/math.20231357
[3]	Ningge Huang, Lily Chen . AVD edge-colorings of cubic Halin graphs. AIMS Mathematics, 2023, 8(11): 27820-27839. doi: 10.3934/math.20231423
[4]	Huifen Ge, Shumin Zhang, Chengfu Ye, Rongxia Hao . The generalized 4-connectivity of folded Petersen cube networks. AIMS Mathematics, 2022, 7(8): 14718-14737. doi: 10.3934/math.2022809
[5]	Fugang Chao, Donghan Zhang . Neighbor sum distinguishing total choice number of IC-planar graphs with restrictive conditions. AIMS Mathematics, 2023, 8(6): 13637-13646. doi: 10.3934/math.2023692
[6]	Kai An Sim, Kok Bin Wong . On the cooling number of the generalized Petersen graphs. AIMS Mathematics, 2024, 9(12): 36351-36370. doi: 10.3934/math.20241724
[7]	Bana Al Subaiei, Ahlam AlMulhim, Abolape Deborah Akwu . Vertex-edge perfect Roman domination number. AIMS Mathematics, 2023, 8(9): 21472-21483. doi: 10.3934/math.20231094
[8]	Yanyi Li, Lily Chen . Injective edge coloring of generalized Petersen graphs. AIMS Mathematics, 2021, 6(8): 7929-7943. doi: 10.3934/math.2021460
[9]	Ali Raza, Mobeen Munir, Tasawar Abbas, Sayed M Eldin, Ilyas Khan . Spectrum of prism graph and relation with network related quantities. AIMS Mathematics, 2023, 8(2): 2634-2647. doi: 10.3934/math.2023137
[10]	Bao-Hua Xing, Nurten Urlu Ozalan, Jia-Bao Liu . The degree sequence on tensor and cartesian products of graphs and their omega index. AIMS Mathematics, 2023, 8(7): 16618-16632. doi: 10.3934/math.2023850

Abstract

1. Introduction

Special functions play essential parts in the applied sciences. Quantum mechanics, numerical analysis, and approximation theory are just a few of the many fields where special functions make an appearance, making them extremely important; see, for example, ^[1,2,3]. Many researchers have performed investigations regarding the different types of special functions. For example, the authors of ^[4,5,6] have studied some degenerate polynomials. Some other sequences of polynomials were investigated in ^[7,8].

Orthogonal polynomials are more widely used in approximation theory than non-orthogonal polynomials. The classical orthogonal polynomials, including Jacobi, Hermite, and Laguerre polynomials, have essential roles in solving different types of differential equations (DEs); see, for example, ^[9,10,11,12]. In addition, there are theoretical results concerned with the classical orthogonal polynomials; see, for example, ^[13,14]. Several authors also addressed the non-orthogonal polynomials from both a theoretical and practical perspective; see, for instance, ^{[15,16,17,18,19,20]}.

Chebyshev polynomials (CPs) play essential roles in a wide variety of fields. A trigonometric expression may be found for each of these polynomials. The recurrence formula is the same for all four kinds of CPs, even though their initials differ. They are particular ones of the classical Jacobi polynomials (JPs). All four kinds were extensively utilized in various problems in numerical analysis. For some contributions that employ these polynomials, one can be referred to ^{[21,22,23,24,25,26,27]}. Some modified CPs were constructed to incorporate some initial and boundary conditions imposed on the given DEs. For example, in ^[28], the authors introduced new modified second-kind CPs and utilized them to treat third-order Emden-Fowler singular DEs.

Several articles explore various generalizations of different polynomials and CPs in particular. The authors in ^[29] have utilized generalized shifted CPs for a class of fractional DEs. The authors in ^[30] studied some generalized CPs. Another type of generalized CPs of the second kind was investigated in ^[31]. A novel class of generalized CPs of the first kind was presented by the authors of ^[32]. In addition, they employed these polynomials in conjunction with the Galerkin method to treat multi-term fractional DEs. A type of generalized CPs was theoretically studied in ^[33]. For other studies on generalized polynomials, one can read ^{[34,35,36,37]}.

Several particular polynomial formulae are very useful in numerical analysis and approximation theory. For example, the derivative expressions of given polynomials as combinations of their original ones are helpful to obtain spectral solutions of various DEs. For example, the authors in ^[38] used the derivatives of the generalized third-kind CPs to obtain solutions of even-order DEs. In contrast, the derivatives of the sixth-kind CPs were utilized in ^[39] to solve a type of Burger's DE. In addition, the operational matrix of derivatives can be constructed from the first-order derivative of a given set of polynomials. This matrix is the core of many approaches to solving different types of DEs; see, for example, ^[40,41]. Formulas for connecting and linearizing different polynomials are also of significance. Some nonlinear DEs may be effectively treated using the linearization formulae; see, for example, ^[42]. Several efforts were dedicated to deriving these formulae; see, for example, ^{[43,44,45,46]}.

Hypergeometric functions (HGFs) are essential tools in studying special functions. They provide an expression for nearly all of the well-known functions and polynomials. New formulas for various special functions can be obtained by performing transformations between HGFs (see, for example, ^[44]). It is worthy of mentioning here also that the coefficients of the expressions related to the derivatives, integrals, moments, connection, and linearization formulas are often expressed in terms of HGFs of different arguments (see, for example, ^{[47,48,49,50]}).

The primary objective of this work is to present a class of generalized CPs. The classical JPs are known to include all four types of CPs. In this paper, the introduced generalized sequence differs from the class of classical JPs. Some fundamental formulas of these polynomials are first introduced, and after that, they are utilized to derive other important problems related to special functions, such as connection and linearization problems. In addition, some definite integrals and weighted definite integrals are computed based on some introduced formulas. According to our knowledge, the paper contains many new formulas and a novel approach.

The current article is structured as follows: Section 2 presents some basic formulas of the UCPs and some orthogonal and non-orthogonal polynomials. The main objective of Section 3 is to obtain novel formulas for the derivatives of the UCPs by using combinations of orthogonal and non-orthogonal polynomials. Section 4 aims to develop the inverse formulae to those provided in Section 3. Section 5 presents some linearization formulas for the UCPs. An application to evaluate some new definite integrals based on the application to the derived formulas is given in Section 6. We end the paper with some discussion and suggest some expected future work in Section 7.

2. Some fundamental properties of the UCPs and other well-known polynomials

This section presents some basic characteristics and relations related to the UCPs. Furthermore, an account of some particular polynomials is provided.

2.1. Some basic formulas concerned with the UCPs

Consider the UCPs that can be generated by using the following recursive formula:

$\begin{equation} G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_ {i}(x)-2x\, G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{i-1}(x)+G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{i-2}(x) = 0, \quad G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{0}(x) = \mathcal{A}, \quad G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{1}(x) = \mathcal{B}\, x+\mathcal{R}. \end{equation}$

(2.1)

It is clear that the polynomial solution of (2.1) $G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{i}(x)$ is a polynomial that generalizes all the well-known four kinds of CPs. The following identities hold:

$\begin{align} T_{i}(x) = &G^{1, 1, 0}_{i}(x), &&U_{i}(x) = G^{1, 2, 0}_{i}(x), \end{align}$

(2.2)

$\begin{align} V_{i}(x) = &G^{1, 2, -1}_{i}(x), &&W_{i}(x) = G^{1, 2, 1}_{i}(x), \end{align}$

(2.3)

where $T_{i}(x), U_{i}(x), V_{i}(x)$ and $W_{i}(x)$ are respectively the well-known four kinds of CPs.

Remark 2.1. Although the four kinds of CPs are special cases of $G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{i}(x)$ as seen from (2.2) and (2.3), they are also special ones of the JPs, but the class of polynomials $G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{i}(x)$ is another generalized class that differs from the JPs class.

Remark 2.2. The analytic formula and its inversion formula are widely recognized as fundamental tools for the theoretical investigation of any collection of polynomials. The following section will provide the derivation of the fundamental formulae for $G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{i}(x)$ .

Now, we will prove a significant theorem, in which we show that $G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{i}(x)$ can be represented as a combination of three consecutive terms of the second-kind CPs.

Theorem 2.1. For all $j\ge 0$ , we have

$\begin{equation} G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{j}(x) = \frac{1}{2} (-2 \mathcal{A}+\mathcal{B}) U_{j-2}(x)+\mathcal{R} U_{j-1}(x)+\frac{\mathcal{B}}{2}\, U_{j}(x). \end{equation}$

(2.4)

Proof. First, assume the polynomial:

$\begin{equation} \theta_{j}(x) = \frac{1}{2} (-2 \mathcal{A}+\mathcal{B}) U_{j-2}(x)+\mathcal{R} U_{j-1}(x)+\frac{\mathcal{B}}{2}\, U_{j}(x). \end{equation}$

(2.5)

Noting that $U_{-2}(x) = -1, \ U_{-1}(x) = 0$ . Obviously, $\theta_{0}(x) = \mathcal{A}, \ \theta_{1}(x) = \mathcal{B}\, x+\mathcal{R}$ . This shows that $\theta_{0}(x) = G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{0}(x)$ and $\theta_{1}(x) = G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{1}(x)$ . So, to prove that $\theta_{j}(x) = G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{j}(x), \ \forall\ j\ge 2$ , it is sufficient to show that they satisfy the same recurrence relation. So, we are going to show the satisfaction of the following recurrence relation for all $j\ge 0$

$\begin{equation} \begin{split} \theta_{j+2}(x)-2\, x\, \theta_{j+1}(x)+\theta_{j}(x) = 0. \end{split} \end{equation}$

(2.6)

Now, we have

$\begin{equation} \begin{split} \theta_{j+2}(x)-2\, x\, \theta_{j+1}(x)+\theta_{j}(x) = &\frac{1}{2} (-2 \mathcal{A}+\mathcal{B}) U_{j}(x)+\mathcal{R} U_{j+1}(x)+\frac{1}{2} \mathcal{B} U_{j+2}(x)\\ &-2 x \left(\frac{1}{2} (-2 \mathcal{A}+\mathcal{B})U_{j-1}(x)+\mathcal{R} U_{j}(x)+\frac{1}{2} \mathcal{B} U_{j+1}(x)\right)\\ &+\frac{1}{2} (-2 \mathcal{A}+\mathcal{B}) U_{j-2}(x)+\mathcal{R} U_{j-1}(x)+\frac{\mathcal{B}}{2}\, U_{j}(x). \end{split} \end{equation}$

(2.7)

If we substitute by the recurrence relation of $U_{j}(x)$ written in the form

$\begin{equation} x\, U_{j}(x) = \frac12\left(U_{j-1}(x)+U_{j+1}(x)\right), \end{equation}$

(2.8)

in the right-hand side of (2.7), then it is not difficult to show that

$\begin{equation} \theta_{j+2}(x)-2\, x\, \theta_{j+1}(x)+\theta_{j}(x) = 0. \end{equation}$

(2.9)

Theorem 2.1 is now proved. □

Now, based on the above theorem, an explicit analytic formula of $G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{i}(x)$ can be deduced.

Theorem 2.2. For every positive number $i$ , the following analytic formula holds:

$\begin{equation} \begin{split} G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{i}(x) = &\sum _{r = 0}^{\left\lfloor \frac{i}{2}\right\rfloor } \frac{(-1)^r 2^{-1+i-2 r} (\mathcal{B} (i-2 r)+2 \mathcal{A} r) (1+i-2 r)_{r-1}}{r!}\, x^{i-2 r}\\ &+\mathcal{R}\, \sum _{r = 0}^{\left\lfloor \frac{i-1}{2}\right\rfloor } \frac{(-1)^r 2^{-1+i-2 r}(i-2 r)_r}{r!}\, x^{i-2 r-1}. \end{split} \end{equation}$

(2.10)

Proof. Based on the expression in (2.4) along with the explicit expression of $U_{j}(x)$ given by

$U_{j}(x) = \sum\limits _{r = 0}^{\left\lfloor \frac{j}{2}\right\rfloor } \frac{(-1)^r 2^{j-2 r} (j-r)!}{(j-2 r)! r!} x^{j-2 r}.$

Formula (2.10) can be obtained. □

The theorems presented next provide the formulae for calculating the moments and inversion of the polynomials $G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{i}(x)$ .

Theorem 2.3. For all non-negative integers (NNIs) $i$ and $m$ , the following moment formula holds

$\begin{equation} x^m\, G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{i}(x) = \frac{1}{2^m}\, \sum\limits_{r = 0}^m \binom{m}{r} G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{i+m-2 r}(x). \end{equation}$

(2.11)

Proof. Easy by applying induction on $m$ based on the recurrence relation (2.1). □

Corollary 2.1. The next formula applies to every non-negative integer (NNI) $m$ .

$\begin{equation} x^m = \frac{1}{2^m\, \mathcal{A}} \sum\limits_{r = 0}^m \binom{m}{r} G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{m-2r}(x). \end{equation}$

(2.12)

Proof. Direct by setting $i = 0$ in Formula (2.11). □

It is useful to introduce the shifted polynomials for $G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{j}(x)$ defined as

$\tilde{G}^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{j}(x) = G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{j}(2x-1).$

The recurrence relation that these polynomials fulfill is

$\begin{equation} \tilde{G}^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{j+2}(x)-2\, (2\, x-1)\, \tilde{G}^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{j+1}(x)+\tilde{G}^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{j}(x) = 0, \quad \tilde{G}^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{0}(x) = \mathcal{A}, \ \tilde{G}^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{0}(x) = \mathcal{R}-\mathcal{B}+2\, \mathcal{B}\, x. \end{equation}$

(2.13)

Now, we give the counterparts results for Theorem 2.3 and Corollary 2.1 for the shifted polynomials $\tilde{G}_{j}^{\mathcal{A}, \mathcal{B}, \mathcal{R}}$ .

Theorem 2.4. The following moment formula applies for all NNIs $r$ and $n$

$\begin{equation} x^r\, \tilde{G}^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{n}(x) = \frac{1}{2^{2r}}\, \sum\limits_{\ell = 0}^{2 r} \binom{2 r}{\ell}\, \tilde{G}^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{n+r-\ell}(x). \end{equation}$

(2.14)

Proof. We can proceed with the proof by induction based on the recurrence relation (2.13). □

Corollary 2.2. The inversion formula of $\tilde{G}^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{j}(x)$ for every NNI $r$ is shown below

$\begin{equation} x^r = \frac{1}{\mathcal{A}\, 2^{2r}} \sum\limits_{\ell = 0}^{2 r} \binom{2 r}{\ell}\, \tilde{G}^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{r-\ell}(x). \end{equation}$

(2.15)

Proof. This formula is a direct special case of Formula (2.14) setting $n = 0$ . □

2.2. An overview on well-known polynomials: both symmetric and nonsymmetric

An introduction to symmetric and nonsymmetric polynomials, both orthogonal and non-orthogonal, is provided in this section.

Let $\phi_{j}(x)$ and $\psi_{j}(x)$ represent, respectively, two sets of symmetric and nonsymmetric polynomials that have the following expressions:

$\begin{align} \phi_{j}(x) = &\sum _{r = 0}^{\left\lfloor \frac{j}{2}\right\rfloor } A_{r, j}\, x^{j-2r}, \end{align}$

(2.16)

$\begin{align} \psi_{j}(x) = &\sum _{r = 0}^{j} B_{r, j}\, x^{j-r}, \end{align}$

(2.17)

where $A_{r, j}$ and $B_{r, j}$ are known coefficients.

Assume also that the inverse formulas to (2.16) and (2.17) can be written as

$\begin{equation} x^j = \sum\limits_{r = 0}^{\left\lfloor \frac{j}{2}\right\rfloor } \bar{A}_{r, j}\, \phi_{j-2r}(x), \end{equation}$

(2.18)

$\begin{equation} x^j = \sum\limits_{r = 0}^{j} \bar{B}_{r, j}\, \psi_{j-r}(x), \end{equation}$

(2.19)

with the known coefficients $\bar{A}_{r, j}$ and $\bar{B}_{r, j}$ .

Among the nonsymmetric polynomials is the general class of the classical JPs, which includes some symmetric classes. The JPs have the following hypergeometric expression:

$\mathcal{P}_{s}^{(\rho, \mu)}(x) = \frac{(\rho+1)_{s}}{s!\, }\ _{2}F_{1}\left.\left( \begin{array}{cc} -s, s+\rho+\mu+1\\ \rho+1 \end{array} \right|\frac{1-x}{2}\right).$

The shifted JPs on $[0, 1]$ can be defined as

$\tilde{P}^{(\rho, \mu)}_{s}(x) = P^{(\rho, \mu)}_{s}(2x-1).$

The ultraspherical polynomials are defined as

$\begin{equation} U_{s}^{(\rho )}(x) = \frac{s!\, \Gamma(\rho +\frac{1}{2})}{\Gamma (s+\rho +\frac{1}{2})}\, P_{s}^{(\rho -\frac{1}{2}, \rho-\frac{1}{2})}(x). \end{equation}$

(2.20)

For a survey on the classical orthogonal polynomials, one can consult ^[51,52].

Now, we give two classes of non-orthogonal polynomials: generalized Fibonacci and generalized Lucas polynomials. $F^{a, b}_{r}(x)$ and $L^{c, d}_{r}(x)$ that were studied ^[53] can be constructed respectively as

$\begin{equation} F^{a, b}_{r}(x) = a\, x\, F^{a, b}_{r-1}(x)+b\, F^{a, b}_{r-2}(x), \quad F^{a, b}_{0}(x) = 1, \ F^{a, b}_{1}(x) = a\, x, \quad r\ge 2, \end{equation}$

(2.21)

$\begin{equation} L^{c, d}_{r}(x) = c\, x\, L^{c, d}_{r-1}(x)+d\, L^{c, d}_{r-2}(x), \quad L^{c, d}_{0}(x) = 2, \ L^{c, d}_{1}(x) = c\, x, \quad r\ge 2. \end{equation}$

(2.22)

Remark 2.3. Important formulas concerning certain polynomials can be derived from their power form representation and associated inversion formula. The four formulas in (2.16)–(2.19) are expressions for the analytic forms and their inversion ones for symmetric and nonsymmetric polynomials. It is clear that these formulas are known if the coefficients $A_{r, j}$ , $B_{r, j}$ , $\bar{A}_{r, j}$ , and $\bar{B}_{r, j}$ are determined. In the following table, we give these coefficients for a group of celebrated polynomials that we will use in this paper. The coefficients for the shifted Jacobi polynomials (SJPs), ultraspherical polynomials (UPs), generalized Laguerre polynomials (LGPs), Hermite polynomials (HPs), generalized Fibonacci polynomials (GFPs), generalized Lucas polynomials (GLuPs), and Bernoulli polynomials (BPs) will be listed in Table 1.

Table 1. Coefficients for analytic forms and their inversion formulas.

Polynomial	$A_{r, j} \, \left(B_{r, j}\right)$	$\bar{A}_{r, j} \, \left(\bar{B}_{r, j}\right)$
SJPs	$\frac{(-1)^r \, (1+\mu)_j \, (1+\rho+\mu)_{2j-r}}{(j-r)! \, r! \, (1+\mu)_{j-r} \, (1+\rho+\mu)_j}$	$\frac{j! \, \Gamma(1+j+\mu) \, (1+2j-2r+\rho+\mu) \, \Gamma(1+j-r+\rho+\mu)}{r! \, \Gamma(1+j-r+\mu) \, \Gamma(2+2j-r+\rho+\mu)}$
UPs	$\frac{(-1)^r \, 2^{j-2r} \, j! \, \Gamma(2\zeta+1) \, \Gamma(j-r+\zeta)}{2 \, (j-2r)! \, r! \, \Gamma(\zeta+1) \, \Gamma(j+2\zeta)}$	$\frac{2^{-j+1} \, (j-2r+\zeta) \, j! \, \Gamma(\zeta+1) \, \Gamma(j-2r+2\zeta)}{(j-2r)! \, r! \, \Gamma(2\zeta+1) \, \Gamma(1+j-r+\zeta)}$
GLPs	$\frac{(-1)^{j-r} \, \binom{j}{r} \, \Gamma(1+j+\rho)}{j! \, \Gamma(1+j-r+\rho)}$	$\frac{(-1)^{-r+j} \, j! \, \Gamma(1+j+\rho)}{r! \, \Gamma(1-r+j+\rho)}$
HPs	$\frac{j! \, (-1)^r \, 2^{j-2r}}{r! \, (j-2r)!}$	$\frac{j!}{2^j \, r! \, (j-2r)!}$
GFPs	$\frac{a^{j-2r} \, b^r \, (1+j-2r)_r}{r!}$	$\frac{(-1)^r \, a^{-j} \, b^r \, (1+j-2r) \, (2+j-r)_{r-1}}{r!}$
GLuPs	$\frac{c^{j-2r} \, d^r \, j \, (1+j-2r)_{r-1}}{r!}$	$\frac{(-1)^r \, c^{-j} \, d^r \, \xi_{j-2r}\, (1+j-r)_r}{r!}$
BPs	$\binom{\ell}{r} \, B_r$	$\frac{\binom{j+1}{j-r}}{j+1}$

| Show Table

DownLoad: CSV

Note that $B_{r}$ in the last row of the table represents the well-known Bernoulli polynomials, where $\xi_{r}$ in the column before the last one is defined as

$\xi_{r} = \begin{cases} \frac12, & r = 0, \\ 1, & r\ge 0. \end{cases}$

3. Some formulas for the derivatives of the UCPs

This section aims to develop new derivative formulas of the UCPs using various other polynomials. The formulae relating the UCPs to other polynomials may be obtained as special cases. More specifically, we'll establish the following expressions:

● The derivatives' expressions of the UCPs in terms of other parameters' UCPs.

● The derivatives expressions of UCPs in terms of some other orthogonal and non-orthogonal polynomials.

3.1. Derivatives of UCPs in terms of other UCPs

We consider the two different UCPs $G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{i}(x)$ and $G^{\bar{\mathcal{A}}, \bar{\mathcal{B}}, \bar{\mathcal{R}}}_{i}(x)$ . For convenience, we will denote $G_{i}(x) = G^{\mathcal{A}, \mathcal{B}, \mathcal{R}}_{i}(x)$ and $\bar{G}_{i}(x) = G^{\bar{\mathcal{A}}, \bar{\mathcal{B}}, \bar{\mathcal{R}}}_{i}(x)$ . We will derive a novel expression that relates the derivatives of $G_{i}(x)$ to $\bar{G}_{i}(x)$ . To begin, it is necessary to establish the following lemma.

Lemma 3.1. Consider a NNI $v$ . We have

$\begin{equation} \begin{split} &\sum _{\ell = 0}^v\, \frac{(-1)^{\ell+1}\, (-\mathcal{B} j-2 \mathcal{A} \ell+2 \mathcal{B} \ell)\, (j-\ell-1)!}{\ell!\, (v-\ell)!\, (j-\ell-v-s)!}\\ & = \frac{(j-v-1)!\, (v+s-1)!\, (-2 \mathcal{B} v (v+s)+2 \mathcal{A} v (-j+v+s)+\mathcal{B} j (2 v+s))}{v!\, s!\, (j-v-s)!}. \end{split} \end{equation}$

(3.1)

Proof. If we let

$\begin{split} &Z_{v, j, s} = \sum _{\ell = 0}^v \frac{(-1)^{\ell+1}\, (-\mathcal{B} j-2 \mathcal{A} \ell+2 \mathcal{B} \ell)\, (j-\ell-1)!}{\ell!\, (v-\ell)!\, (j-\ell-v-s)!}, \end{split}$

then based on Zeilberger's algorithm (^[54]), it is possible to show that $Z_{v, j, s}$ fulfills the following recursive formula

$\begin{split} &(v+s-1)\, (v+s-j-1) \left(2 \mathcal{A} j v-2 \mathcal{B} j v-2 \mathcal{A} v^2+2 \mathcal{B} v^2-\mathcal{B} j s-2 \mathcal{A} v s+2 \mathcal{B} v s\right)\, Z_{v-1, j, s} \\&+(j-v)\, v\, \left(-2 \mathcal{A}+2 \mathcal{B}-2 \mathcal{A} j+2 \mathcal{B} j+4 \mathcal{A} v-4 \mathcal{B} v+2 \mathcal{A} j v-2 \mathcal{B} j v-2 \mathcal{A} v^2+2 \mathcal{B} v^2+2 \mathcal{A} s\right.\\ &\left.-2 \mathcal{B} s-\mathcal{B} j s-2 \mathcal{A} v s+2 \mathcal{B} v s\right) \, Z_{v, j, s} = 0, \end{split}$

with the initial value: $Z_{0, j, s} = 1$ . This first-order recursive formula can be solved quickly to produce

$Z_{v, j, s} = \frac{ (j-v-1)!\, (v+s-1)!\, (-2 \mathcal{B} v (v+s)+2 \mathcal{A} v(-j+v+s)+\mathcal{B} j (2 v+s))}{v!\, s!\, (j-v-s)!}.$

This proves the lemma. □

Theorem 3.1. Consider the NNIs $j$ and $s$ with $j\ge s\ge 1$ . We have

$\begin{equation} \begin{split} D^s G_{j}(x)& = \frac{2^{s-1}}{\bar{\mathcal{A}}\, s!} \sum _{v = 0}^{j-s} \frac{ (j-v-1)!\, (v+s-1)!\, (-2 \mathcal{B} v (v+s)+2 \mathcal{A} v (-j+v+s)+\mathcal{B} j (2 v+s))}{v! (j-v-s)!}\, \bar{G}_{j-s-2v}(x)\\ &+\frac{2^s \mathcal{R} (j-1)!}{\bar{\mathcal{A}}} \sum _{v = 0}^{j-s-1} \frac{(1+s)_v}{v! (j-v-s-1)! (j-v)_v}\, \bar{G}_{j-s-2 v-1}(x). \end{split} \end{equation}$

(3.2)

Proof. From the analytic form in (2.10), it is not difficult to express $D^s G_{j}(x)$ in the form

$\begin{equation} \begin{split} D^s G_{j}(x) = & \sum _{r = 0}^{\left\lfloor \frac{j-s}{2}\right\rfloor } \frac{(-1)^r\, 2^{j-2 r-1}\, (\mathcal{B} (j-2 r)+2 \mathcal{A} r)\, (j-2 r+1)_{r-1} (j-s-2 r+1)_s}{r!}\, x^{j-2 r-s}\\ &+\mathcal{R} \sum _{r = 0}^{\left\lfloor \frac{1}{2} (j-s-1)\right\rfloor } \frac{(-1)^r\, 2^{j-2 r-1}\, (j-2 r)_r\, (j-s-2 r)_s}{r!}\, x^{j-2 r-s-1}. \end{split} \end{equation}$

(3.3)

Utilizing the inversion formula (2.12), we are able to get the expression

$\begin{equation} \begin{split} D^s G_{j}(x)& = \frac{2^{s-1}}{\bar{\mathcal{A}}} \sum _{r = 0}^{\left\lfloor \frac{j-s}{2}\right\rfloor } \frac{(-1)^r\, (\mathcal{B} (j-2 r)+2 \mathcal{A} r)\, (j-r-1)!}{(j-s-2 r)! r!} \sum _{t = 0}^{j-s-2 r} \binom{j-s-2 r}{t} {\bar{G}}_{j-2 r-s-2 t}(x)\\ &+\frac{2^s\, \mathcal{R}}{\bar{\mathcal{A}}}\, \sum _{r = 0}^{\left\lfloor \frac{1}{2} (j-s-1)\right\rfloor } \frac{(-1)^r\, (j-2 r)_r (j-s-2 r)_s}{ r!} \sum _{t = 0}^{j-s-2 r-1} \binom{j-s-2 r-1}{t}\, {\bar{G}}_{j-2 r-s-2 t-1}(x). \end{split} \end{equation}$

(3.4)

Following several algebraic calculations, the preceding formula may be written in the following way:

$\begin{equation} \begin{split} D^s G_{j}(x) = &\frac{2^{s-1}}{\bar{\mathcal{A}}\, s!} \sum _{v = 0}^{j-s} \sum _{\ell = 0}^v \frac{(-1)^{\ell+1} (-\mathcal{B} j-2 \mathcal{A} \ell+2 \mathcal{B} \ell) (j-\ell-1)!}{\ell! (v-\ell)! (j-\ell-v-s)!}{\bar{G}}_{j-s-2v}(x)\\ &+\frac{2^s \mathcal{R}}{\bar{\mathcal{A}}}\, \sum _{v = 0}^{j-s-1} \sum _{\ell = 0}^v \frac{(-1)^\ell (j-\ell-1)!}{\ell! (v-\ell)! (j-\ell-v-s-1)!}{\bar{G}}_{j-s-2 v-1}(x). \end{split} \end{equation}$

(3.5)

Now, to obtain a more simplified formula of (3.5), we make use of the transformation formula:

$\begin{equation} \begin{split} &\sum _{\ell = 0}^v\, \frac{(-1)^\ell\, (j-\ell-1)!}{\ell!\, (v-\ell)!\, (j-\ell-v-s-1)!} = \frac{(j-1)!}{v!\, (j-v-s-1)!}\, \ _{2} F_{1}\left.\left( \begin{array}{cccc} -v, 1-j+v+s\\ 1-j \end{array} \right|1\right), \end{split} \end{equation}$

(3.6)

and accordingly, the Chu-Vandermonde identity (^[51]) can be used to get

$\begin{equation} \sum\limits_{\ell = 0}^v \frac{(-1)^\ell\, (j-\ell-1)!}{\ell!\, (v-\ell)!\, (j-\ell-v-s-1)!} = \frac{(s+1)_v\, (j-v-1)!}{v!\, (j-v-s-1)!}. \end{equation}$

(3.7)

Thanks to (3.7) along with Lemma 3.1, Formula (3.5) is transformed into the following simplified one:

$\begin{split} D^s G_{j}(x) = &\frac{2^{s-1}}{\bar{\mathcal{A}}\, s!} \sum _{v = 0}^{j-s} \frac{ (j-v-1)!\, (v+s-1)!\, (-2 \mathcal{B} v (v+s)+2 \mathcal{A} v (-j+v+s)+\mathcal{B} j (2 v+s))}{v! (j-v-s)!}\, \bar{G}_{j-s-2v}(x)\\ &+\frac{2^s \mathcal{R} (j-1)!}{\bar{\mathcal{A}}}\, \sum _{v = 0}^{j-s-1} \frac{(1+s)_v}{v! (j-v-s-1)! (j-v)_v}\, \bar{G}_{j-s-2 v-1}(x). \end{split}$

This proves Theorem 3.1. □

Remark 3.1. Since Formula (3.2) expresses the derivatives formula of $G_{j}(x)$ in terms of $\bar{G}_{j}(x)$ , so many special formulas can be deduced taking into consideration the four special cases in (2.2) and (2.3). We will now demonstrate these results.

Corollary 3.1. For all $j\ge s\ge 1$ , the following expressions hold

$\begin{align} &D^s T_{j}(x) = \frac{2^{s-1} j}{\bar{A} (s-1)!} \sum _{v = 0}^{j-s} \frac{(j-v-1)! (v+s-1)!}{v! (j-v-s)!}\, G_{j-s-2 v}(x), \end{align}$

(3.8)

$\begin{align} &D^s U_{j}(x) = \frac{2^s}{\bar{A} s!} \sum _{v = 0}^{j-s} \frac{(j-v)! (v+s)!}{v! (j-v-s)!} \, G_{j-s-2 v}(x), \end{align}$

(3.9)

$\begin{align} \begin{split} &D^s V_{j}(x) = \frac{2^s}{\bar{A} s!} \sum _{v = 0}^{j-s} \frac{(j-v)! (v+s)!}{v! (j-v-s)!}\, G_{j-s-2 v}(x) -\frac{2^s}{\bar{A}}\, \sum _{v = 0}^{j-s} \frac{(v+s)!\, (j-v-1)!}{v!\, s!\, (j-v-s-1)!}\, G_{j-s-2 v-1}(x), \end{split} \end{align}$

(3.10)

$\begin{align} \begin{split} &D^s W_{j}(x) = \frac{2^s}{\bar{A} s!} \sum _{v = 0}^{j-s} \frac{(j-v)! (v+s)!}{v! (j-v-s)!} G_{j-s-2 v}(x) +\frac{2^s }{\bar{A}}\, \sum _{v = 0}^{j-s} \frac{(v+s)!\, (j-v-1)!}{v!\, s!\, (j-v-s-1)!}\, G_{j-s-2 v-1}(x). \end{split} \end{align}$

(3.11)

3.2. Derivatives with some other symmetric and nonsymmetric polynomials

This section is interested in deriving some other derivative expressions of the UCPs but in terms of various symmetric and nonsymmetric polynomials. Some of these polynomials are orthogonal, and some others are non-orthogonal.

Theorem 3.2. Consider the NNIs $j$ and $s$ with $j\ge s$ , We have the following derivative expressions as combinations of $U^{(\zeta)}_{j}(x)$

$\begin{equation} \begin{split} D^s &G_{j}(x) = \frac{2^{s-2 \zeta }\, \sqrt{\pi }}{\Gamma (1+s-\zeta )\, \Gamma \left(\frac{1}{2}+\zeta \right)}\times\sum _{v = 0}^{\left\lfloor \frac{j-s}{2}\right\rfloor } \frac{(-j+2 v+s-\zeta )\, (j-v-1)!\, \Gamma (v+s-\zeta )\, \Gamma (j-2 v-s+2 \zeta )}{v!\, (j-2 v-s)!\, \Gamma (1+j-v-s+\zeta )}\\ &\times(2 \mathcal{B} v (v+s-\zeta )+\mathcal{B} j (-2 v-s+\zeta )+2 \mathcal{A} v (j-v-s+\zeta ))\, U^{(\zeta)}_{j-s-2v}(x) +\frac{2^{1+s-2 \zeta }\, \sqrt{\pi }\, \mathcal{R}\, (j-1)!}{\Gamma \left(\frac{1}{2}+\zeta \right)}\\ &\times\sum _{v = 0}^{\left\lfloor \frac{1}{2} (j-s-1)\right\rfloor } \frac{(j-2 v-s+\zeta -1)\, \Gamma (-1+j-2 v-s+2 \zeta )\, (1+s-\zeta )_v}{v!\, (j-2 v-s-1)!\, \Gamma (j-v-s+\zeta )\, (j-v)_v} \, U^{(\zeta)}_{j-s-2v-1}(x). \end{split} \end{equation}$

(3.12)

Proof. Starting from the expression of $D^s G_{j}(x)$ along with the inversion formula of the ultraspherical polynomials leads to the following formula:

$\begin{equation} \begin{split} D^s G_{j}(x) = &\frac{2^s\, \Gamma (1+\zeta )}{\Gamma (1+2 \zeta )} \sum _{r = 0}^{\left\lfloor \frac{j}{2}\right\rfloor }\frac{(-1)^r\, (\mathcal{B} (j-2 r)+2 \mathcal{A} r)\, (j-r-1)!}{r!}\\ &\times\sum _{t = 0}^{\left\lfloor \frac{j-s}{2}\right\rfloor -r} \frac{(j-s-2 (r+t)+\zeta ) \Gamma (j-s-2 (r+t-\zeta ))}{t! \Gamma (1+j-s-2 r-2 t) \Gamma (1+j-s-2 r-t+\zeta )}\, U^{(\zeta)}_{j-2 r-s-2 t}(x)\\ &+\frac{2^{s+1}\, \mathcal{R}\, \Gamma (1+\zeta )}{\Gamma (1+2 \zeta )}\, \sum _{r = 0}^{\left\lfloor \frac{j}{2}\right\rfloor } \frac{(-1)^r\, (j-r-1)!}{r!}\\ &\times\sum _{t = 0}^{\left\lfloor \frac{1}{2} (j-s-1)\right\rfloor -r} \frac{(-1+j-s-2 r-2 t+\zeta )\, \Gamma (-1+j-s-2 r-2 t+2 \zeta )}{t!\, \Gamma (j-s-2 (r+t))\ \Gamma (j-s-2 r-t+\zeta )}\, U^{(\zeta)}_{j-2 r-s-2 t-1}(x). \end{split} \end{equation}$

(3.13)

The above relation can be set in the following form

$\begin{equation} \begin{split} D^s G_{j}(x) = &\frac{2^s\, \Gamma (\zeta +1)}{\Gamma (2 \zeta +1)} \left(\sum _{v = 0}^{\left\lfloor \frac{j-s}{2}\right\rfloor } \frac{(j-2 v-s+\zeta ) \, \Gamma (j-2 v-s+2 \zeta )}{(j-2 v-s)!}\right.\\ &\times\sum _{r = 0}^v \frac{(-1)^{r+1}\, (-\mathcal{B} j-2 \mathcal{A} r+2 \mathcal{B} r)\, (j-r-1)!}{(v-r)!\, r!\, \Gamma (j-v-s-r+\zeta +1)}\, U^{(\zeta)}_{j-s-2v}(x)\\ &+2\, \mathcal{R}\, \sum _{v = 0}^{\left\lfloor \frac{1}{2} (j-s-1)\right\rfloor } \frac{(-j+2 v+s-\zeta +1)\, \Gamma (j-2 v-s+2 \zeta -1)}{(j-2 v-s-1)!}\\ &\left.\sum _{r = 0}^v \frac{(-1)^{r+1} (j-r-1)!}{(v-r)!\, r!\, \Gamma (j-v-s-r+\zeta )}\, U^{(\zeta)}_{j-s-2v-1}(x)\right). \end{split} \end{equation}$

(3.14)

Zeilberger's algorithm aids in finding the following closed forms:

$\begin{align} \begin{split} &\sum _{r = 0}^v \frac{(-1)^{r+1}\, (-\mathcal{B} j-2 \mathcal{A} r+2 \mathcal{B} r)\, (j-r-1)!}{(v-r)!\, r!\, \Gamma (j-v-s-r+\zeta +1)}\\ & = \frac{(-2 \mathcal{B} v (v+s-\zeta )+2 \mathcal{A} v (-j+v+s-\zeta )+\mathcal{B} j (2 v+s-\zeta ))\, (j-v-1)! \Gamma (v+s-\zeta )}{v!\, \Gamma (s-\zeta +1)\, \Gamma (j-v-s+\zeta +1)}, \end{split} \end{align}$

(3.15)

$\begin{align} &\sum _{r = 0}^v \frac{(-1)^{r+1}\, (j-r-1)!}{(v-r)!\, r!\, \Gamma (j-v-s-r+\zeta )} = -\frac{(j-v-1)!\, \Gamma (v+s-\zeta +1)}{v!\, \Gamma (s-\zeta +1)\, \Gamma (j-v-s+\zeta )}, \end{align}$

(3.16)

and therefore, Formula (3.12) can be obtained. □

Corollary 3.2. The UCPs-ultraspherical connection formula is

$\begin{equation} \begin{split} &G_{j}(x) = \frac{2^{-2 \zeta }\, \sqrt{\pi }}{\Gamma \left(\zeta +\frac{1}{2}\right)}\times\sum _{v = 0}^{\left\lfloor \frac{j}{2}\right\rfloor }\\ & \frac{(-j+2 v-\zeta )\, (2 (\mathcal{A}-\mathcal{B}) (j-v) v+\mathcal{B} (j-2 v) \zeta +2 \mathcal{A} v \zeta ) (j-v-1)!\, (1-\zeta )_{v-1}\, \Gamma (j-2 v+2 \zeta )}{(j-2 v)!\, v!\, \Gamma (1+j-v+\zeta )}\, U^{(\zeta)}_{j-2v}(x)\\ &+\frac{2^{1-2 \zeta }\, \sqrt{\pi }\, \mathcal{R}\, (j-1)!}{\Gamma \left(\zeta +\frac{1}{2}\right)} \sum _{v = 0}^{\left\lfloor \frac{j-1}{2}\right\rfloor } \frac{(-1+j-2 v+\zeta )\, (j-v-1)!\, \Gamma (-1+j-2 v+2 \zeta ) (1-\zeta )_v}{v!\, (j-2 v-1)!\, \Gamma (j-v+\zeta )}\, U^{(\zeta)}_{j-2v-1}(x), \\& j\ge 0. \end{split} \end{equation}$

(3.17)

Proof. Formula (3.17) is a specific formula of (3.12) for $s = 0$ . □

Remark 3.2. Since Legendre polynomials and CPs of the first and second kinds are particular ones of special cases of $U^{(\zeta)}_{j}(x)$ , we may infer certain particular formulae from (3.12). The following corollaries display the results.

Corollary 3.3. Let $j\ge s\ge 1$ . We have the following formula:

$\begin{equation} \begin{split} D^s G_{j}(x) = &\frac{2^s}{s!} \sum _{v = 0}^{\left\lfloor \frac{j-s}{2}\right\rfloor } \frac{\xi_{j-s-2 v} (j-v-1)! (v+s-1)! (2\mathcal{B} (j-v) v+\mathcal{B} (j-2 v) s+2 \mathcal{A} v (-j+v+s))}{v! (j-v-s)!}T_{j-s-2 v}(x)\\ &+\frac{2^{1+s} \mathcal{R}}{s!} \sum _{v = 0}^{\left\lfloor \frac{1}{2} (j-s-1)\right\rfloor } \frac{\xi_{j-s-2 v-1} (j-v-1)!\, (v+s)!}{v!\, (j-v-s-1)!}\, T_{j-s-2 v-1}(x). \end{split} \end{equation}$

(3.18)

In particular, we have:

$\begin{equation} G_{j}(x) = \mathcal{B}T_{j}(x)+2 (\mathcal{B}-\mathcal{A}) \sum\limits_{v = 1}^{\left\lfloor \frac{j}{2}\right\rfloor } \xi_{j-2 v}\, T_{j-2 v}(x)+2 \mathcal{R} \sum\limits_{v = 0}^{\left\lfloor \frac{j-1}{2}\right\rfloor } \xi_{j-2 v-1}\, T_{j-2 v-1}(x). \end{equation}$

(3.19)

Proof. Setting $\zeta = 0$ in (3.12) and (3.17) yields respectively (3.18) and (3.19). □

Corollary 3.4. Let $j\ge \mathit{s}\ge 1$ . We have the following formula:

$\begin{equation} \begin{split} D^s G_{j}(x) = &\frac{2^{s-1}}{(s-1)!} \sum _{v = 0}^{\left\lfloor \frac{j-s}{2}\right\rfloor } \frac{(j-v-1)!\, (v+s-2)! (1+j-2v-s)}{v!\, (j-v-s+1)!}\\ &\times(-2 \mathcal{B} v (-1+v+s)+2 \mathcal{A} v (-1-j+v+s)+\mathcal{B} j (-1+2 v+s))\, U_{j-s-2v}(x)\\ &+\frac{2^s\, \mathcal{R}}{(s-1)!} \sum _{v = 0}^{\left\lfloor \frac{1}{2} (j-s-1)\right\rfloor } \frac{(j-2v-s)\, (j-v-1)!\ (v+s-1)!}{v!\, (j-v-s)!}\, U_{j-s-2v-1}(x), \end{split} \end{equation}$

(3.20)

and in particular:

$\begin{equation} \begin{split} G_{j}(x) = &\frac{1}{2} (-2 \mathcal{A}+\mathcal{B}) U_{j-2}(x)+\mathcal{R} U_{j-1}(x)+\frac{\mathcal{B}}{2}\, U_{j}(x). \end{split} \end{equation}$

(3.21)

Proof. Setting $\zeta = 0$ in (3.12) and (3.17) yields respectively (3.20) and (3.21). □

Corollary 3.5. Let $j\ge s\ge 1$ . We have the following formula:

$\begin{equation} \begin{split} D^s G _{j}(x) = &\frac{2^{s-1}\, \sqrt{\pi }}{\Gamma \left(\frac{1}{2}+\mathit{s}\right)} \sum _{v = 0}^{\left\lfloor \frac{j-\mathit{s}}{2}\right\rfloor } \frac{(1+2 j-4v-2\mathit{s})\, (j-v-1)!\, \Gamma \left(-\frac{1}{2}+v+\mathit{s}\right)}{4v! \Gamma \left(\frac{3}{2}+j-v-\mathit{s}\right)}\\ &\times (-2 \mathcal{A} v+4 \mathcal{A} v (-j+v+\mathit{s})+\mathcal{B} (2v-4v(v+\mathit{s})+j (-1+4v+2\mathit{s})))\, P_{j-\mathit{s}-2v}(x)\\ &-2^{s-1} \sqrt{\pi } \mathcal{R} (j-1)! \sum _{v = 0}^{\left\lfloor \frac{1}{2} (j-\mathit{s}-1)\right\rfloor } \frac{(1-2 j+4v+2\mathit{s}) \left(\frac{1}{2}+\mathit{s}\right)_v}{v!\, \Gamma \left(\frac{1}{2}+j-v-\mathit{s}\right)\, (j-v)_v}\, P_{j-\mathit{s}-2v-1}(x), \end{split} \end{equation}$

(3.22)

and in particular:

$\begin{equation} \begin{split} G_{j}(x) = &\sum _{v = 0}^{\left\lfloor \frac{j}{2}\right\rfloor } \frac{(1+2 j-4v) (j-v-1)! \Gamma \left(-\frac{1}{2}+v\right) \left(-\mathcal{B} j+2 (-\mathcal{A}+\mathcal{B}) (1+2 j) v+4 (\mathcal{A}-\mathcal{B}) v^2\right)}{8 v! \Gamma \left(\frac{3}{2}+j-v\right)} P_{j-2v}(x)\\ &-\frac{1}{2} \sqrt{\pi } \mathcal{R} (j-1)!\, \sum _{v = 0}^{\left\lfloor \frac{j-1}{2}\right\rfloor } \frac{(1-2 j+4v) \left(\frac{1}{2}\right)_v}{v! \Gamma \left(\frac{1}{2}+j-v\right) (j-v)_v} P_{j-2v-1}(x). \end{split} \end{equation}$

(3.23)

Proof. Setting $\zeta = \frac12$ in (3.12) and (3.17) yields respectively (3.22) and (3.23). □

Remark 3.3. Similar steps to those followed to prove Theorem 3.2 can be used to obtain derivative expressions for UCPs in terms of other symmetric polynomials. The results of Table 1 are used to derive the desired formulas. The following four theorems exhibit some derivative expressions.

Theorem 3.3. Consider the NNIs $j$ and $\mathit{s}$ with $j\geq \mathit{s}$ . We have

$\begin{equation} \begin{split} D^s G_{j}(x) = &2^{s-1} (j-2)!\sum _{\mathit{v} = 0}^{\left\lfloor \frac{j-\mathit{s}}{2}\right\rfloor } \frac{1}{v! (j-2 v-s)!}\\ &\times\left(2 (-\mathcal{A}+\mathcal{B}) \mathit{v} \, _1F_1(1-v;2-j;-1)+\mathcal{B} (j-1) j \, _1F_1(-v;1-j;-1)\right)\, H_{j-s-2 \mathit{v}}(x)\\ &+2^s \mathcal{R} (j-1)! \sum _{\mathit{v} = 0}^{\left\lfloor \frac{1}{2} (j-s-1)\right\rfloor } \frac{1}{v! (j-2 v-s-1)!} \, _1F_1(-v;1-j;-1)\, H_{j-s-2 v-1}(x). \end{split} \end{equation}$

(3.24)

Theorem 3.4. Consider the NNIs $j$ and $s$ with $j\ge s$ . One has the following derivative expressions:

$\begin{equation} \begin{split} D^s G_{j}(x) = &2^{j-2} a^{-j+s} (j-2)! \sum _{v = 0}^{\left\lfloor \frac{j-s}{2}\right\rfloor } \frac{(-1)^v b^{v-1} (1+j-2 v-s)}{v! (j-v-s+1)!}\\ &\times\left(-a^2 (\mathcal{A}-\mathcal{B}) v (-1-j+v+s)\, _{2} F_{1}\left.\left( \begin{array}{cc} 1-v, -j+v+s\\[0.2cm] 2-j \end{array} \right|-\frac{a^2}{4 b}\right)\right.\\ &\left.+2\, b\, \mathcal{B} (j-1) j \, _{2} F_{1}\left.\left( \begin{array}{cc} -v, -j+v+s\\[0.2cm] 1-j \end{array} \right|-\frac{a^2}{4 b}\right)\right)\, F^{a, b}_{j-s-2 v}(x)\\ &+2^{-1+j} a^{1-j+s} (j-1)! \mathcal{R} \sum _{v = 0}^{\left\lfloor \frac{1}{2} (j-s-1)\right\rfloor } \frac{(-1)^{v+1} b^v (-j+2 v+s)}{v! (j-v-s)!}\\ &\times\, _{2} F_{1}\left.\left( \begin{array}{cc} -v, -j+v+s\\[0.2cm] 1-j \end{array} \right|-\frac{a^2}{4 b}\right)\, F^{a, b}_{j-s-2 v-1}(x). \end{split} \end{equation}$

(3.25)

Theorem 3.5. Consider the NNIs $j$ and $s$ with $j\ge s$ . We have

$\begin{equation} \begin{split} D^s G_{j}(x) = & 2^{j-2} c^{-j+s}\, (j-2)! \sum _{v = 0}^{\left\lfloor \frac{j-s}{2}\right\rfloor } \frac{\xi_{j-s-2 v}\, (-1)^v\, d^{v-1}}{v! (j-v-s)!}\\ &\times\left(-c^2 (\mathcal{A}-\mathcal{B}) v (-j+v+s)\, \ _{2} F_{1}\left.\left( \begin{array}{cc} 1-v, 1-j+v+s\\ 2-j \end{array} \right|-\frac{c^2}{4\, d}\right)\right.\\ &\left.+2\, d\, \mathcal{B} (j-1) j \, _{2} F_{1}\left.\left( \begin{array}{cc} -v, -j+v+s\\ 1-j \end{array} \right|-\frac{c^2}{4\, d}\right)\right)\, L^{c, d}_{j-s-2 v}(x)\\ &+2^{j-1}\, c^{1-j+s}\, \mathcal{R}\, (j-1)!\, \sum _{v = 0}^{\left\lfloor \frac{1}{2} (j-s-1)\right\rfloor } \frac{\xi_{j-s-2 v-1} (-1)^v b^v \, }{v! (j-v-s-1)!}\\ &\times\ _{2} F_{1}\left.\left( \begin{array}{cc} -v, -j+v+1+s\\ 1-j \end{array} \right|-\frac{c^2}{4 \, d}\right)\, L^{c, d}_{j-s-2 v-1}(x). \end{split} \end{equation}$

(3.26)

Remark 3.4. Other expressions of $D^sG_{j}(x)$ in terms of nonsymmetric polynomials are expressed as in (2.17). For example, in the following, we give with proof an expression for $D^sG_{j}(x)$ in terms of Bernoulli polynomials.

Theorem 3.6. Consider the NNIs $j$ and $s$ with $j\ge s$ . We have

$\begin{equation} D^s G_{j}(x) = \sum\limits_{v = 0}^{\left\lfloor \frac{j-s}{2}\right\rfloor } F_{v, j, s}\, B_{j-s-2 v}(x)+\sum\limits_{v = 0}^{\left\lfloor \frac{1}{2} (j-s-1)\right\rfloor }\, \bar{F}_{v, j, s}\, B_{j-s-2 v-1}(x), \end{equation}$

(3.27)

where

$\begin{align*} F_{v, j, s} = &\frac{(-1)^j\, 2^{-j+2 v}\, (2 j-2 v-2)!\, \Gamma \left(\frac{1}{2}-j+2 v\right)}{\sqrt{\pi } (2 v+1)! (j-2 v-s)!}\\ &\times\left(-2 \mathcal{A} v (1+2 v)+\mathcal{B} \left(j (-1+2 j)+2 v-4 j v+4 v^2\right)\right) \\ &+\frac{2^{-1-j-2 v} \mathcal{B} \left( \frac{(-1)^{v+1} 4^j (j-v-1)!}{v!}+\frac{(-1)^j 2^{1+4 v} (2 j-2 v-1)! \Gamma \left(\frac{1}{2}-j+2 v\right)}{\sqrt{\pi } (2 v)!}\right)}{(j-2 v-s)!}, \\ \bar{F}_{v, j, s} = & \frac{2^{-3+j} (j-v-2)! \left(-\frac{1}{2}+j-2 v\right)_v}{(j-2 v-s-1)!} \\ &\times\left(\frac{4 \left(-\mathcal{A} (v+1) (1+2 v)+\mathcal{B} \left(1-\frac{3 j}{2}+j^2+3 v-2 j v+2 v^2\right)+2 (-1+j-v) (v+1) \mathcal{R}\right)}{(2 v+2)!}\right.\\ &+\left.\frac{\left(-\frac{1}{4}\right)^v (2 \mathcal{A} (v+1)+\mathcal{B} (j-2 (v+1)))}{(v+1)!}\right). \end{align*}$

Proof. It is possible to express $D^s G_{j}(x)$ as a result of (2.10).

$\begin{equation} D^s G_{j}(x) = \sum\limits_{r = 0}^{\left\lfloor \frac{j}{2}\right\rfloor } M_{r, j, s}\, x^{j-2 r-s}+\sum\limits_{r = 0}^{\left\lfloor \frac{j}{2}\right\rfloor } S_{r, j, s}\, x^{j-2 r-s-1}, \end{equation}$

(3.28)

with

$\begin{align*} M_{r, j, s} = &\frac{(-1)^r\, 2^{-1+j-2 r}\, (\mathcal{B} (j-2 r)+2 \mathcal{A} r) (j-r-1)!}{r!\, (j-s-2 r)!}, \\ S_{r, j, s} = &\frac{(-1)^r\, 2^{-1+j-2 r}\, \mathcal{R}\, (j-2 r)_r\, (j-s-2 r)_q}{r!}. \end{align*}$

The formula for the inversion of Bernoulli polynomials (see, the last column of Table 1) enables one to get

$\begin{equation} \begin{split} D^s G_{j}(x) = &\sum _{r = 0}^{\left\lfloor \frac{j}{2}\right\rfloor } M_{r, j, s} \sum _{m = 0}^{j-2 r-s} R_{m, j-2 r-s}\, B_{j-2r-s-m}(x)\\ &+\sum _{r = 0}^{\left\lfloor \frac{j-1}{2}\right\rfloor } S_{r, j, s} \sum _{m = 0}^{j-2 r-s-1} R_{m, j-2 r-s-1}\, B_{j-2 r-s-m-1}(x), \end{split} \end{equation}$

(3.29)

where

$R_{r, j} = \frac{\binom{j+1}{j-r}}{j+1}.$

This formula results from extensive manipulations

$\begin{equation} \begin{split} D^s G_{j}(x) = &\sum _{v = 0}^{\left\lfloor \frac{j-s}{2}\right\rfloor } \left(\sum _{\ell = 0}^v M_{\ell, j, s}\, R_{2 v-2 \ell, j-s-2\ell}+\sum _{\ell = 0}^{v-1} S_{\ell, j, s}\, R_{2 v-2 \ell-1, j-s-2 \ell-1}\right)\, B_{j-s-2 v}(x)\\ &+\sum _{v = 0}^{\left\lfloor \frac{1}{2} (j-s-1)\right\rfloor }\left( \sum _{\ell = 0}^v M_{\ell, j, s}\, R_{2 v-2 \ell+1, j-s-2 \ell}+S_{\ell, j, s}\, R_{2v-2 \ell, j-s-2 \ell-1}\right)\, B_{j-s-2 v-1}(x). \end{split} \end{equation}$

(3.30)

The last formula is equivalent to

$\begin{equation} \begin{split} D^s G_{j}(x) = &\sum _{v = 0}^{\left\lfloor \frac{j-s}{2}\right\rfloor } \frac{1}{(j-2 v-s)!} \sum _{\ell = 0}^v \Bigg(\frac{(-1)^{\ell+1}\, 2^{-1+j-2 \ell}\, (-\mathcal{B} j-2 \mathcal{A} \ell+2 \mathcal{B} \ell)\, (j-\ell-1)!}{\ell!\, (2 v-2 \ell+1)!}\\ &+\frac{\mathcal{R}}{(j-2 v-s)!} \sum _{\ell = 0}^{v-1} \frac{(-1)^\ell\, 2^{-1+j-2 \ell}\, (j-\ell-1)!}{\ell! (2 v-2 \ell)!}\Bigg)\, B_{j-s-2 v}(x)\\ &+\sum _{v = 0}^{\left\lfloor \frac{1}{2} (j-s-1)\right\rfloor } \frac{1}{(j-2 v-s-1)!} \sum _{\ell = 0}^v \frac{(-1)^{\ell+1}\, 2^{-1+j-2 \ell}\, (j-\ell-1)!}{\ell!\, (2 v-2 \ell+2)!} \\ &\times\Big(-\mathcal{B} j-2 \mathcal{A} \ell+2 \mathcal{B} \ell+2 (\ell-v-1) \mathcal{R}\Big)\, B_{j-s-2v-1}(x). \end{split} \end{equation}$

(3.31)

Symbolic computation, and in particular Zeilberger's algorithm ^[54], helps to find the three simple forms for the internal sums that appear in (3.31).

$\begin{align} \begin{split} &\sum _{\ell = 0}^v \frac{(-1)^{\ell+1}\, 2^{-1+j-2 \ell} \Big(-\mathcal{B} j-2 \mathcal{A} \ell+2 \mathcal{B} \ell\Big) (j-\ell-1)!}{\ell!\, (2 v-2 \ell+1)!}\\ & = \frac{(-1)^j\, 2^{-j+2 v}\, (2 j-2 v-2)! \Gamma \left(\frac{1}{2}-j+2 v\right)\, \Big(-2 \mathcal{A} v (1+2 v)+\mathcal{B} \left(j (-1+2 j)+2 v-4 j v+4 v^2\right)\Big)}{\sqrt{\pi } (2 v+1)!}, \end{split} \end{align}$

(3.32)

$\begin{align} \begin{split} &\sum _{\ell = 0}^{v-1} \frac{(-1)^\ell 2^{-1+j-2 \ell}\, (j-\ell-1)!}{\ell! (2 v-2 \ell)!} = 2^{-1-j-2 v}\, \Bigg(\frac{(-1)^{v+1}\, 4^j (j-v-1)!}{v!}\Bigg.\\ &\Bigg.+\frac{(-1)^j 2^{1+4 v} (2 j-2 v-1)! \Gamma \left(\frac{1}{2}-j+2 v\right)}{\sqrt{\pi } (2 v)!}\Bigg), \end{split} \end{align}$

(3.33)

$\begin{align} \begin{split} &\sum _{\ell = 0}^v \frac{(-1)^{\ell+1} 2^{-1+j-2 \ell} \Big(-\mathcal{B} j-2 \mathcal{A} \ell+2 \mathcal{B} \ell+2 (\ell-1-v) \mathcal{R}\Big) (j-\ell-1)!}{\ell! (2 v-2 \ell+2)!}\\ & = 2^{-3+j} (j-v-2)! \Bigg(\frac{\left(-\frac{1}{4}\right)^v (2 \mathcal{A} (v+1)+\mathcal{B} (j-2 (v+1)))}{(v+1)!}\Bigg.\\ &\Bigg.+\frac{4 \left(-\mathcal{A} (v+1) (1+2 v)+\mathcal{B} \left(1-\frac{3 j}{2}+j^2+3 v-2 j v+2 v^2\right)+2 (-1+j-v) (v+1) \mathcal{R}\right) \left(-\frac{1}{2}+j-2 v\right)_v}{(2 v+2)!}\Bigg). \end{split} \end{align}$

(3.34)

Substituting the last three summations from Eqs (3.32)–(3.34) into Formula (3.31), we can derive Formula (3.27). Consequently, Theorem 3.6 is proved. □

4. Derivatives' expressions of various celebrated polynomials in terms of UCPs

This section deals with polynomial expressions based on UCPs. Connection formulas between different polynomials with the UCPs can be deduced as special cases.

Theorem 4.1. Consider the NNIs $j, s$ with $j\geq s$ . The following expression holds:

$\begin{equation} D^s U^{(\zeta)}_{j}(x) = \frac{2^{-1+s+2 \zeta }\, j!\, \Gamma \left(\frac{1}{2}+\zeta \right)}{A\, \sqrt{\pi }\, \Gamma (s+\zeta )\, \Gamma (j+2 \zeta )} \sum _{v = 0}^{j-s} \frac{\Gamma (j-v+\zeta )\, \Gamma (v+s+\zeta )}{v!\, (j-v-s)!} \, G_{j-s-2 v}(x). \end{equation}$

(4.1)

Proof. The formula (4.1) can be obtained using the analytic form of $U^{(\zeta)}_{j}(x)$ (Table 1, the second column) along with the inversion formula (2.12). □

Theorem 4.2. Consider the NNIs $j, s$ with $j\ge s$ . The following expression holds:

$\begin{equation} D^s H_{j}(x) = \frac{2^s j!}{\mathcal{A}} \sum\limits_{v = 0}^{j-s} \frac{(-1)^v}{v!} \, _1\tilde{F}_1(-v;1+j-2 v-s;1)\, G_{j-s-2 v}(x), \end{equation}$

(4.2)

where the notation $\ _{p} F_{q}(z)$ is the regularized hypergeometric function, see ^[44].

Proof. The analytic form of $H_{j}(x)$ can be used in conjunction with the inversion formula (2.12) to produce (4.2). □

Theorem 4.3. Consider the NNIs $j, s$ with $j\ge s$ . The following expression holds:

$\begin{equation} D^s F^{(a, b)}_{j}(x) = \frac{2^{-j+s} a^j j!}{\mathcal{A}} \sum\limits_{v = 0}^{j-s} \frac{1}{v! (j-v-s)!}\, \ _{2} F_{1}\left.\left( \begin{array}{cc} -v, -j+v+s\\[0.2cm] -j \end{array} \right|-\frac{4b}{a^2}\right)\, G_{j-s-2 v}(x). \end{equation}$

(4.3)

Proof. The series expression of $F^{a, b}_{j}(x)$ can be used in conjunction with the inversion formula (2.12) to produce (4.3). □

Theorem 4.4. Consider the NNIs $j, s$ with $j\ge s$ . The following expression holds:

$\begin{equation} D^s L^{(c, d)}_{j}(x) = \frac{2^{-j+s}\, c^j\, j!}{\mathcal{A}} \sum\limits_{v = 0}^{j-s} \frac{1}{v!\, (j-v-s)!}\, \ _{2} F_{1}\left.\left( \begin{array}{cc} -v, -j+v+s\\[0.2cm] 1-j \end{array} \right|-\frac{4d}{c^2}\right)\, G_{j-s-2 v}(x). \end{equation}$

(4.4)

Proof. The series expression of $L^{c, d}_{j}(x)$ can be used in conjunction with the inversion formula (2.12) to produce (4.4). □

Theorem 4.5. Consider the NNIs $j, s$ with $j\ge s$ . The following expression holds:

$\begin{equation} \begin{split} D^s P^{(\rho, \mu)}_{j}(x) = & \frac{2^{-2 j+s}\, \Gamma (1+2 j+\rho +\mu )}{\mathcal{A}\, (j-s)!\, \Gamma (1+j+\rho +\mu )} \sum _{v = 0}^{2 (j-s)} \binom{2 (j-s)}{v}\\ &\times\ _{3} F_{2}\left.\left( \begin{array}{cc} -v, -2 j+v+2 s, -j-\mu\\[0.2cm] \frac{1}{2}-j+s, -2 j-\rho -\mu \end{array} \right|1\right)\, G_{j-s-2 v}(x). \end{split} \end{equation}$

(4.5)

Proof. The analytic form of $\tilde{P}^{(\rho, \mu)}_{j}(x)$ yields the following formula

$\begin{equation} D^s \tilde{P}^{(\rho, \mu)}_{j}(x) = \frac{\Gamma (1+j+\mu )}{\Gamma (1+j+\rho +\mu )} \sum _{r = 0}^{j-s} \frac{(-1)^r\, \Gamma (1+2 j-r+\rho +\mu )}{r!\, (j-s-r)!\, \Gamma (1+j-r+\mu )} x^{j-r-s}. \end{equation}$

(4.6)

We utilize the inversion formula of $\tilde{G}_{i}(x)$ , consequently, the next equation can be deduced:

$\begin{equation} \begin{split} D^s \tilde{P}^{(\rho, \mu)}_{j}(x) = &\frac{\Gamma (1+j+\mu )}{\mathcal{A}\, \Gamma (1+j+\rho +\mu )} \sum _{r = 0}^{j-s} \frac{(-1)^r\, 4^{-j+s+r}\, \Gamma (1+2 j-r+\rho +\mu )}{r! (j-s-r)! \Gamma (1+j-r+\mu )} \\ &\times\sum _{t = 0}^{2 (j-r-s)} \binom{2 (j-s-r)}{t} \tilde{G}_{j-r-s-t}(x). \end{split} \end{equation}$

(4.7)

Alternatively, it may be expressed as:

$\begin{equation} \begin{split} D^s \tilde{P}^{(\rho, \mu)}_{j}(x) = &\frac{\Gamma (1+j+\mu )}{\mathcal{A}\, \Gamma (1+j+\rho +\mu )} \sum _{v = 0}^{2 (j-s)}\, \sum _{\ell = 0}^v \frac{(-1)^\ell\, 4^{-j+\ell+s}\, \binom{2 (j-\ell-s)}{v-\ell}\, \Gamma (1+2 j-\ell+\rho +\mu )}{\ell!\, (j-\ell-s)!\, \Gamma (1+j-\ell+\mu )}\, \tilde{G}_{j-s-v}(x). \end{split} \end{equation}$

(4.8)

Accordingly, the last formula can be written in a hypergeometric form as follows:

$\begin{equation} \begin{split} D^s \tilde{P}^{(\rho, \mu)}_{j}(x) = & \frac{2^{-2 j+2s}\, \Gamma (1+2 j+\rho +\mu )}{\mathcal{A}\, (j-s)!\, \Gamma (1+j+\rho +\mu )} \sum _{v = 0}^{2 (j-s)} \binom{2 (j-s)}{v}\\ &\times\ _{3} F_{2}\left.\left( \begin{array}{cc} -v, -2 j+v+2 s, -j-\mu\\[0.2cm] \frac{1}{2}-j+s, -2 j-\rho -\mu \end{array} \right|1\right)\, \tilde{G}_{j-s-v}(x). \end{split} \end{equation}$

(4.9)

Replacing $x$ by $\frac{1+x}{2}$ in (4.8), Formula (4.5) can be obtained. □

Corollary 4.1. Let $j\ge s$ . For $\mu = \rho+1$ , Formula (4.5) reduces to the following formula

$\begin{equation} \begin{split} D^s P^{(\rho, \rho+1)}_{j}(x) = &\frac{2^{1+s+2 \rho }\, \Gamma (1+j+\rho )}{\mathcal{A}\, \sqrt{\pi }\, \Gamma \left(\frac{3}{2}+s+\rho \right) \Gamma (2+j+2 \rho )}\left(\sum _{v = 0}^{j-s} \frac{\Gamma \left(\frac{3}{2}+j-v+\rho \right) \ \Gamma \left(\frac{3}{2}+v+s+\rho \right)}{v! (j-v-s)!}\, G_{j-s-2v}(x)\right.\\ &\left.-\sum _{v = 0}^{j-s-1} \frac{\Gamma \left(\frac{1}{2}+j-v+\rho \right) \Gamma \left(\frac{3}{2}+v+s+\rho \right)}{v! (j-v-s-1)!}\right)\, G_{j-s-2 v-1}(x). \end{split} \end{equation}$

(4.10)

Proof. Setting $\mu = \rho+1$ in (4.5) yields

$\begin{equation} \begin{split} D^s P^{(\rho, \rho+1)}_{j}(x) = &\frac{2^{-2 j+s}\, \Gamma (2 (1+j+\rho ))}{\mathcal{A}\, (j-s)!\, \Gamma (2+j+2 \rho )} \sum _{v = 0}^{2 (j-s)} \binom{2 (j-s)}{v} \\ &\times\ _{3} F_{2}\left.\left( \begin{array}{cc} -v, -2 j+v+2 s, -1-j-\rho\\[0.2cm] \frac{1}{2}-j+s, -1-2 j-2 \rho \end{array} \right|1\right)\, G_{j-s-v}(x). \end{split} \end{equation}$

(4.11)

Zeibreger's algorithm (^[54]) can aid us to sum the $\ _{3} F_{2}(1)$ that appears in the last formula. Setting

$Z_{v, j, s} = \ _{3} F_{2}\left.\left( \begin{array}{cc} -v, -2 j+v+2 s, -1-j-\rho\\[0.2cm] \frac{1}{2}-j+s, -1-2 j-2 \rho \end{array} \right|1\right),$

then $M_{v, j, s}$ meets the next recursive formula

$- \left( v-1 \right) \left( v+2\, \rho+2\, s \right) F_{v-2, j, s}+2\, \left( j-v-s+1 \right) F_{v-1, j, s} + \left( 2\, j-v+1-2\, s \right) \left( 2\, j+2\, \rho-v+2 \right)F_{v, j, s} = 0,$

accompanied by the initial conditions

$F_{0, j, s} = 1, \quad F_{1, j, s} = \frac{-1}{2j+2\rho+1},$

that can be solved to give

$M_{v, j, s} = \frac{1}{\sqrt \pi} \begin{cases} \frac{\Gamma \left(\frac{v+1}{2}\right) \left(\frac{3}{2}+s+\rho \right)_{\frac{v}{2}}}{ \left(\frac{1}{2}+j-\frac{v}{2}-s\right)_{\frac{v}{2}} \left(\frac{3}{2}+j-\frac{v}{2}+\rho \right)_{\frac{v}{2}}}, & v\ \text{even}, \\[0.3cm] \frac{-\Gamma \left(1+\frac{v}{2}\right) \left(\frac{3}{2}+s+\rho \right)_{\frac{1}{2} (v-1)}}{\left(1+j-\frac{v}{2}-s\right)_{\frac{1}{2} (v-1)} \left(1+j-\frac{v}{2}+\rho \right)_{\frac{v+1}{2}}}, & v\ \text{odd}, \end{cases}$

and therefore, Formula (4.10) can be obtained. □

5. Linearization formulas of the UCPs

In this section, we will develop a new linearization formula (LF) for the UCPs based on these polynomials' analytic form and moments formula. Furthermore, some other linearization formulas for UCPs with some other polynomials will also be derived. More precisely, the following linearization problems will be solved:

$\begin{align} G_{i}(x)\, G_{j}(x) & = \sum\limits_{k = 0}^{i+j} L_{k, i, j} G_{i+j-k}(x), \end{align}$

(5.1)

$\begin{align} G_{i}(x)\, \phi_{j}(x) & = \sum\limits_{k = 0}^{i+j} \tilde{L}_{k, i, j}\, G_{i+j-k}(x), \end{align}$

(5.2)

$\begin{align} G_{i}(x)\, \phi_{j}(x) & = \sum\limits_{k = 0}^{i+j} \bar{L}_{k, i, j}\, \phi_{i+j-k}(x), \end{align}$

(5.3)

for certain polynomials $\phi_{j}(x)$ .

5.1. Linearization formula of the UCPs

The following theorem exhibits the LF of $G_{i}(x)$ . This formula generalizes some well-known formulas.

Theorem 5.1. Consider the two NNIs $i$ and $j$ . The following LF applies:

$\begin{equation} \begin{split} G_{i}(x)\, G_{j}(x) = \frac{\mathcal{B}}{2}\, \left(G_{j-i}(x)+G_{j+i}(x)\right)+(\mathcal{B}-\mathcal{A}) \sum _{k = 0}^{i-2}G_{j-i+2 k+2}(x)+\mathcal{R}\, \sum _{k = 0}^{i-1}\, G_{j-i+2 k+1}(x). \end{split} \end{equation}$

(5.4)

Proof. The analytic form in (2.10) leads to obtaining the following formula

$\begin{equation} \begin{split} G_{i}(x)\, G_{j}(x)& = \sum _{r = 0}^{\left\lfloor \frac{i}{2}\right\rfloor } \frac{(-1)^r\, 2^{-1+i-2 r} (\mathcal{B} (i-2 r)+2 \mathcal{A} r)\, (1+i-2 r)_{r-1}}{r!}\, x^{i-2 r}\, G_{j}(x)\\ &+\mathcal{R} \sum _{r = 0}^{\left\lfloor \frac{i-1}{2}\right\rfloor } \frac{(-1)^r\, 2^{-1+i-2 r}\, (i-2 r)_r}{r!}\, \, x^{i-2 r-1}\, G_{j}(x). \end{split} \end{equation}$

(5.5)

As a result of the moment formula (2.11), we obtain the following formula

$\begin{equation} \begin{split} G_{i}(x)\, G_{j}(x) = &\sum _{r = 0}^{\left\lfloor \frac{i}{2}\right\rfloor } \frac{(-1)^r\, 2^{-1+i-2 r}\, (\mathcal{B} (i-2 r)+2 \mathcal{A} r) (1+i-2 r)_{r-1}}{r!} \\ &\times\sum _{\ell = 0}^{i-2 r} 2^{-i+2 r}\, \binom{i-2 r}{\ell}\, G_{i+j-2 r-2 \ell}(x)\\ &+\mathcal{R} \sum _{r = 0}^{\left\lfloor \frac{i-1}{2}\right\rfloor } \frac{(-1)^r\, 2^{-1+i-2 r}\, (i-2 r)_r}{r!} \sum _{\ell = 0}^{i-2 r-1}\, 2^{1-i+2 r}\, \binom{-1+i-2 r}{\ell}\, G_{i+j-2 r-2 \ell-1}(x). \end{split} \end{equation}$

(5.6)

The following formula can be derived from a series of computations:

$\begin{equation} \begin{split} G_{i}(x)\, G_{j}(x) = &\frac{1}{2} \mathcal{B}\, \left(\phi_{j+i}(x)+\phi_{j-i}(x)\right)\\ &+\sum _{v = 1}^{i-1} \sum _{\ell = 0}^v\, \frac{(-1)^\ell\, (\mathcal{B} (i-2 \ell)+2 \mathcal{A} \ell)\, \binom{i-2 \ell}{v-\ell}\, (1+i-2 \ell)_{\ell-1}}{2 \ell!}\, G_{i+j-2 v}(x)\\ &+\mathcal{R}\, \sum _{v = 0}^{i-1} \sum _{\ell = 0}^v \frac{(-1)^\ell \binom{-1+i-2 \ell}{v-\ell} (i-2 \ell)_\ell}{\ell!}\, G_{i+j-2 v-1}(x). \end{split} \end{equation}$

(5.7)

Considering these two identities:

$\begin{align} &\sum _{\ell = 0}^v \frac{(-1)^\ell\, (\mathcal{B} (i-2 \ell)+2 \mathcal{A} \ell) \binom{i-2 \ell}{v-\ell} (1+i-2 \ell)_{\ell-1}}{2 \ell!} = \mathcal{B}-\mathcal{A}, \end{align}$

(5.8)

$\begin{align} &\sum _{\ell = 0}^v \frac{(-1)^\ell\, \binom{-1+i-2 \ell}{v-\ell} (i-2 \ell)_\ell}{\ell!} = 1, \end{align}$

(5.9)

the next simplified LF be acquired:

$\begin{split} G_{i}(x)\, G_{j}(x) = \frac{\mathcal{B}}{2}\, \left(G_{j-i}(x)+G_{j+i}(x)\right)+(\mathcal{B}-\mathcal{A}) \sum _{k = 0}^{i-2}G_{2-i+j+2 k}(x)+\mathcal{R}\, \sum _{k = 0}^{i-1}\, G_{j-i+2 k+1}(x). \end{split}$

This finalizes the proof of Theorem 5.1. □

Remark 5.1. Some well-known LFs can be extracted from the LF (5.4). The following corollary exhibits these formulas.

Corollary 5.1. Consider two NNIs. The following LFs hold:

$\begin{align} T_{i}(x)\, T_{j}(x) = &\frac12\, \left(T_{i-j}(x)+T_{i+j}(x)\right), \end{align}$

(5.10)

$\begin{align} U_{i}(x)\, U_{j}(x) = &\sum\limits_{k = 0}^{i}U_{j-i+2k}(x), \end{align}$

(5.11)

$\begin{align} V_{i}(x)\, V_{j}(x) = &\sum _{k = 0}^i V_{j-i+2 k}(x)-\sum _{k = 0}^{i-1} V_{j-i+2k+1}(x), \end{align}$

(5.12)

$\begin{align} W_{i}(x)\, W_{j}(x) = &\sum _{k = 0}^i W_{j-i+2k}(x)+\sum _{k = 0}^{i-1} W_{j-i+2k+1}(x). \end{align}$

(5.13)

Proof. Formulas (5.10)–(5.13) can be easily obtained as special cases of (5.4) noting the specific classes in (2.2) and (2.3). □

5.2. LFs of the UCPs with some symmetric polynomials

This part is interested in developing other product formulas of the UCPs with some other polynomials.

Theorem 5.2. Consider the two NNIs $i$ and $j$ . The following LF holds

$\begin{equation} G_{i}(x)\, F^{a, b}_{j}(x) = \left(\frac{a}{2}\right)^{j} \sum\limits_{v = 0}^{j} \binom{j}{v}\, \ _{2} F_{1}\left.\left( \begin{array}{cc} -v, -j+v\\[0.2cm] -j \end{array} \right|-\frac{a^2}{4 b}\right)\, G_{j+i-2 v}(x). \end{equation}$

(5.14)

Proof. Starting with the analytic form of $F^{a, b}_{i}(x)$ , we can write

$\begin{equation} G_{i}(x)\, F^{a, b}_{j}(x) = \sum\limits_{r = 0}^{\left\lfloor \frac{j}{2}\right\rfloor } \frac{a^{j-2 r} b^r (1+j-2 r)_r}{r!} x^{j-2 r}\, G_{i}(x). \end{equation}$

(5.15)

Making use of the moment formula (2.11), we get

$\begin{equation} G_{i}(x)\, F^{a, b}_{j}(x) = \sum\limits_{r = 0}^{\left\lfloor \frac{j}{2}\right\rfloor } \frac{\left(\frac{a}{2}\right)^{j-2 r} b^r (1+j-2 r)_r}{r!} \sum\limits_{\ell = 0}^{j-2 r} \binom{j-2 r}{\ell} G_{i+j-2 r-2 \ell}(x), \end{equation}$

(5.16)

which can be written as

$\begin{equation} G_{i}(x)\, F^{a, b}_{j}(x) = \sum\limits_{v = 0}^{j} \sum\limits_{\ell = 0}^v \frac{\left(\frac{a}{2}\right)^{j-2 \ell} b^\ell \binom{j-2 \ell}{v-\ell} (1+j-2 \ell)_\ell}{\ell!}\, G_{j+i-2 v}(x), \end{equation}$

(5.17)

which is also equivalent to

$\begin{equation} G_{i}(x)\, F^{a, b}_{j}(x) = \left(\frac{a}{2}\right)^{j} \sum\limits_{v = 0}^{j} \binom{j}{v} \, \ _{2} F_{1}\left.\left( \begin{array}{cc} -v, -j+v\\[0.2cm] -j \end{array} \right|-\frac{a^2}{4 b}\right)\, G_{j+i-2 v}(x). \end{equation}$

(5.18)

This finalizes the proof of Theorem 5.2. □

Theorem 5.3. Consider the two NNIs $i$ and $j$ . The following LF holds:

$\begin{equation} \begin{split} G_{i}(x)\, F^{a, b}_{j}(x) = &2^{i-1} a^{-i} \mathcal{B}\, \, F^{a, b}_{i+j}(x)+2^{i-1} a^{-i} (-b)^{i} \mathcal{B}\, F^{a, b}_{j-i}(x)\\ &+\frac{(i-2)! 2^{-2+i} a^{-i}}{b} \sum _{v = 1}^{i-1} \frac{(-b)^{v}}{(i-v)! v!} \left(a^2 (\mathcal{A}-\mathcal{B}) (i-v) v \, \ _{2} F_{1}\left.\left( \begin{array}{cc} 1-v, 1-i+v\\[0.2cm] 2-i \end{array} \right|-\frac{a^2}{4 b}\right) \right.\\ &\left.+2 b \mathcal{B} (i-1) i \, _{2} F_{1}\left.\left( \begin{array}{cc} -v, -i+v\\[0.2cm] 1-i \end{array} \right|-\frac{a^2}{4 b}\right) \right)\, F^{a, b}_{j+i-2 v}(x)\\ &+2^{i-1} a^{1-i} \mathcal{R} (i-1)! \sum _{v = 0}^{i-1} \frac{(-b)^{v}}{v! (i-v-1)!}\, \, \ _{2} F_{1}\left.\left( \begin{array}{cc} -v, 1-i+v\\[0.2cm] 1-i \end{array} \right|-\frac{a^2}{4 b}\right)F^{a, b}_{j+i-2v-1}(x). \end{split} \end{equation}$

(5.19)

Proof. Similar to the proof of Theorem 5.2. □

Theorem 5.4. Consider the two NNIs $i$ and $j$ . The following LF holds

$\begin{equation} G_{i}(x)\, U^{(\zeta)}_{j}(x) = \frac{2^{-1+2 \zeta }\, \Gamma \left(\frac{1}{2}+\zeta \right)}{\sqrt{\pi }\, \Gamma (\zeta )\, \Gamma (j+2 \zeta )}\, \sum\limits_{v = 0}^{j} \binom{j}{v}\, \Gamma (j-v+\zeta )\, \Gamma (v+\zeta )\, G_{j+i-2 v}(x). \end{equation}$

(5.20)

Proof. Just like the proof of Theorem 5.2. □

Theorem 5.5. Consider the two NNIs $i$ and $j$ . The following LF holds

$\begin{equation} G_{i}(x)\, H_{j}(x) = \sum\limits_{v = 0}^{j} \binom{j}{v} U(-v, 1+j-2v, 1) G_{j+i-2v}(x), \end{equation}$

(5.21)

where $U(c, d, y)$ denotes the confluent hypergeometric function (see, ^[51]).

Proof. Similar to the proof of Theorem 5.2. □

5.3. LFs of the UCPs with some nonsymmetric polynomials

Here, we give some LFs of the UCPs with some nonsymmetric polynomials. The product formulas with the shifted Jacobi and generalized Lageurre polynomials will be developed.

Theorem 5.6. Consider the two NNIs $i$ and $j$ . The following LF holds

$\begin{equation} \begin{split} \tilde{P}^{(\rho, \mu)}_{i}(x)\, G_{j}(x) = &\frac{1}{2^i}\, \Gamma (1+2 i+\rho +\mu )\, \sum\limits_{v = 0}^i \frac{1}{v!\, (i-v)! \Gamma (1+i+\rho +\mu )} \\ &\times\ _4F_3\left.\left( \begin{array}{cccc} -v, -i+v, -\frac{i}{2}-\frac{\mu }{2}, \frac{1}{2}-\frac{i}{2}-\frac{\mu }{2}\\ \frac{1}{2}, -i-\frac{\rho }{2}-\frac{\mu }{2}, \frac{1}{2}-i-\frac{\rho }{2}-\frac{\mu }{2} \end{array} \right|1\right) \, G_{j+i-2 v}(x)\\ &-\frac{1}{2^{i-1}}\, \frac{(i+\mu )\, \Gamma (2 i+\rho +\mu )}{\Gamma (1+i+\rho +\mu )} \sum\limits_{v = 0}^{i-1} \frac{1}{v! (i-v-1)!} \\ &\times\ _4F_3\left.\left( \begin{array}{cccc} -v, 1-i+v, \frac{1}{2}-\frac{i}{2}-\frac{\mu }{2}, 1-\frac{i}{2}-\frac{\mu }{2}\\ \frac{3}{2}, \frac{1}{2}-i-\frac{\rho }{2}-\frac{\mu }{2}, 1-i-\frac{\rho }{2}-\frac{\mu }{2} \end{array} \right|1\right)\, G_{j+i-2 v-1}(x). \end{split} \end{equation}$

(5.22)

Proof. The analytic form of the shifted JPs (presented in Table 1) allows one to write the following formula:

$\begin{equation} \begin{split} \tilde{P}^{(\rho, \mu)}_{i}(x)\, G_{j}(x) = \frac{(1+\mu )_i}{(1+\rho +\mu )_i} \sum\limits_{r = 0}^i \frac{(-1)^r (1+\rho +\mu )_{2 i-r}}{(i-r)! r! (1+\mu )_{i-r}}\, x^{i-r}\, G_{j}(x), \end{split} \end{equation}$

(5.23)

which turns into

$\begin{equation} \begin{split} \tilde{P}^{(\rho, \mu)}_{i}(x)\, G_{j}(x) = \frac{(1+\mu )_i}{(1+\rho +\mu )_i} \sum\limits_{r = 0}^i \frac{(-1)^r\, 2^{-i+r}\, (1+\rho +\mu )_{2 i-r}}{(i-r)! r! (1+\mu )_{i-r}} \sum\limits_{s = 0}^{i-r}\binom{i-r}{s}\, G_{j+i-r-2 s}(x). \end{split} \end{equation}$

(5.24)

After a series of algebraic computations, (5.24) converts into the following one

$\begin{equation} \begin{split} \tilde{P}^{(\rho, \mu)}_{i}(x)\, G_{j}(x) = & \frac{(1+\mu )_i}{(1+\rho +\mu )_i} \sum\limits_{r = 0}^v \frac{2^{-i+2 r} \binom{i-2 r}{v-r} (1+\rho +\mu )_{2 i-2r}}{(i-2 r)! (2 r)! (1+\mu )_{i-2 r}}\, G_{j+i-2 v}(x)\\ &+\frac{\Gamma (1+i+\mu )}{\Gamma (1+i+\rho +\mu )} \sum\limits_{v = 0}^{i-1} \sum\limits_{r = 0}^v \frac{(-1)^{1+2 r} 2^{1-i+2 r} \Gamma (2 i-2 r+\rho +\mu )}{(i-v-r-1)! (v-r)! (2 r+1)!\Gamma (i-2 r+\mu )}\, G_{j+i-2 v-1}(x). \end{split} \end{equation}$

(5.25)

In virtue of the two identities:

$\begin{align} \begin{split} \sum\limits_{r = 0}^p \frac{2^{-i+2 r} \binom{i-2 r}{p-r} (1+\rho +\mu )_{2 i-2 r}}{(i-2 r)! (2 r)! (1+\mu )_{i-2 r}} = &\frac{2^{-i} \Gamma (1+\mu ) \Gamma (1+2 i+\rho +\mu )}{(i-p)! p! \Gamma (1+i+\mu ) \Gamma (1+\rho +\mu )}\\ &\times\ _4F_3\left.\left( \begin{array}{cccc} -p, -i+p, -\frac{i}{2}-\frac{\mu }{2}, \frac{1}{2}-\frac{i}{2}-\frac{\mu }{2}\\ \frac{1}{2}, -i-\frac{\rho }{2}-\frac{\mu }{2}, \frac{1}{2}-i-\frac{\rho }{2}-\frac{\mu }{2} \end{array} \right|1\right), \end{split} \end{align}$

(5.26)

$\begin{align} \begin{split} \sum\limits_{r = 0}^p &\frac{(-1)^{1+2 r} 2^{1-i+2 r} \Gamma (2 i-2 r+\rho +\mu )}{(i-p-r-1)! (p-r)! (2 r+1)! \Gamma (i-2 r+\mu )} = \frac{-2^{1-i} \Gamma (2 i+\rho +\mu )}{(i-p-1)! p! \Gamma (i+\mu )} \\ &\times \ _4F_3\left.\left( \begin{array}{cccc} -p, 1-i+p, \frac{1}{2}-\frac{i}{2}-\frac{\mu }{2}, 1-\frac{i}{2}-\frac{\mu }{2}\\ \frac{3}{2}, \frac{1}{2}-i-\frac{\rho }{2}-\frac{\mu }{2}, 1-i-\frac{\rho }{2}-\frac{\mu }{2} \end{array} \right|1\right), \end{split} \end{align}$

(5.27)

Formula (5.25) can be written as in (5.22). □

Remark 5.2. The two terminating $\ _4F_3(1)$ can be reduced for some negative choices of $\rho$ and $\mu$ . The following corollaries exhibit some of these results: It is worth mentioning here that some authors investigated the class of JPs whose parameters are certain negative integers (see, ^[55,56]).

Corollary 5.2. Consider the two NNIs $i$ and $j$ . We have

$\begin{equation} \begin{split} \tilde{P}^{(-i, \mu)}_{i}(x)\, G_{j}(x) = &\frac{2^{i} \Gamma \left(\frac{1}{2}+i\right) \Gamma (1+i+\mu )}{\sqrt{\pi } \Gamma (1+\mu )} \left(\sum\limits_{v = 0}^{i} \frac{1}{(2 i-2 v)! (2 v)!} G_{j+i-2 v}(x)\right.\\ &\left.-\sum\limits_{v = 0}^{i-1} \frac{1}{(2 i-2 v-1)! (2 v+1)!} G_{j+i-2 v-1}(x)\right). \end{split} \end{equation}$

(5.28)

Proof. If we substitute $\rho = -i$ into Formula (5.22), it yields

$\begin{equation} \begin{split} \tilde{P}^{(-i, \mu)}_{i}(x)\, G_{j}(x) = &\frac{2^{-i}\, \Gamma (i+\mu +1)}{\Gamma (1+\mu )}\left( \sum\limits_{v = 0}^{i} \frac{1}{v!\, (i-v)!} \ _{2} F_{1}\left.\left( \begin{array}{cc} -v, -i+v\\[0.2cm] \frac{1}{2} \end{array} \right|1\right) \, G_{j+i-2 v}(x)\right.\\ &\left.-2\, \sum\limits_{v = 0}^{i-1} \frac{1}{v!\, (-i-v-1)!} \ _{2} F_{1}\left.\left( \begin{array}{cc} -v, 1-i+v\\[0.2cm] \frac{3}{2} \end{array} \right|1\right)\, \, G_{j+i-2 v-1}(x)\right). \end{split} \end{equation}$

(5.29)

Chu-Vandemomne identity leads to the following two identities:

$\begin{align*} \ _{2} F_{1}\left.\left( \begin{array}{cc} -v, -i+v\\[0.2cm] \frac{1}{2} \end{array} \right|1\right) = &\frac{\sqrt{\pi } \left(i-v+\frac{1}{2}\right)_v}{\Gamma \left(\frac{1}{2}+v\right)}, \\ \ _{2} F_{1}\left.\left( \begin{array}{cc} -v, 1-i+v\\[0.2cm] \frac{3}{2} \end{array} \right|1\right) = &\frac{\sqrt{\pi } \left(i-v+\frac{1}{2}\right)_v}{2 \Gamma \left(\frac{3}{2}+v\right)}, \end{align*}$

and hence, Formula (5.29) reduces to Formula (5.28). □

Remark 5.3. Some other special reduced formulas of the general formula in (5.22) can be also deduced. The details are omitted.

Corollary 5.3. Consider the two NNIs $i$ and $j$ . We have

$\begin{equation} \begin{split} \tilde{P}^{(-i+1, \mu)}_{i}(x)\, G_{j}(x)& = \frac{2^{i-1} \Gamma \left(-\frac{1}{2}+i\right) \Gamma (1+2 i+\rho +\mu )}{\sqrt{\pi } (1+i+\mu ) \Gamma (1+i+\rho +\mu )} \left(\sum\limits_{v = 0}^i \frac{-1+i+2 i^2-4 i v+4 v^2-\mu +2 i \mu }{(2 i-2 v)! (2 v)!}\, G_{j+i-2v}(x)\right.\\ &\left.-\sum\limits_{v = 0}^{i-1} \frac{2 i^2+4 v (v+1)-\mu +i (-1-4 v+2 \mu )}{(2 i-2 v-1)! (2 v+1)!}\, \, G_{j+i-2v-1}(x)\right). \end{split} \end{equation}$

(5.30)

Corollary 5.4. Consider the two NNIs $i$ and $j$ . We have

$\begin{equation} \begin{split} \tilde{P}^{(\rho, -i+1)}_{i}(x)\, G_{j}(x) = & \frac{2^{-i} \Gamma (1+2 i+\rho +\mu )}{\Gamma (1+i+\rho +\mu )} \sum\limits_{v = 0}^{i} \frac{1}{v! (i-v)!}\, G_{j+i-2v}(x)\\ &-\frac{2^{1-i} (i+\mu ) \Gamma (2 i+\rho +\mu )}{\Gamma (1+i+\rho +\mu )} \sum\limits_{v = 0}^{i-1} \frac{1}{v! (i-v-1)!}\, G_{j+i-2v-1}(x). \end{split} \end{equation}$

(5.31)

Corollary 5.5. Consider the two NNIs $i$ and $j$ . We have

$\begin{equation} \begin{split} \tilde{P}^{(\rho, -i+2)}_{i}(x)\, G_{j}(x) = & \frac{2^{-i} \Gamma (1+2 i+\rho +\mu )}{\Gamma (1+i+\rho +\mu )} \sum\limits_{v = 0}^{i} \frac{1+\frac{4 (i-v) v}{(1+i+\rho ) (2+i+\rho )}}{v! (i-v)!}\, G_{j+i-2v}(x)\\ &-\frac{2^{1-i} (i+\mu ) \Gamma (2 i+\rho +\mu )}{\Gamma (1+i+\rho +\mu )} \sum\limits_{v = 0}^{i-1} \frac{1}{v! (i-v-1)!}\, G_{j+i-2v}(x). \end{split} \end{equation}$

(5.32)

6. Closed forms for some integrals and weighted integrals

This section presents new integral and weighted integral formulas based on applying some of the introduced formulas derived in the previous sections.

6.1. Some definite integrals of products involving the UCPs

Corollary 6.1. For every $j\ge 2$ , the following identity holds

$\begin{equation} \int_{0}^{1}\phi_{j}(x)\, dx = S_{j} = \begin{cases} \frac{\mathcal{B}-2 \mathcal{A}}{2 (j-1)}+\frac{\mathcal{B}}{2 (j+1)}+\frac{\mathcal{R} \left(1+(-1)^{1+\frac{j}{2}}\right)}{j}, & j\ \mathit{\text{even}}, \\[0.3cm] \frac{(\mathcal{B}-2 \mathcal{A}) \left(1+(-1)^{\frac{j+1}{2}}\right)}{2 (j-1)}+\frac{\mathcal{B} \left(1+(-1)^{\frac{j-1}{2}}\right)}{2 (j+1)}+\frac{\mathcal{R}}{j}, & j\ \mathit{\text{odd}}. \end{cases} \end{equation}$

(6.1)

Proof. In virtue of Formula (2.4), we can write

$\int_{0}^{1}\phi_{j}(x)\, dx = \frac{1}{2} (-2 \mathcal{A}+\mathcal{B})\int_{0}^{1} U_{j-2}(x)\, dx+\mathcal{R}\int_{0}^{1} U_{j-1}(x)\, dx+\frac{\mathcal{B}}{2}\, \int_{0}^{1} U_{j}(x)\, dx.$

But, since the following integral holds for $U_{j}(x)$ (see, ^[15])

$\int_{0}^{1} U_{j}(x)\, dx = \begin{cases} \frac{1}{1+j}, & j\, \text{even}, \\ \frac{1-(-1)^{\frac{j+1}{2}}}{1+j}, & j\, \text{odd}. \end{cases}$

So it is easy to conclude Formula (6.1). □

Corollary 6.2. For every $i$ and $j$ greater than or equal to $2$ , the following identity holds

$\begin{equation} \int_{0}^{1}\phi_{i}(x)\, \phi_{j}(x)\, dx = \frac{1}{2} \mathcal{B} (S_{j+i}+S_{j-i})+\mathcal{R} \sum\limits_{k = 0}^{i-1} S_{j-i+2k+1}+(\mathcal{B}-\mathcal{A}) \sum\limits_{k = 0}^{i-2} S_{j-i+2k+2}, \end{equation}$

(6.2)

where $S_{j}$ is given as in (6.1).

Proof. The integral formula can be achieved by using LF (5.4) along with the integral formula (6.1). □

6.2. Some weighted integrals of products involving the UCPs

Some weighted integral formulas can be computed based on the derivative expressions of the UCPs and connection formulas with some orthogonal polynomials. Here are some of these results.

Corollary 6.3. For every $j\ge s$ , the following identity holds

$\begin{equation} \begin{split} &\int_{-\infty }^{\infty } e^{-x^2} D^s G_{j}(x)\, H_m(x) \, dx = \\ &\begin{cases} \frac{2^{-1+m+s}\, \sqrt{\pi }\, (j-2)!}{\left(\frac{1}{2} (j-m-s)\right)!}\, \Bigg(\mathcal{B} (j-1) j \, _1F_1\bigg(\frac{1}{2} (-j+m+s);1-j;-1\bigg)\\ +(\mathcal{B}-\mathcal{A}) (j-m-s) \ _1F_1\bigg( \frac{1}{2} (2-j+m+s);2-j;-1\bigg)\Bigg)& (j+m+s)\ \mathit{\text{even}}, \\[0.4cm] \frac{2^{m+s}\, \sqrt{\pi }\, \mathcal{R}\, m!\, (j-1)!}{m!\, \left(\frac{1}{2} (j-m-s-1)\right)!} \, _1F_1\Bigg(\frac{1}{2} (1-j+m+s);1-j;-1\Bigg), & (j+m+s)\ \mathit{\text{odd}}. \end{cases} \end{split} \end{equation}$

(6.3)

Proof. Formula (3.24) leads to the following integral formula:

$\begin{equation} \begin{split} \int_{-\infty }^{\infty } e^{-x^2} D^s G_{j}(x)\, H_m(x) \, dx = & \sum\limits_{v = 0}^{\left\lfloor \frac{j-s}{2}\right\rfloor } M_{v, j, s} \int_{-\infty }^{\infty } e^{-x^2} H_m(x) H_{j-s-2 v}(x) \, dx\\ &+\sum\limits_{v = 0}^{\left\lfloor \frac{1}{2} (j-s-1)\right\rfloor } {\bar{M}}_{v, j, s} \int_{-\infty }^{\infty } e^{-x^2} H_m(x) H_{j-s-2 v-1}(x) \, dx, \end{split} \end{equation}$

(6.4)

where

$\begin{align*} M_{v, j, s} = & \frac{2^{s-1} (j-2)!}{v! (j-2 v-s)!} (2 (-\mathcal{A}+\mathcal{B}) v \, _1F_1(1-v;2-j;-1)+\mathcal{B} (j-1) j \, _1F_1(-v;1-j;-1)), \\ \bar{M}_{v, j, s} = & \frac{2^s \mathcal{R} (j-1)!}{v! (j-2 v-s-1)!} \, _1F_1(-v;1-j;-1). \end{align*}$

The orthogonality relation of Hermite polynomials (^[57]) helps to put (6.4) as

$\begin{equation} \int_{-\infty }^{\infty } e^{-x^2} D^s G_{j}(x)\, H_m(x) \, dx = h_{m}\, \left(\sum\limits_{v = 0}^{\left\lfloor \frac{j-s}{2}\right\rfloor } M_{v, j, s}\, \delta _{m, j-s-2 v}+\sum\limits_{v = 0}^{\left\lfloor \frac{1}{2} (j-s-1)\right\rfloor } \bar{M}_{v, j, s}\, \delta _{m, j-s-2 v-1}\right), \end{equation}$

(6.5)

where $\delta_{i, j}$ is the well-known Kronecker delta functions and $h_{m}$ is given by

$h_{m} = 2^m\, m!\, \sqrt{\pi}.$

Simple computations lead to (6.3). □

Corollary 6.4. For all NNIs $j$ and $m$ , the following identity holds:

$\begin{equation} \begin{split} &\int_{-\infty }^{\infty } e^{-x^2}\, G_{j}(x)\, H_m(x) \, dx = \\ &\begin{cases} \frac{2^{m-1}\, \sqrt{\pi }\, (j-2)!}{\left(\frac{1}{2} (j-m)\right)!}\, \left(\mathcal{B} (j-1) j \, _1F_1\left(\frac{1}{2} (-j+m);1-j;-1\right)\right.\\ +\left.(-\mathcal{A}+\mathcal{B}) (j-m) \, _1F_1\left(\frac{1}{2} (2-j+m);2-j;-1\right)\right)& (j+m)\ \mathit{\text{even}}, \\[0.4cm] \frac{2^{m} \sqrt{\pi } \mathcal{R} m! (j-1)!}{m! \left(\frac{1}{2} (j-m-1)\right)!} \, _1F_1\left(\frac{1}{2} (1-j+m);1-j;-1\right), & (j+m)\ \mathit{\text{odd}}. \end{cases} \end{split} \end{equation}$

(6.6)

Proof. A direct special case of Formula (6.3) for $s = 0$ . □

Corollary 6.5. For every $j\ge s$ , the following identity holds

$\begin{equation} \begin{split} &\int_{-1}^1 \left(1-x^2\right)^{\zeta -\frac{1}{2}} D^s G_{j}(x)\, U^{(\zeta)}_m(x) \, dx = \frac{2^{s-2} \sqrt{\pi }\, \Gamma \left(\frac{1}{2}+\zeta \right)}{\Gamma (1+s-\zeta )}\\ &\times\begin{cases} \frac{\left(\frac{1}{2} (j+m+s)-1\right)!\, \Gamma \left(\frac{1}{2} (j-m+s-2 \zeta )\right)}{\left(\frac{1}{2} (j-m-s)\right)!\, \Gamma \left(\frac{1}{2} (2+j+m-s)+\zeta \right)}\\ \times\left(-\mathcal{A} (j-m-s) (j+m-s+2 \zeta )+\mathcal{B} \left(j^2-(m+s) (m-s+2 \zeta )\right)\right), & (j+m+s)\ \mathit{\text{even}}, \\[0.3cm] \frac{4\, \mathcal{R}\, \Gamma \left(\frac{1}{2} (1+j+m+s)\right) \Gamma \left(\frac{1}{2} (1+j-m+s-2 \zeta )\right)}{\left(\frac{1}{2} (j-m-s)-1\right)!\, \Gamma \left(\frac{1}{2} (1+j+m-s)+\zeta \right)}, & (j+m+s)\ \mathit{\text{odd}}. \end{cases} \end{split} \end{equation}$

(6.7)

Proof. Starting with Formula (3.12) and applying the orthogonality relation of the ultraspherical polynomials ^[58], we may derive Formula (6.7). □

Corollary 6.6. For every $j$ , the following identity holds

$\begin{equation} \begin{split} &\int_{-1}^1 \left(1-x^2\right)^{\zeta -\frac{1}{2}} G_{j}(x)\, U^{(\zeta)}_m(x) \, dx = \frac{1}{4}\, \sqrt{\pi }\, \Gamma \left(\frac{1}{2}+\zeta \right)\\ &\times\begin{cases} \frac{\left(-\mathcal{A} (j-m) (j+m+2 \zeta )+\mathcal{B} \left(j^2-m (m+2 \zeta )\right)\right)\, \left(\frac{j+m}{2}-1\right)!\, (1-\zeta )_{\frac{j-m}{2}-1}}{\left(\frac{j-m}{2}\right)!\, \Gamma \left(\frac{1}{2}(2+j+m)+\zeta \right)}, & (j+m)\ \mathit{\text{even}}, \\[0.3cm] \frac{4\, \mathcal{R}\, \left(\frac{1}{2} (j+m-1)\right)!\, (1-\zeta )_{\frac{1}{2} (j-m+1)-1}}{\left(\frac{1}{2} (j-m-1)\right)!\, \Gamma \left(\frac{1}{2} (1+j+m)+\zeta \right)}, & (j+m)\ \mathit{\text{odd}}. \end{cases} \end{split} \end{equation}$

(6.8)

Proof. Direct from (6.7) setting $s = 0$ . □

7. Conclusions

This research opened new horizons for studying new types of polynomials that satisfy the same recurrence relation of CPs but with general initials. Thus, the solution of this recurrence relation produced generalized polynomials that all four kinds of CPs are special ones of them. We proved an important result for the generalized polynomials: They can be expressed as three terms of consecutive terms of the second kind of CPs. This result enabled us to derive some other fundamental formulas for generalized polynomials. Expressions for the derivatives of these polynomials are given in terms of some other symmetric and nonsymmetric polynomials. Some connection formulas can also be deduced. The LF of the generalized polynomials and some triple product formulas were also deduced. In future work, we aim to investigate other generalized sequences of polynomials and establish some important formulas concerned with them.

Author contributions

Waleed Mohamed Abd-Elhameed: Conceptualization, Methodology, Validation, Formal analysis, Funding acquisition, Investigation, Project administration, Supervision, Writing-Original draft, Writing-review & editing; Omar Mazen Alqubori: Validation, Investigation. All authors have read and approved the final version of the manuscript for publication.

Use of AI tools declaration

The authors declare that they have not used artificial intelligence tools in the creation of this article.

Acknowledgement

This work was funded by the University of Jeddah, Jeddah, Saudi Arabia, under grant No. (UJ-23-DR-254). Therefore, the authors thank the University of Jeddah for its technical and financial support.

Conflict of interest

The authors declare that they have no competing interests.

References

[1]	Modrzewska B, Wyszkowski M (2014) Trace metals content in soils along the state road 51 (north-eastern Poland). Environ Monit Assess 186: 2589-2597. doi: 10.1007/s10661-013-3562-z
[2]	Osakwe SA (2013) Chemical partitioning of iron, cadmium, nickel and chromium in contaminated soils of south-eastern Nigeria. Chem Speciation Bioavailability 25: 71-78. doi: 10.3184/095422913X13581872822530
[3]	Bień JD, Meer J, Rulkens WH, et al. (2005) A GIS-based approach for the long-term prediction of human health risks at contaminated sites. Environ Model Assess 9: 221-226. doi: 10.1007/s10666-005-0909-z
[4]	Jalali M, Karami S, Marj AF (2016) Geostatistical Evaluation of Spatial Variation Related to Groundwater Quality Database: Case Study for Arak Plain Aquifer, Iran. Environ Model Assess 21: 707-719. doi: 10.1007/s10666-016-9506-6
[5]	Krami LK, Amiri F, Sefiyanian A, et al. (2013) Spatial patterns of heavy metals in soil under different geological structures and land uses for assessing metal enrichments. Environ Monit Assess 185: 9871-9888. doi: 10.1007/s10661-013-3298-9
[6]	Goovaerts P (1997) Geostatistics for natural resources evaluation. Oxford Uni. Press, New York, 483.
[7]	Lin WC, Lin YP, Wang YC (2016) A decision-making approach for delineating sites which are potentially contaminated by heavy metals via joint simulation. Environ Pollut 211: 98-110. doi: 10.1016/j.envpol.2015.12.030
[8]	Shen F, Liao R, Ali A, et al. (2017) Spatial distribution and risk assessment of heavy metals in soil near a Pb/Zn smelter in Feng County, China. Ecotoxicol Environ Saf 139: 254-262. doi: 10.1016/j.ecoenv.2017.01.044
[9]	Xie Y, Chen TB, Lei M, et al. (2011) Spatial distribution of soil heavy metal pollution estimated by different interpolation methods: Accuracy and uncertainty analysis. Chemosphere 82: 468-476. doi: 10.1016/j.chemosphere.2010.09.053
[10]	Zawadzki J, Fabjańczyk P (2012) Geostatistical evaluation of lead and zinc concentration in soils of an old mining area with complex land management. Int J Environ Sci Technol 10: 729-742 doi: 10.1007/s13762-012-0132-9
[11]	Namysłowska-Wilczyńska B (2019) Application of Geostatistical Techniques for the Determining of an Anomalous Zone of Copper Ore Deposit in the Area of Polkowice Mine (Region of Lubin-Sieroszowice, SW Part of Poland). Geoinfor Geostat Overv 7: 1-22
[12]	Namysłowska-Wilczyńska B, Wilczyński A (2002) Multivariate estimation and simulationfor environmental data modelling: processing of heavy metals concentration data in soil. Data Sci J 1: 27-44 doi: 10.2481/dsj.1.27
[13]	Ripin SNM, Hasan S, Kamal M (2014) Environmental Geochemical Mapping on Distribution of Metal Contamination in Topsoils Perlis, Malaysia. J Med Bioeng 3: 277-281.
[14]	Cai L, Xu Z, Bao P, et al. (2015). Multivariate and geostatistical analyses of the spatial distribution and source of arsenic and heavy metals in the agricultural soils in Shunde, Southeast China. J Geochem Explor 148: 189-195. doi: 10.1016/j.gexplo.2014.09.010
[15]	Salminen R, Tarvainen T, Demetriades A, et al. (1998) FOREGS Geochemical Mapping Field Manual. Geological Survey of Finland.
[16]	De Vivo B, Lima A, Bove AM, et al. (2006) Environmental Geochemical Atlas of Italy. European Congress on Regional Geoscientific Cartography and Earth Information and Systems Water, 126-127.
[17]	Guillén MT, Delgado J, Albanese S, et al. (2011) Environmental geochemical mapping of Huelva municipality soils (SW Spain) as a tool to determine background and baseline values. J Geochem Explor 109: 59-69. doi: 10.1016/j.gexplo.2011.03.003
[18]	Frentiu T, Ponta M, Levei E, et al. (2008) Preliminary study on heavy metals contamination of soil using solid phase speciation and the influence on groundwater in Bozanta-Baia Mare Area, Romania. Chem Speciation Bioavailability 20: 99-109. doi: 10.3184/095422908X324471
[19]	Malina G (2004) Ecotoxicological and environmental problems associated with the former chemical plant in Tarnowskie Gory, Poland. Toxicology 205: 157-172. doi: 10.1016/j.tox.2004.06.064
[20]	Saito H, Goovaerts P (2000) Geostatistical Interpolation of Positively Skewed and Censored Data in a Dioxin-Contaminated Site. Environ Sci Technol 34: 4228-4235. doi: 10.1021/es991450y
[21]	Fowler D, Skiba U, Nemitz E, et al. (2004). Measuring Aerosol and Heavy Metal Deposition on Urban Woodland and Grass Using Inventories of ²¹⁰Pb and Metal Concentrations in Soil. Water Air Soil Pollut Focus 4: 483-499. doi: 10.1023/B:WAFO.0000028373.02470.ba
[22]	Brunner PH, Rechberger H (2016) Practical Handbook of Material Flow Analysis. CRC Press, 173-178.
[23]	Pleim J, Venkatram A, Yamartino R (1984) ADOM/TADAM model development program. ERT P-b980-520, prepared for OME AES of Canada and the Umweltbundesamt, West Germany, 111.
[24]	Sportisse B (2007) A review of parameterizations for modelling dry deposition and scavenging of radionuclides, Atmos Environ 41: 2683-2698.
[25]	Hopenhayn C (2006) Arsenic in Drinking Water: Impact on Human Health. Elements 2: 103-107. doi: 10.2113/gselements.2.2.103
[26]	Kapaj S, Peterson H, Liber K, et al. (2006) Human Health Effects From Chronic Arsenic Poisoning-A Review. J Environ Sci Health Part A Toxic/Hazard Subst Environ Eng 41: 2399-2428. doi: 10.1080/10934520600873571
[27]	Zawierucha I, Kozłowski C, Malina G (2016) Immobilized materials for removal of toxic metal ions from surface/groundwaters and aqueous waste streams. Environ Sci Processes Impacts 18: 429. doi: 10.1039/C5EM00670H
[28]	Kucharczak-Moryl E, Moryl A, Żmuda R (2014) Environment influence arsenic content in croplands in the zgorzelecki-bogatyński region. Ecological Engineering, No 37: 107-116. Available from: http://www.ineko.net.pl/Wplyw-srodowiska-na-zawartosc-arsenu-w-glebach-uprawnych-rejonu-zgorzelecko-bogatynskiego,283,0,1.html. Accessed 5 May 2016.
[29]	Mikulski S (2010) Gold, arsenic and dopeore. Polish Geological Institute-National Research Institute.
[30]	Kuys K, Ralston J, Sobieraj S, et al. (1989) Methods for the enrichment of bauxite ultrafines. Physicochem Metall Probl 21: 23-26.
[31]	Bernard A (2008) Cadmium & its adverse effects on human health. Indian J Med Res 128: 557-564.
[32]	Kabata-Pendias A, Piotrkowska M (1997) Transfer of cadmium, zinc and lead from soils to plants. The 5th international conference proceedings: transport, fate and effects of silver in the environment, 171-176.
[33]	Kwiatkowska-Malina J, Maciejewska A (2013) Uptake of heavy metals by darnel multifloral (Loliummultiflorum Lam.) at diverse soil reaction and organic matter content. Soil Sci Annu 64: 19-23.
[34]	Maciejewska A, Kwiatkowska J (2001) Contamination of soil with heavy metals along highways as well as in the city of Warsaw, In Obieg pierwiastków w przyrodzie (in Polish), Instytut Ochrony Środowiska: 49-54.
[35]	Maciejewska A, Ociepa E, Kwiatkowska J (2003) Changes in the availability of heavy metals and content of organic carbon in polluted soil after the application of an organic-mineral fertilizer obtained from brown coal. Pol J Soil Sci 36:129-136.
[36]	Duda-Chodak A, Błaszczyk U (2008) The impact of nickel on human health. J Elem 13: 685-696.
[37]	Cempel M, Nikel G (2006) Nickel: A Review of Its Sources and Environmental Toxicology. Pol J Environ Stud 15: 375-382.
[38]	Järup L, Åkesson A (2009) Current status of cadmium as an environmental health problem. Toxicol Appl Pharmacol 238: 201-208. doi: 10.1016/j.taap.2009.04.020
[39]	Mohammed AS, Kapri A, Goel R (2011) Heavy Metal Pollution: Source, Impact, and Remedies: Biomanagement of Metal-Contaminated Soils. Environ Pollut 20: 1-28. doi: 10.1007/978-94-007-1914-9_1
[40]	Pistocchi A, Groenwold J, Lahr J, et al. (2011) Mapping Cumulative Environmental Risks: Examples from the EU NoMiracle Project. Environ Model Assess 16: 119-133. doi: 10.1007/s10666-010-9230-6
[41]	Strzelecki R (2004) Directions and problems of abiotic environment research in Poland conducted by the State Geological Survey. Pol Geol Rev 52: 716-719.
[42]	Institute of Soil Science and Plant Cultivation-State Research Institute (2010) The assessment of soil contamination in Poland. Available from http://www.iung.pulawy.pl/index.php?option=com_content&view=article&id=147:zanieczyszczenia&catid=45:oferta&Itemid=115. Accessed 11 June 2016.

This article has been cited by:

莉周, Vertex Reducible Edge (Total) Coloring of Two Classes of Generalized Petersen Graph, 2023, 13, 2160-7583, 1851, 10.12677/PM.2023.136188

Reader Comments

Your name:*

Email:*
© 2020 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Geosciences

0.9

Metrics

Article views(5471) PDF downloads(551) Cited by(6)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

Figures and Tables

Figures(8) / Tables(1)

AIMS Geosciences

Geostatistical modelling of soil contamination with arsenic, cadmium, lead, and nickel: the Silesian voivodeship, Poland case study

Related Papers:

Abstract

1. Introduction

2. Some fundamental properties of the UCPs and other well-known polynomials

2.1. Some basic formulas concerned with the UCPs

2.2. An overview on well-known polynomials: both symmetric and nonsymmetric

3. Some formulas for the derivatives of the UCPs

3.1. Derivatives of UCPs in terms of other UCPs

3.2. Derivatives with some other symmetric and nonsymmetric polynomials

4. Derivatives' expressions of various celebrated polynomials in terms of UCPs

5. Linearization formulas of the UCPs

5.1. Linearization formula of the UCPs

5.2. LFs of the UCPs with some symmetric polynomials

5.3. LFs of the UCPs with some nonsymmetric polynomials

6. Closed forms for some integrals and weighted integrals

6.1. Some definite integrals of products involving the UCPs

6.2. Some weighted integrals of products involving the UCPs

7. Conclusions

Author contributions

Use of AI tools declaration

Acknowledgement

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Catalog

AIMS Geosciences

Geostatistical modelling of soil contamination with arsenic, cadmium, lead, and nickel: the Silesian voivodeship, Poland case study

Related Papers:

Abstract

1. Introduction

2. Some fundamental properties of the UCPs and other well-known polynomials

2.1. Some basic formulas concerned with the UCPs

2.2. An overview on well-known polynomials: both symmetric and nonsymmetric

3. Some formulas for the derivatives of the UCPs

3.1. Derivatives of UCPs in terms of other UCPs

3.2. Derivatives with some other symmetric and nonsymmetric polynomials

4. Derivatives' expressions of various celebrated polynomials in terms of UCPs

5. Linearization formulas of the UCPs

5.1. Linearization formula of the UCPs

5.2. LFs of the UCPs with some symmetric polynomials

5.3. LFs of the UCPs with some nonsymmetric polynomials

6. Closed forms for some integrals and weighted integrals

6.1. Some definite integrals of products involving the UCPs

6.2. Some weighted integrals of products involving the UCPs

7. Conclusions

Author contributions

Use of AI tools declaration

Acknowledgement

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog