1.
Introduction
Population migration is a common phenomenon. With the migration of population, infectious diseases can easily spread from one area to another, so it is meaningful to consider population migration when studying the spread of infectious diseases [1,2,3,4,5,6,7].
Wang and Mulone [2] established an SIS infectious disease model with standard incidence based on two patches. It is proved that the basic reproduction number is the threshold of the uniform persistence and disappearance of the disease. The dispersal rate of the population will make the infectious disease persist or disappear in all patches. There will be no the phenomena that infectious diseases persists in one patch but disappears in the other.
Sun et al. [3] put forward an SIS epidemic model with media effect in a two patches setting. Under the assumption that the migration matrix is irreducible, it is proved that if the basic reproduction number is greater than 1 then the system persists and solutions converge to an endemic equilibrium and that if the basic reproduction number is less than 1 then solutions tend to an equilibrium without disease.
Gao et al. [5,6] studied an SIS multi-patch model with variable transmission coefficients. Their results show that the basic reproduction number R0 is a threshold parameter of the disease dynamics.
All the patch models referenced above assume that the migration matrix is irreducible. The studies in which the migration matrix is reducible are few. Therefore, based on the case of two patches, we consider that the individuals can only migrate from one patch to the other. In this case, the migration matrix is reducible. It can characterize the phenomenon that individuals migrate in one direction between two regions, such as, from the rural patch to the urban one [8] and from a small community hospital to a large teaching hospital [4].
It is well known that the incidence rate plays an important role in the modeling of infectious disease. Considering the saturation phenomenon for numerous infected individuals, Capasso and Serio [9] first introduce a nonlinear bounded function g(I) to form the interaction term g(I)S in 1978. It can characterize the behavioral changes of individuals, such as wearing masks or reducing their social activities and direct contact with others with the increase of infectious individuals. After that, the saturation incidence rate has attracted much attention and various nonlinear types of incidence rate are employed. The most commonly used types are Holling type Ⅱ λSI1+αI [10,11,12] and βSI1+αS [13], Monod-Haldane type λSI1+αI2 [14], Beddington-DeAngelis type λSI1+αS+βI [15,16,17] and Crowley-Martin type λSI(1+αS)(1+βI) [18,19].
In this paper, we consider infectious disease transmission models with saturation incidence rate. The rest of this paper is organized as follows: In Section 2, we establish a two-patch SIS model with saturating contact rate and one-directing population dispersal. We discuss the existence of disease-free equilibrium, boundary equilibrium and endemic equilibrium and prove the global asymptotic stability of the equilibriums in Section 3. In Section 4, we perform simulations to illustrate the results and analyze the effect of the contact rate and population migration on epidemic transmission. Finally, we discuss in Section 5.
2.
Model formulation
In the two patches, the population is divided into two states: susceptible and infective. Thus we can establish a two-patch SIS model with saturating contact rate and one-directing population dispersal
where Si is the number of susceptible population in patch i (i=1,2), Ii is the number of infective population in patch i (i=1,2), Ai is the recruitment into patch i (i=1,2), di is the natural mortality rate, γi is the recovery rate of an infective individual in patch i (i=1,2), m is the migration rate form patch 1 to patch 2. Since the individuals can migrate from the first patch to the second, patch 1 is the source patch and patch 2 is the sink patch. The initial conditions is
Denote the population in patch i by Ni. Then Ni=Si+Ii. From system (2.1), the differential equations governing the evolution of N1 and N2 are
Obviously, system (2.3) has a unique equilibrium (N∗1,N∗2)=(A1d1+m,A2d2+mA1d2(d1+m)) which is globally asymptotically stable for (2.3). So (2.1) is equivalent the following system
Because limt→∞Ni(t)→N∗i (i=1,2), system (2.4) leads to the following limit system
3.
Mathematical analysis for system (2.5)
3.1. The invariants and equilibriums
Let Ω={(I1,I2)|0≤I1≤N∗1,0≤I2≤N∗2}. Then Ω is invariant region for system (2.5).
Define the basic reproduction number in the two patches respectively by R10=β1A1(d1+γ1+m)2=β1d1+γ1+mN∗1, R20=β2(d2+γ2)N∗2. The basic reproduction number R10 gives the expected secondary infections in the source patch produced by a primary infected individual in the source patch when the population is supposed to be in the disease-free equilibrium. The basic reproduction number R20 gives the expected secondary infections in the sink patch produced by a primary infected individual in the sink patch when the population is supposed to be in the disease-free equilibrium. Then we have the following theorem.
Theorem 3.1. For the system (2.5), we have
(i) The disease-free equilibrium E0:=(0,0) always exists;
(ii) The boundary equilibrium E1:=(0,β2N∗2−d2−γ2(d2+γ2)α2+β2) exists if R20>1;
(iii) There is a unique epidemic equilibrium E∗ if R10>1.
Proof. (ⅰ) can be easily proved.
Let
From Eq (3.1), we can have I1=0 always satisfies Eq (3.1). When I1=0, from Eq (3.2), we have
If R20>1, then I2=β2N∗2−d2−γ2(d2+γ2)α2+β2>0. So The boundary equilibrium E1:=(0,β2N∗2−d2−γ2(d2+γ2)α2+β2) exists if R20>1. The conclusion (ⅱ) is proved.
If R10>1, Eq (3.1) has a positive solution I∗1=β1N∗1−(d1+m+γ1)(d1+m+γ1)α1+β1. Solve Eq (3.2), we have
Substituting I∗1 into Eq (3.3), we have
Since I∗2≥0 is meaning only, we take
So if R10>1, there is a unique epidemic equilibrium E∗=(I∗1,I∗2), where I∗1=β1N∗1−(d1+m+γ1)(d1+m+γ1)α1+β1 and I∗2=(β2N∗2−d2−γ2+mα2I∗1)+√(β2N∗2−d2−γ2+mα2I∗1)2+4[(d2+γ2)α2+β2]mI∗12[(d2+γ2)α2+β2]. The conclusion (ⅲ) is proved.
This completes the proof of the theorem.
From the above analysis, we have the following theorem.
Theorem 3.2. For the system (2.5), we have
(i) If R10<1 and R20<1, there is the disease-free equilibrium E0 only;
(ii) If R10<1 and R20>1, there are the disease-free equilibrium E0 and the boundary equilibrium E1;
(iii) If R10>1 and R20<1, there are the disease-free equilibrium E0 and the epidemic equilibrium E∗;
(iv) If R10>1 and R20>1, there are the disease-free equilibrium E0, the boundary equilibrium E1 and the epidemic equilibrium E∗.
Remark 3.1. Define the basic reproduction number R0 of the system (2.5) by the spectral radius of the next generation matrix [20], we have
where ρ(A) denotes the spectral radius of a matrix A. So from the above analysis, we know that R0=max{R10,R20}.
3.2. The stability of the disease-free equilibrium
The next, we shall discuss the local stability of the disease-free equilibrium firstly. Then we discuss the global asymptotical stability.
Theorem 3.3. For the system (2.5), we have
(i) If R10<1 and R20<1, the disease-free equilibrium E0 is locally asymptotically stable;
(ii) If R10>1 or R20>1, the disease-free equilibrium E0 is unstable.
Proof. The linearized system of (2.5) at the equilibrium E0 is
The associated characteristic equation of the linearized system of (3.4) at the equilibrium E0 is
It is easy to see that the two eigenvalues of characteristic Eq (3.5) are
and
So, when R10<1 and R20<1, the disease-free equilibrium E0 is locally asymptotically stable; However, if R10>1 or R20>1, the disease-free equilibrium E0 is unstable.
Remark 3.2. From Theorem 3.3, we know that for the system (2.5), if R0<1 the disease-free equilibrium E0 is locally asymptotically stable; if R0>1, the disease-free equilibrium E0 is unstable.
Theorem 3.4. For the system (2.5), if R10<1 and R20<1, the disease-free equilibrium E0 is globally asymptotically stable.
Proof. Since Ii1+αiIi≤Ii for i=1,2, from system (2.5), we can obtain that
Define an auxiliary linear system using the right hand side of (3.6) as follows
It can be rewritten as
if R10<1 and R20<1, we can solve (3.7) and know that limt→∞I1(t)=0 and limt→∞I2(t)=0. By the comparison principle [21], we can conclude that when R10<1 and R20<1, all non-negative solutions of (2.5) satisfy limt→∞Ii(t)=0 for i=1,2. So the disease-free equilibrium E0 is globally asymptotically stable.
3.3. The stability of the boundary equilibrium
In this subsection, we will discuss the local stability of the boundary equilibrium firstly. Then discuss the global asymptotical stability.
Theorem 3.5. For the system (2.5), if R10<1 and R20>1, the boundary equilibrium E1 is globally asymptotically stable.
Proof. The Jacobian matrix at the boundary equilibrium E1 of system (2.5) is
The two eigenvalues of the Jacobian matrix are
and
So, when R10<1 and R20>1, λ1<0 and λ2<0. That is the boundary equilibrium E1 is locally asymptotically stable.
For every (I1(0),I2(0))∈Ω, assume the solution of the system (2.5) with initial value (I1(0),I2(0)) is (I1(t),I2(t)). Since
if R10<1, dI1(t)dt<0, then I1(t) is positive and decreasing and limt→∞I1(t)=0. So for sufficiently small positive number ϵ1, there exists a T, such that I1(T)=ϵ1 and I1(t)<ϵ1 when t>T.
The following, we prove that for any ϵ>0, there exists a T∗>T such that |I2(T∗)−β2N∗2−d2−γ2β2+(d2+γ2)α2|<ϵ. And because E1=(0,β2N∗2−d2−γ2β2+(d2+γ2)α2) is locally asymptotically stable, we have E1 is globally asymptotically stable.
Since
if I2(T)<β2N∗2−d2−γ2β2+(d2+γ2)α2, then dI2(t)dt>0 for t>T. So I2(t) is increasing and there exists T∗1 such that |I2(T∗1)−β2N∗2−d2−γ2β2+(d2+γ2)α2|<ϵ;
if I2(T)>β2N∗2−d2−γ2β2+(d2+γ2)α2, there are two cases:
ⅰ) I2(t) is decreasing for t>T. In this case, there exists T∗2>T, such that |I2(T∗2)−β2N∗2−d2−γ2β2+(d2+γ2)α2|<ϵ;
ⅱ) There exists T1>T, such that dI2(T1)dt>0. That is
Since I1(t)<ϵ1 for t>T, we have
Since I2(T)>β2N∗2−d2−γ2β2+(d2+γ2)α2, we have
So
If only ϵ1<(d2+γ2)α2+β2(α2+β2+(d2+γ2)α2β2N∗2−d2−γ2)mϵ, then |I2(T∗2)−β2N∗2−d2−γ2β2+(d2+γ2)α2|<ϵ. It is completed.
3.4. The stability of the epidemic equilibrium
In this subsection, we will discuss the local stability of the epidemic equilibrium firstly and then discuss the global asymptotical stability.
Theorem 3.6. For the system (2.5), if R10>1, the epidemic equilibrium E∗ is locally asymptotically stable.
Proof. The Jacobian matrix at the epidemic equilibrium E∗ of system (2.5) is
The two eigenvalues of the Jacobian matrix are
and
It is easy to see that if R10>1, λ1<0. The next, we need only prove the second eigenvalue λ2<0 if R10>1. Since (1+α2I∗2)2>0, we need only prove β2N∗2−2β2I∗2−2β2α2I∗22−(d2+γ2)(1+α2I∗2)2<0. Let
Since I∗2≥(β2N∗2−d2−γ2+mα1I∗1)(d2+γ2)α2+β2, so
This completes the proof.
Theorem 3.7. For the systeml (2.5), if R10>1, the epidemic equilibrium E∗ is globally asymptotically stable.
Proof. Since E∗ is stable when R10>1, we need only prove E∗ is globally attractive.
Consider the equation
Let
Then f′1(I1)=β1−1−N∗1α1(1+α1I1)2<0. So f1(I1) is a monotonic decreasing function for all I1>0. Furthermore, f1(0)>0, f1(N∗1)<0 and f1(I∗1)=0 when R10>1. That means if I1∈(0,I∗1), f1(I1)>0 and dI1(t)dt>0; if I1∈(I∗1,N∗1), f1(I1)<0 and dI1(t)dt<0. Hence limt→∞I1(t)=I∗1. By Eq (3.3), limt→∞I2(t)=I∗2. Thus E∗ is globally asymptotically stable.
The results about the existence and stability of equilibria are summarized in Table 1.
Remark 3.3. From Theorems 3.5 and 3.7, we know that for the system (2.5), if R0>1, the infectious disease is uniformly persistent. However, the infectious disease is not always uniformly persistent in every patch. If R0>1, but R10<1, the disease is uniformly persistent in the sink patch, but is extinct in the source patch. If R10>1, the disease is always uniformly persistent in every patch. This is a different conclusion resulted by the reducible migration matrix.
Remark 3.4. From Theorem 3.5, in the case that R10<1 and R20>1, the infection does not persist in the source patch but is able to persist in the sink patch. So, in the early stage of the spread of infectious disease, the sink patch should assess the reproduction number R20 reasonably and take control measures timely to prevent the epidemic.
4.
Simulations
In this section, we carry on numerical simulations to verify the theoretical conclusions, reveal the influence of the migration rate form patch 1 to patch 2 on the basic reproduction number, the transmission scale and transmission speed, and discuss the influence of the parameters α1 and α2 that measure the inhibitory effect on the basic reproduction number, the transmission scale and transmission speed.
To numerically illustrate the theoretical results, we need to choose some parameter values (see Table 2).
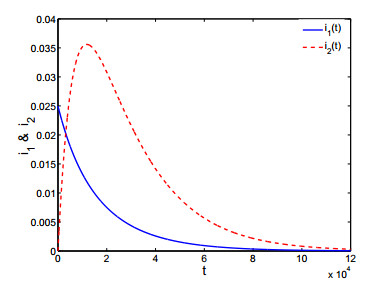
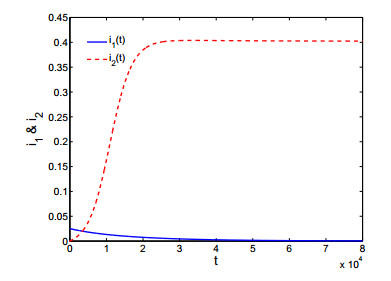
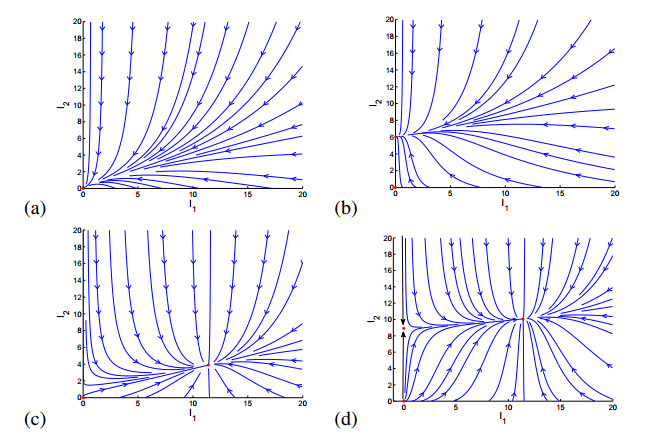
We verify the theoretical conclusions firstly. Denote the density of the infective individuals in patch 1 by i1(t)=I1(t)N∗1. Denote the density of the infective individuals in patch 2 by i2(t)=I2(t)N∗2. Figure 1 shows the evolution of the density of infective individuals in the two patches when R10=0.8889 and R20=0.7500. As predicted by the analytic calculation, the infectious disease in the two patches will disappear eventually. Figure 2 shows the evolution of the density of infective individuals in the two patches when R10=0.8889 and R20=1.8750. We can see the infectious disease will be endemic in patch 2 and the infectious disease in patch 1 will disappear eventually. Figure 3 shows the evolution of the density of infective individuals in the two patches when R10=1.4815 and R20=2.2917. We can see the infectious disease will be endemic in the two patches. And we can see the infectious disease will be endemic in the two patches if R10>1 from the subfigures (c) and (d) of Figure 4.
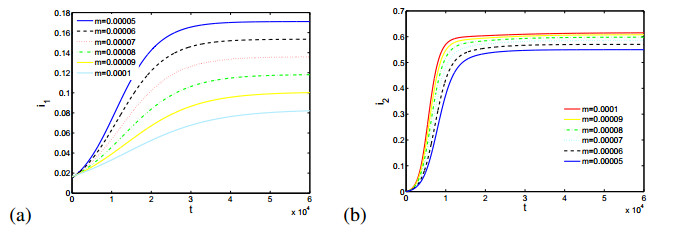
Second, we reveal the influence of the migration rate m on the transmission in Figure 5. With the increasing of m, the density of infective individuals in patch 1 i1 is decreasing, however the density of infective individuals in patch 2 i2 is increasing.
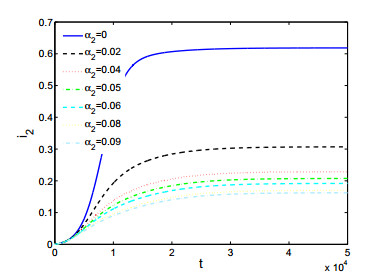
Third, we reveal the parameters α1 and α2 on the transmission scale and transmission speed. We can see that when α1 is increasing, the density of infective individuals in patch 1 i1 is decreasing from Figure 6 and When α2 is increasing, the density of infective individuals in patch 2 i1 is decreasing from Figure 7.
5.
Discussion and conclusions
Many scholars have studied infectious disease transmission with population migration [1,2,3,4,5,6,7], assuming that the migration matrix is irreducible, and found that the propagation dynamics of infectious diseases is determined by the basic reproduction number of the system. When the basic reproduction number is less than 1, the infectious disease eventually becomes extinct; when the basic reproduction number is larger than 1, the infectious disease is epidemic eventually. Since the migration matrix is irreducible, all patches are a connected whole. In all patches, infectious diseases are either extinct or epidemic. That is there is not the phenomenon that infectious diseases are extinct in some patches but epidemic in the others.
Because the studies about the spread of infectious diseases with reducible migration matrix are rare, in this paper, we proposed a two-patch SIS model with saturating contact rate and one-directing population dispersal, discussed the global asymptotic stability of the disease-free equilibrium, the boundary equilibrium and the endemic equilibrium respectively, and revealed the influence of saturating contact rate and migration rate on basic reproduction number and the transmission scale. We have the following main conclusions:
1) If R10>1 then the system tends to a global endemic equilibrium in which infected individuals are present in both patches provided initially there were infected individuals in the source patch; If R10<1 and R20>1 then the system converges to an equilibrium with infected individuals only in the sink patch; If R10<1 and R20<1 then the system converges to the disease-free equilibrium.
2) When migration rate is increasing, the density of infective individuals in the source patch is decreasing; but the density of infective individuals in the sink patch is increasing;
3) With the increasing of the parameter αi (i=1,2) in saturating contact rate, the density of infective individuals in patch i (i=1,2) is decreasing.
The similar conclusions can be obtained for the two patch SI model
We can generalize the current model in many aspects to increase realism. For instance, the infection rate can be given by β(I)SI. We can give the properties on function β(I) such that β(I) is decreasing and tends to 0 when I tends to infinity. The mortality rates of the susceptible and infected individuals are the same in the current model. In fact, the disease-induced death rate can not be neglected sometimes. So the disease-induced death rate can be considered. It is also significant to consider heterogeneous number of contacts for each individual on complex network. There are many paper on this topic [22,23]. One can investigate the multi-patch epidemic model with reducible migration matrix.
Acknowledgments
This work is supported by the National Natural Sciences Foundation of China (Nos.12001501, 12071445, 11571324, 61603351), Shanxi Province Science Foundation for Youths (201901D211216), the Fund for Shanxi '1331KIRT'.
Conflict of interest
All authors declare no conflicts of interest in this paper.









 DownLoad:
DownLoad: