Overview of wind power development over the two past decades (2000-2019) and its role in the Taiwan's energy transition and sustainable development goals

1.   Introduction

Geographically, Taiwan is located in in the East Asia. The East China Sea lies to its north, the Philippine Sea to its east, the Luzon Strait to its south, and the Taiwan Strait to the west. In addition, Taiwan featured its high population density with the majority of population (about 23.6 millions) living in the land area of about 36,000 square kilometers. Although the rapid industrialization and high economic growth of Taiwan in the past decades have resulted in the (GDP) per capita of over US$25,000 in 2018 ^[1], its dependence on imported energy is as high as about 98% ^[2]. Furthermore, the energy supply in Taiwan rapidly went from 94.72 million kiloliters of oil equivalent (KLOE) in 1999 to 148.40 million KLOE in 2019 ^[2]. In order to reduce the dependence on external energy supplies and also mitigate the greenhouse gas (GHG) emissions, the Taiwan government has been actively promoting renewable energy development and energy-saving since the late 1990s ^[3,4,5,6,7]. Among the renewable power technologies, wind power technology is quite mature as compared to hydropower and solar photovoltaic technologies. In addition, the western coast of Taiwan and offshore islands in the Taiwan Strait have quite abundant wind energy due to the strong northeast monsoon. This situation is very similar to the European Union (EU) countries ^[8]. Therefore, the European Unions (EU) and Asian countries are the regions which currently have the highest power potential around the world ^[9]. Soares-Ramos et al. ^[10] gathered and also analyzed the latest information demonstrating the status and trends of existing off-shore wind power above 150 megawatt (MW) in Europe, indicating an increasing trend due to the development aims at reaching about 100 gigawatt (GW) of off-shore wind power in terms of installed capacity by 2030. Based on the installed capacity, Germany currently contributes with about 7105 MW of off-shore wind power above 150 MW, which corresponds to 36% of the large offshore wind power in Europe ^[10]. In Asian countries, China should be the largest wind power producer followed by India ^[11]. By the end of 2018, the cumulative installed capacity of wind power in China and India reached 211.39 GW and 35.14 GW, respectively, which were equivalent to the contributions to global installed capacity by 35.73% and 4.94%, respectively ^[12].

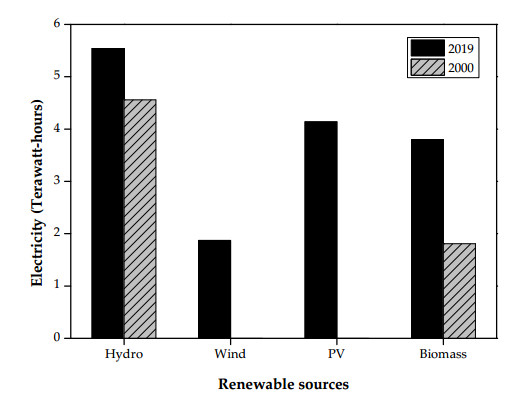
Regarding the electricity supply by renewable sources over the past two decades (2000–2019) ^[2,13], the data indicated an increasing trend from 6.37 terawatt-hours (TW-h) in 2000 to 15.36 TW-h in 2019, which is equivalent to an average annual growth rate of 2.65%. Of total electricity production in 2000 and 2019 ^[2], the data on the electricity supplies as classified by the respective renewable sources were shown in Figure 1. Due to small amounts of electricity generation from wind power and photovoltaic (PV) power in 2000 (i.e., 1.38 and 0.12 GW-h from wind power and PV power, respectively), their corresponding symbols can't be seen in Figure 1 ^[2]. Obviously, the amounts of electricity production from wind power and PV power indicated a roaring growth, but those from conventional hydropower and waste-to-power showed no significant increase. It should be noted that the increasing trend can be divided by two growth periods. During the period of 2000–2009, the wind power rapidly grew from 1.38 gigawatt-hours (GW-h) in 2000 to 786.64 GW-h in 2009. By contrast, the electricity generation by wind power during the period of 2010–2019 only increased from 1,026.34 GW-h in 2010 to 1,871.32 GW-h in 2019. Due to the restriction of available land for installing on-shore wind power system, the "Four-year Wind Power Promotion Plan (2017–2020)", passed by the Taiwan government in 2017, thus focused on the development of off-shore wind farms because many of the world's top 20 sites for offshore wind farms located in the Taiwan Strait by an independent marine energy consultant (i.e., 4C-Offshore).

According to the authorization of the Energy Management Act Amendment in 2016, the Taiwan government approved the "Guidelines on Energy Development" for implementation in 2017 ^[14], so as to achieve the targets of nuclear-free homeland and 20% of total electricity supply from renewable resources by 2025. In this regard, the wind power plays a vital role in the development goals of energy supply diversification and low-carbon society, especially in Taiwan. The aim of this work was to focus on an analysis of progressive wind power development over the two past decades (2000–2019) in Taiwan according to the official statistics by the Ministry of Economic Affairs (MOEA) ^[2]. Therefore, this paper was structured by three key issues. First, a brief description about the Taiwan's wind resource was presented. Subsequently, the wind power development in Taiwan was addressed to be incorporated into the interactive issues, including the regulatory strategies for promoting wind power, the trend analysis of wind power over the two past decades (2000–2019), and the development goals in wind power for 2025. In addition, the carbon reduction benefit of wind power in Taiwan was preliminarily analyzed according to the data on the electricity carbon emission factor and the electricity generation by wind power in 2019. Finally, the discussions on the impacts of the corona virus disease 2019 (COVID-19) pandemic on wind power development were addressed to be in response to the national targets for nuclear-free homeland and 20% renewable electricity generation by 2025 in Taiwan.

2.   Data mining and methodology

As mentioned above, the aim of this study was to analyze wind power development since 2000 and also connect this renewable electricity with the regulatory promotion and national goals by 2025 in Taiwan. Statistical data and regulatory policies are briefly summarized below.

● Energy statistics in Taiwan

The updated data on the statistics and status of power supply by renewable sources in Taiwan were obtained from the official yearbook ^[2], and the website of the central competent agency (i.e., Ministry of Economic Affairs) ^[13].

● Regulatory policies for promoting wind power

The information about the regulatory policies for promoting wind power was based on the relevant laws and national guidelines by accessing the official website ^[15]. The most important laws include the Renewable Energy Development Act. By contrast with the law, the "Guidelines on Energy Development", which was derived from the Energy Management Act, was announced in April 2017 to set national energy policies covering energy security, green economy, environmental sustainability and social equity ^[14].

3.   Wind power resource in Taiwan

Taiwan is a subtropical island, which spans from 119° to 124° east longitude and from 21° to 26° north latitude. Its geographical environment features the Pacific Ocean on the east, the Taiwan Strait on the west, and the Bashi Channel on the south, and the Ryukyu Islands on the north. Taiwan Island is about 394 km long and 144 km wide with about 1,140 km of coastline and a land area of approximately 36,200 km². Due to the subtropical and tropical location at the intersection of East and Southeast Asia, this country has an average temperature of around 24 ℃ and the average annual precipitation of about 2,500 mm. More seriously, the island is often affected by typhoons during the months from June to October, which come from the tropical cyclones of South-East Asia. Typically, the special weather will bring strong winds and torrential rainfall, thus causing heavier damages along the east coast. For example, the eighth named storm and fourth typhoon of the 2009 Pacific typhoon season, the Morakot typhoon caused catastrophic damage in Taiwan between August 6 and August 9, leaving 698 people dead and 58 missing, and making roughly US$3.6 billion in economic loss ^[16]. In this regard, the reasons by tropical cyclone and earthquake must pay attention during the development stage for siting onshore and offshore wind power farms and improving safety factor or seismic capacity.

In spite of tropical cyclones during summer, Taiwan is a windy place along the northwest coast. In the winter reason, the northwest winds from continental China are deflected by the earth's rotation and become a northeast monsoon. By contrast, the southeast winds are deflected into the milder southwest monsoon in summer. From October to March, the winds prevail from the northeast and coincide with the northeast trade winds to reach a velocity of more than 3.8 m/s (i.e., 150 in/sec) in November at Taipei. On the other hand, the Taiwan Strait possesses a special geography which locates between the Central Mountain Range in Taiwan and the Wuyi Mountain in the Fujian Province of Mainland China. These mountains will cause channel effect like a funnel, forming strong winds more than those onshore. Thus, the Taiwan Strait has been recognized by the international organization 4C Offshore as one of the best offshore wind resources around the world ^[17]. It was reported that the average wind speeds of the offshore area along the west coast of Taiwan are about 9.5 m/s, having excellent wind energy potential with high power density of over 1,000 W/m^{2 [18,19]}. The potentials of onshore and offshore wind power in Taiwan have been estimated to be about 4.0 and 70 GW, respectively ^[20,21]. Therefore, there have been a variety of studies on the wind power potentials in Taiwan ^{[18,19,22,23,24,25,26,27,28,29,30]}.

4.   Wind power development in Taiwan

4.1.   Regulatory strategies for promoting wind power

In Taiwan, the Renewable Energy Development Act (REDA), which was first passed on 8 July 2009 and recently revised on 1 May 2019, is a legal foundation for promoting renewable energy and its industry development. As mentioned above, the data on the renewable electricity supply over the past decade (2010–2019) indicated a significant growth, especially in photovoltaic power. For instance, the amounts of electricity generation by photovoltaic power in 2010 and 2019 were 25.59 GW-h and 4,144.09 GW-h, respectively. Obviously, the promotional incentives like feed-in-tariff (FIT) and installation supports or subsidies played a determining role in the installation of renewable power system (including electrical grid connection) ^[31]. Regarding the REDA and renewable energy development in Taiwan, it has been discussed in the literature ^{[6,7,32,33,34,35,36,37]}. Table 1 summarized the feed-in tariffs (FIT) rates of onshore/offshore wind power during the years of 2018–2020 in Taiwan, which can be further pointed out as follows:

1. In order to encourage the industries to invest the small-scale onshore wind power systems (1–30 kW, in terms of installed capacity), the FIT rate is significantly higher than those of onshore wind power systems with over 30 kW.

2. For the large-scale onshore wind power systems (≧ 30 kW), the promotional FIT rates are still beneficial by increasing annual sales of electricity and guiding equipment and technology skill in order to utilize domestic land resources as much as possible.

3. Due to no large-scale offshore wind power system in Taiwan being in operation, the calculations of FIT rates must involve many additional cost items, including pre-planned survey costs, grid-connected costs, fishery compensation costs, decommissioning costs, and other differences in available working days of offshore work, development experience, underwater basic types, and so on. On the other hand, the cost-based data of offshore wind power system will be also referred to the international credible cases from the successfully developed countries like UK, Germany, Netherlands, and Denmark.

4. In order to increase the willingness of domestic financial institutions for participating in green finance credit when considering the future moral hazard of operation, the phased rates will remain the same since 2015. However, the difference between high and low rates will be narrowed to increase the financial planning flexibility.

5. When considering the factors for affecting sea meteorological conditions due to climate change, the financial expenditure control mechanism was introduced below according to the actual electricity generation in the current year.

- The first controlling stage is applied to those with lower than 4,200 kW-h/kW, and the FIT rates listed in Table 1 are used without deduction (i.e., 0.170 US$/kW-h × 1.00).

- The second controlling stage is applied to those with higher than 4,200 kW-h/kW but lower than 4,500 kWh/kW, and the FIT rates are 25 percent off to 0.127 US$/kW-h (i.e., 0.170 × 0.75).

- The third controlling stage is applied to those with higher than 4,500 kW-h/kW, and the FIT rates are 50 percent off to 0.085 US$/kW-h (i.e., 0.170 × 0.50).

4.2.   Trend analysis of wind power over the two past decades (2000–2019)

Although Taiwan is an excellent place for developing wind power, until the late 1990s the government began to take actions for the green energy in response to the energy diversification and climate change mitigation. In 2000, the government actively promoted the use of clean energy in order to reduce GHG emissions by thermal power plants using fossil fuels. In this regard, the central competent authority (i.e., MOEA) announced the "Regulations Governing Wind Power Demonstration System Setting Subsidies" in March 2000 to provide subsidies for wind power equipment. The first wind farm receiving the subsidy was the "Mailiao Wind Power Demonstration System", which is located in middle-western Taiwan (Mailiao Township, Yulin County). The Demonstration System with an installed capacity of 2.64 MW was commercially operated on December 27, 2000. Thereafter, the Taiwan Power Company (Taipower, one of the state-owned enterprises) established 2 demonstration systems, which were located in the Baisha Township (Penghu County, an island in the middle of the Taiwan Strait) with an installed capacity of 2.4 MW and the Chupei Township (Hsinchu County, north-western Taiwan) with an installed capacity of 3.5 MW, respectively. These three wind power demonstration systems successfully drove the Taipower and other private corporations to put efforts into the development of onshore wind energy since 2005. Table 2 summarized the data on the wind power in terms of electricity generation and installed accumulation capacity over the past two decades (2000–2019) ^[2]. Under the efforts by regulatory and promotional measures (including land resource exploration, technical guidance, research survey, and demonstration subsidy), the statistics showed that the accumulative installation capacity of wind power and its electricity generation indicated an increasing trend, but its growth rate became slow since 2011. Obviously, it is more and more difficult to find the available land for the onshore wind power farms. The preliminary planning of offshore wind farms was proposed by the central competent authority (i.e., MOEA) in the early 2010s.

In order to achieve 20% of the power generation from the renewables by 2025, the "Four-year Wind Power Promotion Plan (2017–2020)" was approved by the Taiwan government in 2017. The objectives of this Plan were to raise total onshore wind power in terms of installed capacity to approximately 814 MW and install the offshore wind power with approximately 520 MW in terms of installed capacity. According to the Article 11 of the Renewable Energy Development Act, the MOEA promulgated "Demonstration Incentive Program for Offshore Wind" in July 2012. The incentive fees for installation of demonstration wind turbines included maximal 50% of the installation expenditures and maximal US$8.3 million of operation expenditures. The Government has selected 3 awarded winners, including 2 from the private sectors and 1 from the state-owned company (Taipower). The first phase of two offshore demonstration turbines with a total installed capacity of 8 MW was successfully commissioned in April 2017. In Nov. 2019, 20 6-MW turbines with a total installed capacity of 120 MW started to enter commercial operation. Table 3 listed the amounts of electricity generation from onshore and offshore wind power since 2017 ^[2]. Therefore, the electricity generation by offshore wind power (175.4 GW-h) significantly increased in 2019 compared to that in 2018 (26.7 GW-h).

4.3.   Development goals in wind power for 2025

In order to be in accordance with the goals of phasing out nuclear power and increasing renewable resources to 20% of the Taiwan's electricity supply by 2025, the "Four-year Wind Power Promotion Plan (2017–2020)" set the milestone checkpoints, as listed in Table 4. When comparing the figures in 2020 (Table 4) with those in 2019 (Table 2), the gap filling will heavily depend on the establishment of offshore wind power farms in the next five years. Table 5 further showed the promotion goals by 2020 and 2025 for wind power in terms of electricity generation in Taiwan. These efforts on the wind power not only promote energy diversification and green energy development, but also reduce GHG and other air pollutants emissions due to the combustion of fossil fuels. In addition, the MOEA also evaluated the expected benefits of the Plan completely performed in 2025, which include the following values:

- Lower GHG emissions

The wind power facilities will generate 14 billion GW-h of electricity each year, equivalently cutting GHG emissions by over 7.0 million metric tons annually.

- More green jobs

About 10,000 green energy job opportunities will be created.

- Higher investments

The Plan will spur investments of about US$33 billion.

4.4.   Carbon reduction benefit of wind power in Taiwan

In Taiwan, the Electricity Act was enacted for one of the purposes to reduce the carbon emission, thus promoting the supply diversification of the electricity industry. In this regard, the electricity carbon emission factor (ECEF) shall be stipulated by the electricity industry regulatory authority (i.e., Taiwan Power Company, one of the state-owned enterprises) according to the national energy and carbon reduction policies, and announced on a regular basis ^[38]. The factor can be used by the electricity transmission & distribution enterprises to charge the renewable-energy-based electricity generation enterprises or retailing enterprises that use the electricity supply infrastructure based on the amount and rates of the re-supplied electricity. In addition, this factor was recently connected with the quantified benefits of the GHG emission reduction auditing according to the Greenhouse Gas Reduction and Management Act promulgated in July 2015. Using the data on the generation amount of offshore wind power in 2019 (i.e., 1,871.3 × 10⁶ kW-h or 1,871.3 GW-h, seen in Table 2) and the average default value of 0.539 kg CO₂/kW-h (Figure 2), the equivalent mitigation of CO₂ emission from the wind power systems was thus estimated as follows:

4.5.   COVID-19 impacts on wind power development

Since the first outbreak of coronavirus (COVID-19) in the end of 2019, more than 62 million confirmed cases of COVID-19 have been reported as of 30 December 2020 ^[39]. As a consequence, this pandemic event has impacted on dimensional effects regionally and globally ^[40]. According to the report by the International Energy Agency ^[41], the COVID-19 is expected to delay renewable energy deployment. The lockdown measures (e.g., social distancing and stay at home) have been triggering global supply chain disruption and delays in the project construction, thus causing a direct impact on the installations of renewable electricity system and its future investments ^[42,43]. In Taiwan, the negative effects of COVID-19 could be more serious because of the delays in the supply chains, lacks of international (foreign) professionals and engineers, and risks of not being able to gain government incentives according to the contract schedule (FIT scheme). In this regard, the national targets for nuclear-free homeland and 20 % renewable electricity supply by 2025 will face serious challenges because the COVID-19 pandemic will retard the off-shore wind power.

5.   Conclusions

Taiwan features its high dependence on imported energy by about 98%. On the other hand, the Taiwan Strait (located in the western region of the Taiwan Island) has been recognized as one of the best offshore wind resources around the world, thus possessing high potential for wind power development. In this work, an interactive analysis of Taiwan's wind resources, regulatory measures for promoting wind power, trend analysis of wind power over the two past decades (2000–2019) and its development goals for 2025 were explored to respond to 20% of total electricity generation from renewables by 2025. In addition, the present article may first address the impacts of the corona virus disease 2019 (COVID-19) pandemic on the Taiwan's wind power development and the national energy goals in the near future.

With the implementations of promotional measures, including official subsidies for wind power equipment, FIT mechanism, and financial expenditure mechanism (green financing), the electricity generation by wind power rapidly grew from 1.38 GW-h in 2000 to 1,871.32 GW-h in 2019. However, its growth rate became slow since 2011 due to the difficulty in finding the available land for the onshore wind power farms. Under the Renewable Energy Development Act promulgated in 2010 and revised in 2019, the MOEA announced "Demonstration Incentive Program for Offshore Wind" in July 2012 and further adjusted to "Four-year Wind Power Promotion Plan (2017–2020)" in August 2017. Therefore, the electricity generation by offshore wind power significantly increased in 2019 (175.4 GW-h) compared to that in 2018 (26.7 GW-h). Using the data in 2019 (i.e., 1,871.3 × 10⁶ kW-h) and the average default value (i.e., 0.539 kg CO₂/kW-h), the equivalent mitigation of CO₂ emission from the wind power systems was thus estimated to be about 1.0 Tg (1,000,000 metric tons). Based on the progressive development of wind power and solar photovoltaic power over the past two decades (2000–2019), the national targets for nuclear-free homeland and 20% renewable electricity generation by 2025 would be prospective. However, it should be pointed out that energy saving and energy efficiency improvement must be incorporated into the national targets for the energy sustainability and climate change mitigation, which largely depended on the energy (or electricity) price and the consumer's willingness. On the other hand, the national targets for nuclear-free homeland and 20% renewable electricity supply by 2025 will face serious challenges because the COVID-19 pandemic will retard the wind power development, which is planning about 23% of renewable electricity supply from wind power in terms of installed capacity by 2025.

Funding

This research did not receive any external funding.

Conflict of interest

The author declared that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.