Macromolecular sizes of serum albumins in its aqueous solutions

1.   Introduction

The structure and properties of proteins continue to be in the focus of attention of physicians, chemists, physicists, and other researchers. Features of changes in the protein structure, being dissolved in water and aqueous solutions, are the main research questions for physicists [1]. Herein we want to dwell on transformations that occur with albumin macromolecules in solutions. In this article we will focus on the macromolecules of human serum albumin (HSA) and bovine serum albumin (BSA).

Human serum albumin (HSA) consists of 585 amino acid residues combined into a single macromolecular chain with a molecular weight of 66.5 kDa [2]. In the crystalline state, the HSA macromolecule is folded into a compact conformation of a heart-shaped regular triangular prism with sizes of ∼80 Å and ∼30 Å [3]. Bovine serum albumin (BSA) consists of 583 amino acid residues combined into a macromolecule with a molecular weight of 66.5 kDa and a spatial structure similar to that of HSA [2],[4]. The amino acid sequences of HSA and BSA are 75.52% identical [5].

The domain structure of HSA and BSA is generally accepted. At physiological pH values, the secondary structure of HSA and BSA consists of alpha helices (50–68%) and beta folds (16–18%) stabilized by hydrogen bonds, as well as an unordered part of the macromolecular chain [2],[4],[6]. Due to 17 disulfide bonds between the cysteine residues of the alpha helices, the tertiary structure of these albumins is formed: three domains are formed, each of which is formed by subdomains of three alpha helices, and hydrophobic interactions between the domains determine the globular structure of proteins [2],[4].

Differences in the nucleotide sequences of albumins lead to some change in the hydrophobicity and conformational mobility of the HSA macromolecule compared to the BSA macromolecule [5]. One of the differences in the HSA structure is the presence of two tryptophan residues (Trp134 and Trp213) in the BSA macromolecular sequence, in contrast to one tryptophan residue in the HSA macromolecule (Trp214) [2].

When dissolved in water, as well as in aqueous and biological solutions due to interaction with water, the rigid conformation of albumin macromolecules should violate. To the greatest extent, this applies to those structural features of albumin that are due to Coulomb forces. Upon dissolution, a slight redistribution of locally charged sections of the albumin macromolecule occurs, which changes its hydrophilic and hydrophobic properties. The latter can be the cause of compaction of albumin macromolecules, i.e. reduction in the size of the macromolecule, while the predominance of hydrophilic interactions can contribute to the transformation of a compact domain structure into a quasi-linear structure [2],[5]. Therefore, both hydrophobic and hydrophilic interactions can contribute to the destruction of the domain structure of albumin in solution, which is inherent in the crystalline state of a protein.

The dissolution of albumin macromolecules in water can be considered as an internal structural phase transition. The structural transformations of the albumin macromolecule will also change the structure of the water environment [7],[8]. One of the features of such a phase transition is a change in the size of the macromolecule.

One of the indicators of the structural phase transformations is the hydrodynamic radius change. The latter is studied by various physicochemical methods: dynamic light scattering [9]–[11], small-angle neutron scattering [12]–[14], atomic force microscopy [15], small-angle X-ray scattering [16], pulsed-field gradients NMR spectroscopy [17],[18], gel permeation chromatography [19], capillary viscometry [20].

We focused on methods that allow us to determine the radius of albumin macromolecules as an indicator of conformational transitions using the shear viscosity of macromolecular solutions and the self-diffusion coefficients of macromolecules in them. These methods allow 1) to study the change in the hydrodynamic radius of the macromolecule depending on temperature, concentration, acid-base balance (pH), the presence of salts and the nature of the buffer solutions; 2) to analyze the dynamics of solvent molecules associated by intermolecular interactions with a macromolecule.

To differentiate the temperature, concentration, and acid-base balance (pH) effects on the internal structure of a macromolecule, a comparison is made of the temperature-concentration dependences of the hydrodynamic radius of albumin macromolecules and polyvinyl alcohol (PVA) macromolecules. It is important to note that the PVA macromolecule has an internally disordered, statistically determined structure of the macromolecular coil in solution, while the internal structure of albumin has a strictly determined domain structure of the macromolecular globule, determined by the amino acids sequence.

The macromolecular radius dependence on the conformation changes of albumin macromolecules forms a separate problem and provides a basis for the next step—the study of changes of the macromolecular radius versus solution acid-base balance, which is one of the factors of conformation changes in proteins. At present, the concentration and temperature dependencies of the macromolecular radius of albumin at constant pH values have been obtained, which correlate well with the data obtained by other experimental methods.

2.   Calculation method

2.1.   Determination of the macromolecular radii from the shear viscosity of aqueous solutions

Here, the ratio between weight concentration c_m and volume concentration φ in the solution of macromolecules:

where R is the macromolecular radius; ρ is the solution density; N_A is the Avogadro constant; M is the molecular weight of a substance. Hence, the effective radius of the macromolecule is determined by the ratio: where the mass density is determined by a linear combination: where ρ_w is the mass density of water, ρ_alb is the mass density of the crystalline albumin. For albumin macromolecules d_alb = 2.98·10⁻⁸kg^1/3.

With certain reservations macromolecules in dilute solutions can be approximated as spherical particles. In this assumption, the average viscosity of the dilute solution can be estimated from Einstein's theory [21]:

where η₀ is the solvent (water) viscosity, φ is the volume concentration of macromolecules. The linear dependence of shear viscosity on volume concentration indicates neglect of hydrodynamic interaction between macromolecules.

It follows from equation (4) that ϕ=25λ, where λ=η¯η0−1, and for the effective radius of the macromolecule, considered as a function of excess viscosity, we find:

As shown in [22], formula (5) is only applicable to volume concentrations of φ < 0.1.

Hydrodynamic interactions between macromolecules become essential as the solution concentration increases. Their accounting was first performed by Batchelor [22], who proposed the formula:

Where, By rewriting equation (6) in the form we'll look for φ as an endless series of degrees for λ < (<<)1, The first coefficients of this series take values: By substituting the values of φ obtained in this way, we find: where

Batchelor formula generalizes Einstein formula and turns out to be applicable up to volume concentrations φ ≤ 0.2, which corresponds to the viscosity ratio: η/η₀ < 1.5. Unfortunately, a further generalization of the Batchelor method faces considerable difficulties in finding the higher-order members of the perturbation theory, finding the sum of the series, and even in solving the question of its convergence.

The progress in determining the effective radii of macromolecules is associated with the use of cellular approaches [23], which take into account that hydrodynamic disturbances caused by macromolecular particles are localized mainly within a spherical cell that surrounds the particle. It is also considered that at the cell boundary the normal component of the perturbation rate and the tangential stress components are equal to zero, which is equivalent to the absence of friction on the outer cell surface [23]. Among different versions of cellular approaches, we give preference to the version [23],[24], in which the neighboring macromolecules disturb the hydrodynamic field of the rotating particle. In this case, the properties of symmetry of the inoculum hydrodynamic field and the perturbed hydrodynamic field are used as fully as possible. Works [23],[24] show that the viscosity of dilute solutions of macromolecules is determined by the formula:

where ψ = (R₀/R)³, R₀ is the macromolecule radius, and R is the cell radius. Thus, the task of determining the average viscosity of the macromolecule solution is reduced to establishing the relationship between the model parameter ψ = (R₀/R)³ and the value of specific volume φ = V₀/V measured experimentally, where V₀ is the total volume occupied by macromolecules, and V – the volume of the liquid system. Formula (13) allows us to describe the viscosity behavior of dilute solutions of macromolecules up to the values of the volume concentration of φ ≤ 0.5, which actually coincides with the solution density corresponding to the direct contact of all the nearest neighbors [23],[24]. It is important to note that at concentrations of φ ≤ 0.2 the formulas (13) and (6) are fully equivalent (this fact follows directly from the construction (13)).

We use equation (13) to determine the effective volume concentration:

The effective radius of macromolecules is found directly by the formula (2). An independent method for determining the effective radius of macromolecules is related to their self-diffusion coefficient.

2.2.   Evaluation of macromolecule radii from the self-diffusion coefficient of macromolecules in solution

The dependence of the self-diffusion coefficient on the volume concentration φ is usually presented in the form:

where is the self-diffusion coefficient of a macromolecule in an extremely dilute solution. The same is also represented in another view: where R_D has meaning of the effective radius for a macromolecule. In the case of φ << 1 it can be represented in the form:

To define δR value, let us present the left part of equation (15) as a degree gradation of δRR0 and the right part as a degree gradation of φ. In this way we come to the equation:

As before, we're looking for his solution in the form: δR/R₀ = b₁φ + b₂φ² + …. Equating the coefficients at the same degrees of φ, we find:

In case of the linear nature of the function f(φ): f(φ) = aφ + O(φ²), the effective radius of the macromolecule is changed in the simplest way:

Recall that the above solutions for the effective radius of the macromolecule are fair for volume concentrations of φ ≤ 0.5.

If the dependence of the self-diffusion coefficient on the volume concentration φ is presented in the form of equation (15), where φ turns out to be significantly different from zero, then the application of perturbation theory is unacceptable.

In this case we represent R_D as R_D = R₀ + δR ≡ R₀(1 + u). Then

and where φ₀(c) = (R₀/γ)³. By substituting (22) and (23) in (15), we obtain the following equation for u determining: where f(φ) is the known function of φ.

3.   Results and discussion

In this part, we will consider the successively effective hydrodynamic radii of polyvinyl alcohol (PVA), bovine serum albumin (BSA), and human serum albumin (HSA) macromolecules. In comparison with the latter two, PVA macromolecules have the simplest internal structure, which boils down to a sufficiently dense spherical core and a relatively rarefied periphery. It is assumed that the dense PVA core has a dominant influence on the shear viscosity behavior of its solutions. In the case of BSA and HSA, the character of temperature and temperature dependence of the effective hydrodynamic radius is complicated by the influence of their more complex internal structure. Our results on the size of albumin macromolecules refer to the temperature range up to 318 K, which corresponds to the stage of pre-denaturation of protein macromolecules.

3.1.   Evaluation of macromolecule radii from the self-diffusion coefficient of macromolecules in solution

This section presents the results of the effective hydrodynamic sizes investigation of polyvinyl alcohol (PVA) macromolecules in aqueous solutions [25]. The PVA of Mowiol 6–98 (Kuraray) brand with the average molecular weight M_w = 47 kDa that corresponds to the average number of monomers in a macromolecule p = 1000 and the concentration of OH-groups h = (98.4 ± 0.4) mol.% in a macromolecule was used.

From the small-angle X-ray scattering [26]–[28] it follows that in the condensed state PVA has a flat zigzag-shaped configuration of the macromolecular chain with a period of identity equal to 2.52 Å. When dissolved in water, the macromolecular chain of PVA takes the conformation of the macromolecular coil [29]. Intramolecular hydrogen bonds in PVA macromolecule are stronger than hydrogen bonds between water molecules: ϵH(pp)/kBT=−15.2 and ϵH(pw)/kBT=−12.3 [30],[31]. Therefore, the PVA macromolecule in dilute aqueous solutions gets the conformation of a sufficiently dense macromolecular coil [32]–[37]. The compactization of PVA macromolecular coil in aqueous solution is also due to hydrophobic effects [38],[39].

Temperature and concentration dependencies of the effective radius of PVA macromolecules are restored by experimental values of shear viscosity of aqueous solutions from [25] according to formulas (14), (15), and (2) corresponding to the cell approach [22]–[24]. The results obtained in this way are presented in Figure 1.

As we can see, with increasing temperature and concentration, the effective hydrodynamic radius of a core R_η decreases. Our neglect of the periphery influence on the solution viscosity is confirmed by the analysis performed in [40] by the molecular dynamics method. In this work it is shown that the shape of PVA macromolecule in aqueous solution is close to a spherical one with weakly blurred borders.

The concentration dependencies on temperature, at which the PVA macromolecule radius remains unchanged, were studied in [25]. These lines are characteristic curves that allow tracking the role of the effects of the interaction of macromolecules with solvent and among themselves at the overlap of their edges, as well as the disorder that increases with increasing temperature. Two straight lines intersecting at temperature (315 ± 2) K approximate the characteristic curves for aqueous PVA solutions [25]. As shown in [41],[42] there is a dynamic phase transition in pure water at this temperature, when a significant change in the character of thermal motion of water molecules occurs. Thus, PVA macromolecules in aqueous solutions are indicators of changes in water properties [43],[44].

The results obtained by us specify those given in other publications. In [45], the hydrodynamic radius of a macromolecule RD(PVA)=54.0 Å was obtained from sedimentation coefficients at centrifugation of aqueous PVA solutions (M_w = 44 kDa, h = 88 mol.%), which is slightly less than the value of 65 Å for dilute PVA solutions calculated by us. Some discrepancies of hydrodynamic radii can be explained by the different number of hydroxyl groups (h) in the investigated PVA macromolecules. According to the conclusions of the work [33], with a decrease in the content of hydroxyl groups in a macromolecule, the hydrodynamic radius of PVA macromolecules in aqueous solution decreases due to an increase in the packing density of macromolecule, caused by an increase in the number of hydrophobic interactions between acetate groups and water molecules.

In work [46] by the dynamic light scattering method the R_D values for PVA aqueous solutions with the average number of monomers in a macromolecule p = 500, 2000, 4000, and 8000 were received. Estimating R_D on their basis for p = 1000, we receive RD(PVA)=9.6 Å. The close to it value of hydrodynamic radius (Rη(PVA)=76 Å) of PVA macromolecules (M_w = 38 kDa, h = 97.5 mol %) is obtained from viscosity data on the basis of the Flory approach [46]. This value, corresponding to c = 0.01 wt % (φ = 0.02) at temperature T = 298 K, is close to the size of an ideal polymer chain R_i = r₀N^1/2.

Deviation from our calculated value of 65 Å for diluted PVA solutions may be caused by ignoring the additives specified by us (see equations (21)–(24)). Moreover, viscosity values lead to the size of the dense core, which is slightly smaller than the size of the PVA macromolecule with consideration of the rarefied periphery.

The results of work [47] testify to the growth of the hydrodynamic radius of macromolecules with temperature growth, which is also predicted in [39] using the method of molecular dynamics. Presented on Figure 1 surface of effective radius of PVA macromolecules in aqueous solutions shows constancy of effective radius of macromolecules up to concentrations φ ∼ 0.2 and temperatures T∼330 K. Growth of fluctuations in the peripheral part of the macromolecule with temperature growth should lead to the increase in the periphery size. At the same time, the core size decreases or remains unchanged (see Figure 1).

3.2.   Effective radii of bovine serum albumin macromolecules

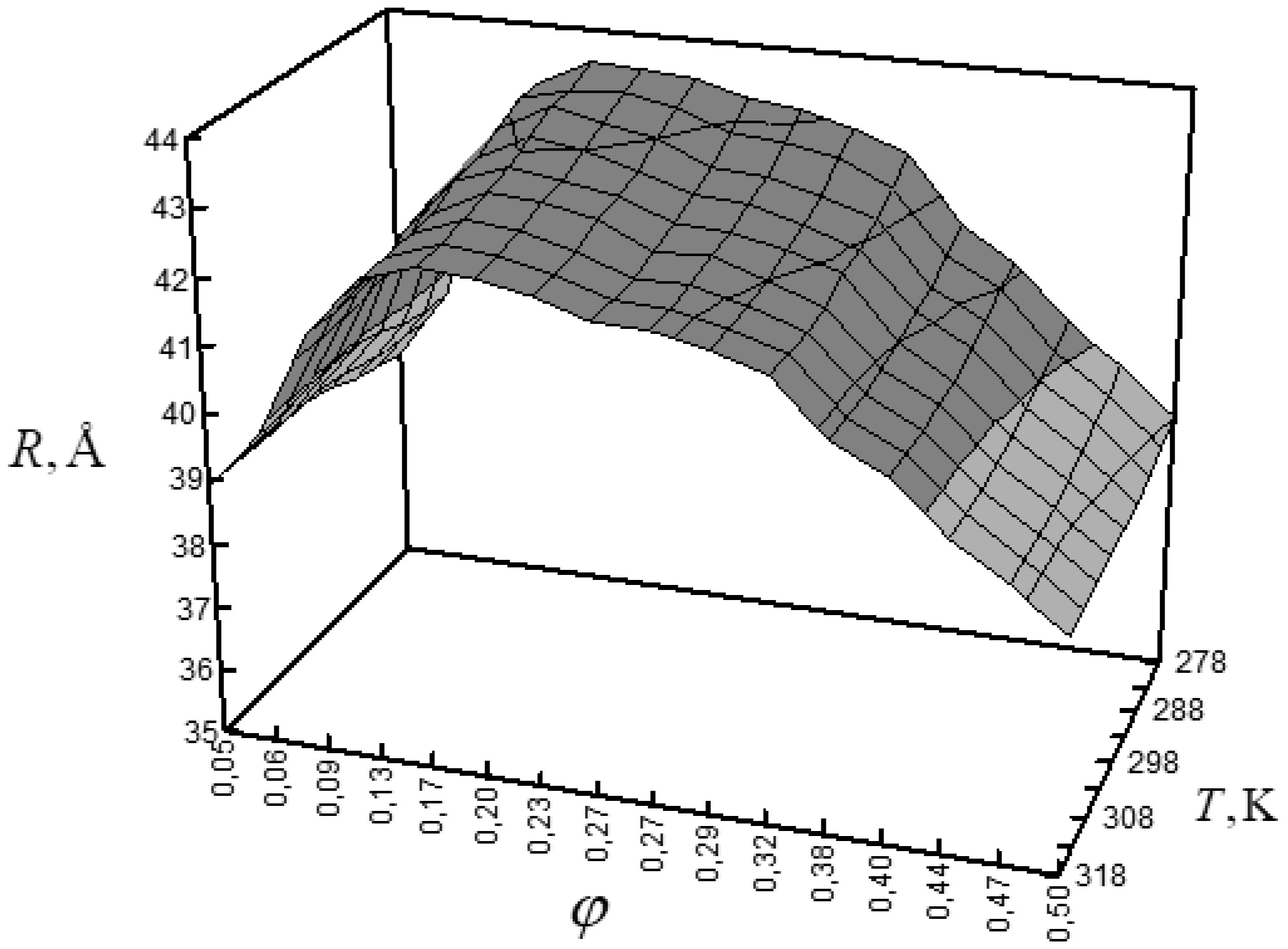
To obtain effective radii of BSA macromolecules, we use experimental values of shear viscosity of BSA aqueous solutions obtained in [48]. Using equations (13), (14), and (2) of the cell approach we obtained temperature and concentration dependences of an effective radius of BSA macromolecules. The surface of effective radius of BSA macromolecules in the concentration range (2.0÷20.0) wt.% (φ = (0.05÷0.49)) and temperatures (278÷318) K at constant pH = 5.2, corresponding to the vicinity of isoelectric point of BSA is presented in Figure 2.

As we can see, at all temperatures studied, the concentration dependencies of the effective radius of BSA macromolecules Rη(BSA) are essentially not monotonous. At concentrations of ∼5 wt.% (φ ≈ 0.17) the Rη(BSA) values take maximum values and fall quickly enough in both directions from the maximum. At concentrations greater than 10 wt.% (φ ≈ 0.32), the concentration dependencies are quasi-linear.

The observed concentration maximum of effective radii Rη(BSA) dependencies corresponds to isolated macromolecules since the transition from isolated macromolecules to macromolecules with overlapping peripheries occurs at the vicinity of 7 wt.% (φ ≈ 0.23) [38]. The maxima position of the effective radius Rη(BSA) is in the physiological range of albumin concentrations (35–55 mg/ml) in plasma [48] and corresponds to the largest surface area of a macromolecule. In its turn it provides maximum access to albumin binding centers for performing one of its main functions–the transport one [2],[49]–[51].

Let us compare the sizes of BSA macromolecules obtained from the analysis of shear viscosity of its aqueous solutions with the available literature data. In [52] the dynamic light scattering for dilute BSA aqueous solutions (temperature T =298K, acid-base balance pH = 5.0, the concentration of ions I = 0.15 M) was used to determine the self-diffusion coefficient D_s = (6.14 ± 0.03)·10⁻¹¹m²/s, which using equations (17) and (21) leads to RD(BSA)=(39.92±0.03) Å. This value practically coincides with 39.90 Å obtained from the analysis of viscosity at c_m = 2.14 wt.% (φ = 0.06), T = 298K, pH = 5.2. It should be emphasized that the pH of solutions in both compared cases is close to the isoelectric point of BCA solutions.

The method of dynamic light scattering to determine the values of self-diffusion coefficient of albumin macromolecules is also used in [53]. At c_m = 1 wt.% (φ = 0.024), T = 296K, pH = 5.0 and I = 0.023 M the hydrodynamic radius is equal to RD(BSA)=37,9 Å. This value also correlates well with that obtained from the analysis of Figure 3, if the corresponding curve is extrapolated to the concentration values of ∼1 wt.% (φ = 0.024).

In [54], the self-diffusion coefficient for dilute BSA aqueous solutions (c_m = 2.79 wt.% (φ = 0.08), T = 298 K, pH = 4.7) was obtained and using equations (17) and (21), one can calculate RD(BSA)=36.9 Å. This value is somewhat less than the value of the hydrodynamic radius Rη(BSA)=(41.50±0.05) Å obtained from the analysis of viscosity of BSA aqueous solution with properties c_m = 2.79 wt.% (φ = 0.08), T = 298 K, pH = 5.2. Here some discrepancy of values of BSA hydrodynamic radii can be connected with the discrepancy of acid-base balances (pH) of solutions and the presence of ions of salts in solutions.

In [55] on the basis of viscosity data for extremely diluted BSA solutions using the generalized Einstein formula for elongated macromolecules [56] the effective length of the large spheroid axis of the revolution was obtained: Rη(BSA)=41.5 Å.

A comparison of the values of effective radii of BSA macromolecules calculated from viscosity data according to equation (13) and self-diffusion coefficient according to equation (17) is presented in Table 1. The properties of the solution – temperature T, acid-base balance pH, and concentration of ions in the solution I–are given in brackets.

Thus, the results obtained by us correlate well with data from other sources. Also, correction of the hydrodynamic radius of albumin macromolecules by equations (20)–(21) allows us to achieve almost complete consistency of data obtained from shear viscosity and self-diffusion. Some ambiguity of macromolecule radii comparison is determined by a mismatch between the pH of a solution and the presence of ions of salts, to which albumin structure is sensitive.

3.3.   Effective radii of human serum albumin macromolecules

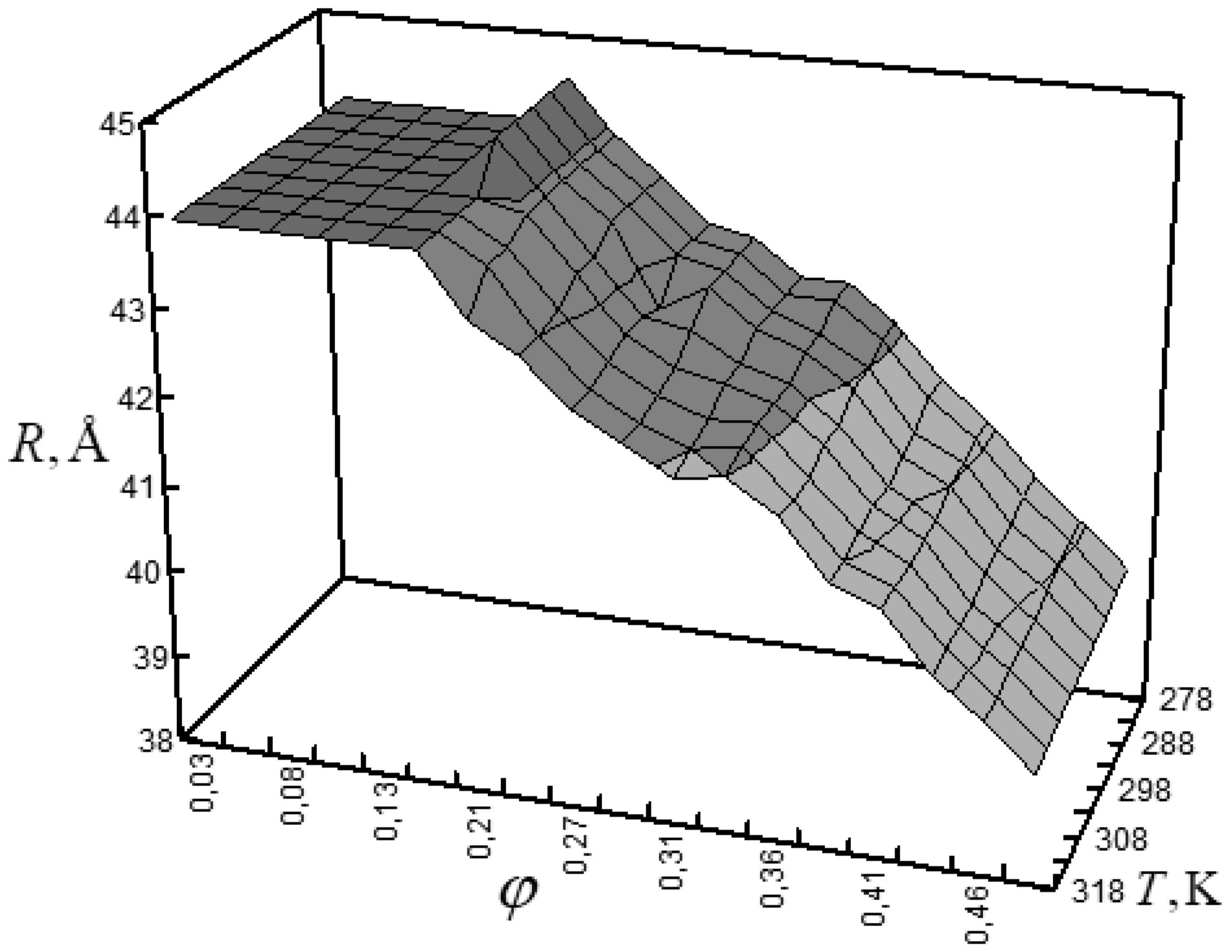
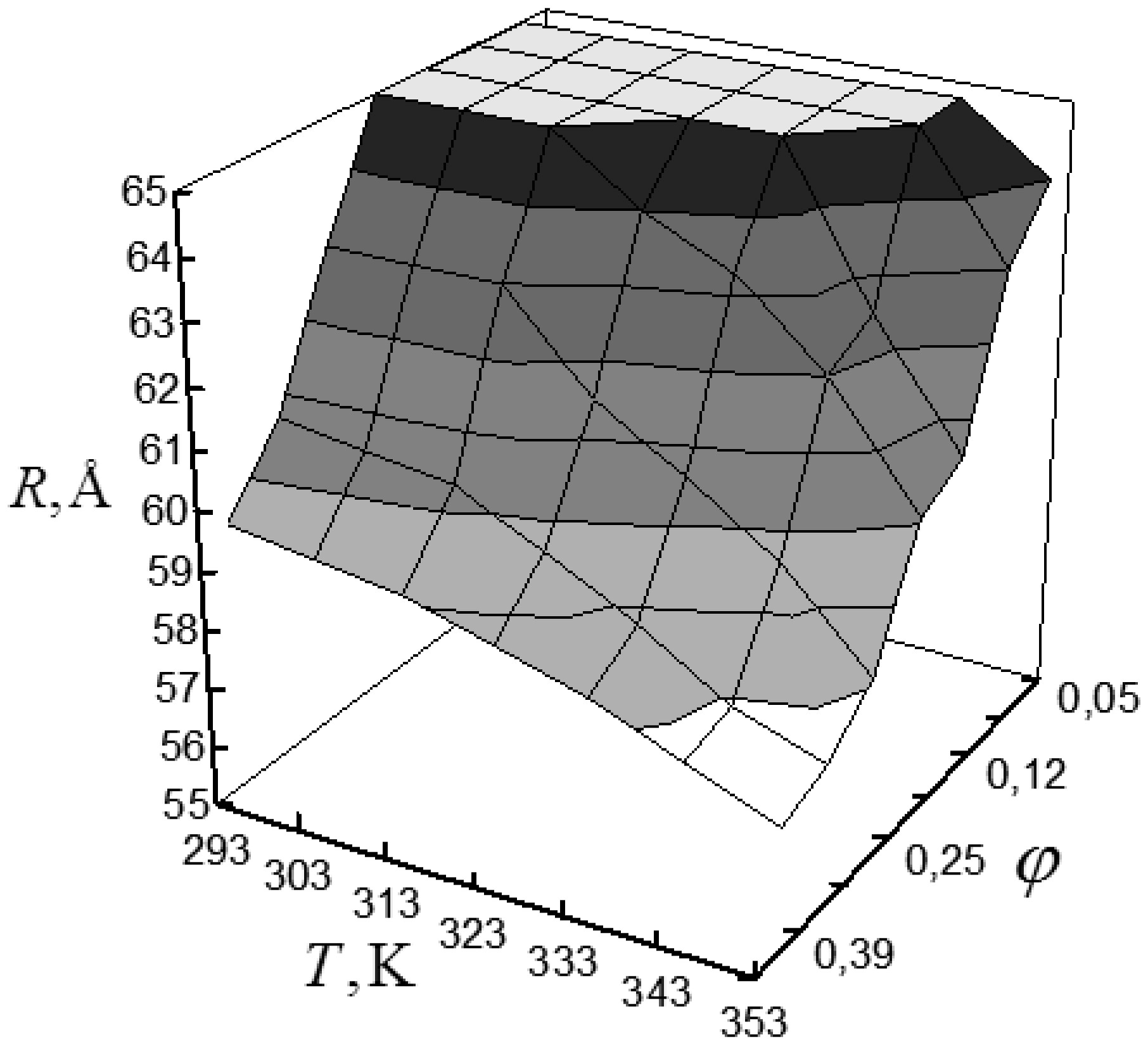
To obtain temperature and concentration dependencies of effective radii of HSA macromolecules we use cell approach equations (13), (14), and (2) and experimental values of shear viscosity in [57]. The surface of effective radii of HSA macromolecules as a function of temperature in the interval (278÷318) K and concentration in the interval (0.82÷18.2) wt.% (φ = (0.03÷0.48)) for the value pH = 7.0 is presented in Figure 4.

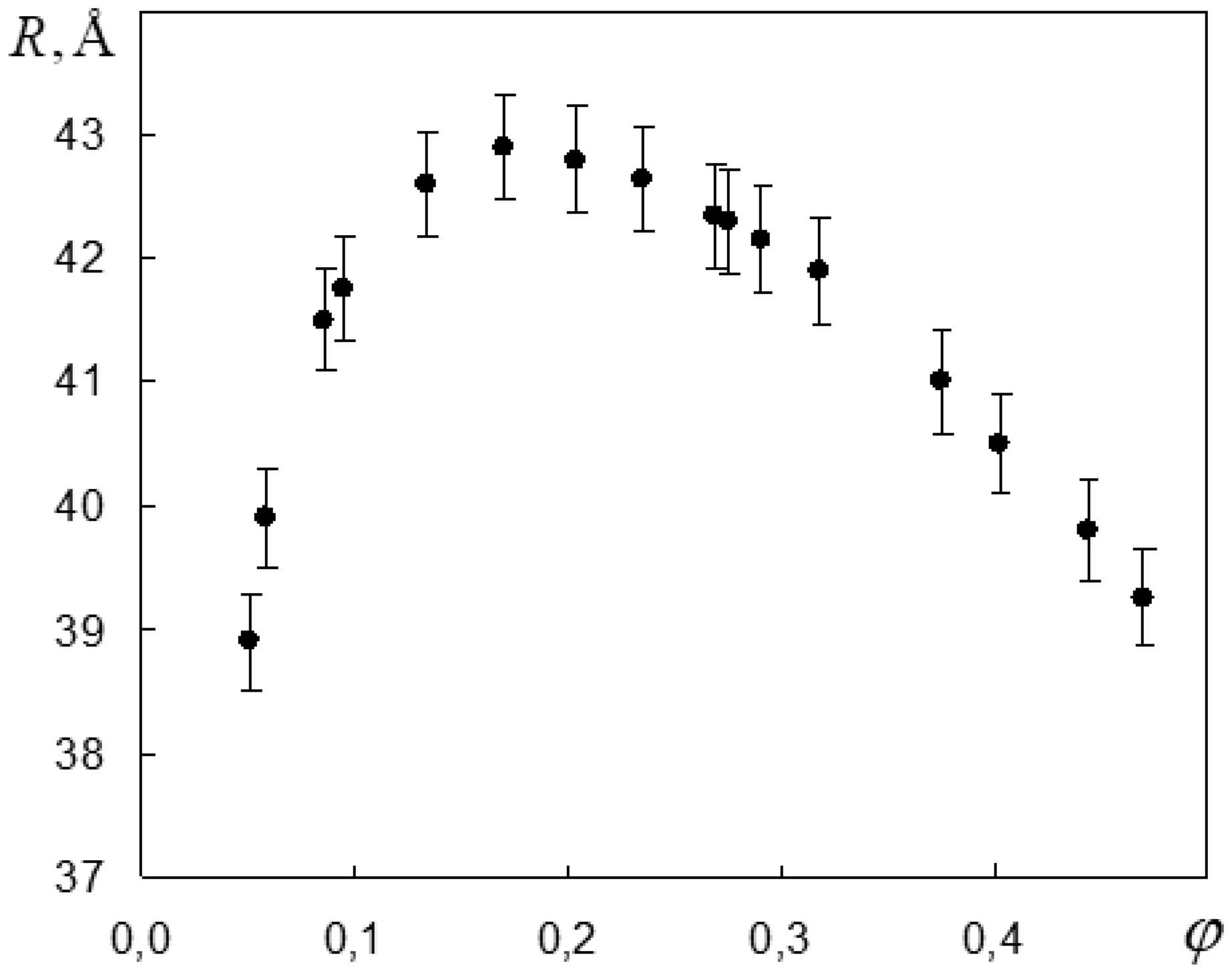
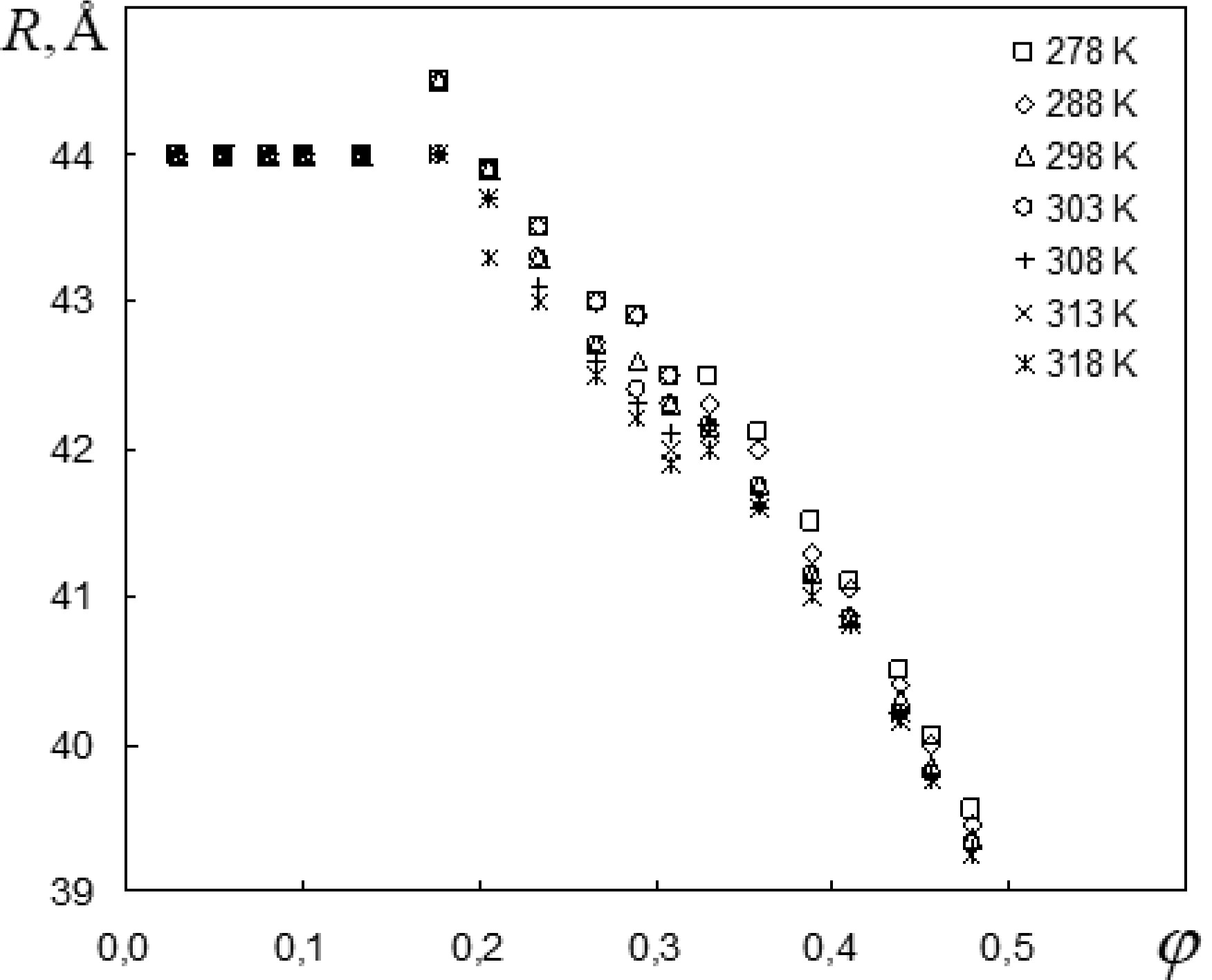
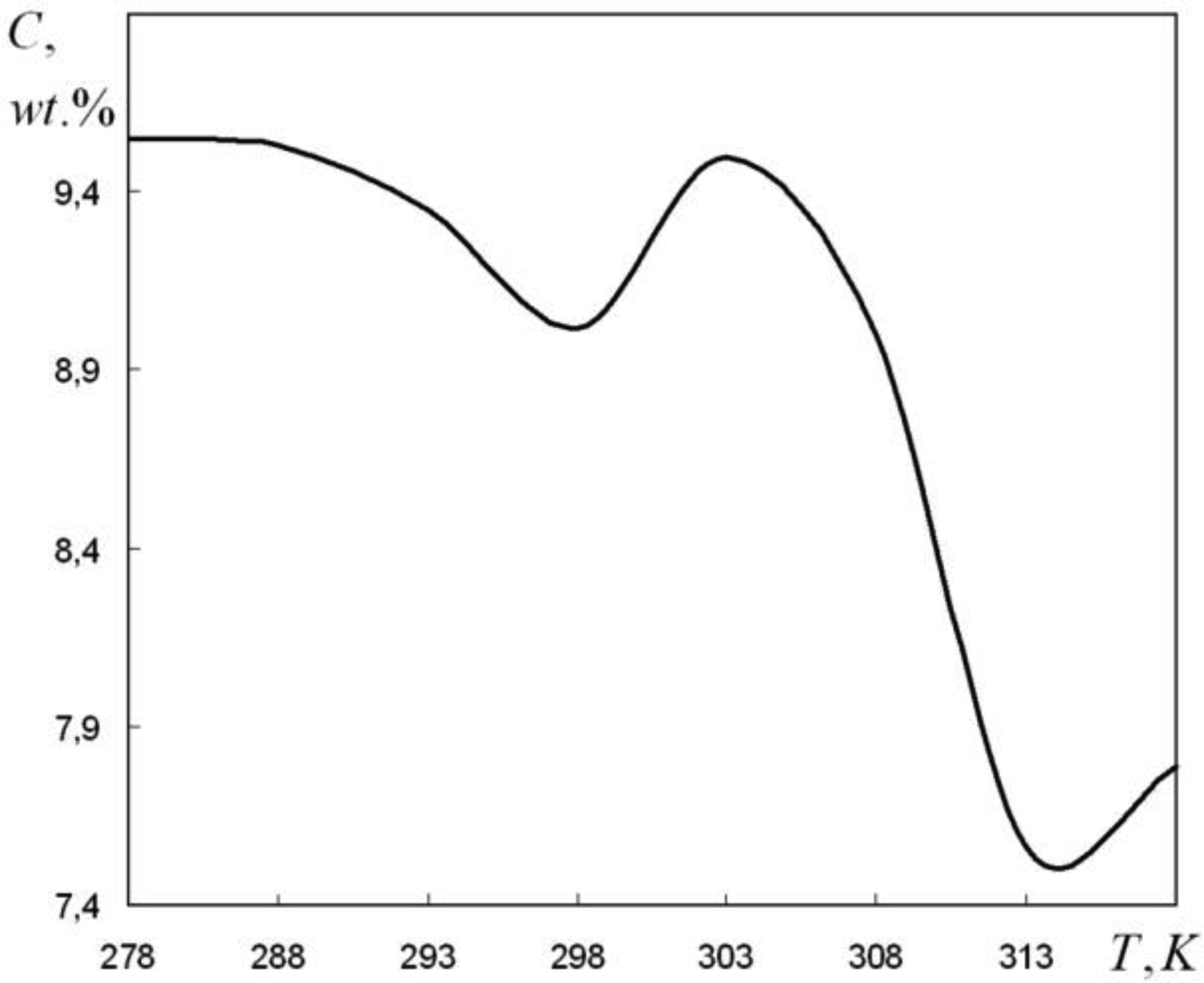
Work [57] shows that three concentration intervals can be identified over the entire temperature range 1) (0.82÷3.65) wt.% (φ = (0.03÷0.14)), where the effective radii of human serum albumin remain unchanged; 2) (4.67÷9.45) wt.% (φ = (0.18÷0.31)), where the effective radii of albumin in aqueous solution decrease and 3) (10.2÷18.2) wt.% (φ = (0.33÷0.48)), where the effective radii of albumin macromolecules with increasing concentration linearly decrease, and the slope is poorly dependent on temperature (Figure 5).

In [57] the dependencies of concentration on temperature are constructed, at which the radius of an HSA macromolecule remains unchanged. The curve that corresponds Rη(HSA)=(42.53±0.05)Å in the concentration interval (7.0÷10.0) wt.% (φ = 0.21÷0.28) has two minimums at temperatures T_min1 = (298±1) K and T_min2 = (314±1) K, one maximum at temperature T_max = (303±1) K (see Figure 6). At the same time, the temperature interval between the local minimums corresponds to the boundaries of warm-blooded organisms [57]. In [58], the change of albumin conformation in the temperature range (293–338) K is associated with the dynamic phase transition occurring in the water at ∼315 K (42 °С). The role of interactions of water with albumin macromolecules increases with the growth of HSA concentration. The authors in [59] attribute the albumin conformation dynamics to changes in water properties occurring in the physiological temperature interval.

Let us compare the sizes of HSA macromolecules obtained from the analysis of the shear viscosity of its aqueous solutions with the available literature data. In [13] using the small-angle neutron scattering some dilute HSA aqueous solutions (I = 0.15 M) were investigated: the results indicate the conformation probability of HSA macromolecule as an asymmetric prolate ellipsoid with a radius of 42.5 Å , which correlates well with our results.

In [59],[60] using the photon correlation spectroscopy and molecular dynamics in dilute HSA aqueous solutions (c_m = 0.1 wt.% (φ = 0.004), T=(298÷323) K, (0.075< I < 0.225) M) the independence of the value of hydrodynamic radius RD(HSA)=(50±3) Å from temperature is shown, that well correlates with the results for concentrations φ < 0.15 obtained by us (see Figure 5). Some discrepancies between our results of Rη(HSA)=44 Å macromolecules of HSA and the value of RD(HSA)=(50±3) Å in [59] can be attributed to the presence of sodium chloride ions in the last solutions.

In [9],[61] somewhat smaller values of the hydrodynamic radius RD(HSA)=(30.5±0.5) Å obtained by the dynamic light scattering for dilute HSA aqueous solutions (c_m = (0.5÷2) wt.% (φ = 0.018÷0.074), T = 298 K, pH = 7.0) are presented. These values of hydrodynamic radii of HSA macromolecules are comparable with the gyration radii of HSA macromolecules, obtained by the small-angle neutron scattering method in [16]. In [9] the independence of the hydrodynamic radius value from the concentration for dilute HSA solutions is observed, which is typical for the temperature-concentration dependence in the interval of dilute HSA solutions obtained by us (φ < 0.15).

The cellular approach (1)–(14) turns out to be a rather simple, efficient method for the determination of the macromolecular radii from the shear viscosity data for the macromolecular solutions. In other hand, the determination of the macromolecular size in the framework of the dynamic light scattering method is a multistage process, each step of which requires the application of certain physical models with their own approximations and errors. An experimental accessibility of the capillary viscometry method and an ability to quickly change the solution composition during the study make it possible to obtain the dependence of the macromolecular size on the temperature, concentration, pH, and ionic composition of the solution, which is important for understanding the complicated dynamics of biomacromolecules in the aqueous solutions.

Thus, the results obtained by us are comparable with the literature sources data. As above, the comparison of macromolecule radii is complicated by the mismatch between pH solution and the presence of salt ions, to which albumin structure is sensitive.

4.   Conclusions

The article is devoted to the discussion of changes in the structure and size of PVA, BSA, and HSA macromolecules in aqueous solutions depending on temperature, concentration, and acid-base balance (pH) of the solution. Special attention is paid to the comparison of hydrodynamic radius values obtained from data on self-diffusion of macromolecules and shear viscosity of aqueous macromolecular solutions. The importance of these issues is determined by the necessity of careful consideration of water influence on protein structure.

The determination of macromolecule radii from the shear viscosity of its solutions using cellular approaches proves to be one of the simplest and most effective methods. An important feature of the cell approach used by us [23],[24] is that it leads to the results that exactly coincide with those obtained by the Einstein equation [21] and the Batchelor equation [22]. It complements and clarifies the features of macromolecule dynamics in aqueous solutions obtained by other methods.

It should be emphasized that the analysis of macromolecule sizes obtained by different methods is extremely important for investigating changes in the macromolecular coil structure due to their interaction with water at the dissolution in it, as well as considering the interaction between the peripheries of different coils, especially at their overlap, and interaction with ions of acids and alkalis dissolved in water.