Choices of financial reporting regimes and techniques and underlying decision-making processes: a case study analysis of a port authority

Gavin C Reid; Julia A Smith; Yu-Lin Hsu; Gavin C Reid; Julia A Smith; Yu-Lin Hsu

doi:10.3934/NAR.2021007

National Accounting Review

2021, Volume 3, Issue 2: 137-151. doi: 10.3934/NAR.2021007

Previous Article Next Article

Research article

Choices of financial reporting regimes and techniques and underlying decision-making processes: a case study analysis of a port authority

1.
School of Economics & Finance, University of St Andrews, St Andrews, Fife, Scotland, UK
2.
Centre for Business Research, Cambridge Judge Business School, University of Cambridge, Cambridge, UK
3.
Department of Accounting and Finance, Strathclyde University Business School, Glasgow, Scotland, UK

Received: 01 February 2021 Accepted: 08 April 2021 Published: 09 April 2021
JEL Codes: C81, D01, G3, L91, M41

This paper examines how financial reporting modes are determined within a company, from the perspective of perceived costs and benefits. The modes investigated include financial reporting regimes (e.g. International Financial Reporting Standards, UK Generally Accepted Accounting Principles) and the financial reporting techniques which support them (e.g. valuing intangibles and investments, treatment of development costs). A stated preference approach is adopted and applied to a fieldwork analysis of the functioning of a large port authority, which was a member of a group and prepared both consolidated and subsidiary accounts. The analysis is largely qualitative, exploring in-depth such matters as key factors in making choices, the decision-making processes behind choices, and the staging of decisions, but underpinned by a quantitative basis, using a metric for determining net benefits of financial reporting regimes and techniques. Our analysis aims to improve our understanding of a company's choice processes underlying its financial reporting, including its handling of complexity and uncertainty, and its use of innovations in techniques and organisational forms for decision support.

Keywords:

Citation: Gavin C Reid, Julia A Smith, Yu-Lin Hsu. Choices of financial reporting regimes and techniques and underlying decision-making processes: a case study analysis of a port authority[J]. National Accounting Review, 2021, 3(2): 137-151. doi: 10.3934/NAR.2021007

Related Papers:

[1]	Venkatarajan Subbarayalu, Subbu Chinnaraman, Athijayamani Ayyanar, Jayaseelan Chinnapalanisamy . Mechanical properties of vinyl ester hybrid composite laminates reinforced with screw pine and glass fiber. AIMS Materials Science, 2024, 11(1): 114-128. doi: 10.3934/matersci.2024007
[2]	Mohd Shahneel Saharudin, Rasheed Atif, Syafawati Hasbi, Muhammad Naguib Ahmad Nazri, Nur Ubaidah Saidin, Yusof Abdullah . Synergistic effects of halloysite and carbon nanotubes (HNTs + CNTs) on the mechanical properties of epoxy nanocomposites. AIMS Materials Science, 2019, 6(6): 900-910. doi: 10.3934/matersci.2019.6.900
[3]	Saif S. Irhayyim, Hashim Sh. Hammood, Hassan A. Abdulhadi . Effect of nano-TiO₂ particles on mechanical performance of Al–CNT matrix composite. AIMS Materials Science, 2019, 6(6): 1124-1134. doi: 10.3934/matersci.2019.6.1124
[4]	Prashant Tripathi, Vivek Kumar Gupta, Anurag Dixit, Raghvendra Kumar Mishra, Satpal Sharma . Development and characterization of low cost jute, bagasse and glass fiber reinforced advanced hybrid epoxy composites. AIMS Materials Science, 2018, 5(2): 320-337. doi: 10.3934/matersci.2018.2.320
[5]	Robiul Islam Rubel, Md. Hasan Ali, Md. Abu Jafor, Md. Mahmodul Alam . Carbon nanotubes agglomeration in reinforced composites: A review. AIMS Materials Science, 2019, 6(5): 756-780. doi: 10.3934/matersci.2019.5.756
[6]	D. M. S. Costa, M. A. R. Loja . Assessing the static behavior of hybrid CNT-metal-ceramic composite plates. AIMS Materials Science, 2016, 3(3): 808-831. doi: 10.3934/matersci.2016.3.808
[7]	Elena Kossovich . Theoretical study of chitosan-graphene and other chitosan-based nanocomposites stability. AIMS Materials Science, 2017, 4(2): 317-327. doi: 10.3934/matersci.2017.2.317
[8]	M. Rajanish, N. V. Nanjundaradhya, Ramesh S. Sharma, H. K. Shivananda, Alok Hegde . Directional Interlaminar Shear Strength (ILSS) of nano-modified epoxy/unidirectional glass fibre composite. AIMS Materials Science, 2018, 5(4): 603-613. doi: 10.3934/matersci.2018.4.603
[9]	Ahmed Ali Farhan Ogaili, Ehsan S. Al-Ameen, Mohammed Salman Kadhim, Muhanad Nazar Mustafa . Evaluation of mechanical and electrical properties of GFRP composite strengthened with hybrid nanomaterial fillers. AIMS Materials Science, 2020, 7(1): 93-102. doi: 10.3934/matersci.2020.1.93
[10]	Nataliya A. Sakharova, Jorge M. Antunes, Andre F. G. Pereira, Jose V. Fernandes . Developments in the evaluation of elastic properties of carbon nanotubes and their heterojunctions by numerical simulation. AIMS Materials Science, 2017, 4(3): 706-737. doi: 10.3934/matersci.2017.3.706

Abstract

1. Introduction

The notion of a pre-Lie algebra has been introduced independently by M. Gerstenhaber in deformation theory of rings and algebras ^[1] and by Vinberg, under the name of left-symmetric algebra, in his theory of homogeneous convex cones ^[2]. Its defining identity is weaker than associativity and lead also to a Lie algebra using commutators. This algebraic structure describes some properties of cochain spaces in Hochschild cohomology of an associative algebra, rooted trees and vector fields on affine spaces. Moreover, it is playing an increasing role in algebra, geometry and physics due to their applications in nonassociative algebras, combinatorics, numerical analysis and quantum field theory, see ^[3,4,5,6].

Hom-type algebras appeared naturally when studying $q$ -deformations of some algebras of vector fields, like Witt and Virasoro algebras. It turns out that the Jacobi identity is no longer satisfied, these new structures involving a bracket and a linear map satisfy a twisted version of the Jacobi identity and define a so called Hom-Lie algebras which form a wider class, see ^[7,8]. Hom-pre-Lie algebras were introduced in ^[9] as a class of Hom-Lie admissible algebras, and play important roles in the study of Hom-Lie bialgebras and Hom-Lie 2-algebras ^[10,11,12]. Recently Hom-pre-Lie algebras were studied from several aspects. Cohomologies of Hom-pre-Lie algebras were studied in ^[13]; The geometrization of Hom-pre-Lie algebras was studied in ^[14]; Universal $\alpha$ -central extensions of Hom-pre-Lie algebras were studied in ^[15]; Hom-pre-Lie bialgebras were studied in ^[16,17]. Furthermore, connections between (Hom-)pre-Lie algebras and various algebraic structures have been established and discussed; like with Rota-Baxter operators, $\mathcal{O}$ -operators, (Hom-)dendriform algebras, (Hom-)associative algebras and Yang-Baxter equation, see ^{[5,18,19,20,21,22]}.

The cohomology of pre-Lie algebras was defined in ^[23] and generalized in a straightforward way to Hom-pre-Lie algebras in ^[13]. Note that the cohomology given there has some restrictions: the second cohomology group can only control deformations of the multiplication, and it can not be applied to study simultaneous deformations of both the multiplication and the homomorphism in a Hom-pre-Lie algebra. The main purpose of this paper is to define a new type of cohomology of Hom-pre-Lie algebras which is richer than the previous one. The obtained cohomology is called the full cohomology and allows to control simultaneous deformations of both the multiplication and the homomorphism in a Hom-pre-Lie algebra. This type of cohomology was established for Hom-associative algebras and Hom-Lie algebras in ^[24,25], and called respectively $\alpha$ -type Hochschild cohomology and $\alpha$ -type Chevalley-Eilenberg cohomology. See ^[26,27,28] for more studies on deformations and extensions of Hom-Lie algebras.

The paper is organized as follows. In Section 2, first we recall some basics of Hom-Lie algebras, Hom-pre-Lie algebras and representations, and then provide our main result defining the full cohomology of a Hom-pre-Lie algebra with coefficients in a given representation. In Section 3, we study one parameter formal deformations of a Hom-pre-Lie algebra, where both the defining multiplication and homomorphism are deformed, using formal power series. We show that the full cohomology of Hom-pre-Lie algebras controls these simultaneous deformations. Moreover, a relationship between deformations of a Hom-pre-Lie algebra and deformations of its sub-adjacent Lie algebra is established. Section 4 deals with abelian extensions of Hom-pre-Lie algebras. We show that the full cohomology fits perfectly and its second cohomology group classifies abelian extensions of a Hom-pre-Lie algebra by a given representation. The proof of the key Lemma to show that the four operators define a cochain complex is lengthy and it is given in the Appendix.

2. The full cohomology of Hom-pre-Lie algebras

In this section, first we recall some basic facts about Hom-Lie algebras and Hom-pre-Lie algebras. Then we introduce the full cohomology of Hom-pre-Lie algebras, which will be used to classify infinitesimal deformations and abelian extensions of Hom-pre-Lie algebras.

Definition 2.1. (see ^[7]) A Hom-Lie algebra is a triple $(\mathfrak g, [\cdot, \cdot]_{ \mathfrak g}, \phi_{ \mathfrak g})$ consisting of a vector space $\mathfrak g$ , a skew-symmetric bilinear map $[\cdot, \cdot]_{ \mathfrak g}:\wedge^2 \mathfrak g\longrightarrow \mathfrak g$ and a homomorphism $\phi_{ \mathfrak g}: \mathfrak g\longrightarrow \mathfrak g$ , satisfying $\phi_{ \mathfrak g}[x, y] = [\phi_{ \mathfrak g}(x), \phi_{ \mathfrak g}(y)]$ and

$\begin{equation} [\phi_{ \mathfrak g}(x), [y, z]_{ \mathfrak g}]_{ \mathfrak g}+[\phi_{ \mathfrak g}(y), [z, x]_{ \mathfrak g}]_{ \mathfrak g}+[\phi_{ \mathfrak g}(z), [x, y]_{ \mathfrak g}]_{ \mathfrak g} = 0, \quad \forall\; x, y, z\in \mathfrak g. \end{equation}$

(2.1)

A Hom-Lie algebra $(\mathfrak g, [\cdot, \cdot]_{ \mathfrak g}, \phi_{ \mathfrak g})$ is said to be regular if $\phi_{ \mathfrak g}$ is invertible.

Definition 2.2. (see ^[29]) A representation of a Hom-Lie algebra $(\mathfrak g, [\cdot, \cdot]_{ \mathfrak g}, \phi_{ \mathfrak g})$ on a vector space $V$ with respect to $\beta\in \mathfrak {gl}(V)$ is a linear map $\rho: \mathfrak g\longrightarrow \mathfrak {gl}(V)$ , such that for all $x, y\in \mathfrak g$ , the following equalities are satisfied:

$\begin{eqnarray} \rho(\phi_{ \mathfrak g}(x))\circ \beta& = &\beta\circ \rho(x), \end{eqnarray}$

(2.2)

$\begin{eqnarray} \rho([x, y]_{ \mathfrak g})\circ \beta& = &\rho(\phi_{ \mathfrak g}(x))\circ\rho(y)-\rho(\phi_{ \mathfrak g}(y))\circ\rho(x). \end{eqnarray}$

(2.3)

We denote a representation of a Hom-Lie algebra $(\mathfrak g, [\cdot, \cdot]_{ \mathfrak g}, \phi_{ \mathfrak g})$ by a triple $(V, \beta, \rho)$ .

Definition 2.3. (see ^[9]) A Hom-pre-Lie algebra $(A, \cdot, \alpha)$ is a vector space $A$ equipped with a bilinear product $\cdot:A\otimes A\longrightarrow A$ , and $\alpha\in \mathfrak {gl}(A)$ , such that for all $x, y, z\in A$ , $\alpha(x \cdot y) = \alpha(x)\cdot \alpha(y)$ and the following equality is satisfied:

$\begin{eqnarray} (x\cdot y)\cdot \alpha(z)-\alpha(x)\cdot (y\cdot z) = (y\cdot x)\cdot \alpha(z)-\alpha(y)\cdot (x\cdot z). \end{eqnarray}$

(2.4)

A Hom-pre-Lie algebra $(A, \cdot, \alpha)$ is said to be regular if $\alpha$ is invertible.

Let $(A, \cdot, \alpha)$ be a Hom-pre-Lie algebra. The commutator $[x, y]_C = x\cdot y-y\cdot x$ defines a Hom-Lie algebra $(A, [\cdot, \cdot]_C, \alpha)$ , which is denoted by $A^C$ and called the sub-adjacent Hom-Lie algebra of $(A, \cdot, \alpha)$ .

Definition 2.4. (see ^[13]) A morphism from a Hom-pre-Lie algebra $(A, \cdot, \alpha)$ to a Hom-pre-Lie algebra $(A', \cdot', \alpha')$ is a linear map $f:A\longrightarrow A'$ such that for all $x, y\in A$ , the following equalities are satisfied:

$\begin{eqnarray} f(x\cdot y)& = &f(x)\cdot' f(y), \quad \forall x, y\in A, \end{eqnarray}$

(2.5)

$\begin{eqnarray} f\circ \alpha& = &\alpha'\circ f. \end{eqnarray}$

(2.6)

Definition 2.5. (see ^[17]) A representation of a Hom-pre-Lie algebra $(A, \cdot, \alpha)$ on a vector space $V$ with respect to $\beta\in \mathfrak {gl}(V)$ consists of a pair $(\rho, \mu)$ , where $\rho:A\longrightarrow \mathfrak {gl}(V)$ is a representation of the sub-adjacent Hom-Lie algebra $A^C$ on $V$ with respect to $\beta\in \mathfrak {gl}(V)$ , and $\mu:A\longrightarrow \mathfrak {gl}(V)$ is a linear map, satisfying, for all $x, y\in A$ :

$\begin{eqnarray} \beta\circ \mu(x)& = &\mu(\alpha(x))\circ \beta, \end{eqnarray}$

(2.7)

$\begin{eqnarray} \mu(\alpha(y))\circ\mu(x)-\mu(x\cdot y)\circ \beta& = &\mu(\alpha(y))\circ\rho(x)-\rho(\alpha(x))\circ\mu(y). \end{eqnarray}$

(2.8)

We denote a representation of a Hom-pre-Lie algebra $(A, \cdot, \alpha)$ by a quadruple $(V, \beta, \rho, \mu)$ . Furthermore, Let $L, R:A\longrightarrow \mathfrak {gl}(A)$ be linear maps, where $L_xy = x\cdot y, R_xy = y\cdot x$ . Then $(A, \alpha, L, R)$ is also a representation, which we call the regular representation.

Let $(A, \cdot, \alpha)$ be a Hom-pre-Lie algebra. In the sequel, we will also denote the Hom-pre-Lie algebra multiplication $\cdot$ by $\omega$ .

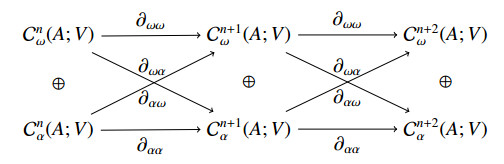
Let $(V, \beta, \rho, \mu)$ be a representation of a Hom-pre-Lie algebra $(A, \omega, \alpha)$ . We define ${\mathcal{C}}^n_\omega(A; V)$ and ${\mathcal{C}}^n_\alpha(A; V)$ respectively by

${\mathcal{C}}^n_\omega(A;V) = \mathrm{Hom}(\wedge^{n-1} A\otimes A, V), \quad {\mathcal{C}}^n_\alpha(A;V) = \mathrm{Hom}(\wedge^{n-2} A\otimes A, V), \quad \forall n\geq 2.$

Define the set of cochains $\tilde{ {\mathcal{C}}}^n(A; V)$ by

$\begin{equation} \left\{\begin{array}{ll} & \tilde{ {\mathcal{C}}}^n(A;V) = {\mathcal{C}}^n_\omega(A;V)\oplus {\mathcal{C}}^n_\alpha(A;V), \quad \forall n\geq 2, \\ & \tilde{ {\mathcal{C}}}^1(A;V) = \mathrm{Hom}(A, V). \end{array}\right. \end{equation}$

(2.9)

For all $(\varphi, \psi) \in \tilde{ {\mathcal{C}}}^n(A; V), x_1, \dots, x_{n+1}\in A$ , we define $\partial_{\omega\omega}: {\mathcal{C}}^n_\omega(A; V)\longrightarrow {\mathcal{C}}^{n+1}_\omega(A; V)$ by

$\begin{eqnarray*} &&(\partial_{\omega\omega}\varphi)(x_1, \dots, x_{n+1})\\ && = \sum\limits_{i = 1}^n(-1)^{i+1}\rho(\alpha^{n-1}(x_i))\varphi(x_1, \dots, \widehat{x_i}, \dots, x_{n+1})\\ &&+\sum\limits_{i = 1}^n(-1)^{i+1}\mu(\alpha^{n-1}(x_{n+1}))\varphi(x_1, \dots, \widehat{x_i}, \dots, x_n, x_i)\\ &&-\sum\limits_{i = 1}^n(-1)^{i+1} \varphi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}\dots, \alpha(x_n), x_i\cdot x_{n+1})\\ &&+\sum\limits_{1\leq i < j\leq n}(-1)^{i+j} \varphi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1})), \end{eqnarray*}$

where the hat corresponds to deleting the element, define $\partial_{\alpha\alpha}: {\mathcal{C}}^n_\alpha(A; V)\longrightarrow {\mathcal{C}}^{n+1}_\alpha(A; V)$ by

$\begin{eqnarray*} &&(\partial_{\alpha\alpha} \psi)(x_1, \dots, x_n)\\ && = \sum\limits_{i = 1}^{n-1}(-1)^i\rho(\alpha^{n-1}(x_i))\psi(x_1, \dots, \widehat{x_i}, \dots, x_n)\\ &&+\sum\limits_{i = 1}^{n-1}(-1)^i\mu(\alpha^{n-1}(x_n))\psi(x_1, \dots, \widehat{x_i}, \dots, x_{n-1}, x_i)\\ &&-\sum\limits_{i = 1}^{n-1}(-1)^i \psi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \alpha(x_{n-1}), x_i\cdot x_n)\\ &&+\sum\limits_{1\leq i < j\leq n-1}(-1)^{i+j-1} \psi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_n)), \end{eqnarray*}$

define $\partial_{\omega\alpha}: {\mathcal{C}}^n_\omega(A; V)\longrightarrow {\mathcal{C}}^{n+1}_\alpha(A; V)$ by

$\begin{eqnarray*} (\partial_{\omega\alpha} \varphi)(x_1, \dots, x_n) = \beta\varphi(x_1, \dots, x_n)-\varphi(\alpha(x_1), \dots, \alpha(x_n)), \end{eqnarray*}$

and define $\partial_{\alpha\omega}: {\mathcal{C}}^n_\alpha(A; V)\longrightarrow {\mathcal{C}}^{n+1}_\omega(A; V)$ by

$\begin{eqnarray*} &&(\partial_{\alpha\omega} \psi)(x_1, \dots, x_{n+1})\\ && = \sum\limits_{1\leq i < j\leq n}(-1)^{i+j}\rho([\alpha^{n-2}(x_i), \alpha^{n-2}(x_j)]_C) \psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_j}, \dots, x_{n+1})\\ &&+\sum\limits_{1\leq i < j\leq n}(-1)^{i+j}\mu(\alpha^{n-2}(x_i)\cdot\alpha^{n-2}(x_{n+1}))\psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_j}, \dots, x_n, x_j)\\ &&-\sum\limits_{1\leq i < j\leq n}(-1)^{i+j}\mu(\alpha^{n-2}(x_j)\cdot\alpha^{n-2}(x_{n+1}))\psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_j}, \dots, x_n, x_i). \end{eqnarray*}$

Define the operator $\tilde{\partial}:\tilde{ {\mathcal{C}}}^n(A; V)\longrightarrow \tilde{ {\mathcal{C}}}^{n+1}(A; V)$ by

$\begin{eqnarray} \tilde{\partial}(\varphi, \psi)& = &(\partial_{\omega\omega}\varphi+\partial_{\alpha\omega}\psi, \partial_{\omega\alpha}\varphi+\partial_{\alpha\alpha}\psi), \quad \forall \varphi \in {\mathcal{C}}^n_\omega(A;V), \psi \in {\mathcal{C}}^n_\alpha(A;V), n\geq 2, \end{eqnarray}$

(2.10)

$\begin{eqnarray} \tilde{\partial}(\varphi)& = &(\partial_{\omega\omega}\varphi, \partial_{\omega\alpha}\varphi), \quad \forall \varphi \in \mathrm{Hom}(A, V). \end{eqnarray}$

(2.11)

The following diagram will explain the above operators:

Lemma 2.6. With the above notations, we have

$\begin{eqnarray} \partial_{\omega\omega}\circ \partial_{\omega\omega}+\partial_{\alpha\omega}\circ \partial_{\omega\alpha}& = &0, \end{eqnarray}$

(2.12)

$\begin{eqnarray} \partial_{\omega\omega}\circ \partial_{\alpha\omega}+\partial_{\alpha\omega}\circ \partial_{\alpha\alpha}& = &0, \end{eqnarray}$

(2.13)

$\begin{eqnarray} \partial_{\omega\alpha}\circ \partial_{\omega\omega}+\partial_{\alpha\alpha}\circ \partial_{\omega\alpha}& = &0, \end{eqnarray}$

(2.14)

$\begin{eqnarray} \partial_{\omega\alpha}\circ \partial_{\alpha\omega}+\partial_{\alpha\alpha}\circ \partial_{\alpha\alpha}& = &0. \end{eqnarray}$

(2.15)

Proof. The proof is given in the Appendix.

Theorem 2.7. The operator $\tilde{\partial}:\tilde{C}^n(A; V)\longrightarrow \tilde{C}^{n+1}(A; V)$ defined as above satisfies $\tilde{\partial}\circ\tilde{\partial} = 0$ .

Proof. When $n\geq 2$ , for all $(\varphi, \psi)\in \tilde{ {\mathcal{C}}}^n(A; V)$ , by (2.10) and Lemma 2.6, we have

$\begin{eqnarray*} \tilde{\partial}\circ\tilde{\partial}(\varphi, \psi) & = &\tilde{\partial}(\partial_{\omega\omega}\varphi+\partial_{\alpha\omega}\psi, \partial_{\omega\alpha}\varphi+\partial_{\alpha\alpha}\psi)\\ & = &(\partial_{\omega\omega}\partial_{\omega\omega}\varphi+\partial_{\omega\omega}\partial_{\alpha\omega}\psi+\partial_{\alpha\omega}\partial_{\omega\alpha}\varphi+\partial_{\alpha\omega}\partial_{\alpha\alpha}\psi, \\ &&\partial_{\omega\alpha}\partial_{\omega\omega}\varphi+\partial_{\omega\alpha}\partial_{\alpha\omega}\psi+\partial_{\alpha\alpha}\partial_{\omega\alpha}\varphi+\partial_{\alpha\alpha}\partial_{\alpha\alpha}\psi)\\ & = &0. \end{eqnarray*}$

When $n = 1$ , for all $\varphi \in \mathrm{Hom}(A, V)$ , by (2.11) and Lemma 2.6, we have

$\begin{eqnarray*} \tilde{\partial}\circ\tilde{\partial}(\varphi) & = &\tilde{\partial}(\partial_{\omega\omega}\varphi, \partial_{\omega\alpha}\varphi)\\ & = &(\partial_{\omega\omega}\partial_{\omega\omega}\varphi+\partial_{\alpha\omega}\partial_{\omega\alpha}\varphi, \partial_{\omega\alpha}\partial_{\omega\omega}\varphi+\partial_{\alpha\alpha}\partial_{\omega\alpha}\varphi)\\ & = &0. \end{eqnarray*}$

This finishes the proof.

We denote the set of closed $n$ -cochains by $\tilde{Z}^n(A; V)$ and the set of exact $n$ -cochains by $\tilde{B}^n(A; V)$ . We denote by $\tilde{H}^n(A; V) = \tilde{Z}^n(A; V)/\tilde{B}^n(A; V)$ the corresponding cohomology groups.

Definition 2.8. Let $(V, \beta, \rho, \mu)$ be a representation of a Hom-pre-Lie algebra $(A, \cdot, \alpha)$ . The cohomology of the cochain complex $(\oplus_{n = 1}^\infty\tilde{C}^n(A; V), \partial)$ is called the full cohomology of the Hom-pre-Lie algebra $(A, \cdot, \alpha)$ with coefficients in the representation $(V, \beta, \rho, \mu)$ .

We use $\tilde{\partial}_{ \mathrm{reg}}$ to denote the coboundary operator of the Hom-pre-Lie algebra $(A, \cdot, \alpha)$ with coefficients in the regular representation. The corresponding cohomology group will be denoted by $\tilde{H}^n(A; A)$ .

Remark 2.9. Compared with the cohomology theory of Hom-pre-Lie algebras studied in ^[13], the above full cohomology contains more informations. In the next section, we will see that the second cohomology group can control simultaneous deformations of the multiplication and the homomorphism in a Hom-pre-Lie algebra.

3. Formal deformations of Hom-pre-Lie algebras

In this section, we study formal deformations of Hom-pre-Lie algebras using the cohomology theory established in the last section. We show that the infinitesimal of a formal deformation is a 2-cocycle and depends only on its cohomology class. Moreover, if the cohomology group $\tilde{H}^2(A; A)$ is trivial, then the Hom-pre-Lie algebra is rigid.

Definition 3.1. Let $(A, \omega, \alpha)$ be a Hom-pre-Lie algebra, $\omega_t = \omega+\sum_{i = 1}^{+\infty} \omega_it^i:A[[t]]\otimes A[[t]]\longrightarrow A[[t]]$ be a $\mathbb K[[t]]$ -bilinear map and $\alpha_t = \alpha+\sum_{i = 1}^{+\infty} \alpha_it^i:A[[t]]\longrightarrow A[[t]]$ be a $\mathbb K[[t]]$ -linear map, where $\omega_i:A\otimes A\longrightarrow A$ and $\alpha_i:A\longrightarrow A$ are linear maps. If $(A[[t]], \omega_t, \alpha_t)$ is still a Hom-pre-Lie algebra, we say that $\{\omega_i, \alpha_i\}_{i\geq1}$ generates a $1$ -parameter formal deformation of a Hom-pre-Lie algebra $(A, \omega, \alpha)$ .

If $\{\omega_i, \alpha_i\}_{i\geq1}$ generates a $1$ -parameter formal deformation of a Hom-pre-Lie algebra $(A, \omega, \alpha)$ , for all $x, y, z\in A$ and $n = 1, 2, \dots$ , we then have

$\begin{equation} \sum\limits_{i+j+k = n\atop i, j, k\geq 0}\omega_i(\omega_j(x, y), \alpha_k(z))-\omega_i(\alpha_j(x), \omega_k(y, z))-\omega_i(\omega_j(y, x), \alpha_k(z))+\omega_i(\alpha_j(y), \omega_k(x, z)) = 0. \end{equation}$

(3.1)

Moreover, we have

$\begin{eqnarray} &&\sum\limits_{i+j+k = n\atop 0 < i, j, k\leq n-1 }\omega_i(\omega_j(x, y), \alpha_k(z))-\omega_i(\alpha_j(x), \omega_k(y, z))-\omega_i(\omega_j(y, x), \alpha_k(z))+\omega_i(\alpha_j(y), \omega_k(x, z))\\ & = &(\partial_{\omega\omega}\omega_n+\partial_{\alpha\omega}\alpha_n)(x, y, z). \end{eqnarray}$

(3.2)

For all $x, y\in A$ and $n = 1, 2, \dots$ , we have

$\begin{equation} \sum\limits_{i+j+k = n\atop i, j, k\geq 0}\omega_i(\alpha_j(x), \alpha_k(y))-\sum\limits_{i+j = n\atop i, j\geq 0}\alpha_i(\omega_j(x, y)) = 0. \end{equation}$

(3.3)

Moreover, we have

$\begin{equation} \sum\limits_{i+j+k = n\atop 0 < i, j, k\leq n-1}\omega_i(\alpha_j(x), \alpha_k(y))-\sum\limits_{i+j = n\atop i, j > 0}\alpha_i(\omega_j(x, y)) = (\partial_{\omega\alpha}\omega_n+\partial_{\alpha\alpha}\alpha_n)(x, y). \end{equation}$

(3.4)

Proposition 3.2. Let $(\omega_t = \omega+\sum_{i = 1}^{+\infty} \omega_it^i, \alpha_t = \alpha+\sum_{i = 1}^{+\infty} \alpha_it^i)$ be a $1$ -parameter formal deformation of a Hom-pre-Lie algebra $(A, \omega, \alpha)$ . Then $(\omega_1, \alpha_1)$ is a $2$ -cocycle of the Hom-pre-Lie algebra $(A, \omega, \alpha)$ with coefficients in the regular representation.

Proof. When $n = 1$ , by (3.1), we have

$\begin{eqnarray*} \nonumber 0& = &(x\cdot y)\cdot\alpha_1(z)+\omega_1(x, y)\cdot\alpha(z)+\omega_1(x\cdot y, \alpha(z))-\alpha(x)\cdot\omega_1(y, z)\\ \nonumber &&-\alpha_1(x)\cdot (y\cdot z)-\omega_1(\alpha(x), y\cdot z)-(y\cdot x)\cdot\alpha_1(z)-\omega_1(y, x)\cdot\alpha(z)\\ \nonumber &&-\omega_1(y\cdot x, \alpha(z))+\alpha(y)\cdot\omega_1(x, z)+\alpha_1(y)\cdot(x\cdot z)+\omega_1(\alpha(y), x\cdot z)\\ & = &-(\partial_{\omega\omega}\omega_1+\partial_{\alpha\omega}\alpha_1)(x, y, z), \end{eqnarray*}$

and by (3.3), we have

$\begin{eqnarray*} \nonumber 0& = &\omega_1(\alpha(x), \alpha(y))+\alpha_1(x)\cdot \alpha(y)+\alpha(x)\cdot \alpha_1(y)-\alpha(\omega_1(x, y))-\alpha_1(x\cdot y)\\ & = &-(\partial_{\omega\alpha}\omega_1+\partial_{\alpha\alpha}\alpha_1)(x, y), \end{eqnarray*}$

which implies that $\tilde{\partial}_{ \mathrm{reg}}(\omega_1, \alpha_1) = 0$ . Thus, $(\omega_1, \alpha_1)$ is a $2$ -cocycle of the Hom-pre-Lie algebra $(A, \omega, \alpha)$ .

Definition 3.3. The $2$ -cocycle $(\omega_1, \alpha_1)$ is called the infinitesimal of the $1$ -parameter formal deformation $(A[[t]], \omega_t, \alpha_t)$ of the Hom-pre-Lie algebra $(A, \omega, \alpha)$ .

Definition 3.4. Let $(\omega_t' = \omega+\sum_{i = 1}^{+\infty} \omega_i't^i, \alpha_t' = \alpha+\sum_{i = 1}^{+\infty} \alpha_i't^i)$ and $(\omega_t = \omega+\sum_{i = 1}^{+\infty} \omega_it^i, \alpha_t = \alpha+\sum_{i = 1}^{+\infty} \alpha_it^i)$ be two $1$ -parameter formal deformations of a Hom-pre-Lie algebra $(A, \omega, \alpha)$ . A formal isomorphism from $(A[[t]], \omega_t', \alpha_t')$ to $(A[[t]], \omega_t, \alpha_t)$ is a power series $\Phi_t = \sum_{i = 0}^{+\infty} \varphi_it^i$ , where $\varphi_i:A\longrightarrow A$ are linear maps with $\varphi_0 = { {\rm{Id}}}$ , such that

$\begin{eqnarray} \Phi_t\circ \omega_t'& = &\omega_t \circ(\Phi_t\times \Phi_t), \end{eqnarray}$

(3.5)

$\begin{eqnarray} \alpha_t\circ \Phi_t& = &\Phi_t\circ \alpha_t'. \end{eqnarray}$

(3.6)

Two $1$ -parameter formal deformations $(A[[t]], \omega_t', \alpha_t')$ and $(A[[t]], \omega_t, \alpha_t)$ are said to be equivalent if there exists a formal isomorphism $\Phi_t = \sum_{i = 0}^{+\infty} \varphi_it^i$ from $(A[[t]], \omega_t', \alpha_t')$ to $(A[[t]], \omega_t, \alpha_t)$ .

Theorem 3.5. Let $(A, \omega, \alpha)$ be a Hom-pre-Lie algebra.If two $1$ -parameter formal deformations $(\omega_t' = \omega+\sum_{i = 1}^{+\infty} \omega_i't^i, \alpha_t' = \alpha+\sum_{i = 1}^{+\infty} \alpha_i't^i)$ and $(\omega_t = \omega+\sum_{i = 1}^{+\infty} \omega_it^i, \alpha_t = \alpha+\sum_{i = 1}^{+\infty} \alpha_it^i)$ are equivalent, then there infinitesimals $(\omega_1', \alpha_1')$ and $(\omega_1, \alpha_1)$ are in the same cohomology class of $\tilde{H}^2(A; A)$ .

Proof. Let $(\omega_t', \alpha_t')$ and $(\omega_t, \alpha_t)$ be two $1$ -parameter formal deformations. By Proposition 3.2, we have $(\omega_1', \alpha_1')$ and $(\omega_1, \alpha_1) \in \tilde{Z}^2(A; A)$ . Let $\Phi_t = \sum_{i = 0}^{+\infty} \varphi_it^i$ be the formal isomorphism. Then for all $x, y\in A$ , we have

$\begin{eqnarray*} \omega_t'(x, y) & = &\Phi_t^{-1}\circ\omega_t(\Phi_t(x), \Phi_t(y))\\ & = &({ {\rm{Id}}}-\varphi_1 t+\dots)\omega_t\big(x+\varphi_1(x)t+\dots, y+\varphi_1(y)t+\dots\big)\\ & = &({ {\rm{Id}}}-\varphi_1 t+\dots)\Big(x\cdot y+\big(x\cdot\varphi_1(y)+\varphi_1(x)\cdot y+\omega_1(x, y)\big)t+\dots\Big)\\ & = &x\cdot y+\Big(x\cdot\varphi_1(y)+\varphi_1(x)\cdot y+\omega_1(x, y)-\varphi_1(x\cdot y)\Big)t+\dots. \end{eqnarray*}$

Thus, we have

$\begin{eqnarray*} \omega_1'(x, y)-\omega_1(x, y)& = &x\cdot\varphi_1(y)+\varphi_1(x)\cdot y-\varphi_1(x\cdot y)\\ & = &\partial_{\omega\omega}\varphi_1(x, y), \end{eqnarray*}$

which implies that $\omega_1'-\omega_1 = \partial_{\omega\omega}\varphi_1.$

For all $x\in A$ , we have

$\begin{eqnarray*} \alpha_t'(x) & = &\Phi_t^{-1}\circ\alpha_t(\Phi_t(x))\\ & = &({ {\rm{Id}}}-\varphi_1 t+\dots)\alpha_t\big(x+\varphi_1(x)t+\dots\big)\\ & = &({ {\rm{Id}}}-\varphi_1 t+\dots)\Big(\alpha(x)+\big(\alpha(\varphi_1(x))+\alpha_1(x))t+\dots\Big)\\ & = &\alpha(x)+\Big(\alpha(\varphi_1(x))+\alpha_1(x)-\varphi_1(\alpha(x))\Big)t+\dots. \end{eqnarray*}$

Thus, we have

$\begin{equation} \nonumber\alpha_1'(x)-\alpha_1(x) = \alpha(\varphi_1(x))-\varphi_1(\alpha(x)) = \partial_{\omega\alpha}\varphi_1(x), \end{equation}$

which implies that $\alpha_1'-\alpha_1 = \partial_{\omega\alpha}\varphi_1.$

Thus, we have $(\omega_1'-\omega_1, \alpha_1'-\alpha_1)\in \tilde{B}^2(A; A)$ . This finishes the proof.

Definition 3.6. A $1$ -parameter formal deformation $(A[[t]], \omega_t, \alpha_t)$ of a Hom-pre-Lie algebra $(A, \omega, \alpha)$ is said to be trivial if it is equivalent to $(A, \omega, \alpha)$ , i.e. there exists $\Phi_t = \sum_{i = 0}^{+\infty} \varphi_it^i$ , where $\varphi_i:A\longrightarrow A$ are linear maps with $\varphi_0 = { {\rm{Id}}}$ , such that

$\begin{eqnarray} \Phi_t\circ \omega_t& = &\omega \circ(\Phi_t\times \Phi_t), \end{eqnarray}$

(3.7)

$\begin{eqnarray} \alpha \circ\Phi_t& = &\Phi_t\circ\alpha_t. \end{eqnarray}$

(3.8)

Definition 3.7. Let $(A, \omega, \alpha)$ be a Hom-pre-Lie algebra. If all $1$ -parameter formal deformations are trivial, we say that $(A, \omega, \alpha)$ is rigid.

Theorem 3.8. Let $(A, \omega, \alpha)$ be a Hom-pre-Lie algebra. If $\tilde{H}^2(A; A) = 0$ , then $(A, \omega, \alpha)$ is rigid.

Proof. Let $(\omega_t = \omega+\sum_{i = 1}^{+\infty} \omega_it^i, \alpha_t = \alpha+\sum_{i = 1}^{+\infty} \alpha_it^i)$ be a $1$ -parameter formal deformation and assume that $n\geq1$ is the minimal number such that at least one of $\omega_n$ and $\alpha_n$ is not zero. By (3.2), (3.4) and $\tilde{H}^2(A; A) = 0$ , we have $(\omega_n, \alpha_n)\in \tilde{B}^2(A; A)$ . Thus, there exists $\varphi_n \in \tilde{ {\mathcal{C}}}^1(A; A)$ such that $\omega_n = \partial_{\omega\omega}(-\varphi_n)$ and $\alpha_n = \partial_{\omega\alpha}(-\varphi_n)$ . Let $\Phi_t = { {\rm{Id}}}+\varphi_nt^n$ and define a new formal deformation $(\omega_t', \alpha_t')$ by $\omega_t'(x, y) = \Phi_t^{-1}\circ\omega_t(\Phi_t(x), \Phi_t(y))$ and $\alpha_t'(x) = \Phi_t^{-1}\circ\alpha_t(\Phi_t(x))$ . Then $(\omega_t', \alpha_t')$ and $(\omega_t, \alpha_t)$ are equivalent. By a straightforward computation, for all $x, y\in A$ , we have

$\begin{eqnarray*} \omega_t'(x, y) & = &\Phi_t^{-1}\circ\omega_t(\Phi_t(x), \Phi_t(y))\\ & = &({ {\rm{Id}}}-\varphi_n t^n+\dots)\omega_t\big(x+\varphi_n(x)t^n, y+\varphi_n(y)t^n\big)\\ & = &({ {\rm{Id}}}-\varphi_n t^n+\dots)\Big(x\cdot y+\big(x\cdot\varphi_n(y)+\varphi_n(x)\cdot y+\omega_n(x, y)\big)t^n+\dots\Big)\\ & = &x\cdot y+\Big(x\cdot\varphi_n(y)+\varphi_n(x)\cdot y+\omega_n(x, y)-\varphi_n(x\cdot y)\Big)t^n+\dots. \end{eqnarray*}$

Thus, we have $\omega_1' = \omega_2' = \dots = \omega_{n-1}' = 0$ . Moreover, we obtain

$\begin{eqnarray*} \omega_n'(x, y)& = &\varphi_n(x)\cdot y+x\cdot \varphi_n(y)+\omega_n(x, y)-\varphi_n(x\cdot y)\\ & = &\partial_{\omega\omega}\varphi_n(x, y)+\omega_n(x, y)\\ & = &0. \end{eqnarray*}$

For all $x\in A$ , we get

$\begin{eqnarray*} \alpha_t'(x) & = &\Phi_t^{-1}\circ\alpha_t(\Phi_t(x))\\ & = &({ {\rm{Id}}}-\varphi_n t^n+\dots)\alpha_t\big(x+\varphi_n(x)t^n\big)\\ & = &({ {\rm{Id}}}-\varphi_1 t^n+\dots)\Big(\alpha(x)+\big(\alpha(\varphi_n(x))+\alpha_n(x))t^n+\dots\Big)\\ & = &\alpha(x)+\Big(\alpha(\varphi_n(x))+\alpha_n(x)-\varphi_n(\alpha(x))\Big)t^n+\dots. \end{eqnarray*}$

Thus, $\alpha_1' = \alpha_2' = \dots = \alpha_{n-1}' = 0$ , and

$\begin{eqnarray*} \alpha_n'(x, y)& = &\alpha(\varphi_n(x))+\alpha_n(x)-\varphi_n(\alpha(x))\\ & = &\partial_{\omega\alpha}\varphi_n(x)+\alpha_n(x)\\ & = &0. \end{eqnarray*}$

By repeating this process, we obtain that $(A[[t]], \omega_t, \alpha_t)$ is equivalent to $(A, \omega, \alpha)$ . The proof is finished.

At the end of this section, we recall $1$ -parameter formal deformations of Hom-Lie algebras, and establish the relation between $1$ -parameter formal deformations of Hom-pre-Lie algebras and $1$ -parameter formal deformations of Hom-Lie algebras.

Definition 3.9. (see ^[25]) Let $(\mathfrak g, [\cdot, \cdot]_{ \mathfrak g}, \phi_{ \mathfrak g})$ be a Hom-Lie algebra, $[\cdot, \cdot]_t = [\cdot, \cdot]_{ \mathfrak g}+\sum_{i = 1}^{+\infty} \bar{\omega}_it^i: \mathfrak g[[t]]\wedge \mathfrak g[[t]]\longrightarrow \mathfrak g[[t]]$ be a $\mathbb K[[t]]$ -bilinear map and $\phi_t = \phi_{ \mathfrak g}+\sum_{i = 1}^{+\infty} \phi_it^i: \mathfrak g[[t]]\longrightarrow \mathfrak g[[t]]$ be a $\mathbb K[[t]]$ -linear map, where $\bar{\omega}_i: \mathfrak g\otimes \mathfrak g\longrightarrow \mathfrak g$ and $\phi_i: \mathfrak g\longrightarrow \mathfrak g$ are linear maps. If $(\mathfrak g[[t]], [\cdot, \cdot]_t, \phi_t)$ is still a Hom-Lie algebra, we say that $\{\bar{\omega}_i, \phi_i\}_{i\geq1}$ generates a $1$ -parameter formal deformation of a Hom-Lie algebra $(\mathfrak g, [\cdot, \cdot]_{ \mathfrak g}, \phi_{ \mathfrak g})$ .

Proposition 3.10. Let $(\omega_t = \omega+\sum_{i = 1}^{+\infty} \omega_it^i, \alpha_t = \alpha+\sum_{i = 1}^{+\infty} \alpha_it^i)$ be a $1$ -parameter formal deformation of a Hom-pre-Lie algebra $(A, \omega, \alpha)$ , then

$\{\omega_i-\omega_i\circ \sigma, \alpha_i\}_{i\geq1}$

generates a $1$ -parameter formal deformation of the sub-adjacent Hom-Lie algebra $A^C$ , where $\sigma:A\otimes A\longrightarrow A\otimes A$ is the flip operator defined by $\sigma(x\otimes y) = y\otimes x$ for all $x, y\in A$ .

Proof. Let $(A[[t]], \omega_t, \alpha_t)$ be a $1$ -parameter formal deformation of a Hom-pre-Lie algebra $(A, \omega, \alpha)$ . For all $x, y\in A$ , we have

$\begin{eqnarray*} \omega_t(x, y)-\omega_t(y, x)& = &\omega(x, y)-\omega(y, x)+\sum\limits_{i = 1}^{+\infty} \omega_i(x, y)t^i-\sum\limits_{i = 1}^{+\infty} \omega_i(y, x)t^i\\ & = &[x, y]_C+\sum\limits_{i = 1}^{+\infty}(\omega_i-\omega_i\circ \sigma)(x, y)t^i, \end{eqnarray*}$

and

$\begin{equation} \nonumber\alpha_t\circ(\omega_t(x, y)-\omega_t(y, x)) = \omega_t(\alpha_t(x), \alpha_t(y))-\omega_t(\alpha_t(y), \alpha_t(x)). \end{equation}$

Therefore, $\{\omega_i-\omega_i\circ \sigma, \alpha_i\}_{i\geq1}$ generates a $1$ -parameter formal deformation of the sub-adjacent Hom-Lie algebra $A^C$ .

4. Abelian extensions of Hom-pre-Lie algebras

In this section, we study abelian extensions of Hom-pre-Lie algebras using the cohomological approach. We show that abelian extensions are classified by the cohomology group $\tilde{H}^2(A; V)$ .

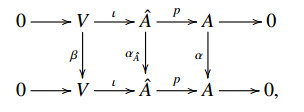
Definition 4.1. Let $(A, \cdot, \alpha)$ and $(V, \cdot_V, \beta)$ be two Hom-pre-Lie algebras. An extension of $(A, \cdot, \alpha)$ by $(V, \cdot_V, \beta)$ is a short exact sequence of Hom-pre-Lie algebra morphisms:

where $(\hat{A}, \cdot_{\hat{A}}, \alpha_{\hat{A}})$ is a Hom-pre-Lie algebra.

It is called an abelian extension if $(V, \cdot_V, \beta)$ is an abelian Hom-pre-Lie algebra, i.e. for all $u, v\in V, u\cdot_V v = 0$ .

Definition 4.2. A section of an extension $(\hat{A}, \cdot_{\hat{A}}, \alpha_{\hat{A}})$ of a Hom-pre-Lie algebra $(A, \cdot, \alpha)$ by $(V, \cdot_V, \beta)$ is a linear map $s:A\longrightarrow \hat{A}$ such that $p\circ s = {\rm{Id}}_A$ .

Let $(\hat{A}, \cdot_{\hat{A}}, \alpha_{\hat{A}})$ be an abelian extension of a Hom-pre-Lie algebra $(A, \cdot, \alpha)$ by $(V, \beta)$ and $s:A\longrightarrow \hat{A}$ a section. For all $x, y\in A$ , define linear maps $\theta:A\otimes A\longrightarrow V$ and $\xi:A\longrightarrow V$ respectively by

$\begin{eqnarray} \theta(x, y)& = &s(x)\cdot_{\hat{A}}s(y)-s(x\cdot y), \end{eqnarray}$

(4.1)

$\begin{eqnarray} \xi(x)& = &\alpha_{\hat{A}}(s(x))-s(\alpha(x)). \end{eqnarray}$

(4.2)

And for all $x, y\in A, u\in V$ , define $\rho, \mu:A\longrightarrow \mathfrak {gl}(V)$ respectively by

$\begin{eqnarray} \rho(x)(u)& = &s(x)\cdot_{\hat{A}} u, \end{eqnarray}$

(4.3)

$\begin{eqnarray} \mu(x)(u)& = &u\cdot_{\hat{A}} s(x). \end{eqnarray}$

(4.4)

Obviously, $\hat{A}$ is isomorphic to $A\oplus V$ as vector spaces. Transfer the Hom-pre-Lie algebra structure on $\hat{A}$ to that on $A\oplus V$ , we obtain a Hom-pre-Lie algebra $(A\oplus V, \diamond, \phi)$ , where $\diamond$ and $\phi$ are given by

$\begin{eqnarray} (x+u)\diamond (y+v)& = &x\cdot y+\theta(x, y)+\rho(x)(v)+\mu(y)(u), \quad \forall \; x, y\in A, u, v\in V, \end{eqnarray}$

(4.5)

$\begin{eqnarray} \phi(x+u)& = &\alpha(x)+\xi(x)+\beta(u), \quad \forall \; x\in A, u\in V. \end{eqnarray}$

(4.6)

Theorem 4.3. With the above notations, we have

${\rm{(i) }}$ $(V, \beta, \rho, \mu)$ is a representation of the Hom-pre-Lie algebra $(A, \cdot, \alpha)$ ,

${\rm{(ii)}}$ $(\theta, \xi)$ is a $2$ -cocycle of the Hom-pre-Lie algebra $(A, \cdot, \alpha)$ with coefficients in the representation $(V, \beta, \rho, \mu)$ .

Proof. For all $x\in A$ , $v\in V$ , by the definition of a Hom-pre-Lie algebra, we have

$\begin{equation} \nonumber\phi(x\diamond v)-\phi(x)\diamond \phi(v) = \beta(\rho(x)(v))-\rho(\alpha(x))\beta(v) = 0, \end{equation}$

which implies that

$\begin{equation} \beta\circ \rho(x) = \rho(\alpha(x))\circ\beta. \end{equation}$

(4.7)

Similarly, we have

$\begin{equation} \beta\circ \mu(x) = \mu(\alpha(x))\circ\beta. \end{equation}$

(4.8)

For all $x, y\in A$ , $v\in V$ , by the definition of a Hom-pre-Lie algebra, we have

$\begin{eqnarray} &&(x\diamond y)\diamond\phi(v)-\phi(x)\diamond (y\diamond v)-(y\diamond x)\diamond\phi(v)+\phi(y)\diamond (x\diamond v)\\ & = &(x\cdot y+\theta(x, y))\diamond \beta(v)-(\alpha(x)+\xi(x))\diamond\rho(y)(v)\\ &&-(y\cdot x+\theta(y, x))\diamond \beta(v)+(\alpha(y)+\xi(y))\diamond\rho(x)(v)\\ & = &\rho(x\cdot y)\beta(v)-\rho(\alpha(x))\rho(y)(v)-\rho(y\cdot x)\beta(v)+\rho(\alpha(y))\rho(x)(v)\\ & = &\rho([x, y]_C)\beta(v)-\rho(\alpha(x))\rho(y)(v)+\rho(\alpha(y))\rho(x)(v)\\ & = &0, \end{eqnarray}$

which implies that

$\begin{equation} \rho([x, y]_C)\circ\beta = \rho(\alpha(x))\circ\rho(y)-\rho(\alpha(y))\circ\rho(x). \end{equation}$

(4.9)

Similarly, we have

$\begin{equation} \mu(\alpha(y))\circ\mu(x)-\mu(x\cdot y)\circ \beta = \mu(\alpha(y))\circ\rho(x)-\rho(\alpha(x))\circ\mu(y). \end{equation}$

(4.10)

By (4.7), (4.8), (4.9), (4.10), we obtain that $(V, \beta, \rho, \mu)$ is a representation.

For all $x, y\in A$ , by the definition of a Hom-pre-Lie algebra, we have

$\begin{eqnarray} &&\phi(x\diamond y)-\phi(x)\diamond \phi(y)\\ & = &\phi\big(x\cdot y+\theta(x, y)\big)-\alpha(x)\cdot \alpha(y)-\theta(\alpha(x), \alpha(y))-\rho(\alpha(x))\xi(y)-\mu(\alpha(y))\xi(x)\\ & = &\xi(x\cdot y)+\beta(\theta(x, y))-\theta(\alpha(x), \alpha(y))-\rho(\alpha(x))\xi(y)-\mu(\alpha(y))\xi(x)\\ & = &\partial_{\omega\alpha}\theta(x, y)+\partial_{\alpha\alpha}\xi(x, y)\\ & = &0, \end{eqnarray}$

which implies that

$\begin{equation} \partial_{\omega\alpha}\theta+\partial_{\alpha\alpha}\xi = 0. \end{equation}$

(4.11)

For all $x, y, z\in A$ , by the definition of a Hom-pre-Lie algebra, we have

$\begin{eqnarray} &&(x\diamond y)\diamond\phi(z)-\phi(x)\diamond (y\diamond z)-(y\diamond x)\diamond\phi(z)+\phi(y)\diamond (x\diamond z)\\ & = &\big(x\cdot y+\theta(x, y)\big)\diamond(\alpha(z)+\xi(z))-(\alpha(x)+\xi(x))\diamond\big(y\cdot z +\theta(y, z)\big)\\ &&-\big(y\cdot x+\theta(y, x)\big)\diamond(\alpha(z)+\xi(z))+(\alpha(y)+\xi(y))\diamond\big(x\cdot z +\theta(x, z)\big)\\ & = &\theta(x\cdot y, \alpha(z))+\mu(\alpha(z))\theta(x, y)-\theta(\alpha(x), y\cdot z)-\rho(\alpha(x))\theta(y, z)\\ &&-\theta(y\cdot x, \alpha(z))-\mu(\alpha(z))\theta(y, x)+\theta(\alpha(y), x\cdot z)+\rho(\alpha(y))\theta(x, z)\\ &&+\rho(x\cdot y)\xi(z)-\mu(y\cdot z)\xi(x)-\rho(y\cdot x)\xi(z)+\mu(x\cdot z)\xi(y)\\ & = &-\partial_{\omega\omega}\theta(x, y, z)-\partial_{\alpha\omega}\xi(x, y, z)\\ & = &0, \end{eqnarray}$

which implies that

$\begin{equation} \partial_{\omega\omega}\theta+\partial_{\alpha\omega}\xi = 0. \end{equation}$

(4.12)

By Eqs (4.11) and (4.12), we obtain that $\tilde{\partial}(\theta, \xi) = 0$ , which implies that $(\theta, \xi)$ is a $2$ -cocycle of the Hom-pre-Lie algebra $(A, \cdot, \alpha)$ . The proof is finished.

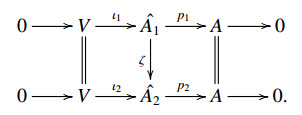
Definition 4.4. Let $(\hat{A_1}, \cdot_{\hat{A_1}}, \alpha_{\hat{A_1}})$ and $(\hat{A_2}, \cdot_{\hat{A_2}}, \alpha_{\hat{A_2}})$ be two abelian extensions of a Hom-pre-Lie algebra $(A, \cdot, \alpha)$ by $(V, \beta)$ . They are said to be isomorphic if there exists a Hom-pre-Lie algebra isomorphism $\zeta:(\hat{A_1}, \cdot_{\hat{A_1}}, \alpha_{\hat{A_1}})\longrightarrow (\hat{A_2}, \cdot_{\hat{A_2}}, \alpha_{\hat{A_2}})$ such that the following diagram is commutative:

Proposition 4.5. With the above notations, we have

${\rm(i)}$ Two different sections of an abelian extension of a Hom-pre-Lie algebra $(A, \cdot, \alpha)$ by $(V, \beta)$ give rise to the same representation of $(A, \cdot, \alpha)$ ,

${\rm(ii)}$ Isomorphic abelian extensions give rise to the same representation of $(A, \cdot, \alpha)$ .

Proof. ${\rm(i)}$ Let $(\hat{A}, \cdot_{\hat{A}}, \alpha_{\hat{A}})$ be an abelian extension of a Hom-pre-Lie algebra $(A, \cdot, \alpha)$ by $(V, \beta)$ . Choosing two different sections $s_1, s_2:A\longrightarrow \hat{A}$ , by equations (4.3), (4.4) and Theorem 4.3, we obtain two representations $(V, \beta, \rho_1, \mu_1)$ and $(V, \beta, \rho_2, \mu_2)$ . Define $\varphi:A\longrightarrow V$ by $\varphi(x) = s_1(x)-s_2(x)$ . Then for all $x\in A$ , we have

$\begin{eqnarray*} \rho_1(x)(u)-\rho_2(x)(u)& = &s_1(x)\cdot_{\hat{A}} u-s_2(x)\cdot_{\hat{A}} u\\ & = &(\varphi(x)+s_2(x))\cdot_{\hat{A}} u-s_2(x)\cdot_{\hat{A}} u\\ & = &\varphi(x)\cdot_{\hat{A}} u\\ & = &0, \end{eqnarray*}$

which implies that $\rho_1 = \rho_2$ . Similarly, we have $\mu_1 = \mu_2$ . This finishes the proof.

${\rm(ii)}$ Let $(\hat{A_1}, \cdot_{\hat{A_1}}, \alpha_{\hat{A_1}})$ and $(\hat{A_2}, \cdot_{\hat{A_2}}, \alpha_{\hat{A_2}})$ are two isomorphic abelian extensions of a Hom-pre-Lie algebra $(A, \cdot, \alpha)$ by $(V, \beta)$ . Let $s_1:A_1\longrightarrow \hat{A_1}$ and $s_2:A_2\longrightarrow \hat{A_2}$ be two sections of $(\hat{A_1}, \cdot_{\hat{A_1}}, \alpha_{\hat{A_1}})$ and $(\hat{A_2}, \cdot_{\hat{A_2}}, \alpha_{\hat{A_2}})$ respectively. By equations (4.3), (4.4) and Theorem 4.3, we obtain that $(V, \beta, \rho_1, \mu_1)$ and $(V, \beta, \rho_2, \mu_2)$ are their representations respectively. Define $s'_1:A_1\longrightarrow \hat{A_1}$ by $s'_1 = \zeta^{-1}\circ s_2$ . Since $\zeta:(\hat{A_1}, \cdot_{\hat{A_1}}, \alpha_{\hat{A_1}})\longrightarrow (\hat{A_2}, \cdot_{\hat{A_2}}, \alpha_{\hat{A_2}})$ is a Hom-pre-Lie algebra isomorphism satisfying the commutative diagram in Definition 4.4, by $p_2\circ \zeta = p_1$ , we have

$\begin{equation} p_1\circ s'_1 = p_2\circ \zeta \circ \zeta^{-1}\circ s_2 = {\rm{Id}}_A. \end{equation}$

(4.13)

Thus, we obtain that $s'_1$ is a section of $(\hat{A_1}, \cdot_{\hat{A_1}}, \alpha_{\hat{A_1}})$ . For all $x\in A, u\in V$ , we have

$\begin{eqnarray*} \rho_1(x)(u)& = &s'_1(x) \cdot_{\hat{A_1}} u\\ & = &(\zeta^{-1}\circ s_2)(x)\cdot_{\hat{A_1}} u\\ & = &\zeta^{-1}(s_2(x) \cdot_{\hat{A_2}} u)\\ & = &\rho_2(x)(u), \end{eqnarray*}$

which implies that $\rho_1 = \rho_2$ . Similarly, we have $\mu_1 = \mu_2$ . This finishes the proof.

So in the sequel, we fix a representation $(V, \beta, \rho, \mu)$ of a Hom-pre-Lie algebra $(A, \cdot, \alpha)$ and consider abelian extensions that induce the given representation.

Theorem 4.6. Abelian extensions of a Hom-pre-Lie algebra $(A, \cdot, \alpha)$ by $(V, \beta)$ are classified by $\tilde{H}^2(A; V)$ .

Proof. Let $(\hat{A}, \cdot_{\hat{A}}, \alpha_{\hat{A}})$ be an abelian extension of a Hom-pre-Lie algebra $(A, \cdot, \alpha)$ by $(V, \beta)$ . Choosing a section $s:A\longrightarrow \hat{A}$ , by Theorem 4.3, we obtain that $(\theta, \xi)\in \tilde{Z}^2(A; V)$ . Now we show that the cohomological class of $(\theta, \xi)$ does not depend on the choice of sections. In fact, let $s_1$ and $s_2$ be two different sections. Define $\varphi:A\longrightarrow V$ by $\varphi(x) = s_1(x)-s_2(x)$ . Then for all $x, y\in A$ , we have

$\begin{eqnarray*} \theta_1(x, y)& = &s_1(x)\cdot_{\hat{A}} s_1(y)-s_1(x\cdot y)\\ & = &\big(s_2(x)+\varphi(x)\big)\cdot_{\hat{A}} \big(s_2(y)+\varphi(y)\big)-s_2(x\cdot y)-\varphi(x\cdot y)\\ & = &s_2(x)\cdot_{\hat{A}} s_2(y)+\rho(x)\varphi(y)+\mu(y)\varphi(x)-s_2(x\cdot y)-\varphi(x\cdot y)\\ & = &\theta_2(x, y)+\partial_{\omega\omega}\varphi(x, y), \end{eqnarray*}$

which implies that $\theta_1-\theta_2 = \partial_{\omega\omega}\varphi$ .

For all $x\in A$ , we have

$\begin{eqnarray*} \xi_1(x)& = &\alpha_{\hat{A}}(s_1(x))-s_1(\alpha(x))\\ & = &\alpha_{\hat{A}}(\varphi(x)+s_2(x))-\varphi(\alpha(x))-s_2(\alpha(x))\\ & = &\alpha_{\hat{A}}(\varphi(x))+\alpha_{\hat{A}}(s_2(x))-\varphi(\alpha(x))-s_2(\alpha(x))\\ & = &\xi_2(x)+\beta(\varphi(x))-\varphi(\alpha(x)), \end{eqnarray*}$

which implies that $\xi_1-\xi_2 = \partial_{\omega\alpha}\varphi$ .

Therefore, we obtain that $(\theta_1-\theta_2, \xi_1-\xi_2)\in \tilde{B}^2(A; V)$ , $(\theta_1, \xi_1)$ and $(\theta_2, \xi_2)$ are in the same cohomological class.

Now we prove that isomorphic abelian extensions give rise to the same element in $\tilde{H}^2(A; V)$ . Assume that $(\hat{A_1}, \cdot_{\hat{A_1}}, \alpha_{\hat{A_1}})$ and $(\hat{A_2}, \cdot_{\hat{A_2}}, \alpha_{\hat{A_2}})$ are two isomorphic abelian extensions of a Hom-pre-Lie algebra $(A, \cdot, \alpha)$ by $(V, \beta)$ , and $\zeta:(\hat{A_1}, \cdot_{\hat{A_1}}, \alpha_{\hat{A_1}})\longrightarrow (\hat{A_2}, \cdot_{\hat{A_2}}, \alpha_{\hat{A_2}})$ is a Hom-pre-Lie algebra isomorphism satisfying the commutative diagram in Definition 4.4. Assume that $s_1:A\longrightarrow \hat{A_1}$ is a section of $\hat{A_1}$ . By $p_2\circ \zeta = p_1$ , we have

$\begin{equation} p_2\circ (\zeta\circ s_1) = p_1\circ s_1 = {\rm{Id}}_A. \end{equation}$

(4.14)

Thus, we obtain that $\zeta\circ s_1$ is a section of $\hat{A_2}$ . Define $s_2 = \zeta\circ s_1$ . Since $\zeta$ is an isomorphism of Hom-pre-Lie algebras and $\zeta\mid_V = {\rm{Id}}_V$ , for all $x, y\in A$ , we have

$\begin{eqnarray*} \theta_2(x, y)& = &s_2(x)\cdot_{\hat{A_2}} s_2(y)-s_2(x\cdot y)\\ & = &(\zeta\circ s_1)(x)\cdot_{\hat{A_2}}(\zeta\circ s_1)(y)-(\zeta\circ s_1)(x\cdot y)\\ & = &\zeta\big(s_1(x)\cdot_{\hat{A_1}} s_1(y)-s_1(x\cdot y)\big)\\ & = &\theta_1(x, y), \end{eqnarray*}$

and

$\begin{eqnarray*} \xi_2(x)& = &\alpha_{\hat{A_2}}(s_2(x))-s_2(\alpha(x))\\ & = &\alpha_{\hat{A_2}}(\zeta(s_1(x)))-\zeta(s_1(\alpha(x)))\\ & = &\zeta(\alpha_{\hat{A_1}}(s_1(x))-s_1(\alpha(x)))\\ & = &\xi_1(x). \end{eqnarray*}$

Thus, isomorphic abelian extensions gives rise to the same element in $\tilde{H}^2(A; V)$ .

Conversely, given two 2-cocycles $(\theta_1, \xi_1)$ and $(\theta_2, \xi_2)$ , by Eqs (4.5) and (4.6), we can construct two abelian extensions $(A\oplus V, \diamond_1, \phi_1)$ and $(A\oplus V, \diamond_2, \phi_2)$ . If $(\theta_1, \xi_1), (\theta_2, \xi_2)\in \tilde{H}^2(A; V)$ , then there exists $\varphi:A\longrightarrow V$ , such that $\theta_1 = \theta_2+\partial_{\omega\omega}\varphi$ and $\xi_1 = \xi_2+\partial_{\omega\alpha}\varphi$ . We define $\zeta:A\oplus V\longrightarrow A\oplus V$ by

$\begin{equation} \zeta(x+u) = x+u+\varphi(x), \quad \forall \; x\in A, u\in V. \end{equation}$

(4.15)

For all $x, y\in A, u, v\in V$ , by $\theta_1 = \theta_2+\partial_{\omega\omega}\varphi$ , we have

$\begin{eqnarray} &&\zeta\big((x+u)\diamond_1(y+v)\big)- \zeta(x+u)\diamond_2\zeta(y+v)\\ & = &\zeta\big(x\cdot y+\theta_1(x, y)+\rho(x)(v)+\mu(y)(u)\big)-\big(x+u+\varphi(x)\big)\diamond_2 \big(y+v+\varphi(y)\big)\\ & = &\theta_1(x, y)+\varphi(x\cdot y)-\theta_2(x, y)-\rho(x)\varphi(y)-\mu(y)\varphi(x)\\ & = &\theta_1(x, y)-\theta_2(x, y)-\partial_{\omega\omega}\varphi(x, y)\\ & = &0, \end{eqnarray}$

(4.16)

and for all $x\in A, u\in V$ , by $\xi_1 = \xi_2+\partial_{\omega\alpha}\varphi$ , we have

$\begin{eqnarray} &&\zeta \circ\phi_1(x+u)-\phi_1\circ \zeta(x+u)\\ & = &\zeta\big(\alpha(x)+\xi_1(x)+\beta(u)\big)-\phi_2\big(x+u+\varphi(x)\big)\\ & = &\xi_1(x)+\varphi(\alpha(x))-\xi_2(x)-\beta(\varphi(x))\\ & = &\xi_1(x)-\xi_2(x)-\partial_{\omega\alpha}\varphi(x)\\ & = &0, \end{eqnarray}$

(4.17)

which implies that $\zeta$ is a Hom-pre-Lie algebra isomorphism from $(A\oplus V, \diamond_1, \phi_1)$ to $(A\oplus V, \diamond_2, \phi_2)$ . Moreover, it is obvious that the diagram in Definition 4.4 is commutative. This finishes the proof.

Appendix: The proof of Lemma 2.6

By straightforward computations, for all $x_1, \dots, x_{n+2}\in A$ , we have

$\begin{eqnarray} &&\partial_{\omega\omega}(\partial_{\omega\omega}\varphi)(x_1, \dots, x_{n+2})\\ & = &\sum\limits_{i = 1}^{n+1}(-1)^{i+1}\rho(\alpha^n(x_i))(\partial_{\omega\omega}\varphi)(x_1, \dots, \widehat{x_i}, \dots, x_{n+2})\\ &&+\sum\limits_{i = 1}^{n+1}(-1)^{i+1}\mu(\alpha^n(x_{n+2}))(\partial_{\omega\omega}\varphi)(x_1, \dots, \widehat{x_i}, \dots, x_{n+1}, x_i)\\ &&-\sum\limits_{i = 1}^{n+1}(-1)^{i+1} (\partial_{\omega\omega}\varphi)(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \alpha(x_{n+1}), x_i\cdot x_{n+2})\\ &&+\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j} (\partial_{\omega\omega}\varphi)([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+2}))\\ & = &\sum\limits_{1\leq j < i\leq n+1}(-1)^{i+1}(-1)^{j+1}\rho(\alpha^n(x_i))\rho(\alpha^{n-1}(x_j))\varphi(x_1, \dots, \widehat{x_j}, \dots, \widehat{x_i}, \dots, x_{n+2}) \end{eqnarray}$

(4.18)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+1}(-1)^j\rho(\alpha^n(x_i))\rho(\alpha^{n-1}(x_j))\varphi(x_1, \dots, \widehat{x_i}, \dots, \widehat{x_j}, \dots, x_{n+2}) \end{eqnarray}$

(4.19)

$\begin{eqnarray} &&+\sum\limits_{1\leq j < i\leq n+1}(-1)^{i+1}(-1)^{j+1}\rho(\alpha^n(x_i))\mu(\alpha^{n-1}(x_{n+2}))\varphi(x_1, \dots, \widehat{x_j}, \dots, \widehat{x_i}, \dots, x_{n+1}, x_j) \end{eqnarray}$

(4.20)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+1}(-1)^j\rho(\alpha^n(x_i))\mu(\alpha^{n-1}(x_{n+2}))\varphi(x_1, \dots, \widehat{x_i}, \dots, \widehat{x_j}, \dots, x_{n+1}, x_j) \end{eqnarray}$

(4.21)

$\begin{eqnarray} &&-\sum\limits_{1\leq j < i\leq n+1}(-1)^{i+1}(-1)^{j+1}\rho(\alpha^n(x_i))\varphi(\alpha(x_1), \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_i)}, \dots, \alpha(x_{n+1}), x_j\cdot x_{n+2}) \end{eqnarray}$

(4.22)

$\begin{eqnarray} &&-\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+1}(-1)^j\rho(\alpha^n(x_i))\varphi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), x_j\cdot x_{n+2}) \end{eqnarray}$

(4.23)

$+\sum\limits_{1\leq j < k < i\leq n+1}(-1)^{i+1}(-1)^{j+k}\rho(\alpha^n(x_i))\varphi([x_j, x_k]_C, \alpha(x_1), \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_i)}, \dots, \alpha(x_{n+2}))$

(4.24)

$\begin{eqnarray} &&+\sum\limits_{1\leq j < i < k\leq n+1}(-1)^{i+1}(-1)^{j+k-1}\rho(\alpha^n(x_i))\varphi([x_j, x_k]_C, \alpha(x_1), \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_k)}, \dots, \alpha(x_{n+2})) \end{eqnarray}$

(4.25)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+1}(-1)^{j+k}\rho(\alpha^n(x_i))\varphi([x_j, x_k]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_k)}, \dots, \alpha(x_{n+2})) \end{eqnarray}$

(4.26)

$\begin{eqnarray} &&+\sum\limits_{1\leq j < i\leq n+1}(-1)^{i+1}(-1)^{j+1}\mu(\alpha^n(x_{n+2}))\rho(\alpha^{n-1}(x_j))\varphi(x_1, \dots, \widehat{x_j}, \dots, \widehat{x_i}, \dots, x_{n+1}, x_i) \end{eqnarray}$

(4.27)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+1}(-1)^j\mu(\alpha^n(x_{n+2}))\rho(\alpha^{n-1}(x_j))\varphi(x_1, \dots, \widehat{x_i}, \dots, \widehat{x_j}, \dots, x_{n+1}, x_i) \end{eqnarray}$

(4.28)

$\begin{eqnarray} &&+\sum\limits_{1\leq j < i\leq n+1}(-1)^{i+1}(-1)^{j+1}\mu(\alpha^n(x_{n+2}))\mu(\alpha^{n-1}(x_i))\varphi(x_1, \dots, \widehat{x_j}, \dots, \widehat{x_i}, \dots, x_{n+1}, x_j) \end{eqnarray}$

(4.29)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+1}(-1)^j\mu(\alpha^n(x_{n+2}))\mu(\alpha^{n-1}(x_i))\varphi(x_1, \dots, \widehat{x_i}, \dots, \widehat{x_j}, \dots, x_{n+1}, x_j) \end{eqnarray}$

(4.30)

$\begin{eqnarray} &&-\sum\limits_{1\leq j < i\leq n+1}(-1)^{i+1}(-1)^{j+1}\mu(\alpha^n(x_{n+2}))\varphi(\alpha(x_1), \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_i)}, \dots, \alpha(x_{n+1}), x_j\cdot x_i) \end{eqnarray}$

(4.31)

$\begin{eqnarray} &&-\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+1}(-1)^j\mu(\alpha^n(x_{n+2}))\varphi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), x_j\cdot x_i) \end{eqnarray}$

(4.32)

$\begin{eqnarray} &&+\sum\limits_{1\leq j < k < i\leq n+1}(-1)^{i+1}(-1)^{j+k}\mu(\alpha^n(x_{n+2}))\varphi([x_j, x_k]_C, \alpha(x_1), \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_k)}, \end{eqnarray}$

(4.33)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_i)}, \dots, \alpha(x_{n+1}), \alpha(x_i))\\ &&+\sum\limits_{1\leq j < i < k\leq n+1}(-1)^{i+1}(-1)^{j+k-1}\mu(\alpha^n(x_{n+2}))\varphi([x_j, x_k]_C, \alpha(x_1), \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_i)}, \end{eqnarray}$

(4.34)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_k)}, \dots, \alpha(x_{n+1}), \alpha(x_i))\\ &&+\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+1}(-1)^{j+k}\mu(\alpha^n(x_{n+2}))\varphi([x_j, x_k]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \end{eqnarray}$

(4.35)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_k)}, \dots, \alpha(x_{n+1}), \alpha(x_i))\\ &&-\sum\limits_{1\leq j < i\leq n+1}(-1)^{i+1}(-1)^{j+1}\rho(\alpha^n(x_j))\varphi(\alpha(x_1), \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_i)}, \dots, \alpha(x_{n+1}), x_i\cdot x_{n+2}) \end{eqnarray}$

(4.36)

$\begin{eqnarray} &&-\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+1}(-1)^j\rho(\alpha^n(x_j))\varphi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), x_i\cdot x_{n+2}) \end{eqnarray}$

(4.37)

$\begin{eqnarray} &&-\sum\limits_{1\leq j < i\leq n+1}(-1)^{i+1}(-1)^{j+1}\mu(\alpha^{n-1}(x_i \cdot x_{n+2}))\varphi(\alpha(x_1), \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_i)}, \dots, \alpha(x_{n+1}), \alpha(x_j)) \end{eqnarray}$

(4.38)

$\begin{eqnarray} &&-\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+1}(-1)^j\mu(\alpha^{n-1}(x_i \cdot x_{n+2}))\varphi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), \alpha(x_j)) \end{eqnarray}$

(4.39)

$\begin{eqnarray} &&+\sum\limits_{1\leq j < i\leq n+1}(-1)^{i+1}(-1)^{j+1}\varphi(\alpha^2(x_1), \dots, \widehat{\alpha^2(x_j)}, \dots, \widehat{\alpha^2(x_i)}, \dots, \alpha^2(x_{n+1}), \alpha(x_j)\cdot(x_i\cdot x_{n+2})) \end{eqnarray}$

(4.40)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+1}(-1)^j\varphi(\alpha^2(x_1), \dots, \widehat{\alpha^2(x_i)}, \dots, \widehat{\alpha^2(x_j)}, \dots, \alpha^2(x_{n+1}), \alpha(x_j)\cdot(x_i\cdot x_{n+2})) \end{eqnarray}$

(4.41)

$\begin{eqnarray} &&-\sum\limits_{1\leq j < k < i\leq n+1}(-1)^{i+1}(-1)^{j+k}\varphi([\alpha(x_j), \alpha(x_k)]_C, \alpha^2(x_1), \dots, \widehat{\alpha^2(x_j)}, \dots, \widehat{\alpha^2(x_k)}, \dots, \end{eqnarray}$

(4.42)

$\begin{eqnarray} &&\widehat{\alpha^2(x_i)}, \dots, \alpha^2(x_{n+1}), \alpha(x_i \cdot x_{n+2}))\\ &&-\sum\limits_{1\leq j < i < k\leq n+1}(-1)^{i+1}(-1)^{j+k-1}\varphi([\alpha(x_j), \alpha(x_k)]_C, \alpha^2(x_1), \dots, \widehat{\alpha^2(x_j)}, \dots, \widehat{\alpha^2(x_i)}, \dots, \end{eqnarray}$

(4.43)

$\begin{eqnarray} &&\widehat{\alpha^2(x_k)}, \dots, \alpha^2(x_{n+1}), \alpha(x_i \cdot x_{n+2}))\\ &&-\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+1}(-1)^{j+k}\varphi([\alpha(x_j), \alpha(x_k)]_C, \alpha^2(x_1), \dots, \widehat{\alpha^2(x_i)}, \dots, \widehat{\alpha^2(x_j)}, \dots, \end{eqnarray}$

(4.44)

$\begin{eqnarray} &&\widehat{\alpha^2(x_k)}, \dots, \alpha^2(x_{n+1}), \alpha(x_i \cdot x_{n+2}))\\ &&+\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j}\rho(\alpha^{n-1}[x_i, x_j]_C)\varphi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+2})) \end{eqnarray}$

(4.45)

$\begin{eqnarray} &&+\sum\limits_{1\leq k < i < j\leq n+1}(-1)^{i+j}(-1)^k\rho(\alpha^n(x_k)))\varphi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+2})) \end{eqnarray}$

(4.46)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < k < j\leq n+1}(-1)^{i+j}(-1)^{k+1}\rho(\alpha^n(x_k)))\varphi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+2})) \end{eqnarray}$

(4.47)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+j}(-1)^k\rho(\alpha^n(x_k)))\varphi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_k)}, \dots, \alpha(x_{n+2})) \end{eqnarray}$

(4.48)

$\begin{eqnarray} &&+\sum\limits_{1\leq k < i < j\leq n+1}(-1)^{i+j}(-1)^k\mu(\alpha^n(x_{n+2})))\varphi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_i)}, \dots, \end{eqnarray}$

(4.49)

$\begin{eqnarray} &&\widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), \alpha(x_k))\\ &&+\sum\limits_{1\leq i < k < j\leq n+1}(-1)^{i+j}(-1)^{k+1}\mu(\alpha^n(x_{n+2})))\varphi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_k)}, \dots, \end{eqnarray}$

(4.50)

$\begin{eqnarray} &&\widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), \alpha(x_k))\\ &&+\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+j}(-1)^k\mu(\alpha^n(x_{n+2})))\varphi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \end{eqnarray}$

(4.51)

$\begin{eqnarray} &&\widehat{\alpha(x_k)}, \dots, \alpha(x_{n+1}), \alpha(x_k))\\ &&+\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j}\mu(\alpha^n(x_{n+2}))\varphi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), [x_i, x_j]_C) \end{eqnarray}$

(4.52)

$\begin{eqnarray} &&-\sum\limits_{1\leq k < i < j\leq n+1}(-1)^{i+j}(-1)^k\varphi(\alpha[x_i, x_j]_C, \alpha^2(x_1), \dots, \widehat{\alpha^2(x_k)}, \dots, \widehat{\alpha^2(x_i)}, \dots, \widehat{\alpha^2(x_j)}, \end{eqnarray}$

(4.53)

$\begin{eqnarray} &&\dots, \alpha^2(x_{n+1}), \alpha(x_k) \cdot \alpha(x_{n+2}))\\ &&-\sum\limits_{1\leq i < k < j\leq n+1}(-1)^{i+j}(-1)^{k+1}\varphi(\alpha[x_i, x_j]_C, \alpha^2(x_1), \dots, \widehat{\alpha^2(x_i)}, \dots, \widehat{\alpha^2(x_k)}, \dots, \widehat{\alpha^2(x_j)}, \end{eqnarray}$

(4.54)

$\begin{eqnarray} &&\dots, \alpha^2(x_{n+1}), \alpha(x_k) \cdot \alpha(x_{n+2}))\\ &&-\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+j}(-1)^k\varphi(\alpha[x_i, x_j]_C, \alpha^2(x_1), \dots, \widehat{\alpha^2(x_i)}, \dots, \widehat{\alpha^2(x_j)}, \dots, \widehat{\alpha^2(x_k)}, \end{eqnarray}$

(4.55)

$\begin{eqnarray} &&\dots, \alpha^2(x_{n+1}), \alpha(x_k) \cdot \alpha(x_{n+2}))\\ &&-\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j}\varphi(\alpha^2(x_1), \dots, \widehat{\alpha^2(x_i)}, \dots, \widehat{\alpha^2(x_j)}, \dots, \alpha^2(x_{n+1}), [x_i, x_j]_C \cdot \alpha(x_{n+2})) \end{eqnarray}$

(4.56)

$\begin{eqnarray} &&+\sum\limits_{1\leq k < l < i < j\leq n+1}(-1)^{i+j}(-1)^{k+l}\varphi([\alpha(x_k), \alpha(x_l)]_C, \alpha[x_i, x_j]_C, \alpha^2(x_1), \dots, \widehat{\alpha^2(x_k)}, \dots, \end{eqnarray}$

(4.57)

$\begin{eqnarray} &&\widehat{\alpha^2(x_l)}, \dots, \widehat{\alpha^2(x_i)}, \dots, \widehat{\alpha^2(x_j)}, \dots, \alpha^2(x_{n+2}))\\ &&+\sum\limits_{1\leq k < i < l < j\leq n+1}(-1)^{i+j}(-1)^{k+l+1}\varphi([\alpha(x_k), \alpha(x_l)]_C, \alpha[x_i, x_j]_C, \alpha^2(x_1), \dots, \widehat{\alpha^2(x_k)}, \dots, \end{eqnarray}$

(4.58)

$\begin{eqnarray} &&\widehat{\alpha^2(x_i)}, \dots, \widehat{\alpha^2(x_l)}, \dots, \widehat{\alpha^2(x_j)}, \dots, \alpha^2(x_{n+2}))\\ &&+\sum\limits_{1\leq i < k < l < j\leq n+1}(-1)^{i+j}(-1)^{k+l}\varphi([\alpha(x_k), \alpha(x_l)]_C, \alpha[x_i, x_j]_C, \alpha^2(x_1), \dots, \widehat{\alpha^2(x_i)}, \dots, \end{eqnarray}$

(4.59)

$\begin{eqnarray} &&\widehat{\alpha^2(x_k)}, \dots, \widehat{\alpha^2(x_l)}, \dots, \widehat{\alpha^2(x_j)}, \dots, \alpha^2(x_{n+2}))\\ &&+\sum\limits_{1\leq i < j < k < l\leq n+1}(-1)^{i+j}(-1)^{k+l}\varphi([\alpha(x_k), \alpha(x_l)]_C, \alpha[x_i, x_j]_C, \alpha^2(x_1), \dots, \widehat{\alpha^2(x_i)}, \dots, \end{eqnarray}$

(4.60)

$\begin{eqnarray} &&\widehat{\alpha^2(x_j)}, \dots, \widehat{\alpha^2(x_k)}, \dots, \widehat{\alpha^2(x_l)}, \dots, \alpha^2(x_{n+2}))\\ &&+\sum\limits_{1\leq i < k < j < l\leq n+1}(-1)^{i+j}(-1)^{k+l+1}\varphi([\alpha(x_k), \alpha(x_l)]_C, \alpha[x_i, x_j]_C, \alpha^2(x_1), \dots, \widehat{\alpha^2(x_i)}, \dots, \end{eqnarray}$

(4.61)

$\begin{eqnarray} &&\widehat{\alpha^2(x_k)}, \dots, \widehat{\alpha^2(x_j)}, \dots, \widehat{\alpha^2(x_l)}, \dots, \alpha^2(x_{n+2}))\\ &&+\sum\limits_{1\leq k < i < j < l\leq n+1}(-1)^{i+j}(-1)^{k+l}\varphi([\alpha(x_k), \alpha(x_l)]_C, \alpha[x_i, x_j]_C, \alpha^2(x_1), \dots, \widehat{\alpha^2(x_k)}, \dots, \end{eqnarray}$

(4.62)

$\begin{eqnarray} &&\widehat{\alpha^2(x_i)}, \dots, \widehat{\alpha^2(x_j)}, \dots, \widehat{\alpha^2(x_l)}, \dots, \alpha^2(x_{n+2}))\\ &&+\sum\limits_{1\leq k < i < j\leq n+1}(-1)^{i+j}(-1)^k\varphi([[x_i, x_j]_C, \alpha(x_k)]_C, \alpha^2(x_1), \dots, \widehat{\alpha^2(x_k)}, \dots, \widehat{\alpha^2(x_i)}, \dots, \widehat{\alpha^2(x_j)}, \dots, \alpha^2(x_{n+2})) \end{eqnarray}$

(4.63)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < k < j\leq n+1}(-1)^{i+j}(-1)^{k+1}\varphi([[x_i, x_j]_C, \alpha(x_k)]_C, \alpha^2(x_1), \dots, \widehat{\alpha^2(x_i)}, \dots, \widehat{\alpha^2(x_k)}, \dots, \widehat{\alpha^2(x_j)}, \dots, \alpha^2(x_{n+2})) \end{eqnarray}$

(4.64)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+j}(-1)^k\varphi([[x_i, x_j]_C, \alpha(x_k)]_C, \alpha^2(x_1), \dots, \widehat{\alpha^2(x_i)}, \dots, \widehat{\alpha^2(x_j)}, \dots, \widehat{\alpha^2(x_k)}, \dots, \alpha^2(x_{n+2})). \end{eqnarray}$

(4.65)

The terms (4.22) and (4.37), (4.23) and (4.36), (4.24) and (4.48), (4.25) and (4.47), (4.26) and (4.46), (4.33) and (4.51), (4.34) and (4.50), (4.35) and (4.49) cancel each other. By the definition of the sub-adjacent Hom-Lie algebra, the sum of (4.63), (4.64) and (4.65) is zero. By the antisymmetry condition, the term (4.57) and (4.60), (4.58) and (4.61), (4.59) and (4.62) cancel each other. By the definition of the sub-adjacent Lie bracket, the sum of (4.31), (4.32) and (4.52) is zero. By the definition of Hom-pre-Lie algebras, the sum of (4.40), (4.41) and (4.56) is zero. Since $\alpha$ is an algebra morphism, the term (4.42) and (4.55), (4.43) and (4.54), (4.44) and (4.53) cancel each other.

Since $(V, \beta, \rho, \mu)$ is a representation of the Hom-pre-Lie algebra $(A, \cdot, \alpha)$ , the sum of (4.18) and (4.19) can be written as

$\begin{equation} \sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j+1}\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C)\beta\varphi(x_1, \dots, \widehat{x_i}, \dots, \widehat{x_j}, \dots, x_{n+2}), \end{equation}$

(4.66)

the sum of (4.20), (4.28) and (4.29) can be written as

$\begin{equation} -\sum\limits_{1\leq j < i\leq n+1}(-1)^{i+j+1}\mu(\alpha^{n-1}(x_i) \cdot\alpha^{n-1}(x_{n+2}))\beta\varphi(x_1, \dots, \widehat{x_j}, \dots, \widehat{x_i}, \dots, x_{n+1}, x_j), \end{equation}$

(4.67)

and the sum of (4.21), (4.27) and (4.30) can be written as

$\begin{equation} \sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j+1}\mu(\alpha^{n-1}(x_i) \cdot\alpha^{n-1}(x_{n+2}))\beta\varphi(x_1, \dots, \widehat{x_i}, \dots, \widehat{x_j}, \dots, x_{n+1}, x_j). \end{equation}$

(4.68)

Thus, we have

$\begin{eqnarray*} \nonumber&&\partial_{\omega\omega}(\partial_{\omega\omega}\varphi)(x_1, \dots, x_{n+2})\\ & = &\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j+1}\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C)\beta\varphi(x_1, \dots, \widehat{x_i}, \dots, \widehat{x_j}, \dots, x_{n+2}), \\ &&+\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j+1}\mu(\alpha^{n-1}(x_i) \cdot\alpha^{n-1}(x_{n+2}))\beta\varphi(x_1, \dots, \widehat{x_i}, \dots, \widehat{x_j}, \dots, x_{n+1}, x_j)\\ &&-\sum\limits_{1\leq j < i\leq n+1}(-1)^{i+j+1}\mu(\alpha^{n-1}(x_i) \cdot\alpha^{n-1}(x_{n+2}))\beta\varphi(x_1, \dots, \widehat{x_j}, \dots, \widehat{x_i}, \dots, x_{n+1}, x_j)\\ &&-\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j+1}\rho(\alpha^{n-1}[x_i, x_j]_C)\varphi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+2}))\\ &&-\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j+1}\mu(\alpha^{n-1}(x_i \cdot x_{n+2}))\varphi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), \alpha(x_j))\\ &&+\sum\limits_{1\leq j < i\leq n+1}(-1)^{i+j+1}\mu(\alpha^{n-1}(x_i \cdot x_{n+2}))\varphi(\alpha(x_1), \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_i)}, \dots, \alpha(x_{n+1}), \alpha(x_j)). \end{eqnarray*}$

For all $x_1, \dots, x_{n+2}\in A$ , we have

$\begin{eqnarray*} &&\partial_{\alpha\omega}(\partial_{\omega\alpha}\varphi)(x_1, \dots, x_{n+2})\\ & = &\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j}\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C) (\partial_{\omega\alpha}\varphi)(x_1, \dots, \hat{x_i}, \dots, \hat{x_j}, \dots, x_{n+2})\\ &&+\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j}\mu(\alpha^{n-1}(x_i)\cdot\alpha^{n-1}(x_{n+2}))(\partial_{\omega\alpha}\varphi)(x_1, \dots, \hat{x_i}, \dots, \hat{x_j}, \dots, x_{n+1}, x_j)\\ &&-\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j}\mu(\alpha^{n-1}(x_j)\cdot\alpha^{n-1}(x_{n+2}))(\partial_{\omega\alpha}\varphi)(x_1, \dots, \hat{x_i}, \dots, \hat{x_j}, \dots, x_{n+1}, x_i)\\ & = &\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j}\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C)\beta\varphi(x_1, \dots, \widehat{x_i}, \dots, \widehat{x_j}, \dots, x_{n+2}), \\ &&-\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j}\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C)\varphi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+2}))\\ &&+\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j}\mu(\alpha^{n-1}(x_i) \cdot\alpha^{n-1}(x_{n+2}))\beta\varphi(x_1, \dots, \widehat{x_i}, \dots, \widehat{x_j}, \dots, x_{n+1}, x_j)\\ &&-\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j}\mu(\alpha^{n-1}(x_i) \cdot\alpha^{n-1}(x_{n+2}))\varphi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), \alpha(x_j))\\ &&-\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j}\mu(\alpha^{n-1}(x_j) \cdot\alpha^{n-1}(x_{n+2}))\beta\varphi(x_1, \dots, \widehat{x_i}, \dots, \widehat{x_j}, \dots, x_{n+1}, x_i)\\ &&+\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j}\mu(\alpha^{n-1}(x_j) \cdot\alpha^{n-1}(x_{n+2}))\varphi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), \alpha(x_i)).\\ \end{eqnarray*}$

Since $\alpha$ is an algebra morphism, we obtain that $\partial_{\omega\omega}\circ \partial_{\omega\omega}+\partial_{\alpha\omega}\circ \partial_{\omega\alpha}$ = 0.

For all $x_1, \dots, x_{n+2}\in A$ , we have

$\begin{eqnarray} &&\partial_{\omega\omega}(\partial_{\alpha\omega}\psi)(x_1, \dots, x_{n+2})\\ & = &\sum\limits_{i = 1}^{n+1}(-1)^{i+1}\rho(\alpha^n(x_i))(\partial_{\alpha\omega}\psi)(x_1, \dots, \widehat{x_i}, \dots, x_{n+2})\\ &&+\sum\limits_{i = 1}^{n+1}(-1)^{i+1}\mu(\alpha^n(x_{n+2}))(\partial_{\alpha\omega}\psi)(x_1, \dots, \widehat{x_i}, \dots, x_{n+1}, x_i)\\ &&-\sum\limits_{i = 1}^{n+1}(-1)^{i+1}(\partial_{\alpha\omega}\psi)(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \alpha(x_{n+1}), x_i\cdot x_{n+2})\\ &&+\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j} (\partial_{\alpha\omega}\psi)([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+2}))\\ & = &\sum\limits_{1\leq j < k < i\leq n+1}(-1)^{i+1}(-1)^{j+k}\rho(\alpha^n(x_i))\rho([\alpha^{n-2}(x_j), \alpha^{n-2}(x_k)]_C)\psi(x_1, \dots, \hat{x_j}, \dots, \hat{x_k} \dots, \hat{x_i}, \dots, x_{n+2}) \end{eqnarray}$

(4.69)

$\begin{eqnarray} &&+\sum\limits_{1\leq j < i < k\leq n+1}(-1)^{i+1}(-1)^{j+k-1}\rho(\alpha^n(x_i))\rho([\alpha^{n-2}(x_j), \alpha^{n-2}(x_k)]_C)\psi(x_1, \dots, \hat{x_j}, \dots, \hat{x_i} \dots, \hat{x_k}, \dots, x_{n+2}) \end{eqnarray}$

(4.70)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+1}(-1)^{j+k}\rho(\alpha^n(x_i))\rho([\alpha^{n-2}(x_j), \alpha^{n-2}(x_k)]_C)\psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_j} \dots, \hat{x_k}, \dots, x_{n+2}) \end{eqnarray}$

(4.71)

$\begin{eqnarray} &&+\sum\limits_{1\leq j < k < i\leq n+1}(-1)^{i+1}(-1)^{j+k}\rho(\alpha^n(x_i))\mu(\alpha^{n-2}(x_j)\cdot\alpha^{n-2}(x_{n+2}))\psi(x_1, \dots, \hat{x_j}, \dots, \hat{x_k} \dots, \hat{x_i}, \dots, x_{n+1}, x_k) \end{eqnarray}$

(4.72)

$\begin{eqnarray} &&+\sum\limits_{1\leq j < i < k\leq n+1}(-1)^{i+1}(-1)^{j+k-1}\rho(\alpha^n(x_i))\mu(\alpha^{n-2}(x_j)\cdot\alpha^{n-2}(x_{n+2}))\psi(x_1, \dots, \hat{x_j}, \dots, \hat{x_i} \dots, \hat{x_k}, \dots, x_{n+1}, x_k) \end{eqnarray}$

(4.73)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+1}(-1)^{j+k}\rho(\alpha^n(x_i))\mu(\alpha^{n-2}(x_j)\cdot\alpha^{n-2}(x_{n+2}))\psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_j} \dots, \hat{x_k}, \dots, x_{n+1}, x_k) \end{eqnarray}$

(4.74)

$\begin{eqnarray} &&-\sum\limits_{1\leq j < k < i\leq n+1}(-1)^{i+1}(-1)^{j+k}\rho(\alpha^n(x_i))\mu(\alpha^{n-2}(x_k)\cdot\alpha^{n-2}(x_{n+2}))\psi(x_1, \dots, \hat{x_j}, \dots, \hat{x_k} \dots, \hat{x_i}, \dots, x_{n+1}, x_j) \end{eqnarray}$

(4.75)

$\begin{eqnarray} &&-\sum\limits_{1\leq j < i < k\leq n+1}(-1)^{i+1}(-1)^{j+k-1}\rho(\alpha^n(x_i))\mu(\alpha^{n-2}(x_k)\cdot\alpha^{n-2}(x_{n+2}))\psi(x_1, \dots, \hat{x_j}, \dots, \hat{x_i} \dots, \hat{x_k}, \dots, x_{n+1}, x_j) \end{eqnarray}$

(4.76)

$\begin{eqnarray} &&-\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+1}(-1)^{j+k}\rho(\alpha^n(x_i))\mu(\alpha^{n-2}(x_k)\cdot\alpha^{n-2}(x_{n+2}))\psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_j} \dots, \hat{x_k}, \dots, x_{n+1}, x_j) \end{eqnarray}$

(4.77)

$\begin{eqnarray} &&+\sum\limits_{1\leq j < k < i\leq n+1}(-1)^{i+1}(-1)^{j+k}\mu(\alpha^n(x_{n+2}))\rho([\alpha^{n-2}(x_j), \alpha^{n-2}(x_k)]_C)\psi(x_1, \dots, \hat{x_j}, \dots, \hat{x_k} \dots, \hat{x_i}, \dots, x_{n+1}, x_i) \end{eqnarray}$

(4.78)

$\begin{eqnarray} &&+\sum\limits_{1\leq j < i < k\leq n+1}(-1)^{i+1}(-1)^{j+k-1}\mu(\alpha^n(x_{n+2}))\rho([\alpha^{n-2}(x_j), \alpha^{n-2}(x_k)]_C)\psi(x_1, \dots, \hat{x_j}, \dots, \hat{x_i} \dots, \hat{x_k}, \dots, x_{n+1}, x_i) \end{eqnarray}$

(4.79)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+1}(-1)^{j+k}\mu(\alpha^n(x_{n+2}))\rho([\alpha^{n-2}(x_j), \alpha^{n-2}(x_k)]_C)\psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_j} \dots, \hat{x_k}, \dots, x_{n+1}, x_i) \end{eqnarray}$

(4.80)

$\begin{eqnarray} &&+\sum\limits_{1\leq j < k < i\leq n+1}(-1)^{i+1}(-1)^{j+k}\mu(\alpha^n(x_{n+2}))\mu(\alpha^{n-2}(x_j)\cdot\alpha^{n-2}(x_i))\psi(x_1, \dots, \hat{x_j}, \dots, \hat{x_k} \dots, \hat{x_i}, \dots, x_{n+1}, x_k) \end{eqnarray}$

(4.81)

$\begin{eqnarray} &&+\sum\limits_{1\leq j < i < k\leq n+1}(-1)^{i+1}(-1)^{j+k-1}\mu(\alpha^n(x_{n+2}))\mu(\alpha^{n-2}(x_j)\cdot\alpha^{n-2}(x_i))\psi(x_1, \dots, \hat{x_j}, \dots, \hat{x_i} \dots, \hat{x_k}, \dots, x_{n+1}, x_k) \end{eqnarray}$

(4.82)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+1}(-1)^{j+k}\mu(\alpha^n(x_{n+2}))\mu(\alpha^{n-2}(x_j)\cdot\alpha^{n-2}(x_i))\psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_j} \dots, \hat{x_k}, \dots, x_{n+1}, x_k) \end{eqnarray}$

(4.83)

$\begin{eqnarray} &&-\sum\limits_{1\leq j < k < i\leq n+1}(-1)^{i+1}(-1)^{j+k}\mu(\alpha^n(x_{n+2}))\mu(\alpha^{n-2}(x_k)\cdot\alpha^{n-2}(x_i))\psi(x_1, \dots, \hat{x_j}, \dots, \hat{x_k} \dots, \hat{x_i}, \dots, x_{n+1}, x_j) \end{eqnarray}$

(4.84)

$\begin{eqnarray} &&-\sum\limits_{1\leq j < i < k\leq n+1}(-1)^{i+1}(-1)^{j+k-1}\mu(\alpha^n(x_{n+2}))\mu(\alpha^{n-2}(x_k)\cdot\alpha^{n-2}(x_i))\psi(x_1, \dots, \hat{x_j}, \dots, \hat{x_i} \dots, \hat{x_k}, \dots, x_{n+1}, x_j) \end{eqnarray}$

(4.85)

$\begin{eqnarray} &&-\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+1}(-1)^{j+k}\mu(\alpha^n(x_{n+2}))\mu(\alpha^{n-2}(x_k)\cdot\alpha^{n-2}(x_i))\psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_j} \dots, \hat{x_k}, \dots, x_{n+1}, x_j) \end{eqnarray}$

(4.86)

$\begin{eqnarray} &&-\sum\limits_{1\leq j < k < i\leq n+1}(-1)^{i+1}(-1)^{j+k}\rho([\alpha^{n-1}(x_j), \alpha^{n-1}(x_k)]_C)\psi(\alpha(x_1), \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_k)}, \end{eqnarray}$

(4.87)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_i)}, \dots, \alpha(x_{n+1}), x_i\cdot x_{n+2})\\ &&-\sum\limits_{1\leq j < i < k\leq n+1}(-1)^{i+1}(-1)^{j+k-1}\rho([\alpha^{n-1}(x_j), \alpha^{n-1}(x_k)]_C)\psi(\alpha(x_1), \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_i)}, \end{eqnarray}$

(4.88)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_k)}, \dots, \alpha(x_{n+1}), x_i\cdot x_{n+2})\\ &&-\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+1}(-1)^{j+k}\rho([\alpha^{n-1}(x_j), \alpha^{n-1}(x_k)]_C)\psi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \end{eqnarray}$

(4.89)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_k)}, \dots, \alpha(x_{n+1}), x_i\cdot x_{n+2})\\ &&-\sum\limits_{1\leq j < k < i\leq n+1}(-1)^{i+1}(-1)^{j+k}\mu(\alpha^{n-1}(x_j)\cdot\alpha^{n-2}(x_i\cdot x_{n+2}))\psi(\alpha(x_1), \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_k)}, \end{eqnarray}$

(4.90)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_i)}, \dots, \alpha(x_{n+1}), \alpha(x_k))\\ &&-\sum\limits_{1\leq j < i < k\leq n+1}(-1)^{i+1}(-1)^{j+k-1}\mu(\alpha^{n-1}(x_j)\cdot\alpha^{n-2}(x_i\cdot x_{n+2}))\psi(\alpha(x_1), \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_i)}, \end{eqnarray}$

(4.91)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_k)}, \dots, \alpha(x_{n+1}), \alpha(x_k))\\ &&-\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+1}(-1)^{j+k}\mu(\alpha^{n-1}(x_j)\cdot\alpha^{n-2}(x_i\cdot x_{n+2}))\psi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \end{eqnarray}$

(4.92)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_k)}, \dots, \alpha(x_{n+1}), \alpha(x_k))\\ &&+\sum\limits_{1\leq j < k < i\leq n+1}(-1)^{i+1}(-1)^{j+k}\mu(\alpha^{n-1}(x_k)\cdot\alpha^{n-2}(x_i\cdot x_{n+2}))\psi(\alpha(x_1), \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_k)}, \end{eqnarray}$

(4.93)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_i)}, \dots, \alpha(x_{n+1}), \alpha(x_j))\\ &&+\sum\limits_{1\leq j < i < k\leq n+1}(-1)^{i+1}(-1)^{j+k-1}\mu(\alpha^{n-1}(x_k)\cdot\alpha^{n-2}(x_i\cdot x_{n+2}))\psi(\alpha(x_1), \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_i)}, \end{eqnarray}$

(4.94)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_k)}, \dots, \alpha(x_{n+1}), \alpha(x_j))\\ &&+\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+1}(-1)^{j+k}\mu(\alpha^{n-1}(x_k)\cdot\alpha^{n-2}(x_i\cdot x_{n+2}))\psi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \end{eqnarray}$

(4.95)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_k)}, \dots, \alpha(x_{n+1}), \alpha(x_j))\\ &&+\sum\limits_{1\leq k < l < i < j\leq n+1}(-1)^{i+j}(-1)^{k+l}\rho([\alpha^{n-1}(x_k), \alpha^{n-1}(x_l)]_C)\psi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_k)}, \dots, \end{eqnarray}$

(4.96)

$\begin{eqnarray} &&\widehat{\alpha(x_l)}, \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+2}))\\ &&+\sum\limits_{1\leq k < i < l < j\leq n+1}(-1)^{i+j}(-1)^{k+l-1}\rho([\alpha^{n-1}(x_k), \alpha^{n-1}(x_l)]_C)\psi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_k)}, \dots, \end{eqnarray}$

(4.97)

$\begin{eqnarray} &&\widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_l)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+2}))\\ &&+\sum\limits_{1\leq k < i < j < l\leq n+1}(-1)^{i+j}(-1)^{k+l}\rho([\alpha^{n-1}(x_k), \alpha^{n-1}(x_l)]_C)\psi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_k)}, \dots, \end{eqnarray}$

(4.98)

$\begin{eqnarray} &&\widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_l)}, \dots, \alpha(x_{n+2}))\\ &&+\sum\limits_{1\leq i < k < l < j\leq n+1}(-1)^{i+j}(-1)^{k+l}\rho([\alpha^{n-1}(x_k), \alpha^{n-1}(x_l)]_C)\psi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \end{eqnarray}$

(4.99)

$\begin{eqnarray} &&\widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_l)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+2}))\\ &&+\sum\limits_{1\leq i < k < j < l\leq n+1}(-1)^{i+j}(-1)^{k+l-1}\rho([\alpha^{n-1}(x_k), \alpha^{n-1}(x_l)]_C)\psi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \end{eqnarray}$

(4.100)

$\begin{eqnarray} &&\widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_l)}, \dots, \alpha(x_{n+2}))\\ &&+\sum\limits_{1\leq i < j < k < l\leq n+1}(-1)^{i+j}(-1)^{k+l}\rho([\alpha^{n-1}(x_k), \alpha^{n-1}(x_l)]_C)\psi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \end{eqnarray}$

(4.101)

$\begin{eqnarray} &&\widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_l)}, \dots, \alpha(x_{n+2}))\\ &&+\sum\limits_{1\leq k < i < j\leq n+1}(-1)^{i+j}(-1)^k\rho([\alpha^{n-2}[x_i, x_j]_C, \alpha^{n-1}(x_k)]_C)\psi(\alpha(x_1), \dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_i)}, \end{eqnarray}$

(4.102)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+2}))\\ &&+\sum\limits_{1\leq i < k < j\leq n+1}(-1)^{i+j}(-1)^{k+1}\rho([\alpha^{n-2}[x_i, x_j]_C, \alpha^{n-1}(x_k)]_C)\psi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_k)}, \end{eqnarray}$

(4.103)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+2}))\\ &&+\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+j}(-1)^k\rho([\alpha^{n-2}[x_i, x_j]_C, \alpha^{n-1}(x_k)]_C)\psi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \end{eqnarray}$

(4.104)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_k)}, \dots, \alpha(x_{n+2}))\\ &&+\sum\limits_{1\leq k < l < i < j\leq n+1}(-1)^{i+j}(-1)^{k+l}\mu(\alpha^{n-1}(x_k)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_k)}, \dots, \end{eqnarray}$

(4.105)

$\begin{eqnarray} &&\widehat{\alpha(x_l)}, \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), \alpha(x_l))\\ &&+\sum\limits_{1\leq k < i < l < j\leq n+1}(-1)^{i+j}(-1)^{k+l-1}\mu(\alpha^{n-1}(x_k)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_k)}, \dots, \end{eqnarray}$

(4.106)

$\begin{eqnarray} &&\widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_l)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), \alpha(x_l))\\ &&+\sum\limits_{1\leq k < i < j < l\leq n+1}(-1)^{i+j}(-1)^{k+l}\mu(\alpha^{n-1}(x_k)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_k)}, \dots, \end{eqnarray}$

(4.107)

$\begin{eqnarray} &&\widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_l)}, \dots, \alpha(x_{n+1}), \alpha(x_l))\\ &&+\sum\limits_{1\leq i < k < l < j\leq n+1}(-1)^{i+j}(-1)^{k+l}\mu(\alpha^{n-1}(x_k)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \end{eqnarray}$

(4.108)

$\begin{eqnarray} &&\widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_l)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), \alpha(x_l))\\ &&+\sum\limits_{1\leq i < k < j < l\leq n+1}(-1)^{i+j}(-1)^{k+l-1}\mu(\alpha^{n-1}(x_k)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \end{eqnarray}$

(4.109)

$\begin{eqnarray} &&\widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_l)}, \dots, \alpha(x_{n+1}), \alpha(x_l))\\ &&+\sum\limits_{1\leq i < j < k < l\leq n+1}(-1)^{i+j}(-1)^{k+l}\mu(\alpha^{n-1}(x_k)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \end{eqnarray}$

(4.110)

$\begin{eqnarray} &&\widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_l)}, \dots, \alpha(x_{n+1}), \alpha(x_l))\\ &&-\sum\limits_{1\leq k < l < i < j\leq n+1}(-1)^{i+j}(-1)^{k+l}\mu(\alpha^{n-1}(x_l)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_k)}, \dots, \end{eqnarray}$

(4.111)

$\begin{eqnarray} &&\widehat{\alpha(x_l)}, \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), \alpha(x_k))\\ &&-\sum\limits_{1\leq k < i < l < j\leq n+1}(-1)^{i+j}(-1)^{k+l-1}\mu(\alpha^{n-1}(x_l)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_k)}, \dots, \end{eqnarray}$

(4.112)

$\begin{eqnarray} &&\widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_l)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), \alpha(x_k))\\ &&-\sum\limits_{1\leq k < i < j < l\leq n+1}(-1)^{i+j}(-1)^{k+l}\mu(\alpha^{n-1}(x_l)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_k)}, \dots, \end{eqnarray}$

(4.113)

$\begin{eqnarray} &&\widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_l)}, \dots, \alpha(x_{n+1}), \alpha(x_k))\\ &&-\sum\limits_{1\leq i < k < l < j\leq n+1}(-1)^{i+j}(-1)^{k+l}\mu(\alpha^{n-1}(x_l)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \end{eqnarray}$

(4.114)

$\begin{eqnarray} &&\widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_l)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), \alpha(x_k))\\ &&-\sum\limits_{1\leq i < k < j < l\leq n+1}(-1)^{i+j}(-1)^{k+l-1}\mu(\alpha^{n-1}(x_l)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \end{eqnarray}$

(4.115)

$\begin{eqnarray} &&\widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_l)}, \dots, \alpha(x_{n+1}), \alpha(x_k))\\ &&-\sum\limits_{1\leq i < j < k < l\leq n+1}(-1)^{i+j}(-1)^{k+l}\mu(\alpha^{n-1}(x_l)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_i, x_j]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \end{eqnarray}$

(4.116)

$\begin{eqnarray} &&\widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_l)}, \dots, \alpha(x_{n+1}), \alpha(x_k))\\ &&+\sum\limits_{1\leq k < i < j\leq n+1}(-1)^{i+j}(-1)^k\mu(\alpha^{n-2}[x_i, x_j]_C\cdot\alpha^{n-1}(x_{n+2}))\psi(\alpha(x_1), \dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_i)}, \end{eqnarray}$

(4.117)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), \alpha(x_k))\\ &&+\sum\limits_{1\leq i < k < j\leq n+1}(-1)^{i+j}(-1)^{k+1}\mu(\alpha^{n-2}[x_i, x_j]_C\cdot\alpha^{n-1}(x_{n+2}))\psi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_k)}, \end{eqnarray}$

(4.118)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), \alpha(x_k))\\ &&+\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+j}(-1)^k\mu(\alpha^{n-2}[x_i, x_j]_C\cdot\alpha^{n-1}(x_{n+2}))\psi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \end{eqnarray}$

(4.119)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_k)}, \dots, \alpha(x_{n+1}), \alpha(x_k))\\ &&-\sum\limits_{1\leq k < i < j\leq n+1}(-1)^{i+j}(-1)^k\mu(\alpha^{n-1}(x_k)\cdot\alpha^{n-1}(x_{n+2}))\psi(\alpha(x_1), \dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_i)}, \end{eqnarray}$

(4.120)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), [x_i, x_j]_C)\\ &&-\sum\limits_{1\leq i < k < j\leq n+1}(-1)^{i+j}(-1)^{k+1}\mu(\alpha^{n-1}(x_k)\cdot\alpha^{n-1}(x_{n+2}))\psi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_k)}, \end{eqnarray}$

(4.121)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), [x_i, x_j]_C)\\ &&-\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+j}(-1)^k\mu(\alpha^{n-1}(x_k)\cdot\alpha^{n-1}(x_{n+2}))\psi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \\ \nonumber&&\dots, \widehat{\alpha(x_k)}, \dots, \alpha(x_{n+1}), [x_i, x_j]_C), \end{eqnarray}$

(4.122)

and

$\begin{eqnarray} &&\partial_{\alpha\omega}(\partial_{\alpha\alpha} \psi)(x_1, \dots, x_{n+2})\\ & = &\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j}\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C)(\partial_{\alpha\alpha} \psi)(x_1, \dots, \hat{x_i}, \dots, \hat{x_j}, \dots, x_{n+2})\\ &&+\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j}\mu(\alpha^{n-1}(x_i)\cdot\alpha^{n-1}(x_{n+2}))(\partial_{\alpha\alpha} \psi)(x_1, \dots, \hat{x_i}, \dots, \hat{x_j}, \dots, x_{n+1}, x_j)\\ &&-\sum\limits_{1\leq i < j\leq n+1}(-1)^{i+j}\mu(\alpha^{n-1}(x_j)\cdot\alpha^{n-1}(x_{n+2}))(\partial_{\alpha\alpha} \psi)(x_1, \dots, \hat{x_i}, \dots, \hat{x_j}, \dots, x_{n+1}, x_i)\\ & = &\sum\limits_{1\leq k < i < j\leq n+1}(-1)^{i+j}(-1)^k\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C)\rho(\alpha^{n-1}(x_k))\psi(x_1, \dots, \hat{x_k}, \dots, \hat{x_i}, \dots, \hat{x_j}\dots, x_{n+2}) \end{eqnarray}$

(4.123)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < k < j\leq n+1}(-1)^{i+j}(-1)^{k+1}\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C)\rho(\alpha^{n-1}(x_k))\psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_k}, \dots, \hat{x_j}\dots, x_{n+2}) \end{eqnarray}$

(4.124)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+j}(-1)^k\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C)\rho(\alpha^{n-1}(x_k))\psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_j}, \dots, \hat{x_k}, \dots, x_{n+2}) \end{eqnarray}$

(4.125)

$\begin{eqnarray} &&+\sum\limits_{1\leq k < i < j\leq n+1}(-1)^{i+j}(-1)^k\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C)\mu(\alpha^{n-1}(x_{n+2}))\psi(x_1, \dots, \hat{x_k}, \dots, \hat{x_i}, \dots, \hat{x_j}\dots, x_{n+1}, x_k) \end{eqnarray}$

(4.126)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < k < j\leq n+1}(-1)^{i+j}(-1)^{k+1}\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C)\mu(\alpha^{n-1}(x_{n+2}))\psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_k}, \dots, \hat{x_j}\dots, x_{n+1}, x_k) \end{eqnarray}$

(4.127)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+j}(-1)^k\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C)\mu(\alpha^{n-1}(x_{n+2}))\psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_j}, \dots, \hat{x_k}\dots, x_{n+1}, x_k) \end{eqnarray}$

(4.128)

$\begin{eqnarray} &&-\sum\limits_{1\leq k < i < j\leq n+1}(-1)^{i+j}(-1)^k\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C)\psi(\alpha(x_1), \dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_i)}, \end{eqnarray}$

(4.129)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), x_k\cdot x_{n+2})\\ &&-\sum\limits_{1\leq i < k < j\leq n+1}(-1)^{i+j}(-1)^{k+1}\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C)\psi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_k)}, \end{eqnarray}$

(4.130)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), x_k\cdot x_{n+2})\\ &&-\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+j}(-1)^k\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C)\psi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \end{eqnarray}$

(4.131)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_k)}, \dots, \alpha(x_{n+1}), x_k\cdot x_{n+2})\\ &&+\sum\limits_{1\leq k < l < i < j\leq n+1}(-1)^{i+j}(-1)^{k+l-1}\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C)\psi([x_k, x_l]_C, \alpha(x_1), \dots, \widehat{\alpha(x_k)}, \end{eqnarray}$

(4.132)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_l)}, \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+2}))\\ &&+\sum\limits_{1\leq k < i < l < j\leq n+1}(-1)^{i+j}(-1)^{k+l}\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C)\psi([x_k, x_l]_C, \alpha(x_1), \dots, \widehat{\alpha(x_k)}, \end{eqnarray}$

(4.133)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_l)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+2}))\\ &&+\sum\limits_{1\leq k < i < j < l\leq n+1}(-1)^{i+j}(-1)^{k+l-1}\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C)\psi([x_k, x_l]_C, \alpha(x_1), \dots, \widehat{\alpha(x_k)}, \end{eqnarray}$

(4.134)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_l)}, \dots, \alpha(x_{n+2}))\\ &&+\sum\limits_{1\leq i < k < l < j\leq n+1}(-1)^{i+j}(-1)^{k+l-1}\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C)\psi([x_k, x_l]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \end{eqnarray}$

(4.135)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_l)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+2}))\\ &&+\sum\limits_{1\leq i < k < j < l\leq n+1}(-1)^{i+j}(-1)^{k+l}\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C)\psi([x_k, x_l]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \end{eqnarray}$

(4.136)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_l)}, \dots, \alpha(x_{n+2}))\\ &&+\sum\limits_{1\leq i < j < k < l\leq n+1}(-1)^{i+j}(-1)^{k+l-1}\rho([\alpha^{n-1}(x_i), \alpha^{n-1}(x_j)]_C)\psi([x_k, x_l]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \end{eqnarray}$

(4.137)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_l)}, \dots, \alpha(x_{n+2}))\\ &&+\sum\limits_{1\leq k < i < j\leq n+1}(-1)^{i+j}(-1)^k\mu(\alpha^{n-1}(x_i)\cdot\alpha^{n-1}(x_{n+2}))\rho(\alpha^{n-1}(x_k))\psi(x_1, \dots, \hat{x_k}, \dots, \hat{x_i}, \dots, \hat{x_j}\dots, x_{n+1}, x_j) \end{eqnarray}$

(4.138)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < k < j\leq n+1}(-1)^{i+j}(-1)^{k+1}\mu(\alpha^{n-1}(x_i)\cdot\alpha^{n-1}(x_{n+2}))\rho(\alpha^{n-1}(x_k))\psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_k}, \dots, \hat{x_j}\dots, x_{n+1}, x_j) \end{eqnarray}$

(4.139)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+j}(-1)^k\mu(\alpha^{n-1}(x_i)\cdot\alpha^{n-1}(x_{n+2}))\rho(\alpha^{n-1}(x_k))\psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_j}, \dots, \hat{x_k}\dots, x_{n+1}, x_j) \end{eqnarray}$

(4.140)

$\begin{eqnarray} &&+\sum\limits_{1\leq k < i < j\leq n+1}(-1)^{i+j}(-1)^k\mu(\alpha^{n-1}(x_i)\cdot\alpha^{n-1}(x_{n+2}))\mu(\alpha^{n-1}(x_j))\psi(x_1, \dots, \hat{x_k}, \dots, \hat{x_i}, \dots, \hat{x_j}\dots, x_{n+1}, x_k) \end{eqnarray}$

(4.141)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < k < j\leq n+1}(-1)^{i+j}(-1)^{k+1}\mu(\alpha^{n-1}(x_i)\cdot\alpha^{n-1}(x_{n+2}))\mu(\alpha^{n-1}(x_j))\psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_k}, \dots, \hat{x_j}\dots, x_{n+1}, x_k) \end{eqnarray}$

(4.142)

$\begin{eqnarray} &&+\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+j}(-1)^k\mu(\alpha^{n-1}(x_i)\cdot\alpha^{n-1}(x_{n+2}))\mu(\alpha^{n-1}(x_j))\psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_j}, \dots, \hat{x_k}\dots, x_{n+1}, x_k) \end{eqnarray}$

(4.143)

$\begin{eqnarray} &&-\sum\limits_{1\leq k < i < j\leq n+1}(-1)^{i+j}(-1)^k\mu(\alpha^{n-1}(x_i)\cdot\alpha^{n-1}(x_{n+2}))\psi(\alpha(x_1), \dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_i)}, \end{eqnarray}$

(4.144)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), x_k\cdot x_j)\\ &&-\sum\limits_{1\leq i < k < j\leq n+1}(-1)^{i+j}(-1)^{k+1}\mu(\alpha^{n-1}(x_i)\cdot\alpha^{n-1}(x_{n+2}))\psi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_k)}, \end{eqnarray}$

(4.145)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), x_k\cdot x_j)\\ &&-\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+j}(-1)^k\mu(\alpha^{n-1}(x_i)\cdot\alpha^{n-1}(x_{n+2}))\psi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \end{eqnarray}$

(4.146)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_k)}, \dots, \alpha(x_{n+1}), x_k\cdot x_j)\\ &&+\sum\limits_{1\leq k < l < i < j\leq n+1}(-1)^{i+j}(-1)^{k+l-1}\mu(\alpha^{n-1}(x_i)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_k, x_l]_C, \alpha(x_1), \dots, \widehat{\alpha(x_k)}, \end{eqnarray}$

(4.147)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_l)}, \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), \alpha(x_j))\\ &&+\sum\limits_{1\leq k < i < l < j\leq n+1}(-1)^{i+j}(-1)^{k+l}\mu(\alpha^{n-1}(x_i)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_k, x_l]_C, \alpha(x_1), \dots, \widehat{\alpha(x_k)}, \end{eqnarray}$

(4.148)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_l)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), \alpha(x_j))\\ &&+\sum\limits_{1\leq k < i < j < l\leq n+1}(-1)^{i+j}(-1)^{k+l-1}\mu(\alpha^{n-1}(x_i)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_k, x_l]_C, \alpha(x_1), \dots, \widehat{\alpha(x_k)}, \end{eqnarray}$

(4.149)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_l)}, \dots, \alpha(x_{n+1}), \alpha(x_j))\\ &&+\sum\limits_{1\leq i < k < l < j\leq n+1}(-1)^{i+j}(-1)^{k+l-1}\mu(\alpha^{n-1}(x_i)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_k, x_l]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \end{eqnarray}$

(4.150)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_l)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), \alpha(x_j))\\ &&+\sum\limits_{1\leq i < k < j < l\leq n+1}(-1)^{i+j}(-1)^{k+l}\mu(\alpha^{n-1}(x_i)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_k, x_l]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \end{eqnarray}$

(4.151)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_l)}, \dots, \alpha(x_{n+1}), \alpha(x_j))\\ &&+\sum\limits_{1\leq i < j < k < l\leq n+1}(-1)^{i+j}(-1)^{k+l-1}\mu(\alpha^{n-1}(x_i)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_k, x_l]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \end{eqnarray}$

(4.152)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_l)}, \dots, \alpha(x_{n+1}), \alpha(x_j))\\ &&-\sum\limits_{1\leq k < i < j\leq n+1}(-1)^{i+j}(-1)^k\mu(\alpha^{n-1}(x_j)\cdot\alpha^{n-1}(x_{n+2}))\rho(\alpha^{n-1}(x_k))\psi(x_1, \dots, \hat{x_k}, \dots, \hat{x_i}, \dots, \hat{x_j}\dots, x_{n+1}, x_i) \end{eqnarray}$

(4.153)

$\begin{eqnarray} &&-\sum\limits_{1\leq i < k < j\leq n+1}(-1)^{i+j}(-1)^{k+1}\mu(\alpha^{n-1}(x_j)\cdot\alpha^{n-1}(x_{n+2}))\rho(\alpha^{n-1}(x_k))\psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_k}, \dots, \hat{x_j}\dots, x_{n+1}, x_i) \end{eqnarray}$

(4.154)

$\begin{eqnarray} &&-\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+j}(-1)^k\mu(\alpha^{n-1}(x_j)\cdot\alpha^{n-1}(x_{n+2}))\rho(\alpha^{n-1}(x_k))\psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_j}, \dots, \hat{x_k}\dots, x_{n+1}, x_i) \end{eqnarray}$

(4.155)

$\begin{eqnarray} &&-\sum\limits_{1\leq k < i < j\leq n+1}(-1)^{i+j}(-1)^k\mu(\alpha^{n-1}(x_j)\cdot\alpha^{n-1}(x_{n+2}))\mu(\alpha^{n-1}(x_i))\psi(x_1, \dots, \hat{x_k}, \dots, \hat{x_i}, \dots, \hat{x_j}\dots, x_{n+1}, x_k) \end{eqnarray}$

(4.156)

$\begin{eqnarray} &&-\sum\limits_{1\leq i < k < j\leq n+1}(-1)^{i+j}(-1)^{k+1}\mu(\alpha^{n-1}(x_j)\cdot\alpha^{n-1}(x_{n+2}))\mu(\alpha^{n-1}(x_i))\psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_k}, \dots, \hat{x_j}\dots, x_{n+1}, x_k) \end{eqnarray}$

(4.157)

$\begin{eqnarray} &&-\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+j}(-1)^k\mu(\alpha^{n-1}(x_j)\cdot\alpha^{n-1}(x_{n+2}))\mu(\alpha^{n-1}(x_i))\psi(x_1, \dots, \hat{x_i}, \dots, \hat{x_j}, \dots, \hat{x_k}\dots, x_{n+1}, x_k) \end{eqnarray}$

(4.158)

$\begin{eqnarray} &&+\sum\limits_{1\leq k < i < j\leq n+1}(-1)^{i+j}(-1)^k\mu(\alpha^{n-1}(x_j)\cdot\alpha^{n-1}(x_{n+2}))\psi(\alpha(x_1), \dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_i)}, \end{eqnarray}$

(4.159)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), x_k\cdot x_i)\\ &&+\sum\limits_{1\leq i < k < j\leq n+1}(-1)^{i+j}(-1)^{k+1}\mu(\alpha^{n-1}(x_j)\cdot\alpha^{n-1}(x_{n+2}))\psi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_k)}, \end{eqnarray}$

(4.160)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), x_k\cdot x_i)\\ &&+\sum\limits_{1\leq i < j < k\leq n+1}(-1)^{i+j}(-1)^k\mu(\alpha^{n-1}(x_j)\cdot\alpha^{n-1}(x_{n+2}))\psi(\alpha(x_1), \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \end{eqnarray}$

(4.161)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_k)}, \dots, \alpha(x_{n+1}), x_k\cdot x_i)\\ &&-\sum\limits_{1\leq k < l < i < j\leq n+1}(-1)^{i+j}(-1)^{k+l-1}\mu(\alpha^{n-1}(x_j)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_k, x_l]_C, \alpha(x_1), \dots, \widehat{\alpha(x_k)}, \end{eqnarray}$

(4.162)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_l)}, \dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), \alpha(x_i))\\ &&-\sum\limits_{1\leq k < i < l < j\leq n+1}(-1)^{i+j}(-1)^{k+l}\mu(\alpha^{n-1}(x_j)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_k, x_l]_C, \alpha(x_1), \dots, \widehat{\alpha(x_k)}, \end{eqnarray}$

(4.163)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_l)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), \alpha(x_i))\\ &&-\sum\limits_{1\leq k < i < j < l\leq n+1}(-1)^{i+j}(-1)^{k+l-1}\mu(\alpha^{n-1}(x_j)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_k, x_l]_C, \alpha(x_1), \dots, \widehat{\alpha(x_k)}, \end{eqnarray}$

(4.164)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_i)}, \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_l)}, \dots, \alpha(x_{n+1}), \alpha(x_i))\\ &&-\sum\limits_{1\leq i < k < l < j\leq n+1}(-1)^{i+j}(-1)^{k+l-1}\mu(\alpha^{n-1}(x_j)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_k, x_l]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \end{eqnarray}$

(4.165)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_l)}, \dots, \widehat{\alpha(x_j)}, \dots, \alpha(x_{n+1}), \alpha(x_i))\\ &&-\sum\limits_{1\leq i < k < j < l\leq n+1}(-1)^{i+j}(-1)^{k+l}\mu(\alpha^{n-1}(x_j)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_k, x_l]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \end{eqnarray}$

(4.166)

$\begin{eqnarray} &&\dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_l)}, \dots, \alpha(x_{n+1}), \alpha(x_i))\\ &&-\sum\limits_{1\leq i < j < k < l\leq n+1}(-1)^{i+j}(-1)^{k+l-1}\mu(\alpha^{n-1}(x_j)\cdot\alpha^{n-1}(x_{n+2}))\psi([x_k, x_l]_C, \alpha(x_1), \dots, \widehat{\alpha(x_i)}, \\ \nonumber&&\dots, \widehat{\alpha(x_j)}, \dots, \widehat{\alpha(x_k)}, \dots, \widehat{\alpha(x_l)}, \dots, \alpha(x_{n+1}), \alpha(x_i)). \end{eqnarray}$

(4.167)

By the definition of the sub-adjacent Hom-Lie algebra, the sum of (4.102), (4.103) and (4.104) is zero. By the definition of Hom-pre-Lie algebras, the sum of (4.90), (4.95) and (4.118) is zero, the sum of (4.91), (4.92) and (4.119) is zero, the sum of (4.93), (4.94) and (4.117) is zero. Obviously, the sum of (4.96) and (4.137) is zero, the sum of (4.97) and (4.136) is zero, the sum of (4.98) and (4.135) is zero, the sum of (4.99) and (4.134) is zero, the sum of (4.100) and (4.133) is zero, the sum of (4.101) and (4.132) is zero, the sum of (4.87) and (4.131) is zero, the sum of (4.88) and (4.130) is zero, the sum of (4.89) and (4.129) is zero, the sum of (4.105) and (4.152) is zero, the sum of (4.106) and (4.151) is zero, the sum of (4.107) and (4.150) is zero, the sum of (4.108) and (4.149) is zero, the sum of (4.109) and (4.148) is zero, the sum of (4.110) and (4.147) is zero, the sum of (4.111) and (4.167) is zero, the sum of (4.112) and (4.166) is zero, the sum of (4.113) and (4.165) is zero, the sum of (4.114) and (4.164) is zero, the sum of (4.115) and (4.163) is zero, the sum of (4.116) and (4.162) is zero. By the definition of the sub-adjacent Lie bracket, the sum of (4.120), (4.145) and (4.146) is zero, the sum of (4.121), (4.144) and (4.161) is zero, the sum of (4.122), (4.159) and (4.160) is zero. Since $(V, \beta, \rho)$ is a representation of the sub-adjacent Hom-Lie algebra $A^C$ , the sum of (4.69), (4.70), (4.71), (4.123), (4.124) and (4.125) is zero. Since $(V, \beta, \rho, \mu)$ is a representation of the Hom-pre-Lie algebra $(A, \cdot, \alpha)$ , the sum of (4.73), (4.74), (4.78), (4.82), (4.83), (4.128), (4.138), (4.139), (4.143) and (4.158) is zero, the sum of (4.72), (4.77), (4.79), (4.81), (4.86), (4.127), (4.140), (4.142), (4.153) and (4.157) is zero, the sum of (4.75), (4.76), (4.80), (4.84), (4.85), (4.126), (4.141), (4.154), (4.155) and (4.156) is zero. Thus, we have $\partial_{\omega\omega}\circ \partial_{\alpha\omega}+\partial_{\alpha\omega}\circ \partial_{\alpha\alpha} = 0$ .

Similarly, we have $\partial_{\omega\alpha}\circ \partial_{\omega\omega}+\partial_{\alpha\alpha}\circ \partial_{\omega\alpha} = 0$ and $\partial_{\omega\alpha}\circ \partial_{\alpha\omega}+\partial_{\alpha\alpha}\circ \partial_{\alpha\alpha} = 0$ . This finishes the proof.

Acknowledgments

We give warmest thanks to Yunhe Sheng for helpful comments that improve the paper. This work is supported by National Natural Science Foundation of China (Grant No. 12001226).

Conflict of interest

The authors declare there is no conflicts of interest.

References

[1]	Adamowicz W, Louviere J, Williams M (1994) Combining Revealed and Stated Preference Methods for Valuing Environmental Amenities. J Environ Econ Manage 26: 271-292. doi: 10.1006/jeem.1994.1017
[2]	Ball R (2006) International Financial Reporting Standards (IFRS): Pros and Cons for Investors. Accounting Bus Res 36: 5-27. doi: 10.1080/00014788.2006.9730040
[3]	Boden R, Froud J (1996) Obeying the rules: Accounting for regulatory compliance costs in the United Kingdom. Accounting Organ Society 21: 529-547. doi: 10.1016/0361-3682(95)00034-8
[4]	Bruggemann U, Hitz J, Sellhorn T (2013) Intended and Unintended Consequences of Mandatory IFRS Adoption: A Review of Extant Evidence and Suggestions for Future Research. Eur Accounting Rev 22: 1-37. doi: 10.1080/09638180.2012.718487
[5]	Cazavan-Jeny A, Jeanjean T, Joos P (2011) Accounting choice and future performance: The case of R & D accounting in France. J Accounting Public Policy 30: 145-165. doi: 10.1016/j.jaccpubpol.2010.09.016
[6]	Covaleski MA, Dirsmith MW (1990) Dialectic tension, double reflexivity and the everyday accounting researcher: on using qualitative methods. Accounting Organ Society 15: 543-573. doi: 10.1016/0361-3682(90)90034-R
[7]	Fields TD, Lys TZ, Vincent L (2001) Empirical research on accounting choice. J Accounting Econ 31: 255-307. doi: 10.1016/S0165-4101(01)00028-3
[8]	Graham JR, Harvey CR, Rajgopal S (2005) The economic implications of corporate financial reporting. J Accounting Econ 40: 3-73. doi: 10.1016/j.jacceco.2005.01.002
[9]	Haka SF (1987) Capital budgeting techniques and firm specific contingencies: a correlational analysis. Accounting Organ Society 12: 31-48. doi: 10.1016/0361-3682(87)90014-6
[10]	Hensher DA (1994) Stated Preference Analysis of Travel Choices: The State of Practice. Transportation 21: 107-133. doi: 10.1007/BF01098788
[11]	Holthausen RW (1990) Accounting method choice. J Accounting Econ 12: 207-218. doi: 10.1016/0165-4101(90)90047-8
[12]	Messier WF, Quick LA, Vandervelde SD (2014) The influence of process accountability and accounting standard type on auditor usage of a status quo heuristic. Accounting Organ Society 39: 59-74. doi: 10.1016/j.aos.2013.12.002
[13]	Power B, Reid GC (2018) Decision support for firm performance by real options analytics. Managerial Decis Econ 39: 56-64. doi: 10.1002/mde.2867
[14]	Reid GC, Smith JA (2007) Reporting in Small Firms. Financ Manage, 31-33.
[15]	Schipper K (2010) How Can We Measure the Costs and Benefits of Changes in Financial Reporting Standards? Accounting Bus Res 40: 309-327. doi: 10.1080/00014788.2010.9663406
[16]	Schlapfer F, Schmitt M, Roschewiz A (2008) Competitive Politics, Simplified Heuristics, and Preference for Public Goods. Ecol Econ 65: 574-589. doi: 10.1016/j.ecolecon.2007.08.008

NAR-03-02-007-s001.pdf

Reader Comments

Your name:*

Email:*
© 2021 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

National Accounting Review

3.2

Metrics

Article views(2126) PDF downloads(138) Cited by(2)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

National Accounting Review

Choices of financial reporting regimes and techniques and underlying decision-making processes: a case study analysis of a port authority

Related Papers:

Abstract

1. Introduction

2. The full cohomology of Hom-pre-Lie algebras

3. Formal deformations of Hom-pre-Lie algebras

4. Abelian extensions of Hom-pre-Lie algebras

Appendix: The proof of Lemma 2.6

Acknowledgments

Conflict of interest

References

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Catalog

Abstract

1. Introduction

2. The full cohomology of Hom-pre-Lie algebras

3. Formal deformations of Hom-pre-Lie algebras

4. Abelian extensions of Hom-pre-Lie algebras

Appendix: The proof of Lemma 2.6

Acknowledgments

Conflict of interest

References

National Accounting Review

Choices of financial reporting regimes and techniques and underlying decision-making processes: a case study analysis of a port authority

Related Papers:

Abstract

1. Introduction

2. The full cohomology of Hom-pre-Lie algebras

3. Formal deformations of Hom-pre-Lie algebras

4. Abelian extensions of Hom-pre-Lie algebras

Appendix: The proof of Lemma 2.6

Acknowledgments

Conflict of interest

References

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog

Abstract

1. Introduction

2. The full cohomology of Hom-pre-Lie algebras

3. Formal deformations of Hom-pre-Lie algebras

4. Abelian extensions of Hom-pre-Lie algebras

Appendix: The proof of Lemma 2.6

Acknowledgments

Conflict of interest

References