1. Introduction
The use of fullerenes in polymer films and composites has become increasingly popular due to their ability to produce singlet oxygen [1], as well as their unique optical [2,3,4,5], magnetic [6,7], electronic [8,9,10], physical [11], and photophysical [1,12,13,14] properties. Though fullerenes have many potential uses, as-prepared, they are completely insoluble in aqueous solutions and only sparingly soluble in common organic solvents, monomers, and polymers, greatly limiting their utility. Fortunately, chemical modification of fullerenes may greatly enhance solubility and processability, thereby leading to a practical realization of many of these unique attributes [15,16]. As a result, a library of reactions has evolved which enable the surface functionalization of fullerenes creating interesting derivatives, which are more amenable to the preparation of fullerene polymer nanocomposites.
Of these, free-radical reactions continue to be an important methodology for the functionalization of fullerenes. The phrase “radical sponge” is often used to emphasize the high radical scavenging activity that C60 exhibits due to the combination of a favorable electron affinity (ca. 2.7-2.8 eV) and large number (30) of conjugated double bonds, which can readily react with radicals. [17] The addition of 11 phenyl groups, 15 benzyl groups, and 34 methyl groups are among the first examples of radical additions to C60 [18,19,20]. A review by Tzirakis et al. [18] summarizes the radical addition of C-, Si-, O-, S-, P-, N-, and metal- centered radicals as well as hydrogen and halogen radicals to fullerenes. In addition, select phosphorylated fullerene derivatives have been shown to possess biological activities [21,22] and optical properties [23,24]. Directly related to this work, C60 was also shown to readily react with commercial initiators such as 2, 2’-azobisisobutyronitrile (AIBN), which was discovered during the free-radical polymerization of methyl methacrylate (MMA) and styrene in the presence of C60 [25]. Ford et al. [26] later demonstrated the high reactivity of C60 with other thermal initiators, such as dimethyl azobisisobutyrate (DMAIB).
Compared to empty cage fullerenes, such as C60, functionalization of Sc3N@C80, a C80 cage encapsulating a Sc3N complex within, can be less straightforward. A stabilizing effect due to the electrostatic relationship between the Sc3N cluster and the C80 cage exists, and the electronic structure of [Sc3N]6+@[C80]6− results in a reduced reactivity in most reactions common to the derivatization of C60, whose reactivity can be compared to an electron-deficient alkene. Although numerous radical additions are successful with C60, only a few radical additions of metallic nitride fullerenes (MNFs), such as Sc3N@C80, have been documented [27,28,29,30]. In 2007, Shustova et al. [30] derivatized Sc3N@C80 via radical addition of CF3. Later, Shu et al. [29] derivatized Sc3N@C80 with carbon-centered radicals generated from diethyl malonate catalyzed by manganese (III) acetate. Shu et al. [28] also demonstrated the reaction of Sc3N@C80 with photochemically generated benzyl radicals producing a benzyl adduct, Sc3N@C80(CH2C6H5)2, in 82% yield. To a large extent, chemical reactivity is believed to be controlled by the identity of the metal encapsulated, as discussed in a recent report by Echegoyen et al. [31], where the Y3N@C80-diethyl malonate monoadduct was successfully prepared under conditions which would not produce the comparable Sc3N@C80 adduct. This difference in chemical reactivity was attributed to the encapsulated cluster, which affected the reactivity and the regiochemistry of the addition.
Numerous functionalization methods have been employed to increase the solubility, and therefore, the processability of fullerenes. An important breakthrough in polymer photovoltaics was achieved upon functionalizing pristine C60, yielding phenyl-C61-butyric acid methyl ester (PCBM), which exhibits enhanced solubility (~50 mg mL−1 in chlorobenzene) compared to pristine C60 [32]. In 2009, Troshin et al. [33] prepared various C60 and C70 methanofullerenes closely resembling the structure of PCBM to determine structure-property relationships as a function of functionality. The solubilities of the resulting methanofullerenes was found to vary greatly, ranging from 4 mg mL−1 to ~130 mg mL−1, however, a correlation between functionality and solubility was not determined. Furthermore, both empty cage fullerenes and MNFs have been highly hydroxylated yielding water soluble fullerols exhibiting biological activity [34,35,36,37,38] and radical-scavenging ability [39,40,41,42,43] with negligible toxicity [44,45]. Therefore, fullerols have attracted much attention for their use in medical and cosmetic applications.
Specific to this work, fullerenes and their derivatives are also well-known photosensitizers due to their unique electronic π-system [1,5,46,47]. The use of C60 fullerene as a photosensitizer to generate singlet oxygen as well as C60’s photophysical properties have been well documented [1,12,14,48,49,50,51]. Upon irradiation the fullerene is converted to an excited triplet state though intersystem crossing. The excited fullerene triplet can then react directly with molecular oxygen via energy transfer generating singlet oxygen, a highly reactive form of molecular oxygen. Once generated, singlet oxygen can be used in a variety of organic reactions such as the ene reaction and the Diels-Alder reaction [52,53]. The ability of a few fullerene derivatives to produce singlet oxygen has also been explored, where singlet oxygen production was found to decrease with an increase in the number addends bound to the cage but was independent of addend functionality [46,54,55,56]. Functionalized fullerenes that generate singlet oxygen have been exploited for their use in biological applications such photodynamic therapy and antimicrobial coatings [55,57].
In order to utilize the unique properties of fullerenes, many efforts have been made to incorporate fullerenes and fullerene derivatives into a polymer matrix via covalent linkages or blending. Polymer blends and cross-linked networks containing various fullerenes show great promise in the applications of optoelectronic devices, energy conversion systems, magnetic resonance imaging (MRI) contrast agents, photovoltaic devices, oxygen sensors, and as antimicrobial and decontaminating agents [3,51,58,59]. Recently, singlet oxygen generating fullerenes have been utilized as polymer constituents to create stimuli-responsive adhesives and antimicrobial coatings [51,60]. It has been demonstrated that the adhesive properties of rubber-based elastomeric adhesives can be affected when blended with singlet oxygen generators, such as fullerenes, by utilizing a singlet oxygen mediated crosslinking reaction [60]. Singlet oxygen generating fullerenes have also been exploited as potential self-decontaminating agents due to singlet oxygen’s ability to react with olefins, dienes, aromatics, peptides, and sulfur atoms, which are found in chemical warfare agents [61]. To date, fullerenes have been incorporated into polymer architectures including polystyrene (PS) [62,63], polyurethanes [61,64,65], and poly(methyl) methacrylate (PMMA) [66]. However, the work herein, evaluates the potential for incorporation of select C60 and Sc3N@C80 fullerene derivatives into a thiol-ene polymer matrix, which is a relatively new area of research. Thiol-ene networks are inherently a good model matrix due to its well-defined network, possessing narrow glass transition temperatures, which allow us to probe subtle physical changes, such as enthalpic relaxation, photodegradation, network free volume, molecular weight between crosslinks, and elasticity of the network [67,68,69].
Thiol-ene photopolymerizations occur rapidly through a step growth radical polymerization reaction, do not require solvents for processing, produce optically clear products, and exhibit good thermal and mechanical properties [68,69]. The uniformly structured networks of thiol-ene films allows tuning of properties depending upon the chemical structures of the thiols and enes. Furthermore, thiol-ene photopolymerizations are unique in that they are not inhibited by oxygen and may be polymerizable without the addition of photoinitiators [69,70]. The rate of polymerization of the thiol-ene reaction is directly related to the resulting electron density of the ene, where ene reactivity generally decreases with decreasing electron density. Therefore, electron rich enes polymerize much faster than electron poor enes [69,71,72]. The rate of polymerization of thiols can be affected by inductive resonance; therefore, propionate esters react faster than glycolate esters and both propionate and glycolate esters react much faster than alkyl thiols [69].
The incorporation of fullerenes into thiol-ene composite materials has not been widely studied, and little is known about the consequences of incorporating singlet oxygen generating species into these polymer nanocomposites. Our group’s interest lies in the preparation of novel fullerene derivatives as additives to thiol-ene composites and the characterization of the resulting networks. Derivatization of C60 and Sc3N@C80 with P- and C-centered radicals, resulting from the photochemical decomposition of bis(2, 4, 6-trimethylbenzoyl)-phenylphosphineoxide (TMB-PPO), was investigated as a function of C60 to TMB:PPO molar ratios. Fullerene derivatives possessing greatly enhanced solubilities were produced and incorporated into thiol-ene nanocomposites at various percent loadings. To our knowledge, this is the first report of functionalizing Sc3N@C80 with a phosphorous ligand.
2. Materials and Method
2.1. Materials
Trimethylolpropane diallyl ether (TMPDE) (90%), 1, 3, 5-triallyl-1, 3, 5-triazine-2, 4, 6(1H, 3H, 5H)-trione (TTT) (98%), trimethylolpropane tris (3-mercaptopropionate) (TMPMP) (>95%), pentaerythritol tetrakis (3-mercaptopropionate) (PETMP) (>95%), 2, 2’-azobisisobutyronitrile (AIBN, 98%), benzene (99%), chloroform (>99.8%), dichloromethane (DCM) (>99.5%), methanol (MeOH) (>99.8%), ethanol (EtOH) (>99.5%), acetone (>99.5%), and chloroform-d (99.8% atom D) were obtained from the Sigma Aldrich Chemical Company (St. Louis, MO). Bis(2, 4, 6-trimethylbenzoyl)-phenylphosphineoxide (TMB-PPO) was purchased from Ciba Specialty Chemicals. C60 (99.6%) and Sc3N@C80 (MNF) (95%) fullerenes were obtained from SES Research (Houston, TX). All chemicals were used as received.
2.2. Preparation of Fullerene Derivatives
C60 (75 mg, 104.2 µmol) and TMB-PPO (261 mg, 624.4 µmol) were added to benzene (150 mL) and sonicated until dissolved, as determined by a resulting clear purple-red solution. The solution was placed in a rayonet photochemical reactor containing sixteen, 350 nm λmax bulbs for 1 hr, at which time a precipitate forms. The resulting dark brown heterogeneous solution was concentrated under reduced pressure. Methanol (100 mL) was then added to the concentrated sample to dissolve any unreacted TMB-PPO from the precipitate of brown solids. The precipitate was separated via filtration using a 0.45 µm nylon filter membrane, followed by drying at 50 °C overnight to give C60(TMB-PPO)x in crude isolated yields of ~95%. Further purification was afforded via multiple passes on a gravity chromatography column (silica gel- SiliCycle® 40-63 µm), using a gradient solvent mixture from 100% DCM to 10% MeOH/90% DCM. A coffee colored narrow band was isolated and vacuum oven dried at 50 °C overnight to yield black crystals in 80% recovered yield. A similar method was utilized to prepare Sc3N@C80(TMB-PPO)y derivatives. Sc3N@C80 (5 mg, 4.5 µmol) and TMB-PPO (11.5 mg, 27.5 µmol) were added to benzene (100 mL), sonicated, and placed in the rayonet photoreactor for 1 hr. The resulting tan heterogeneous solution was then filtered using a 0.45 µm nylon filter membrane. Solids were washed 3x with additional benzene (100 mL) to remove unreacted Sc3N@C80 and TMB-PPO starting materials, followed by drying at 50 °C overnight to give Sc3N@C80(TMB-PPO)3 in crude isolated yields of ~ 95%. The purified fullerene derivatives were characterized via thermogravimetric analysis (TGA), FTIR spectroscopy, and MALDI.
2.3. Preparation of Fullerene-Containing Thiol-Ene Nanocomposites
Fullerene containing thiol-ene (TE) films were prepared by adding a known mass of the C60(TMB-PPO)5 derivative to a TE monomer mix of either PETMP:TTT or TMPMP:TMPDE monomers at varying TE monomer concentrations of 1:1 to 1:0.75 molar equivalents. AIBN thermal initiator (1 wt% solids) was added to the sample, and the resulting mixture was combined in a high shear mixer (FlackTec Inc SpeedMixerTM) at 2,400 rpm for 5 min. DCM (2-10 mL) was added to the sample to aid in uniform mixing. Any residual solvent was removed under reduced pressure, and the resulting clear sample was added to a 10 g Teflon release mold and placed in a 50-65 °C oven for at least 24 hrs to ensure complete polymerization. A similar method was utilized for the incorporation of Sc3N@C80(TMB-PPO)3 into the TE film. Sc3N@C80(TMB-PPO)3 (1.5 wt%) was added to TMPMP:TMPDE monomer mix prepared using a 1:0.75 molar ratio of thiol and ene. AIBN and acetone (~20 mL) were added to the sample, which was then mixed under high shear. Residual solvent was removed, and the concentrated sample was added to a Teflon release mold and placed in a 65-85 °C oven until fully cured. Five different sets of fullerene-TE films were prepared: C60(TMB-PPO)5 films prepared using PETMP:TTT and TMPMP:TMPDE monomers at 1:1 and at 1:0.75 molar equivalents at five different loadings of C60 (0, 1, 5, 10, and 20 wt%) and a Sc3N@C80(TMB-PPO)3 film using TMPMP:TMPDE monomers at 1:0.75 molar equivalents with a Sc3N@C80(TMB-PPO)3 loading of 1.5 wt % for a total of 21 films.
2.4. Characterization
2.4.1. Thermogravimetric Analysis (TGA)
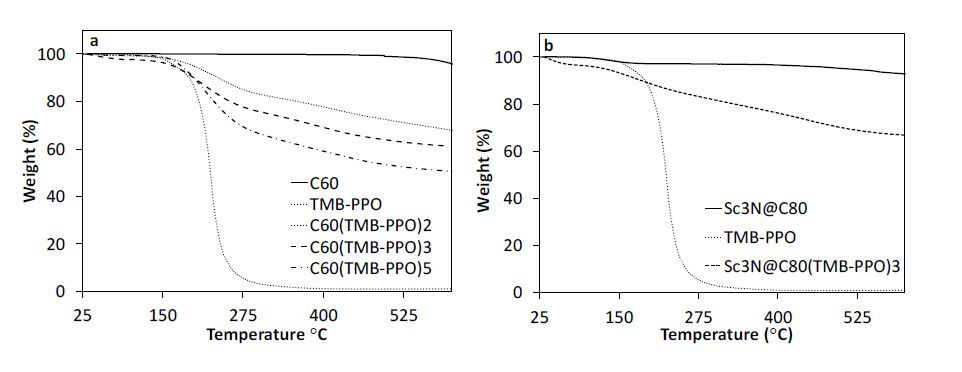
The thermal stability of the prepared fullerene derivatives and fullerene-TE nanocomposites were evaluated using a TA instrments Q500 serices thermogravimentric analysis (TGA) instrument by monitoring change in weight as a function of temperature under nitrogen. The samples (~ 10 mg) were analyzed in aluminum pans over the temperature range of 25-600 °C at a heating rate of 10 °C/min. The number of addends attached to the fullerene cage was estimated by calculating the ratio of the weight loss at 115-570 °C to the weight loss at temperatures above 570 °C [73]. The thermal degradation onset temperature was reported as the temperature corresponding to 10% mass loss.
2.4.2. Solubility Studies
The solubility of C60(TMB-PPO)x in common solvents was determined using a method adapted from Troshin et al. [33] In general, saturated solutions of the fullerene derivatives were prepared by mixing an excess amount of fullerene derivative (>25 mg) in 1 mL solvent (MeOH, EtOH, acetone, DCM, chloroform, and benzene) at room temperature (~25 °C) for 24 hrs. Following mixing, the prepared saturated solutions were filtered using 0.25 µm PTFE syringe filters into a glass vial of known mass, and solvent was removed at 50 °C under reduced pressure.
2.4.3. FT-IR, 1H-NMR, Elemental Analysis (EA), and Mass Spectrometry (MS), and XPS
Fourier transform infrared spectroscopy(FT-IR) analysis was performed in the 500-4000 cm-1 range using a Nicolet Nexus 470 FT-IR spectrometer equipped with a diamond crystal ATR accessory. 1H-NMR spectrums were obtained using a Bruker 400 MHz NMR in chloroform-d, and all shifts are reported relative to chloroform-d at 7.24 ppm. Vacuum dried samples (50 °C for 48 hrs) were sent to a commercial analytical company, Galbraith Laboratories Inc. (Knoxville, TN) for elemental analysis. Matrix-Assisted Laser Desorption/Ionization MALDI-MS of C60(TMB-PPO)5 and Sc3N@C80(TMB-PPO)3 was obtained using a MicroFlex Bruker mass spectrometer with a laser power of ~90%. The matrix was prepared by dissolving 2, 5-dihydroxybenzoic acid (1 mg) in 1 mL of a mixed solvent of 0.1% trifluoroacetic acid (TFA)/DCM (1:2, v/v). The C60(TMB-PPO)5 adduct (1 mg/mL), dissolved in DCM, was premixed with the prepared matrix at a 1:2000 molar analyte-to-matrix ratio, then applied to the target plate, followed by solvent evaporation. A ProteoMassTM mix containing bradykinin fragment 1-7 (757.3997 Da), angiotensin II (human) (1,046.5423 Da), and insulin (bovine) (5,730.6087 Da), dissolved in acetonitrile, was used as references. Digested samples were sent to a commercial analytic company, Bonner Analytical Testing Co. (Hattiesburg, MS) for inductively-coupled plasma mass spectrometry (ICP-MS). Prior to ICP-MS analysis, a known mass of sample (~1.5 mg) was added to a digestion tube containing concentrated sulfuric acid (3.5 mL) and H2O2 (30 %, 3.5 mL). The resulting mixture was heated to 200 °C until no solids were visual and solutions were clear (~6 hrs). Upon cooling to room temperature, resulting solutions were filtered using 0.2 µm PTFE syringe filters and diluted with water yielding a 50 mL sample. X-ray photoelectron spectroscopy (XPS) analysis was performed at the Naval Research Laboratory (NRL) (Washington, DC) using a Thermo Scientific K-Alpha X-ray photoelectron spectrometer equipped with monochromatic Al Kα radiation. Survey spectra were acquired from −10 to 1350 eV with 200.00 eV pass energy, 25 ms dwell time, and 1.000 eV step size. High resolution elemental scans were performed for C 1s, N 1s, O 1s, P 2p, and Sc 2p. 10 scans were collected and averaged for C, N, and O, while 30 scans were averaged for P 2p. Each scan employed 30 eV pass energy, 50 ms dwell time, and 0.150 eV step size. Peak fitting and integration were performed via Smart Fitting on Avantage XPS software.
2.4.4. Singlet Oxygen Studies
Direct singlet oxygen solution based studies were performed using a method adapted by Barker et al. [74] C60(TMB-PPO)x (100 µmol) samples were prepared by dissolving C60(TMB-PPO)x in benzene and sonicating for 5 min or until fully dissolved. Benzene (2 mL) was added to a fluorometer cuvette with a septum cap and stir bar, and then 10 µL of the prepared sample was added to the cuvette via microsyringe. The resulting sample was then excited at 350 nm, and an emission scan was obtained from 1200-1350 nm. Any singlet oxygen produced was directly detected via the phosphorescence of singlet oxygen at 1270 nm using a Photon Technology International (PTI) FeliX32 InGaAs-TE photodiode with pre-amplifier NIR detector. Following each emission scan obtained, more sample (10-100 µL) was added to the cuvette to obtain calibration curves. Analogous methods were employed to obtain a calibration curve for the singlet oxygen generation of pure C60 (10 µM) for comparison.
Thin films for singlet oxygen studies were prepared by coating Teflon containers with prepared TMPMP:TMPDE monomer mix containing C60(TMB-PPO)5 (1 and 10 wt% C60) or 1.5 wt% Sc3N@C80(TMB-PPO)3 and curing at 65 °C for at least 24 hrs. Films were prepared at different thicknesses to yield similar transparencies for singlet oxygen studies. Resulting films were then adhered to one side of a flurometer cuvet containing benzene. An excitation scan was obtained from 300-600 nm with the emission set to 1270 nm, and emission scans were obtained from 1200-1350 nm while exciting at 420 nm.
2.4.5. Gel Fractions
Gel fractions of the prepared films were obtained by dissolving a known mass of the film in benzene, allowing the samples to rest at room temperature for 24 hrs, and recovering the remaining film. Residual solvent was removed at 25 °C under reduced pressure. Gel fractions were recorded as the final mass of the film after solvent removal over the initial mass and is expressed as a percent. UV-Vis was obtained on the resulting solution to determine the amount of extractable C60(TMB-PPO)5, through comparisons to known standards.
2.4.6. Differential Scanning Calorimetry (DSC)
The glass transition temperature (Tg) of films was determined using a TA Instruments Q2000 modulated differential scanning calorimetry (DSC). The thermally cured films (~10 mg) were monitored over the temperature range of −50 to 150 °C in a heat/cool/heat cycle at 5 °C /min. The Tg was obtained from the second heating cycle.
2.4.7. Transmission Electron Microscopy (TEM)
Fullerene-containing TE nanocomposites were analyzed using a Zeiss EM-900 TEM at 50kV. TEM samples were prepared using a Leica EM FC6 ultramicrotome with cryochamber cooled to −60 °C and sliced using glass knives. Samples were applied to 300 mesh copper grids, purchased from Electron Microscopy Sciences.
2.4.8. Film Photodegradation Studies
FT-IR samples were prepared for photodegradation studies by diluting the resulting C60(TMB-PPO)5 TE monomer mix with DCM, yielding a 10 wt % solid solution. The dilute solution (100 µL) was then spin casted onto sodium chloride salt plates using an ED101D Digital Photo Resist Spinner (Headway Research, TX) at 1000 rpm for 60s. Coated salt plates were then placed in a 65 °C oven for at least 48 hrs allowing the films to cure. The concentration of C60 varied from 0-10 wt%, and irradiation time spanned from 0-4 hrs under a high-pressure mercury-xenon lamp. FT-IR measurements were taken before and after irradiation using a PerkinElmer Spectrum 100 spectrometer in the 450-4000 cm−1 range.
3. Results and Discussion
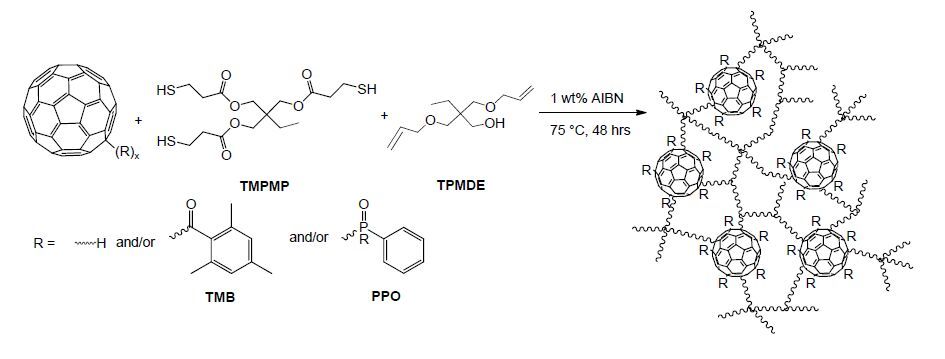
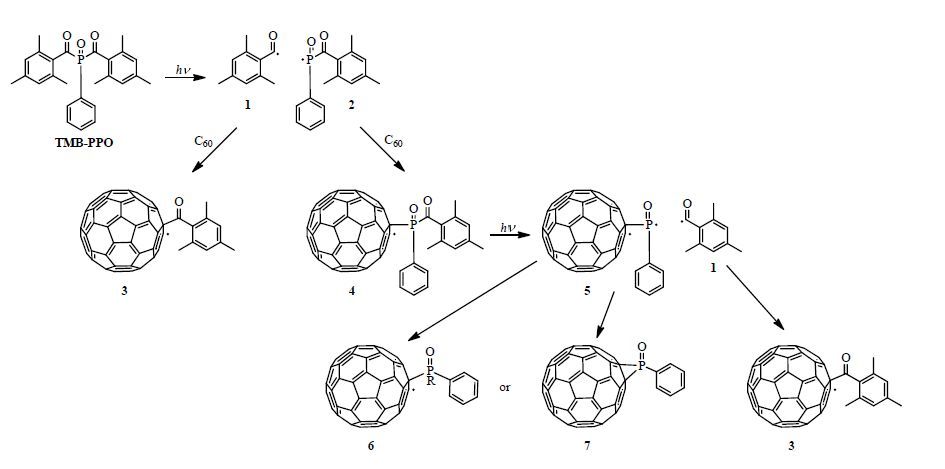
In general, C60 and Sc3N@C80 derivatives were prepared via radical addition of the photodecomposition products from the commercial photoinitiator (TMB-PPO), according to Scheme 1. The reaction of C60 with thermal initiators AIBN and DMAIB has also been reported [25,26]. As illustrated in Scheme 1, both C- and P-centered radicals are produced during photodecomposition, which may either add to C=C or terminate through hydrogen abstraction or coupling reactions [75]. A brief description of the variety of radicals and products that may be produced in Scheme 1 is as follows. TMB-PPO undergoes an initial α-cleavage yielding a benzoyl (1) and an (acyl) phosphinoyl (2) radical, both of which may react with C60 yielding the C60-benzoyl radical adduct (3) and a C60-(acyl) phosphinoyl radical (4), respectively. The C60-(acyl) phosphinoyl radical (4) can undergo a second α-cleavage forming another benzoyl (1) and a C60-phosphinoyl radical (5). The benzoyl radical (1) produced can then react with another C60, yielding another C60-benzoyl radical adduct (3), and the C60-phosphinoyl radical (5) produced can with undergo hydrogen abstraction yielding product 6 or couple yielding product 7
Structure-reactivity relationships based on the number of addends bound to C60 was determined by varying the molar ratio of C60 to TMB:PPO. Derivatives prepared during this study and the corresponding fullerene:TMB-PPO molar ratios are reported in Table 1. Using thermogravimetric analysis (TGA), the maximum number of TMB-PPO addends bound to C60 was found to be five. Representative TGA plots of fullerene derivatives are provided in Figure 1, including C60, Sc3N@C80, and TMB-PPO for comparison. The number of addends increases with excess TMB-PPO only to a ratio of C60:TMB-PPO of 1:6, and above this ratio, higher order adducts were not observed and the isolated yield of soluble products decreased. Therefore, the optimal ratio of 1:6 was chosen for Sc3N@C80, which resulted in an Sc3N@C80 adduct containing three TMP-PPO addends. The thermal degradation onset temperatures of the resulting adducts were also determined from TGA and are reported in Table 1. As expected, the thermal degradation onset temperatures of the resulting adducts fall between 190-236 °C, indicating a slightly elevated thermal stability over TMB-PPO alone (191 °C) but decreases with increasing functionality.
Table 1.Derivative functionality and thermal stability as a function of fullerene:TMP-PPO reactivity
| Sample ID
|
Fullerene:TMB-PPO Molar Ratioa
|
Avg. # Addendsb
|
% Yieldc
|
Degradation Onset ( °C)d
|
% Chare
|
| C60(TMB-PPO)x
|
1:1
|
2
|
> 95
|
236
|
68
|
|
|
1:3
|
3
|
> 95
|
208
|
61
|
|
|
1:6
|
5
|
> 95
|
199
|
48
|
|
|
1:12
|
5
|
> 85
|
-
|
-
|
| Sc3N@C80(TMB-PPO)3
|
1:6
|
3
|
> 95
|
190
|
63
|
| TMB-PPO
|
-
|
-
|
-
|
191
|
1
|
| C60f
|
-
|
-
|
-
|
642
|
95
|
| Sc3N@C80
|
-
|
-
|
-
|
719
|
93
|
| a Using a rayonet photoreactor, samples were irradiated in benzene at 350 nm for 1 hr.; b calculated from TGA plots as reported by Singh et al.; c isolated yields based on the average molecular mass of the derivative population; d calculated from TGA plots as the temperature corresponding to 10% mass loss; e% char is reported as the mass remaining above 600 °C from TGA analysis; and f C60 control. |
A primary goal of this work was to prepare fullerene derivatives having increased solubility in common organic solvents and miscibility with TE monomers. Solubility studies were performed using a method adapted from Troshin et al. [33] and are reported in Table 2. The number of TMB-PPO addends bound to C60 was found to dramatically change the solubility of the adducts. In general, increasing the number of addends resulted in an increase in solubility of the resulting fullerene derivatives. The addition of five TMB-PPO addends was found to increase C60’s solubility to a remarkably high value of ~260 mg mL−1 in DMC. However, the addition of two and three TMB-PPO addends only increased the solubility up to ~18-19 mg mL−1 also in DMC. The increase in solubility is attributed to the addition of carbonyl and phosphine oxide functional groups, which increase polarity of the derivative.
Table 2.Solubility of fullerene derivatives in common solvents at 20 °C reported in mg mL−1
|
MeOH
|
EtOH
|
Acetone
|
DCM
|
CHCl3
|
Benzene
|
| Dielectric constant
|
33
|
24.3
|
21
|
9.1
|
4.8
|
2.28
|
| C60 [76]
|
0
|
0.001
|
0.001
|
0.26
|
0.16
|
1.7
|
| C60(TMB-PPO)2
|
0.2
|
0.3
|
0.5
|
18
|
14
|
8
|
| C60(TMB-PPO)3
|
0.4
|
1
|
1.3
|
19
|
15
|
11
|
| C60(TMB-PPO)5
|
6
|
3
|
40
|
260
|
219
|
91
|
| Sc3N@C80
|
0
|
0
|
0
|
0.1
|
0.8
|
1.1
|
| Sc3N@C80(TMB-PPO)3
|
1.5
|
1.4
|
1.3
|
0
|
0
|
0
|
| a Average of triplicate measurements, all values within ±10%. |
Further characterization was performed on the most soluble derivative of each fullerene, i.e. C60(TMB-PPO)5 and Sc3N@C80(TMB-PPO)3. FT-IR collected on the purified C60(TMB-PPO)5 and Sc3N@C80(TMB-PPO)3 samples support the successful attachment of TMB-PPO, through the presence of shifts in characteristic peaks (Supporting Information Figures S1 and S2). An observed shift in the C=OTMB from 1680 to 1707 cm−1 and 1731 cm−1 is observed for C60(TMB-PPO)5 and Sc3N@C80(TMB-PPO)3, respectively. A comparable shift results in the P=OPPO peaks from 1201 and 1222 cm−1 to 1223 and 1242 cm−1 for C60(TMB-PPO)5 and 1261 cm−1 for Sc3N@C80(TMB-PPO)3. MALDI-MS analysis on the C60(TMB-PPO)5 yielded significant peaks at m/z 720 and 1386 corresponding to pure C60 and C60 with ~5 TMB-PPO addends attached. Sc3N@C80(TMB-PPO)3 yielded peaks a m/z 1109 corresponding to pure Sc3N@C80, suggesting loss of functionality during laser ablation which is a common occurance with fullerene derivatives. (Supporting Information Figures S3 and S4). EA experimental percent compositions of C 78.6, H 4.4, O 15.6, P 1.4 were obtained for C60(TMB-PPO)5 and suggested the attachment of 5 TMB-PPO addends at a ratio of 0.7 PPO to 4.3 TMB (1PPO:6TMB) addends per C60, which correlates well with TGA and MALDI. ICP-MS and XPS were obtained for both C60(TMB-PPO)5 and Sc3N@C80(TMB-PPO)3. ICP-MS suggests that 1.6% of the C60(TMB-PPO)5 sample consists of phosphorous, which correlates with elemental analysis. IPC also suggests the binding of ~2.25 phosphorous addends per Sc3N@C80, where TGA suggested a total of three addends bound to Sc3N@C80 indicating a higher binding of phosphorous to Sc3N@C80 than C60. ICP data was also found to correlate well with FT-IR, where phosphine oxide peaks were stronger than the carbonyl peaks for the Sc3N@C80(TMB-PPO)3 sample. XPS data, along with FT-IR, suggests the binding of phosphine oxide to C60 and Sc3N@C80. In summary, shifts in the characteristic TMB-PPO peaks observed in the FT-IR, in combination with MALDI and the increased solubilities, supports the successful derivatization of C60 and Sc3N@C80 with TMB-PPO.
Since the cleavage reactions of TMB-PPO yields a 2:1 ratio of TMB and PPO radicals (Scheme 1), both of which can readily react with C60, 1H-NMR was performed to determine the relative ratio of TMB and PPO addends bound to C60(TMB-PPO)5. As observed in Supporting Information Figure S5, the TMB-PPO starting material possesses well-resolved and unique hydrogen chemical shifts for both TMB (Ha, 7.86 δ; Hb, 7.53 δ; Hc, 7.40 δ) and PPO (Hd, 6.77 δ; He, 2.23 δ; Hf, 2.12δ), allowing for the easy integration of 1H-NMR peak areas. Unlike the starting material, the C60(TMB-PPO)5 adduct yields broad peaks for TMB (Ha, Hb, Hc, 7.57 δ) and PPO (Hd, He, Hf, 2.13 δ) addends, which is suggestive of a population of isomers and not a single isomer. However, although broad peaks are present in the 1H-NMR spectrum of the C60(TMB-PPO)5 derivative, regions characteristic of TMB and PPO do not overlap, allowing for successful integration. The relative ratio of TMB to PPO addends was determined by integration and normalizing for the number of hydrogens. The 1H-NMR data further supports a ratio of 0.7 PPO to 4.3 TMB (1PPO:6TMB) addends per C60, which correlates well with EA and ICP-MS.
Table 3.Efficiency of singlet oxygen generation by derivatives
| Derivatives
|
Derivatives (µM)
|
C60 (µM)
|
Relative
Efficiency
|
| Sc3N@C80
|
0.3
|
0.1
|
−3
|
| C60(TMB-PPO)2
|
9
|
0.9
|
−10
|
| C60(TMB-PPO)3
|
13
|
1
|
−13
|
| C60(TMB-PPO)5
|
25
|
1
|
−25
|
| Singlet oxygen was directly detected via phosphoresce at 1270 nm at λex = 350 nm. |
Direct singlet oxygen studies were performed using a method previously reported by Barker et al. [74] In general, solution based assays were performed through the direct detection of the singlet oxygen phosphorescence (1270 nm) produced upon exciting a catalytic amount of C60(TMB-PPO)x and Sc3N@C80(TMB-PPO)3 adducts at 350 nm. Emission spectrums were obtained for pristine C60 and C60(TMB-PPO)x derivatives to obtain normalized responses yielding C60 equivalent correlation concentrations, which are reported in Table 3 and Supporting Information Figure S6. In general, an expected trend was observed where emission intensity increased with increasing concentration and the generation of singlet oxygen decreased upon increased derivatization of C60. A previous study by Hamano et al. [46] showed a similar trend where the efficiency of fullerene derivatives to produce singlet oxygen was not dependent on the type of added but was dependent on the number of addends, where efficiency decreased with an increase in functionality. Singlet oxygen studies were also performed on Sc3N@C80(TMB-PPO)3. Interestingly, although Sc3N@C80 itself was shown to produce singlet oxygen, derivatization greatly diminished the response in the adduct to below confidence.
Enhanced solubility in organic media for the derivatives enabled the preparation of fullerene-TE nanocomposites, according to Scheme 2. C60(TMB-PPO)5 and Sc3N@C80(TMB-PPO)3 were added to a TE monomer mix of either PETMP:TTT or TMPMP:TMPDE (Figure 2) at two TE molar equivalents of 1:1 or 1:0.75. The concentration of the ene was reduced in one series in anticipation of fullerene derivatives acting as a reactive ene and capturing radicals generated during the thermal polymerization of the composites. Two monomer compositions were explored, a rigid system, PETMP:TTT (Tg 56), and a flexible system, TMPMP:TMPDE (Tg -32), to allow a broader scope of structure-property determinations, with regard to thermal and mechanical properties. Five different sets of fullerene-TE films were prepared (Table 4). Representative images of the resulting TE films, placed in order of increasing C60 loading, are provided in Figure 3 and Supporting Information Figures S7-S9.
Table 4.Physical and mechanical analysis of fullerene containing TE nanocomposites
| Monomers/Sample IDa
|
Wt % C60(TMB-PPO)5
|
Ene/Thiol ratio
|
Gel %
|
% Extractable C60(TMB-PPO)5
|
Glass Transition Temperature (Tg) DSC
|
Thermal Degradation Onset ( °C)d
|
% Chare
|
| PETMP:TTT-1-0
|
0
|
1
|
99
|
-
|
56
|
352
|
7.2
|
| PETMP:TTT-1-1
|
2
|
1
|
99
|
0
|
60
|
349
|
8.8
|
| PETMP:TTT-1-5
|
10
|
1
|
99
|
0
|
42
|
339
|
13.8
|
| PETMP:TTT-1-10
|
19
|
1
|
88
|
15
|
19
|
328
|
20.5
|
| PETMP:TTT-1-20*
|
39
|
1
|
47*
|
63*
|
9*
|
257
|
29.6
|
| PETMP:TTT-0.75-0
|
0
|
0.75
|
99
|
-
|
31
|
348
|
7.7
|
| PETMP:TTT-0.75-1
|
2
|
0.75
|
99
|
0
|
38
|
342
|
8.9
|
| PETMP:TTT-0.75-5
|
10
|
0.75
|
99
|
0
|
41
|
338
|
13.8
|
| PETMP:TTT-0.75-10
|
19
|
0.75
|
99
|
0
|
41
|
323
|
18.2
|
| PETMP:TTT-0.75-20
|
39
|
0.75
|
98
|
5
|
33
|
274
|
27.7
|
| TMPMP:TMPDE-1-0
|
0
|
1
|
99
|
-
|
-32
|
323
|
1.4
|
| TMPMP:TMPDE-1-1
|
2
|
1
|
97
|
1
|
-28
|
316
|
2.9
|
| TMPMP:TMPDE-1-5
|
10
|
1
|
95
|
2
|
-25
|
301
|
7.3
|
| TMPMP:TMPDE-1-10
|
19
|
1
|
93
|
3
|
-20
|
288
|
13.3
|
| TMPMP:TMPDE-1-20
|
39
|
1
|
93
|
7
|
-5
|
279
|
23.4
|
| TMPMP:TMPDE-0.75-0
|
0
|
0.75
|
91
|
-
|
-33
|
335
|
1.7
|
| TMPMP:TMPDE-0.75-1
|
2
|
0.75
|
99
|
0
|
-28
|
309
|
3.1
|
| TMPMP:TMPDE-0.75-5
|
10
|
0.75
|
95
|
2
|
-27
|
294
|
10.1
|
| TMPMP:TMPDE-0.75-10
|
19
|
0.75
|
94
|
5
|
-20
|
293
|
15.2
|
| TMPMP:TMPDE-0.75-20
|
39
|
0.75
|
93
|
6
|
-4
|
263
|
28.8
|
| TMPMP:TMPDE-0.75-1.5 Sc3N@C80
|
2.4
|
0.75
|
96
|
0
|
-33
|
296
|
5.7
|
| a Samples identified by monomer composition (PETMP:TTT or TMPMP:TMPDE), ene/thiol ratio (1 or 0.75), and % equivalent loading C60 (0, 1, 5, 10, or 20%). |
As seen in Figure 4, the incorporation of C60(TMB-PPO)5 into the TE films results in a visually more uniform and continuous composition compared to the addition of pure C60. A general and expected trend was observed, where higher loadings of C60 resulted in increasingly darker films (Figure 3). Curing time was found to be dependent on monomer identity and C60 loading, where curing times increased with an increase in C60 loading and with a decrease in monomer rigidity. We attribute the increased curing times to the persistent radical scavenging ability of fullerenes. Furthermore, the increased curing times for TMPMP:TMPDE compared to the PETMP:TTT monomers is expected due to the lower monomer functionality. A composite was considered cured coincident with the disappearance of the monomer SH and C=C absorptions.
The gel percents and extractable C60(TMB-PPO)5 from the composites were analyzed to assess the nature and degree of incorporation of the derivatives. As seen in Table 4 and Supporting Information Figure S10, all films at low loadings of C60 (<5%) yield good gel percent values (>90%). Furthermore, all films prepared using the flexible monomers (TMPMP:TMPDE) yield similar gel percents, all above 90%. However, a very different trend was observed with films prepared using the rigid (PETMP:TTT) monomers with higher loadings of C60 (>10%), where the ene deficient PETMP:TTT-0.75 films exhibit significantly higher gel percent values (99% and 98%) compared to the PETMP:TTT-1 film series (88% and 47%).
The amount of C60(TMB-PPO)5 extracted during gel percent studies was also analyzed and is reported in Table 4 and Supporting Information Figure S11. In general, the amount of C60(TMB-PPO)5 was found to compare well with the gel percent values obtained. For all samples, an expected trend in the amount of C60(TMB-PPO)5 extracted increased as a function of C60 loading. All samples prepared with TMPMP:TMPDE monomers, as well as, the PETMP:TTT-0.75 films resulted in films with moderately low (<7%) amounts of extracted C60(TMB-PPO)5. Both gel percent values and the amount of C60(TMB-PPO)5 extracted from the prepared films suggests that C60 is being covalently incorporated into the films, as observed by high gel percent values and a low amount of C60(TMB-PPO)5 extracted from all films except the PETMP:TTT-1 10 and 20 wt % film series, which were crumbly films of poor quality.
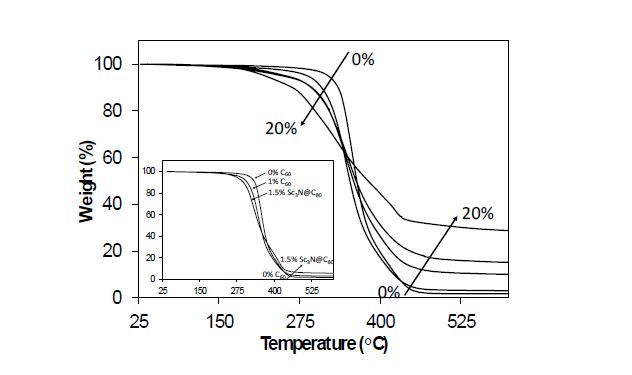
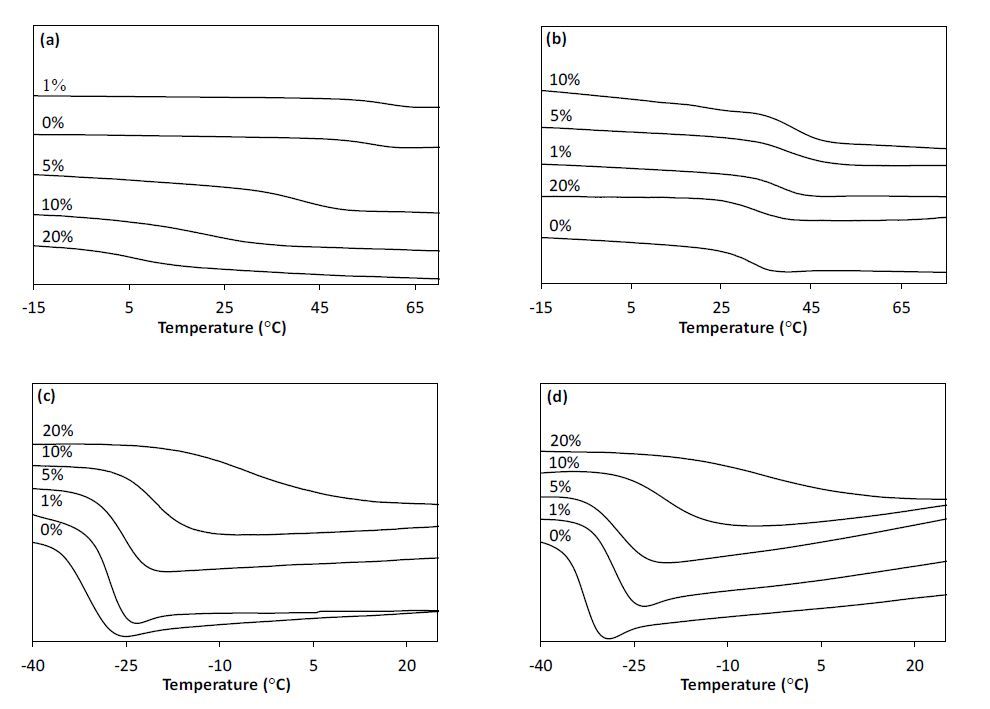
The thermal and mechanical properties of the resulting TE films were explored as a function of C60 loading, alkyl ene concentration, and monomer rigidity. Thermal stability of the resulting films was determined using TGA, and representative TGA plots are provided in Figure 5 as well as Supporting Information Figure S12. Thermal degradation onset temperatures and % char were calculated from TGA and are recorded in Table 4. For both film series, a decrease in thermal stability is observed with an increase in C60 loading. This is attributed to a more favorable breaking of the fullerene-addend bond, allowing the fullerene to re-establish conjugation. Reports of increased thermal stability on polymer networks containing blended fullerene derivatives suggest that optimal stabilization occurs at low loadings of C60 (0.4-0.8 wt%), where higher loadings increase aggregation, thus deforming the morphological interactions between polymer chains and negatively impacting the networks [66]. Another study of the thermal stability of C60-containing PS and PMMA concluded that increased thermal stability of C60-containing networks occurred only with blended C60 due to its superior ability of the π-system to “trap” radicals resulting from thermal degradation, while C60 networks containing multiple covalent attachments to the network lacked this characteristic, thus demonstrating decreased thermal stability [77].
All films were analyzed using DSC and obtained Tg values, along with the temperature step width of the Tg, are reported in Table 4 and graphed in Figure 6 and Supporting Information Figure 13. For the flexible films series, a general trend was observed where the Tg increases (~28 °C) and a broadening of step width of the Tg (~20 °C) was observed as a function of C60 loading independent of alkyl ene concentration. However, the Tg for the rigid film series was found to be depended on both C60 loading and alkyl ene concentration, where the Tg of the control (0% C60) PETMP:TTT films decreased significantly (25 °C) with a decrease in alkyl ene concentration. The change in Tg of the control films for the rigid system, as a function of alkyl ene concentration, suggest that small changes in the network of the PETMP:TTT films yields significant changes in Tg. Furthermore, an interesting trend is observed with the PETMP:TTT-1 film series where the Tg increases initially (4 °C) with C60 loading (1 wt%), then declines (51 °C) as C60 loading continues to increase. However, with the PETMP:TTT-0.75 film series, the Tg increases (10 °C) and remains constant (±3 °C) up to 20 wt% loading, at which time the Tg decreases (8 °C). Furthermore, the step width of the PETMP:TTT film series was found to only vary ~5 °C. Another trend was observed with DSC, where the enthalpic relaxation peak is only observed for the TMPMP:TMPDE films up to 5 wt % C60 loading and for the rigid ene deficient control (PETMP:TTT-0.75-0) film. This phenomenon is not surprising considering enthalpy relaxation is known to decrease with increasing monomer rigidity [67]. The variations in the observed Tg, broadening of the Tg step width, and the loss of enthalpy relaxation as a function of C60 loading, alkyl ene concentration, and monomer rigidity, further supports the covalent incorporation of C60 into the TE matrix.
Increasing C60 loadings resulted in higher residual mass for each loaded film, reported as % char in Table 4. This remaining mass is attributed to the high thermal stability of the C60 and Sc3N@C80 cage [78,79]. It should be noted that the remaining mass of each sample was greater than the combined mass remaining for the control sample plus fullerene loading, which has been observed in other studies and is believed to be due to one of the following possibilities. Either strong interactions occurring between the fullerene cage and the polymer network or the initiator that requires temperatures greater than 600 ⁰C to dissociate, or aggregation of the derivatives within the polymer network [61]. Since the thermal stability of the nanocomposites were negatively impacted by the addition of the fullerene adducts, it does not seem likely that the increase in % char is due to strong interaction between the fullerene adducts and polymer network.
Further characterization was afforded on the prepared films to assess the dispersibility of the C60(TMB-PPO)5 adducts within the polymer matrix, and TEM images of the 1 and 10 wt% composites are provided in Figure 7 and Supporting Information Figures S14-S22. An interesting phenomena of larger aggregates were observed within the rigid PETMP:TTT film series; however, the fullerene containing TMPMP:TMPDE films appeared continuous throughout. The aggregation of C60(TMB-PPO)5 within the PETMP:TTT films could be due to increased reactivity/functionality or changes in miscibility with PETMP and TTT monomers, excluding the C60(TMB-PPO)5 during polymerization and resulting in phase segregation.
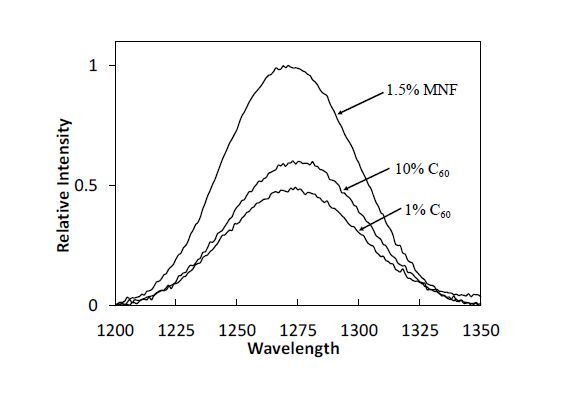
Composites were evaluated for the production of singlet oxygen. Direct observation studies of singlet oxygen phosphorescence were performed on the TMPMP:TMPDE-0.75 films containing 1 and 10 wt% C60 and 1.5 wt% Sc3N@C80 films. Thickness was adjusted to yield films of comparable transparencies, assuming that the singlet oxygen observed was produced at or near the surface of the sample, not in the bulk material. As seen in Figure 8, the emission spectrum of the fullerene containing films shows a phosphorescence peak centered at 1270 nm supporting the formation of singlet oxygen, and in general phosphorescence increases with fullerene loading. Surprisingly, the highest phosphorescence yield correlated to the Sc3N@C80 films, where crosslinking reactions may have served to adjust orbital positions to promote a more favorable energy transfer to molecular oxygen.
Generating singlet oxygen within a polymer matrix has the potential to degrade the network through hydrogen abstraction, scission reactions, and the oxidation of network functional groups. With the confirmed generation of singlet oxygen from the composites, a brief study was performed on the TMPMP:TMPDE-0.75 fullerene composites to characterize significant changes in the network as a function of radiation exposure time and fullerene concentration. Thin samples were cast onto salt plates and monitored before and after exposure. After irradiation, all samples, including the control sample, resulted in amber colored films. For ease of comparison, the FT-IR spectrums of C60(TMB-PPO)5 containing TMPMP:TMPDE TE films are shown from 4000-2500 cm−1 in Supporting Information Figure S24, from 1800-1550 cm−1 in Supporting Information Figure S24; and from 1500-500 cm−1 in Supporting Information Figure S26. Overall, relatively minor additional photodegradation is promoted at low fullerene loadings above that which is observed by the controls. A couple general statements regarding photostability and the generation of oxygenated species in the networks include (1) the growth of broad absorption bands centered around 3300 cm−1 suggests the formation of hydroperoxide products, which increases with C60 loading and increased singlet oxygen production, and (2) higher loadings of C60 may lead to a higher fraction of unreacted enes, which are well-known reagents for reactions with singlet oxygen, the “ene” reaction. Other absorption bands yield evidence for modest increases in photodegradation at higher fullerene loadings, such as the broadening of the carbonyl stretch at 1739 cm−1 suggesting the formation of new carbonyl moieties upon generating singlet oxygen (Supporting Information Figure S25). However, previous work by our group has demonstrated that in polymer composites bearing residual enes and readily abstractable hydrogens, the in-situ generation of singlet oxygen can be devastating to network physical properties in a very short time scale [60].
4. Conclusion
Chemical modification of fullerenes has been shown to greatly enhance solubility and processability of fullerenes. As a result, a library of reactions has evolved which enable the surface functionalization of fullerenes creating interesting derivatives, which are more amenable to the preparation of fullerene polymer nanocomposites. Of these, radical addition reactions continue to be an important methodology. C60 and Sc3N@C80 derivatives were prepared via radical addition of the photodecomposition products from the commercial photoinitiator TMB-PPO, yielding C60(TMB-PPO)5 and Sc3N@C80(TMB-PPO)3 as preferred soluble derivatives obtained in high yields (>95%). The solubility of C60(TMB-PPO)5 was found to increase to a remarkably high value of ~ 260 mg mL-1 in DMC. Further characterization of the mixture of isomers using standard techniques suggests an overall 1PPO:6TMB ratio of addends, reflecting the increased reactivity of the carbon radical. Although a higher percentage of PPO is observed in the Sc3N@C80(TMB-PPO)3 population, perhaps due to reverse electronic requirements of the substrate. Visually dispersed TE nanocomposites with low extractables were prepared using two monomer compositions of PETMP:TTT (Tg 56) and TMPMP:TMPDE (Tg -32) with increasing fullerene derivative loading and at two different TE molar equivalents (1:1 or 1:0.75) to probe network structure-property relationships. The thermal and mechanical properties of the resulting TE films were explored as a function of C60 loading, alkyl ene concentration, and monomer rigidity. Thermal stability of the derivatives and the resulting networks was determined using TGA and was found to decrease with an increase in monomer functionality and at high fullerene loadings, respectively. Which is attributed to the favorable breaking of the fullerene-addend bond, allowing the fullerene to re-establish conjugation. All films were also analyzed using DSC and Tg values were obtained. The Tg of the flexible film series was found to increase as a function of C60 loading independent of alkyl ene concentration. Whereas, the Tg of the rigid film series was found to be dependent on both C60 loading and alkyl ene concentration. Furthermore, enthalpic relaxations observed with DSC decreased with an increase in C60 loading and monomer rigidity. TMPMP:TMPDE composite networks show well-dispersed derivatives via TEM imaging, and PETMP:TTT composites show phase separation in TEM, which is supported by the observed Tg’s. Singlet oxygen generation of the derivatives decreases with increased functionality; however this is compensated for by the tremendous increase in solubility in organic solvents and miscibility with monomers. Most importantly, singlet oxygen generation from the composites increased with fullerene derivative loading, with good photostability of the networks.
Acknowledgments
Financial support for this work was provided through the NSF GK-12 Program-University of Southern Mississippi, “Connections in the Classroom: Molecules to Muscles” award #0947944, the National Science Foundation (NSF) CAREER program award #CHE-0847481, and the NSF instrumentation grant #CHE-0840390. The authors would also like to thank David Delatte and Jessica Douglas from USM for obtaining DSC data and help with TEM images, respectively. The authors would also like to thank James H. Wynne and Jeffrey G. Lundin from NRL for obtaining XPS data.
Conflict of Interest
The authors declare no conflict of interest.









 DownLoad:
DownLoad: