Cellular deformation characterization of human breast cancer cells under hydrodynamic forces

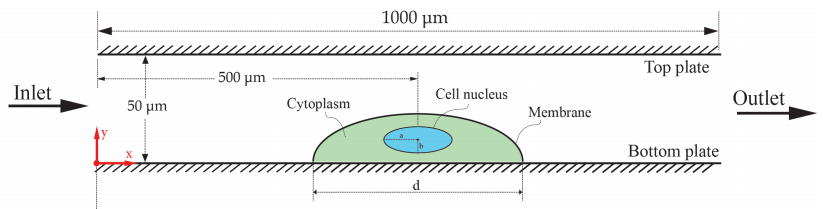
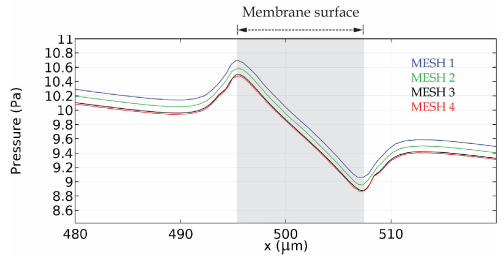
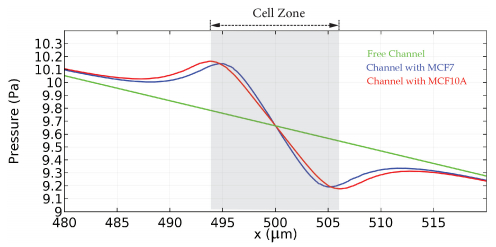
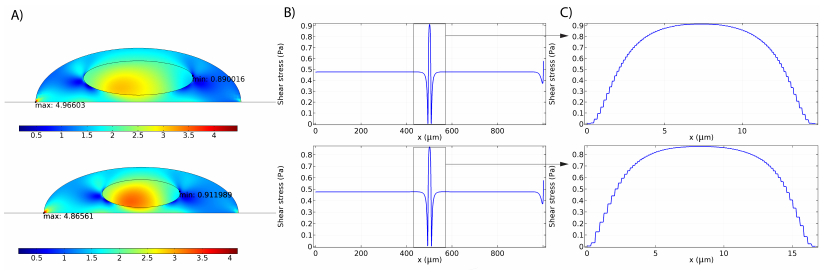
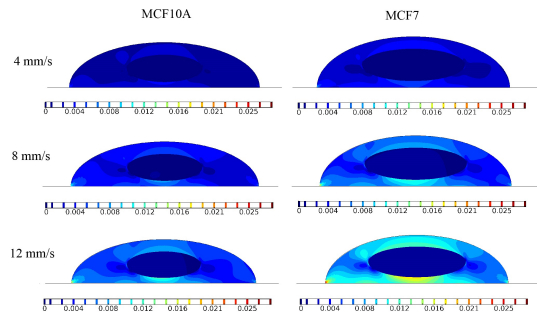
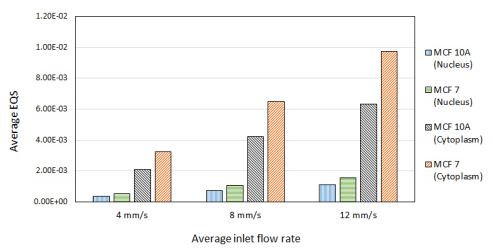
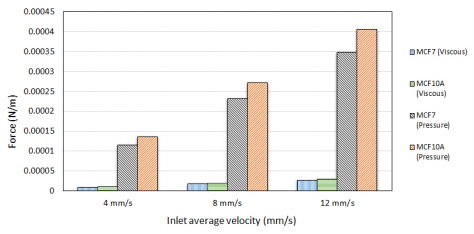
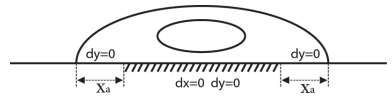
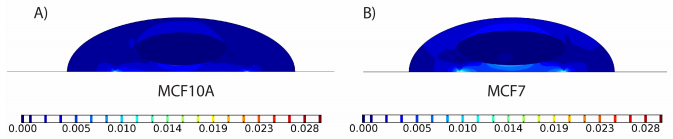
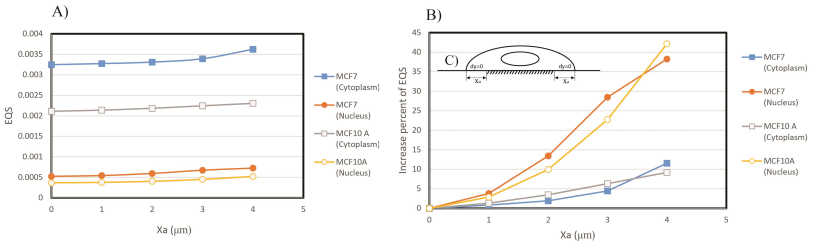
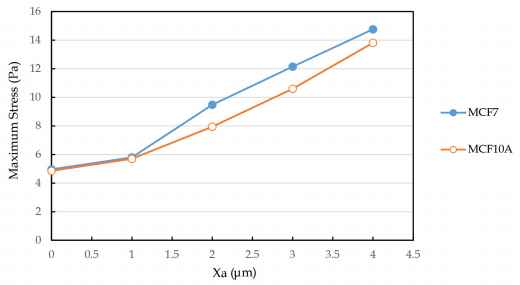
Understanding how cells sense mechanical forces, and how respond biologically to themis an interesting and quickly-progressing area. Cells within their microenvironment are subjected tovarious physical forces such as mechanical loads and shear stress. Cells respond and adjust to theseforces by mechanotransduction mechanism in which deformation and mechanical forces are convertedinto biomechanical signals. To quantify mechanotransduction responses and to correctly interpretthe behavior of cell under in vitro stimulation, magnitude and distribution of the stresses on the cellmembrane should be characterized. In this study, a 2D Finite Element Model is introduced to simulatethe deformation of individual benign (MCF10A) and malignant (MCF7) human breast cancer cellsunder hydrodynamic forces. A fluid-structure interaction method is implemented to model fluid flowand the adherent single cells inside a microchannel to study the nature of mechanical forces (viscousand pressure) and to determine their contribution to the deformation of cells. Due to the differentmechanical properties, cells respond differently to the forces exerted by the fluid flow. It was foundthat the maximum stress and strain take place at the interface of the adherent cell and channel wall. Also, under the same boundary conditions, nucleolus and cytoplasm of an individual malignant cellundergo more deformation comparing a single benign cell. Furthermore, it was observed that both two cell lines experience much more stress when their attached area to the substrate is reduced.