1. Introduction

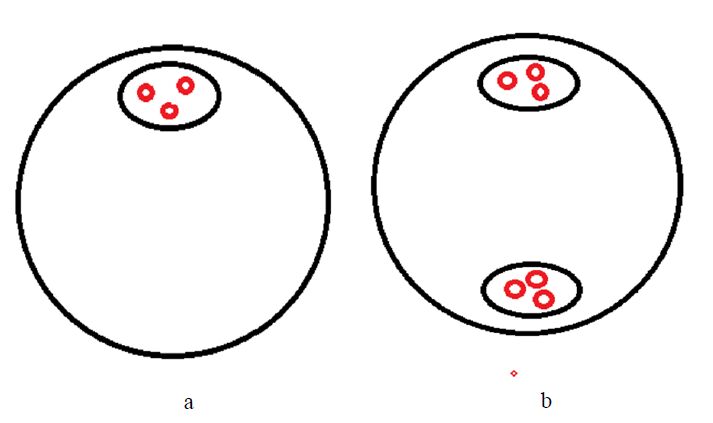
The Berg-Purcell ^[1] result, models the cell as a sphere with N circular binding sites distributed uniformly over the surface of the cell. The binding sites are of radius a, and the sphere is of radius R. The receptors or binding sites are totally reactive, in that when a ligand diffuses up to the site they are completely absorbed or transformed and the site is again available for another reaction. We are assuming that the reaction is diffusion-controlled (i.e. the rate limiting step is the diffusion process). The concentration of ligands is given by c(r) with diffusion coefficient D. The concentration of ligands far away from the cell isgiven by c˳, a constant. The diffusion-limited rate constant is given by k=J/c˳, where J is the reactive flux of ligands to the sphere. And ${\text{J}}=\int {} \partial {\text{c}}/\partial {\text{r}}\left| {_{\text{R}}} \right.(4\pi {R^2})$. It is assumed that the steady state has been achieved and the flux is constant. The expression for the Berg-Purcell rate constant is given by equation (2), where 4πDR is the rate constant for a uniformly reactive spherical cell. The uniform distribution of reactive sites over a sphere, while a useful model in some cases^[2], rules out systems where chemoreceptors are located over a small area or areas of the cell^[3,4,5,6]. Prior to this, single binding sites located at one ^[7,8,9,10]and both^[11,12] poles of a sphere have been considered. In this paper, the Berg-Purcell ^[1] model is generalized to clusters of receptors located at one and both poles of a cell. Heretofore this has not been accomplished. See Figures 1a and 1b. The diffusion-controlled rate constant, for multiple reactive sites located at one and both poles of a spherical cell are derived and studied in the current work. The cluster model is superior to the Berg-Purcell model, when the cell has its receptors located at one or both the poles. An example of this is bacterial chemotaxis. This is where bacteria respond to chemicals in their environment. They move towards favorable chemicals and away from unfavorable ones. Recent experimental evidence shows that the receptors on such a bacterial cell are located at one or both poles ^[3]. We will show in this paper that the rate constant, for a partially covered patch, is almost identical to one that is completely covered by receptors. This behavior, has been seen previously, for a whole sphere partially covered by reactive sites ^[1,13]. We will also show there is a significant drop in the diffusion-controlled reaction rate from the Berg-Purcell rate to the cluster model rate. In this paper we start by showing the method used in deriving the rate constant for multiple binding sites. We then derive and discuss the rate constant for multiple reactive sites at one pole on a spherical cell. Next we consider the case where the cluster of reactive sites is located at both poles of a spherical cell. Finally we summarize our results and draw conclusions.

---

2. Materials and Methods

First we demonstrate the solution technique for a problem that has been solved previously ^[13]. We then apply it to many sites at the poles. If we consider a cell as a partially reactive sphere, that is

one whose rate constant is obtained by using the Collins and Kimball theory ^[14]. This rate constant is given by ^[13].

${\text{k}} = 4\pi {\text{DRK}}/\left( {4\pi {\text{DR}} + {\text{K}}} \right)$

(1)

Here ĸ is a measure of diffusion control. As given before ^[13], if we set ĸ=N4Da, where N is the number of reactive sites of radius a, in which 4Da is the diffusion controlled rate constant of a circular site embedded in a non-reactive planar surface, we obtain the Berg-Purcell result ^[1].

${{\text{k}}_{{\text{BP}}}} = {\text{ }}4\pi {\text{DRNa}}/\left( {\pi {\text{R}} + {\text{Na}}} \right)$

(2)

This assumption is valid, given that there are many sites relatively far apart. The above result was found previously, by using an analogy with electrostatics ^[1]. We see in the limit of large N, the Smoluchowski result is obtained (i.e. for a totally reactive sphere).

${\text{k}} = 4\pi {\text{DR}}$

(3)

---

3. Results

---

3.1. The rate constant for a diffusion-controlled reaction at multiple sites located on one pole of a Sphere

Here we derive the rate constant for the multiple site problem, where the receptors are located at one pole of a sphere. See Figure 1a. We want to solve Laplace’s equation in spherical polar coordinates

${\partial ^2}c(r,Ɵ)/\partial {r^2} + (2/r)\partial c(r,Ɵ)/\partial r + 1/{r^2}(1/sin Ɵ)\partial /\partial Ɵ(\sin Ɵ \partial c(r,Ɵ)/\partial Ɵ) = 0$

(4)

Where c(r, ϴ) gives the ligand concentration. The solution to this problem is

$c(r,Ɵ) = \alpha + \sum {_l^\infty } {\text{ }}{a_l}{P_l}(cosƟ)/{r^{(l + 1)}}$

(5)

Subject to

$c˳ = {\lim _{r \to \infty }}c(r,Ɵ)$

(6)

c˳ is the bulk concentration of ligand and the P_l(cosƟ) are Legendre polynomials of order l. The reactive boundary conditions are given by

$c\left( {r,Ɵ} \right) = 0 \qquad \qquad \qquad 0 \leq Ɵ \leq Ɵ˳$

(7)

and

$\partial c(r,Ɵ)/\partial r\left| {_{r = R}} \right. = 0 \qquad \qquad Ɵ˳ \leq Ɵ \leq \pi$

(8)

As done previously ^[7,11], equation (7) is replaced by the constant flux boundary condition

$\partial c(r,)/\partial r\left| {_{r = R}} \right. = Q \qquad \qquad \qquad 0 \leq Ɵ \leq Ɵ˳$

(9)

Q is evaluated by

$4\pi {{\text{R}}^2}{\text{D}}\int_0^{Ɵ˳} {} \partial c/\partial r\left| {_R\sin Ɵ} \right.d Ɵ= {ĸ_1}\int_0^{Ɵ˳} {} c(R,Ɵ)sin Ɵ d Ɵ$

(10)

Here ĸ₁ is a measure of diffusion control. The diffusion-controlled rate constant is given by

${{\text{k}}_{{\text{DC}}}} = (2\pi {{\text{R}}^2}{\text{D}}/{\text{c}}˳)\int_0^{Ɵ˳} {} \partial c/\partial r\left| {_R\sin Ɵ} \right.d Ɵ$

(11)

Applying the boundary conditions to equation (5) ^[7], we obtain for the partially diffusion-controlled rate constant for one polar reactive site on a spherical cell.

$\begin{gathered} {{\text{k}}_{{\text{DC}}}} = 8\pi {\text{DR}}{ĸ_1}{(1 - cos(Ɵ˳))^2}/\{ 16\pi {\text{DR(}}1 - cos Ɵ˳) + 2{ĸ_1}{{\text{(}}1 - cos Ɵ˳)^2} \hfill \\ {ĸ_1}\sum\nolimits_{l = 1} {} {[{P_{{\text{l}} - 1}}(cos Ɵ˳) - {P_{{\text{l}} + 1}}(cos Ɵ˳)]^2}/({\text{l}} + 1)({\text{l}} + 1/2)\} \hfill \\ \end{gathered}$

(12)

As discussed previously, if we replace ĸ₁ in equation (12) by N4Da, we obtain the diffusion-limited rate constant for N uniformly circular reactive sites of radius a located on a spherical cap of half-angle Ɵ˳ at one pole on a sphere (see Figure 1a)

$\begin{gathered} {{\text{k}}_{{\text{DC}}}} = 8\pi {\text{DR}}{ĸ_1}{(1 - cos(Ɵ˳))^2}{\text{Na}}/\{ 16\pi {\text{DR(}}1 - cos Ɵ˳) + 2{\text{Na}}{{\text{(}}1 - cos Ɵ˳)^2} \hfill \\ {\text{Na}}\sum\nolimits_{l = 1} {} {[{P_{{\text{l}} - 1}}(cos Ɵ˳) - {P_{{\text{l}} + 1}}(cos Ɵ˳)]^2}/({\text{l}} + 1)({\text{l}} + 1/2)\} \hfill \\ \end{gathered}$

(13)

Letting ϴ˳→π in equation (13), we obtain the Berg-Purcell rate as given by equation (2)

The fraction of receptors on the patch is given by

${\text{f}} {\text{ = }} {\text{(N/2)(}}{{\text{a}}^2}{\text{/}}{{\text{R}}^2}{\text{)(1/(1 - cos}} ϴ˳{\text{))}}$

(14)

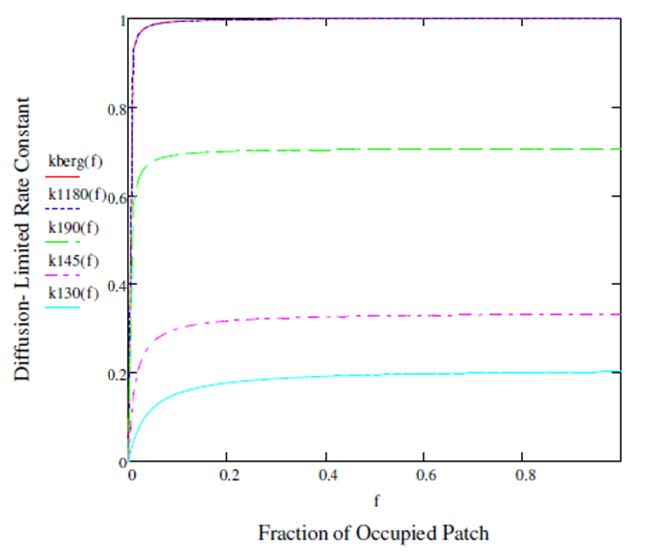
Figure 2 shows k1Ɵ˳=k_{DC /}4πDR plotted against the fraction of receptors for different patch sizes (30, 45, 90 and 180 degrees along with the Berg-Purcell result, Equation (2)/4πDR). One can see that the proper limiting behavior occurs when Ɵ˳→ 180 degrees (e.g. there is complete agreementwith the Berg-Purcell result). One also can see that when the fraction of the occupied patch is 15% or greater, the patch behaves as if it were completely absorbing (independent of the patch size). This indicates that the many site model, can be replaced by the one site model. Meaning the rate constant (13) for the cluster problem can be replaced by the simpler one site ^[9] result (ĸ₁→∞ in equation (12). This is given by

${{\text{k}}_{{\text{DC}}}} = 8\pi {\text{DR}}{(1 - cos Ɵ˳)^2}/\{ 2{(1 - cos Ɵ˳)^2} + \sum\nolimits_{l = 1} {} {[{P_{{\text{l}} - 1}}(cos Ɵ˳) - {P_{{\text{l}} + 1}}(cos Ɵ˳)]^2}/({\text{l}} + 1)({\text{l}} + 1/2)\}$

(15)

Finally it can be seen that rate constant for the receptor clusters is significantly lower than the Berg-Purcell result (30% for a 90 degree patch). All calculations were carried out using an R/a=1000.

---

3.2. The rate constant for a diffusion-controlled reaction at multiple sites located on both poles of a Sphere

Here we derive the rate constant for multiple sites, where the receptors are located at both poles of a sphere. See Figure 1b. We want to solve equation (4), where the solution is again given by equation (5).Here the solution is subject to the same boundary conditions as before with 3 exceptions. The first is the addition of

$\partial c(r,Ɵ)/\partial Ɵ = 0 \qquad Ɵ = \pi /2$

(16)

This says that the net flux normal to the equatorial plane vanishes for all r. It reduces the two site problem to a one site problem. Second, for the current problem Ɵ varies from 0 to π/2. Whereas in the previous problem, Ɵ varied from 0 to π. As a consequence of this, the boundary conditions must be modified accordingly. Finally, equation (10) becomes for this problem

$2\pi {{\text{R}}^2}{\text{D}}\int_0^{Ɵ˳} {} \partial c/\partial r\left| {_R\sin Ɵ} \right.d Ɵ = {ĸ_2}\int_0^{Ɵ˳} {} c(R,Ɵ)sin Ɵ d Ɵ$

(17)

since we are working in a half space. Here ĸ₂ is a measure of diffusion control for the two site problem. The partially diffusion-controlled rate constant for the two site problem is given by

$\begin{gathered} {{\text{k}}_{{\text{DC}}}} = 16\pi {\text{DR}}{ĸ_2}{(1 - cos( Ɵ˳))^2}/\{ 16\pi {\text{DR(}}1 - cos Ɵ˳) + 4{ĸ_2}{{\text{(}}1 - cos Ɵ˳)^2} \hfill \\ {ĸ_2}\sum\nolimits_{l = 1} {} {[{P_{{\text{2l}} - 1}}(cos Ɵ˳) - {P_{{\text{2l}} + 1}}(cos Ɵ˳)]^2}/(2{\text{l}} + 1/2)({\text{l}} + 1/2)\} \hfill \\ \end{gathered}$

(18)

As mentioned before, if we replace ĸ₂ in equation (18) by (2N) 4Da(since there are 2 patches), we obtain the diffusion-controlled rate constant for N uniformly circular reactive sites of radius a located on each spherical cap of half-angle Ɵ˳ at both poles on a sphere (See Figure 1b).

$\begin{gathered} {{\text{k}}_{{\text{DC}}}} = 16\pi {\text{DR}}{\text{Na}}{(1 - cos( Ɵ˳))^2}/\{ 16\pi {\text{DR(}}1 - cos Ɵ˳) + 4{\text{Na}}{{\text{(}}1 - cos Ɵ˳)^2} \hfill \\ {\text{Na}}\sum\nolimits_{l = 1} {} {[{P_{{\text{2l}} - 1}}(cos Ɵ˳) - {P_{{\text{2l}} + 1}}(cos Ɵ˳)]^2}/(2{\text{l}} + 1/2)({\text{l}} + 1/2)\} \hfill \\ \end{gathered}$

(19)

Letting Ɵ˳π/2 we obtain the Berg-Purcell result for two patches

${{\text{k}}_{{\text{BP}}}} = 4\pi {\text{DR}}\left( {2{\text{N}}} \right){\text{a}}/\left( {\pi {\text{R}} + \left( {2{\text{N}}} \right){\text{a}}} \right)$

(20)

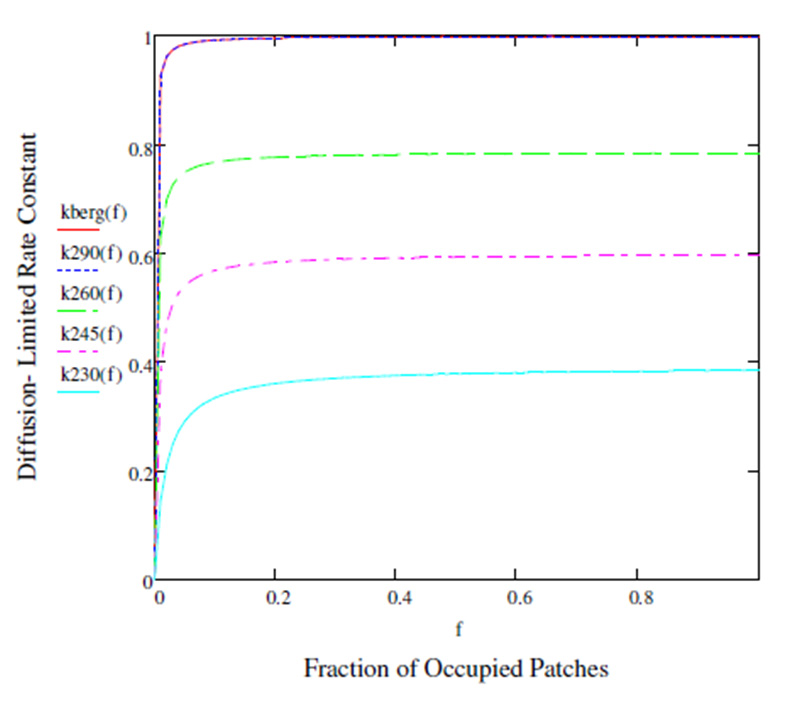
Figure 3 shows k2Ɵ˳=k_DC/4πDR plotted against the fraction of receptors for different patch sizes (30, 45, 60 and 90 degrees along with the Berg-Purcell result, equation (20)/4πDR). Here the fractional coverage is the same as equation (14), because ϴ˳ ranges from 0 to π/2. The proper limitingbehavior occurs for Ɵ˳ → π/2 (agreement with the Berg-Purcell result). Here, for multiple sites at two poles of the sphere, we see that for fractional coverages of 15% or greater, the patches behave as if they were both totally absorbing (independent of the patch size). This shows that the cluster problem at two poles on a sphere reduce to the two site problem ^[11] for fractional coverages in this range (e.g. Equation (19) can be replaced by equation (18) for ĸ₂ → ∞.) which is

${{\text{k}}_{{\text{DC}}}} = 16\pi {\text{DR}}{(1 - cos Ɵ˳)^2}/\{ 4{(1 - cos Ɵ˳)^2} + \sum\nolimits_{l = 1} {} {[{P_{{\text{2l}} - 1}}(cos Ɵ˳) - {P_{{\text{2l}} + 1}}(cos Ɵ˳)]^2}/(2{\text{l}} + 1/2)({\text{l}} + 1/2)\}$

(21)

Finally figure 3 shows that the cluster site rate constant is substantially less than the Berg-Purcell rate (40% for Ɵ˳=45 degrees).

---

4. Summary and Conclusion

In this paper we have derived expressions for the diffusion-controlled rate constant for multiple binding sites on one and both poles of a sphere. We have found that for fractional coverages greater than 15%, simplification occurs in the models used to describe diffusion-controlled asymmetric reactions for a spherical cell or protein. Also the polar models show a large reduction in the magnitude of the rate constant from the Berg-Purcell result as might be expected. For bacterial chemotaxis, one would expect the predicted rate constant for the cluster model to be smaller than the predicted rate constant for the Berg-Purcell model by as much as 35% for a 30 degree patch for fractional coverages greater than 15% for R/a values around1000. This shows that a sphere uniformly covered by reactive sites is not always the best model to use. It should be noted that for R/a values around 100, the fractional coverage cutoff point is 30% as opposed to 15% (obtained for R/a values around 1000 discussed in the paper).

---

Conflict of Interest

There is no conflict of interest regarding this paper.

---