Mean time to infection by small diffusing droplets containing SARS-CoV-2 during close social contacts

1.   Introduction

Gorenstein homological algebra, roughly speaking, which is a relative version of homological algebra with roots on one hand in commutative algebra and on the other hand in modular representation theory of finite groups, has been developed to a high level. We refer the reader to ^[6] for some basic knowledge. It is well known that a very natural and important way to study homological algebra is by extending the homological theory on the category of modules to one on the category of complexes. Based on this idea, Gorenstein homological theory of complexes concerned many scholars, see ^{[5,8,12,20,22,27]} and so on. In particular, Gorensein injective complexes were introduced and studied by Enochs and García Rozas in ^[5]. It was shown that for each $n\in\mathbb{Z}$, a complex $C$ is Gorenstein-injective if and only if $C_n$ is a Gorenstein-injective module over an $n$-Gorenstein ring. Liu and Zhang proved that this result holds over left Noetherian rings ^[12]. These have been further developed by Yang ^[22], Yang and Liu ^[27], independently.

Cartan-Eilenberg projective and injective complexes play important roles not only in the category of complexes but also in homotopy category of complexes, see [2,Sections 12.4 and 12.5]. In 2011, Enochs further studied Cartan-Eilenberg projective, injective and flat complexes, and meanwhile, introduced and investigated Cartan-Eilenberg Gorenstein-injective complexes in ^[4]. In particular, he proved that a complex $X$ is Cartan-Eilenberg Gorenstein-injective if and only if $\mathrm{B}_n(X)$ and $\mathrm{H}_n(X)$ are Gorenstein-injective modules for all $n\in\mathbb{Z}$. Recently, Cartan-Eilenberg complexes have attracted a lot of attention. For instance, Yang and Liang in ^[23,24] studied the notion of Cartan-Eilenberg Gorenstein projective and flat complexes. For a self-orthogonal class $\mathscr{W}$ of modules, Lu et al. studied Cartan-Eilenberg $\mathscr{W}$-Gorenstein complexes and stability ^[17].

The notion of $m$-complexes has important applications in theoretical physics, quantum theory and representation theory of algebras, and has concerned many authors, see ^{[1,7,9,11,14,15,16,19,25,26,28]} and so on. For instance, Bahiraei, Gillespie, Iyama, Yang and their collaborators ^{[1,9,11,19,26,28]} investigate the homotopy category and the derived category of $m$-complexes. Yang and Wang in ^[28] introduce the notions of dg-projective and dg-injective $m$-complexes, and study the existence of homotopy resolutions for $m$-complexes. Estrada investigates the notion of Gorenstein-injective $m$-complexes in ^[7]. Recently, Lu et al. in ^[13,15,16] investigate the notions of Cartan-Eilenberg $m$-complexes and Gorenstein $m$-complexes. For a self-orthogonal subcategory $\mathscr{W}$ of an abelian category $\mathscr{A}$, it is shown that a Cartan-Eilenberg injective $m$-complex can be divided into direct sums of an injective $m$-complex and an injetive grade module, a $m$-complex $G$ is Gorenstein-injective if and only if $G$ is a $m$-complex consisting of Gorenstein modules which improves a result of Estrada in ^[7].

The motivation of this paper comes from the following incomplete diagram of related notions:

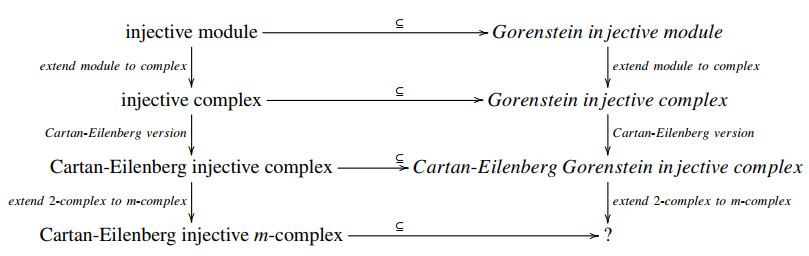
The main purpose of the present paper is to define and investigate Cartan-Eilenberg Gorenstein injective $m$-complexes such that the above diagram will be completed. We establish the following result which gives a relationship between a Cartan-Eilenberg Gorenstein-injective $m$-complex and the corresponding level modules, cycle modules, boundary modules and homology modules.

Theorem 1.1. (= Theorem 3.8) Let $G$ be a m-complex. Then the following conditions are equivalent$:$

$(1)$ $G$ is a Cartan-Eilenberg Gorenstein-injective m-complex.

$(2)$ $G_n$, $\mathrm{Z}_n^{t}(G)$, $\mathrm{B}_n^{t}(G)$ and $\mathrm{H}_n^{t}(G)$ are Gorenstein-injective modules for each $n\in\mathbb{Z}$ and $t = 1, 2, \ldots, m$.

The stability of Gorenstein categories, initiated by Sather-Wagstaff et al. in ^[18], is an important research subject, and has also been considered by Ding, Liu, Lu, Xu and so on, see ^[17,21]. In this paper, as an application of Theorem 1.1 we prove a stability result for Cartan-Eilenberg Gorenstein-injective $m$-complexes, see Theorem 4.2.

We conclude this section by summarizing the contents of this paper. Section 2 contains necessary notation and definitions for use throughout this paper. In section 3, we mainly give an equivalent characterization of Cartan-Eilenberg Gorenstein-injective $m$-complexes. An application of Theorem 1.1 is given in Section 4.

In what follows, we will use the abbreviation 'CE' for Cartan-Eilenberg.

2.   Preliminaries

In this section we recall some necessary notation and definitions. Throughout the paper, $R$ denotes an associative ring with an identity and by the term "module" we always mean a left $R$-module. For two modules $M$ and $N$, we will let $\mathrm{Hom}_R(M, N)$ denote the group of morphisms from $M$ to $N$. Ext$^i_R$ for $i\geq0$ denotes the groups we get from the right derived functor of Hom.

A $m$-complex $X$ ($m\geq 2$) is a sequence of left $R$-modules

satisfying $d^m = d^X_{n+1}d^X_{n+2}\cdots d^X_{n+m} = 0$ for any $n\in\mathbb{Z}$. That is, composing any $m$-consecutive morphisms gives $0$. So a 2-complex is a chain complex in the usual sense. We use $d^l_{n_X}$ to denote $d^X_{n-l+1}\cdots d^X_{n-1}d^X_{n}$. A chain map or simply map $f:X\rightarrow Y$ of $m$-complexes is a collection of morphisms $f_n:X_n\rightarrow Y_n$ making all the rectangles commute. In this way we get a category of $m$-complexes of left $R$-modules, denoted by $\mathcal{C}_m(R)$, whose objects are $m$-complexes and whose morphisms are chain maps. This is an abelian category having enough projectives and injectives. Let $C$ and $D$ be $m$-complexes. We use $\mathrm{Hom}_{\mathcal{C}_m(R)}(C, D)$ to denote the abelian group of morphisms from $C$ to $D$ and Ext$^i_{\mathcal{C}_m(R)}(C, D)$ for $i\geq0$ to denote the groups we get from the right derived functor of Hom. In particular, we use $\mathrm{Hom}(C, D)$ to denote the abelian group of morphisms from $C$ to $D$ whenever $m = 2$.

Unless stated otherwise, $m$-complexes will always the $m$-complexes of left $R$-modules. For a $m$-complex $X$, there are $m-1$ choices for homology. Indeed for $t = 1, \cdots, m$, we define $\mathrm{Z}^t_n(X) = \mathrm{Ker}(d_{n-t+1}\cdots d_{n-1}d_n)$, $\mathrm{B}^t_n(X) = \mathrm{Im}(d_{n+1}d_{n+2}\cdots d_{n+t})$, and $\mathrm{H}^t_n(X) = \mathrm{Z}^t_n(X)/\mathrm{B}^{m-t}_n(X)$ the amplitude homology modules of $X$. In particular, we have

and

We say $X$ is $m$-exact, or just exact, if $\mathrm{H}^t_n(X) = 0$ for all $n$ and $t$. Given a left $R$-module $A$, we define $m$-complexes $\mathrm{D}^t_n(A)$ for $t = 1, \cdots, m$ as follows: $\mathrm{D}^t_n(A)$ consists of $A$ in degrees $n, n-1, \cdots, n-t+1$, all joined by identity morphisms, and $0$ in every other degree.

Two chain maps $f, g:X\rightarrow Y$ are called chain homotopic, or simply homotopic if there exists a collection of morphisms $\{s_n:X_n\rightarrow Y_{n+m-1}\}$ such that

If $f$ and $g$ are homotopic, then we write $f\sim g$. We call a chain map $f$ null homotopic if $f\sim0$. There exists an additive category $\mathcal{K}_m(R)$, called the homotopy category of $m$-complexes, whose objects are the same as those of $\mathcal{C}_m(R)$ and whose Hom sets are the $\sim$ equivalence classes of Hom sets in $\mathcal{C}_m(R)$. An isomorphism in $\mathcal{K}_m(R)$ is called a homotopy equivalence. We define the shift functor $\Theta : \mathcal{C}_m(R)\longrightarrow \mathcal{C}_m(R)$ by

for $X = (X_i, d_i^X)\in\mathcal{C}_m(R)$. The $m$-complex $\Theta(\Theta X)$ is denoted $\Theta^2X$ and inductively we define $\Theta^nX$ for all $n\in\mathbb{Z}$. This induces the shift functor $\Theta : \mathcal{K}_m(R)\longrightarrow \mathcal{K}_m(R)$ which is a triangle functor.

Recall from [6,Definition 10.1.1] that a left $R$-module $M$ is called Gorenstein-injective if there exists an exact sequence of injective left $R$-modules

with $M\cong \mathrm{Ker}(E_0\rightarrow E_1)$ and which remains exact after applying $\mathrm{Hom}_R(I, -)$ for any injective left $R$-module $I$.

A complex $I$ is injective if and only if $I$ is exact with $\mathrm{Z}_n(I)$ injective in $R$-Mod for each $n\in\mathbb{Z}$. A complex $X$ is said to be Gorenstein-injective if there exists an exact sequence of injective complexes

with $X\cong \mathrm{Ker}(E^0\rightarrow E^1)$ and which remains exact after applying $\mathrm{Hom}(I, -)$ for any injective complex $I$ [8,Definition 3.2.1].

A complex $I$ is said to be CE injective if $I, \mathrm{Z}(I), \mathrm{B}(I)$ and $\mathrm{H}(I)$ are complexes consisting of injective modules [4,Definition 3.1]. A sequence of complexes

is $\mathrm{C}\mathrm{E}$ exact if $\cdots\rightarrow C^{-1}\rightarrow C^{0}\rightarrow C^{1}\rightarrow \cdots$ and $\cdots\rightarrow \mathrm{Z}(C^{-1})\rightarrow \mathrm{Z}(C^{0})\rightarrow \mathrm{Z}(C^{1})\rightarrow \cdots$ are exact by [4,Lemma 5.2 and Definition 5.3]. Eonchs also introduced and studied the concept of CE Gorenstein-injective complexes, see [4,Definition 8.4]. A complex $G$ is said to be CE Gorenstein-injective, if there exists a CE exact sequence

of CE injective complexes such that $G = \mathrm{Ker}(I^{0}\rightarrow I^{1})$ and the sequence remains exact when $\mathrm{Hom}(E, -)$ is applied to it for any CE injective complex $E$.

Remark 2.1. $(1)$ For any injective module $E$, $E$ is a Gorenstein-injective module and $\cdots\longrightarrow 0\longrightarrow E\stackrel{1} \longrightarrow E\longrightarrow 0\longrightarrow\cdots$ is an injective complex.

$(2)$ For any Gorenstein-injective module $E$, $\cdots\longrightarrow 0\longrightarrow E\longrightarrow 0\longrightarrow\cdots$ is a Gorenstein-injective complex by [27,Proposition 2.8].

$(3)$ According to [4,Theorem 8.5] and [27,Proposition 2.8], any injective complex is CE Gorenstein-injective, and any CE Gorenstein-injective complex is Gorenstein-injective.

$(4)$ Note that strongly Gorenstein-injective modules are Gorenstein-injective by [3,Definition 2.1]. The Gorenstein injective modules are not necessarily injective by [3,Example 2.5]. Thus Gorenstein-injective complexes and CE Gorenstein-injective complexes are not necessarily injective by [4,Theorem 8.5] and [27,Proposition 2.8].

3.   Cartan-Eilenberg Gorenstein-injective $m$-complexes

In this section, we will give an equivalent characterization on Cartan-Eilenberg Gorenstein-injective $m$-complexes. To this end, we first give some preparations.

Definition 3.1. ([16,Definition 1]) A sequence of $m$-complexes

is said to be CE exact if

$(1)$ $\cdots\rightarrow C^{-1}_n\rightarrow C^{0}_n\rightarrow C^{1}_n\rightarrow \cdots$,

$(2)$ $\cdots\rightarrow \mathrm{Z}^t_n(C^{-1})\rightarrow \mathrm{Z}^t_n(C^{0})\rightarrow \mathrm{Z}^t_n(C^{1})\rightarrow \cdots$,

$(3)$ $\cdots\rightarrow \mathrm{B}^t_n(C^{-1})\rightarrow \mathrm{B}^t_n(C^{0})\rightarrow \mathrm{B}^t_n(C^{1})\rightarrow \cdots$,

$(4)$ $\cdots\rightarrow C^{-1}/\mathrm{Z}^t_n(C^{-1})\rightarrow C^{0}/\mathrm{Z}^t_n(C^{0})\rightarrow C^{1}/\mathrm{Z}^t_n(C^{1})\rightarrow \cdots$,

$(5)$ $\cdots\rightarrow C^{-1}/\mathrm{B}^t_n(C^{-1})\rightarrow C^{0}/\mathrm{B}^t_n(C^{0})\rightarrow C^{1}/\mathrm{B}^t_n(C^{1})\rightarrow \cdots$,

$(6)$ $\cdots\rightarrow \mathrm{H}^t_n(C^{-1})\rightarrow \mathrm{H}^t_n(C^{0})\rightarrow \mathrm{H}^t_n(C^{1})\rightarrow \cdots$ are all exact for $t = 1, \cdots, m$ and $n\in\mathbb{Z}$. $

Remark 3.2. ([16,Remark 1]) Obviously, in the above definition, exactness of $(1)$ and $(2) ($or $(1)$ and $(3)$, or $(1)$ and $(4)$, or $(2)$ and $(3)$, or $(2)$ and $(5)$, or $(3)$ and $(4)$, or $(3)$ and $(5)$, or $(4)$ and $(5))$ implies exactness of all $(1)$–$(6)$.

Definition 3.3. ([16,Remark 2]) A $m$-complex $I$ is called CE CE if $I_n, \mathrm{Z}^t_n(I), \mathrm{B}^t_n(I), \mathrm{H}^t_n(I)$ are injective left $R$-modules for $n\in\mathbb{Z}$ and $t = 1, \cdots, m$.

The following lemma palys an important role in the proof of Theorem 3.8.

Lemma 3.4. ([16,Proposition 3] and [15,Proposition 4.1]) Let $I$ be a $m$-complex. Then

$(1)$ I is injective if and only if $I = \bigoplus\limits_{n\in\mathbb{Z}}\mathrm{D}^m_{n+m-1}(E_n)$, where $E_n$ is an injective module for each $n\in\mathbb{Z}$.

$(2)$ $I$ is $CE$ injective if and only if $I$ can be divided into direct sums $I = I^{'} \bigoplus I^{''}$, where $I^{'}$ is an injective $m$-complex and $I^{''}$ is a graded module with $I^{''}_n$ injective modules. Specifically,

Here, we give the notion of CE Gorenstein-injective objects in the category of $m$-complexes.

Definition 3.5. A $m$-complex $G$ is said to be CE Gorenstein-injective, if there exists a $CE$ exact sequence

of CE injective $m$-complexes such that

$(1)$ $G = \mathrm{Ker}(I^{0}\rightarrow I^{1});$

$(2)$ the sequence remains exact when $\mathrm{Hom}_{\mathcal{C}_m(R)}(E, -)$ is applied to it for any CE injective $m$-complex $V$.

In this case, $\mathbb{I}$ is called a complete $CE$ injective resolution of G.

Remark 3.6. (1) It is clear that any CE injective $m$-complex is CE Gorenstein-injective.

(2) Any CE Gorenstein-injective $m$-complex is Gorenstein-injective.

(3) Take $m = 2$. Then CE Gorenstein-injective $m$-complexes are CE Gorenstein-injective complexes.

The following lemma is used to prove Theorem 3.8.

Lemma 3.7. ([25,Lemma 2.2]) For any module $M$, $X, Y\in\mathcal{C}_m(R)$ and $n\in\mathbb{Z}, \; i = 1, 2, \cdots, m$, we have the following natural isomorphisms.

$(1)$ $\mathrm{Hom}_{\mathcal{C}_m(R)}(\mathrm{D}_n^m(M), Y) \cong \mathrm{Hom}_{R}(M, Y_n)$.

$(2)$ $\mathrm{Hom}_{\mathcal{C}_m(R)}(X, \mathrm{D}_{n+m-1}^m(M)) \cong \mathrm{Hom}_{R}(X_{n}, M)$.

$(3)$ $\mathrm{Hom}_{\mathcal{C}_m(R)}(\mathrm{D}_n^i(M), Y) \cong \mathrm{Hom}_{R}(M, \mathrm{Z}_n^i(Y))$.

$(4)$ $\mathrm{Hom}_{\mathcal{C}_m(R)}(X, \mathrm{D}_n^i(M)) \cong \mathrm{Hom}_{R}(X_{n-(i-1)}/\mathrm{B}_{n-(i-1)}^i(Y), M)$.

$(5)$ $\mathrm{Ext}^1_{\mathcal{C}_m(R)}(\mathrm{D}_n^m(M), Y) \cong \mathrm{Ext}^1_{R}(M, Y_n)$.

$(6)$ $\mathrm{Ext}^1_{\mathcal{C}_m(R)}(X, \mathrm{D}_{n+m-1}^m(M)) \cong \mathrm{Ext}^1_{R}(X_{n}, M)$.

$(7)$ If $Y$ is $m$-exact, then $\mathrm{Ext}^1_{\mathcal{C}_m(R)}(\mathrm{D}_n^i(M), Y) \cong \mathrm{Ext}^1_{R}(M, \mathrm{Z}_n^i(Y))$.

$(8)$ If $X$ is $m$-exact, then $\mathrm{Ext}^1_{\mathcal{C}_m(R)}(X, \mathrm{D}_n^i(M)) \cong \mathrm{Ext}^1_{R}(X_{n-(i-1)}/\mathrm{B}_{n-(i-1)}^i(X), M)$.

Now, we give the following result which gives an equivalent characterization of $m$-complexes which extends the corresponding result [4,Theorem 8.5] to the setting of $m$-complexes.

Theorem 3.8. Let $G$ be a m-complex. Then the following conditions are equivalent$:$

$(1)$ $G$ is a $CE$ Gorenstein-injective m-complex.

$(2)$ $G_n$, $\mathrm{Z}_n^{t}(G)$, $\mathrm{B}_n^{t}(G)$ and $\mathrm{H}_n^{t}(G)$ are Gorenstein-injective modules for each $n\in\mathbb{Z}$ and $t = 1, 2, \ldots, m$.

In this case, $G_n/\mathrm{Z}_n^{t}(G)$ and $G_n/\mathrm{B}_n^{t}(G)$ are Gorenstein-injective modules.

Proof. (1) $\Rightarrow$(2). Suppose that

is a complete CE injective resolution of $G$ such that $G = \text{Ker}\, (I^{0}\rightarrow I^{-1})$. Then, there is an exact sequence of injective modules

such that $G_{n} = \text{Ker}(I^{0}_{n}\rightarrow I^{-1}_{n})$ for all $n\in \mathbb{Z}$ by Definition 3.3. For any injective module $E$, $\mathrm{D}^m_{n}(E)$ is a CE injective $m$-complex for each $n\in \mathbb{Z}$ by Definition 3.3 again. It follows from Lemma 3.7 that there is a natural isomorphism

for all $i, n\in \mathbb{Z}.$ Then the following commutative diagram with the first row exact

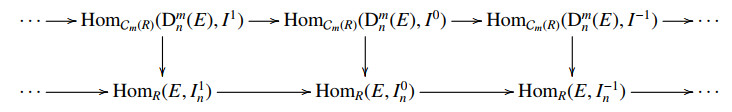
yields that the lower row is exact. Hence, $G_{n}$ is a Gorenstein-injective module.

We notice that $\text{Z}^t_{n}(I^{i}) = I^{i}_{n}$ for $t = m$. So we only to show that Z$^{t}_{n}(G)$ is a Gorenstein-injective module for $t = 1, 2, \cdots, m-1$. Since $\mathbb{I}$ is CE exact, then, for any $n\in \mathbb{Z}$ and $t = 1, 2, \cdots, m-1$, the sequence

is exact with Z$^t_{n}(I^{i})$ injective module since $I^{i}$ is CE injective for each $i\in \mathbb{Z}$.

For any injective module $E$, $\mathrm{D}^{t}_{n}(E)$ is a CE injective $m$-complex for each $n\in \mathbb{Z}$ and $t = 1, 2, \cdots, m$, and there is a natural isomorphism Hom$_{\mathcal{C}_m(R)}(\mathrm{D}^{t}_{n}(E), I^{i}) \cong \text{Hom}_{R}(E, \text{Z}^{t}_{n}(I^{i}))$ by Lemma 3.7 for each $i\in \mathbb{Z}$. Applying Hom$_{\mathcal{C}_m(R)}(\mathrm{D}^{t}_{n}(E), -)$ to the sequence $\mathbb{I}$, we obtain that $\text{Z}^{t}_{n}(\mathbb{I})$ is $\text{Hom}_{R}(E, -)$ exact. Note that Z$^{t}_{n}(G) = \text{Ker}\, (\text{Z}^{t}_{n}(I^{0})\rightarrow \text{Z}^{t}_{n}(I^{-1}))$. Thus Z$^{t}_{n}(G)$ is a Gorenstein-injective module.

Meanwhile, there are exact sequences of modules

and

for all $n\in \mathbb{Z}$ and $t = 1, 2, \cdots, m$. Then we get that $\text{B}^{t}_{n-t}(G)$ and $\text{H}^{t}_{n}(G)$ are Gorenstein-injective by [10,Theorem 2.6].

(2) $\Rightarrow$ (1). For a fixed $n\in \mathbb{Z}$, there exist the following exact sequences of modules

with $\text{B}^{m-1}_{n-i}(G)$ and $\text{H}^1_{n}(G)$ Gorenstein-injective modules for $i = 0, 1, 2, \cdots, m-1.$

Suppose $E^i$ is a complete injective resolution of $\text{B}^{m-1}_{n}(G)$, and $F^n$ is a complete injective resolution of $\text{H}^1_{n}(G)$ respectively, $i = 0, 1, 2, \cdots, m-1$, $n\in\mathbb{Z}$. In an iterative way, we can construct a complete injective resolution of $G_{n+m}$

by the Horseshoe Lemma.

Put

and define

for any $(x_n, x_{n-1}, \cdots, x_{n-m+2}, x_{n-m+1}, y_n)\in I^{l}_n$. Then $(I^{l}, d_{n})$ is a $m$-complex and $G_n\cong\mathrm{Ker}(I^l_{n}\rightarrow I^{l-1}_{n})$. It is easily seen that $I^{l}$ is a CE injective $m$-complex for all $l\in \mathbb{Z}$ and $G = \text{Ker}\, (I^{l}\rightarrow I^{l-1})$. For any $n\in \mathbb{Z}$ and $t = 1, 2, \cdots, m$,

is a complete injective resolution of Z$^t_{n}(G)$, and

is a complete injective resolution of $G_{n}$, so they both are exact. Hence, we can get that

is CE exact by Remark 3.2.

It remains to prove that, for any CE injective $m$-complex $I$, $\mathbb{I}$ is still exact when Hom$_{\mathcal{C}_m(R)}(I, -)$ applied to it. However, it suffices to prove that the assertion holds when we pick $I$ particularly as $I = \mathrm{D}_n^m(E)$ and $I = \mathrm{D}_n^1(E)$ for any injective module $E$ and all $n\in \mathbb{Z}$ by Lemma 3.4. Note that $\mathbb{I}_{n}$ and Z$^t_{n}(\mathbb{I})$ are complete injective resolutions, hence from Lemma 3.7, the desired result follows.

Moreover, if $G$ is a CE Gorenstein-injective $m$-complex. then $G_n/\mathrm{Z}_n^{t}(G)$ and $G_n/\mathrm{B}_n^{t}(G)$ are also Gorenstein-injective modules by [10,Theorem 2.6].

Take $m = 2$ in Theorem 3.8. We obtain the following corollary which is a main result of ^[4].

Corollary 3.9. [4,Theorem 8.5] Let $G$ be a complex. Then the following conditions are equivalent$:$

$(1)$ $G$ is a $CE$ Gorenstein-injective complex.

$(2)$ $G_n$, $\mathrm{Z}_n(G)$, $\mathrm{B}_n(G)$ and $\mathrm{H}_n(G)$ are Gorenstein-injective modules for each $n\in\mathbb{Z}$.

Take $m = 3$ in Theorem 3.8. We obtain the following result.

Corollary 3.10. Let $G$ be a 3-complex. Then the following conditions are equivalent$:$

$(1)$ $G$ is a $CE$ Gorenstein-injective 3-complex.

$(2)$ $G_n$, $\mathrm{Z}_n^1(G)$, $\mathrm{B}_n^1(G)$ , $\mathrm{H}_n^1(G)$, $\mathrm{Z}_n^2(G)$, $\mathrm{B}_n^2(G)$ and $\mathrm{H}_n^2(G)$ are Gorenstein-injective modules for each $n\in\mathbb{Z}$.

The following result establishes a relationship between an $m$-exact $m$-complex and its cycle modules under certain hypotheses.

Proposition 3.11. Let C be a m-exact m-complex with $\mathrm{Hom}_R(\mathrm{D}^1_n(E), C)$ exact for any injective module $E$. Then C is a Gorenstein-injective complex if and only if $\mathrm{Z}^t_n(C)$ is a Gorenstein-injective module for each $n\in\mathbb{Z}$ and $t = 1, 2, \cdots, m.$

Proof. $(\Rightarrow)$ Let $C$ be a Gorenstein-injective $m$-complex. There exists an exact sequence of injective $m$-complexes

with $C = \mathrm{Ker}f^0$ such that $\mathbb{I}$ is $\mathrm{Hom}_{\mathcal{C}_m({R})}(E, -)$ exact for any injective $m$-complex $E$. We also have $\mathrm{Ker}(f^i)$ is $m$-exact for all $i\in\mathbb{Z}$ since $\mathrm{Ker}(f^0) = C$ and $I^i$ are $m$-exact.

Applying Hom$_{\mathcal{C}_m({R})}(\mathrm{D}^t_n(R), -)$ to the sequence $(\star)$, there is a commutative diagram:

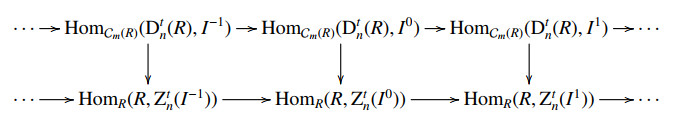
with the upper exact since $\mathrm{Ext}^1_{\mathcal{C}_m({R})}(\mathrm{D}^t_n(R), \mathrm{Ker}f^i) = 0$ by Lemma 3.7. Then the second row is exact. Thus we obtain an exact sequence of injective modules

with $\mathrm{Z}^t_n(C)\cong \mathrm{Ker}(\mathrm{Z}^t_n(I^{0}) \rightarrow\mathrm{Z}_n(I^{1}))$. So we only need to show that $\mathrm{Hom}_R(E, -)$ leave the sequence $(\star\star)$ exact for any injective module $E$.

Let $E$ be an injective module and $g:E\rightarrow \mathrm{Z}^{t}_{n}(C)$ be a morphism of modules. Since $\mathrm{Hom}_R(\mathrm{D}^1_n(E), C)$ is $m$-exact, there exists a morphism $f:E\rightarrow C_{n+m-t}$ such that the following diagram:

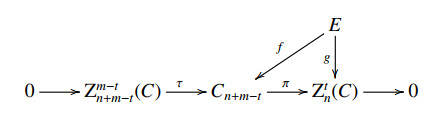
commutes.

Note that $\mathbb{I}$ is $\mathrm{Hom}_{\mathcal{C}_m({R})} (\mathrm{D}^m_{n+m-t}(E), -)$ exact since $\mathrm{D}^m_{n+m-t}(E)$ is an injective $m$-complex by [15,Proporition 4.1 and Corollary 4.4]. For the exact sequence

there is an exact sequence

It follows from Lemma 3.7 that

is exact. Thus, for $f\in \mathrm{Hom}_R(E, C_{n+m-t})$, there exists a morphism $h:E\rightarrow I^{-1}_{n+m-t}$ such that $f = h\rho$. Therefore, we obtain the following commutative diagram:

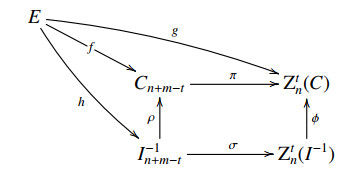
where

and

is induced by the morphism $I^{-1}\rightarrow C$, which means $g = \phi\sigma h$. We notice that

Then

is exact. Similarly, we can prove that

is exact. Hence, $\mathrm{Hom}_R(E, -)$ leaves the sequence $(\star\star)$ exact. This completes the proof.

$(\Leftarrow)$ Note that there is an exact sequence

and $\mathrm{Z}^{m-t}_{n+m-t}(C)$ and $\mathrm{Z}^t_n(C)$ are Gorenstein-injective modules. Then $C_{n}$ is a Gorenstein-injective module for each $n\in\mathbb{Z}$ by [10,Theorem 2.6], as desired.

As an immediately consequence of Proposition 3.11, we establish the following Corollary which appears in [22,Theorem 4].

Corollary 3.12. Let C be an exact complex with $\mathrm{Hom}_R(E, C)$ exact for any injective module $E$. Then C is a Gorenstein-injective complex if and only if $\mathrm{Z}_n(C)$ is a Gorenstein-injective module for each $n\in\mathbb{Z}$.

4.   An application

In this section, as an application of Theorem 3.8, we investigate the stability of CE Gorenstein-injective categories of CE Gorenstein-injective $m$-complexes. That is, we show that an iteration of the procedure used to define the CE Gorenstein-injective $m$-complexes yields exactly the CE Gorenstein-injective $m$-complexes. We first introduce the notion of two-degree CE Gorenstein-injective $m$-complexes.

Definition 4.1. A $m$-complex C is said to be two-degree CE Gorenstein-injective if there is a CE exact sequence of CE Gorenstein-injective $m$-complexes

such that $C\cong \mathrm{Ker}(G^0\rightarrow G^1)$ and the functors $\mathrm{Hom}_{\mathcal{C}_m({R})}(H, -)$ leave $\mathbb{G}$ exact whenever $H$ is a CE Gorenstein-injective $m$-complex. In this case, $\mathbb{G}$ is called a complete CE Gorenstein-injective resolution of C.

It is obvious that any CE Gorenstein-injective $m$-complex is two-degree CE Gorenstein-injective$.$ In the following, we prove that two-degree CE Gorenstein-injective $m$-complexes are CE Gorenstein-injective.

Theorem 4.2. Let C be a $m$-complex. Then the following statements are equivalent$:$

$(1)$ C is two-degree CE Gorenstein-injective.

$(2)$ C is a CE Gorenstein-injective.

Proof. $(2)\Rightarrow(1)$ is trivial.

$(1)\Rightarrow(2)$. We need to show that $G_n$, $\mathrm{Z}_n^{t}(G)$, $\mathrm{B}_n^{t}(G)$ and $\mathrm{H}_n^{t}(G)$ are Gorenstein-injective modules for each $n\in\mathbb{Z}$ and $t = 1, 2, \ldots, m$ by Theorem 3.8. By (1), there exists a CE exact sequence of CE Gorenstein-injective $m$-complexes

with $C = \mathrm{Ker}(G^0\rightarrow G^1)$ and such that the functor Hom$_{\mathcal{C}_m({R})}(H, -)$ leave $\mathbb{G}$ exact whenever $H$ is a CE Gorenstein-injective $m$-complex. Then there is an exact sequence of Gorenstein-injective modules

with $C_n = \mathrm{Ker}(G_n^0\rightarrow G_n^1)$ for all $n\in\mathbb{Z}$ by Theorem 3.8.

Let $E$ be an injective module. Then $\mathrm{D}^m_{n}(E)$ is a CE Gorenstein-injective $m$-complex for each $n\in\mathbb{Z}$ by Theorem 3.8. We apply the functor $\mathrm{Hom}_{\mathcal{C}_m({R})}(\mathrm{D}^m_{n}(E), -)$ to the sequence $(†)$, we get the following exact sequence

by Lemma 3.7. Thus, $C_n$ is a Gorenstein-injective module for each $n\in\mathbb{Z}$ by Corollary 3.12.

There also exists an exact sequence

of Gorenstein-injective modules with $\mathrm{Z}^t_n(C) = \mathrm{Ker}(\text{Z}^t_{n}(G^{0}) \rightarrow \text{Z}^t_{n}(G^{1}))$ for all $n\in\mathbb{Z}$ and $t = 1, 2, \cdots, m.$

We notice that, for any injective module $E$, $\mathrm{D}^t_{n}(E)$ is CE Gorenstein-injective $m$-complexes for each $n\in\mathbb{Z}$ and $t = 1, 2, \cdots, m$ by Theorem 3.8. Applying the functor $\mathrm{Hom}_{\mathcal{C}_m({R})}(\mathrm{D}^t_{n}(E), -)$ to the sequence $(†)$, we get the following exact sequence

by Lemma 3.7. Hence, $\mathrm{Z}^t_n(C)$ is a Gorenstein-injective module for each $n\in\mathbb{Z}$ by Corollary 3.12 again.

Meanwhile, there are exact sequences of modules

and

for all $n\in \mathbb{Z}$ and $t = 1, 2, \cdots, m$. Then we get that $\text{B}^{t}_{n-t}(G)$ and $\text{H}^{t}_{n}(G)$ are Gorenstein-injective by [10,Theorem 2.6]. Therefore, $C$ is a CE Gorenstein-injective $m$-complexes using Theorem 3.8 again.

Take $m = 2$, as a consequence of Theorem 4.2, we obtain the following corollary which establishes the stability for CE Gorenstein-injective complexes.

Corollary 4.3. Let C be a complex. Then the following statements are equivalent$:$

$(1)$ C is a two-degree CE Gorenstein-injective complex.

$(2)$ C is a CE Gorenstein-injective complex.

Take $m = 3$, as a consequence of Theorem 4.2, we obtain the following corollary.

Corollary 4.4. Let C be a 3-complex. Then the following statements are equivalent$:$

$(1)$ C is two-degree CE Gorenstein-injective 3-complex.

$(2)$ C is a CE Gorenstein-injective 3-complex.

We can construct the following example by Corollary 3.10 and Corollary 4.4.

Example 4.5. The 3-complex

is $CE$ Gorenstein-injective if and only if $G$ is a Gorenstein-injective module if and only if $H$ is two-degree CE Gorenstein-injective.

Acknowledgments

This work was partially supported by National Natural Science Foundation of China (No. 12061061), Innovation Team Projec of Northwest Minzu University (No. 1110130131), and first-rate discipline of Northwest Minzu University. We would like to thank the referee for a careful reading of the paper and for many useful comments and suggestions.

Conflict of Interest

All authors declare no conflicts of interest.