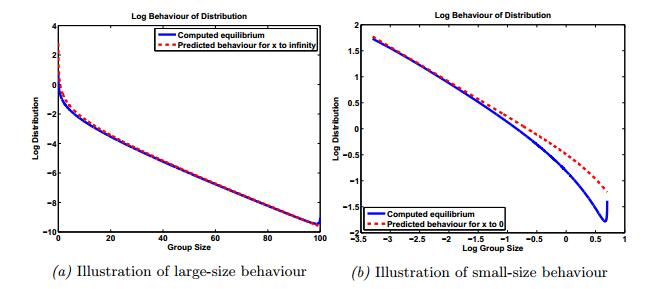
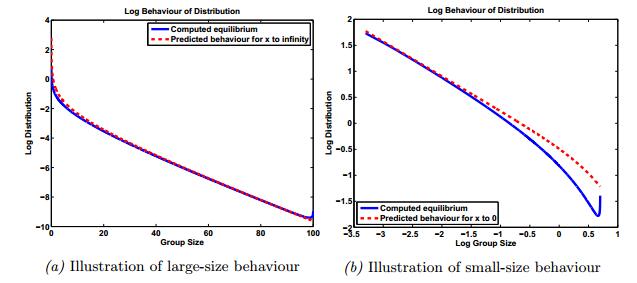
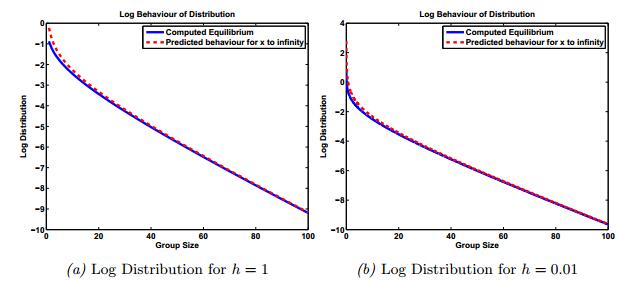
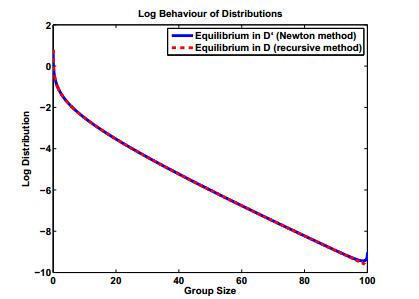
We study numerically a coagulation-fragmentation model derived by Niwa [17] and further elaborated by Degond et al. [5]. In [5] a unique equilibrium distribution of group sizes is shown to exist in both cases of continuous and discrete group size distributions. We provide a numerical investigation of these equilibria using three different methods to approximate the equilibrium: a recursive algorithm based on the work of Ma et. al. [12], a Newton method and the resolution of the time-dependent problem. All three schemes are validated by showing that they approximate the predicted small and large size asymptotic behaviour of the equilibrium accurately. The recursive algorithm is used to investigate the transition from discrete to continuous size distributions and the time evolution scheme is exploited to show uniform convergence to equilibrium in time and to determine convergence rates.
Citation: Pierre Degond, Maximilian Engel. Numerical approximation of a coagulation-fragmentation model for animal group size statistics[J]. Networks and Heterogeneous Media, 2017, 12(2): 217-243. doi: 10.3934/nhm.2017009
Related Papers:
| [1] |
Jicheng Li, Beibei Liu, Hao-Tian Wu, Yongjian Hu, Chang-Tsun Li .
Jointly learning and training: using style diversification to improve domain generalization for deepfake detection. Electronic Research Archive, 2024, 32(3): 1973-1997.
doi: 10.3934/era.2024090
|
| [2] |
Qing Tian, Canyu Sun .
Structure preserved ordinal unsupervised domain adaptation. Electronic Research Archive, 2024, 32(11): 6338-6363.
doi: 10.3934/era.2024295
|
| [3] |
Bingsheng Li, Na Li, Jianmin Ren, Xupeng Guo, Chao Liu, Hao Wang, Qingwu Li .
Enhanced spectral attention and adaptive spatial learning guided network for hyperspectral and LiDAR classification. Electronic Research Archive, 2024, 32(7): 4218-4236.
doi: 10.3934/era.2024190
|
| [4] |
Huixia Liu, Zhihong Qin .
Deep quantization network with visual-semantic alignment for zero-shot image retrieval. Electronic Research Archive, 2023, 31(7): 4232-4247.
doi: 10.3934/era.2023215
|
| [5] |
Junjie Zhao, Junfeng Wu, James Msughter Adeke, Guangjie Liu, Yuewei Dai .
EITGAN: A Transformation-based Network for recovering adversarial examples. Electronic Research Archive, 2023, 31(11): 6634-6656.
doi: 10.3934/era.2023335
|
| [6] |
Qing Tian, Yanan Zhu, Yao Cheng, Chuang Ma, Meng Cao .
Unsupervised domain adaptation through transferring both the source-knowledge and target-relatedness simultaneously. Electronic Research Archive, 2023, 31(2): 1170-1194.
doi: 10.3934/era.2023060
|
| [7] |
Yu Wang .
Bi-shifting semantic auto-encoder for zero-shot learning. Electronic Research Archive, 2022, 30(1): 140-167.
doi: 10.3934/era.2022008
|
| [8] |
Jian Liu, Zhen Yu, Wenyu Guo .
The 3D-aware image synthesis of prohibited items in the X-ray security inspection by stylized generative radiance fields. Electronic Research Archive, 2024, 32(3): 1801-1821.
doi: 10.3934/era.2024082
|
| [9] |
Qing Tian, Heng Zhang, Shiyu Xia, Heng Xu, Chuang Ma .
Cross-view learning with scatters and manifold exploitation in geodesic space. Electronic Research Archive, 2023, 31(9): 5425-5441.
doi: 10.3934/era.2023275
|
| [10] |
Ziqing Yang, Ruiping Niu, Miaomiao Chen, Hongen Jia, Shengli Li .
Adaptive fractional physical information neural network based on PQI scheme for solving time-fractional partial differential equations. Electronic Research Archive, 2024, 32(4): 2699-2727.
doi: 10.3934/era.2024122
|
Abstract
We study numerically a coagulation-fragmentation model derived by Niwa [17] and further elaborated by Degond et al. [5]. In [5] a unique equilibrium distribution of group sizes is shown to exist in both cases of continuous and discrete group size distributions. We provide a numerical investigation of these equilibria using three different methods to approximate the equilibrium: a recursive algorithm based on the work of Ma et. al. [12], a Newton method and the resolution of the time-dependent problem. All three schemes are validated by showing that they approximate the predicted small and large size asymptotic behaviour of the equilibrium accurately. The recursive algorithm is used to investigate the transition from discrete to continuous size distributions and the time evolution scheme is exploited to show uniform convergence to equilibrium in time and to determine convergence rates.
References
|
[1]
|
Possible universality in the size distribution of fish schools. Phys. Rev. E (1995) 51: 5220-5223. 
|
|
[2]
|
Scaling in animal group-size distributions. Proc. Natl. Acad. Sci. USA (1999) 96: 4472-4477. 
|
|
[3]
|
Convergence of a finite volume scheme for coagulation-fragmentation equations. Comm. Math. Sciences (2008) 77: 851-882. 
|
|
[4]
|
(2011) Functional Analysis, Sobolev Spaces and Partial Differential Equations. New York: Springer-Verlag. |
|
[5]
|
Coagulation-fragmentation model for animal group-size statistics. J. Nonlinear Sci. (2017) 27: 379-424. 
|
|
[6]
|
Numerical simulation of the Smoluchowski coagulation equation. SIAM J. Sci. Comput. (2004) 25: 2004-2028. 
|
|
[7]
|
A finite volume preserving scheme on nonuniform meshes and for multidimensional coalescence. SIAM J. Sci. Comput. (2012) 34: B840-B860. 
|
|
[8]
|
The steady-state distributions of coagulation-fragmentation processes. J. Math. Biol. (1998) 37: 1-27. 
|
|
[9]
|
The dynamics of group formations. Math. Biosc. (1995) 128: 243-264. 
|
|
[10]
|
An accurate and efficient discrete formulation of aggregation population balance equation. Kinet. Relat. Models (2016) 9: 373-391. 
|
|
[11]
|
Moment preserving finite volume schemes for solving population balance equations incorporating aggregation, breakage, growth and source terms. Math. Models Methods Appl. Sci. (2013) 23: 1235-1273. 
|
|
[12]
|
A first principles derivation of animal group size distributions. J. Theoret. Biol. (2011) 283: 35-43. 
|
|
[13]
|
Efficient solution of population balance equations with discontinuities by finite elements. Chem. Eng. Sci. (2002) 57: 1107-1119. 
|
|
[14]
|
A finite element analysis of the steady state population balance equation for particulate systems: Aggregation and growth. Comput. Chem. Eng. (1996) 20: 261-266. 
|
|
[15]
|
Mathematical model for the size distributions of fish schools. Comp. Math. Appl. (1996) 32: 79-88. 
|
|
[16]
|
School size statistics of fish. J. Theoret. Biol. (1998) 195: 351-361. 
|
|
[17]
|
Power-Law versus exponential distributions of animal group sizes. J. Theoret. Biol. (2003) 224: 451-457. 
|
|
[18]
|
Space-irrelevant scaling law for fish school sizes. J. Theoret. Biol. (2004) 228: 347-357. 
|
|
[19]
|
Dynamical aspects of animal grouping: Swarms, schools, rocks, and herds. Adv. Biophys. (1986) 22: 1-94. |
|
[20]
|
Finite-element scheme for solution of the dynamic population balance equation. AIChE Journal (2003) 49: 1127-1139. 
|
|
[21]
|
Population balances for particulate processes-a volume approach. Chem. Eng. Sci. (2002) 57: 2287-2303. 
|











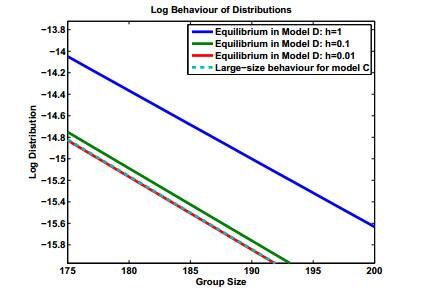
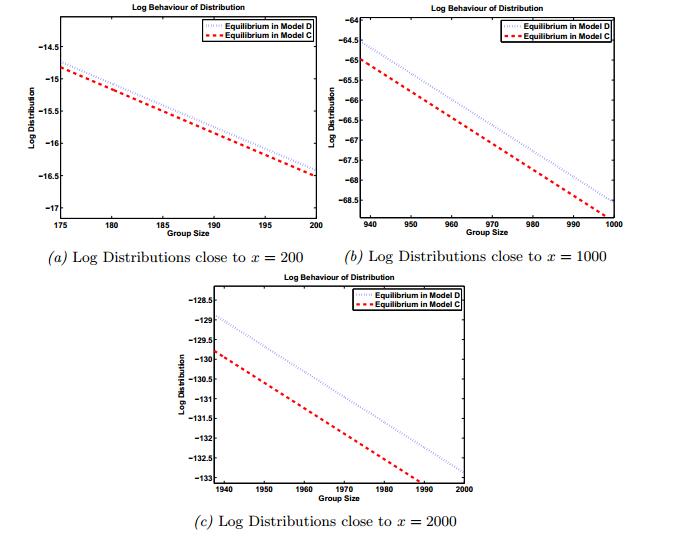
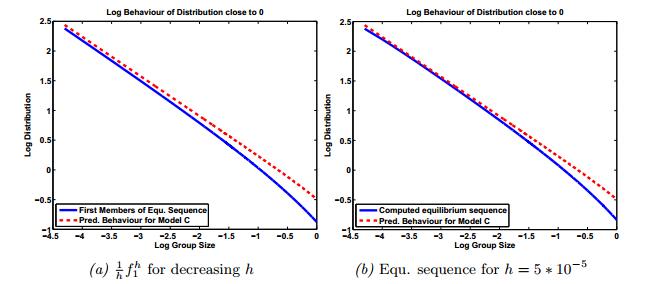
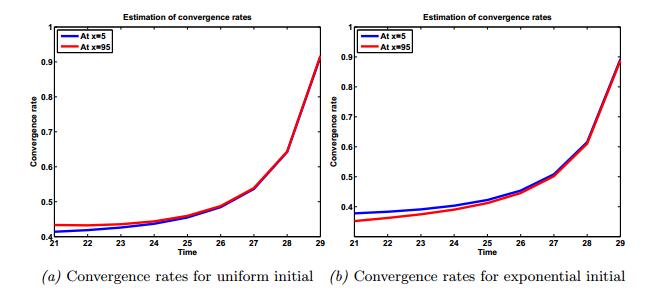

 DownLoad:
DownLoad: